Biosorption of Triphenyl Methane Dyes (Malachite Green and Crystal Violet) from Aqueous Media by Alfa (Stipa tenacissima L.) Leaf Powder
Abstract
1. Introduction
2. Results
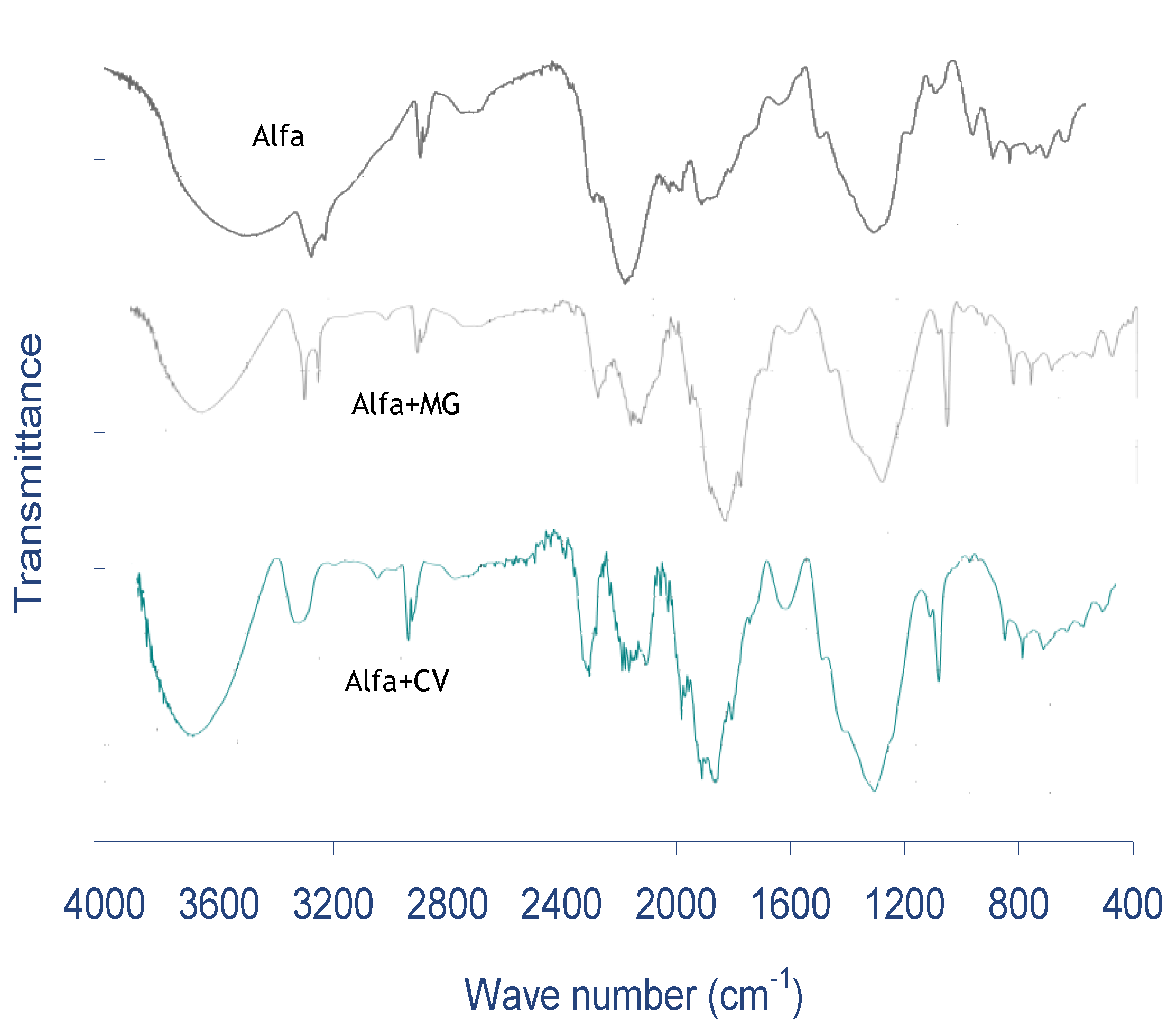
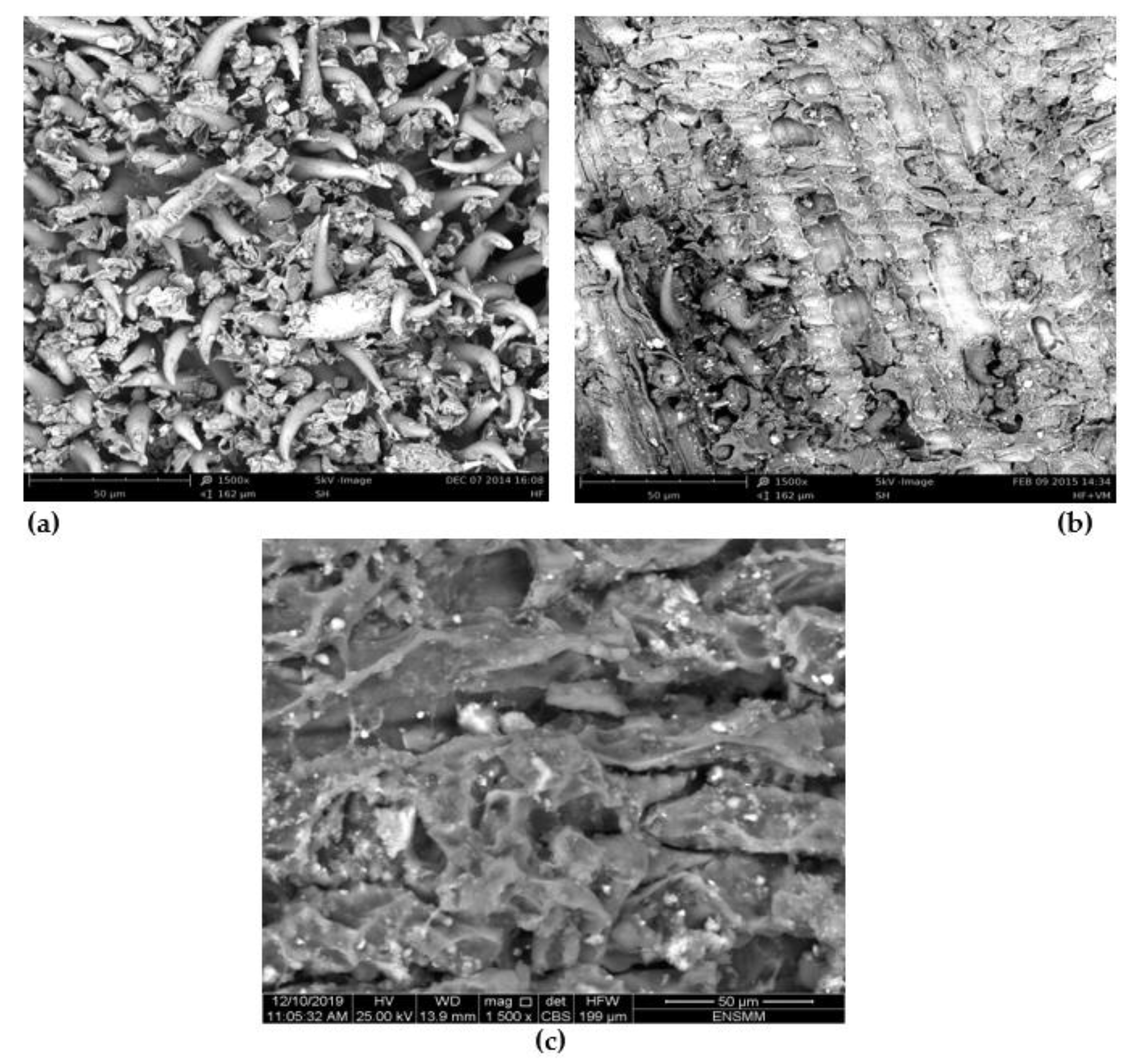
2.1. Characterization of ALP
2.2. Influence of Operating Conditions
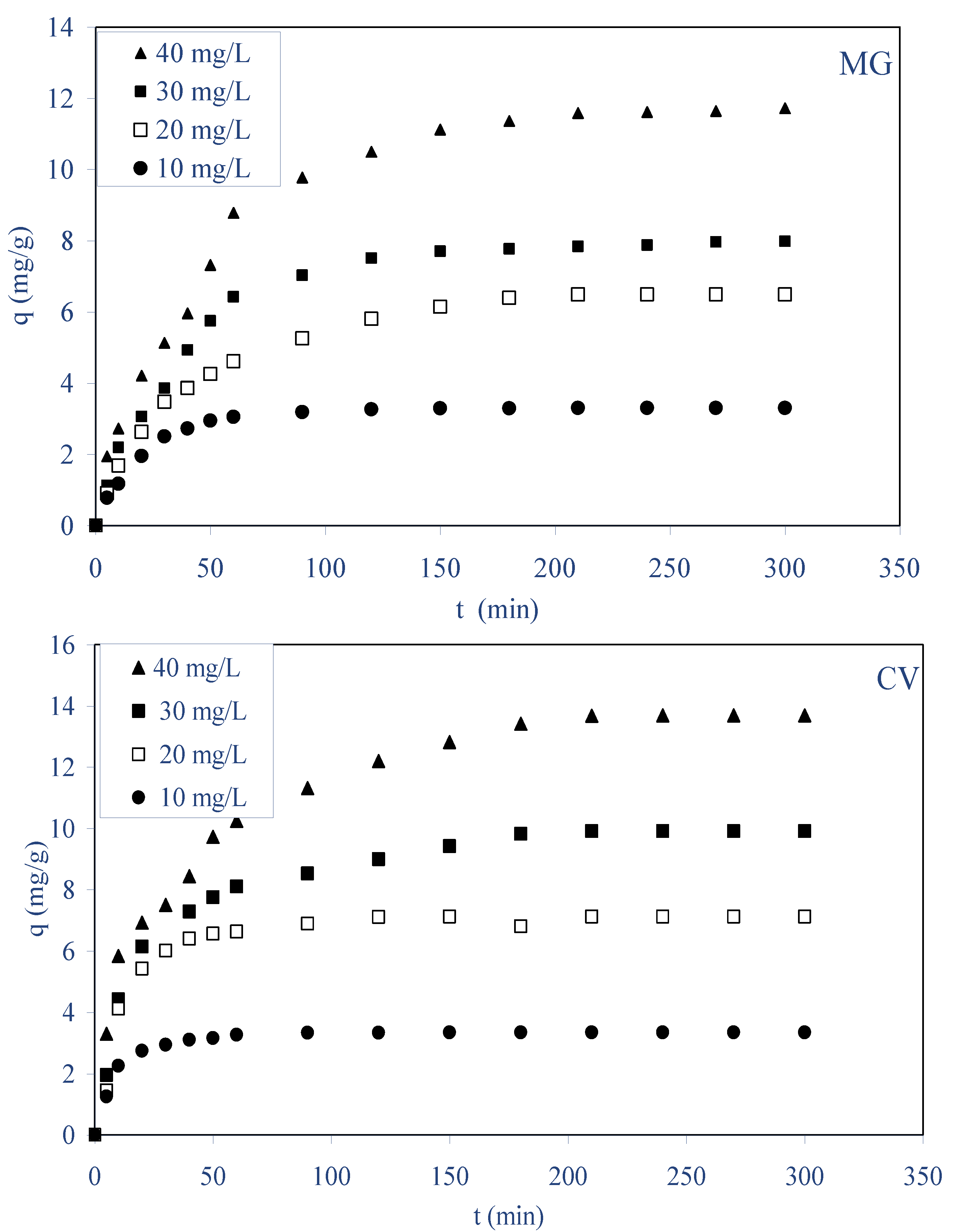
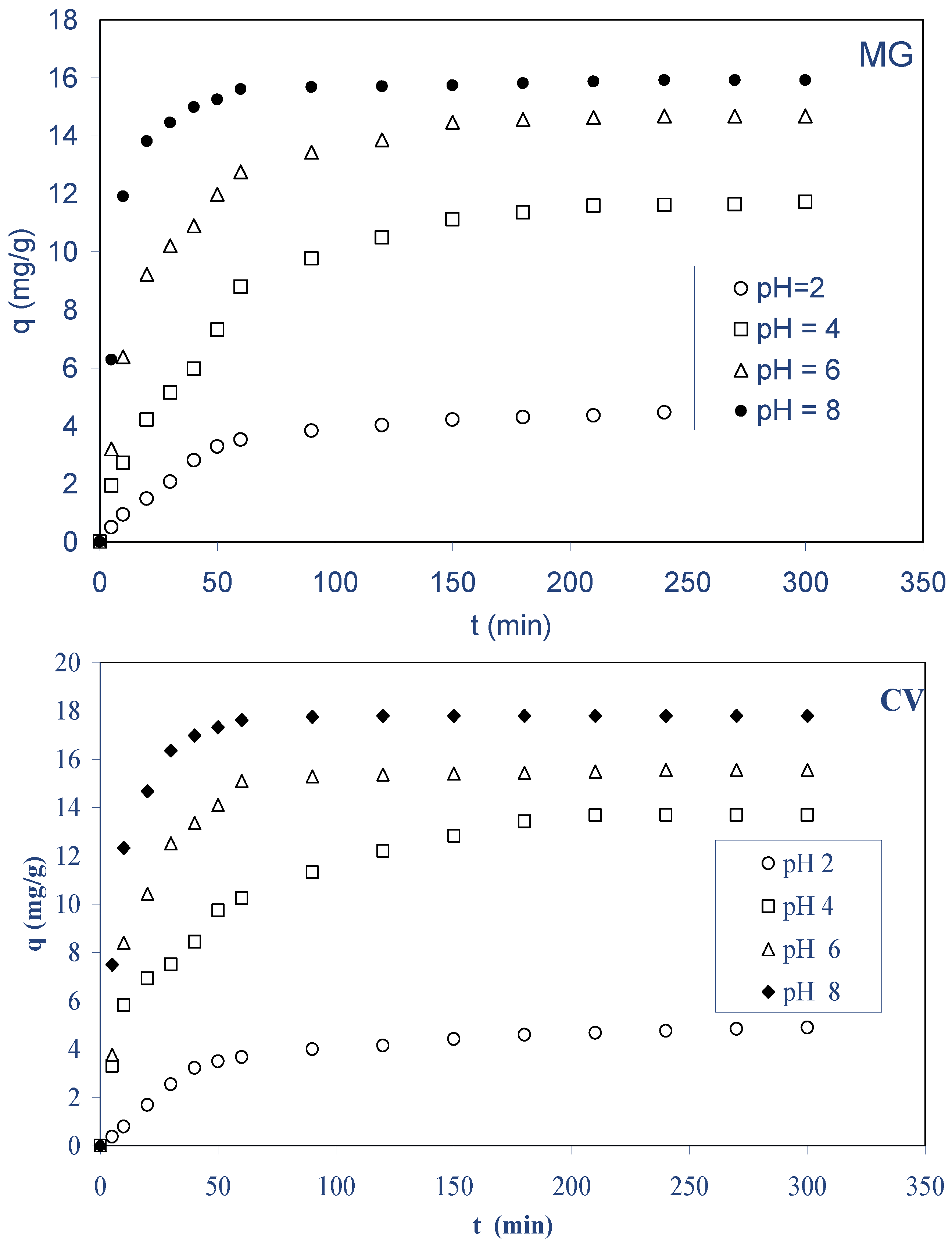
2.2.1. Effect of Initial Dye Concentration and Contact Time
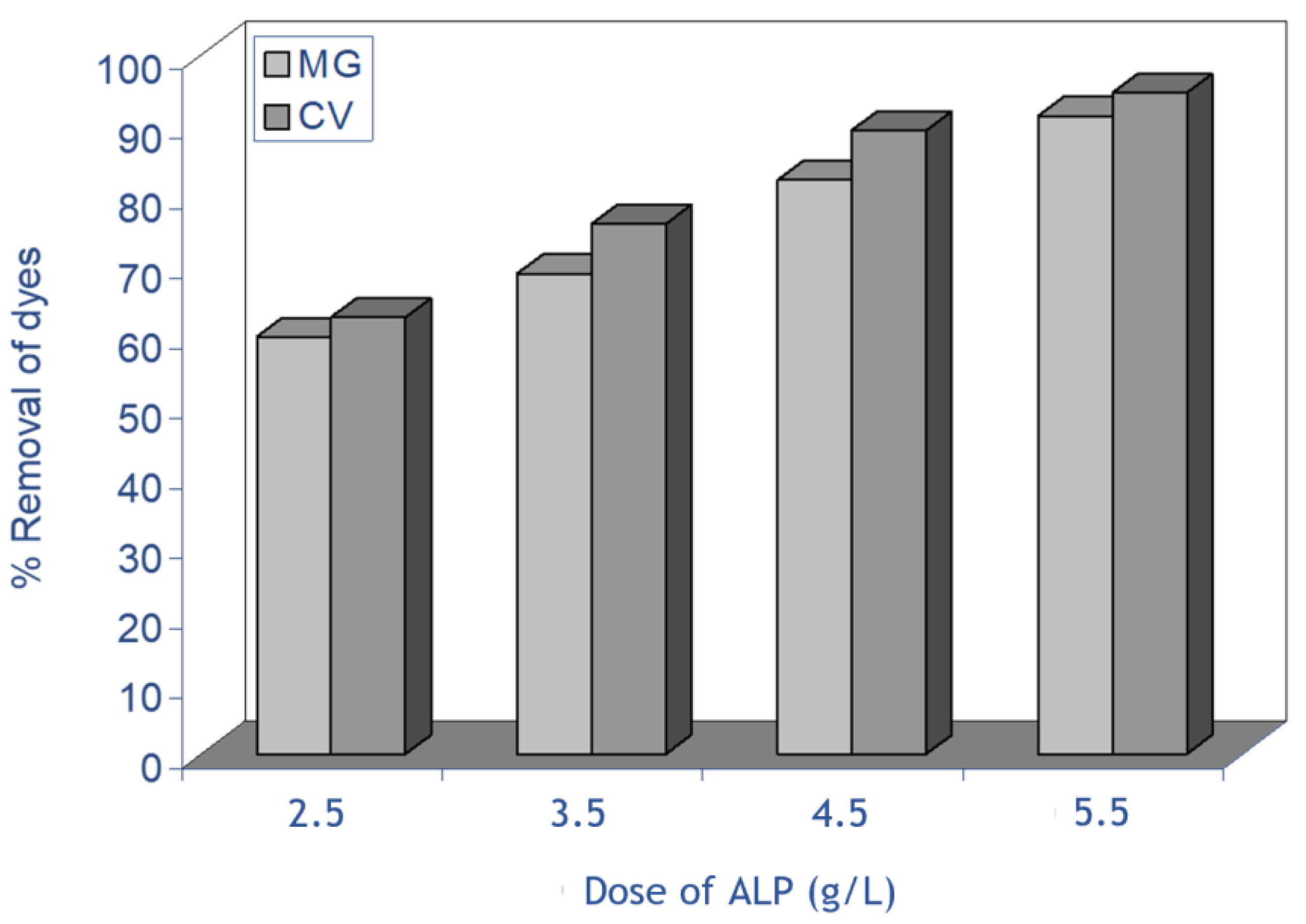
2.2.2. Effect of Biosorbent Dosage
2.2.3. Effect of Initial pH
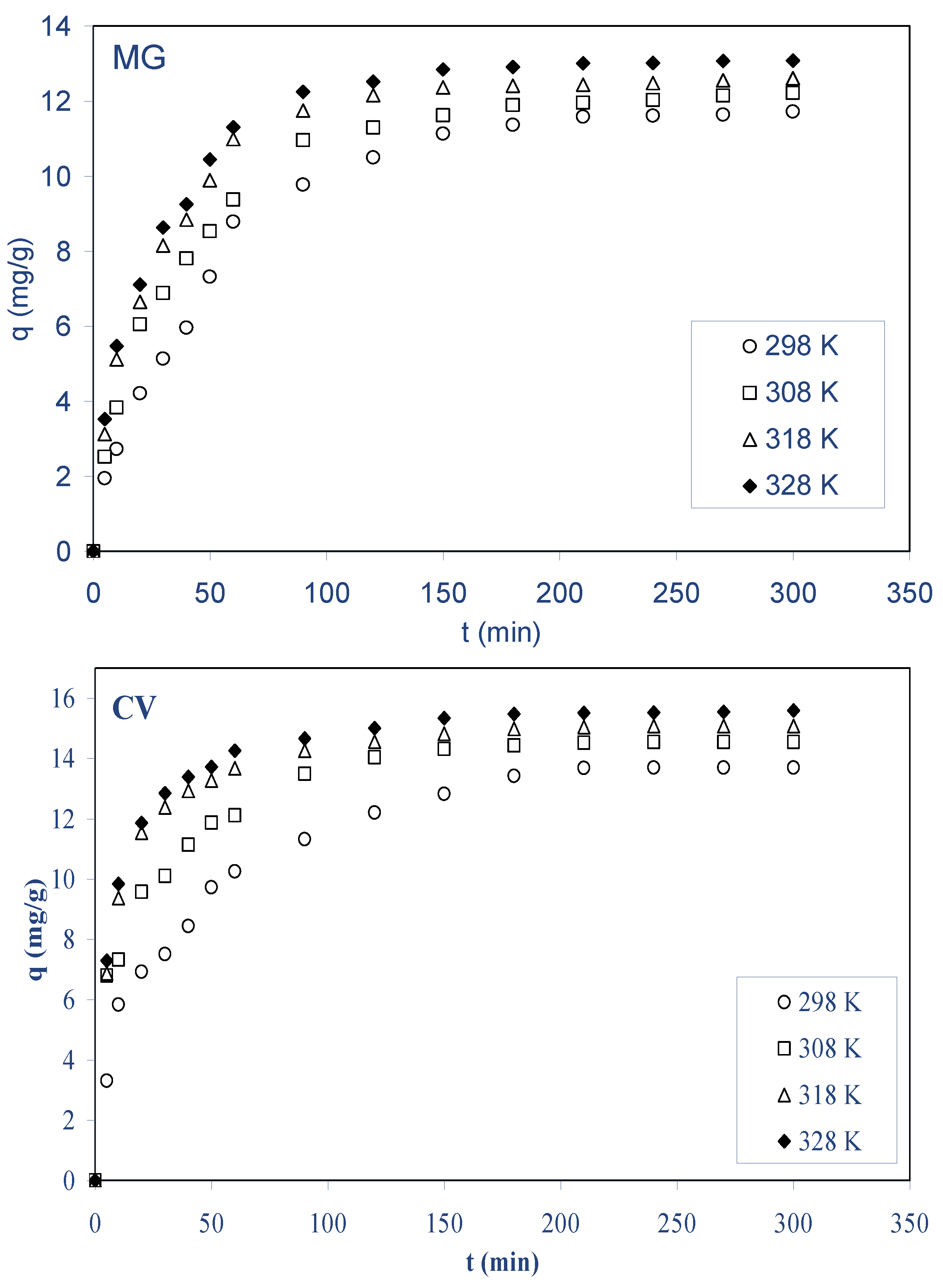
2.2.4. Effect of Temperature
2.2.5. Effect of Ionic Strength
2.3. Modeling of Biosorption Kinetics
2.3.1. Pseudo-First-Order Model
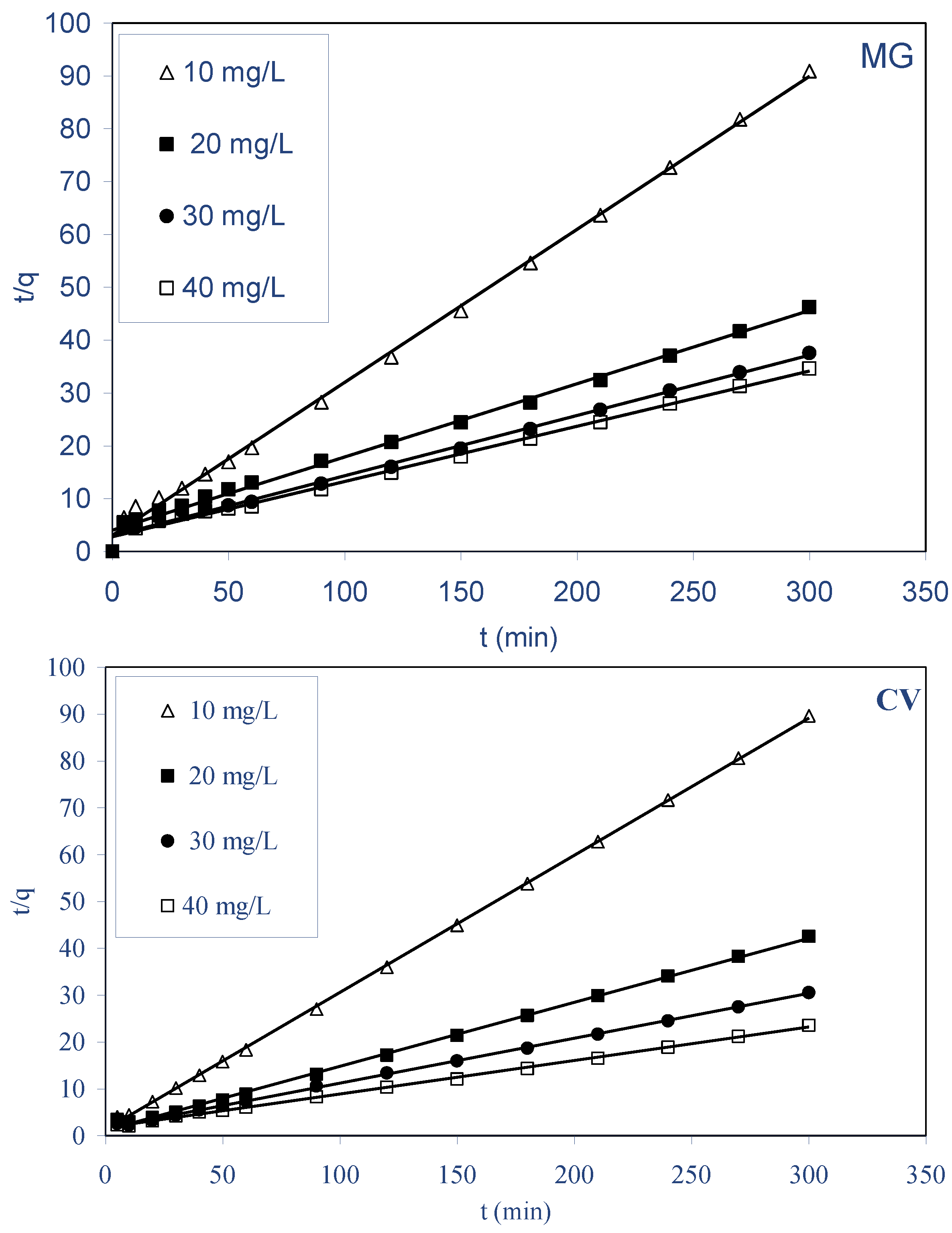
2.3.2. Pseudo-Second-Order Model
2.3.3. Elovich Model
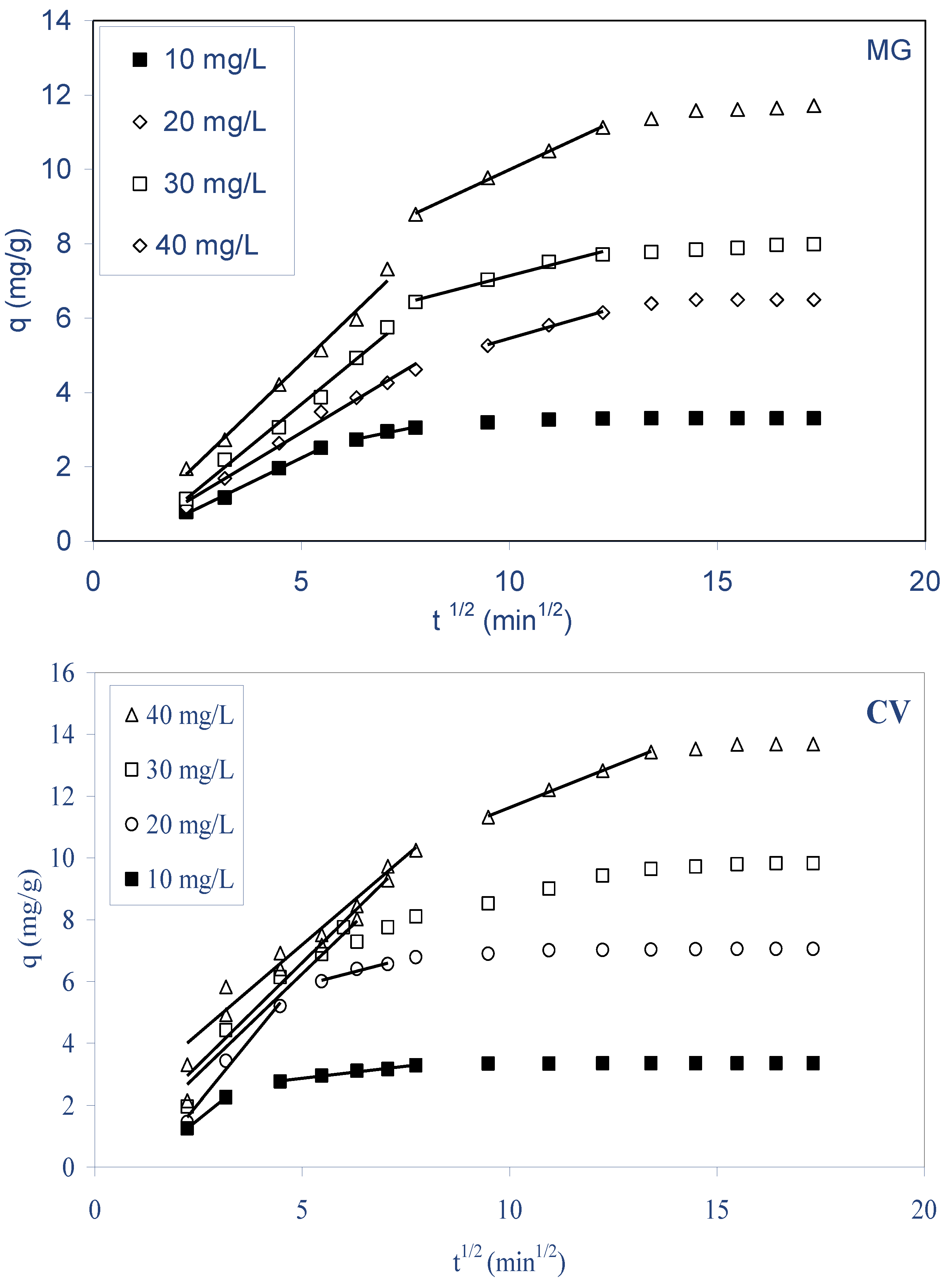
2.4. Mechanism of Dyes Biosorption
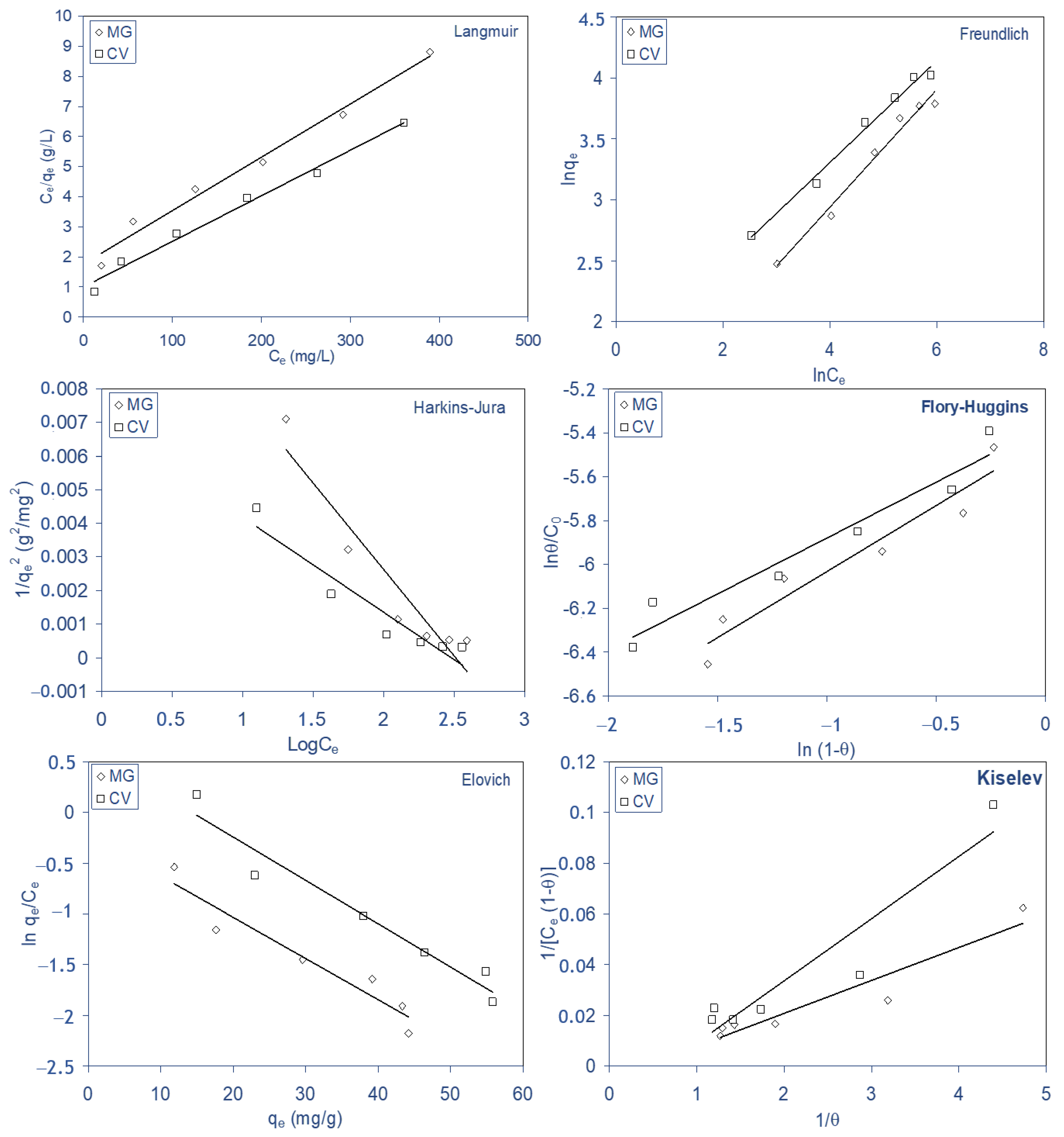
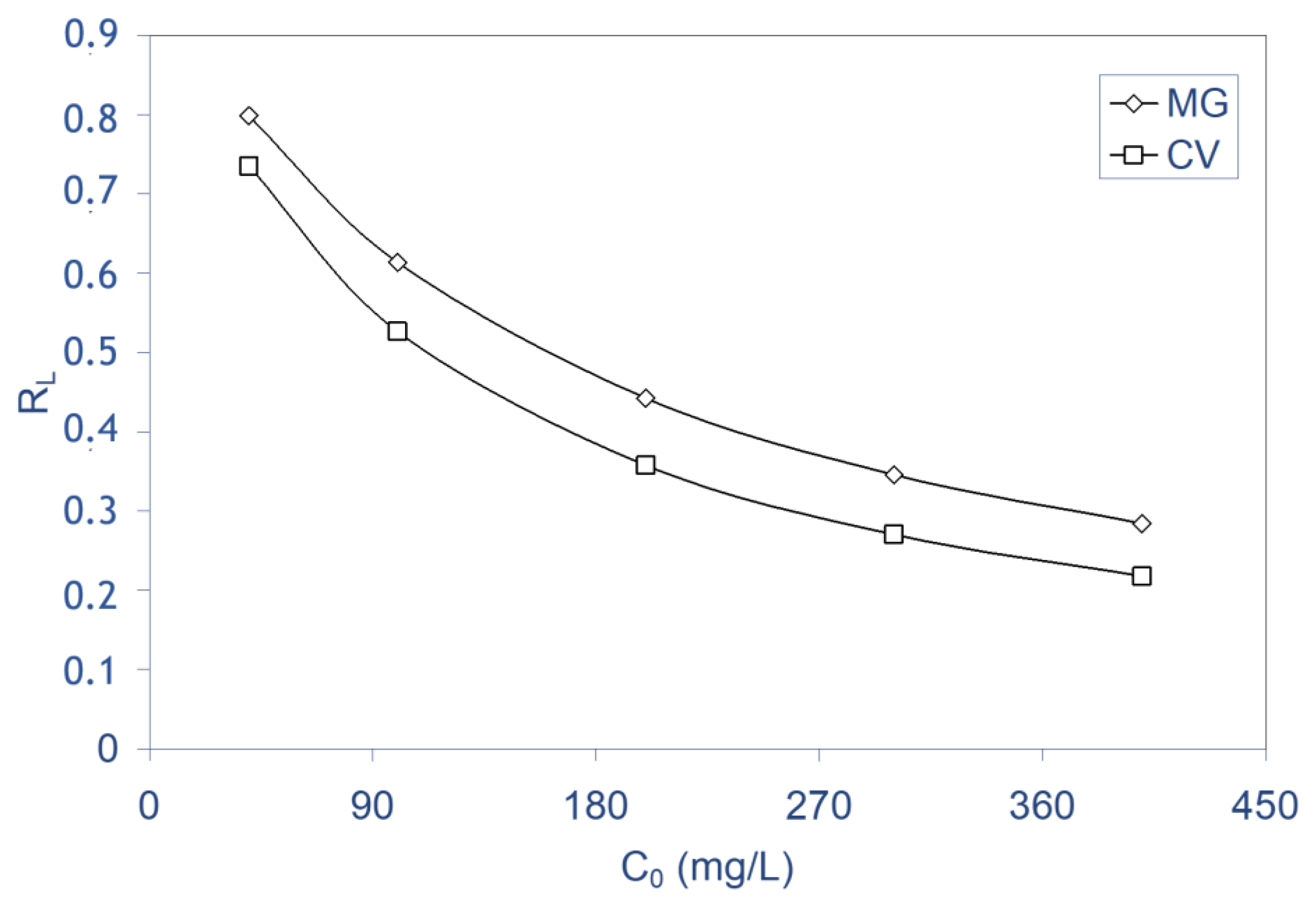
2.5. Biosorption Isotherms
3. Materials and Methods
3.1. Preparation and Characterization of ALP as Biosorbent
3.2. Preparation of Dye Solutions
3.3. Procedures of Biosorption
4. Conclusions
- ALP can be used as an alternative biosorbent for cationic dyes MG and CV removal from aqueous solutions. The advantages of this biosorbent are high availability, low cost, and good biosorption capacity.
- The amounts of acid groups were higher than the basic groups, and this result determines the nature of the ALP surface that enhances the biosorption of the basic dyes from the aqueous phase. Additionally, the FTIR reveals the principal functional groups, which might be implicated in MG and CV biosorption such as OH of the phenol group of cellulose and lignin. The SEM of ALP displays that it is heterogeneous with the presence of spines and a rough, porous surface structure, which also improves the biosorption of dyes, and the X-ray diffraction technique indicates a characteristic shape of ALP that is amorphous in nature. The ALP presents a pHpzc of 6.3.
- The biosorption process was influenced by a number of factors such as contact time and the initial dyes’ concentration, ALP biosorbent dose, initial pH of solution, temperature, and salt ionic strength concentration.
- For both dyes, the biosorption rate increased with increase in initial concentration, the biosorbent (ALP), temperature, initial pH of solution, and contact time, contrary to salt concentration, while stirring speed and total volume of the reaction mixture remained constant.
- The highest biosorption of both dyes was obtained at a pH greater than or equal to 6.
- For both dyes, the information obtained from biosorption isotherms at diverse temperatures (298–328 K) were employed to determine thermodynamic parameters such as ΔG˚, ΔH˚, and ΔS˚ of biosorption. The findings demonstrate that the biosorption is a physical process, spontaneous and endothermic in nature.
- The kinetics biosorption data for both dyes were found to obey to pseudo-second-order kinetics with a good correlation, and for the diffusion mechanism studies, the obtained results reveal that intraparticle diffusion is not the only rate-limiting step, but other processes may control the rate of biosorption for both dyes on ALP, and the equilibrium data were best described by the Langmuir and Freundlich isotherm model for both dyes. The maximum monolayer biosorption capacity was 90.10 and 110.98 mg g−1 for MG and CV, respectively, at 298 K.
- The dimensionless separation factor (RL) showed that ALP could be used for removal of cationic dyes (MG and CV) from aqueous media.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Sulyman, M.; Namiesnik, J.; Gierak, A. Adsorptive Removal of Aqueous Phase Crystal Violet Dye by Low-Cost Activated Carbon Obtained from Date Palm (L.) Dead Leaflets. Eng. Prot. Environ. 2016, 19, 611–631. [Google Scholar] [CrossRef]
- Wan, X.S.; Zhou, Y.; Jiang, Y.; Sun, C. The removal of basic dyes from aqueous solutions using agricultural by-products. J. Hazard. Mater. 2008, 157, 374–385. [Google Scholar]
- Kadirvelu, K.; Palanival, M.; Kalpana, R.; Rajeswari, S. Activated carbon from an agricultural by-product for the treatment of dyeing industry. Waste Water. Bioresour. Technol. 2000, 74, 263–265. [Google Scholar] [CrossRef]
- Moawed, E.A.; Abulkibash, A.B.; El-Shahat, M.F. Synthesis, characterization of iodopolyurethane foam and its application in removing of aniline blue and crystal violet from laundry waste water. J. Taibah Univ. Sci. 2015, 9, 80–88. [Google Scholar] [CrossRef]
- Moawed, E.A.; El-Shahat, M.F. Equilibrium, kinetic and thermodynamic studies of the removal of triphenyl methane dyes from wastewater using iodopolyurethane powder. J. Taibah Univ. Sci. 2016, 10, 46–55. [Google Scholar] [CrossRef]
- Robinson, T.; Mcmullan, G.; Marchant, R.; Nigam, P. Remediation of dyes in textiles effluent: A critical review on current treatment technologies with a proposed alternative. Biresour. Technol. 2001, 77, 247–255. [Google Scholar] [CrossRef] [PubMed]
- Appusamy, A.; John, I.; Ponnusamy, K.; Ramalingam, A. Removal of crystal violet dye from aqueous solution using triton X-114 surfactant via cloud point extraction. Eng. Sci. Technol. Int. J. 2014, 17, 137–144. [Google Scholar] [CrossRef]
- Nawar, S.; Doma, H. Removal of dyes from effluents using low-cost agricultural by-products. Sci. Total Environ. 1989, 79, 271–279. [Google Scholar] [CrossRef]
- Laskar, N.; Kumar, U. Adsorption of Crystal Violet from Wastewater by Modified Bambusa Tulda. KSCE J. Civ. Eng. 2018, 22, 2755–2763. [Google Scholar] [CrossRef]
- Daneshvar, N.; Ayazloo, M.; Khataee, A.R.; Pourhassan, M. Biological decolorization of dye solution containing Malachite Green by microalgae Cosmariumsp. Bioresour. Technol. 2007, 98, 1176–1182. [Google Scholar] [CrossRef]
- Haou, S.; Guechi, E.K.; Benabdesselam, S.; Hamdaoui, O. Effect of ultrasound on biosorption kinetics of Acid blue 25 from aqueous media by using cycads palm bark as novel biosorbent. Desalin. Water Treat. 2021, 225, 413–421. [Google Scholar] [CrossRef]
- Guechi, E.K.; Hamdaoui, O. Sorption of malachite green from aqueous solution by potato peel: Kinetics and equilibrium modeling using non-linear analysis method. Arab. J. Chem. 2016, 9, S416–S424. [Google Scholar] [CrossRef]
- Guechi, E.K.; Hamdaoui, O. Evaluation of potato peel as a novel adsorbent for the removal of Cu (II) from aqueous solutions: Equilibrium, kinetic, and thermodynamic studies. Desalin. Water Treat. 2016, 57, 10677–11068. [Google Scholar] [CrossRef]
- Guechi, E.K.; Benabdesselam, S. Removal of cadmium and copper from aqueous media by biosorption on cattail (Thypha angustifolia) leaves: Kinetic and isotherm studies. Desalin. Water Treat. 2020, 173, 367–382. [Google Scholar] [CrossRef]
- Badescu, I.S.; Bulgariu, D.; Ahmad, I.; Bulgariu, L. Valorisation possibilities of exhausted biosorbents loaded with metal ions. Rev. J. Environ. Manag. 2018, 224, 288–297. [Google Scholar] [CrossRef] [PubMed]
- Guechi, E.K.; Hamdaoui, O.; Benabdesselam, S. Kinetic and thermodynamic study for the removal of cadmium (II) ions from aqueous media by Aucoumea klaineana sawdust. Desalin. Water Treat. 2021, 209, 447–453. [Google Scholar] [CrossRef]
- Zhu, R.; Wang, X.; Panther, J.G.; Wang, Q.; Chakir, S.; Ding, Y.; Huang, Y.; Wang, H. Micro/nanostructured MgO hollow spheres with selective adsorption performance and their application for fluoride monitoring in water. Sep. Purif. Technol. 2022, 299, 121703. [Google Scholar] [CrossRef]
- Paiva, M.C.; Ammar, I.; Campos, A.R.; Cheikh, R.B.; Cunha, A.M. Alfa fibres: Mechanical, morphological and interfacial characterization. Compos. Sci. Technol. 2015, 67, 1132–1138. [Google Scholar] [CrossRef]
- Cerda, A. The effect of patchy distribution of Stipa tenacissima L. On runoff and erosion. J. Arid Environ. 1997, 36, 37–51. [Google Scholar] [CrossRef]
- Nadji, H.; Salon, M.C.B.; Bruzzesse, C.; Benaboura, A.; Belgacem, M.N. Chemical composition and pulp properties of Alfa. Cellul. Chem. Technol. 2006, 40, 45–52. [Google Scholar]
- Guechi, E.K.; Beggas, D. Removal of cadmium (II) from water using fibre fruit lufa as biosorbent. Desalin. Water Treat. 2017, 94, 181–188. [Google Scholar] [CrossRef]
- Guechi, E.K. Equilibrium, kinetics and mechanism for the removal of Rhodamine B by adsorption on Okoume (Aucoumea Klaineana) sawdust from aqueous media. Desalin. Water Treat. 2017, 94, 164–173. [Google Scholar] [CrossRef]
- Guechi, E.K.; Hamdaoui, O. Biosorption of methylene blue from aqueous solution by potato (Solanum tuberosum) peel: Equilibrium modelling, kinetic, and thermodynamic Studies. Desalin. Water Treat. 2016, 57, 10270–10285. [Google Scholar] [CrossRef]
- Silva, L.S.; Lima, L.C.B.; Silva, F.C.; Matos, J.M.E.; Santos, L.S.S.; Junior, M.R.M.C.; Sousa, K.S.; Silva Filho, E.C. Dye anionic sorption in aqueous solution onto a cellulose surface chemically modified with amino ethanethiol. Chem. Eng. J. 2013, 218, 89–98. [Google Scholar] [CrossRef]
- Ahmad, A.; Rafatullah, M.; Sulaiman, O.; Ibrahim, M.H.; Hashim, R. Scavenging behaviour of meranti sawdust in the removal of methylene blue from aqueous solution. J. Hazard. Mater. 2009, 170, 357–365. [Google Scholar] [CrossRef] [PubMed]
- Toumi, B.; Hamdi, L.; Salem, L.; Allia, K. Batch adsorption of methylene blue from aqueous solutions by untreated Alfa grass. Desalin. Water Treat. 2013, 53, 806–817. [Google Scholar] [CrossRef]
- Hemsas, S.; Hachemi, M. Removal of Astrazon yellow dye from aqueous solutions by sorption onto Stipa tenacissima L. Alfa fibers as a natural adsorbent. Alger. J. Environ. Sci. Technol. AJEST 2020, 6, 1–9. [Google Scholar]
- Namasivayam, C.; Kavitha, D. IR, XRD and SEM studies on the mechanism of adsorption of dyes and phenols by coir pith carbon from aqueous phase. Microchem. J. 2006, 2, 43–48. [Google Scholar] [CrossRef]
- Robalds, A.; Dreijalte, L.; Bikovens, O.; Klavins, M. A novel peat based biosorbent for the removal of phosphate from synthetic and real wastewater and possible utilization of spent sorbent in land application. Desalin. Water Treat. 2016, 57, 13285–13294. [Google Scholar] [CrossRef]
- Abdelmouleh, M.; Boufi, S.; Belgacem, M.N.; Dufresne, A. Short natural-fibre reinforced polyethylene and natural rubber composites: Effect of silane coupling agents and fibres loading. Compos. Sci. Technol. 2007, 67, 1627–1639. [Google Scholar] [CrossRef]
- Bessadok, A.; Marais, S.; Gouanve, F.; Colasse, L.; Zimmerlin, I.; Roudesli, S.; Metayer, M. Effect of chemical treatments of Alfa (Stipa tenacissima) fibres on water-sorption properties. Compos. Sci. Technol. 2007, 67, 685–697. [Google Scholar] [CrossRef]
- Senthilkumaar, S.; Varadarajan, P.R.; Porkodi, K.; Subbhuraam, C.V. Adsorption of methylene blue onto jute fiber carbon: Kinetics and equilibrium studies. J. Colloid Interface Sci. 2005, 284, 78–82. [Google Scholar] [CrossRef] [PubMed]
- Guechi, E.K.; Hamdaoui, O. Cattail leaves as a novel biosorbent for the removal of malachite green from liquid phase: Data analysis by non-linear technique. Desalin. Water Treat. 2013, 51, 3371–3380. [Google Scholar] [CrossRef]
- Vasanth, K.; Sivanesan, S.; Ramamurthi, V. Adsorption of malachite green onto Pithophora sp., a fresh water alga: Equilibrium and kinetic modelling. Process Biochem. 2005, 40, 2865–2872. [Google Scholar]
- Maksudur Rahman Khan, M.; Wasikur Rahman, M.; Ong, H.R.; Binti, A.; Cheng, I.C.K. Tea dust as a potential low-cost adsorbent for the removal of crystal violet from aqueous solution. Desalin. Water Treat. 2016, 57, 1–11. [Google Scholar]
- Nakkeeran, E.; Saranya, N.; Giri Nandagopal, M.S.; Santhiagu, A.; Selvaraju, N. Hexavalent chromium removal from aqueous solutions by a novel powder, prepared from Colocasia esculenta leaves. Int. J. Phytoremediat. 2016, 18, 812–821. [Google Scholar] [CrossRef]
- Khattri, S.D.; Singh, M.K. Removal of malachite green from dye wastewater using neem sawdust by adsorption. J. Hazard. Mater. 2009, 167, 1089–1094. [Google Scholar] [CrossRef]
- Mall, I.D.; Srivastava, V.C.; Agarwal, N.K.; Mishra, I.M. Adsorptive removal of malachite green dye from aqueous solution by bagasse fly ash and activated carbon-kinetic study and equilibrium isotherm analyses. Colloids Surf. A Physicochem. Eng. Asp. 2005, 264, 17–28. [Google Scholar] [CrossRef]
- Guechi, E.K.; Bendebane, F.; Aisset, A.; Malaoui, R. Sorption of mercury (II) from aqueous solution by Okoume sawdust. Desalin. Water Treat. 2012, 38, 285–292. [Google Scholar] [CrossRef]
- Niyaz, M.; Farhood, M.; Khorramfar, N.S.; Amini, F.; Arami, M. Synthesis, characterization and dye removal ability of high-capacity polymeric adsorbent: Polyaminoimide homopolymer. J. Hazard. Mater. 2011, 198, 87–94. [Google Scholar]
- Lakshmipathy, R.; Sarada, N.C. Adsorptive removal of basic cationic dyes from aqueous solution by chemically protonated watermelon (Citrullus lanatus) rind biomass. Desalin. Water Treat. 2014, 52, 6175–6184. [Google Scholar] [CrossRef]
- Bayramoglu, G.; Altintas, B.; Yakup Arica, M. Adsorption kinetics and thermodynamic parameters of cationic dyes from aqueous solutions by using a new strong cation-exchange resin. Chem. Eng. J. 2009, 152, 339–346. [Google Scholar] [CrossRef]
- Srivastava, S.K.; Singh, A.K.; Sharma, A. Studies on the uptake of lead and zinc by lignin obtained from black liquor, a paper industry waste material. Environ. Technol. 1994, 15, 353–361. [Google Scholar] [CrossRef]
- Hamdaoui, O.; Saoudi, F.; Chiha, M.; Naffrechoux, E. Sorption of malachite green by a novel sorbent, dead leaves of plane tree: Equilibrium and kinetic modeling. J. Chem. Eng. 2008, 143, 73–84. [Google Scholar] [CrossRef]
- Ghodbane, I.; Nouri, L.; Hamdaoui, O.; Chiha, M. Kinetic and equilibrium study for the sorption of cadmium (II) ions from aqueous phase by eucalyptus bark. J. Hazard. Mater. 2008, 152, 148–1582. [Google Scholar] [CrossRef]
- Altinisik, A.; Gur, E.; Seki, Y. A natural sorbent, Luffa cylindrical for the removal of a model basic dye. J. Hazard. Mater. 2010, 179, 658–664. [Google Scholar] [CrossRef]
- Hameed, B.H. Grass waste: A novel sorbent for the removal of basic dye from aqueous solution. J. Hazard. Mater. 2009, 166, 233–238. [Google Scholar] [CrossRef]
- Hameed, B.H.; El-Khaiary, M.I. Malachite green adsorption by rattan sawdust: Isotherm, kinetic and mechanism modeling. J. Hazard. Mater. 2008, 159, 574–579. [Google Scholar] [CrossRef]
- Allen, S.J.; Mckay, G.; Khader, K.Y.H. Intraparticle diffusion of a basic dye during adsorption onto sphagnum peat. Environ. Pollut. 1989, 56, 39–50. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, J.; Zhao, R.; Li, C.; Li, Y.; Zhang, C. Adsorption of basic dyes on activated carbon prepared from Polygonum orientale Linn: Equilibrium, kinetic and thermodynamic studies. Desalination 2010, 254, 68–74. [Google Scholar] [CrossRef]
- Nasuha, N.; Hameed, B.H.; Mohd Din, A.T. Rejected tea as a potential low-cost adsorbent for the removal of methylene blue. J. Hazard. Mater. 2010, 175, 126–132. [Google Scholar] [CrossRef]
- Coşkun, Y.; Aksuner, İ.; Yanik, N.J. Sandpaper Wastes as Adsorbent for the Removal of Brilliant Green and Malachite Green Dye. Acta Chim. Slov. 2019, 66, 402–413. [Google Scholar] [CrossRef] [PubMed]
- Yan, Y.; Han, H.; Dai, Y.; Zhu, H.; Liu, W.; Tang, X.; Gan, W.; Li, H. Nb2CTx MXene Nanosheets for Dye Adsorption. ACS Appl. Nano Mater. 2021, 11, 11763–11769. [Google Scholar] [CrossRef]
- Hamdaoui, O.; Naffrechoux, E. Modeling of adsorption isotherms of phenol and chlorophenols onto granular activated carbon Part I. Two-parameter models and equations allowing determination of thermodynamic parameters. J. Hazard. Mater. 2007, 147, 381–394. [Google Scholar] [CrossRef]
- Karim, A.H.; Jalil, A.; Triwahyono, A.S.; Sidik, S.M.; Kamarudin, N.H.N.; Jusoh, R.; Jusoh, N.W.C.; Hameed, B.H. Amino modified mesostructured silica nanoparticles for efficient adsorption of methylene blue. J. Colloid Interface Sci. 2012, 386, 307–314. [Google Scholar] [CrossRef]
- Horsfall, M.; Spiff, A.I. Equilibrium sorption study of Al3+, Co2+ and Ag2+ in aqueous solutions by fluted pumpkin (Telfairia occidentalis HOOK) waste biomass. Acta Chim. Slov. 2005, 52, 174–181. [Google Scholar]
- Debrassi, A.; Rodrigues, C.A. Adsorption of Cationic Dyes from Aqueous Solution by Termite Feces, a Non-Conventional Adsorbent. Clean–Soil Air Water 2011, 39, 549–556. [Google Scholar] [CrossRef]
- Annadurai, G.; Juang, R.S.; Lee, D.J. Use of cellulose-based wastes for adsorption of dyes from aqueous solutions. J. Hazard. Mater. 2002, 92, 263–274. [Google Scholar] [CrossRef] [PubMed]
- Ahmad, R. Studies on Adsorption of Crystal Violet Dye from Aqueous Solution onto Coniferous Pinus Bark Powder (CPBP). J. Hazard. Mater. 2009, 171, 767–773. [Google Scholar] [CrossRef]
- Silveira, M.B.; Pavan, S.F.A.; Gelos, N.F.; Lima, E.C.; Dias, S.L.P. Punica granatum Shell Preparation, Characterization, and Use for Crystal Violet Removal from Aqueous Solution. Clean–Soil Air Water 2014, 42, 939–946. [Google Scholar] [CrossRef]
- Khattri, S.D.; Singh, M.K. Colour removal from dye wastewater using sugar cane dust as an adsorbent. Adsorpt. Sci. Technol. 1999, 17, 269–282. [Google Scholar] [CrossRef]
- El-Sayed, G.O. Removal of Methylene Blue and Crystal Violet from Aqueous Solutions by Palm Kernel Fiber. Desalination 2011, 272, 225–232. [Google Scholar] [CrossRef]
- Pavan, F.A.; Camacho, E.S.; Lima, E.C.; Dotto, G.L.; Branco, V.T.A.; Dias, S.L.P. Formosa papaya seed powder (FPSP): Preparation, characterization and application as an alternative adsorbent for the removal of crystal violet from aqueous phase. J. Environ. Chem. Eng. 2014, 2, 230–238. [Google Scholar] [CrossRef]
- Madhavakrishnan, S.; Manickavasagam, K.; Vasanthakumar, R.; Resappan, K.; Mohanraj, R.; Pattabhi, S. Adsorption of Crystal Violet Dye from Aqueous Solution Using Ricinus communis Pericarp Carbon as an Adsorbent. J. Chem. 2009, 6, 1109–1116. [Google Scholar]
- Porkodi, K.; Kumar, K.V. Equilibrium, Kinetics and Mechanism Modeling and Simulation of Basic and Acid Dyes Sorption onto Jute Fiber Carbon: Eosin Yellow, Malachite Green and Crystal Violet Single Component Systems. J. Hazard. Mater. 2007, 143, 311–327. [Google Scholar] [CrossRef] [PubMed]
- Namasivayam, C.; Kumar, M.D.; Selvi, K.; Begum, R.A.; Vanathi, T.; Yamuna, R.T. Waste Coir Pith—A Potential Biomass for the Treatment of Dyeing Wastewaters. Biomass Bioenergy 2001, 21, 477–483. [Google Scholar] [CrossRef]
- Banerjee, S.; Sharma, Y.C. Equilibrium and kinetic studies for removal of malachite green from aqueous solution by a low cost activated carbon. J. Ind. Eng. Chem. 2013, 19, 1099–1105. [Google Scholar] [CrossRef]
- Singh, H.; Chauhan, G.; Jain, A.K.; Sharma, S.K. Adsorptive potential of agricultural wastes for removal of dyes from aqueous solutions. J. Environ. Chem. Eng. 2017, 5, 122–135. [Google Scholar] [CrossRef]
- Zhang, J.; Li, Y.; Zhang, C.; Jing, Y. Adsorption of malachite green from aqueous solution onto carbon prepared from Arundo donax root. J. Hazard. Mater. 2008, 150, 774–782. [Google Scholar] [CrossRef]
- Basar, C.A. Applicability of the various adsorption models of three dyes adsorption onto activated carbon prepared waste apricot. J. Hazard. Mater. 2006, 135, 232–241. [Google Scholar] [CrossRef]
- Sartape, A.S.; Mandhare, A.M.; Jadhav, V.V.; Raut, P.D.; Anuse, M.A.; Kolekar, S.S. Removal of malachite green dye from aqueous solution with adsorption technique using Limonia acidissima (wood apple) shell as low-cost adsorbent. Arab. J. Chem. 2017, 10, S3229–S3238. [Google Scholar] [CrossRef]
- Webber, T.W.; Chakkravorti, R.K. Pore and solid diffusion models for fixed-bed adsorbers. AlChE J. 1974, 20, 228–238. [Google Scholar] [CrossRef]
- Belala, Z.; Jeguirim, M.; Belhachemi, M.; Addoun, F.; Trouve, G. Biosorption of basic dye from aqueous solutions by Date Stones and Palm-Trees Waste: Kinetic, equilibrium and thermodynamic studies. Desalination 2011, 271, 80–87. [Google Scholar] [CrossRef]
- Boehm, H.P. Chemical identification of surface groups. Adv. Catal. 1966, 16, 179–274. [Google Scholar]
- Oickle, A.M.; Goertzen, S.L.; Hopper, K.R.; Abdalla, Y.O.; Andreas, H.A. Standardization of the Boehm titration. Part II. Method of agitation, effect of filtering and dilute titrant. Carbon 2010, 48, 3313–3322. [Google Scholar] [CrossRef]

| Component | Value |
|---|---|
| Ash | 2.5 |
| Extractives in: | |
| hot water | 4.88 |
| ethanol–toluene | 6 |
| ether | 2.03 |
| Lignin | 24.3 |
| Cellulose | 44.1 |
| Pentosans | 26.8 |
| Uronic acids | 4.66 |
| Concentration Groups | Carboxylic | Lactonic | Phenolic | Carbonylic and Quinonic | Acid | Basic | Total |
|---|---|---|---|---|---|---|---|
| Value (mequiv·g−1) | 0.008 | 0.340 | 1.395 | 2.213 | 3.948 | 0.242 | 4.190 |
| Dye | T (K) | ΔG° (kJ/mol) | ΔH° (kJ/mol) | ΔS° (kJ/mol·K) |
|---|---|---|---|---|
| MG | 298 | 5.52 | 1.99 | 25.3 |
| 308 | 2.25 | |||
| 318 | 2.5 | |||
| 328 | 2.61 | |||
| CV | 298 | 14.81 | 15.24 | 100.9 |
| 308 | 16.25 | |||
| 318 | 17.32 | |||
| 328 | 18.25 |
| Model | Initial MG Concentration (mg/L) | Initial CV Concentration (mg/L) | ||||||
|---|---|---|---|---|---|---|---|---|
| Pseudo-first order | 10 | 20 | 30 | 40 | 10 | 20 | 30 | 40 |
| qe (exp) (mg/g) | 3.23 | 6.48 | 8.67 | 8.67 | 3.55 | 7.11 | 9.91 | 13.75 |
| qe (cal) (mg/g) | 1.06 | 2.71 | 5.34 | 5.34 | 1.57 | 5.72 | 3.3 | 12.73 |
| k1 (min−1) | 0.02 | 0.021 | 0.025 | 0.025 | 0.04 | 0.045 | 0.021 | 0.032 |
| r | 0.981 | 0.997 | 0.988 | 0.988 | 0.978 | 0.972 | 0.968 | 0.967 |
| Pseudo-second order | 10 | 20 | 30 | 40 | 10 | 20 | 30 | 40 |
| k2 (g/mg min) | 0.062 | 0.027 | 0.004 | 0.038 | 0.076 | 0.019 | 0.006 | 0.009 |
| qe (cal) (mg/g) | 3.45 | 6.2 | 8.77 | 11..56 | 3.6 | 7.34 | 9.45 | 13.93 |
| h (mg/g min) | 0.187 | 0.328 | 0.249 | 0.0403 | 0.888 | 1.696 | 0.696 | 0.908 |
| r | 0.999 | 0.998 | 0.996 | 0.994 | 0.999 | 0.999 | 0.998 | 0.997 |
| Elovich | 10 | 20 | 30 | 40 | 10 | 20 | 30 | 40 |
| α (mg/g min) | 0.778 | 0.635 | 0.613 | 0.801 | 1.815 | 0.583 | 0.947 | 0.271 |
| β (g/mg) | 0.342 | 0.445 | 0.501 | 0.628 | 0.364 | 0.531 | 0.562 | 1.312 |
| r | 0.982 | 0.988 | 0.959 | 0.969 | 0.994 | 0.982 | 0.955 | 0.97 |
| qm (mg/g) | References | |
|---|---|---|
| Crystal violet | ||
| AC—from date palm leaflets | 36.63 | [1] |
| Modified Bambusa tulda | 20.84 | [9] |
| Termite feces | 75.71 | [57] |
| Banana peel | 12.2 | [58] |
| Coniferous pinus bark | 32.78 | [59] |
| Punica granatum shell | 50.21 | [60] |
| Sugarcane dust | 3.42 | [61] |
| Palm kernel fiber | 78.9 | [62] |
| Orange peel | 14.3 | [41] |
| Watermelon rind | 104.76 | [41] |
| Formosa papaya seed powder | 85.99 | [63] |
| Ricinus communis pericarp carbon | 48 | [64] |
| Jute fiber carbon | 27.74 | [65] |
| Coir pith | 2.56 | [66] |
| Alfa leaf powder (ALP) | 110.98 | This work |
| Malachite green | ||
| Wheat bran | 66.57 | [2] |
| Rice bran | 68.97 | [2] |
| Potato peel | 35.61 | [12] |
| Dead leaves of plane tree | 33.23 | [44] |
| Luffa cylindrical | 29.2 | [46] |
| Coconut coir activated carbon | 27.44 | [67] |
| Rattan sawdust | 62.71 | [48] |
| Citrus limetta peel | 8.73 | [68] |
| Zea mays cob | 16.72 | [69] |
| Arundo donax root carbon | 8.69 | [70] |
| AC prepared waste apricot | 116.27 | [71] |
| Wood apple shell | 34.56 | [72] |
| Alfa leaf powder | 90.1 | This work |
| Element | Value | Element | Value |
|---|---|---|---|
| MG | CV | ||
| Langmuir | qm (mg/g) | 90.1 | 110.98 |
| b × 103 (L/mg) | 6.3 | 8.99 | |
| r | 0.995 | 0.998 | |
| RL (40–400 mg/L) | 0.79–0.28 | 0.73–0.21 | |
| Freundlich | KF | 2.74 | 5.14 |
| nF | 2.1 | 2.7 | |
| r | 0.994 | 0.998 | |
| Harkins–Jura | A | 196.07 | 357.14 |
| B | 2.531 | 2.721 | |
| r | 0.949 | 0.951 | |
| Flory–Huggins | KF-H × 103 | 4.65 | 4.38 |
| nF-H | 0.51 | 0.602 | |
| r | 0.971 | 0.961 | |
| Kiselev | K1 | 0.013 | 0.0245 |
| Kn | −0.04 | −0.628 | |
| r | 0.932 | 0.943 | |
| Elovich | KE | 0.032 | 0.078 |
| qm | 24.63 | 23.47 | |
| r | 0.958 | 0.963 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ouettar, L.; Guechi, E.-K.; Hamdaoui, O.; Fertikh, N.; Saoudi, F.; Alghyamah, A. Biosorption of Triphenyl Methane Dyes (Malachite Green and Crystal Violet) from Aqueous Media by Alfa (Stipa tenacissima L.) Leaf Powder. Molecules 2023, 28, 3313. https://doi.org/10.3390/molecules28083313
Ouettar L, Guechi E-K, Hamdaoui O, Fertikh N, Saoudi F, Alghyamah A. Biosorption of Triphenyl Methane Dyes (Malachite Green and Crystal Violet) from Aqueous Media by Alfa (Stipa tenacissima L.) Leaf Powder. Molecules. 2023; 28(8):3313. https://doi.org/10.3390/molecules28083313
Chicago/Turabian StyleOuettar, Lamia, El-Khamssa Guechi, Oualid Hamdaoui, Nadia Fertikh, Fethi Saoudi, and Abudulaziz Alghyamah. 2023. "Biosorption of Triphenyl Methane Dyes (Malachite Green and Crystal Violet) from Aqueous Media by Alfa (Stipa tenacissima L.) Leaf Powder" Molecules 28, no. 8: 3313. https://doi.org/10.3390/molecules28083313
APA StyleOuettar, L., Guechi, E.-K., Hamdaoui, O., Fertikh, N., Saoudi, F., & Alghyamah, A. (2023). Biosorption of Triphenyl Methane Dyes (Malachite Green and Crystal Violet) from Aqueous Media by Alfa (Stipa tenacissima L.) Leaf Powder. Molecules, 28(8), 3313. https://doi.org/10.3390/molecules28083313







