In-Depth Performance Analysis and Comparison of Monolithic and Particulate Zwitterionic Hydrophilic Interaction Liquid Chromatography Polymer Columns
Abstract
1. Introduction
2. Results and Discussion
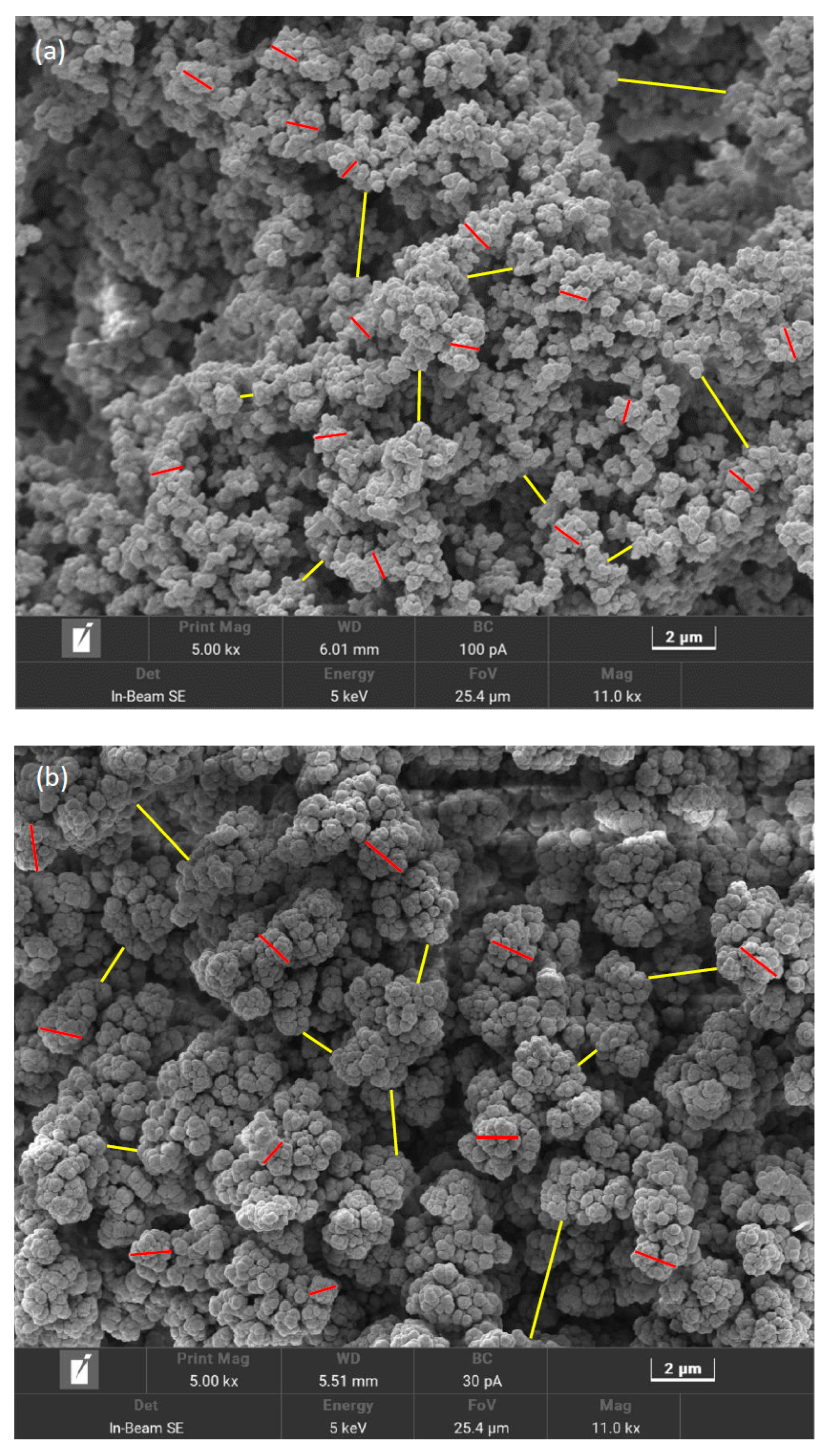
2.1. Geometrical Characterization
2.2. Evaluation of Column Performance
2.2.1. Plate Height Curves
2.2.2. Permeability Measurements
2.2.3. Reduced Plate Height Curves
2.2.4. Kinetic Plot Analysis
3. Materials and Methods
3.1. Reagents and Materials
3.2. Instrumentation
3.3. Samples and Mobile Phases
3.4. Plate Height Measurements
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bayatloo, M.R.; Tabani, H.; Nojavan, S.; Alexovič, M.; Ozkan, S.A. Liquid-Phase Microextraction Approaches for Preconcentration and Analysis of Chiral Compounds: A Review on Current Advances. Crit. Rev. Anal. Chem. 2022, 1–15. [Google Scholar]
- Schiesel, S.; Lämmerhofer, M. Multitarget quantitative metabolic profiling of hydrophilic metabolites in fermentation broths of β -lactam antibiotics production by HILIC–ESI–MS/MS. Anal. Bioanal. Chem. 2010, 396, 1655–1679. [Google Scholar] [CrossRef] [PubMed]
- Nelis, M.; Decraecker, L.; Boeckxstaens, G.; Augustijns, P.; Cabooter, D. Development of a HILIC-MS/MS method for the quantification of histamine and its main metabolites in human urine samples. Talanta 2020, 220, 121328. [Google Scholar] [CrossRef] [PubMed]
- Díez, C.; Guillarme, D.; Spörri, A.S.; Cognard, E.; Ortelli, D.; Edder, P.; Rudaz, S. Aminoglycoside analysis in food of animal origin with a zwitterionic stationary phase and liquid chromatography–tandem mass spectrometry. Anal. Chim. Acta 2015, 882, 127–139. [Google Scholar] [CrossRef] [PubMed]
- Kahsay, G.; Song, H.; Van Schepdael, A.; Cabooter, D.; Adams, E. Hydrophilic interaction chromatography (HILIC) in the analysis of antibiotics. J. Pharm. Biomed. Anal. 2014, 87, 142–154. [Google Scholar] [CrossRef]
- Ikegami, T.; Horie, K.; Saad, N. Highly efficient analysis of underivatized carbohydrates using monolithic-silica-based capillary hydrophilic interaction (HILIC) HPLC. Anal. Bioanal. Chem. 2008, 391, 2533–2542. [Google Scholar] [CrossRef]
- Spicer, V.; Krokhin, O.V. Peptide retention time prediction in hydrophilic interaction liquid chromatography. Comparison of separation selectivity between bare silica and bonded stationary phases. J. Chromatogr. A 2018, 1534, 75–84. [Google Scholar] [CrossRef]
- Gilar, M.; Jaworski, A. Retention behavior of peptides in hydrophilic-interaction chromatography. J. Chromatogr. A 2011, 1218, 8890–8896. [Google Scholar] [CrossRef]
- Periat, A.; Fekete, S.; Cusumano, A.; Veuthey, J.; Beck, A.; Lauber, M.; Guillarme, D. Potential of hydrophilic interaction chromatography for the analytical characterization of protein biopharmaceuticals. J. Chromatogr. A 2016, 1448, 81–92. [Google Scholar] [CrossRef]
- Alpert, A.J. Hydrophilic-interaction chromatography for the separation of peptides, nucleic acids and other polar compounds. J. Chromatogr. A 1990, 499, 177–196. [Google Scholar] [CrossRef]
- McCalley, D.V.; Neue, U.D. Estimation of the extent of the water-rich layer associated with the silica surface in hydrophilic interaction chromatography. J. Chromatogr. A 2008, 1192, 225–229. [Google Scholar] [CrossRef] [PubMed]
- Buszewski, B.; Noga, S. Hydrophilic interaction liquid chromatography (HILIC)-a powerful separation technique. Anal. Bioanal. Chem. 2012, 402, 231–247. [Google Scholar] [CrossRef] [PubMed]
- Jandera, P. Stationary and mobile phases in hydrophilic interaction chromatography: A review. Anal. Chim. Acta 2011, 692, 1–25. [Google Scholar] [CrossRef] [PubMed]
- Heaton, J.C.; Mccalley, D.V. Comparison of the kinetic performance and retentivity of sub-2 µm core–shell, hybrid and conventional bare silica phases in hydrophilic interaction chromatography. J. Chromatogr. A 2014, 1371, 106–116. [Google Scholar] [CrossRef] [PubMed]
- Chauve, B.; Guillarme, D.; Cléon, P.; Veuthey, J.L. Evaluation of various HILIC materials for the fast separation of polar compounds. J. Sep. Sci. 2010, 33, 752–764. [Google Scholar] [CrossRef]
- Jiang, Z.; William, N.; Liu, Z. Preparation and application of hydrophilic monolithic columns. J. Chromatogr. A 2011, 1218, 2350–2361. [Google Scholar] [CrossRef]
- Desmet, G.; Broeckhoven, K. Equivalence of the different Cm- and Cs-term expressions used in liquid chromatography and a geometrical model uniting them. Anal. Chem. 2008, 80, 8076–8088. [Google Scholar] [CrossRef]
- Giddings, C. Dynamics of Chromatography: Principles and Theory; CRC Press: New York, NY, USA, 1965. [Google Scholar]
- Lapidus, L.; Amundson, N.R. Mathematics of Adsorption in Beds. VI. The Effect of Longitudinal Diffusion in Ion Exchange and Chromatographic Columns. J. Phys. Chem. 1952, 56, 984–988. [Google Scholar] [CrossRef]
- Horvath, C.; Lin, H.-J. Movement and band spreading liquid chromatography. J. Chromatogr. A 1976, 126, 401–420. [Google Scholar] [CrossRef]
- Li, H.; Desmet, G.; Jiang, Z.; Cabooter, D. On the occurrence of very low intra-particle diffusion rates in zwitterionic hydrophilic interaction liquid chromatography polymer columns. J. Chromatogr. A 2022, 1683, 463531. [Google Scholar] [CrossRef]
- Sohn, H.Y.; Moreland, C. The effect of particle size distribution on packing density. Can. J. Chem. Eng. 1968, 46, 162–167. [Google Scholar] [CrossRef]
- Afeyan, N.B.; Gordon, N.; Mazsaroff, I.; Varady, L.; Fulton, S.P.; Yang, Y.B.; Regnier, F.E. Flow-through particles for the high-performance liquid chromatographic separation of biomolecules: Perfusion chromatography. J. Chromatogr. A 1990, 519, 1–29. [Google Scholar] [CrossRef]
- Desmet, G.; Song, H.; Makey, D.; Stoll, D.R.; Cabooter, D. Experimental investigation of the retention factor dependency of eddy dispersion in packed bed columns and relation to knox’s empirical model parameters. J. Chromatogr. A 2020, 1626, 461339. [Google Scholar] [CrossRef]
- Deridder, S.; Desmet, G. New insights in the velocity dependency of the external mass transfer coefficient in 2D and 3D porous media for liquid chromatography. J. Chromatogr. A 2012, 1227, 194–202. [Google Scholar] [CrossRef] [PubMed]
- Bear, J. Dynamics of Fluids in Porous Media; Dover Publications: New York, NY, USA, 1988. [Google Scholar]
- Halasz, I.; Görlitz, G. Optimal Parameters in High Speed Liquid Chromatography (HPLC). Angew. Chem. Int. Ed. 1982, 21, 50–61. [Google Scholar] [CrossRef]
- Desmet, G.; Broeckhoven, K.; De Smet, J.; Deridder, S.; Baron, G.V.; Gzil, P. Errors involved in the existing B-term expressions for the longitudinal diffusion in fully porous chromatographic media Part I: Computational data in ordered pillar arrays and effective medium theory. J. Chromatogr. A 2008, 1188, 171–188. [Google Scholar] [CrossRef]
- De Villiers, A.; Lestremau, F.; Szucs, R.; Gélébart, S.; David, F.; Sandra, P. Evaluation of ultra performance liquid chromatography. Part I. Possibilities and limitations. J. Chromatogr. A 2006, 1127, 60–69. [Google Scholar] [CrossRef]
- Hsieh, S.; Jorgenson, J.W. Preparation and evaluation of slurry-packed liquid chromatography microcolumns with inner diameters from 12 to 33 μm. Anal. Chem. 1996, 68, 1212–1217. [Google Scholar] [CrossRef]
- Desmet, G.; Clicq, D.; Gzil, P. Geometry-independent plate height representation methods for the direct comparison of the kinetic performance of LC supports with a different size or morphology. Anal. Chem. 2005, 77, 4058–4070. [Google Scholar] [CrossRef]
- Clicq, D.; Heinisch, S.; Rocca, J.L.; Cabooter, D.; Gzil, P.; Desmet, G. Use of the kinetic plot method to analyze commercial high-temperature liquid chromatography systems II. Practically constrained performance comparison. J. Chromatogr. A 2007, 1146, 193–201. [Google Scholar] [CrossRef]
- Eeltink, S.; Decrop, W.M.C.; Steiner, F.; Ursem, M.; Cabooter, D.; Desmet, G.; Kok, W.T. Use of kinetic plots for the optimization of the separation time in ultra-high-pressure LC. J. Sep. Sci. 2010, 33, 2629–2635. [Google Scholar] [CrossRef] [PubMed]
- Jiang, Z.; Smith, N.W.; Ferguson, P.D.; Taylor, M.R. Hydrophilic interaction chromatography using methacrylate-based monolithic capillary column for the separation of polar analytes. Anal. Chem. 2007, 79, 1243–1250. [Google Scholar] [CrossRef] [PubMed]
- Pena-Pereira, F.; Wojnowski, W.; Tobiszewski, M. AGREE—Analytical GREEnness Metric Approach and Software. Anal. Chem. 2020, 92, 10076–10082. [Google Scholar] [CrossRef] [PubMed]
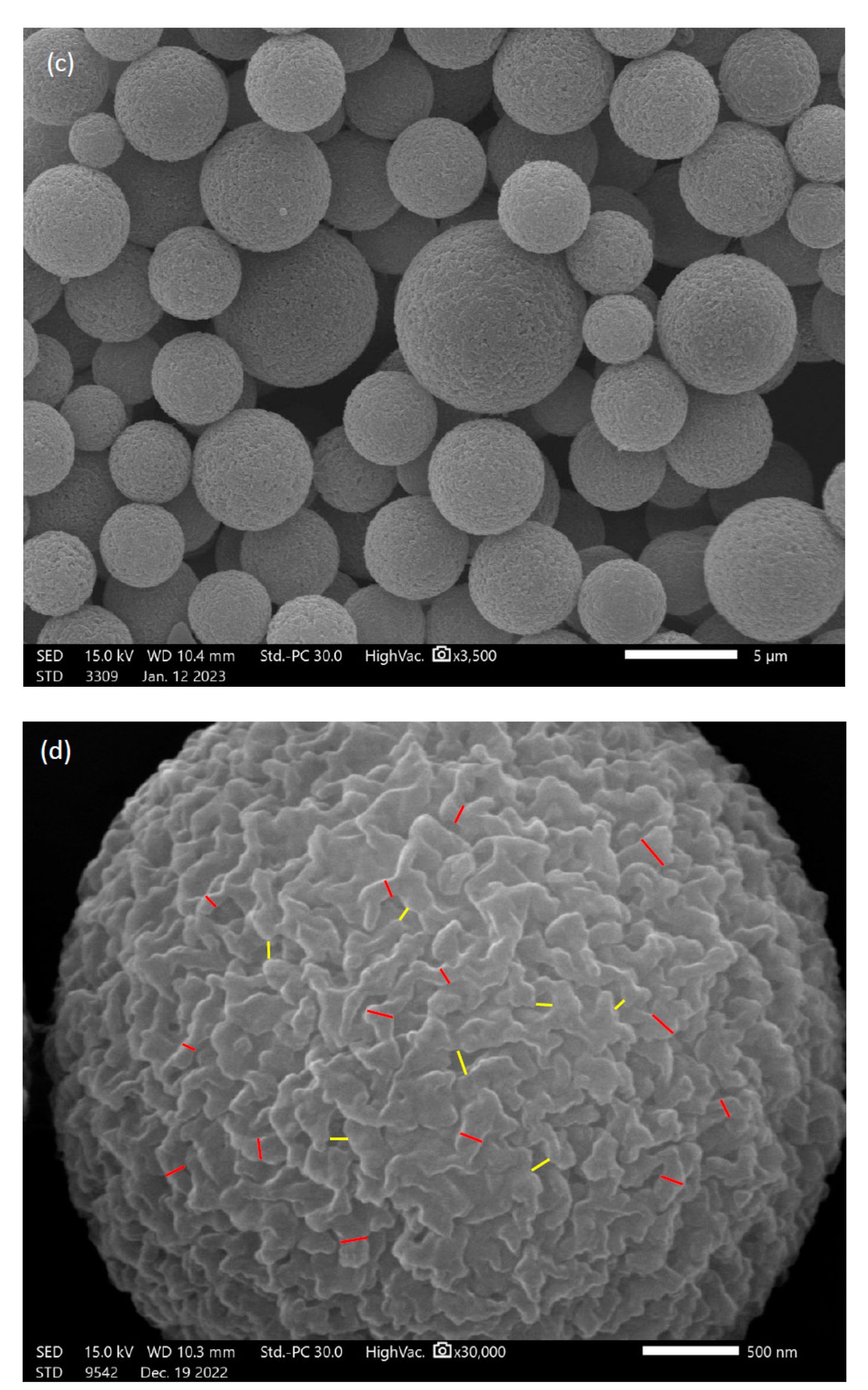

 ), k″ = 2.9–3.7 (
), k″ = 2.9–3.7 ( ), k″ = 4.7–6.4 (
), k″ = 4.7–6.4 ( ), k″ = 6.4–7.3 (
), k″ = 6.4–7.3 ( ), k″ = 9.7–10.5 (
), k″ = 9.7–10.5 ( ). Mobile-phase conditions are given in Table S1.
). Mobile-phase conditions are given in Table S1.
 ), k″ = 2.9–3.7 (
), k″ = 2.9–3.7 ( ), k″ = 4.7–6.4 (
), k″ = 4.7–6.4 ( ), k″ = 6.4–7.3 (
), k″ = 6.4–7.3 ( ), k″ = 9.7–10.5 (
), k″ = 9.7–10.5 ( ). Mobile-phase conditions are given in Table S1.
). Mobile-phase conditions are given in Table S1.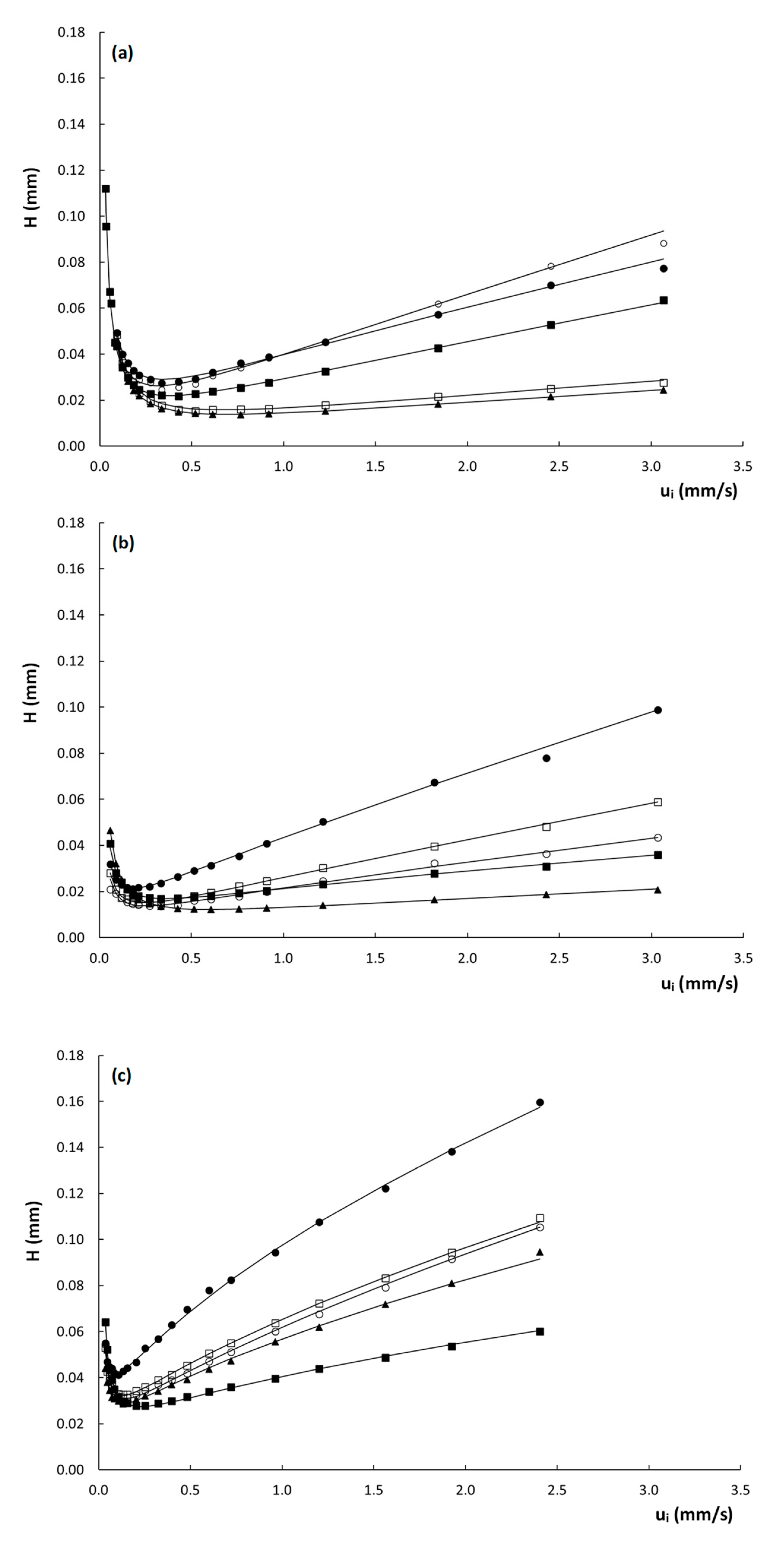
 ) poly(SPE-co-EDMA) monolithic column, (
) poly(SPE-co-EDMA) monolithic column, ( ) poly(SPE-co-MBA) monolithic column, (
) poly(SPE-co-MBA) monolithic column, ( ) ZIC-pHILIC column. The error bars are the standard deviations obtained from three replicate measurements.
) ZIC-pHILIC column. The error bars are the standard deviations obtained from three replicate measurements.
 ) poly(SPE-co-EDMA) monolithic column, (
) poly(SPE-co-EDMA) monolithic column, ( ) poly(SPE-co-MBA) monolithic column, (
) poly(SPE-co-MBA) monolithic column, ( ) ZIC-pHILIC column. The error bars are the standard deviations obtained from three replicate measurements.
) ZIC-pHILIC column. The error bars are the standard deviations obtained from three replicate measurements.
 ), k″= 2.9–3.7 (
), k″= 2.9–3.7 ( ), k″= 4.7–6.4 (
), k″= 4.7–6.4 ( ), k″= 6.4–7.3 (
), k″= 6.4–7.3 ( ), k″= 9.7–10.5 (
), k″= 9.7–10.5 ( ). Mobile-phase conditions as in Table S1.
). Mobile-phase conditions as in Table S1.
 ), k″= 2.9–3.7 (
), k″= 2.9–3.7 ( ), k″= 4.7–6.4 (
), k″= 4.7–6.4 ( ), k″= 6.4–7.3 (
), k″= 6.4–7.3 ( ), k″= 9.7–10.5 (
), k″= 9.7–10.5 ( ). Mobile-phase conditions as in Table S1.
). Mobile-phase conditions as in Table S1.

 ) poly(SPE-co-EDMA) monolithic column, (
) poly(SPE-co-EDMA) monolithic column, ( ) poly(SPE-co-MBA) monolithic column, (
) poly(SPE-co-MBA) monolithic column, ( ) ZIC-pHILIC column. Component = uracil, having k″ ≅ 2 on all three materials.
) ZIC-pHILIC column. Component = uracil, having k″ ≅ 2 on all three materials.
 ) poly(SPE-co-EDMA) monolithic column, (
) poly(SPE-co-EDMA) monolithic column, ( ) poly(SPE-co-MBA) monolithic column, (
) poly(SPE-co-MBA) monolithic column, ( ) ZIC-pHILIC column. Component = uracil, having k″ ≅ 2 on all three materials.
) ZIC-pHILIC column. Component = uracil, having k″ ≅ 2 on all three materials.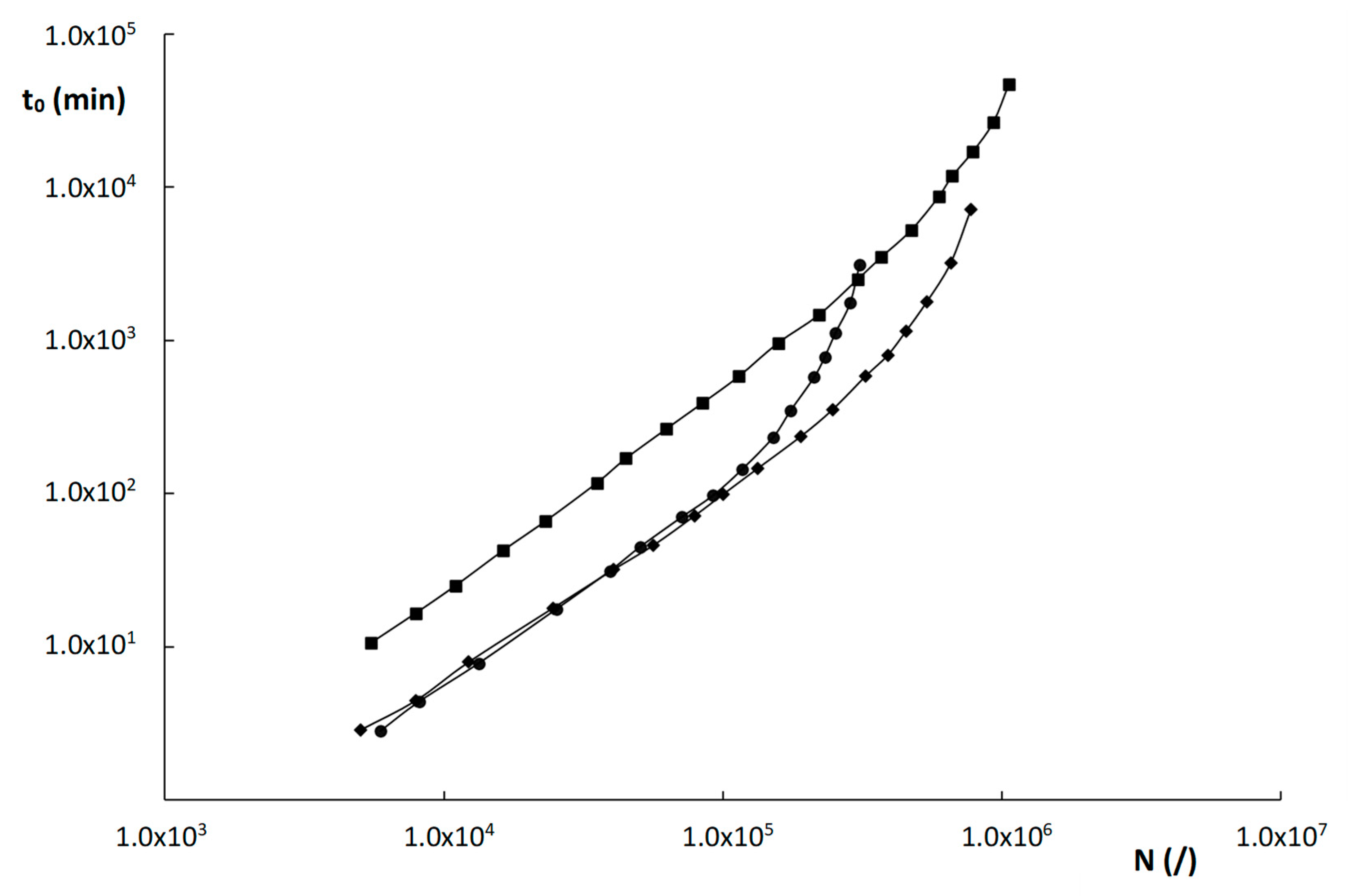
| Column | (µm) | (µm) | or (µm) | dHalasz (µm) | |||
|---|---|---|---|---|---|---|---|
| poly(SPE-co-EDMA) | 0.7 ± 0.1 | 0.8 ± 0.4 | 1.5 ± 0.4 | 5.2 | 0.6923 | 0.7830 | 0.2948 |
| poly(SPE-co-MBA) | 1.7 ± 0.3 | 1.6 ± 0.7 | 3.3 ± 0.8 | 5.7 | 0.6995 | 0.7370 | 0.1248 |
| ZIC-pHILIC | / | / | 4.7 ± 1.1 | 5.5 | 0.4398 | 0.6040 | 0.2931 |
| Column | Compound | k″ | Hmin (mm) | Equation (10) BFIT | Equation (10) BPP | ||||
|---|---|---|---|---|---|---|---|---|---|
| A (mm1/2/s1/2) | B (mm²/s) | C (s) | A (mm1/2/s1/2) | B (mm²/s) | C (s) | ||||
| SPE-co-EDMA | Uracil | 1.92 | 2.17 × 10−2 | 1.38 × 10−2 | 3.35 × 10−3 | 1.22 × 10−2 | 1.25 × 10−2 | 3.48 × 10−3 | 1.30 × 10−2 |
| Uracil | 3.08 | 2.76 × 10−2 | 2.29 × 10−2 | 3.80 × 10−3 | 1.31 × 10−2 | 2.89 × 10−2 | 3.27 × 10−3 | 8.74 × 10−3 | |
| Thiourea | 4.96 | 1.37 × 10−2 | 6.90 × 10−3 | 3.83 × 10−3 | 3.71 × 10−3 | 7.59 × 10−3 | 3.70 × 10−3 | 3.21 × 10−3 | |
| Thiourea | 7.00 | 1.53 × 10−2 | 8.43 × 10−3 | 4.11 × 10−3 | 4.11 × 10−3 | 9.53 × 10−3 | 3.91 × 10−3 | 3.42 × 10−3 | |
| Hypoxanthine | 10.29 | 2.47 × 10−2 | 1.46 × 10−2 | 3.53 × 10−3 | 2.18 × 10−2 | 1.39 × 10−2 | 3.64 × 10−3 | 2.23 × 10−2 | |
| SPE-co-MBA | Uracil | 1.86 | 1.70 × 10−2 | 1.78 × 10−2 | 2.08 × 10−3 | 1.38 × 10−3 | 1.56 × 10−2 | 2.31 × 10−3 | 2.80 × 10−3 |
| Adenosine | 2.93 | 2.12 × 10−2 | 2.22 × 10−2 | 1.53 × 10−3 | 1.96 × 10−2 | 1.96 × 10−2 | 1.80 × 10−3 | 2.11 × 10−2 | |
| Thiourea | 4.73 | 1.22 × 10−2 | 8.80 × 10−3 | 2.66 × 10−3 | 1.63 × 10−3 | 6.25 × 10−3 | 3.05 × 10−3 | 3.20 × 10−3 | |
| Uridine | 6.37 | 1.48 × 10−2 | 1.25 × 10−2 | 1.47 × 10−3 | 1.21 × 10−2 | 1.21 × 10−2 | 1.72 × 10−3 | 1.20 × 10−2 | |
| Inosine | 9.69 | 1.36 × 10−2 | 1.42 × 10−2 | 1.30 × 10−3 | 5.98 × 10−3 | 1.41 × 10−2 | 1.25 × 10−3 | 6.04 × 10−3 | |
| ZIC-pHILIC | Uracil | 2.18 | 2.78 × 10−2 | 3.84 × 10−2 | 2.07 × 10−3 | 0.00 | 3.92 × 10−2 | 1.84 × 10−3 | 0.00 |
| Adenosine | 3.70 | 4.11 × 10−2 | 8.70 × 10−2 | 1.37 × 10−3 | 9.12 × 10−3 | 8.31 × 10−2 | 1.50 × 10−3 | 1.26 × 10−2 | |
| Uridine | 6.39 | 2.87 × 10−2 | 4.98 × 10−2 | 1.29 × 10−3 | 5.71 × 10−3 | 4.42 × 10−2 | 1.61 × 10−3 | 1.05 × 10−2 | |
| Uridine | 7.31 | 3.26 × 10−2 | 5.45 × 10−2 | 1.50 × 10−3 | 9.31 × 10−3 | 5.84 × 10−2 | 1.25 × 10−3 | 5.97 × 10−3 | |
| Uridine | 10.54 | 3.14 × 10−2 | 4.71 × 10−2 | 1.62 × 10−3 | 1.31 × 10−2 | 5.33 × 10−2 | 1.33 × 10−3 | 7.41 × 10−3 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Jiang, Z.; Desmet, G.; Cabooter, D. In-Depth Performance Analysis and Comparison of Monolithic and Particulate Zwitterionic Hydrophilic Interaction Liquid Chromatography Polymer Columns. Molecules 2023, 28, 2902. https://doi.org/10.3390/molecules28072902
Li H, Jiang Z, Desmet G, Cabooter D. In-Depth Performance Analysis and Comparison of Monolithic and Particulate Zwitterionic Hydrophilic Interaction Liquid Chromatography Polymer Columns. Molecules. 2023; 28(7):2902. https://doi.org/10.3390/molecules28072902
Chicago/Turabian StyleLi, Haibin, Zhengjin Jiang, Gert Desmet, and Deirdre Cabooter. 2023. "In-Depth Performance Analysis and Comparison of Monolithic and Particulate Zwitterionic Hydrophilic Interaction Liquid Chromatography Polymer Columns" Molecules 28, no. 7: 2902. https://doi.org/10.3390/molecules28072902
APA StyleLi, H., Jiang, Z., Desmet, G., & Cabooter, D. (2023). In-Depth Performance Analysis and Comparison of Monolithic and Particulate Zwitterionic Hydrophilic Interaction Liquid Chromatography Polymer Columns. Molecules, 28(7), 2902. https://doi.org/10.3390/molecules28072902








