Electronic, Optical, Thermoelectric and Elastic Properties of RbxCs1−xPbBr3 Perovskite
Abstract
1. Introduction
2. Results and Discussion
2.1. Structural Properties
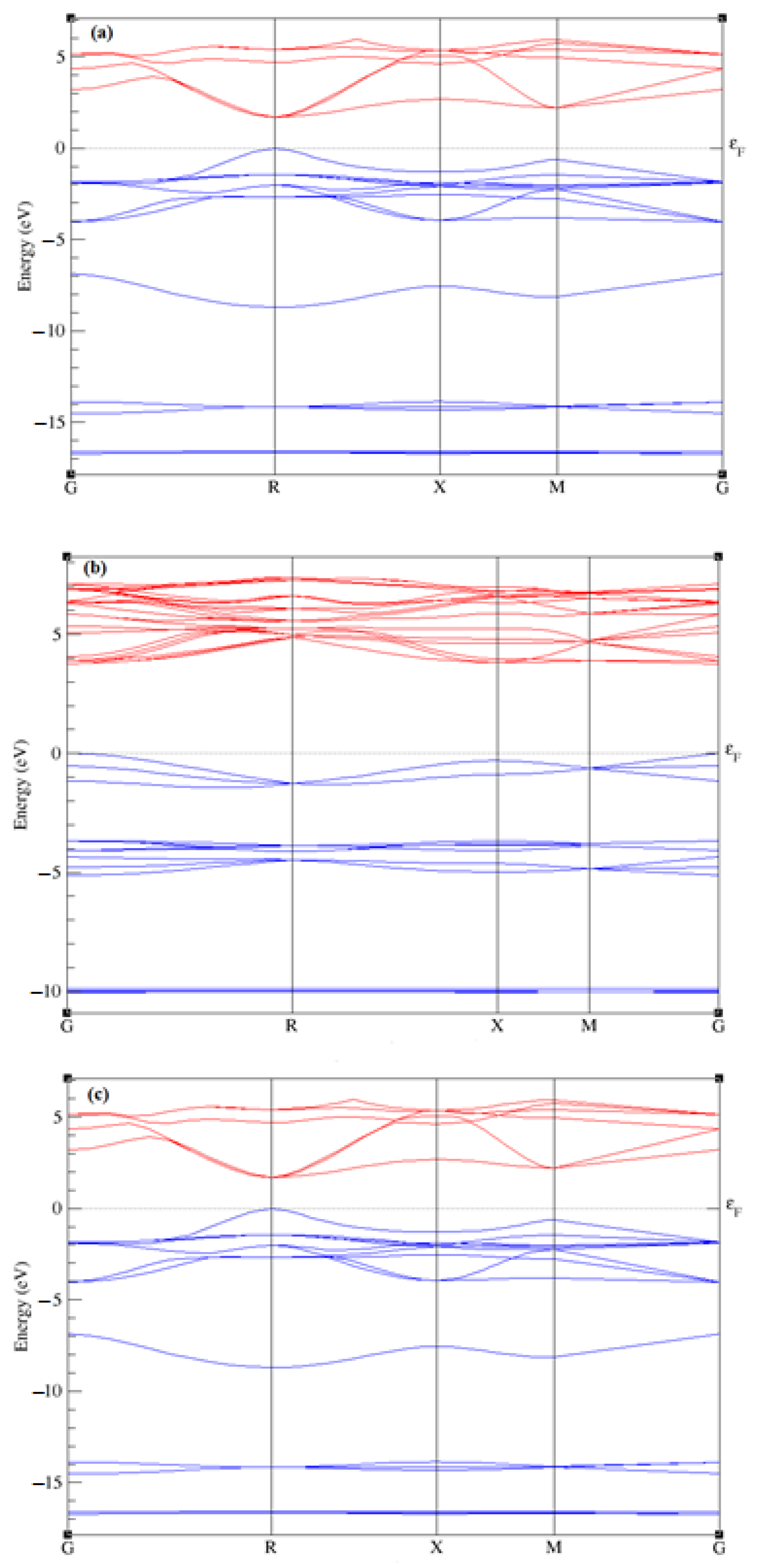
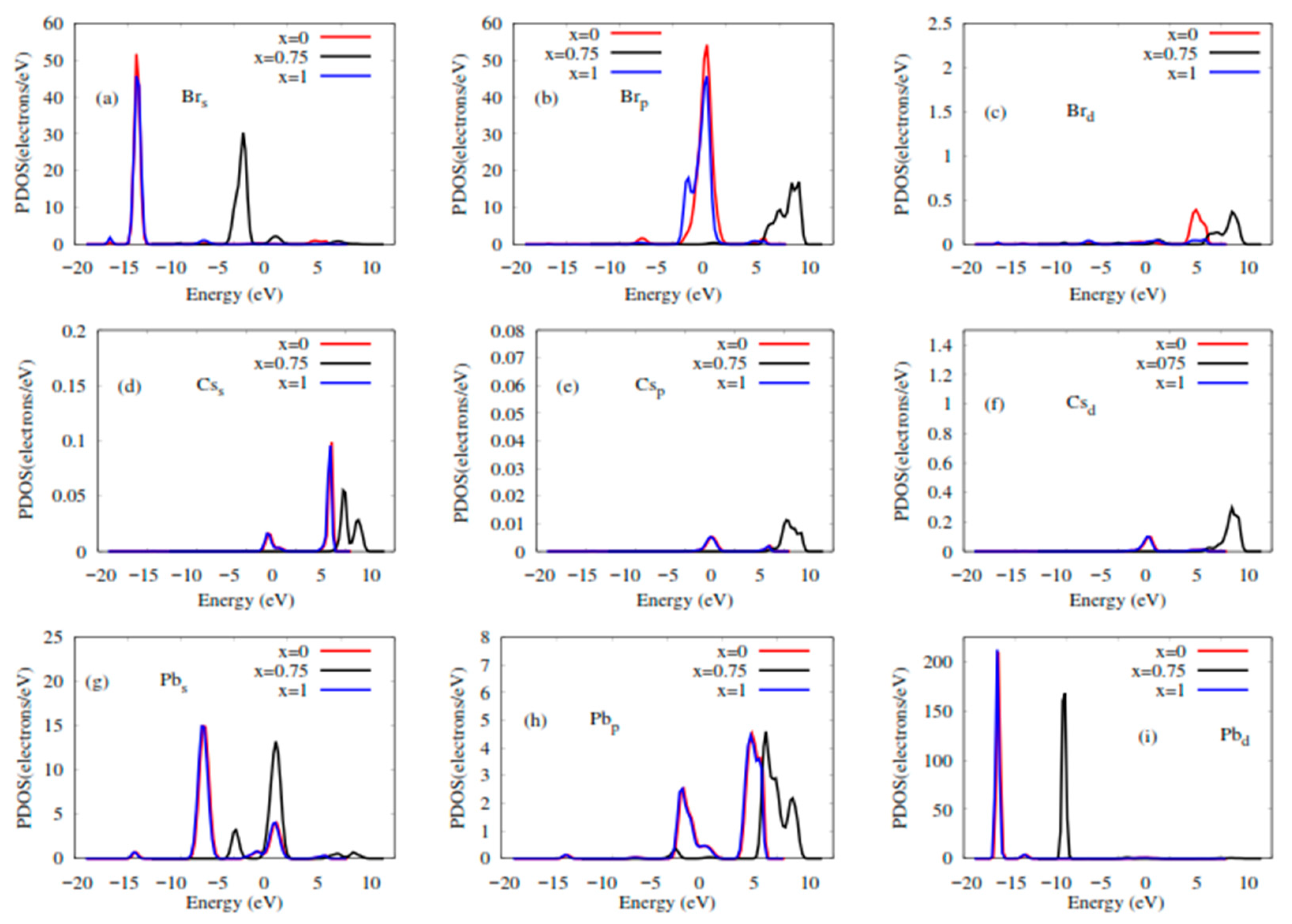
2.2. Elastic and Electronic Properties
2.3. Optical Properties
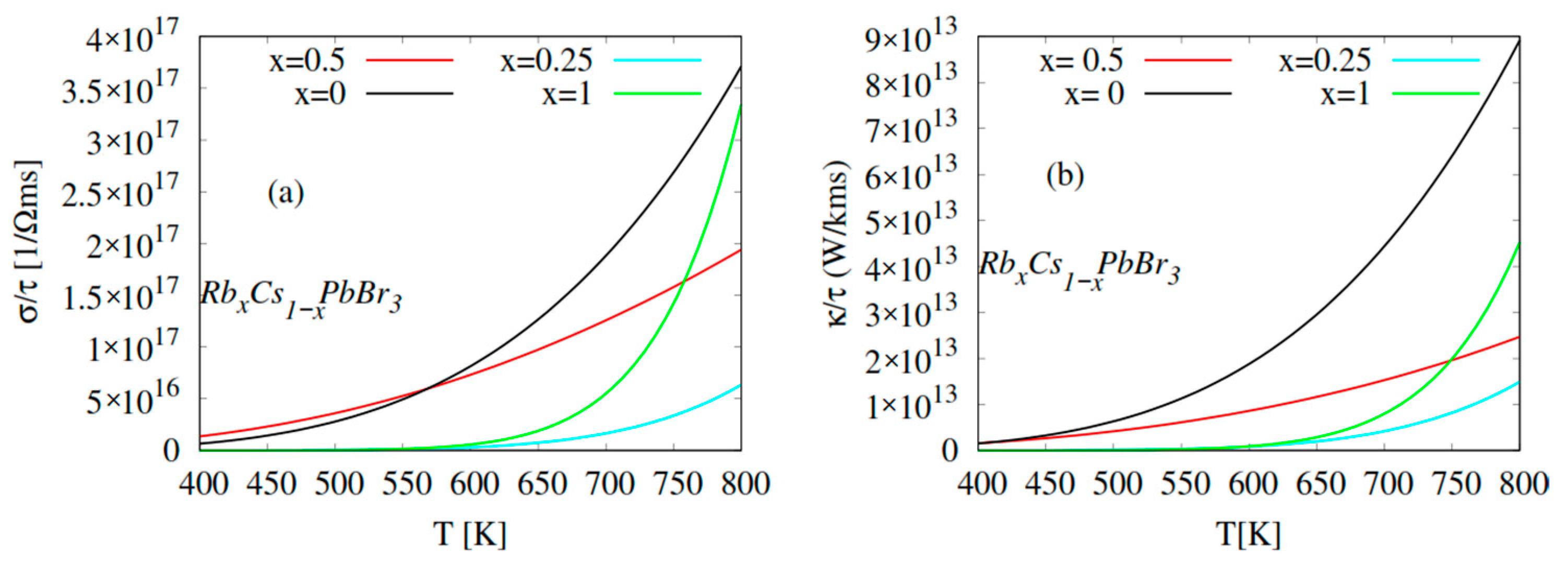
2.4. Thermoelectric Properties
3. Materials and Methods
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Gao, Y.; Wu, Y.; Lu, H.; Chen, C.; Liu, Y.; Bai, X.; Yang, L.; Yu, W.W.; Dai, Q.; Zhang, Y. CsPbBr3 perovskite nanoparticles as additive for environmentally stable perovskite solar cells with 20.46% efficiency. Nano Energy 2019, 59, 517–526. [Google Scholar] [CrossRef]
- Chung, I.; Lee, B.; He, J.; Chang, R.P.H.; Kanatzidis, M.G. All-solid-state dye-sensitized solar cells with high efficiency. Nature 2012, 485, 486–489. [Google Scholar] [CrossRef] [PubMed]
- Ge, Y.; Ye, F.; Xiao, M.; Wang, H.; Wang, C.; Liang, J.; Hu, X.; Guan, H.; Cui, H.; Ke, W.; et al. Internal Encapsu-lation for Lead Halide Perovskite Films for Efficient and Very Stable Solar Cells. Adv. Energy Mater. 2022, 12, 2200361. [Google Scholar] [CrossRef]
- Kim, Y.H.; Cho, H.; Heo, J.H.; Kim, T.S.; Myoung, N.; Lee, C.L.; Im, S.H.; Lee, T.W. Multicolored Organic/Inorganic Hybrid Perovskite Light-Emitting Diodes. Adv. Mater. 2015, 27, 1248. [Google Scholar] [CrossRef] [PubMed]
- Stranks, S.D.; Snaith, H.J. Metal-halide perovskites for photovoltaic and light-emitting devices. Nat. Nanotechnol. 2015, 10, 391–402. [Google Scholar] [CrossRef] [PubMed]
- Saidaminov, M.I.; Adinolfi, V.; Comin, R.; Abdelhady, A.L.; Peng, W.; Dursun, I.; Yuan, M.; Hoogland, S.; Sargent, E.H.; Bakret, O.M. Planar-integrated single-crystalline perovskite photodetectors. Nat. Commun. 2015, 6, 8724. [Google Scholar] [CrossRef] [PubMed]
- Hong, E.; Li, Z.; Yan, T.; Fang, X. Surface-Tension-Dominant Crystallization of 2D Perovskite Single Crystals for Vertically Oriented Hetero-/Homo-Structure Photodetectors. Nano Lett. 2022, 22, 8662. [Google Scholar] [CrossRef] [PubMed]
- Dong, K.; Zhou, H.; Xiao, M.; Gui, P.; Gao, Z.; Yao, F.; Shao, W.; Liu, C.; Tao, C.; Ke, W.; et al. Semi-transparent, high-performance lead-free Cs3Bi2I9 single crystal self-driven photodetector. Appl. Phys. Lett. 2022, 120, 191102. [Google Scholar] [CrossRef]
- Li, Z.; Hong, E.; Zhang, X.; Deng, M.; Fang, X. Perovskite-Type 2D Materials for High-Performance Photodetectors. J. Phys. Chem. Lett. 2022, 13, 1215–1225. [Google Scholar] [CrossRef]
- Li, Z.; Liu, X.; Zuo, C.; Yang, W.; Fang, X. Supersaturation-Controlled Growth of Monolithically Integrated Lead-Free Halide Perovskite Single-Crystalline Thin Film for High-Sensitivity Photodetectors. Adv. Mater. 2021, 33, 2103010. [Google Scholar] [CrossRef]
- Liu, Y.; Sun, J.; Yang, Z.; Yang, D.; Ren, X.; Xu, H.; Yang, Z.; Liu, S.F. 20-mm-Large Single-Crystalline Formamidinium-Perovskite Wafer for Mass Production of Integrated Photodetectors. Adv. Opt. Mater. 2016, 4, 1829–1837. [Google Scholar] [CrossRef]
- Chen, S.; Roh, K.; Lee, J.; Chong, W.K.; Lu, Y.; Mathews, N.; Sum, T.C.; Nurmikko, A. A Photonic Crystal Laser from Solution Based Organo-Lead Iodide Perovskite Thin Films. ACS Nano 2016, 10, 3959–3967. [Google Scholar] [CrossRef] [PubMed]
- Babu, K.E.; Murali, N.; Babu, K.V.; Shibeshi, P.T.; Veeraiah, V. Structural, Elastic, Electronic, and Optical Properties of Cubic Perovskite CsCaCl3 Compound: An ab initio Study. Act. Phys. Pol. A 2014, 125, 1179. [Google Scholar] [CrossRef]
- Clark, S.J.; Donaldson, J.D.; Harvey, J.A. Evidence for the Direct Population of Solid-state Bands by Nonbonding Electron Pairs in Compounds of the Type CsM”X, (M” = Ge, Sn, Pb; X=CI, Br, I). J. Mater. Chem. 1995, 5, 1813. [Google Scholar] [CrossRef]
- Chang, Y.H.; Park, C.H.; Matsuishi, K. First-principles study of the Structural and the electronic properties of the lead-Halide-based inorganic-organic perovskites (CH3NH3) PbX3 and CsPbX3 (X = Cl, Br, I). Korean Phys. Soc. 2004, 44, 889. [Google Scholar]
- Zhao, Y.Q.; Ma, Q.R.; Liu, B.; Yu, Z.L.; Cai, M.Q. Pressure-induced strong ferroelectric polarization in tetra-phase perovskite CsPbBr3. Phys. Chem. Chem. Phys. 2018, 20, 14718. [Google Scholar] [CrossRef] [PubMed]
- Stroppa, A.; Quarti, C.; Angelis, F.D.; Picozzi, S. Ferroelectric Polarization of CH3NH3PbI3: A Detailed Study Based on Density Functional Theory and Symmetry Mode Analysis. J. Phys. Chem. Lett. 2015, 6, 2223. [Google Scholar] [CrossRef]
- Yang, Y.; You, J. Make perovskite solar cells stable. Nature 2017, 544, 155–156. [Google Scholar] [CrossRef]
- Han, Y.; Meyer, S.; Dkhissi, Y.; Weber, K.; Pringle, J.M.; Bach, U.; Spiccia, L.; Cheng, Y.B. Degradation observations of en-capsulated planar CH3NH3PbI3 perovskite solar cells at high temperatures and humidity. J. Mater. Chem. A 2015, 3, 8139. [Google Scholar] [CrossRef]
- Xiao, C.; Li, Z.; Guthrey, H.; Moseley, J.; Yang, Y.; Wozny, S.; Moutinho, H.; To, B.; Berry, J.J.; Gorman, B.; et al. Mechanisms of Electron-Beam-Induced Damage in Perovskite Thin Films Revealed by Cathodoluminescence Spectroscopy. J. Phys. Chem. C 2015, 119, 26904–26911. [Google Scholar] [CrossRef]
- Zhang, Z.; Wu, C.; Wang, D.; Liu, G.; Zhang, Q.; Luo, W.; Qi, X.; Guo, X.; Zhang, Y.; Lao, Y.; et al. Im-provement of Cs2AgBiBr6 double perovskite solar cell by rubidium doping. Org. Electron. 2019, 74, 204. [Google Scholar] [CrossRef]
- Machiba, H.; Oku, T.; Kishimoto, T.; Ueoka, N.; Suzuki, A. Fabrication and evaluation of K-doped MA0.8FA0.1K0.1PbI3(Cl) perovskite solar cells. Chem. Phys. Lett. 2019, 730, 117–123. [Google Scholar] [CrossRef]
- Fan, Y.; Qin, H.; Ye, W.; Liu, M.; Huang, F.; Zhong, D. Improving the stability of methylammonium lead iodide perovskite solar cells by cesium doping. Thin Solid Films 2018, 667, 40–47. [Google Scholar] [CrossRef]
- Yuan, Y.; Xu, R.; Xu, H.T.; Hong, F.; Xu, F.; Wang, L.J. Nature of the band gap of halide perovskites ABX3 (A = CH3NH3, Cs; B = Sn, Pb; X = Cl, Br, I): First-principles calculations. Chin. Phys. B 2015, 24, 116302. [Google Scholar] [CrossRef]
- Yi, C.; Luo, J.; Meloni, S.; Boziki, A.; Ashari-Astani, N.; Graetzel, C.; Zakeeruddin, S.M.; Roethlisberger, U.; Graetzel, M. En-tropic stabilization of mixed A-cation ABX3 metal halide perovskites for high performance perovskite solar cells. Energy Environ. Sci. 2016, 9, 656. [Google Scholar] [CrossRef]
- Lang, L.; Yang, J.-H.; Liu, H.-R.; Xiang, H.; Gong, X. First-principles study on the electronic and optical properties of cubic ABX3 halide perovskites. Phys. Lett. A 2014, 378, 290–293. [Google Scholar] [CrossRef]
- Wang, Z.; Shi, Z.; Li, T.; Chen, Y.; Huang, W. Stability of Perovskite Solar Cells: A Prospective on the Substitution of the A Cation and X Anion. Angew. Chem. Int. Ed. 2017, 56, 1190–1212. [Google Scholar] [CrossRef]
- Bu, T.; Liu, X.; Zhou, Y.; Yi, J.; Huang, X.; Luo, L.; Xiao, J.; Ku, Z.; Peng, Y.; Huang, F.; et al. A novel quad-ruple-cation absorber for universal hysteresis elimination for high efficiency and stable perovskite solar cells. Energy Environ. Sci. 2017, 10, 2509. [Google Scholar] [CrossRef]
- Shai, X.; Zuo, L.; Sun, P.; Liao, P.; Huang, W.; Yao, E.-P.; Li, H.; Liu, S.; Shen, Y.; Yang, Y.; et al. Efficient planar perovskite solar cells using halide Sr-substituted Pb perovskite. Nano Energy 2017, 36, 213–222. [Google Scholar] [CrossRef]
- Zhao, W.G.; Yao, Z.; Yu, F.Y.; Yang, D.; Liu, S.Z. Alkali metal doping for improved CH3NH3PbI3 perovskite solar cells. Adv. Sci. 2018, 5, 1700131. [Google Scholar] [CrossRef]
- Nam, J.K.; Chai, S.U.; Cha, W.; Choi, Y.J.; Kim, W.; Jung, M.S.; Kwon, J.; Kim, D.; Park, J.H. Potassium Incorporation for Enhanced Performance and Stability of Fully Inorganic Cesium Lead Halide Perovskite Solar Cells. Nano Lett. 2017, 17, 2028–2033. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.Y.; Lin, H.Y.; Chiang, K.M.; Tsai, W.L.; Huang, Y.C.; Tsao, C.S.; Lin, H.W. All-Vacuum-Deposited Stoichiometrically Balanced Inorganic Cesium Lead Halide Perovskite Solar Cells with Stabilized Efficiency Exceeding 11%. Adv. Mater. 2017, 29, 1605290. [Google Scholar] [CrossRef] [PubMed]
- Lau, C.F.J.; Deng, X.; Ma, Q.; Zheng, J.; Yun, J.S.; Green, M.A.; Huang, S.; Ho-Baillie, A.W.Y. CsPbIBr2 Perovskite Solar Cell by Spray Assisted Deposition. ACS. Energy Lett. 2016, 1, 573. [Google Scholar] [CrossRef]
- Ma, Q.; Huang, S.; Wen, X.; Green, M.A.; Ho-Baillie, A.W.Y. Hole Transport Layer Free Inorganic CsPbIBr2 Perovskite Solar Cell by Dual Source Thermal Evaporation. Adv. Energy Mater. 2016, 6, 1502202. [Google Scholar] [CrossRef]
- Kulbak, M.; Gupta, S.; Kedem, N.; Levine, I.; Bendikov, T.; Hodes, G.; Cahen, D. Cesium Enhances Long-Term Stability of Lead Bromide Perovskite-Based Solar Cells. J. Phys. Chem. Lett. 2016, 7, 167–172. [Google Scholar] [CrossRef] [PubMed]
- Wu, B.; Zhou, Y.; Xing, G.; Xu, Q.; Garces, H.F.; Solanki, A.; Goh, T.W.; Padture, N.P.; Sum, T.C. Long Minority-Carrier Dif-fusion Length and Low Surface-Recombination Velocity in Inorganic Lead-Free CsSnI3 Perovskite Crystal for Solar Cells. Adv. Funct. Mater. 2017, 27, 1604818. [Google Scholar] [CrossRef]
- Frolova, L.A.; Anokhin, D.V.; Piryazev, A.A.; Luchkin, S.Y.; Dremova, N.N.; Stevenson, K.J.; Troshin, P.A. Highly Efficient All-Inorganic Planar Heterojunction Perovskite Solar Cells Produced by Thermal Coevaporation of CsI and PbI2. J. Phys. Chem. Lett. 2017, 8, 67–72. [Google Scholar] [CrossRef]
- Kim, Y.G.; Kim, T.-Y.; Oh, J.H.; Choi, K.S.; Kim, Y.-J.; Kim, S.Y. Cesium lead iodide solar cells controlled by annealing temperature. Phys. Chem. Chem. Phys. 2017, 19, 6257–6263. [Google Scholar] [CrossRef]
- Trots, D.M.; Myagkota, S.V. High-temperature structural evolution of cesium and rubidium triiodoplumbates. J. Phys. Chem. Solids 2008, 69, 2520–2526. [Google Scholar] [CrossRef]
- Zhao, Z.; Xu, W.; Pan, G.; Liu, Y.; Yang, M.; Hua, S.; Chen, X.; Peng, H.; Song, H. Enhancing the exciton emission of CsPbCl3 perovskite quantum dots by incorporation of Rb+ ions. Mater. Res. Bull. 2019, 112, 142–146. [Google Scholar] [CrossRef]
- Sebastian, M.; Peters, J.A.; Stoumpos, C.C.; Im, J.; Kostina, S.S.; Liu, Z.; Kanatzidis, M.G.; Freeman, A.J.; Wessels, B.W. Excitonic emissions and above-band-gap luminescence in the single-crystal perovskite semiconductors CsPbBr3 and CsPbCl3. Phys. Rev. B 2015, 92, 235210. [Google Scholar] [CrossRef]
- Park, J.P.; Lee, T.K.; Kwak, S.K.; Kim, S.-W. Formation of bright-green-color-emitting perovskite CsPbBr3 in a bulk state using a simple recrystallization process. Dye. Pigment. 2017, 144, 151–157. [Google Scholar] [CrossRef]
- He, H.; Tang, B.; Ma, Y. Controlled synthesis of quantum confined CsPbBr3 perovskite nanocrystals under ambient conditions. Nanotechnology 2018, 29, 055601. [Google Scholar] [CrossRef] [PubMed]
- Du, X.; Wu, G.; Cheng, J.; Dang, H.; Ma, K.; Zhang, Y.-W.; Tan, P.-F.; Chen, S. High-quality CsPbBr3 perovskite nanocrystals for quantum dot light-emitting diodes. RSC Adv. 2017, 7, 10391–10396. [Google Scholar] [CrossRef]
- Stoumpos, C.C.; Malliakas, C.D.; Peters, J.A.; Liu, Z.; Sebastian, M.; Im, J.; Chasapis, T.C.; Wibowo, A.C.; Chung, D.Y.; Freeman, A.J.; et al. Crystal Growth of the Perovskite Semiconductor CsPbBr3: A New Material for High-Energy Radiation Detection. Cryst. Growth Des. 2013, 13, 2722–2727. [Google Scholar] [CrossRef]
- Murtaza, G.; Ahmad, I. First principle study of the structural and optoelectronic properties of cubic perovskites CsPbM3 (M = Cl, Br, I). Phys. B 2011, 406, 3222. [Google Scholar] [CrossRef]
- Duong, T.; Wu, Y.; Shen, H.; Peng, J.; Fu, X.; Jacobs, D.; Wang, E.C.; Kho, T.C.; Fong, K.C.; Stocks, M.; et al. Rubidium Multication Perovskite with Op-timized Bandgap for Perovskite-Silicon Tandem with over 26% Efficiency. Adv. Energy Mater. 2017, 7, 1700228. [Google Scholar] [CrossRef]
- Mahmood, Q.; Hassan, M.; Rashid, M.; Haq, B.U.; Laref, A. The systematic study of mechanical, thermoelectric and optical properties of lead based halides by first principle approach. Phys. B Condens. Matter 2019, 571, 87–92. [Google Scholar] [CrossRef]
- Zhang, X.; Ma, Q.; Li, R.; Lin, C.; Huang, D.; Cheng, Y. The mechanism of alkali doping in CsPbBr3: A first-principles per-spective. J. Appl. Phys. 2021, 129, 165110. [Google Scholar] [CrossRef]
- Zheng, F.; Takenaka, H.; Wang, F.; Koocher, N.Z.; Rappe, A.M. First-principles calculation of the bulk photovoltaic effect in CH3NH3PbI3 and CH3NH3PbI3-xClx. J. Phys. Chem. Lett. 2015, 6, 31. [Google Scholar] [CrossRef]
- Castelli, I.E.; García-Lastra, J.M.; Thygesen, K.S.; Jacobsen, K.W. Bandgap calculations and trends of organometal halide perovskites. APL Mater. 2014, 2, 081514. [Google Scholar] [CrossRef]
- Myagkota, S.; Gloskovskii, A.; Gladyshevskii, R.; Voloshynovskii, A. Luminescent kinetic characteristics of CsPbCl3 aggregates dispersed in Rb1-xCsxCl matrices. Condens. Matter Phys. 2003, 6, 325. [Google Scholar] [CrossRef]
- Moreira, R.L.; Dias, A. Comment on “Prediction of lattice constant in cubic perovskites”. J. Phys. Chem. Solids 2007, 68, 1617. [Google Scholar] [CrossRef]
- Nikl, M.; Nitsch, K.; Chval, J.; Somma, F.; Phani, A.R.; Santucci, S. Optical and structural properties of ternary nanoaggregates in CsI-PbI2 co-evaporated thin films. J. Phys. Condens. Matter. 2000, 12, 1939. [Google Scholar] [CrossRef]
- Qian, J.; Xu, B.; Tian, W. A comprehensive theoretical study of halide perovskites ABX3. Org. Electron. 2016, 37, 61–73. [Google Scholar] [CrossRef]
- Kulbak, M.; Cahen, D.; Hodes, G. How Important Is the Organic Part of Lead Halide Perovskite Photovoltaic Cells? Efficient CsPbBr3 Cells. J. Phys. Chem. Lett. 2015, 6, 2452–2456. [Google Scholar] [CrossRef]
- Beal, R.E.; Slotcavage, D.J.; Leijtens, T.; Bowring, A.R.; Belisle, R.A.; Nguyen, W.H.; Burkhard, G.F.; Hoke, E.T.; McGehee, M.D. Cesium Lead Halide Perovskites with Improved Stability for Tandem Solar Cells. J. Phys. Chem. Lett. 2016, 7, 746. [Google Scholar] [CrossRef]
- Zhang, H.; Yuan, R.; Jin, M.; Zhang, Z.; Yu, Y.; Xiang, W.; Liang, X. Rb+-doped CsPbBr3 quantum dots with multi-color stabilized in borosilicate glass via crystallization. J. Eur. Ceram. Soc. 2020, 40, 94–102. [Google Scholar] [CrossRef]
- Shi, Y.; Xi, J.; Lei, T.; Yuan, F.; Dai, J.; Ran, C.; Dong, H.; Jiao, B.; Hou, X.; Wu, Z. Rubidium Doping for Enhanced Perfor-mance of Highly Efficient Formamidinium-Based Perovskite Light-Emitting Diodes. ACS Appl. Mater. Interfaces 2018, 10, 9849–9857. [Google Scholar] [CrossRef]
- Jiang, L.; Guo, J.; Liu, H.; Zhu, M.; Zhou, X.; Wu, P.; Li, C. Prediction of lattice constant in cubic perovskites. J. Phys. Chem. Solids 2006, 67, 1531–1536. [Google Scholar] [CrossRef]
- Pitriana, P.; Wungu, T.D.K.; Herman; Hidayat, R. The characteristics of band structures and crystal binding in all-inorganic perovskite APbBr3 studied by the first principle calculations using the Density Functional Theory (DFT) method. Results Phys. 2019, 15, 102592. [Google Scholar] [CrossRef]
- Kim, S.H.; Park, K.-D.; Lee, H.S. Growth Kinetics and Optical Properties of CsPbBr3 Perovskite Nanocrystals. Energies 2021, 14, 275. [Google Scholar] [CrossRef]
- Yan, W.; Mao, L.; Zhao, P.; Mertens, A.; Dottermusch, S.; Hu, H.; Jin, Z.; Richards, B.S. Determination of complex optical constants and photovoltaic device design of all-inorganic CsPbBr3 perovskite thin films. Opt. Express 2020, 28, 15706–15717. [Google Scholar] [CrossRef]
- Zhao, M.; Shi, Y.; Dai, J.; Lian, J. Ellipsometric study of the complex optical constants of a CsPbBr3 perovskite thin film. J. Mater. Chem. C 2018, 6, 10450–10455. [Google Scholar] [CrossRef]
- Yang, D.; Cheng, X.; Liu, Y.; Shen, C.; Xu, Z.; Zheng, X.; Jiang, T. Dielectric properties of a CsPbBr3 quantum dot solution in the terahertz region. Appl. Opt. 2017, 56, 2878–2885. [Google Scholar] [CrossRef] [PubMed]
- Scott, J.F.; Bohn, H.G.; Schenk, W. Ionic Wiedemann–Franz law. Appl. Phys. Lett. 2000, 77, 2599. [Google Scholar] [CrossRef]
- Li, Z.; Xiao, C.; Fan, S.; Deng, Y.; Zhang, W.; Ye, B.; Xie, Y. Dual vacancies: An effective strategy realizing synergistic optimi-zation of thermoelectric property in BiCuSeO. J. Am. Chem. Soc. 2015, 137, 6587. [Google Scholar] [CrossRef]
- Pei, Y.; May, A.F.; Snyder, G.J. Self-tuning the carrier concentration of PbTe/Ag2Te composites with excess Ag for high ther-moelectric performance. Adv. Energy Mater. 2011, 1, 291. [Google Scholar] [CrossRef]
- Fu, J.; Su, X.; Xie, H.; Yan, Y.; Liu, W.; You, Y.; Cheng, X.; Uher, C.; Tang, X. Understanding the combustion process for the synthesis of mechanically robust SnSe thermoelectrics. Nano Energy 2018, 44, 53–62. [Google Scholar] [CrossRef]
- Yu, H.S.; Li, S.L.; Truhlar, D.G. Perspective: Kohn-Sham density functional theory descending a stair-case. J. Chem. Phys. 2016, 145, 130901. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, B864–B871. [Google Scholar] [CrossRef]
- Kohn, W.; Becke, A.D.; Parr, R.G. Density functional theory of electronic structure. J. Phys. Chem. 1996, 100, 12974. [Google Scholar] [CrossRef]
- Burke, K. Perspective on density functional theory. J. Chem. Phys. 2012, 136, 150901. [Google Scholar] [CrossRef]
- Gonze, X.; Amadond, B.; Anglade, P.M.; Beuken, J.M.; Bottin, F.; Boulangera, P.; Bruneval, F.; Caliste, D.; Caracas, R.; Côté, M.; et al. ABINIT: First-principles approach to material and nanosystem properties. Comput. Phys. Commun. 2009, 180, 2582. [Google Scholar] [CrossRef]
- Gonze, X.; Beuken, J.-M.; Caracas, R.; Detraux, F.; Fuchs, M.; Rignanese, G.-M.; Sindic, L.; Verstraete, M.; Zerah, G.; Jollet, F.; et al. First-principles computation of material properties: The ABINIT software project. Comput. Mater. Sci. 2002, 25, 478–492. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple John. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef] [PubMed]
- Kohn, W.; Sham, L.J. Self-consistent equations including exchange and correlation effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188. [Google Scholar] [CrossRef]
- Sakata, M.; Nishiwaki, T.; Harada, J. Neutron Diffraction Study of the Structure of Cubic CsPbBr3. J. Phys. Soc. Jpn. 1979, 47, 232–233. [Google Scholar] [CrossRef]
- Madsen, G.K.; Singh, D.J. BoltzTraP. A code for calculating band-structure dependent quantities. Comput. Phys. Commun. 2006, 175, 67–71. [Google Scholar] [CrossRef]


| Present Work a (Å) | Other Works a (Å) | Experimental a (Å) |
|---|---|---|
| 5.93 | 5.74 [15] 5.84 [29] 5.94 [60] 5.99 [58,60] | 5.87 [60] 5.90 [57] |
| Elastic Constants (GPa) | Theoretical Eg (eV) | Experimental Eg (eV) |
|---|---|---|
| B11 = 163.30 B12 = 84.51 B44 = 10.93 | 1.70 (x = 0), 3.76 (x = 0.75) and 1.71 (x = 1): our work 1.769, 1.756: GGA-PBE [61] 1.148, 1.197: LDA-PZ [61] | 2.383 [62] 2.25 [45], 2.36 [56], and 1.90 [57] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ouaaka, E.; Aazza, M.; Bouymajane, A.; Cacciola, F. Electronic, Optical, Thermoelectric and Elastic Properties of RbxCs1−xPbBr3 Perovskite. Molecules 2023, 28, 2880. https://doi.org/10.3390/molecules28072880
Ouaaka E, Aazza M, Bouymajane A, Cacciola F. Electronic, Optical, Thermoelectric and Elastic Properties of RbxCs1−xPbBr3 Perovskite. Molecules. 2023; 28(7):2880. https://doi.org/10.3390/molecules28072880
Chicago/Turabian StyleOuaaka, Elmustafa, Mustapha Aazza, Aziz Bouymajane, and Francesco Cacciola. 2023. "Electronic, Optical, Thermoelectric and Elastic Properties of RbxCs1−xPbBr3 Perovskite" Molecules 28, no. 7: 2880. https://doi.org/10.3390/molecules28072880
APA StyleOuaaka, E., Aazza, M., Bouymajane, A., & Cacciola, F. (2023). Electronic, Optical, Thermoelectric and Elastic Properties of RbxCs1−xPbBr3 Perovskite. Molecules, 28(7), 2880. https://doi.org/10.3390/molecules28072880








