Influencing Factors and Evaluation of the Self-Healing Behavior of Asphalt Binder Using Molecular Dynamics Simulation Method
Abstract
1. Introduction
2. Molecular Model Construction and Evaluation Method of Self-Healing Behavior
2.1. Construction of Asphalt Molecular Model
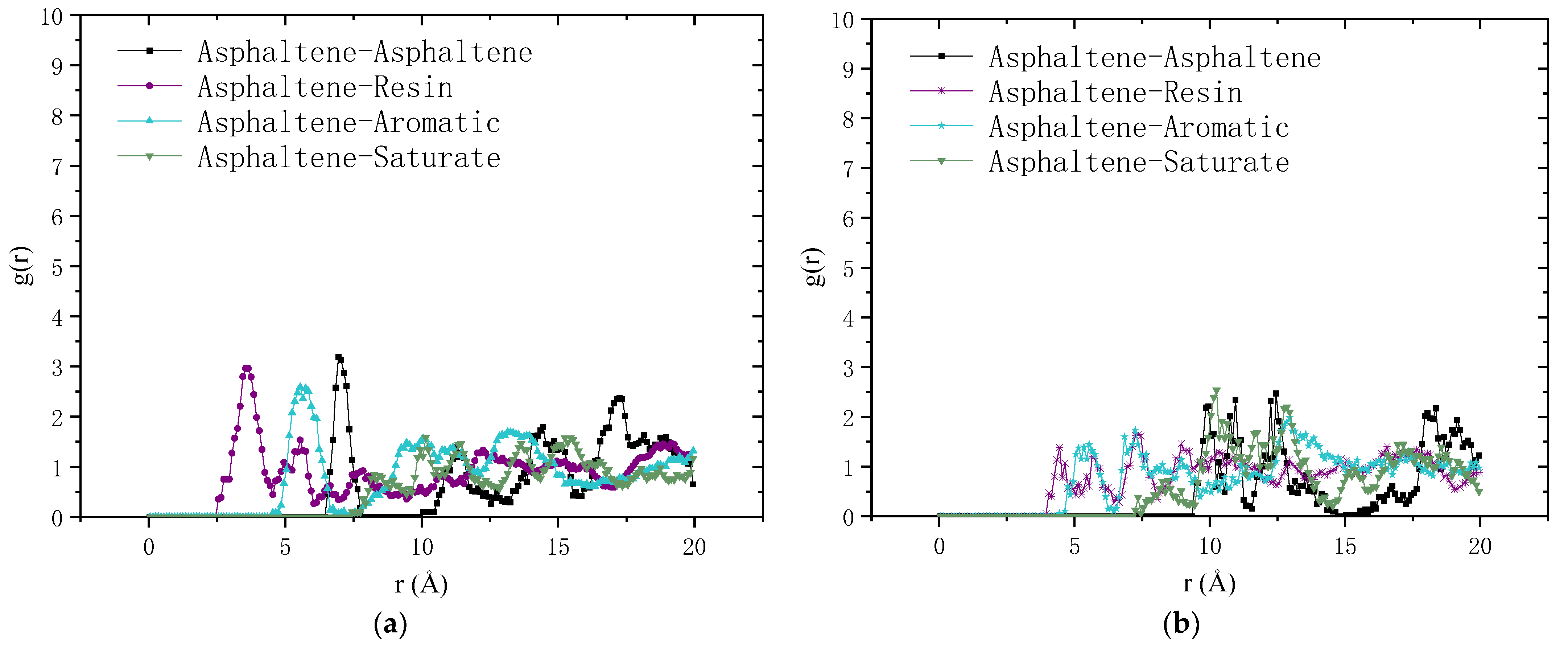
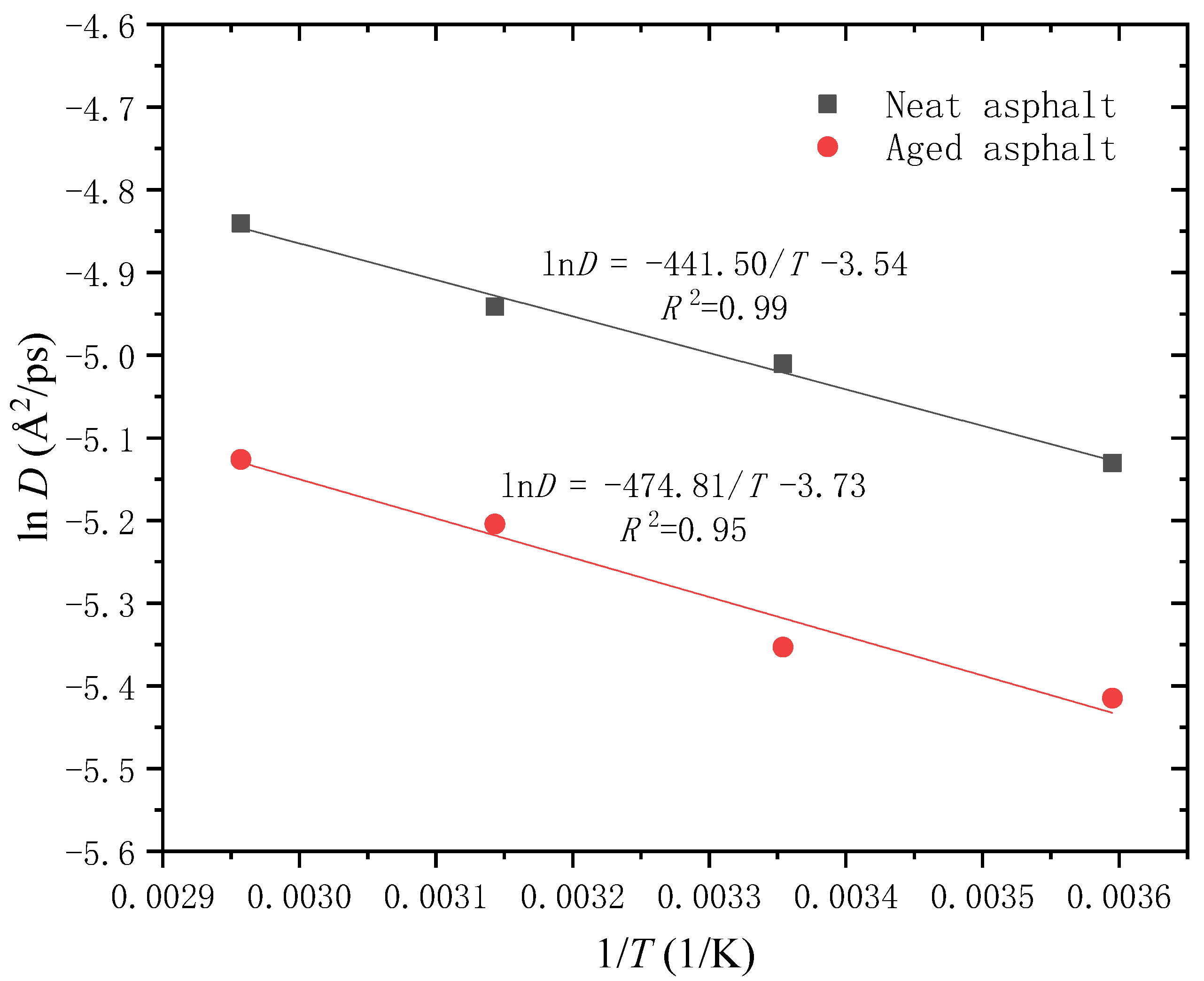
2.2. Verification of Asphalt Molecular Model
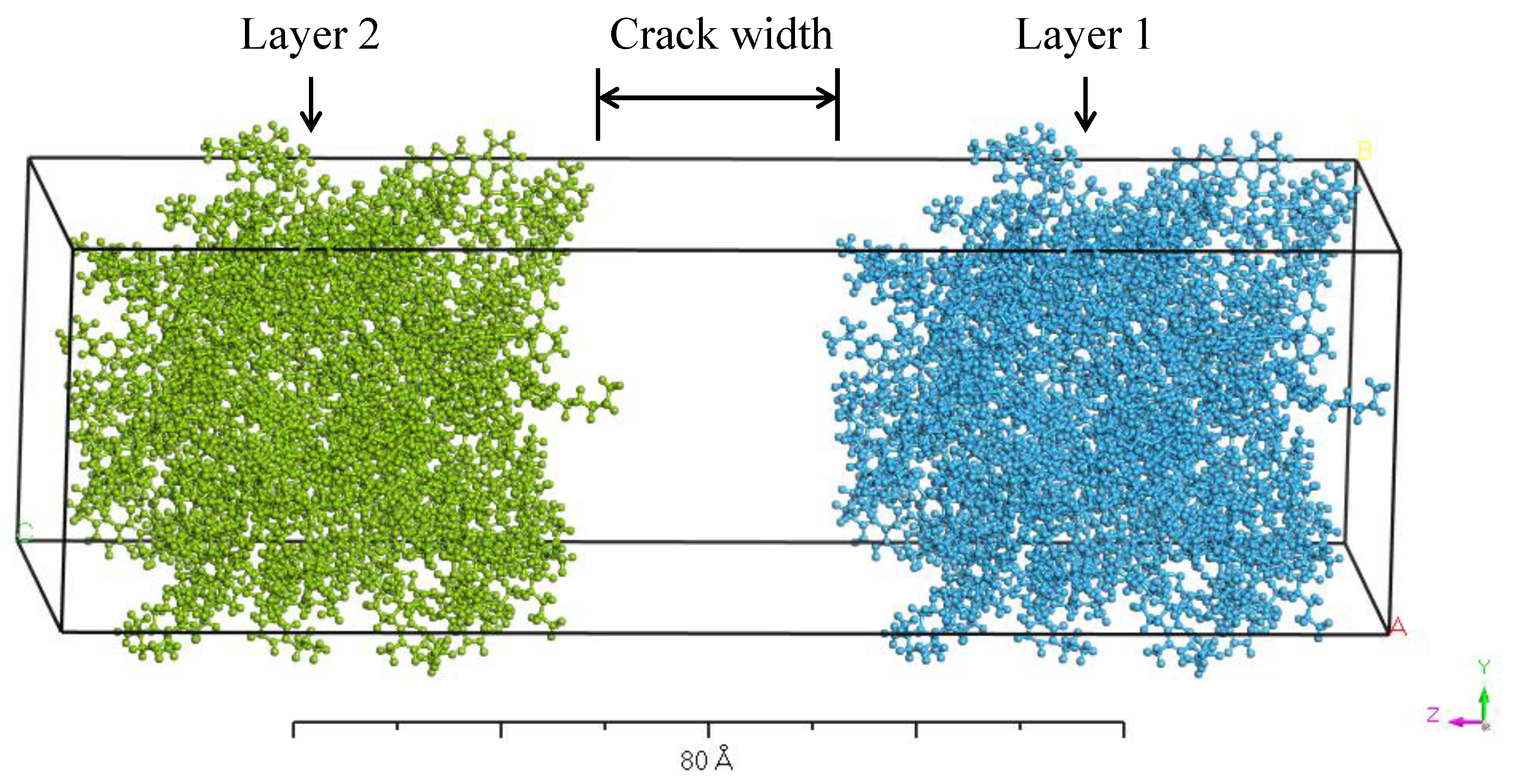
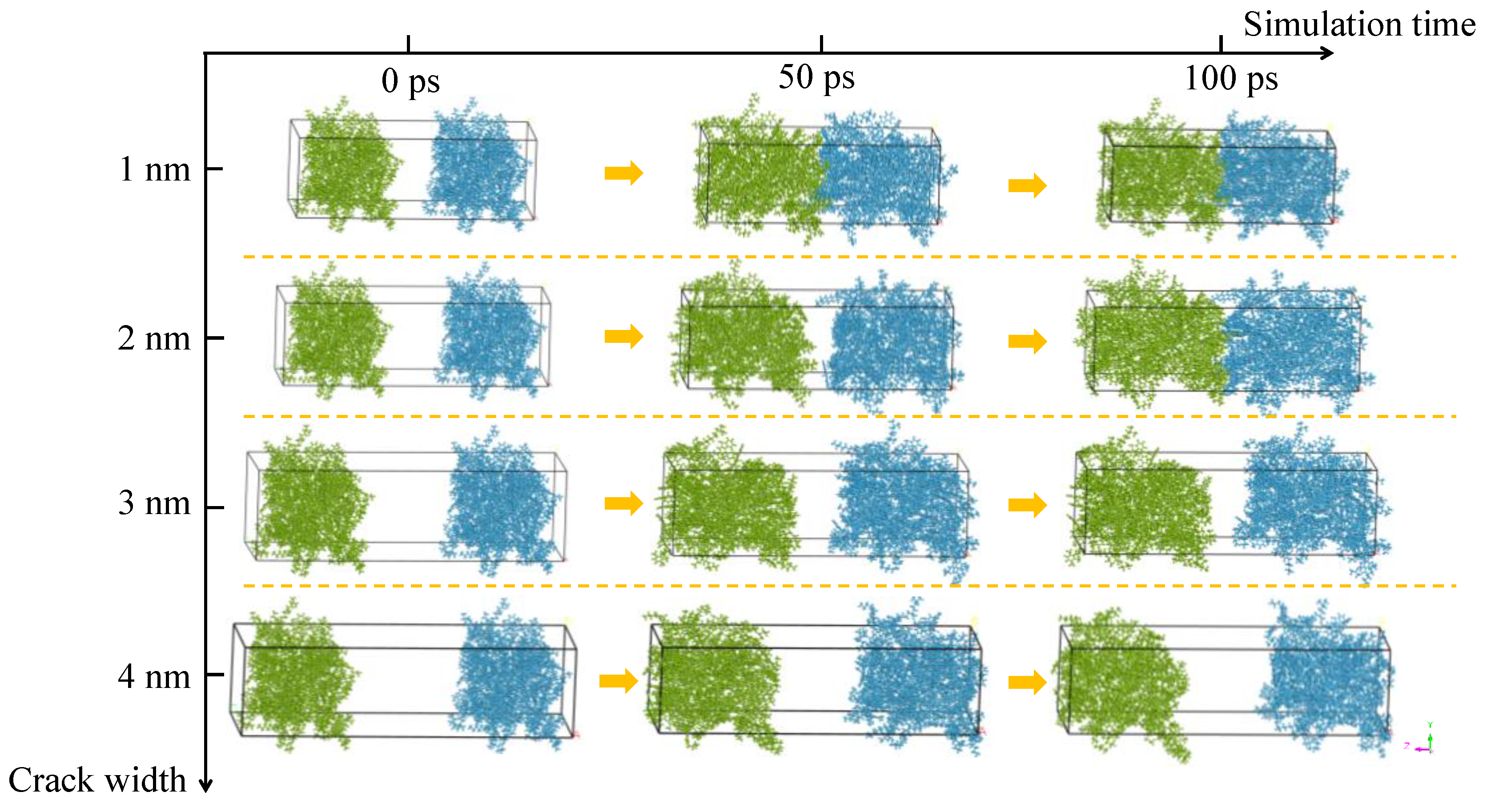
2.3. Development of Asphalt Self-Healing Model
2.4. Evaluation Method of Self-Healing Behavior
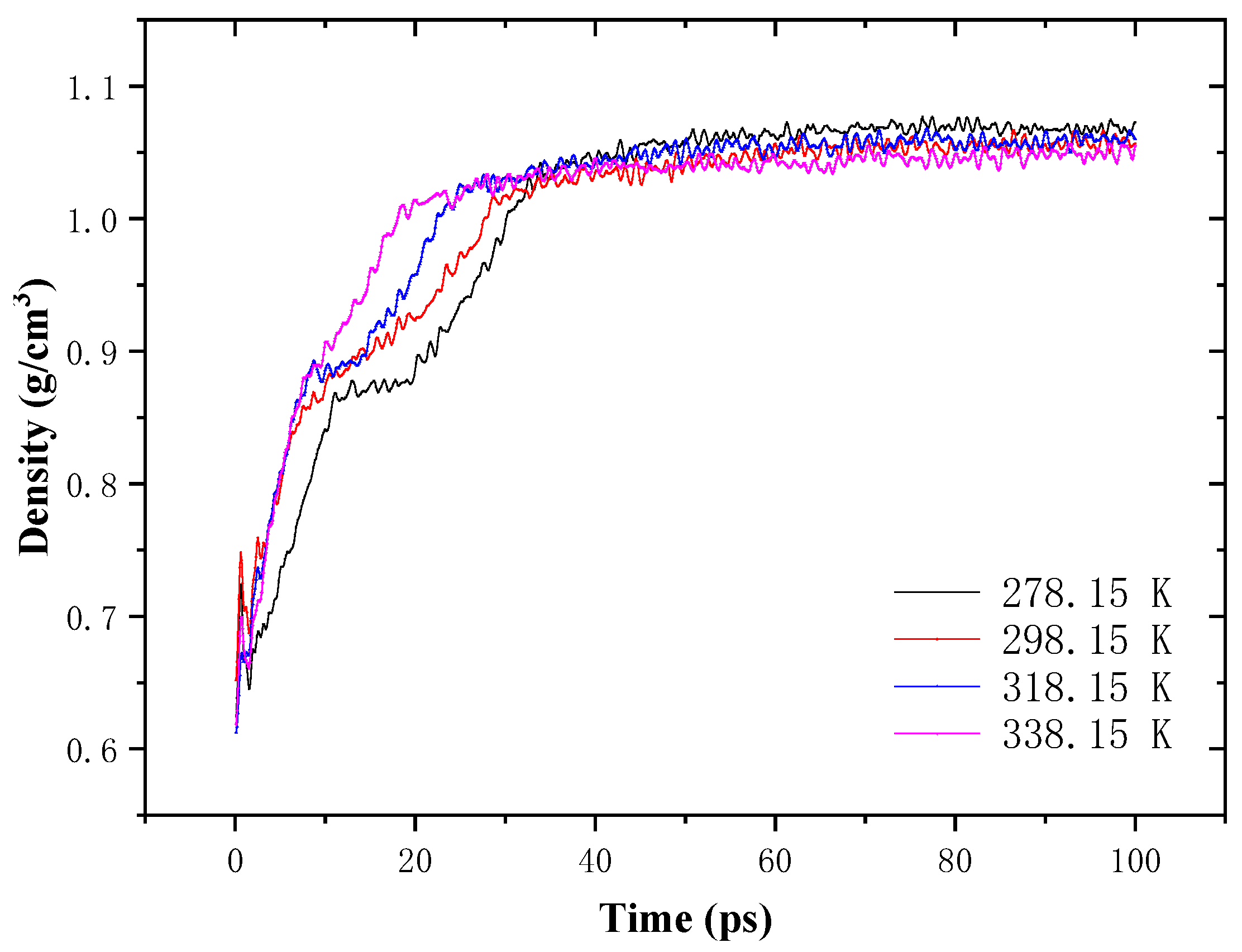
2.4.1. Crack Closing Stage
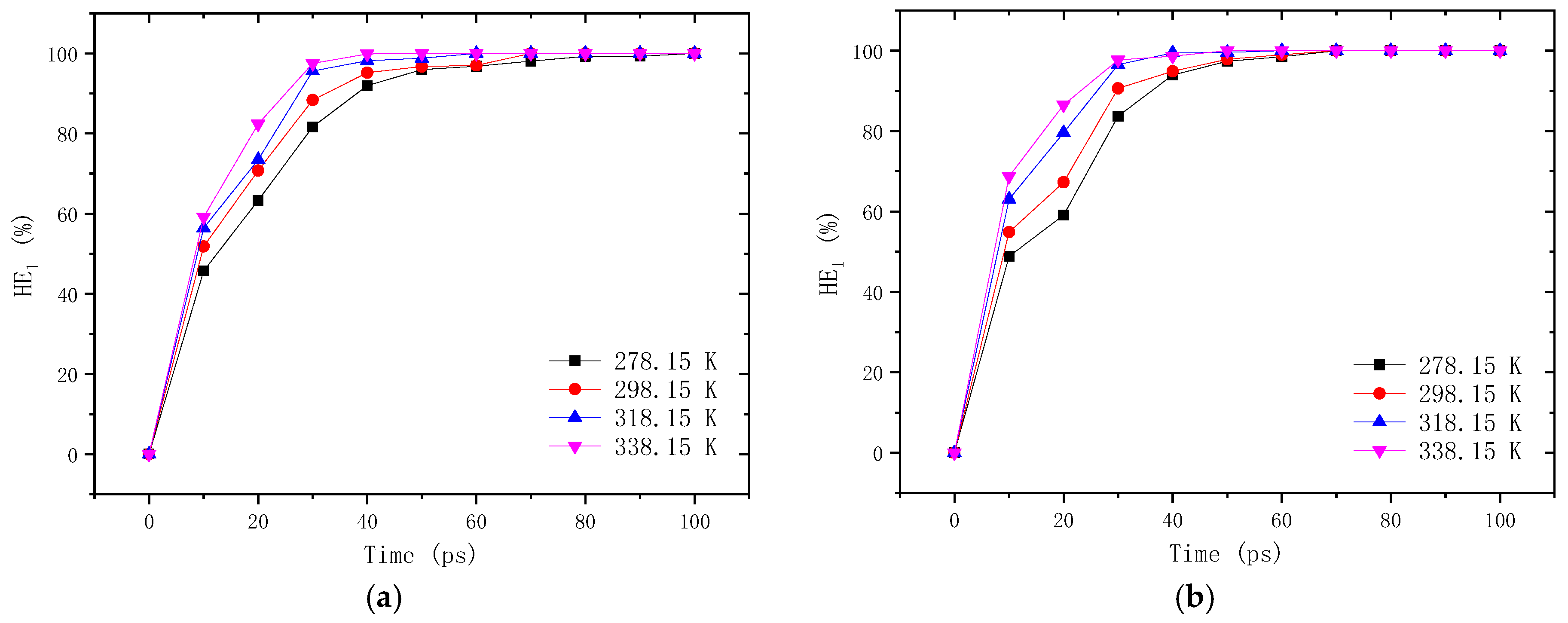
2.4.2. Intrinsic Healing Stage
3. Results and Discussion
3.1. Influencing Factors of Self-Healing Behavior
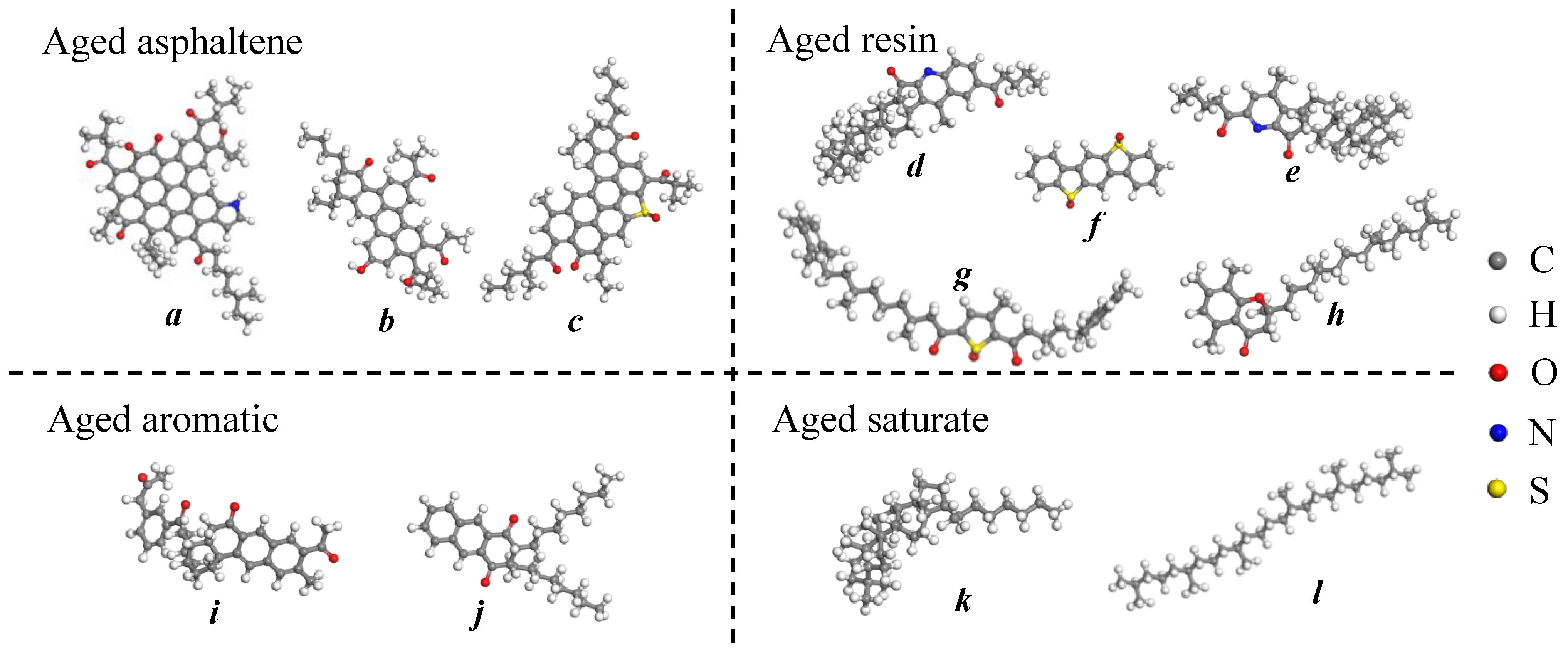
3.1.1. Oxidative Aging
- (1)
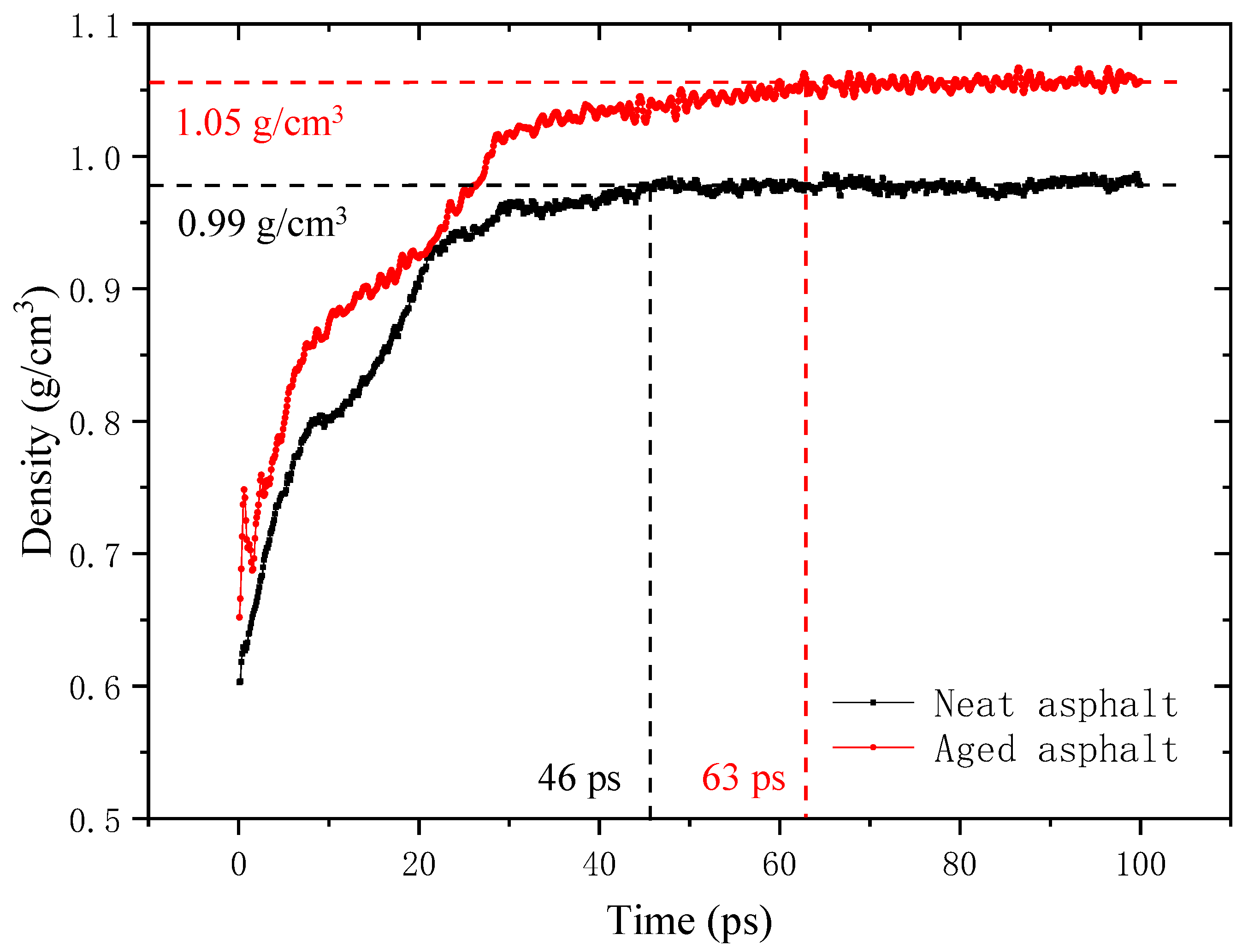
- At the crack closing stage, the density increases sharply with time until approaching the real density of undamaged asphalt binder, indicating that the crack is gradually closed. The oxidative aging reduces the growth ratio of the density and prolongs the simulation time required for density recovery;
- (2)
- At the intrinsic healing stage, the asphalt molecules further diffuse across the closed crack interfaces to reach a greater equilibrium and randomization situation, contributing a lot to the strength recovery of the model. The density of the asphalt self-healing model stays at a stable value, and the aged asphalt binder has a higher density caused by the introduced oxygen atoms.
3.1.2. Damage Degree
3.1.3. Healing Temperature
3.2. Evaluation of Self-Healing Efficiency
3.2.1. Crack Closing Stage
3.2.2. Intrinsic Healing Stage
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, D.Q.; Sun, G.Q.; Zhu, X.Y.; Ye, F.Y.; Xu, J.Y. Intrinsic Temperature Sensitive Self-Healing Character of Asphalt Binders Based on Molecular Dynamics Simulations. Fuel 2018, 211, 609–620. [Google Scholar] [CrossRef]
- Bhasin, A.; Motamed, A. Analytical Models to Characterise Crack Growth in Asphaltic Materials and Healing in Asphalt Binders. Int. J. Pavement Eng. 2011, 12, 371–383. [Google Scholar] [CrossRef]
- Qiu, J. Self-Healing of Asphalt Mixtures; Delft University of Technology: Delft, Netherlands, 2012. [Google Scholar]
- Li, Y.; Hao, P.W.; Zhang, M.Y. Fabrication, Characterization and Assessment of the Capsules Containing Rejuvenator for Improving the Self-Healing Performance of Asphalt Materials: A Review. J. Clean. Prod. 2021, 287, 125079. [Google Scholar] [CrossRef]
- Xu, G.J.; Wang, H. Molecular Dynamics Study of Oxidative Aging Effect on Asphalt Binder Properties. Fuel 2017, 188, 1–10. [Google Scholar] [CrossRef]
- Wang, Y.H.; Zhao, K.C.; Glover, C.; Chen, L.; Wen, Y.; Chong, D.; Hu, C.C. Effects of Aging on the Properties of Asphalt at the Nanoscale. Constr. Build. Mater. 2015, 80, 244–254. [Google Scholar] [CrossRef]
- Lv, Q.; Huang, W.D.; Zhu, X.Y.; Xiao, F.P. On the Investigation of Self-Healing Behavior of Bitumen and Its Influencing Factors. Mater. Des. 2017, 117, 7–17. [Google Scholar] [CrossRef]
- Bhasin, A.; Bommavaram, R.; Greenfield, M.L.; Little, D.N. Use of Molecular Dynamics to Investigate Self-Healing Mechanisms in Asphalt Binders. J. Mater. Civ. Eng. 2011, 23, 485–492. [Google Scholar] [CrossRef]
- Qiu, J.; van de Ven, M.; Wu, S.; Yu, J.; Molenaar, A. Evaluating Self Healing Capability of Bituminous Mastics. Exp. Mech. 2012, 52, 1163–1171. [Google Scholar] [CrossRef]
- García, Á. Self-Healing of Open Cracks in Asphalt Mastic. Fuel 2012, 93, 264–272. [Google Scholar] [CrossRef]
- Hung, A.M.; Mousavi, M.; Fini, E.H. Implication of Wax on Hindering Self-Healing Processes in Bitumen. Appl. Surf. Sci. 2020, 523, 146449. [Google Scholar] [CrossRef]
- Wang, P. Interfacial Enhancement Mechanism and Rheological Properties of Composited Polymer Modified Asphalt with Carbon Nanotubes; Harbin Institute of Technology: Harbin, China, 2017. [Google Scholar]
- Guo, F.C.; Zhang, J.P.; Pei, J.Z.; Zhou, B.C.; Hu, Z. Study on the Mechanical Properties of Rubber Asphalt by Molecular Dynamics Simulation. J. Mol. Model. 2019, 25, 365. [Google Scholar] [CrossRef]
- Xu, G.; Wang, H. Diffusion and Interaction Mechanism of Rejuvenating Agent with Virgin and Recycled Asphalt Binder: A Molecular Dynamics Study. Mol. Simul. 2018, 44, 1433–1443. [Google Scholar] [CrossRef]
- Xu, G.; Wang, H. Molecular Dynamics Study of Interfacial Mechanical Behavior between Asphalt Binder and Mineral Aggregate. Constr. Build. Mater. 2016, 121, 246–254. [Google Scholar] [CrossRef]
- Yu, T.J.; Zhang, H.T.; Wang, Y. Multi-Gradient Analysis of Temperature Self-Healing of Asphalt Nano-Cracks Based on Molecular Simulation. Constr. Build. Mater. 2020, 250, 118859. [Google Scholar] [CrossRef]
- Sun, D.Q.; Lin, T.B.; Zhu, X.Y.; Tian, Y.; Liu, F.L. Indices for Self-Healing Performance Assessments Based on Molecular Dynamics Simulation of Asphalt Binders. Comput. Mater. Sci. 2016, 114, 86–93. [Google Scholar] [CrossRef]
- Sun, D.Q.; Yu, F.; Li, L.H.; Lin, T.B.; Zhu, X.Y. Effect of Chemical Composition and Structure of Asphalt Binders on Self-Healing. Constr. Build. Mater. 2017, 133, 495–501. [Google Scholar] [CrossRef]
- Gao, X.W.; Liu, Z.H. Self-Healing Mechanism of Bio-Oil Recycled Asphalt. China J. Highw. Transp. 2019, 32, 235–242. [Google Scholar]
- He, L.; Li, G.N.; Lv, S.T.; Gao, J.; Kowalski, K.J.; Valentin, J.; Alexiadis, A. Self-Healing Behavior of Asphalt System Based on Molecular Dynamics Simulation. Constr. Build. Mater. 2020, 254, 119225. [Google Scholar] [CrossRef]
- Bommavaram, R.R. Evaluation of Healing in Asphalt Binders Using Dynamic Shear Rheometer and Molecular Modeling Techniques; Texas A&M University: College Station, TX, USA, 2008. [Google Scholar]
- Lesueur, D. The Colloidal Structure of Bitumen: Consequences on the Rheology and on the Mechanisms of Bitumen Modification. Adv. Colloid Interface Sci. 2009, 145, 42–82. [Google Scholar] [CrossRef]
- Li, D.D.; Greenfield, M.L. Chemical Compositions of Improved Model Asphalt Systems for Molecular Simulations. Fuel 2014, 115, 347–356. [Google Scholar] [CrossRef]
- Pan, J.; Tarefder, R.A. Investigation of Asphalt Aging Behaviour due to Oxidation Using Molecular Dynamics Simulation. Mol. Simul. 2016, 42, 667–678. [Google Scholar] [CrossRef]
- Qiu, J.; van de Ven, M.; Molenaar, A. Crack-Healing Investigation in Bituminous Materials. J. Mater. Civ. Eng. 2013, 25, 864–870. [Google Scholar] [CrossRef]
- Bhasin, A.; Little, D.N.; Bommavaram, R.; Vasconcelos, K.; Little, D.N.; Bommavaram, R.; Vasconcelos, K. A Framework to Quantify the Effect of Healing in Bituminous Materials Using Material Properties. Road Mater. Pavement Des. 2008, 9, 219–242. [Google Scholar] [CrossRef]
- Andrade, C. Calculation of Chloride Diffusion Coefficients in Concrete from Ionic Migration Measurements. Cem. Concr. Res. 1993, 23, 724–742. [Google Scholar] [CrossRef]
- Sun, D.Q.; Lin, T.B.; Zhu, X.Y.; Cao, L.H. Calculation and Evaluation of Activation Energy as a Self-Healing Indication of Asphalt Mastic. Constr. Build. Mater. 2015, 95, 431–436. [Google Scholar] [CrossRef]
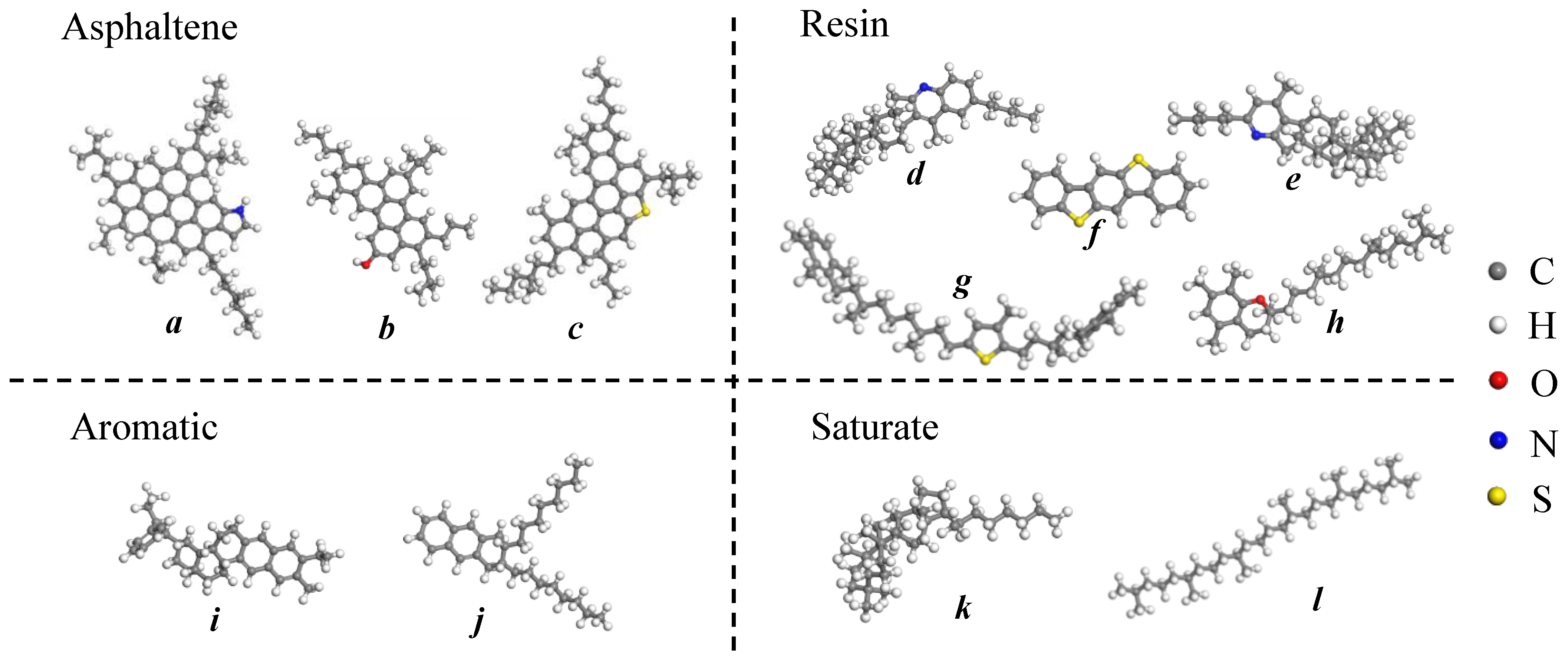






| Chemical Component | Code | Chemical Formula | Number |
|---|---|---|---|
| Asphaltene | a | C66H81N | 2 |
| b | C42H54O | 3 | |
| c | C51H62S | 3 | |
| Resin | d | C40H59N | 4 |
| e | C36H57N | 4 | |
| f | C18H10S2 | 15 | |
| g | C40H60S | 4 | |
| h | C29H50O | 5 | |
| Aromatic | i | C35H44 | 11 |
| j | C30H46 | 13 | |
| Saturate | k | C35H62 | 4 |
| l | C30H62 | 4 |
| Parameter | Aging Condition | 278.15 K | 298.15 K | 318.15 K | 338.15 K | Measured Value |
|---|---|---|---|---|---|---|
| Density (g/cm3) | Unaged | 1.005 | 0.992 | 0.989 | 0.965 | 0.96–1.04 [16] |
| Aged | 1.060 | 1.051 | 1.046 | 1.037 | ||
| Solubility parameter (J/cm3)0.5 | Unaged | 18.11 | 17.84 | 17.78 | 17.19 | 15.3–23.0 [16] |
| Aged | 19.37 | 19.13 | 18.85 | 18.70 |
| Temperature | Neat Asphalt Binder | Aged Asphalt Binder | ||||
|---|---|---|---|---|---|---|
| Fitting Formula | R2 | D (Å2/ps) | Fitting Formula | R2 | D (Å2/ps) | |
| 278.15 K | y = 0.0355x + 1.6673 | 0.99 | 0.0059 | y = 0.0267 x + 1.4740 | 0.99 | 0.0045 |
| 298.15 K | y = 0.0400x + 1.7195 | 0.99 | 0.0067 | y = 0.0284x + 1.6704 | 0.99 | 0.0047 |
| 318.15 K | y = 0.0429x + 2.48699 | 0.98 | 0.0072 | y = 0.0330x + 1.7441 | 0.99 | 0.0055 |
| 338.15 K | y = 0.0474x + 2.6928 | 0.97 | 0.0079 | y = 0.0356x + 2.0758 | 0.99 | 0.0059 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Zhang, H.; Wu, Z.; Sun, B. Influencing Factors and Evaluation of the Self-Healing Behavior of Asphalt Binder Using Molecular Dynamics Simulation Method. Molecules 2023, 28, 2860. https://doi.org/10.3390/molecules28062860
Li Y, Zhang H, Wu Z, Sun B. Influencing Factors and Evaluation of the Self-Healing Behavior of Asphalt Binder Using Molecular Dynamics Simulation Method. Molecules. 2023; 28(6):2860. https://doi.org/10.3390/molecules28062860
Chicago/Turabian StyleLi, Yan, Haiwei Zhang, Zirui Wu, and Bowei Sun. 2023. "Influencing Factors and Evaluation of the Self-Healing Behavior of Asphalt Binder Using Molecular Dynamics Simulation Method" Molecules 28, no. 6: 2860. https://doi.org/10.3390/molecules28062860
APA StyleLi, Y., Zhang, H., Wu, Z., & Sun, B. (2023). Influencing Factors and Evaluation of the Self-Healing Behavior of Asphalt Binder Using Molecular Dynamics Simulation Method. Molecules, 28(6), 2860. https://doi.org/10.3390/molecules28062860





