Biomimetic Liquid Metal–Elastomer Composited Foam with Adjustable Thermal Conductivity for Heat Control
Abstract
:1. Introduction
2. Results and Discussion
2.1. Materials
2.2. Fabrication of the B-LM-ECF
2.3. Characterizations and Measurements
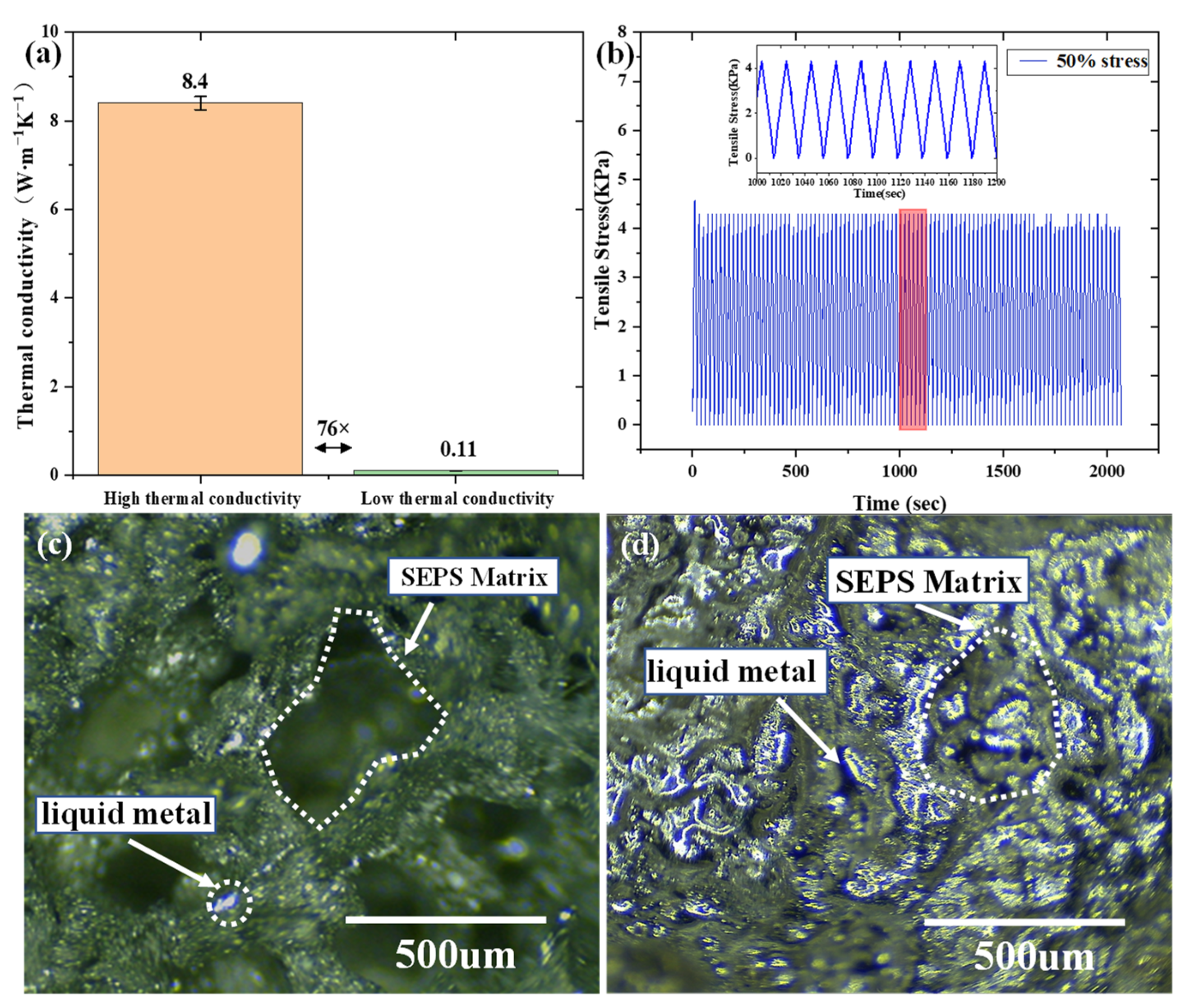
2.4. Mechanical Properties and Micromorphology of B-LM-ECF
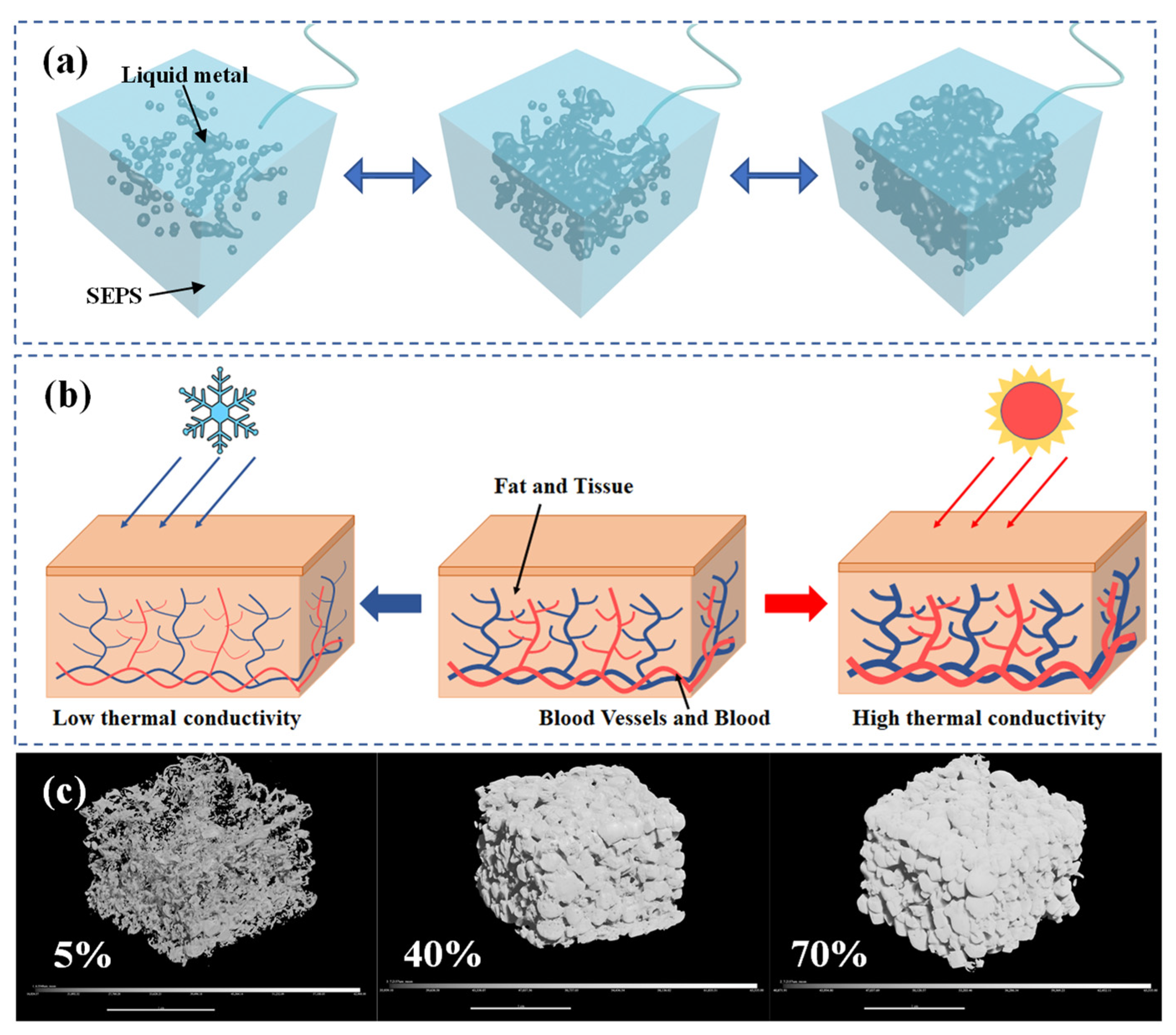
2.5. Mechanism of the Thermal Regulating of B-LM-ECF
2.6. Thermal Properties and Applications of B-LM-ECF
3. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Burger, N.; Laachachi, A.; Ferriol, M.; Lutz, M.; Toniazzo, V.; Ruch, D. Review of thermal conductivity in composites: Mechanisms, parameters and theory. Prog. Polym. Sci. 2016, 61, 1–28. [Google Scholar] [CrossRef]
- Xu, X.; Chen, J.; Zhou, J.; Li, B. Thermal conductivity of polymers and their nanocomposites. Adv. Mater. 2018, 30, 1705544. [Google Scholar] [CrossRef] [PubMed]
- Zhou, W.; Cheng, Y.; Chen, K.; Xie, G.; Wang, T.; Zhang, G. Thermal conductivity of amorphous materials. Adv. Funct. Mater. 2020, 30, 1903829. [Google Scholar] [CrossRef]
- Fraleoni-Morgera, A.; Chhikara, M. Polymer-based nano-composites for thermal insulation. Adv. Eng. Mater. 2019, 21, 1801162. [Google Scholar] [CrossRef]
- Li, B.; Zhao, G.; Wang, G.; Zhang, L.; Gong, J.; Shi, Z. Super High-Expansion Poly (Lactic Acid) Foams with Excellent Oil-Adsorption and Thermal-Insulation Properties Fabricated by Supercritical CO2 Foaming. Adv. Sustain. Syst. 2021, 5, 2000295. [Google Scholar] [CrossRef]
- Ma, Z.; Liu, X.; Xu, X.; Liu, L.; Yu, B.; Maluk, C.; Huang, G.; Wang, H.; Song, P. Bioinspired, highly adhesive, nanostructured polymeric coatings for superhydrophobic fire-extinguishing thermal insulation foam. ACS Nano 2021, 15, 11667–11680. [Google Scholar] [CrossRef]
- Rizvi, A.; Chu, R.; Park, C. Scalable fabrication of thermally insulating mechanically resilient hierarchically porous polymer foams. ACS Appl. Mater. Interfaces 2018, 10, 38410–38417. [Google Scholar] [CrossRef]
- Ruckdeschel, P.; Philipp, A.; Retsch, M. Understanding thermal insulation in porous, particulate materials. Adv. Funct. Mater. 2017, 27, 1702256. [Google Scholar] [CrossRef]
- Cui, Y.; Gong, H.; Wang, Y.; Li, D.; Bai, H. A thermally insulating textile inspired by polar bear hair. Adv. Mater. 2018, 30, 1706807. [Google Scholar] [CrossRef]
- George, K.; Mohanty, S.; Biswal, M.; Nayak, S. Thermal insulation behaviour of Ethylene propylene diene monomer rubber/kevlar fiber based hybrid composites containing Nanosilica for solid rocket motor insulation. J. Appl. Polym. Sci. 2021, 138, 49934. [Google Scholar] [CrossRef]
- Alves, P.; Dias, D.; Pontinha, A. Silica Aerogel-Rubber Composite: A Sustainable Alternative for Buildings’ Thermal Insulation. Molecules 2022, 27, 7127. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Liu, X.; Nie, X.; Yang, W.; Wang, Y.; Yu, R.; Shui, J. Multifunctional organic–inorganic hybrid aerogel for self-cleaning, heat-insulating, and highly efficient microwave absorbing material. Adv. Funct. Mater. 2019, 29, 1807624. [Google Scholar] [CrossRef]
- Yan, M.; Pan, Y.; Cheng, X.; Zhang, Z.; Deng, Y.; Lun, Z.; Gong, L.; Gao, M.; Zhang, H. “Robust–Soft” Anisotropic Nanofibrillated Cellulose Aerogels with Superior Mechanical, Flame-Retardant, and Thermal Insulating Properties. ACS Appl. Mater. Interfaces 2021, 13, 27458–27470. [Google Scholar] [CrossRef]
- Zhang, J.; Cheng, Y.; Xu, C.; Gao, M.; Zhu, M.; Jiang, L. Hierarchical interface engineering for advanced nanocellulosic hybrid aerogels with high compressibility and multifunctionality. Adv. Funct. Mater. 2021, 31, 2009349. [Google Scholar] [CrossRef]
- Zuo, B.; Yuan, B. Flame-retardant cellulose nanofiber aerogel modified with graphene oxide and sodium montmorillonite and its fire-alarm application. Polym. Adv. Technol. 2021, 32, 1877–1887. [Google Scholar] [CrossRef]
- Cho, J.; Park, J.; An, J. Low thermal conductivity of atomic layer deposition yttria-stabilized zirconia (YSZ) thin films for thermal insulation applications. J. Eur. Ceram. Soc. 2017, 37, 3131–3136. [Google Scholar] [CrossRef]
- Dai, Z.; Li, Z.; Li, L.; Xu, G. Synthesis and thermal properties of antimony doped tin oxide/waterborne polyurethane nanocomposite films as heat insulating materials. Polym. Adv. Technol. 2011, 22, 1905–1911. [Google Scholar] [CrossRef]
- Ge, C.; Zhai, W. Cellular thermoplastic polyurethane thin film: Preparation, elasticity, and thermal insulation performance. Ind. Eng. Chem. Res. 2018, 57, 4688–4696. [Google Scholar] [CrossRef]
- Kundu, S.; Das, A.; Basu, A.; Abdullah, M.; Mukherjee, A. Guar gum benzoate nanoparticle reinforced gelatin films for enhanced thermal insulation, mechanical and antimicrobial properties. Carbohydr. Polym. 2017, 170, 89–98. [Google Scholar] [CrossRef]
- Wallner, G.; Hausner, R.; Hegedys, H.; Schobermayr, H.; Lang, R. Application demonstration and performance of a cellulose triacetate polymer film based transparent insulation wall heating system. Sol. Energy 2006, 80, 1410–1416. [Google Scholar] [CrossRef]
- Chung, C.-Y.; Lee, M.-T.; Tsai, M.-Y.; Chu, C.-H.; Lin, S.-J. High thermal conductive diamond/Cu–Ti composites fabricated by pressureless sintering technique. Appl. Therm. Eng. 2014, 69, 208–213. [Google Scholar] [CrossRef]
- Firkowska, I.; Boden, A.; Boerner, B.; Reich, S. The origin of high thermal conductivity and ultralow thermal expansion in copper–graphite composites. Nano Lett. 2015, 15, 4745–4751. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; Jiang, P.; Xie, L. Ferroelectric polymer/silver nanocomposites with high dielectric constant and high thermal conductivity. Appl. Phys. Lett. 2009, 95, 242901. [Google Scholar] [CrossRef]
- Liu, M.; Ma, Y.; Wu, H.; Wang, R. Metal matrix–metal nanoparticle composites with tunable melting temperature and high thermal conductivity for phase-change thermal storage. ACS Nano 2015, 9, 1341–1351. [Google Scholar] [CrossRef] [PubMed]
- Sun, Z.; Li, J.; Yu, M.; Kathaperumal, M.; Wong, C.-P. A review of the thermal conductivity of silver-epoxy nanocomposites as encapsulation material for packaging applications. Chem. Eng. J. 2022, 446, 137319. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, Q.; Hou, L.; Liu, Y.; Li, Z. Ultralight, Ultraflexible, Anisotropic, Highly Thermally Conductive Graphene Aerogel Films. Molecules 2021, 26, 6867. [Google Scholar] [CrossRef] [PubMed]
- Kargar, F.; Barani, Z.; Balinskiy, M.; Magana, A.; Lewis, J.; Balandin, A. Dual-functional graphene composites for electromagnetic shielding and thermal management. Adv. Electron. Mater. 2019, 5, 1800558. [Google Scholar] [CrossRef]
- Min, P.; Liu, J.; Li, X.; An, F.; Liu, P.; Shen, Y.; Koratkar, N.; Yu, Z. Thermally conductive phase change composites featuring anisotropic graphene aerogels for real-time and fast-charging solar-thermal energy conversion. Adv. Funct. Mater. 2018, 28, 1805365. [Google Scholar] [CrossRef]
- Naghibi, S.; Kargar, F.; Wright, D.; Huang, C.; Mohammadzadeh, A.; Barani, Z.; Salgado, R.; Balandin, A. Noncuring graphene thermal interface materials for advanced electronics. Adv. Electron. Mater. 2020, 6, 1901303. [Google Scholar] [CrossRef]
- Pan, X.; Shen, L.; Schenning, A.; Bastiaansen, C. Transparent, high-thermal-conductivity ultradrawn polyethylene/graphene nanocomposite films. Adv. Mater. 2019, 31, 1904348. [Google Scholar] [CrossRef]
- Song, S.; Park, K.; Kim, B.; Choi, Y.; Jun, G.; Lee, D.; Kong, B.; Paik, K.; Jeon, S. Enhanced thermal conductivity of epoxy–graphene composites by using non-oxidized graphene flakes with non-covalent functionalization. Adv. Mater. 2013, 25, 732–737. [Google Scholar] [CrossRef] [PubMed]
- Zhong, B.; Dong, H.; Luo, Y.; Zhang, D.; Jia, Z.; Jia, D.; Liu, F. Simultaneous reduction and functionalization of graphene oxide via antioxidant for highly aging resistant and thermal conductive elastomer composites. Compos. Sci. Technol. 2017, 151, 156–163. [Google Scholar] [CrossRef]
- Guan, C.; Qin, Y.; Li, L.; Wang, M.; Lin, C.-T.; He, X.; Nishimura, K.; Yu, J.; Yi, J.; Jiang, N. Highly thermally conductive polymer composites with barnacle-like nano-crystalline Diamond@ Silicon carbide hybrid architecture. Compos. Part B Eng. 2020, 198, 108167. [Google Scholar] [CrossRef]
- Lee, E.; Salgado, R.; Lee, B.; Sumant, A.; Rajh, T.; Johnson, C.; Balandin, A.; Shevchenko, E. Design of lithium cobalt oxide electrodes with high thermal conductivity and electrochemical performance using carbon nanotubes and diamond particles. Carbon 2018, 129, 702–710. [Google Scholar] [CrossRef]
- Mizuuchi, K.; Inoue, K.; Agari, Y.; Morisada, Y.; Sugioka, M.; Tanaka, M.; Takeuchi, T.; Kawahara, M.; Makino, Y. Thermal conductivity of diamond particle dispersed aluminum matrix composites fabricated in solid–liquid co-existent state by SPS. Compos. Part B Eng. 2011, 42, 1029–1034. [Google Scholar] [CrossRef]
- Wang, L.; Li, J.; Che, Z.; Wang, X.; Zhang, H.; Wang, J.; Kim, M. Combining Cr pre-coating and Cr alloying to improve the thermal conductivity of diamond particles reinforced Cu matrix composites. J. Alloy. Compd. 2018, 749, 1098–1105. [Google Scholar] [CrossRef]
- Xu, B.; Hung, S.-W.; Hu, S.; Shao, C.; Guo, R.; Choi, J.; Kodama, T.; Chen, F.-R.; Shiomi, J. Scalable monolayer-functionalized nanointerface for thermal conductivity enhancement in copper/diamond composite. Carbon 2021, 175, 299–306. [Google Scholar] [CrossRef]
- Zhang, L.; Wei, Q.; An, J.; Ma, L.; Zhou, K.; Ye, W.; Yu, Z.; Gan, X.; Lin, C.-T.; Luo, J. Construction of 3D interconnected diamond networks in Al-matrix composite for high-efficiency thermal management. Chem. Eng. J. 2020, 380, 122551. [Google Scholar] [CrossRef]
- Cho, J.; Losego, M.; Zhang, H.; Kim, H.; Zuo, J.; Petrov, I.; Cahill, D.; Braun, P. Electrochemically tunable thermal conductivity of lithium cobalt oxide. Nat. Commun. 2014, 5, 4035. [Google Scholar] [CrossRef]
- Lu, Q.; Huberman, S.; Zhang, H.; Song, Q.; Wang, J.; Vardar, G.; Hunt, A.; Waluyo, I.; Chen, G.; Yildiz, B. Bi-directional tuning of thermal transport in SrCoOx with electrochemically induced phase transitions. Nat. Mater. 2020, 19, 655–662. [Google Scholar] [CrossRef]
- Zhang, X.; Li, J.; Lin, J.; Tong, P.; Wang, M.; Wang, X.; Tong, H.; Zhang, Y.; Song, W.; Sun, Y. High-contrast, reversible change of thermal conductivity in hexagonal nickel-iron sulfides. Acta Mater. 2021, 208, 116709. [Google Scholar] [CrossRef]
- Yao, B.; Xu, X.; Li, H.; Han, Z.; Hao, J.; Yang, G.; Xie, Z.; Chen, Y.; Liu, W.; Wang, Q. Soft liquid-metal/elastomer foam with compression-adjustable thermal conductivity and electromagnetic interference shielding. Chem. Eng. J. 2021, 410, 128288. [Google Scholar] [CrossRef]
- Liang, X.; Fan, A.; Li, Z.; Wei, N.; Fan, W.; Liang, H.; Wang, H.; Bi, P.; Li, S.; Wu, X. Highly Regulatable Heat Conductance of Graphene–Sericin Hybrid for Responsive Textiles. Adv. Funct. Mater. 2022, 32, 2111121. [Google Scholar] [CrossRef]
- Harish, S.; Ishikawa, K.; Chiashi, S.; Shiomi, J.; Maruyama, S. Anomalous thermal conduction characteristics of phase change composites with single-walled carbon nanotube inclusions. J. Phys. Chem. C 2013, 117, 15409–15413. [Google Scholar] [CrossRef]
- Ho, C.; Powell, R.; Liley, P. Thermal conductivity of the elements. J. Phys. Chem. Ref. Data 1972, 1, 279–421. [Google Scholar] [CrossRef]
- Mehra, N.; Mu, L.; Ji, T.; Li, Y.; Zhu, J. Moisture driven thermal conduction in polymer and polymer blends. Compos. Sci. Technol. 2017, 151, 115–123. [Google Scholar] [CrossRef]
- Sun, P.; Wu, Y.; Gao, J.; Cheng, G.; Chen, G.; Zheng, R. Room temperature electrical and thermal switching CNT/hexadecane composites. Adv. Mater. 2013, 25, 4938–4943. [Google Scholar] [CrossRef] [PubMed]
- Zheng, Q.; Zhu, G.; Diao, Z.; Banerjee, D.; Cahill, D. High contrast thermal conductivity change in Ni–Mn–In heusler alloys near room temperature. Adv. Eng. Mater. 2019, 21, 1801342. [Google Scholar] [CrossRef]
- Zheng, R.; Gao, J.; Wang, J.; Chen, G. Reversible temperature regulation of electrical and thermal conductivity using liquid–solid phase transitions. Nat. Commun. 2011, 2, 289. [Google Scholar] [CrossRef]
- Lü, X.; Tang, H.; Wang, H.; Meng, X.; Li, F. Ultra-soft thermal self-healing liquid-metal-foamed composite with high thermal conductivity. Compos. Sci. Technol. 2022, 226, 109523. [Google Scholar] [CrossRef]
- Gouveia, B.; Kim, Y.; Shaevitz, J.; Petry, S.; Stone, H.; Brangwynne, C. Capillary forces generated by biomolecular condensates. Nature 2022, 609, 255–264. [Google Scholar] [CrossRef] [PubMed]
- Levin, A.; Hakala, T.; Schnaider, L.; Bernardes, G.; Gazit, E.; Knowles, T. Biomimetic peptide self-assembly for functional materials. Nat. Rev. Chem. 2020, 4, 615–634. [Google Scholar] [CrossRef]
- Li, J.; Li, H.; Xu, L.; Wang, L.; Hu, Z.; Liu, L.; Huang, Y.; Kotov, N. Biomimetic nanoporous aerogels from branched aramid nanofibers combining high heat insulation and compressive strength. SmartMat 2021, 2, 76–87. [Google Scholar] [CrossRef]
- Liu, J.; Zhorabek, F.; Chau, Y. Biomaterial design inspired by membraneless organelles. Matter 2022, 5, 2787–2812. [Google Scholar] [CrossRef]
- Liu, J.; Zhorabek, F.; Dai, X.; Huang, J.; Chau, Y. Minimalist design of an Intrinsically disordered protein-Mimicking Scaffold for an artificial membraneless organelle. ACS Cent. Sci. 2022, 8, 493–500. [Google Scholar] [CrossRef]
- Wang, D.; Peng, H.; Yu, B.; Zhou, K.; Pan, H.; Zhang, L.; Li, M.; Liu, M.; Tian, A.; Fu, S. Biomimetic structural cellulose nanofiber aerogels with exceptional mechanical, flame-retardant and thermal-insulating properties. Chem. Eng. J. 2020, 389, 124449. [Google Scholar] [CrossRef]
- Dickey, M.; Chiechi, R.; Larsen, R.; Weiss, E.; Weitz, D.; Whitesides, G. Eutectic gallium-indium (EGaIn): A liquid metal alloy for the formation of stable structures in microchannels at room temperature. Adv. Funct. Mater. 2008, 18, 1097–1104. [Google Scholar] [CrossRef]
- Burleigh, D.; Gagliani, J. Thermal conductivity of PVC foams for spacecraft applications. Therm. Conduct. 1989, 20, 41–45. [Google Scholar]
- Caps, R.; Heinemann, U.; Fricke, J.; Keller, K. Thermal conductivity of polyimide foams. Int. J. Heat Mass Transf. 1997, 40, 269–280. [Google Scholar] [CrossRef]
- Zhang, H.; Fang, W.-Z.; Li, Y.-M.; Tao, W.-Q. Experimental study of the thermal conductivity of polyurethane foams. Appl. Therm. Eng. 2017, 115, 528–538. [Google Scholar] [CrossRef]
- Almanza, O.; Rodríguez-Pérez, M.; De Saja, J. Applicability of the transient plane source method to measure the thermal conductivity of low-density polyethylene foams. J. Polym. Sci. Part B Polym. Phys. 2004, 42, 1226–1234. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, H.; Lü, X.; Meng, X.; Wang, H.; Bai, G.; Bao, W. Biomimetic Liquid Metal–Elastomer Composited Foam with Adjustable Thermal Conductivity for Heat Control. Molecules 2023, 28, 1688. https://doi.org/10.3390/molecules28041688
Tang H, Lü X, Meng X, Wang H, Bai G, Bao W. Biomimetic Liquid Metal–Elastomer Composited Foam with Adjustable Thermal Conductivity for Heat Control. Molecules. 2023; 28(4):1688. https://doi.org/10.3390/molecules28041688
Chicago/Turabian StyleTang, Hongyao, Xiaozhou Lü, Xiangyu Meng, Hai Wang, Guanghui Bai, and Weimin Bao. 2023. "Biomimetic Liquid Metal–Elastomer Composited Foam with Adjustable Thermal Conductivity for Heat Control" Molecules 28, no. 4: 1688. https://doi.org/10.3390/molecules28041688
APA StyleTang, H., Lü, X., Meng, X., Wang, H., Bai, G., & Bao, W. (2023). Biomimetic Liquid Metal–Elastomer Composited Foam with Adjustable Thermal Conductivity for Heat Control. Molecules, 28(4), 1688. https://doi.org/10.3390/molecules28041688







