Tautomeric Equilibrium in 1-Benzamidoisoquinoline Derivatives
Abstract
1. Introduction
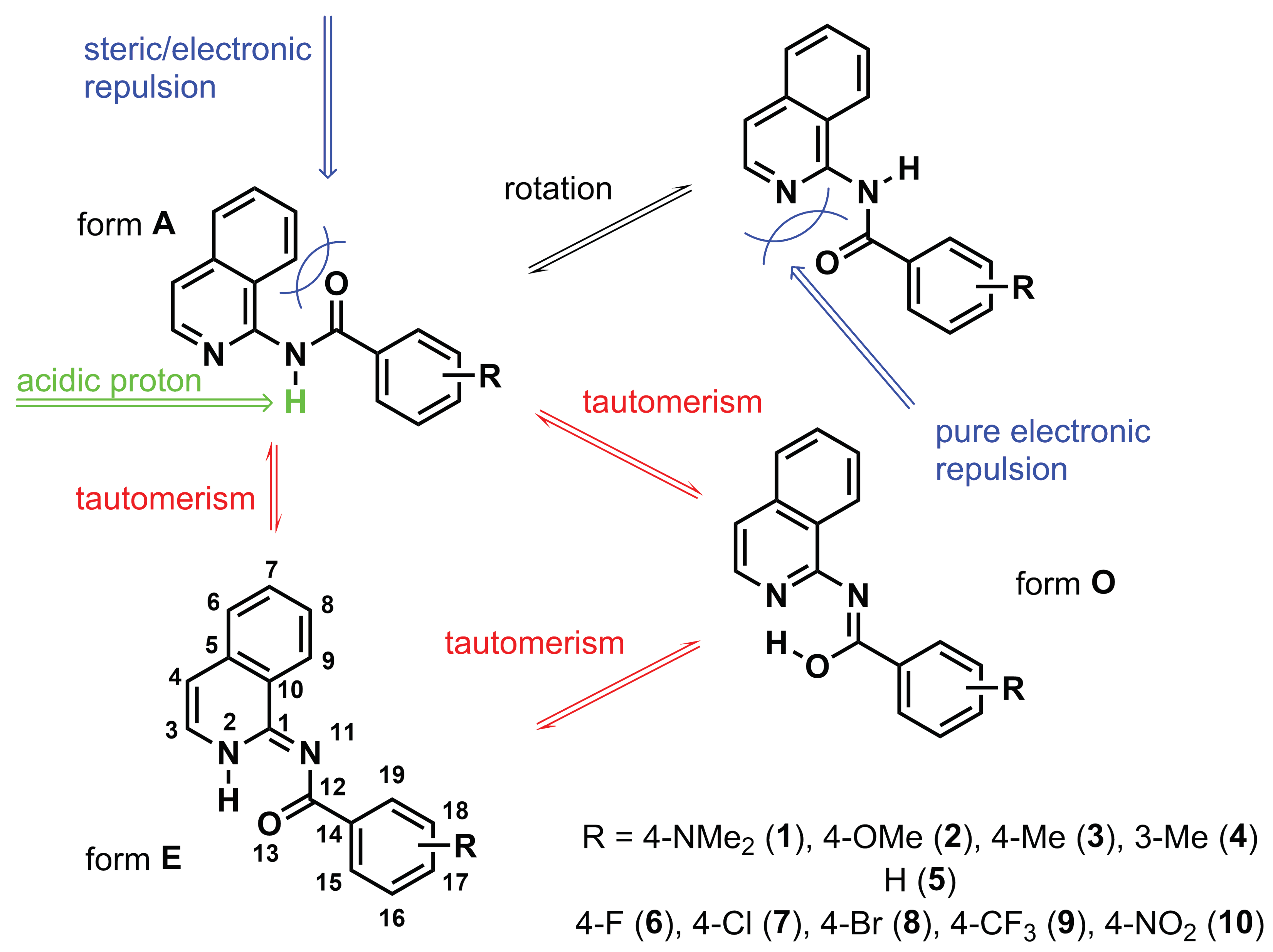
2. Results
2.1. Experiments
2.2. Theoretical Calculations
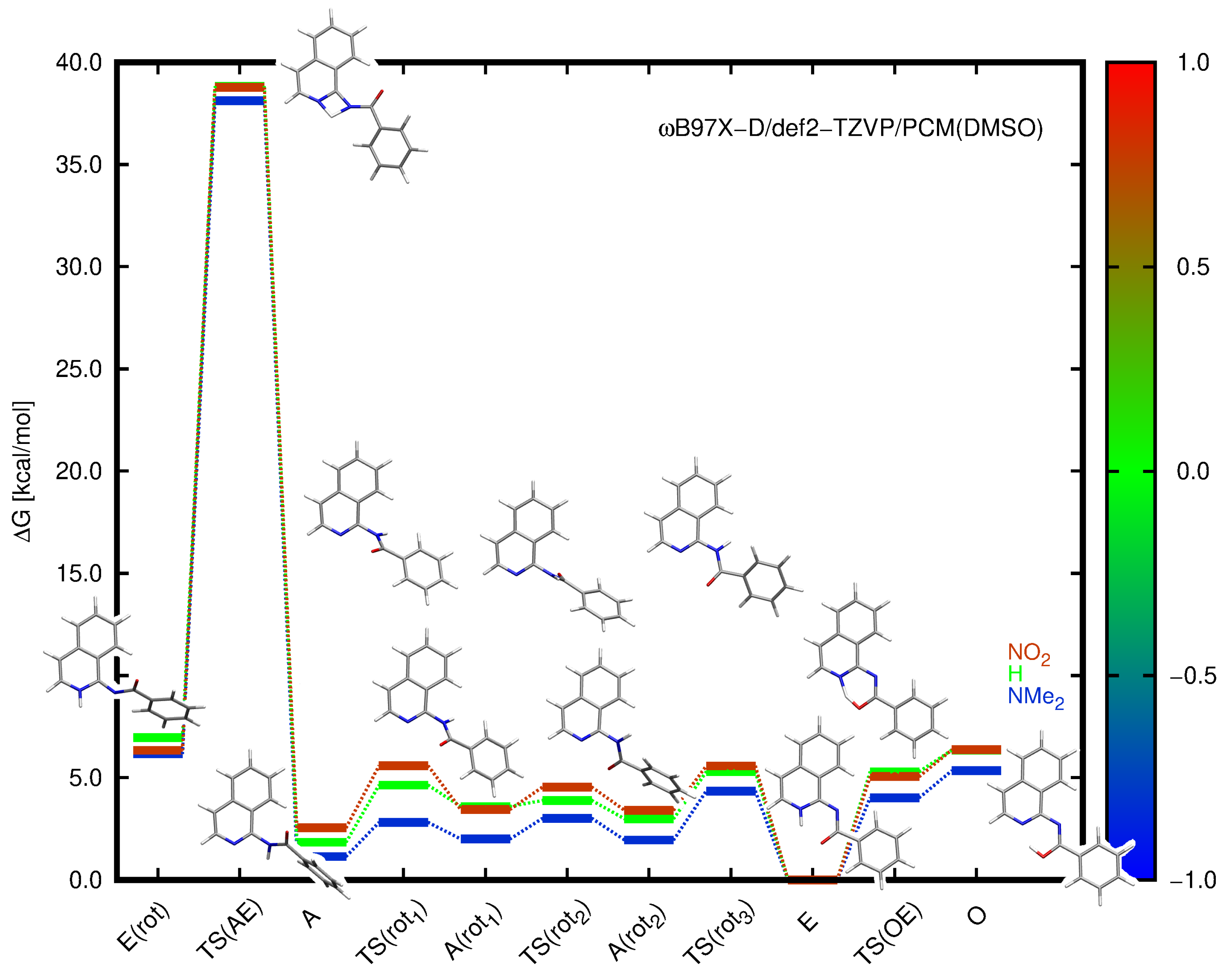
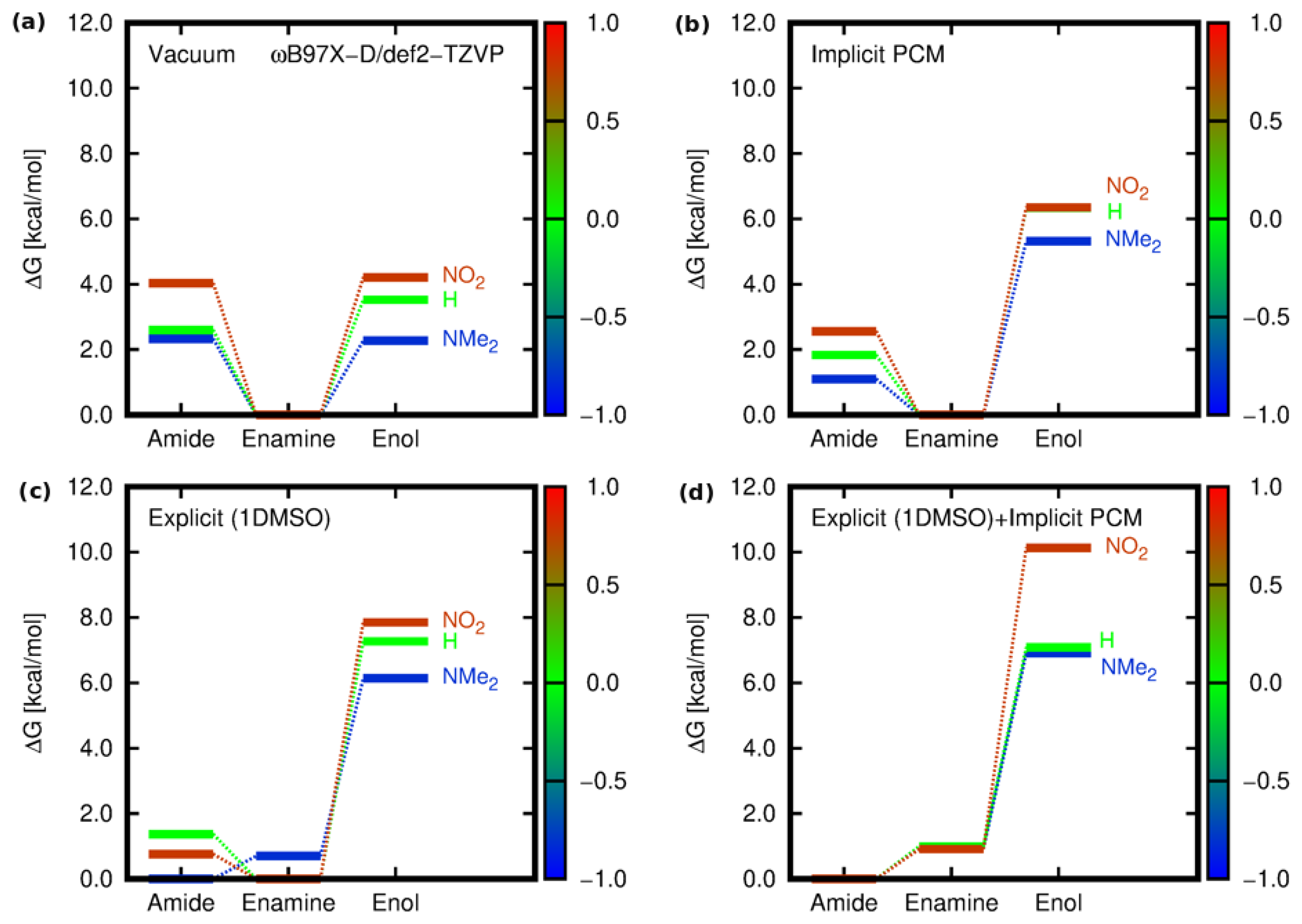
2.2.1. Tautomeric Equilibrium in Solvent
2.2.2. Barriers to Proton Transfer and Internal Rotation
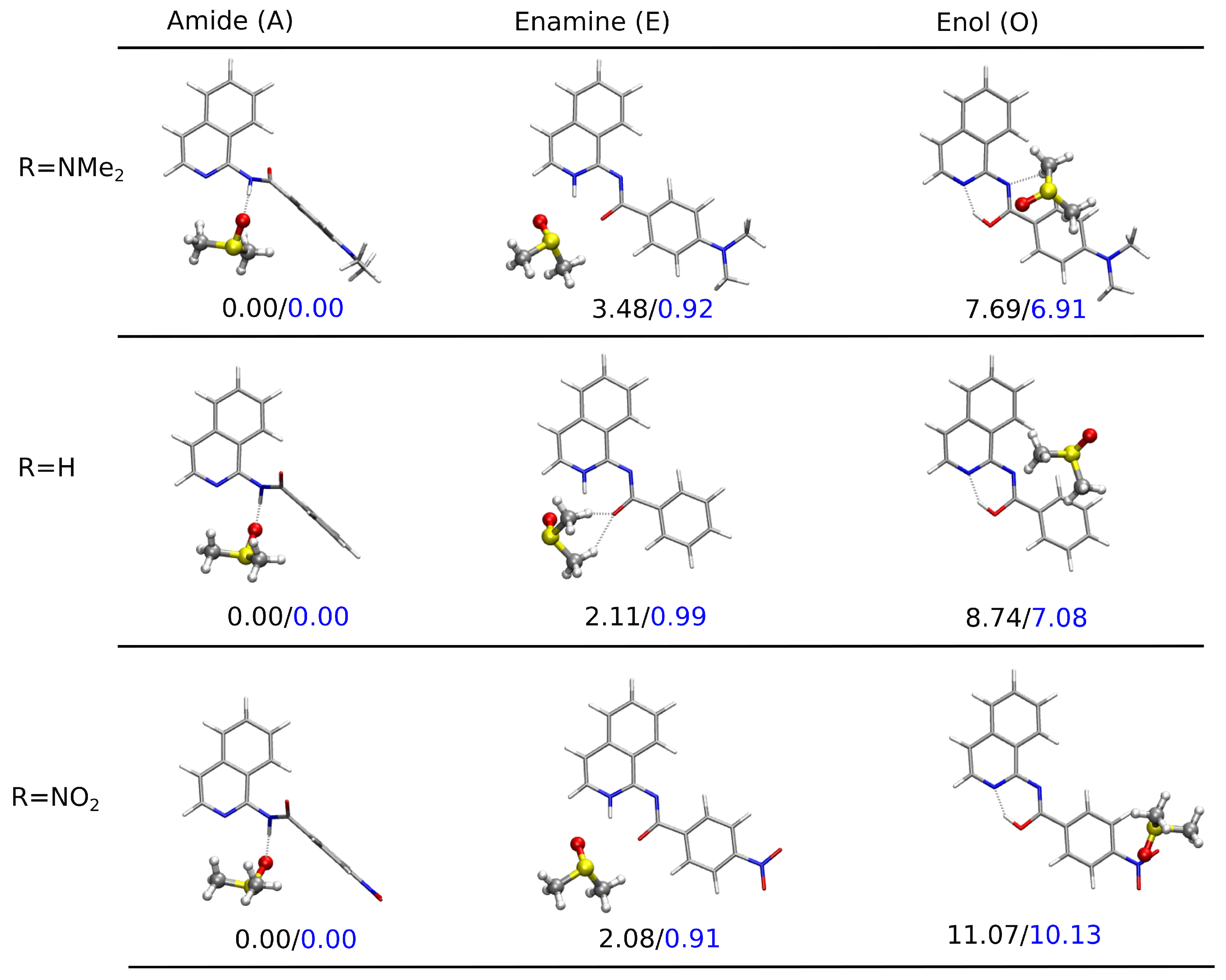
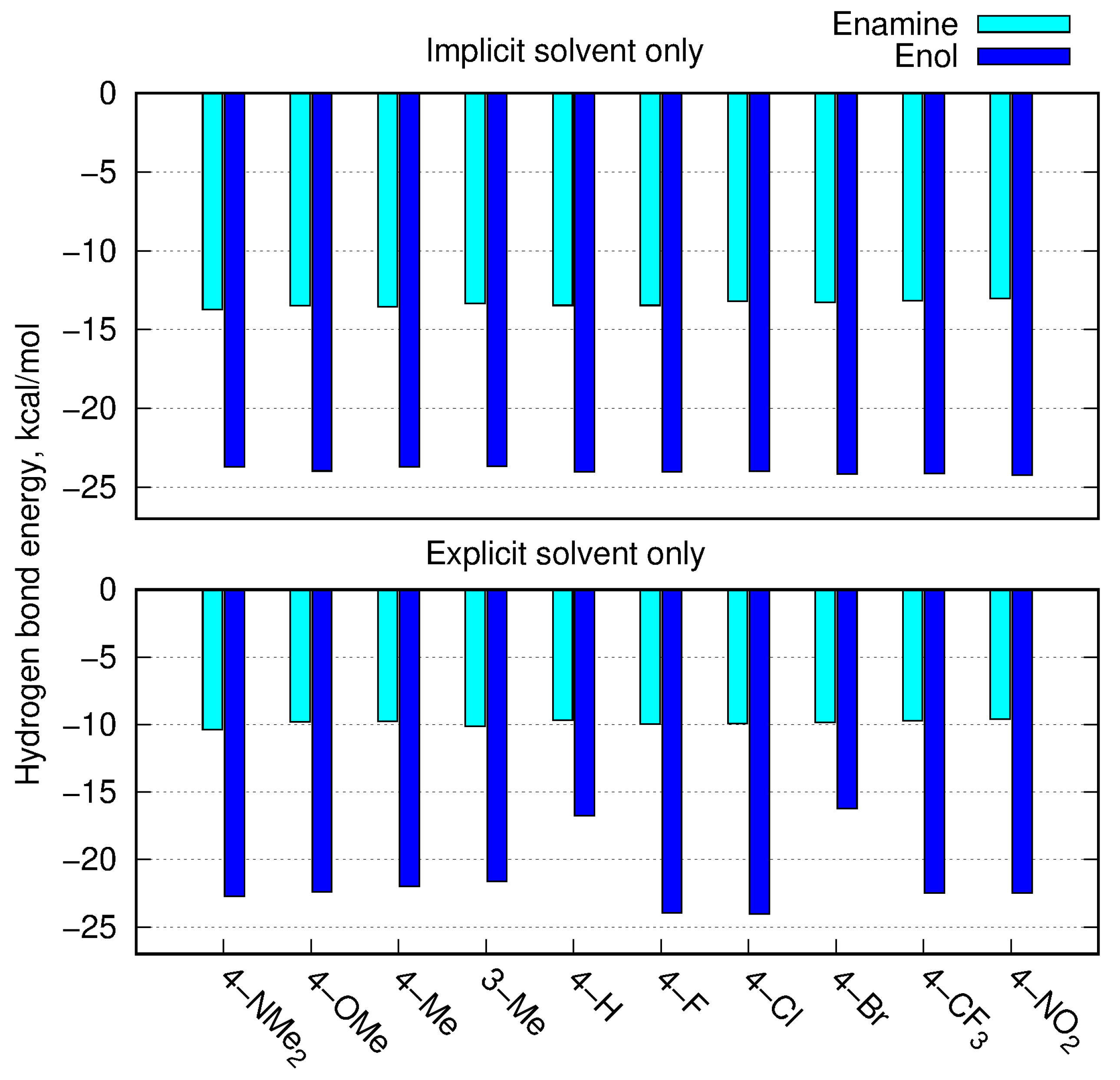
2.2.3. Non-Covalent Interactions
3. Materials and Methods
3.1. Synthesis
3.1.1. Method A
3.1.2. Method B
3.2. Technical Details for Theoretical Calculations
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kolehmainen, E.; Ośmiałowski, B.; Nissinen, M.; Kauppinen, R.; Gawinecki, R. Substituent and Temperature Controlled Tautomerism of 2-Phenacylpyridine: The Hydrogen Bond as a Configurational Lock of (Z )-2-(2-hydroxy-2-phenylvinyl)pyridine. J. Chem. Soc. Perkin Trans. 2000, 2, 2185–2191. [Google Scholar] [CrossRef]
- Slocombe, L.; Al-Khalili, J.S.; Sacchi, M. Quantum and Classical Effects in DNA Point Mutations: Watson-Crick Tautomerism in AT and GC Base Pairs. Phys. Chem. Chem. Phys. 2021, 23, 4141–4150. [Google Scholar] [CrossRef] [PubMed]
- Ishizuka, T.; Sakashita, R.; Iwanaga, O.; Morimoto, T.; Mori, S.; Ishida, M.; Toganoh, M.; Takegoshi, K.; Osuka, A.; Furuta, H. NH Tautomerism of N-Confused Porphyrin: Solvent/Substituent Effects and Isomerization Mechanism. J. Phys. Chem. A 2020, 124, 5756–5769. [Google Scholar] [CrossRef]
- Pérez, A.; Tuckerman, M.E.; Hjalmarson, H.P.; von Lilienfeld, O.A. Enol Tautomers of Watson-Crick Base Pair Models Are Metastable Because of Nuclear Quantum Effects. J. Am. Chem. Soc. 2010, 132, 11510–11515. [Google Scholar] [CrossRef]
- Friedberg, E.C. DNA Damage and Repair. Nature 2003, 421, 436–440. [Google Scholar] [CrossRef]
- Sedgwick, A.C.; Wu, L.; Han, H.H.; Bull, S.D.; He, X.P.; James, T.D.; Sessler, J.L.; Tang, B.Z.; Tian, H.; Yoon, J. Excited-State Intramolecular Proton-Transfer (ESIPT) Based Fluorescence Sensors and Imaging Agents. Chem. Soc. Rev. 2018, 47, 8842–8880. [Google Scholar] [CrossRef] [PubMed]
- Kwiatkowski, A.; Kolehmainen, E.; Ośmiałowski, B. Conformational and Tautomeric Control by Supramolecular Approach in Ureido-N-iso-propyl,N’-4-(3-pyridin-2-one)pyrimidine. Molecules 2019, 24, 2491. [Google Scholar] [CrossRef] [PubMed]
- Sharif, S.; Denisov, G.S.; Toney, M.D.; Limbach, H.H. NMR Studies of Coupled Low- and High-Barrier Hydrogen Bonds in Pyridoxal-5‘-phosphate Model Systems in Polar Solution. J. Am. Chem. Soc. 2007, 129, 6313–6327. [Google Scholar] [CrossRef]
- Kolehmainen, E.; Ośmiałowski, B.; Krygowski, T.M.; Kauppinen, R.; Nissinena, M.; Gawinecki, R. Substituent and Temperature Controlled Tautomerism: Multinuclear Magnetic Resonance, X-ray, and Theoretical Studies on 2-Phenacylquinolines. J. Chem. Soc. Perkin Trans. 2000, 2, 1259–1266. [Google Scholar] [CrossRef]
- Gawinecki, R.; Kolehmainen, E.; Loghmani-Khouzani, H.; Ośmiałowski, B.; Lovász, T.; Rosa, P. Effect of π-Electron Delocalization on Tautomeric Equilibria – Benzoannulated 2-Phenacylpyridines. Eur. J. Org. Chem. 2006, 2006, 2817–2824. [Google Scholar] [CrossRef]
- Frey, J.; Rappoport, Z. Observation of an Amide Enol of Bis(2,4,6-triisopropylphenyl)acetic Acid. J. Am. Chem. Soc. 1996, 118, 3994–3995. [Google Scholar] [CrossRef]
- Basheer, A.; Rappoport, Z. Enols of Amides Activated by the 2,2,2-Trichloroethoxycarbonyl Group. J. Org. Chem. 2004, 69, 1151–1160. [Google Scholar] [CrossRef]
- Basheer, A.; Rappoport, Z. Oxa-ene Reaction of Enols of Amides with 4-Phenyl-1,2,4-triazoline-3,5-dione. J. Org. Chem. 2008, 73, 184–190. [Google Scholar] [CrossRef] [PubMed]
- O’Ferrall, R.A.M.; Murray, B.A. 1H and 13C NMR Spectra of α-Heterocyclic Ketones and Assignment of Keto, Enol and Enaminone Tautomeric Structures. J. Chem. Soc. Perkin Trans. 1994, 2, 2461–2470. [Google Scholar] [CrossRef]
- Kleinpeter, E. Quantification and Visualization of the Anisotropy Effect in NMR Spectroscopy by Through-Space NMR Shieldings. Annu. Rep. Nmr Spectrosc. 2014, 82, 115–166. [Google Scholar] [CrossRef]
- Ośmiałowski, B.; Kolehmainen, E. Comment on “Non-symmetric Substituted Ureas Locked in an (E,Z) Conformation: An Unusual Anion Binding via Supramolecular Assembly” by M. Olivari, C. Caltagirone, A. Garau, F. Isaia, M. E. Light, V. Lippolis, R. Montis and M. A. Scorciapino, New J. Chem., 2013, 37, 663. New J. Chem. 2014, 38, 2701–2703. [Google Scholar] [CrossRef]
- Mardirossian, N.; Head-Gordon, M. Thirty Years of Density Functional Theory in Computational Chemistry: An Overview and Extensive Assessment of 200 Density Functionals. Mol. Phys. 2017, 115, 2315–2372. [Google Scholar] [CrossRef]
- Weigend, F.; Ahlrichs, R. Balanced Basis Sets of Split Valence, Triple Zeta Valence and Quadruple Zeta Valence Quality for H to Rn: Design and Assessment of Accuracy. Phys. Chem. Chem. Phys. 2005, 7, 3297–3305. [Google Scholar] [CrossRef] [PubMed]
- Karton, A.; O’Reilly, R.J.; Radom, L. Assessment of Theoretical Procedures for Calculating Barrier Heights for a Diverse Set of Water-Catalyzed Proton-Transfer Reactions. J. Phys. Chem. A 2012, 116, 4211–4221. [Google Scholar] [CrossRef]
- Oziminski, W.P. Theoretical Study on the Solvent Influence on 1,2,3-Triazole Tautomeric Equilibrium. A Comparison of Incremental Microsolvation and Continuum Solvation Model Approaches. Tetrahedron 2013, 69, 3197–3205. [Google Scholar] [CrossRef]
- Montgomery, J.A., Jr.; Frisch, M.J.; Ochterski, J.W.; Petersson, G.A. A Complete Basis Set Model Chemistry. VI. Use of Density Functional Geometries and Frequencies. J. Chem. Phys. 1999, 110, 2822. [Google Scholar] [CrossRef]
- Montgomery, J.A., Jr.; Frisch, M.J.; Ochterski, J.W.; Petersson, G.A. A Complete Basis Set Model Chemistry. VII. Use of the Minimum Population Localization Method. J. Chem. Phys. 2000, 112, 6532. [Google Scholar] [CrossRef]
- Léon, I.; Tasinato, N.; Spada, L.; Alonso, E.R.; Mata, S.; Balbi, A.; Puzzarini, C.; Alonso, J.L.; Barone, V. Looking for the Elusive Imine Tautomer of Creatinine: Different States of Aggregation Studied by Quantum Chemistry and Molecular Spectroscopy. ChemPlusChem 2021, 86, 1374–1386. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.S.; Ahn, D.S.; Chung, S.Y.; Kim, S.K.; Lee, S. Tautomerization of Adenine Facilitated by Water: Computational Study of Microsolvation. J. Phys. Chem. A 2007, 111, 8007–8012. [Google Scholar] [CrossRef] [PubMed]
- Mata, S.; Cortijo, V.; Caminati, W.; Alonso, J.L.; Sanz, M.E.; López, J.C.; Blanco, S. Tautomerism and Microsolvation in 2-Hydroxypyridine/2-Pyridone. J. Phys. Chem. A 2010, 114, 11393–11398. [Google Scholar] [CrossRef] [PubMed]
- Simm, G.N.; Türtscher, P.L.; Reiher, M. Systematic Microsolvation Approach with a Cluster-Continuum Scheme and Conformational Sampling. J. Comput. Chem. 2020, 41, 1144–1155. [Google Scholar] [CrossRef]
- Raczynska, E.D.; Kaminska, B. Variations of the Tautomeric Preferences and π-Electron Delocalization for the Neutral and Redox Forms of Purine when Proceeding from the Gas Phase (DFT) to Water (PCM). J. Mol. Model. 2013, 19, 3947–3960. [Google Scholar] [CrossRef]
- Espinosa, E.; Molins, E.; Lecomte, C. Hydrogen Bond Strengths Revealed by Topological Analyses of Experimentally Observed Electron Densities. Chem. Phys. Lett. 1998, 285, 170–173. [Google Scholar] [CrossRef]
- Bannwarth, C.; Caldeweyher, E.; Ehlert, S.; Hansen, A.; Pracht, P.; Seibert, J.; Spicher, S.; Grimme, S. Extended Tight-Binding Quantum Chemistry Methods. WIREs Comput. Mol. Sci. 2020, 11, e1493. [Google Scholar] [CrossRef]
- Bannwarth, C.; Ehlert, S.; Grimme, S. GFN2-xTB—An Accurate and Broadly Parametrized Self-Consistent Tight-Binding Quantum Chemical Method with Multipole Electrostatics and Density-Dependent Dispersion Contributions. J. Chem. Theory Comput. 2019, 15, 1652–1671. [Google Scholar] [CrossRef]
- Pracht, P.; Bohle, F.; Grimme, S. Automated Exploration of the Low-Energy Chemical Space with Fast Quantum Chemical Methods. Phys. Chem. Chem. Phys. 2020, 22, 7169–7192. [Google Scholar] [CrossRef]
- Chai, J.D.; Head-Gordon, M. Long-range Corrected Hybrid Density Functionals with Damped Atom–Atom Dispersion Corrections. Phys. Chem. Chem. Phys. 2008, 10, 6615–6620. [Google Scholar] [CrossRef]
- Smith, D.G.A.; Burns, L.A.; Simmonett, A.C.; Parrish, R.M.; Schieber, M.C.; Galvelis, R.; Kraus, P.; Kruse, H.; Remigio, R.D.; Alenaizan, A.; et al. Psi4 1.4: Open-Source Software for High-Throughput Quantum Chemistry. J. Chem. Phys. 2020, 152, 184108. [Google Scholar] [CrossRef]
- Parker, T.M.; Burns, L.A.; Parrish, R.M.; Ryno, A.G.; Sherrill, C.D. Levels of Symmetry Adapted Perturbation Theory (SAPT). I. Efficiency and Performance for Interaction Energies. J. Chem. Phys. 2014, 140, 094106. [Google Scholar] [CrossRef] [PubMed]
- Jeziorski, B.; Moszynski, R.; Szalewicz, K. Perturbation Theory Approach to Intermolecular Potential Energy Surfaces of van der Waals Complexes. Chem. Rev. 1994, 94, 1887–1930. [Google Scholar] [CrossRef]
- Hohenstein, E.G.; Sherrill, C.D. Wavefunction Methods for Noncovalent Interactions. WIREs Comput. Mol. Sci. 2012, 2, 304–326. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16 Revision C.01; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Neese, F.; Wennmohs, F.; Becker, U.; Riplinger, C. The ORCA Quantum Chemistry Program Package. J. Chem. Phys. 2020, 152, 224108. [Google Scholar] [CrossRef]
- Keith, T.A. AIMAll (Version 19.10.12). 2019. Available online: http://aim.tkgristmill.com/ (accessed on 18 January 2023).
- Cremer, D.; Kraka, E. A description of the chemical bond in terms of local properties 87 of electron density and energy. Croat. Chim. Acta 1984, 57, 1259–1281. [Google Scholar]
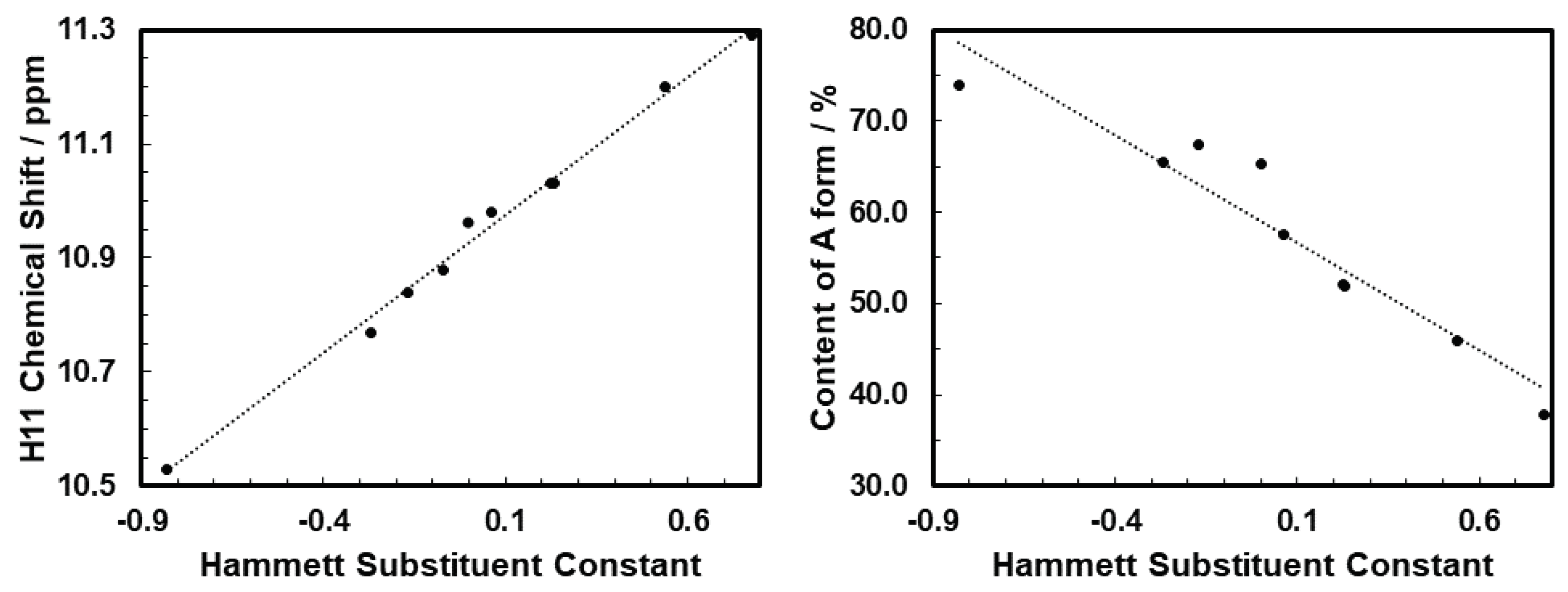

| Comp. (R) | (H2) | (H11) | (H3) | (H9) | Fraction (%) | |
|---|---|---|---|---|---|---|
| 1E (4-NMe) | −0.83 | 14.87 | 8.86 | 7.09 | 26 | |
| 1A | 10.53 | 8.35 | 7.91 | 74 | ||
| 2E (4-OMe) | −0.27 | 14.86 | 8.90 | 7.18 | 35 | |
| 2A | 10.77 | 8.37 | 7.94 | 65 | ||
| 3E (4-Me) | −0.17 | 14.89 | 8.93 | 7.21 | 33 | |
| 3A | 10.84 | 8.37 | 7.95 | 67 | ||
| 4E (3-Me) | −0.07 | 14.98 | 8.93 | 7.22 | 32 | |
| 4A | 10.88 | 8.38 | 7.97 | 68 | ||
| 5E (4-H) | 0.00 | 14.83 | 8.92 | 7.23 | 35 | |
| 5A | 10.96 | 8.39 | 7.98 | 65 | ||
| 6E (4-F) | 0.06 | 14.80 | 8.92 | 7.24 | 42 | |
| 6A | 10.98 | 8.38 | 7.97 | 58 | ||
| 7E (4-Cl) | 0.23 | 14.83 | 8.91 | 7.25 | 48 | |
| 7A | 11.03 | 8.38 | 7.98 | 52 | ||
| 8E (4-Br) | 0.23 | 14.83 | 8.91 | 7.26 | 48 | |
| 8A | 11.03 | 8.38 | 7.97 | 52 | ||
| 9E (4-CF) | 0.54 | 14.85 | 8.94 | 7.32 | 54 | |
| 9A | 11.20 | 8.40 | 8.03 | 46 | ||
| 10E (4-NO) | 0.78 | 14.82 | 8.95 | 7.34 | 62 | |
| 10A | 11.29 | 8.39 | 8.03 | 38 |
| B97X-D/def2-TZVP | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Vacuum | Implicit | Explicit | Hybrid | |||||||||
| Substituent | A | E | O | A | E | O | A | E | O | A | E | O |
| 1 (4-NMe) | 2 | 96 | 2 | 13 | 87 | 0 | 77 | 23 | 0 | 83 | 17 | 0 |
| 10 (4-NO) | 0 | 100 | 0 | 1 | 99 | 9 | 21 | 79 | 0 | 83 | 17 | 0 |
| Implicit Solvent | Explicit Solvent | Explicit–Implicit Solvent | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Compound | A | E | O | A | E | O | A | E | O |
| 1 (4-NMe) | 1.10 | 0.00 | 5.32 | 0.00 | 0.70 | 6.14 | 0.00 | 0.92 | 6.91 |
| 2 (4-OMe) | 2.56 | 0.00 | 5.59 | 1.45 | 0.00 | 7.99 | 0.00 | 1.72 | 8.43 |
| 3 (4-Me) | 2.85 | 0.00 | 6.78 | 0.95 | 0.00 | 8.15 | 0.00 | 1.69 | 7.71 |
| 4 (3-Me) | 1.42 | 0.00 | 5.43 | 0.14 | 0.00 | 6.75 | 0.00 | 2.33 | 6.36 |
| 5 (H) | 1.83 | 0.00 | 6.34 | 1.37 | 0.00 | 7.27 | 0.00 | 0.99 | 7.08 |
| 6 (4-F) | 2.78 | 0.00 | 6.17 | 1.09 | 0.00 | 7.58 | 0.00 | 0.46 | 6.82 |
| 7 (4-Cl) | 2.68 | 0.00 | 5.88 | 0.65 | 0.00 | 7.74 | 0.00 | 0.64 | 6.97 |
| 8 (4-Br) | 2.67 | 0.00 | 5.97 | 0.41 | 0.00 | 8.30 | 0.00 | 1.13 | 7.30 |
| 9 (4-CF) | 0.35 | 0.00 | 4.34 | 0.60 | 0.00 | 8.19 | 0.00 | 2.54 | 8.41 |
| 10 (4-NO) | 2.56 | 0.00 | 6.35 | 0.76 | 0.00 | 7.85 | 0.00 | 0.91 | 10.13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rybczyński, P.; Kaczmarek-Kędziera, A.; Iglesias-Reguant, A.; Plażuk, D.; Ośmiałowski, B. Tautomeric Equilibrium in 1-Benzamidoisoquinoline Derivatives. Molecules 2023, 28, 1101. https://doi.org/10.3390/molecules28031101
Rybczyński P, Kaczmarek-Kędziera A, Iglesias-Reguant A, Plażuk D, Ośmiałowski B. Tautomeric Equilibrium in 1-Benzamidoisoquinoline Derivatives. Molecules. 2023; 28(3):1101. https://doi.org/10.3390/molecules28031101
Chicago/Turabian StyleRybczyński, Patryk, Anna Kaczmarek-Kędziera, Alex Iglesias-Reguant, Damian Plażuk, and Borys Ośmiałowski. 2023. "Tautomeric Equilibrium in 1-Benzamidoisoquinoline Derivatives" Molecules 28, no. 3: 1101. https://doi.org/10.3390/molecules28031101
APA StyleRybczyński, P., Kaczmarek-Kędziera, A., Iglesias-Reguant, A., Plażuk, D., & Ośmiałowski, B. (2023). Tautomeric Equilibrium in 1-Benzamidoisoquinoline Derivatives. Molecules, 28(3), 1101. https://doi.org/10.3390/molecules28031101






