Isotropic Contact Properties in Monolayer GeAs Field-Effect Transistors
Abstract
:1. Introduction
2. Results and Discussion
2.1. Interface and Device Models
2.2. Structure and Electronic Properties of the ML GeAs/Metal Interfaces
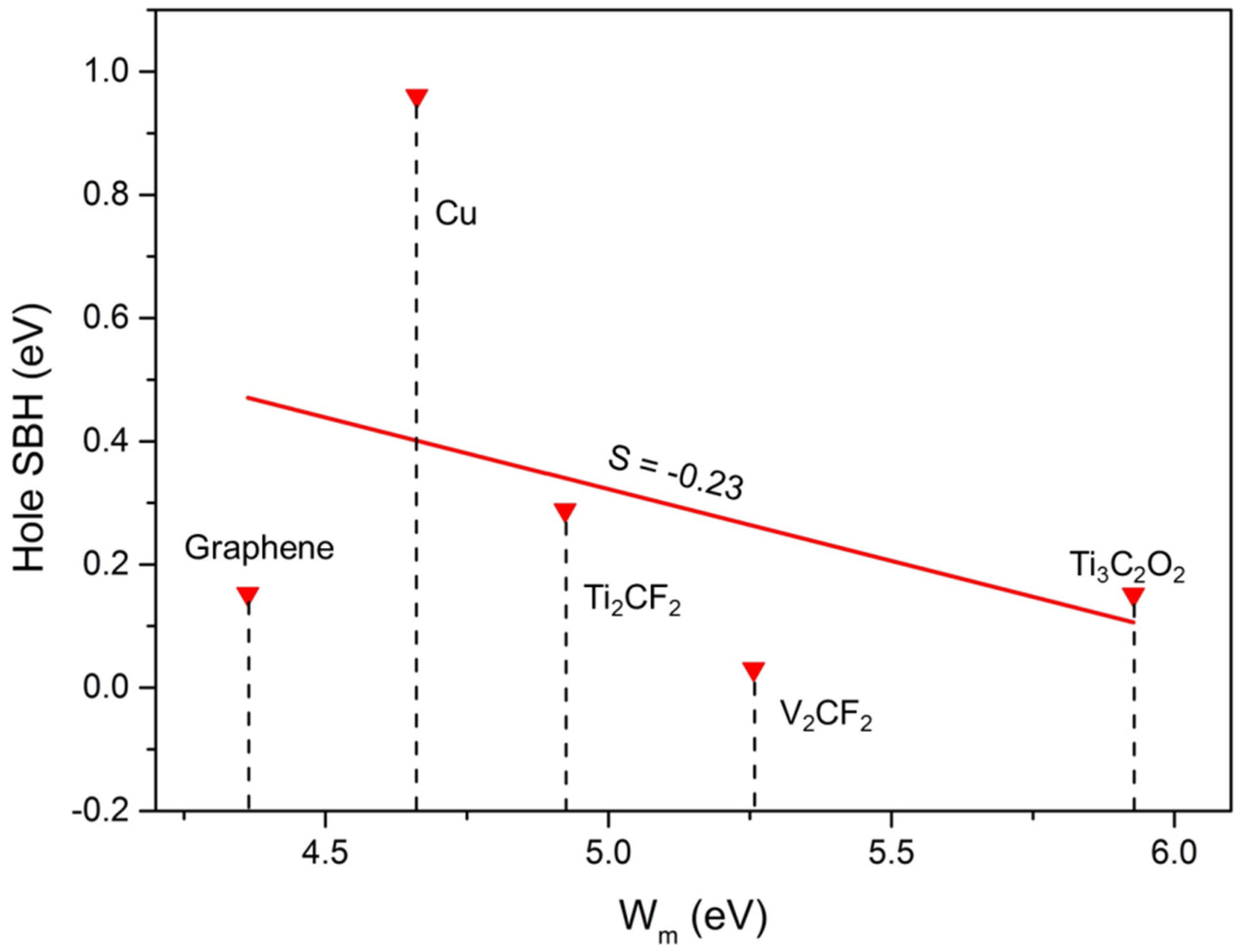
2.3. Schottky Barriers in the ML GeAs FETs
2.3.1. Vertical Schottky Barriers in the ML GeAs FETs
2.3.2. Lateral Schottky Barriers in the ML GeAs FETs
2.4. Discussion
3. Computational Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhao, J.; Liu, H.; Yu, Z.; Quhe, R.; Zhou, S.; Wang, Y.; Liu, C.C.; Zhong, H.; Han, N.; Lu, J.; et al. Rise of silicene: A competitive 2D material. Prog. Mater. Sci. 2016, 83, 24–151. [Google Scholar] [CrossRef]
- Ngo, T.D.; Huynh, T.; Jung, H.; Ali, F.; Jeon, J.; Choi, M.S.; Yoo, W.J. Modulation of Contact Resistance of Dual-Gated MoS(2) FETs Using Fermi-Level Pinning-Free Antimony Semi-Metal Contacts. Adv. Sci. 2023, 10, e2301400. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Zhang, J.; Ye, S.; Song, J.; Qu, J. Progress Report on Property, Preparation, and Application of Bi2O2Se. Adv. Funct. Mater. 2020, 30, 2004480. [Google Scholar] [CrossRef]
- Li, L.; Yu, Y.; Ye, G.J.; Ge, Q.; Ou, X.; Wu, H.; Feng, D.; Chen, X.H.; Zhang, Y. Black phosphorus field-effect transistors. Nat. Nanotechnol. 2014, 9, 372–377. [Google Scholar] [CrossRef] [PubMed]
- Reich, E.S. Phosphorene excites materials scientists. Nature 2014, 509, 19. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Wang, J.; Zheng, X.; Zhang, K.; Liu, X. Two dimensional monolayers TetraHex-CX(2) (X = N, P, As, and Sb) with superior electronic, mechanical and optical properties. Phys. Chem. Chem. Phys. 2022, 24, 29601–29608. [Google Scholar] [CrossRef] [PubMed]
- Wei, W.; Yang, S.; Wang, G.; Zhang, T.; Pan, W.; Cai, Z.; Yang, Y.; Zheng, L.; He, P.; Wang, L.; et al. Bandgap engineering of two-dimensional C3N bilayers. Nat. Electron. 2021, 4, 486–494. [Google Scholar] [CrossRef]
- Dong, S.; Hu, Z.Y.; Wei, P.; Han, J.; Wang, Z.; Liu, J.; Su, B.L.; Zhao, D.; Liu, Y. All-Inorganic Perovskite Single-Crystal Photoelectric Anisotropy. Adv. Mater. 2022, 34, e2204342. [Google Scholar] [CrossRef]
- Fang, Y.; Wang, F.; Wang, R.; Zhai, T.; Huang, F. 2D NbOI(2): A Chiral Semiconductor with Highly In-Plane Anisotropic Electrical and Optical Properties. Adv. Mater. 2021, 33, e2101505. [Google Scholar] [CrossRef]
- Zeng, H.; Chen, R.S.; Yao, G. Tunable Electronic Properties and Potential Applications of 2D GeP/Graphene van der Waals Heterostructure. Adv. Electron. Mater. 2020, 6, 1901024. [Google Scholar] [CrossRef]
- Zhang, J.; Shang, C.; Dai, X.; Zhang, Y.; Zhu, T.; Zhou, N.; Xu, H.; Yang, R.; Li, X. Effective Passivation of Anisotropic 2D GeAs via Graphene Encapsulation for Highly Stable Near-Infrared Photodetectors. ACS Appl. Mater. Interfaces 2023, 15, 13281–13289. [Google Scholar] [CrossRef]
- Barreteau, C.; Michon, B.; Besnard, C.; Giannini, E. High-pressure melt growth and transport properties of SiP, SiAs, GeP, and GeAs 2D layered semiconductors. J. Cryst. Growth 2016, 443, 75–80. [Google Scholar] [CrossRef]
- Jung, C.S.; Kim, D.; Cha, S.; Myung, Y.; Shojaei, F.; Abbas, H.G.; Lee, J.A.; Cha, E.H.; Park, J.; Kang, H.S. Two-dimensional GeAs with a visible range band gap. J. Mater. Chem. A 2018, 6, 9089–9098. [Google Scholar] [CrossRef]
- Zhou, Z.; Long, M.; Pan, L.; Wang, X.; Zhong, M.; Blei, M.; Wang, J.; Fang, J.; Tongay, S.; Hu, W.; et al. Perpendicular Optical Reversal of the Linear Dichroism and Polarized Photodetection in 2D GeAs. ACS Nano 2018, 12, 12416–12423. [Google Scholar] [CrossRef] [PubMed]
- Dushaq, G.; Villegas, J.E.; Paredes, B.; Tamalampudi, S.R.; Rasras, M.S. Anisotropic Van Der Waals 2D GeAs Integrated on Silicon Four-Waveguide Crossing. J. Light. Technol. 2023, 41, 1784–1789. [Google Scholar] [CrossRef]
- Hou, S.; Guo, Z.; Xiong, T.; Wang, X.; Yang, J.; Liu, Y.-Y.; Niu, Z.-C.; Liu, S.; Liu, B.; Zhai, S.; et al. Optical and electronic anisotropy of a 2D semiconductor SiP. Nano Res. 2022, 15, 8579–8586. [Google Scholar] [CrossRef]
- Dai, X.; Qin, F.; Qiu, C.; Zhou, L.; Huang, J.; Cheng, F.; Bi, X.; Zhang, C.; Li, Z.; Tang, M.; et al. Selective substitution induced anomalous phonon stiffening within quasi-one-dimensional P—P chains in SiP2. Nano Res. 2022, 16, 1107–1114. [Google Scholar] [CrossRef]
- Kim, J.H.; Han, G.H.; Moon, B.H. GeAs as an emerging p-type van der Waals semiconductor and its application in p-n photodiodes. Nanotechnology 2023, 34, 315201. [Google Scholar] [CrossRef]
- Zhang, J.; Duan, L.; Zhou, N.; Zhang, L.; Shang, C.; Xu, H.; Yang, R.; Wang, X.; Li, X. Modulating the Function of GeAs/ReS(2) van der Waals Heterojunction with its Potential Application for Short-Wave Infrared and Polarization-Sensitive Photodetection. Small 2023, 19, e2303335. [Google Scholar] [CrossRef]
- Wang, Z.; Luo, P.; Han, B.; Zhang, X.; Zhao, S.; Wang, S.; Chen, X.; Wei, L.; Yang, S.; Zhou, X.; et al. Strong In-Plane Anisotropic SiP(2) as a IV-V 2D Semiconductor for Polarized Photodetection. ACS Nano 2021, 15, 20442–20452. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, S.; Sui, F.; Qi, R.; Dong, S.; Yang, P.; Yue, F. Abnormal vibrational anisotropy and thermal properties of a two-dimensional GeAs semiconductor. Phys. Chem. Chem. Phys. 2023, 25, 3745–3751. [Google Scholar] [CrossRef] [PubMed]
- Lin, W.; Liang, S.-D.; Li, J.; Yao, D.-X. Phonon dispersions and electronic structures of two-dimensional IV-V compounds. Carbon 2021, 172, 345–352. [Google Scholar] [CrossRef]
- Yang, S.; Liu, Y.; Wu, M.; Zhao, L.-D.; Lin, Z.; Cheng, H.-C.; Wang, Y.; Jiang, C.; Wei, S.-H.; Huang, L.; et al. Highly-anisotropic optical and electrical properties in layered SnSe. Nano Res. 2018, 11, 554–564. [Google Scholar] [CrossRef]
- Yang, H.; Jussila, H.; Autere, A.; Komsa, H.-P.; Ye, G.; Chen, X.; Hasan, T.; Sun, Z. Optical Waveplates Based on Birefringence of Anisotropic Two-Dimensional Layered Materials. ACS Photonics 2017, 4, 3023–3030. [Google Scholar] [CrossRef]
- Yang, S.; Yang, Y.; Wu, M.; Hu, C.; Shen, W.; Gong, Y.; Huang, L.; Jiang, C.; Zhang, Y.; Ajayan, P.M. Highly In-Plane Optical and Electrical Anisotropy of 2D Germanium Arsenide. Adv. Funct. Mater. 2018, 28, 1707379. [Google Scholar] [CrossRef]
- Guo, J.; Liu, Y.; Ma, Y.; Zhu, E.; Lee, S.; Lu, Z.; Zhao, Z.; Xu, C.; Lee, S.J.; Wu, H.; et al. Few-Layer GeAs Field-Effect Transistors and Infrared Photodetectors. Adv. Mater. 2018, 30, 1705934. [Google Scholar] [CrossRef] [PubMed]
- Zacharia, R.; Ulbricht, H.; Hertel, T. Interlayer cohesive energy of graphite from thermal desorption of polyaromatic hydrocarbons. Phys. Rev. B 2004, 69, 155406. [Google Scholar] [CrossRef]
- Ziambaras, E.; Kleis, J.; Schröder, E.; Hyldgaard, P. Potassium intercalation in graphite: A van der Waals density-functional study. Phys. Rev. B 2007, 76, 155425. [Google Scholar] [CrossRef]
- Cheng, A.Q.; He, Z.; Zhao, J.; Zeng, H.; Chen, R.S. Monolayered Silicon and Germanium Monopnictide Semiconductors: Excellent Stability, High Absorbance, and Strain Engineering of Electronic Properties. ACS Appl. Mater. Interfaces 2018, 10, 5133–5139. [Google Scholar] [CrossRef]
- Zhou, L.; Guo, Y.; Zhao, J. GeAs and SiAs monolayers: Novel 2D semiconductors with suitable band structures. Physica E 2018, 95, 149–153. [Google Scholar] [CrossRef]
- Lee, K.; Kamali, S.; Ericsson, T.; Bellard, M.; Kovnir, K. GeAs: Highly Anisotropic van der Waals Thermoelectric Material. Chem. Mater. 2016, 28, 2776–2785. [Google Scholar] [CrossRef]
- Xia, F.; Wang, H.; Jia, Y. Rediscovering black phosphorus as an anisotropic layered material for optoelectronics and electronics. Nat. Commun. 2014, 5, 4458. [Google Scholar] [CrossRef] [PubMed]
- Liu, E.; Fu, Y.; Wang, Y.; Feng, Y.; Liu, H.; Wan, X.; Zhou, W.; Wang, B.; Shao, L.; Ho, C.-H.; et al. Integrated digital inverters based on two-dimensional anisotropic ReS2 field-effect transistors. Nat. Commun. 2015, 6, 6991. [Google Scholar] [CrossRef] [PubMed]
- Dushaq, G.; Rasras, M. Planar Multilayered 2D GeAs Schottky Photodiode for High-Performance Visible-Near-Infrared Photodetection. ACS Appl. Mater. Interfaces 2021, 13, 21499–21506. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.; Zeng, H.; Wang, D.; Yao, G. Electron transport properties of 2D IV-V semiconductors and their improvement by graphene contact. Appl. Surf. Sci. 2020, 519, 146203. [Google Scholar] [CrossRef]
- Chuang, H.J.; Chamlagain, B.; Koehler, M.; Perera, M.M.; Yan, J.; Mandrus, D.; Tomanek, D.; Zhou, Z. Low-Resistance 2D/2D Ohmic Contacts: A Universal Approach to High-Performance WSe2, MoS2, and MoSe2 Transistors. Nano Lett. 2016, 16, 1896–1902. [Google Scholar] [CrossRef]
- Liu, Y.; Xiao, H.; Goddard, W.A., 3rd. Schottky-Barrier-Free Contacts with Two-Dimensional Semiconductors by Surface-Engineered MXenes. J. Am. Chem. Soc. 2016, 138, 15853–15856. [Google Scholar] [CrossRef]
- Takahashi, T.; Tokailin, H.; Sagawa, T. Angle-resolved ultraviolet photoelectron spectroscopy of the unoccupied band structure of graphite. Phys. Rev. B 1985, 32, 8317–8324. [Google Scholar] [CrossRef]
- Muhibbullah, M.; Ikuma, Y. Refutation of the short report “On the impossibility of Photoelectron ejection by electromagnetic wave”. Optik 2020, 202, 163734. [Google Scholar] [CrossRef]
- Ashcroft, N.W.; Mermin, N.D. Solid State Physics; Saunders College Publishing: Philadelphia, PA, USA, 1988. [Google Scholar]
- Li, L.; Wang, W.; Gong, P.; Zhu, X.; Deng, B.; Shi, X.; Gao, G.; Li, H.; Zhai, T. 2D GeP: An Unexploited Low-Symmetry Semiconductor with Strong In-Plane Anisotropy. Adv. Mater. 2018, 30, e1706771. [Google Scholar]
- Pan, Y.; Wang, Y.; Ye, M.; Quhe, R.; Zhong, H.; Song, Z.; Peng, X.; Yu, D.; Yang, J.; Shi, J.; et al. Monolayer Phosphorene–Metal Contacts. Chem. Mater. 2016, 28, 2100–2109. [Google Scholar] [CrossRef]
- Lee, G.-H.; Kim, S.; Jhi, S.-H.; Lee, H.-J. Ultimately short ballistic vertical graphene Josephson junctions. Nat. Commun. 2015, 6, 6181. [Google Scholar] [CrossRef]
- Dong, M.M.; Zhang, G.P.; Li, Z.L.; Wang, M.L.; Wang, C.K.; Fu, X.X. Anisotropic interfacial properties of monolayer C(2)N field effect transistors. Phys. Chem. Chem. Phys. 2020, 22, 28074–28085. [Google Scholar] [CrossRef]
- Pan, Y.; Dan, Y.; Wang, Y.; Ye, M.; Zhang, H.; Quhe, R.; Zhang, X.; Li, J.; Guo, W.; Yang, L.; et al. Schottky Barriers in Bilayer Phosphorene Transistors. ACS Appl. Mater. Interfaces 2017, 9, 12694–12705. [Google Scholar] [CrossRef]
- Pan, Y.; Dai, J.; Liu, Z.; Wu, M.; Hu, H.; Lu, J. Ohmic contacts of monolayer Tl2O field-effect transistors. J. Mater. Sci. 2020, 55, 11439–11450. [Google Scholar] [CrossRef]
- Yan, J.; Zhang, X.; Pan, Y.; Li, J.; Shi, B.; Liu, S.; Yang, J.; Song, Z.; Zhang, H.; Ye, M.; et al. Monolayer tellurene–metal contacts. J. Mater. Chem. C 2018, 6, 6153–6163. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, S.; Li, Q.; Quhe, R.; Yang, C.; Guo, Y.; Zhang, X.; Pan, Y.; Li, J.; Zhang, H.; et al. Schottky barrier heights in two-dimensional field-effect transistors: From theory to experiment. Rep. Prog. Phys. 2021, 84, 056501. [Google Scholar] [CrossRef]
- Shu, Y.; Liu, Y.; Cui, Z.; Xiong, R.; Zhang, Y.; Xu, C.; Zheng, J.; Wen, C.; Wu, B.; Sa, B. Efficient Ohmic Contact in Monolayer CrX2N4 (X = C, Si) Based Field-Effect Transistors. Adv. Electron. Mater. 2023, 9, 2201056. [Google Scholar] [CrossRef]
- Liu, X.; Choi, M.S.; Hwang, E.; Yoo, W.J.; Sun, J. Fermi Level Pinning Dependent 2D Semiconductor Devices: Challenges and Prospects. Adv. Mater. 2022, 34, 2108425. [Google Scholar] [CrossRef]
- Kang, J.; Liu, W.; Sarkar, D.; Jena, D.; Banerjee, K. Computational Study of Metal Contacts to Monolayer Transition-Metal Dichalcogenide Semiconductors. Phys. Rev. X 2014, 4, 031005. [Google Scholar] [CrossRef]
- Kim, C.; Moon, I.; Lee, D.; Choi, M.S.; Ahmed, F.; Nam, S.; Cho, Y.; Shin, H.J.; Park, S.; Yoo, W.J. Fermi Level Pinning at Electrical Metal Contacts of Monolayer Molybdenum Dichalcogenides. ACS Nano 2017, 11, 1588–1596. [Google Scholar] [CrossRef]
- Liu, Y.; Weiss, N.O.; Duan, X.; Cheng, H.-C.; Huang, Y.; Duan, X. Van der Waals heterostructures and devices. Nat. Rev. Mater. 2016, 1, 16042. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558–561. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Atomistix ToolKit, version 2019; QuantumWise a/s: Copenhagen, Denmark, 2023.
- Taylor, J.; Guo, H.; Wang, J. Ab initio modeling of quantum transport properties of molecular electronic devices. Phys. Rev. B 2001, 63, 245407. [Google Scholar] [CrossRef]
- Brandbyge, M.; Mozos, J.-L.; Ordejón, P.; Taylor, J.; Stokbro, K. Density-functional method for nonequilibrium electron transport. Phys. Rev. B 2002, 65, 165401. [Google Scholar] [CrossRef]
- Smidstrup, S.; Markussen, T.; Vancraeyveld, P.; Wellendorff, J.; Schneider, J.; Gunst, T.; Verstichel, B.; Stradi, D.; Khomyakov, P.A.; Vej-Hansen, U.G.; et al. QuantumATK: An integrated platform of electronic and atomic-scale modelling tools. J. Phys. Condens. Matter 2020, 32, 015901. [Google Scholar] [CrossRef]
- Zhang, X.; Pan, Y.; Ye, M.; Quhe, R.; Wang, Y.; Guo, Y.; Zhang, H.; Dan, Y.; Song, Z.; Li, J.; et al. Three-layer phosphorene-metal interfaces. Nano Res. 2017, 11, 707–721. [Google Scholar] [CrossRef]
- Das, S.; Zhang, W.; Demarteau, M.; Hoffmann, A.; Dubey, M.; Roelofs, A. Tunable Transport Gap in Phosphorene. Nano Lett. 2014, 14, 5733–5739. [Google Scholar] [CrossRef]

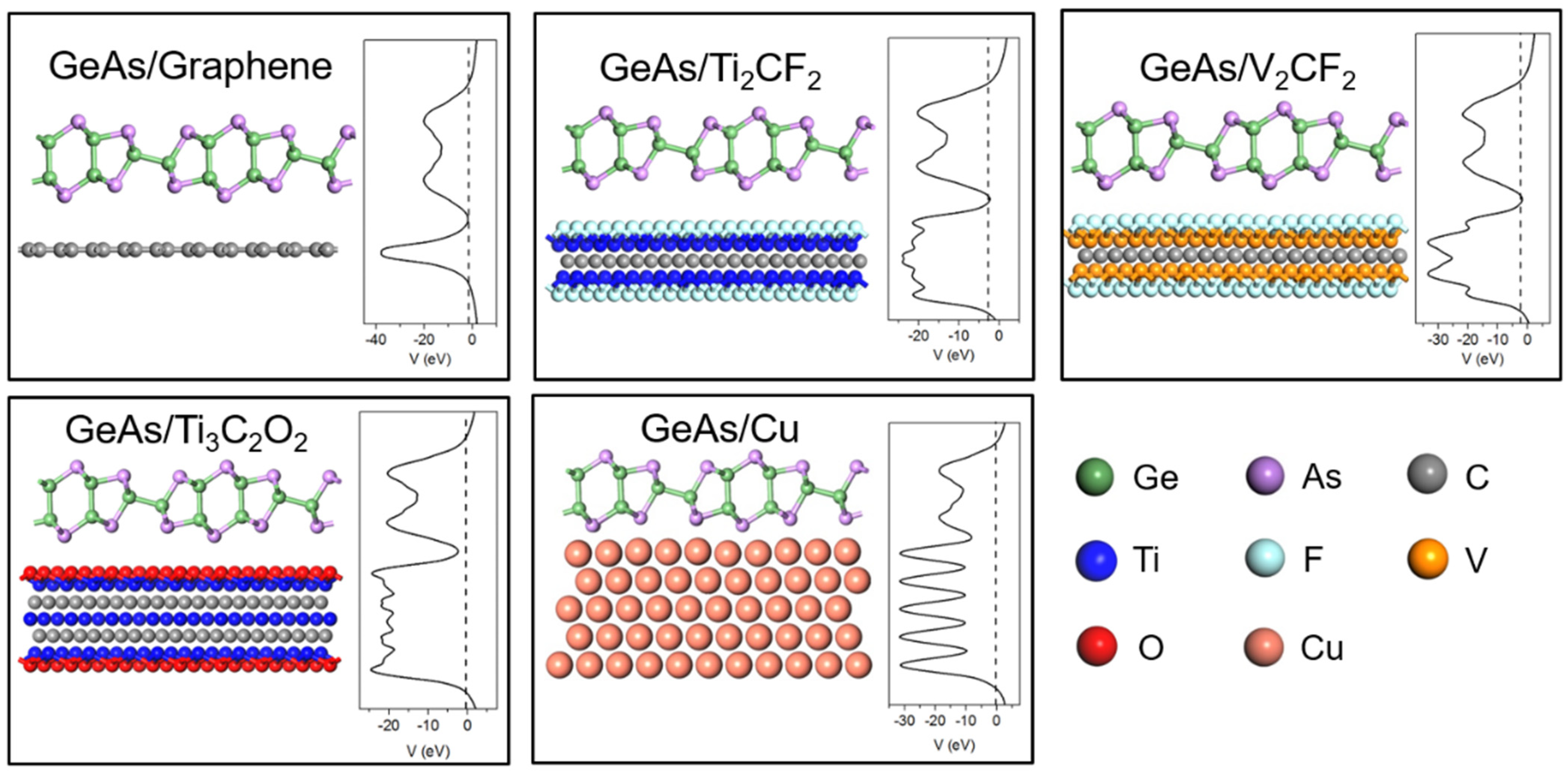
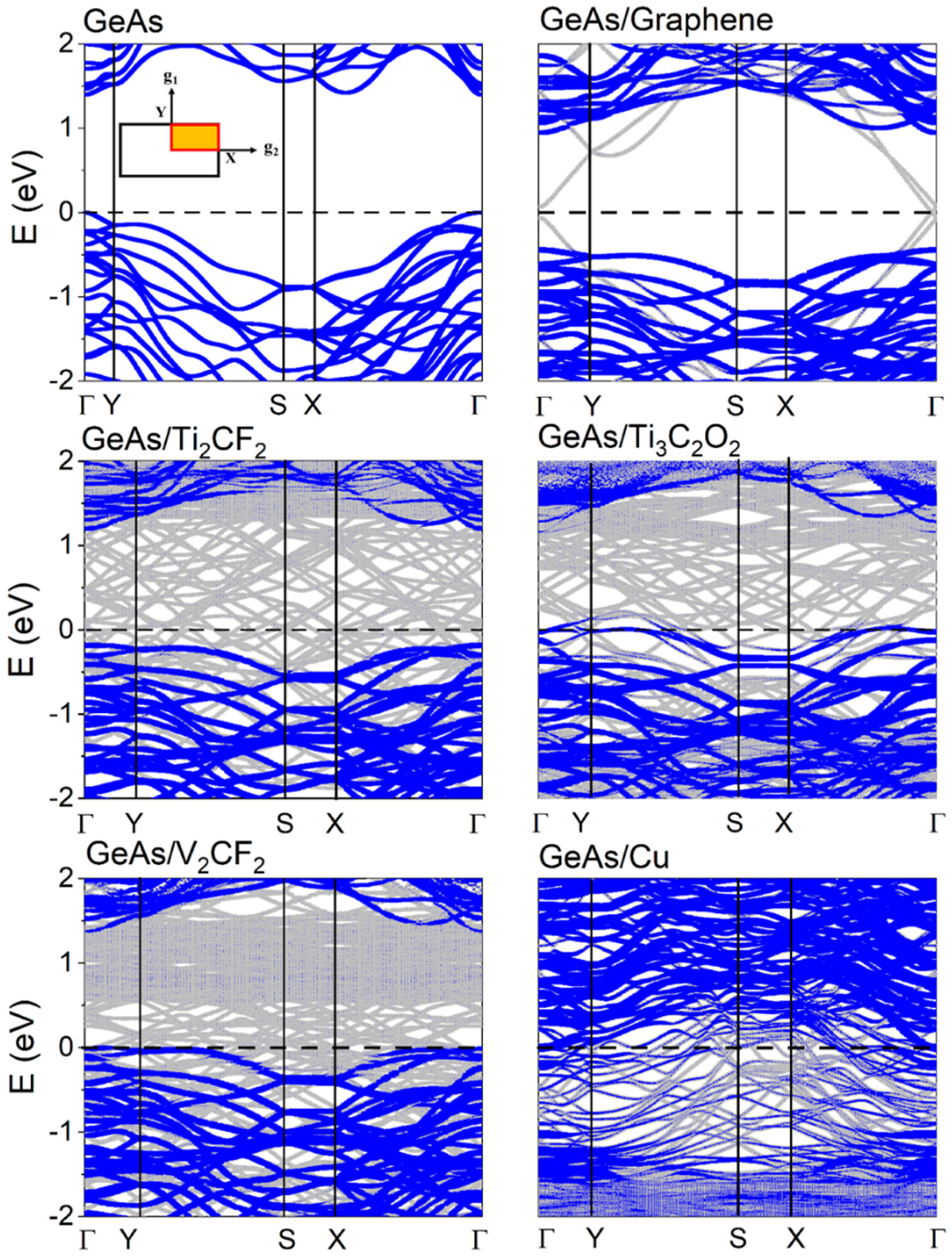
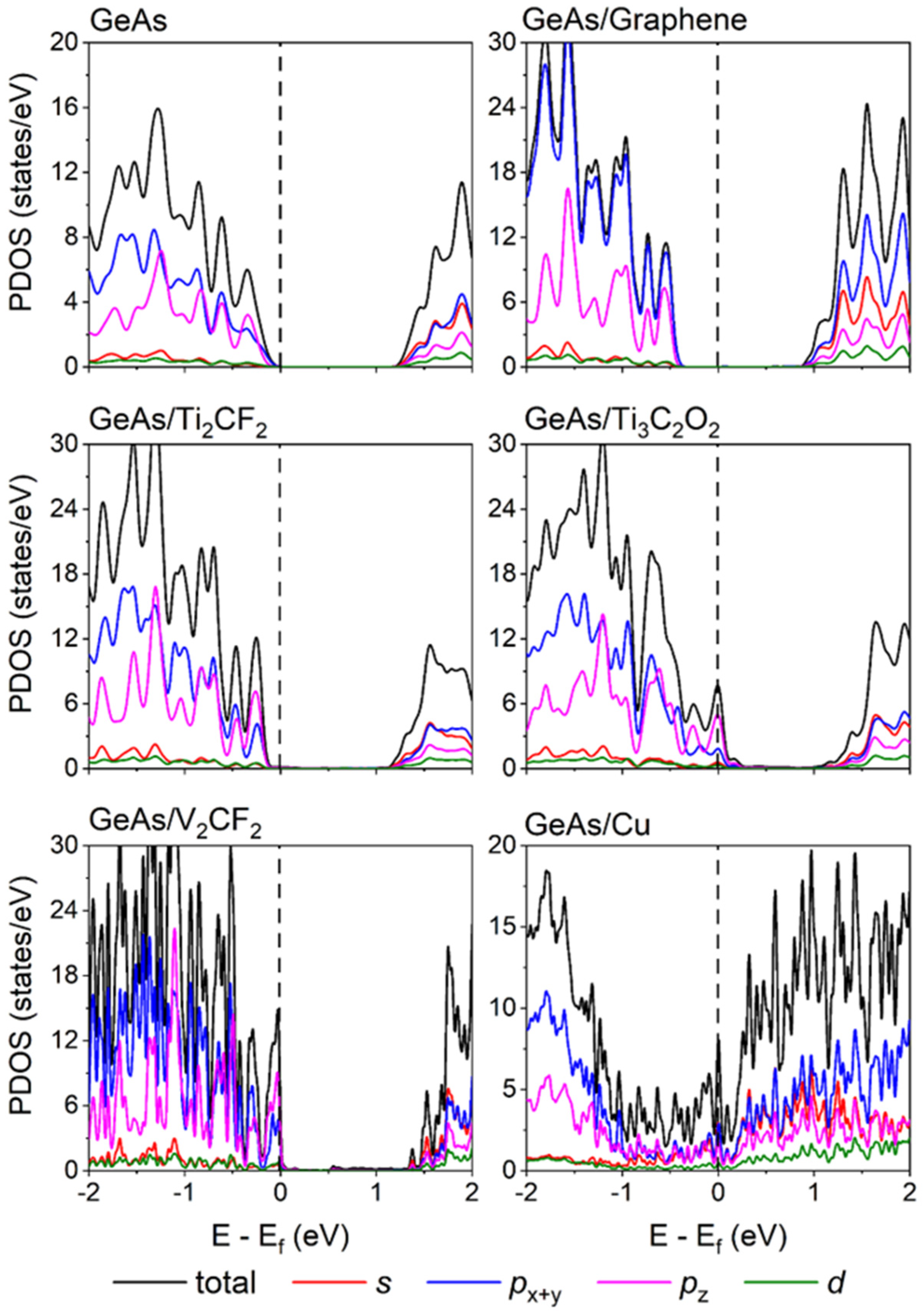
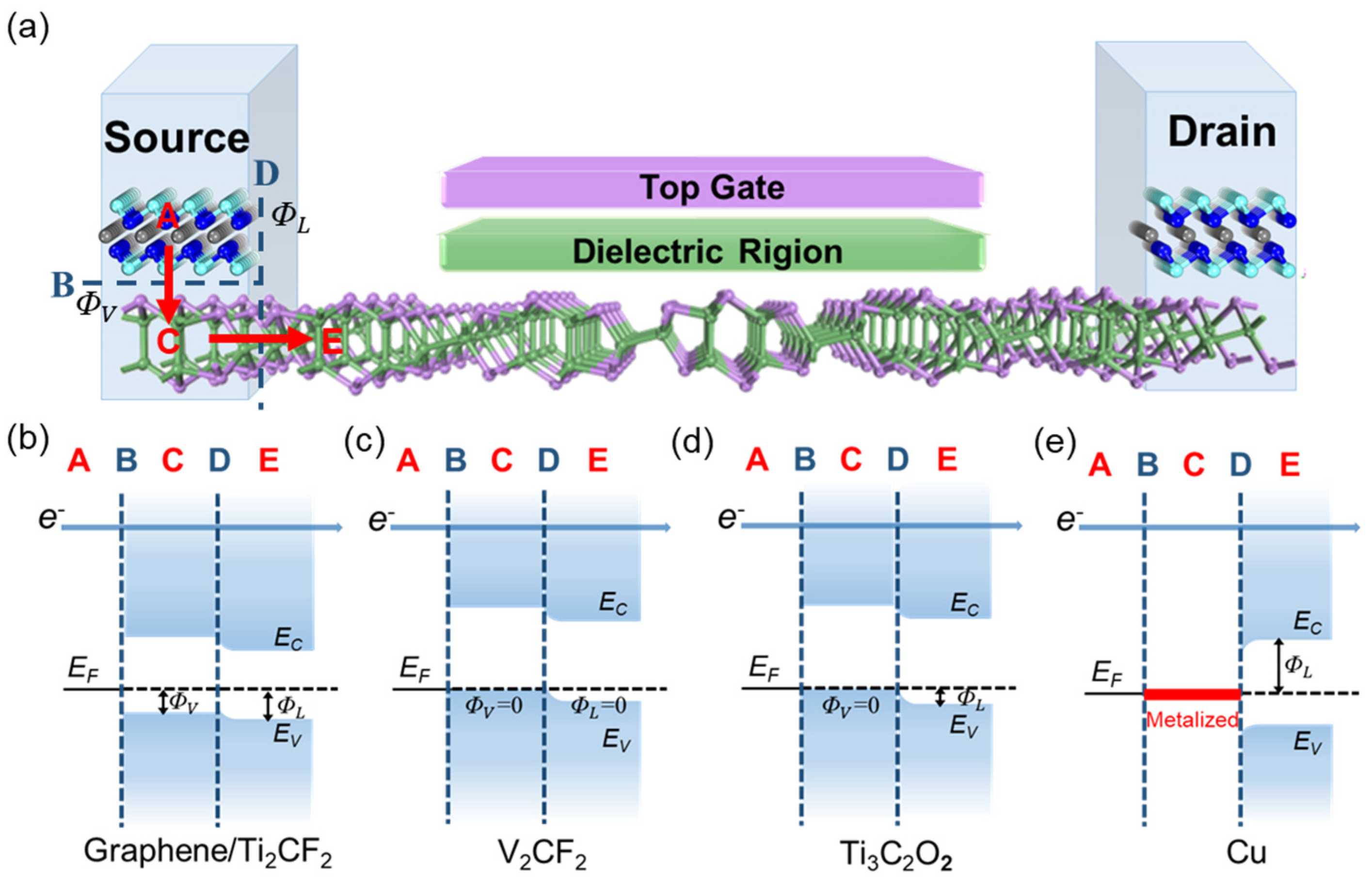


| Graphene | Ti2CF2 | Ti3C2O2 | V2CF2 | Cu | |
|---|---|---|---|---|---|
| (%) | 2.36 | 3.04 | 3.18 | 3.09 | 0.32 |
| dz (Å) | 3.28 | 2.67 | 2.58 | 2.63 | 2.04 |
| Eb (eV/atom) | 0.39 | 0.99 | 0.91 | 0.97 | 2.96 |
| W (eV) | 4.70 | 4.96 | 5.60 | 5.26 | 4.54 |
| Wm (eV) | 4.36 | 4.92 | 5.93 | 5.26 | 4.66 |
| (eV) | 0.33 | 0.15 | 0 | 0 | 0 |
| (eV) | 1.52 | 1.18 | 1.18 | 1.42 | 0.57 |
| (eV) | 0.11 | 0.21 | 0.20 | 0.00 | 0.72 |
| (eV) | 1.49 | 1.32 | 1.30 | 1.42 | 0.64 |
| (eV) | 0.15 | 0.29 | 0.12 | 0.00 | 0.96 |
| (Å) | 68.1 | 67.1 | 67.1 | 67.1 | 66.8 |
| (Å) | 54.7 | 53.7 | 53.8 | 53.6 | 54.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, W.; Liu, H.; Zou, F.; Niu, Y.; Zhao, Y.; Cong, Y.; Pan, Y.; Li, Q. Isotropic Contact Properties in Monolayer GeAs Field-Effect Transistors. Molecules 2023, 28, 7806. https://doi.org/10.3390/molecules28237806
Song W, Liu H, Zou F, Niu Y, Zhao Y, Cong Y, Pan Y, Li Q. Isotropic Contact Properties in Monolayer GeAs Field-Effect Transistors. Molecules. 2023; 28(23):7806. https://doi.org/10.3390/molecules28237806
Chicago/Turabian StyleSong, Weiqi, Haosong Liu, Feihu Zou, Yize Niu, Yue Zhao, Yao Cong, Yuanyuan Pan, and Qiang Li. 2023. "Isotropic Contact Properties in Monolayer GeAs Field-Effect Transistors" Molecules 28, no. 23: 7806. https://doi.org/10.3390/molecules28237806
APA StyleSong, W., Liu, H., Zou, F., Niu, Y., Zhao, Y., Cong, Y., Pan, Y., & Li, Q. (2023). Isotropic Contact Properties in Monolayer GeAs Field-Effect Transistors. Molecules, 28(23), 7806. https://doi.org/10.3390/molecules28237806








