Rotational Barriers in N-Benzhydrylformamides: An NMR and DFT Study
Abstract
1. Introduction
2. Results and Discussion
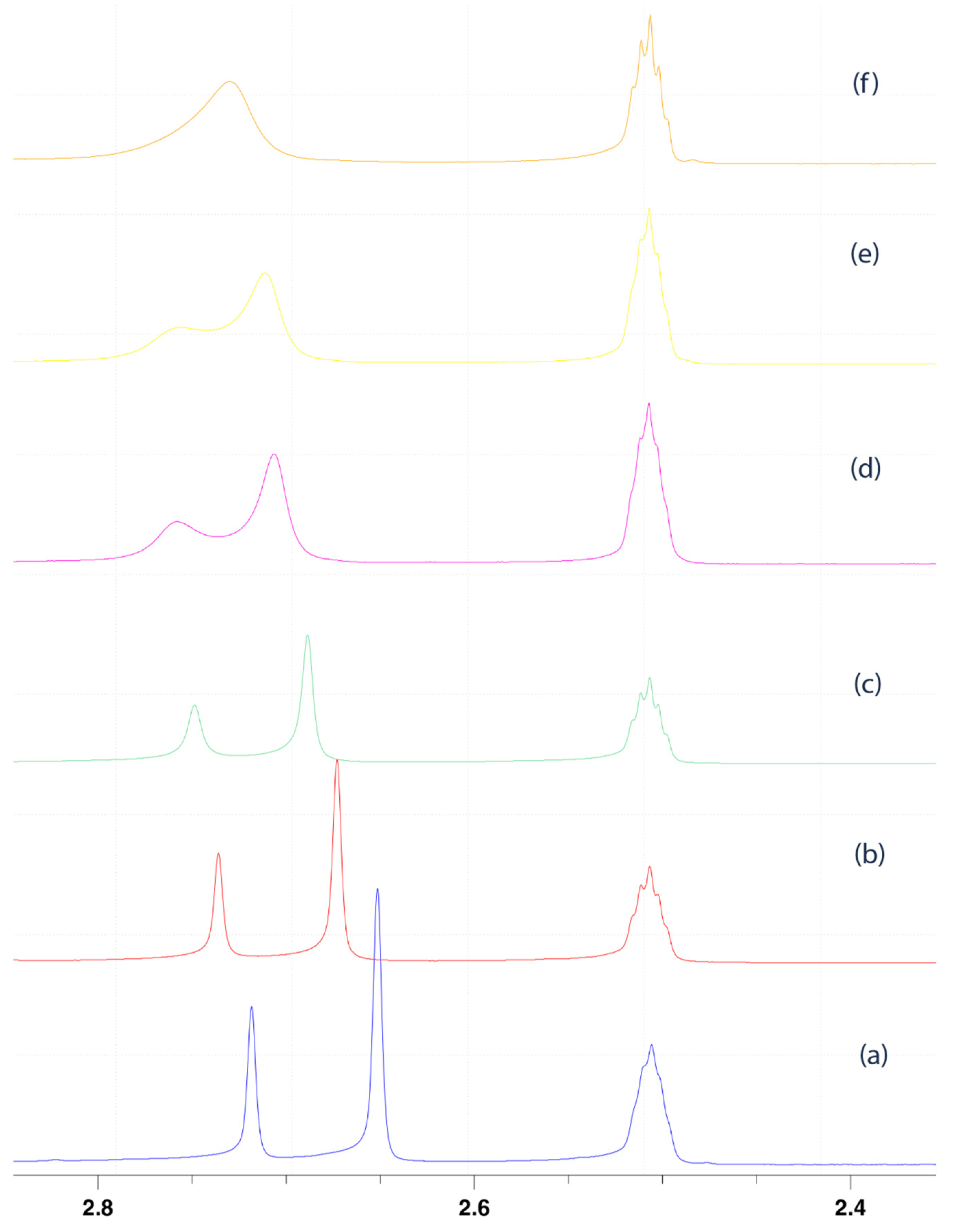
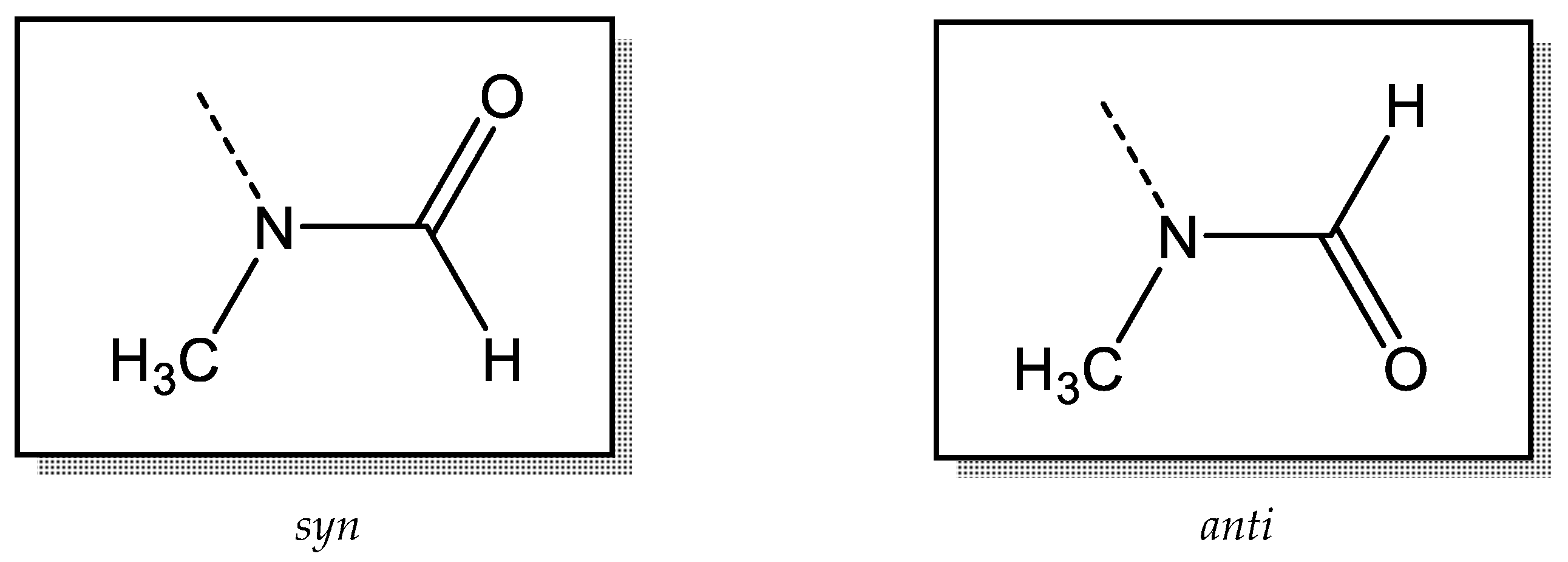
2.1. Investigation of the Internal Rotation by NMR
2.2. Calculation of the Internal Rotational Barriers by the DFT Method
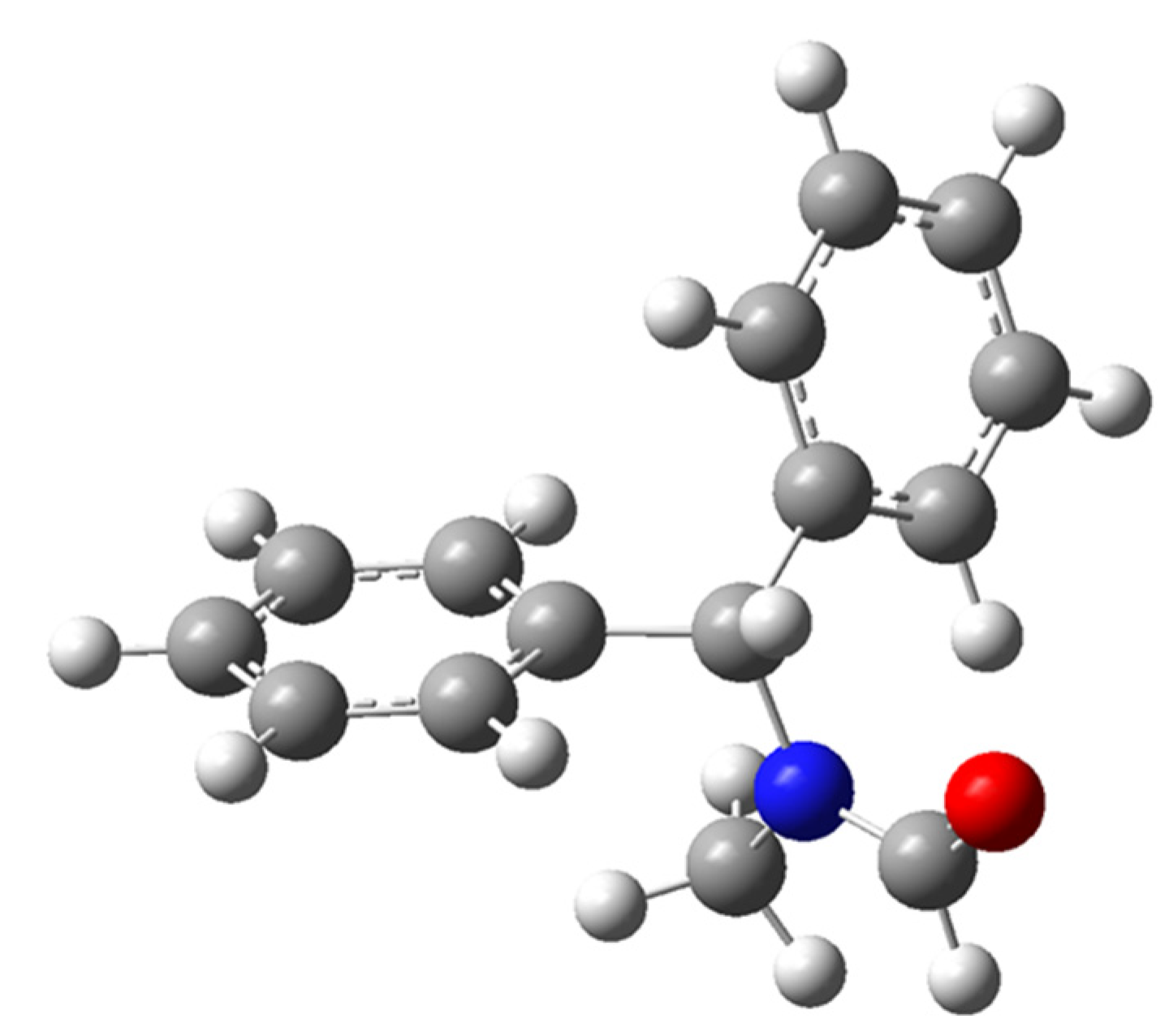
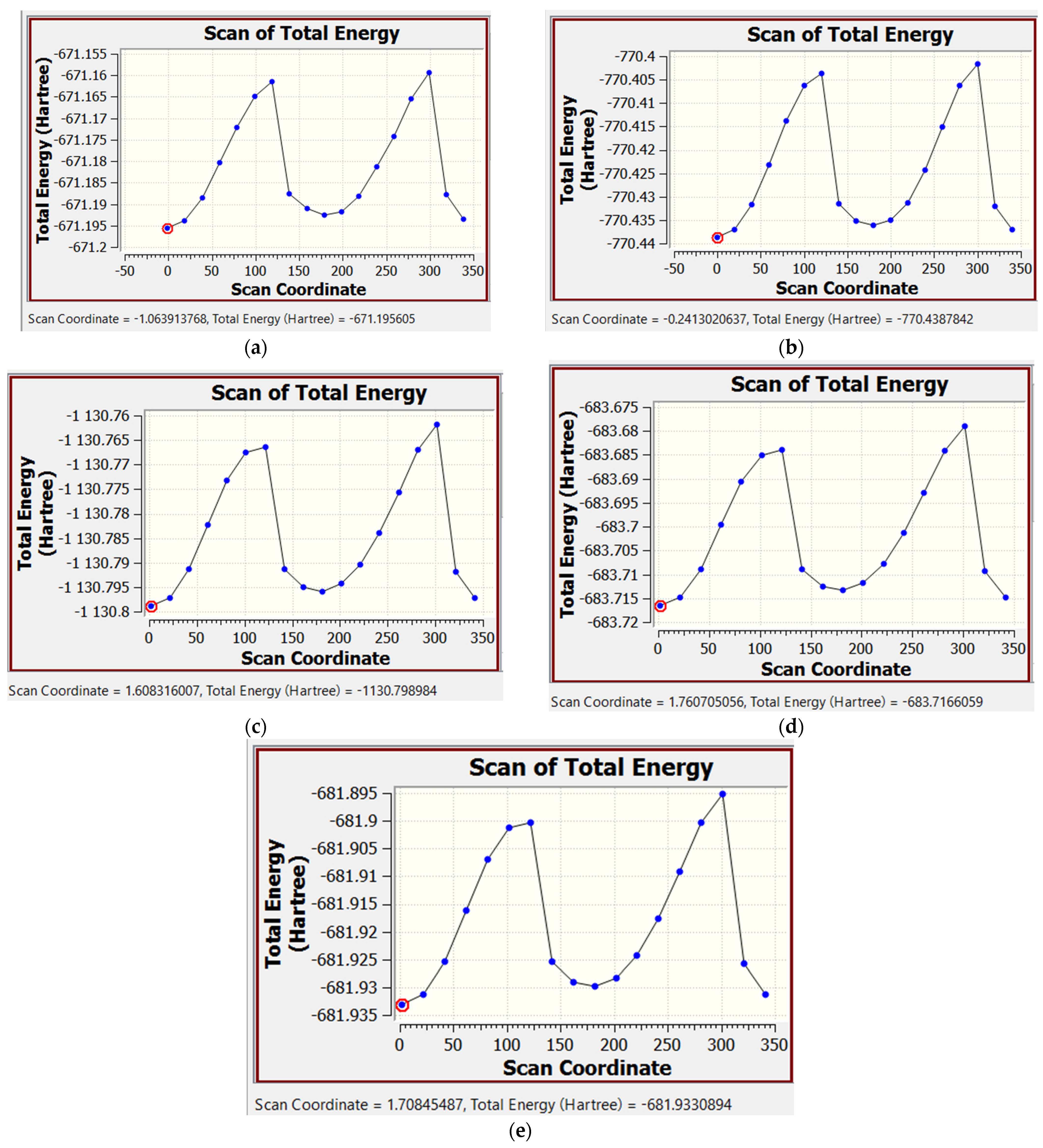
2.2.1. Calculation of the Rotational Barriers in N-Benzhydryl-N-Methylformamide
2.2.2. Calculation of Rotational Barriers in N-Benzhydrylformamide and Its ortho-Substituted Derivatives
3. Materials and Methods
3.1. The NMR Experiments
3.2. Quantum Chemical Calculations
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Librowski, T.; Kubacka, M.; Meusel, M.; Scolari, S.; Müller, C.E.; Gütschow, M. Evaluation of anticonvulsant and analgesic effects of benzyl- and benzhydryl ureides. Eur. J. Pharmacol. 2007, 559, 138–149. [Google Scholar] [CrossRef] [PubMed]
- Siddiqui, N.; Alam, M.S.; Stables, J.P. Synthesis and anticonvulsant properties of 1-(amino-N-arylmethanethio)-3-(1-substituted benzyl-2, 3-dioxoindolin-5-yl) urea derivatives. Eur. J. Med. Chem. 2011, 46, 2236–2242. [Google Scholar] [CrossRef] [PubMed]
- Krasnov, V.A.; Gorshkova, V.K.; Bakibaev, A.A.; Saratikov, A.S. Synthesis and anticonvulsive activity of fluorine-substituted benzhydrylamides. Khimiko-Farmatsevticheskii Zhurnal. 1997, 31, 368–369. [Google Scholar] [CrossRef]
- Roy, D.; Panda, G. Benzhydryl Amines: Synthesis and Their Biological Perspective. ACS Omega 2019. [Google Scholar] [CrossRef] [PubMed]
- Bakibaev, A.A.; Gorshkova, V.K.; Saratikov, A.S. Antihypoxic properties of organic compounds (a review). Khimiko-Farmatsevticheskii Zhurnal. 1997, 31, 3–16. [Google Scholar] [CrossRef]
- Hua, C.; Li, X.-G.; Xu, S.-M. N-Benzhydrylformamide. Acta Crystallogr. 2007, E63, o144–o145. [Google Scholar] [CrossRef]
- Bakibaev, A.A.; Yagovkin, A.Y.; Filimonov, V.D. Urea in organic synthesis. V. Reactions of aromatic ketones and 1,2-diketones with ureas in formic acid. Zhurnal. Organicheskoi Khimii 1991, 7, 1512–1520. [Google Scholar]
- Huggins, M.T.; Kesharwani, T.; Buttrick, J.; Nicholson, C. Variable Temperature NMR Experiment Studying Restricted Bond Rotation. J. Chem. Educ. 2020, 97, 1425–1429. [Google Scholar] [CrossRef]
- Kang, Y.K.; Park, H.S. Internal rotation about the C–N bond of amides. J. Mol. Struct. Theochem. 2004, 676, 171–176. [Google Scholar] [CrossRef]
- Quintanilla-Licea, R.; Colunga-Valladares, J.; Caballero-Quintero, A.; Rodríguez-Padilla, C.; Tamez-Guerra, R.; Gómez-Flores, R.; Waksman, N. NMR Detection of Isomers Arising from Restricted Rotation of the C-N Amide Bond of N-Formyl-o-toluidine and N,N′-bis-Formyl-o-tolidine. Molecules 2002, 7, 662–673. [Google Scholar] [CrossRef]
- Zhao, Y.; Truhlar, D.G. The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: Two new functionals and systematic testing of four M06-class functionals and 12 other functionals. Theor. Chem. Acc. 2008, 120, 215–241. [Google Scholar] [CrossRef]
- Mennucci, B.; Cancès, E.; Tomasi, J. Evaluation of Solvent Effects in Isotropic and Anisotropic Dielectrics and in Ionic Solutions with a Unified Integral Equation Method: Theoretical Bases, Computational Implementation, and Numerical Applications. J. Phys. Chem. B. 1997, 101, 10506–10517. [Google Scholar] [CrossRef]
- Yang, Y.; Weaver, M.N.; Merz, K.M. Assessment of the “6-31+G** + LANL2DZ” Mixed Basis Set Coupled with Density Functional Theory Methods and the Effective Core Potential: Prediction of Heats of Formation and Ionization Potentials for First-Row-Transition-Metal Complexes. J. Phys. Chem. A 2009, 113, 9843–9851. [Google Scholar] [CrossRef]
- Pauling, L. The Nature of the Chemical Bond and the Structure of Molecules and Crystals, 3rd ed.; Cornell University Press: Ithaca, NY, USA, 1960; 644p. [Google Scholar]
- van Lenthe, E.; Baerends, E.J.; Snijders, J.G. Relativistic regular two-component Hamiltonians. J. Chem. Phys. 1993, 99, 4597–4610. [Google Scholar] [CrossRef]
- Fogarasi, G.; Szalay, P.G. High-Level Electron Correlation Calculations on Formamide and the Resonance Model. J. Phys. Chem. A 1997, 101, 1400–1408. [Google Scholar] [CrossRef]
- Kemnitz, C.R.; Loewen, M.J. “Amide Resonance” Correlates with a Breadth of C−N Rotation Barriers. J. Am. Chem. Soc. 2007, 129, 2521–2528. [Google Scholar] [CrossRef]
- Otani, Y.; Liu, X.; Ohno, H.; Wang, S.; Zhai, L.; Su, A.; Kawahata, M.; Yamaguchi, K.; Ohwada, T. Amide nitrogen pyramidalization changes lactam amide spinning. Nat. Commun. 2019, 10, 461. [Google Scholar] [CrossRef]
- Mayo, S.L.; Olafson, B.D.; Goddard, W.A. DREIDING: A generic force field for molecular simulations. J. Phys. Chem. 1990, 94, 8897–8909. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Krishnan, R.; Binkley, J.S.; Seeger, R.; Pople, J.A. Self-consistent molecular orbital methods. XX. A basis set for correlated wave functions. J. Chem. Phys. 1980, 72, 650. [Google Scholar] [CrossRef]
- Spitznagel, G.W.; Clark, T.; Schleyer, P.R.; Hehre, W.J. An evaluation of the performance of diffuse function-augmented basis sets for second row elements, Na-Cl. J. Comput. Chem. 1987, 8, 1109–1116. [Google Scholar] [CrossRef]
- Wadt, W.R.; Hay, P.J. Ab initio effective core potentials for molecular calculations. Potentials for main group elements Na to Bi. J. Chem. Phys. 1985, 82, 284–298. [Google Scholar] [CrossRef]
- Keith, T.A.; Millam, J.M. GaussView, Version 6, Roy Dennington; Semichem Inc.: Shawnee Mission, KS, USA, 2016. [Google Scholar]
- Rolfes, J.D.; Neese, F.; Pantazis, D.A. All-electron scalar relativistic basis sets for the elements Rb–Xe. J. Comput. Chem. 2020. [Google Scholar] [CrossRef] [PubMed]
- Neese, F.; Wennmohs, F.; Becker, U.; Riplinger, C. The ORCA quantum chemistry program package. J. Chem. Phys. 2020, 152, 224108. [Google Scholar] [CrossRef] [PubMed]




| Compound | Nconf | |||
|---|---|---|---|---|
| BHFA | 42 | 21.50 | 2.49 | 1.40 |
| BHFA-oF | 54 | 20.11 | 5.81 | 1.40 |
| BHFA-oCl | 42 | 20.42 (20.57) | 8.68 (8.23) | 1.61 |
| BHFA-oBr | 45 | 20.36 (20.62) | 9.53 (8.68) | 1.69 |
| BHFA-oI | 47 | 21.40 (21.15) | 9.76 (9.19) | 1.67 |
| BHFA-NMe | 66 | 22.65 | 3.06 | 0.15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sadvakassova, M.Z.; Khlebnikov, A.I.; Bakibaev, A.A.; Kotelnikov, O.A.; Erkassov, R.S.; Yelubay, M.A.; Issabayeva, M.A. Rotational Barriers in N-Benzhydrylformamides: An NMR and DFT Study. Molecules 2023, 28, 535. https://doi.org/10.3390/molecules28020535
Sadvakassova MZ, Khlebnikov AI, Bakibaev AA, Kotelnikov OA, Erkassov RS, Yelubay MA, Issabayeva MA. Rotational Barriers in N-Benzhydrylformamides: An NMR and DFT Study. Molecules. 2023; 28(2):535. https://doi.org/10.3390/molecules28020535
Chicago/Turabian StyleSadvakassova, Madina Zh., Andrei I. Khlebnikov, Abdigali A. Bakibaev, Oleg A. Kotelnikov, Rakhmetulla Sh. Erkassov, Madeniyet A. Yelubay, and Manar A. Issabayeva. 2023. "Rotational Barriers in N-Benzhydrylformamides: An NMR and DFT Study" Molecules 28, no. 2: 535. https://doi.org/10.3390/molecules28020535
APA StyleSadvakassova, M. Z., Khlebnikov, A. I., Bakibaev, A. A., Kotelnikov, O. A., Erkassov, R. S., Yelubay, M. A., & Issabayeva, M. A. (2023). Rotational Barriers in N-Benzhydrylformamides: An NMR and DFT Study. Molecules, 28(2), 535. https://doi.org/10.3390/molecules28020535







