Molecular Dynamics Simulation of Hydrogels Based on Phosphorylcholine-Containing Copolymers for Soft Contact Lens Applications
Abstract
:1. Introduction
2. Results and Discussion
- block M13H37 (B13),
- block M37H13 (B37),
- random with 13 MPC and 37 HEMA mers,HMH6MH4MH4MH4MHMHMHMH11MHM2H2M2H (R13),
- random with 37 MPC and 13 HEMA mers,M10HM4H2M6H3M5H4MHM5HM3HM3 (R37),
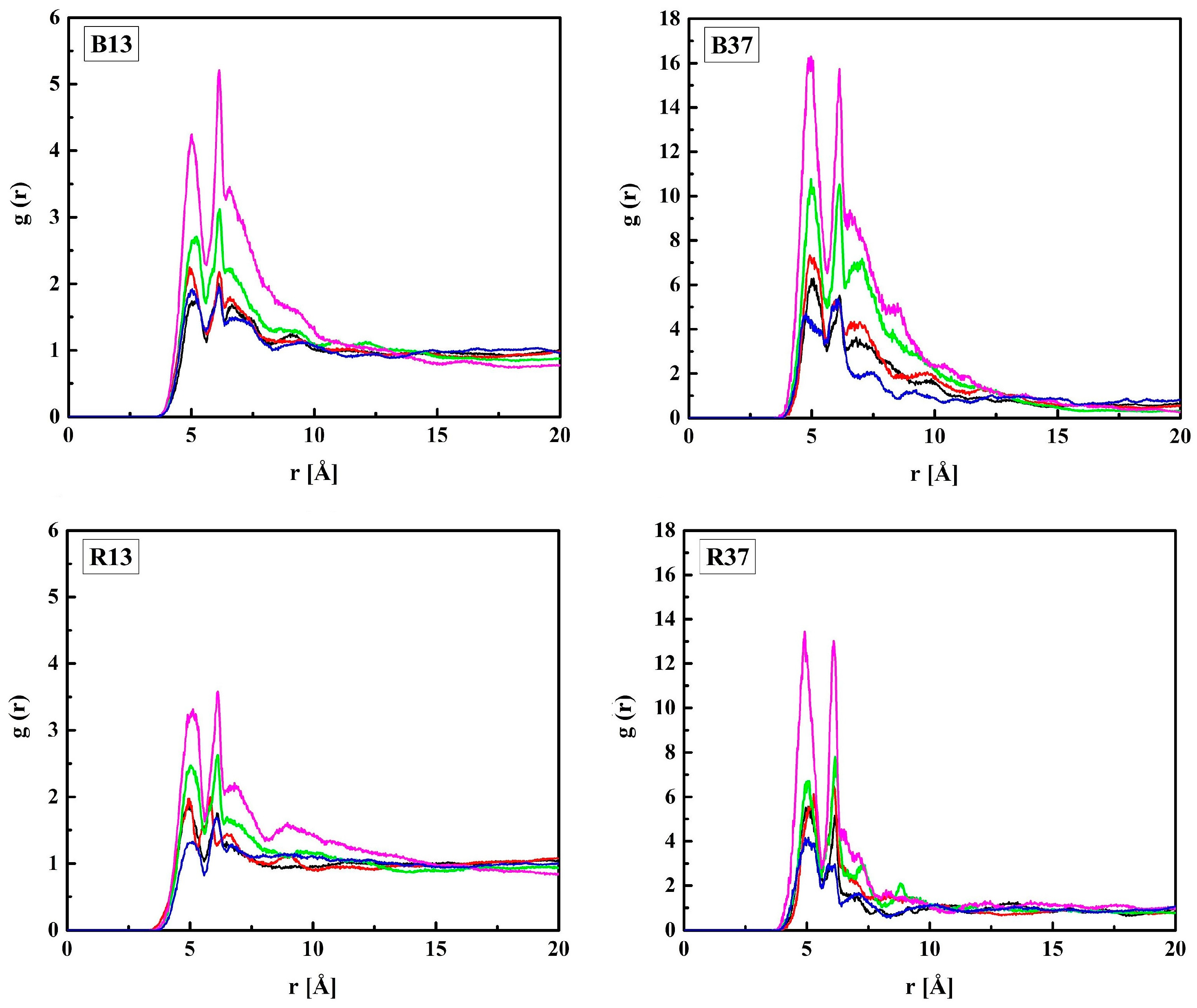
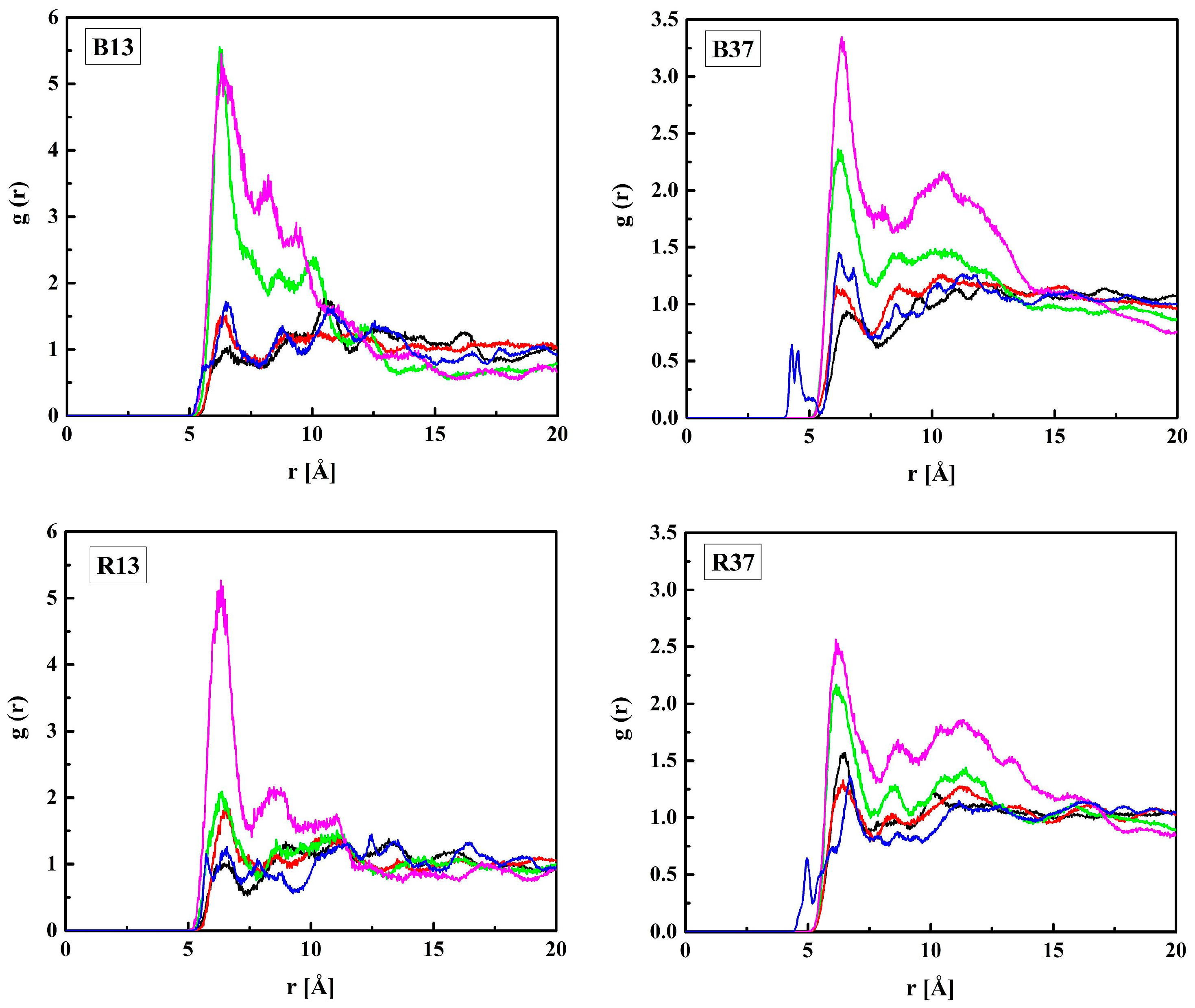
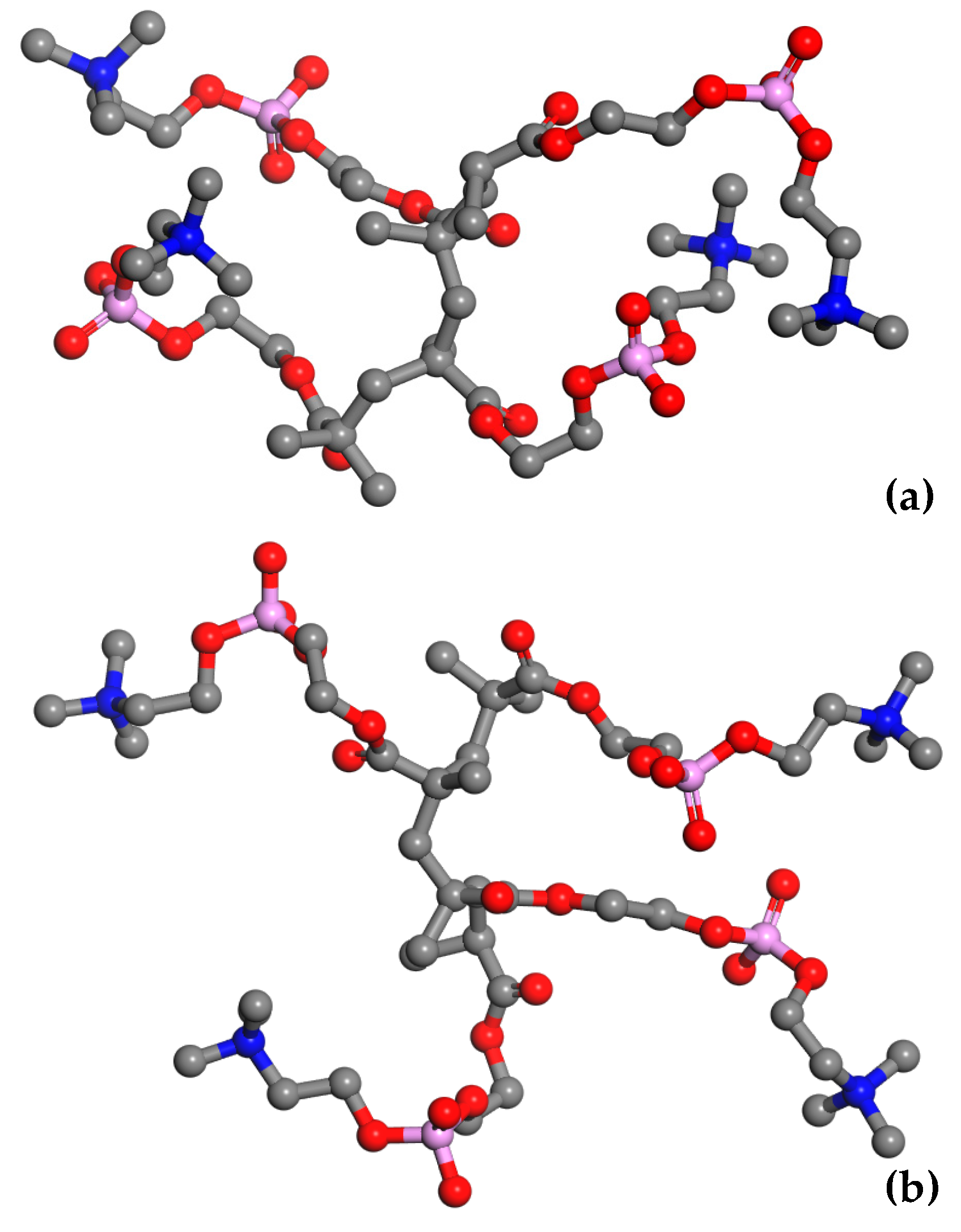
2.1. Structure and Chain Packing
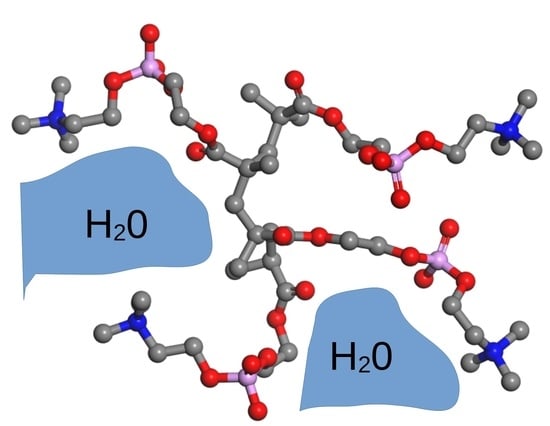
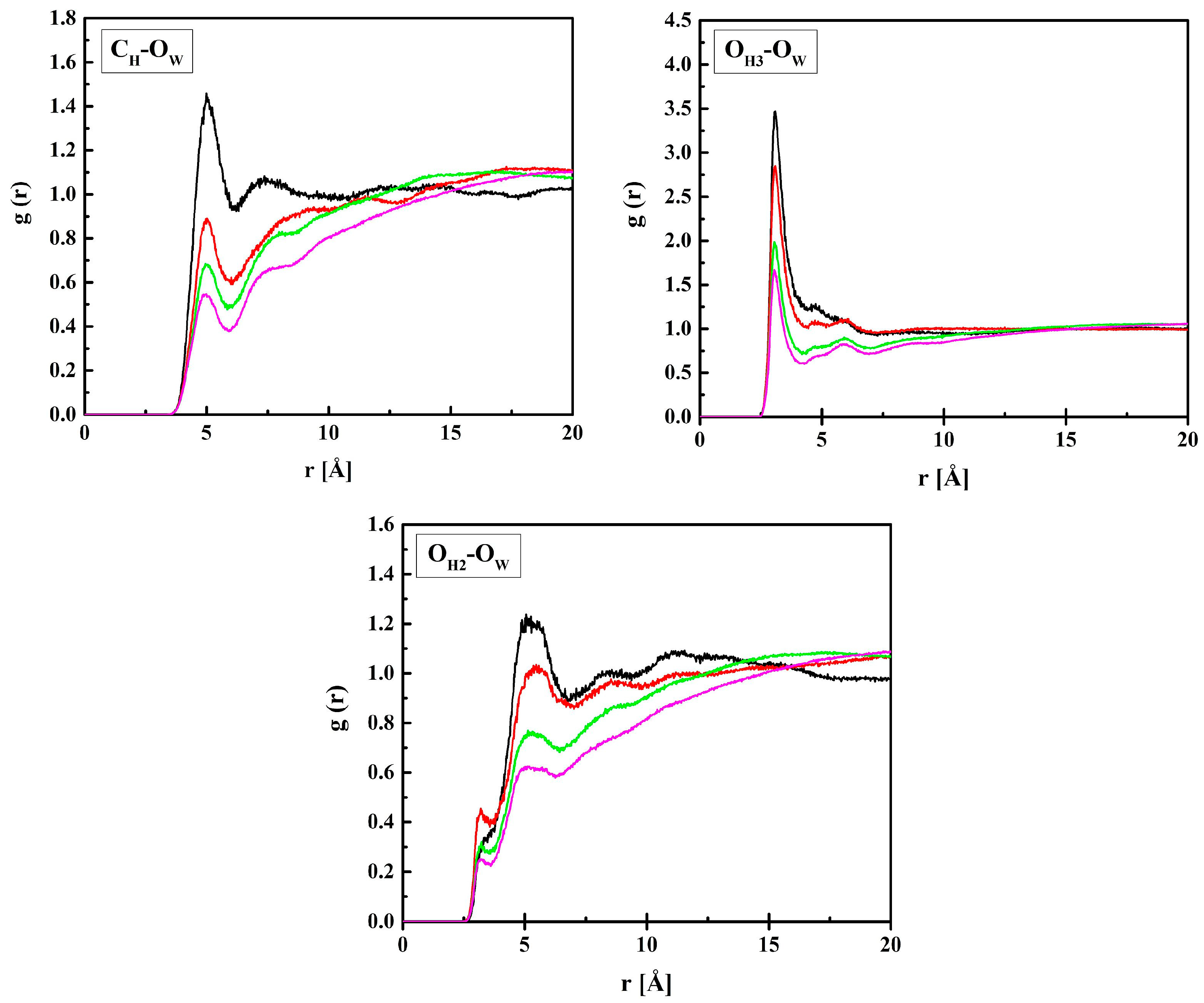
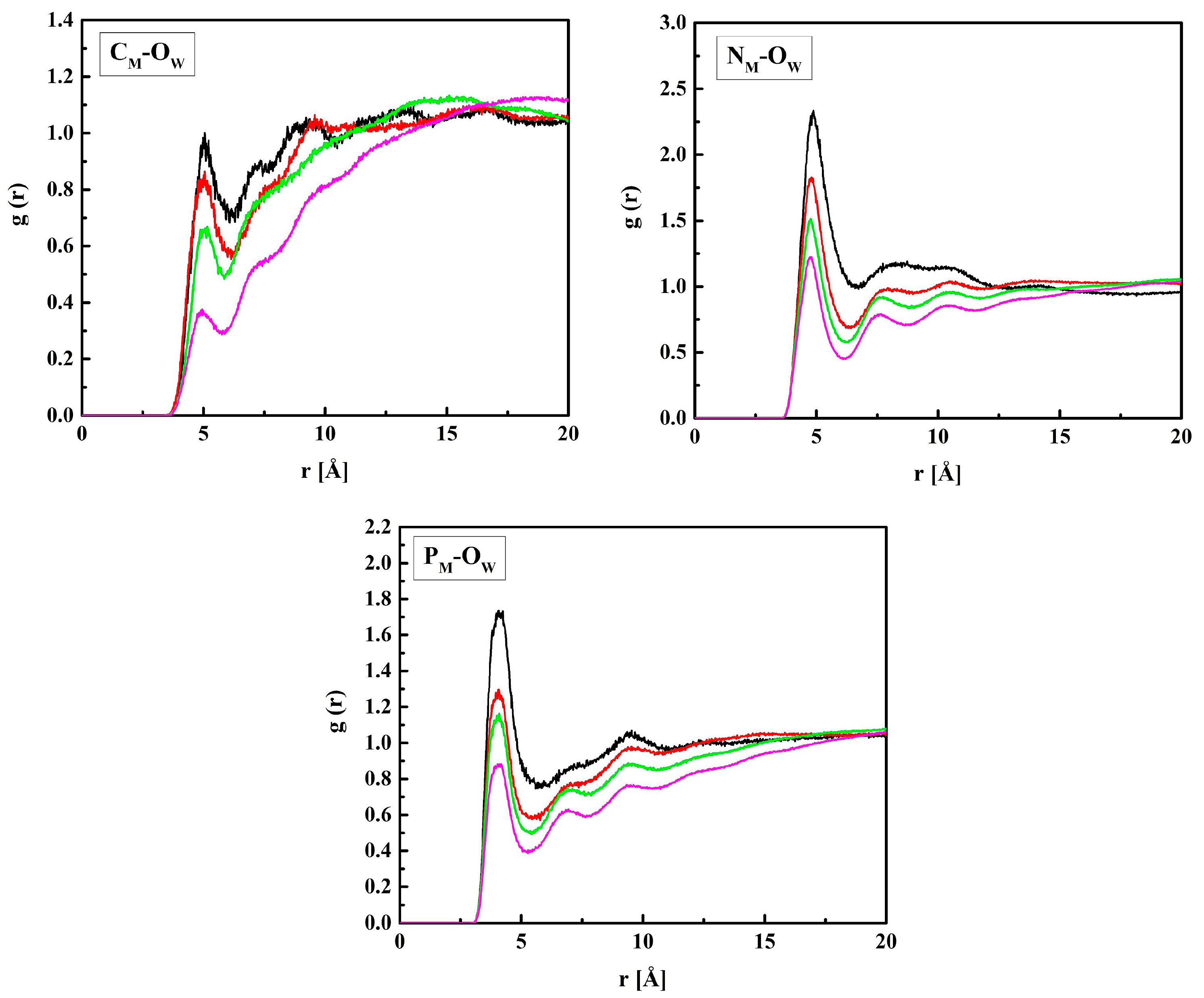
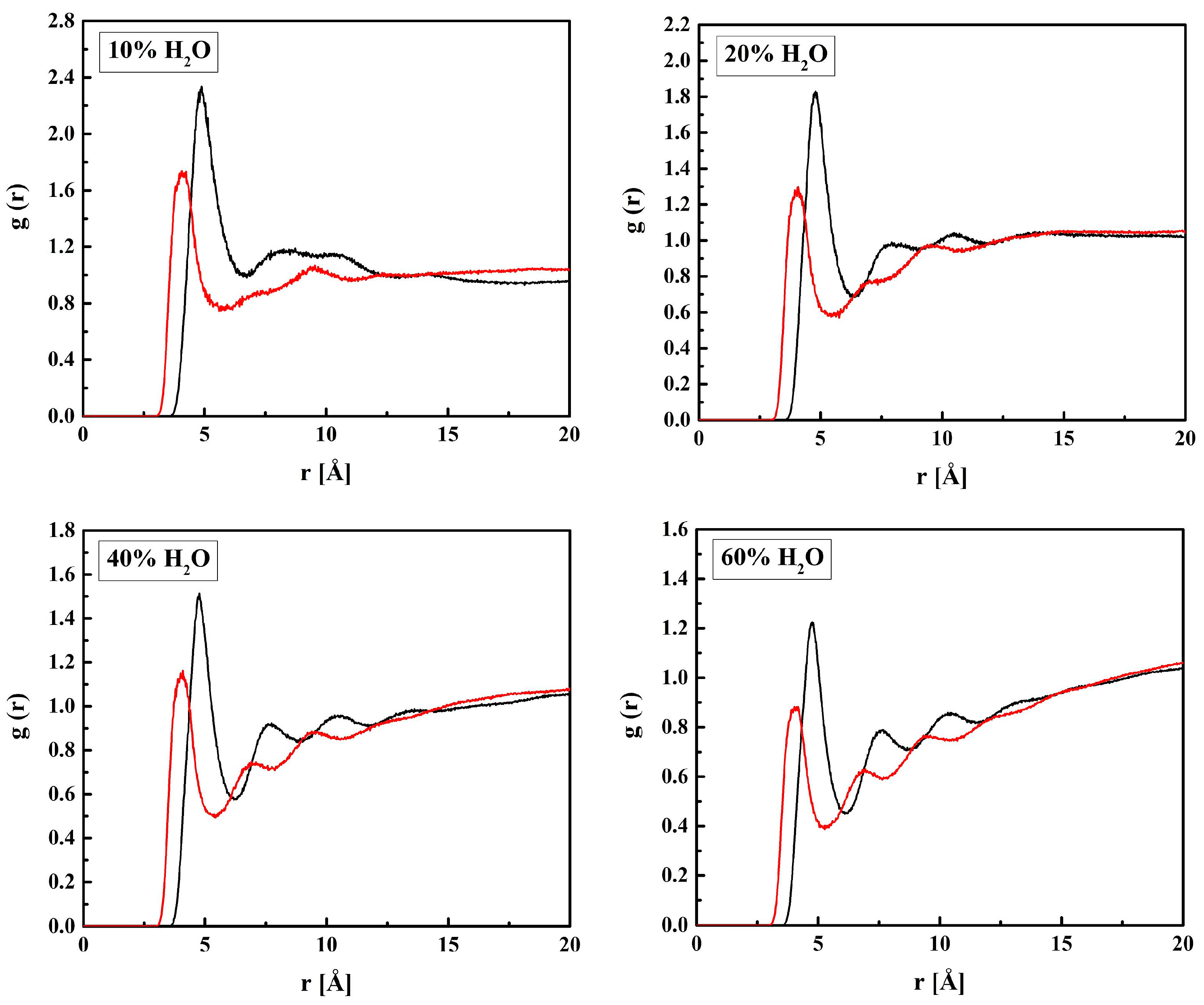
2.2. Distribution of Water Molecules
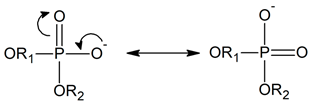
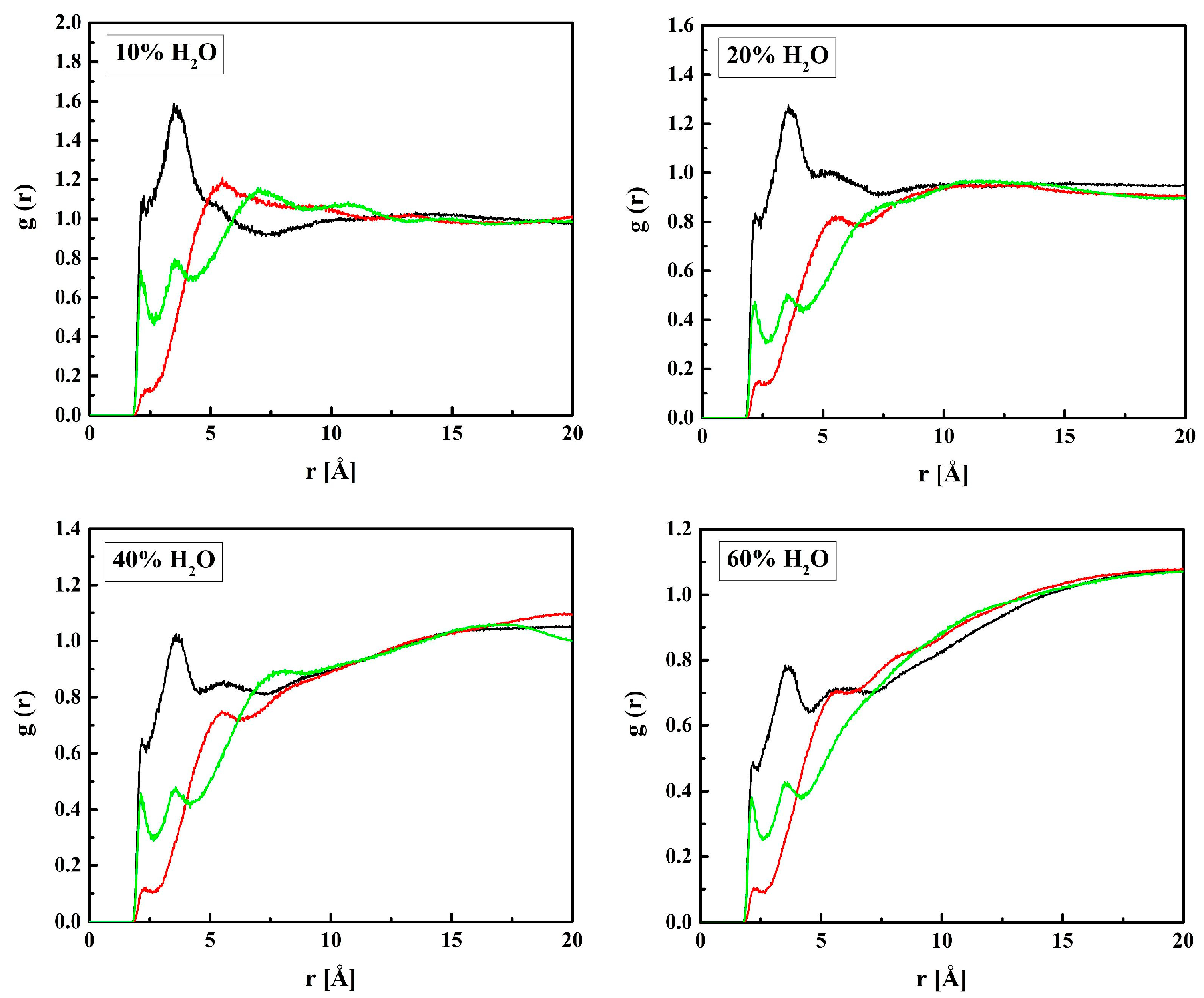
2.3. Hydrogen Bonding
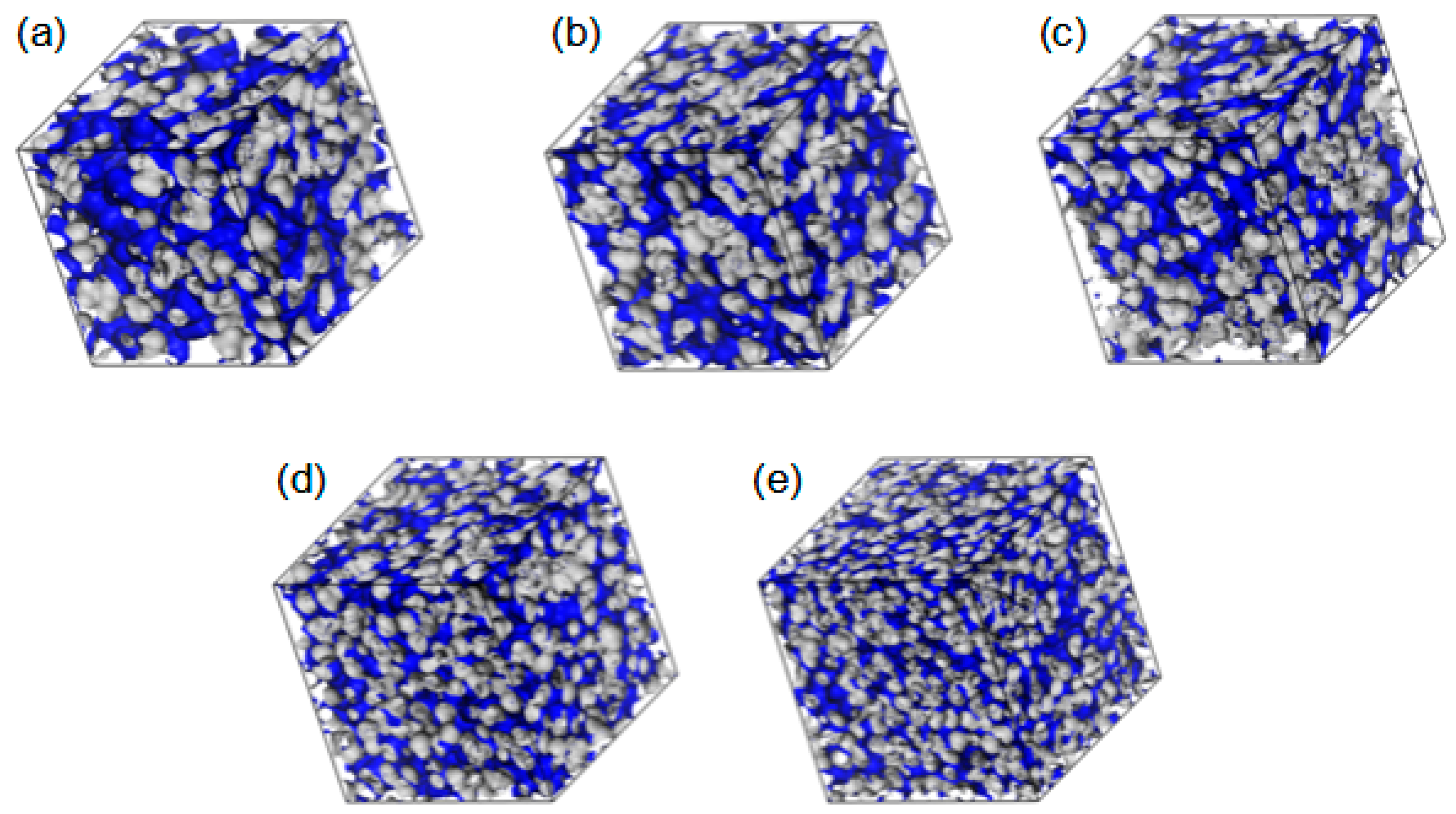
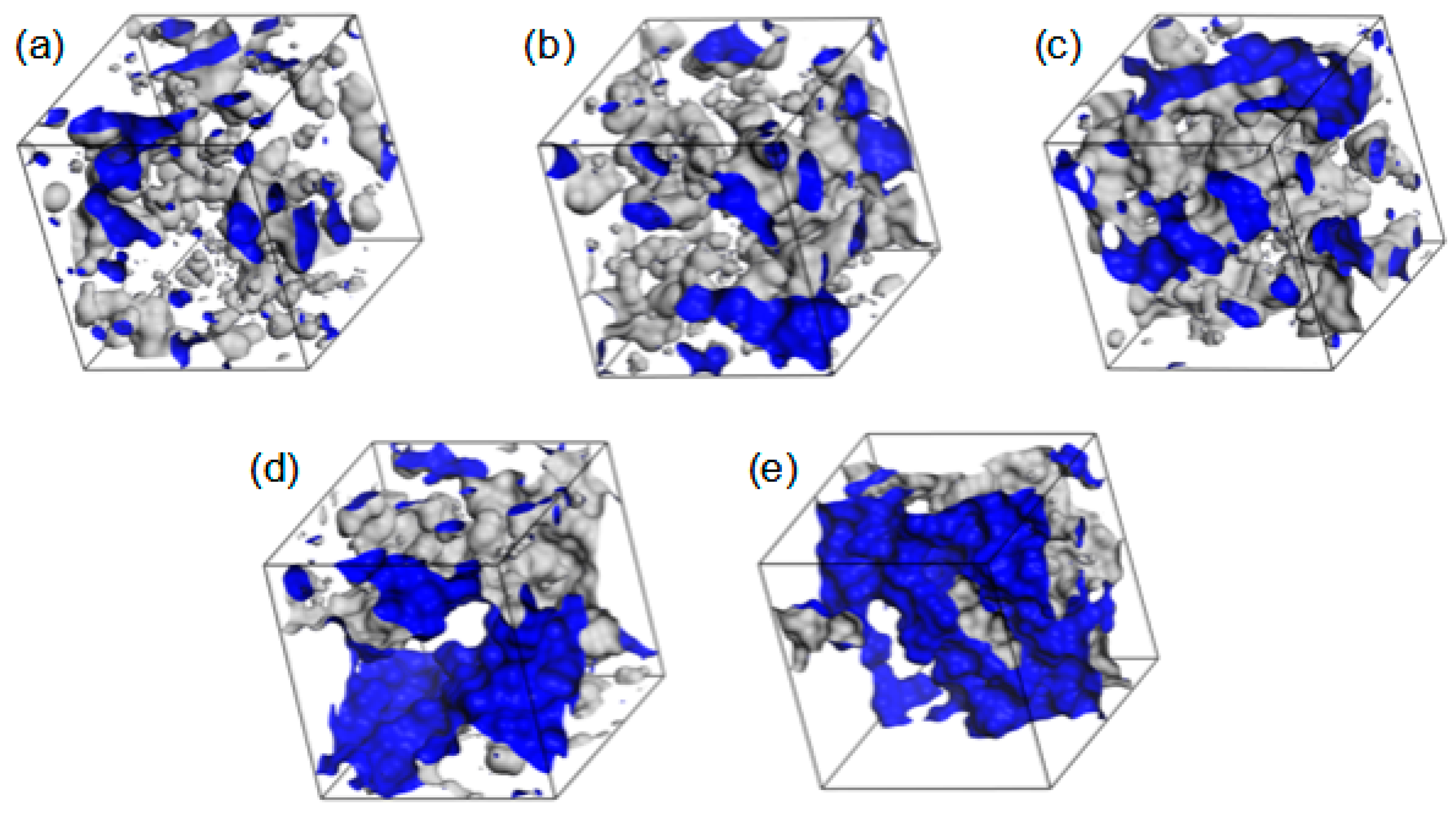
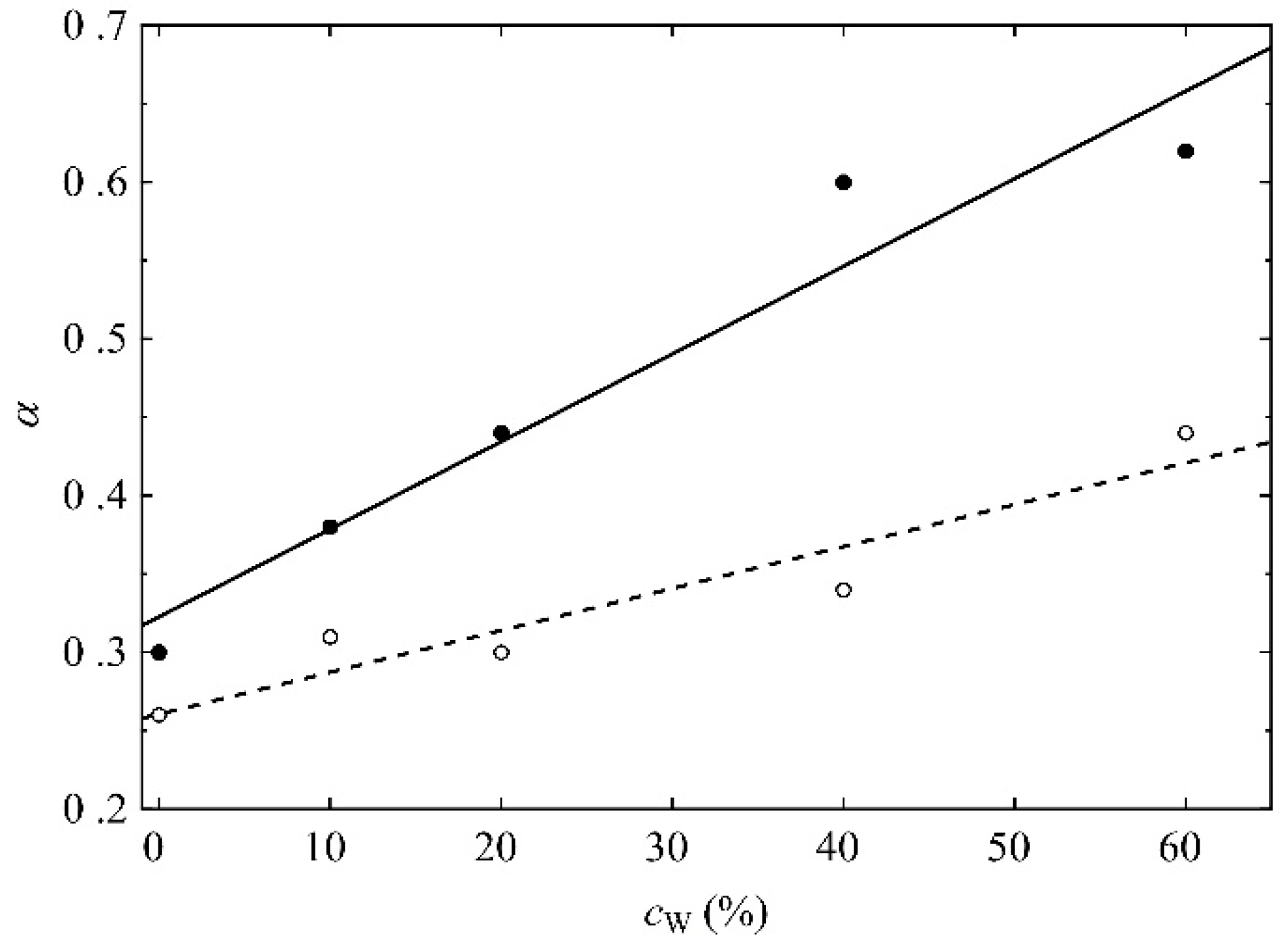
2.4. Free Volumes
2.5. Dynamics of the Polymer Chains
2.6. Dynamics of Water Molecules
3. Computer Simulations
3.1. Model Construction and Simulation Details
3.2. Characteristics of the Simulated System
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Ahmed, E.M. Hydrogel: Preparation, characterization, and applications: A review. J. Adv. Res. 2015, 6, 105–121. [Google Scholar] [CrossRef]
- Hoffman, A.S. Hydrogels for biomedical applications. Adv. Drug Deliv. Rev. 2012, 64, 18–23. [Google Scholar] [CrossRef]
- Caló, E.; Khutoryanskiy, V.V. Biomedical applications of hydrogels: A review of patents and commercial products. Eur. Polym. J. 2015, 65, 252–267. [Google Scholar] [CrossRef]
- Franco, P.; De Marco, I. Contact lenses as ophthalmic drug delivery systems: A review. Polymers 2021, 13, 1102. [Google Scholar] [CrossRef]
- Ottenbrite, R.M.; Park, K.; Okano, T. Biomedical Applications of Hydrogels Handbook; Springer: New York, NY, USA, 2010. [Google Scholar]
- Mantha, S.; Pillai, S.; Khayambashi, P.; Upadhyay, A.; Zhang, Y.; Tao, O.; Pham, H.M.; Tran, S.D. Smart hydrogels in tissue engineering and regenerative medicine. Materials 2019, 12, 3323. [Google Scholar] [CrossRef]
- Kirchhof, S.; Goepferich, A.M.; Brandl, F.P. Hydrogels in ophthalmic applications. Eur. J. Pharm. Biopharm. 2015, 95, 227–238. [Google Scholar] [CrossRef]
- Wichterle, O.; Lim, D. Hydrophilic gels for biological use. Nature 1960, 185, 117–118. [Google Scholar] [CrossRef]
- Nicolson, P.C.; Vogt, J. Soft contact lens polymers: An evolution. Biomaterials 2001, 22, 3273–3283. [Google Scholar] [CrossRef]
- Chaudhari, P.; Ghate, V.M.; Lewis, S.A. Next-generation contact lenses: Towards bioresponsive drug delivery and smart technologies in ocular therapeutics. Eur. J. Pharm. Biopharm. 2021, 161, 80–99. [Google Scholar] [CrossRef]
- Montheard, J.P.; Chatzopoulos, M.; Chappard, D. 2-Hydroxyethyl methacrylate (HEMA): Chemical properties and applications in biomedical fields. J. Macromol. Sci. Rev. Macromol. Chem. Phys. 1992, 32, 1–34. [Google Scholar] [CrossRef]
- Arica, M.Y.; Senel, S.; Alaeddinoglu, N.G.; Patir, S.; Denizli, A. Invertase immobilized on spacer-arm attached poly(hydroxyethyl methacrylate) membrane: Preparation and properties. J. Appl. Polym. Sci. 2000, 75, 1685–1692. [Google Scholar] [CrossRef]
- Hacker, M.C.; Krieghoff, J.; Mikos, A.G. Synthetic polymers. In Principles of Regenerative Medicine, 2nd ed.; Atala, A., Lanza, R., Thomson, J., Nerem, R., Eds.; Elsevier Academic Press: San Diego, CA, USA, 2011; pp. 587–622. [Google Scholar]
- Hernandez-Martínez, A.R.; Lujan-Montelongo, J.A.; Silva-Cuevas, C.; Mota-Morales, J.D.; Cortez-Valadez, M.; de Jesus Ruíz-Baltazar, Á.; Cruz, M.; Herrera-Ordonez, J. Swelling and methylene blue adsorption of poly (N,N-dimethylacrylamide-co-2-hydroxyethyl methacrylate) hydrogel. React. Funct. Polym. 2018, 122, 75–84. [Google Scholar] [CrossRef]
- Jeria, M.; Pizarro, G.D.C.; Marambio, O.G.; Huerta, M.; Valencia, P.; Rivas, B.L. Copolymers of 2-hydroxyethylmethacrylate and N-maleoylglycine: Synthesis, characterization, and swelling behavior. J. Appl. Polym. Sci. 2005, 98, 1897–1902. [Google Scholar] [CrossRef]
- Stapleton, F.; Stretton, S.; Papas, E.; Skotnitsky, C.; Sweeney, D.F. Silicone hydrogel contact lenses and the ocular surface. Ocul. Surf. 2006, 4, 24–43. [Google Scholar] [CrossRef]
- Liesegang, T.J. Physiologic changes of the cornea with contact lens wear. CLAO J. 2002, 28, 12–27. [Google Scholar]
- Jones, L. Modern contact lens materials: A clinical performance update. Contact Lens Spectr. 2002, 17, 24–35. [Google Scholar]
- Tighe, B. Soft lens materials. In Contact Lens Practice, 1st ed.; Efron, N., Ed.; Butterworth-Heinemann: Oxford, UK, 2002; pp. 71–84. [Google Scholar]
- Maldonado-Codina, C. Soft lens materials. In Contact Lens Practice, 2nd ed.; Efron, N., Ed.; Butterworth-Heinemann: Oxford, UK, 2010; pp. 67–85. [Google Scholar]
- Krylov, N.A.; Pentkovsky, V.M.; Efremov, R.G. Nontrivial behavior of water in the vicinity and inside lipid bilayers as probed by molecular dynamics simulations. ACS Nano 2013, 7, 9428–9442. [Google Scholar] [CrossRef]
- Ishihara, K.; Mu, M.; Konno, T.; Inoue, Y.; Fukazawa, K. The unique hydration state of poly(2-methacryloyloxyethyl phosphorylcholine). J. Biomater. Sci. Polym. Ed. 2017, 28, 884–899. [Google Scholar] [CrossRef]
- Kitano, H.; Imai, M.; Mori, T.; Gemmei-Ide, M.; Yokoyama, Y.; Ishihara, K. Structure of water in the vicinity of phospholipid analogue copolymers as studied by vibrational spectroscopy. Langmuir 2003, 19, 10260–10266. [Google Scholar] [CrossRef]
- Morisaku, T.; Watanabe, J.; Konno, T.; Takai, M.; Ishihara, K. Hydration of phosphorylcholine groups attached to highly swollen polymer hydrogels studied by thermal analysis. Polymer 2008, 49, 4652–4657. [Google Scholar] [CrossRef]
- Debnath, A.; Mukherjee, B.; Ayappa, K.G.; Maiti, P.K.; Lin, S.T. Entropy and dynamics of water in hydration layers of a bilayer. J. Chem. Phys. 2010, 133, 174704. [Google Scholar] [CrossRef] [PubMed]
- Berkowitz, M.L.; Vácha, R. Aqueous solutions at the interface with phospholipid bilayers. Acc. Chem. Res. 2012, 45, 74–82. [Google Scholar] [CrossRef]
- Ishihara, K.; Nomura, H.; Mihara, T.; Kurita, K.; Iwasaki, Y.; Nakabayashi, N. Why do phospholipid polymers reduce protein adsorption? Biomed. Mater. Res. 1998, 39, 323–330. [Google Scholar] [CrossRef]
- Zhang, Z.; Chen, S.; Chang, Y.; Jiang, S. Surface grafted sulfobetaine polymers via atom transfer radical polymerization as superlow fouling coatings. J. Phys. Chem. B 2006, 110, 10799–10804. [Google Scholar] [CrossRef]
- Ishihara, K. Blood-compatible surfaces with phosphorylcholine-based polymers for cardiovascular medical devices. Langmuir 2018, 35, 1778–1787. [Google Scholar] [CrossRef] [PubMed]
- Ishihara, K.; Ziats, N.P.; Tierney, B.P.; Nakabayashi, N.; Anderson, J.M. Protein adsorption from human plasma is reduced on phospholipid polymers. J. Biomed. Mater. Res. 1991, 25, 1397–1407. [Google Scholar] [CrossRef] [PubMed]
- Lemp, M.A.; Caffery, B.; Lebow, K.; Lembach, R.; Park, J.; Foulks, G.; Hall, B.; Bowers, R.; McGarvey, S.; Young, G. Omafilcon A (Proclear) soft contact lenses in a dry eye population. CLAO J. 1999, 25, 40–47. [Google Scholar]
- Li, L.; Wang, J.H.; Xin, Z. Synthesis and biocompatibility of a novel silicone hydrogel containing phosphorylcholine. Eur. Polym. J. 2011, 47, 1795–1803. [Google Scholar] [CrossRef]
- Taddei, P.; Balducci, F.; Simoni, R.; Monti, P. IR and thermal study of a new highly biocompatible phosphorylcholine-based contact lens. J. Mol. Struct. 2005, 744–747, 507–514. [Google Scholar] [CrossRef]
- Lewis, A.L. Phosphorylcholine-based polymers and their use in the prevention of biofouling. Colloids Surf. B 2000, 18, 261–275. [Google Scholar] [CrossRef]
- Ishihara, K.; Fukazawa, K.; Sharma, V.; Liang, S.; Shows, A.; Dunbar, D.C.; Zheng, Y.; Ge, J.; Zhang, S.; Hong, Y.; et al. Antifouling silicone hydrogel contact lenses with a bioinspired 2-methacryloyloxyethyl phosphorylcholine polymer surface. ACS Omega 2021, 6, 7058–7067. [Google Scholar] [CrossRef]
- Jenkins, A.D.; Kratochvíl, P.; Stepto, R.F.T.; Suter, U.W. Glossary of basic terms in polymer science. Pure Appl. Chem. 1996, 68, 2287–2311. [Google Scholar] [CrossRef]
- Sun, D.; Zhou, J. Effect of water content on microstructures and oxygen permeation in PSiMA–IPN–PMPC hydrogel: A molecular simulation study. Chem. Eng. Sci. 2012, 78, 236–245. [Google Scholar] [CrossRef]
- Wu, C. Cooperative behavior of poly(vinyl alcohol) and water as revealed by molecular dynamics simulations. Polymer 2010, 51, 4452–4460. [Google Scholar] [CrossRef]
- Tamai, Y.; Tanaka, H.; Nakanishi, K. Molecular dynamics study of polymer—Water interaction in hydrogels. 1. Hydrogen-bond structure. Macromolecules 1996, 29, 6750–6760. [Google Scholar] [CrossRef]
- Tamai, Y.; Tanaka, H.; Nakanishi, K. Molecular dynamics study of polymer—Water interaction in hydrogels. 2. Hydrogen-bond dynamics. Macromolecules 1996, 29, 6761–6769. [Google Scholar] [CrossRef]
- Tamai, Y.; Tanaka, H. Permeation of small penetrants in hydrogels. Fluid Phase Equilibria 1998, 144, 441–448. [Google Scholar] [CrossRef]
- Chiessi, E.; Cavalieri, F.; Paradossi, G. Water and polymer dynamics in chemically cross-linked hydrogels of poly(vinyl alcohol): A molecular dynamics simulation study. J. Phys. Chem. B 2007, 111, 2820–2827. [Google Scholar] [CrossRef]
- Jiang, X.; Wang, C.; Han, Q. Molecular dynamic simulation on the state of water in poly(vinyl alcohol) hydrogel. Comput. Theor. Chem. 2017, 1102, 15–21. [Google Scholar] [CrossRef]
- Karlsson, G.E.; Gedde, U.W.; Hedenqvist, M.S. Molecular dynamics simulation of oxygen diffusion in dry and water-containing poly(vinyl alcohol). Polymer 2004, 45, 3893–3900. [Google Scholar] [CrossRef]
- Bermejo, J.S.; Ugarte, C.M. Influence of water content on structure and mobility of polyvinyl alcohol: A molecular dynamics simulation. J. Chem. Phys. 2008, 129, 154907. [Google Scholar] [CrossRef]
- Bermejo, J.S.; Ugarte, C.M. Chemical crosslinking of PVA and prediction of material properties by means of fully atomistic MD simulation. Macromol. Theory Simul. 2009, 18, 259–267. [Google Scholar] [CrossRef]
- Wei, Q.; Zhang, Y.; Wang, Y.; Chai, W.; Yang, M.; Zeng, W.; Wang, M. Study of the effects of water content and temperature on polyacrylamide/polyvinyl alcohol interpenetrating network hydrogel performance by a molecular dynamics method. e-Polymers 2015, 15, 301–309. [Google Scholar] [CrossRef]
- Zhao, Z.J.; Wang, Q.; Zhang, L.; Wu, T. Structured water and water-polymer interactions in hydrogels of molecularly imprinted polymers. J. Phys. Chem. B 2008, 112, 7515–7521. [Google Scholar] [CrossRef] [PubMed]
- Pozuelo, J.; Compañ, V.; González-Méijome, J.M.; González, M.; Mollá, S. Oxygen and ionic transport in hydrogel and silicone-hydrogel contact lens materials: An experimental and theoretical study. J. Membr. Sci. 2014, 452, 62–72. [Google Scholar] [CrossRef]
- Lee, S.G.; Brunello, G.F.; Jang, S.S.; Bucknall, D.G. Molecular dynamics simulation study of P(VP-co-HEMA) hydrogels: Effect of water content on equilibrium structures and mechanical properties. Biomaterials 2009, 30, 6130–6141. [Google Scholar] [CrossRef] [PubMed]
- Lee, S.G.; Brunello, G.F.; Jang, S.S.; Lee, J.H.; Bucknall, D.G. Effect of monomeric sequence on mechanical properties of P(VP-co-HEMA) hydrogels at low-hydration. J. Phys. Chem. B 2009, 113, 6604–6612. [Google Scholar] [CrossRef]
- Filipecka, K.; Miedziński, R.; Sitarz, M.; Filipecki, J.; Makowska-Janusik, M. Optical and vibrational properties of phosphorylcholine-based contact lenses—Experimental and theoretical investigations. Spectrochim. Acta A 2017, 176, 83–90. [Google Scholar] [CrossRef]
- Oldiges, C.; Tönsing, T.; Wittler, K. Molecular dynamics simulation of structural, mobility effects between dilute aqueous CH3CN solution and crosslinked PAA—Part 2. Dynamics. Phys. Chem. Chem. Phys. 2002, 4, 5135–5141. [Google Scholar] [CrossRef]
- Wu, C.; Xu, W. Atomistic simulation study of absorbed water influence on structure and properties of crosslinked epoxy resin. Polymer 2007, 48, 5440–5448. [Google Scholar] [CrossRef]
- Perova, T.S.; Vij, J.K.; Xu, H. Fourier transform infrared study of poly(2-hydroxyethyl methacrylate) PHEMA. Colloid Polym. Sci. 1997, 275, 323–332. [Google Scholar] [CrossRef]
- Coyle, F.C.; Martin, S.J.; McBrierty, V.J. Dynamics of water molecules in polymers. J. Mol. Liq. 1996, 69, 95–116. [Google Scholar] [CrossRef]
- Tamai, Y.; Tanaka, H.; Nakanishi, K. Molecular simulation of permeation of small penetrants through membranes. 1. Diffusion coefficients. Macromolecules 1994, 27, 4498–4508. [Google Scholar] [CrossRef]
- Nagel, C.; Schmidtke, E.; Gunther-Schade, K.; Hofmann, D.; Fritsch, D.; Strunskus, T. Free volume distributions in glassy polymer membranes: Comparison between molecular modeling and experiments. Macromolecules 2000, 33, 2242–2248. [Google Scholar] [CrossRef]
- Van der Berg, C. Food water relationships: Progress and integration, comments and thoughts. In Water Relationships in Foods; Levine, H., Slade, L., Eds.; Springer Science & Business Media: New York, NY, USA, 1991; pp. 21–28. [Google Scholar]
- Rouse, P.E., Jr. A theory of the linear viscoelastic properties of dilute solutions of coiling polymers. J. Chem. Phys. 1953, 21, 1272–1280. [Google Scholar] [CrossRef]
- Zimm, B.H. Dynamics of polymer molecules in dilute solution: Viscoelasticity, flow birefringence and dielectric loss. J. Chem. Phys. 1956, 24, 269–278. [Google Scholar] [CrossRef]
- Rubinstein, M.; Colby, R.H. Polymer Physics; Oxford University Press: New York, NY, USA, 2003. [Google Scholar]
- Dietrich, O. Diffusion Coefficients of Water. Available online: https://dtrx.de/od/diff/ (accessed on 9 August 2023).
- Lee, S.G.; Jang, S.S.; Kim, J.; Kim, G. Distribution and diffusion of water in model epoxy molding compound: Molecular dynamics simulation approach. IEEE Trans. Adv. Packag. 2009, 33, 333–339. [Google Scholar]
- Materials Studio, Version 8.0.100.21; Accelrys Software Inc.: San Diego, CA, USA, 2014.
- Mayo, S.L.; Olafson, B.D.; Goddard, W.A. Dreiding—A generic force-field for molecular simulations. J. Phys. Chem. 1990, 94, 8897–8909. [Google Scholar] [CrossRef]
- Jang, S.S.; Goddard, W.A.; Kalani, M.Y.S. Mechanical and transport properties of the poly(ethylene oxide)-poly(acrylic acid) double network hydrogel from molecular dynamic simulations. J. Phys. Chem. B 2007, 111, 1729–1737. [Google Scholar] [CrossRef]
- Li, C.; Coons, E.; Strachan, A. Material property prediction of thermoset polymers by molecular dynamics simulations. Acta Mech. 2014, 225, 1187–1196. [Google Scholar] [CrossRef]
- Verlet, L. Computer “experiments” on classical fluids. I. Thermodynamical properties of Lennard-Jones molecules. Phys. Rev. 1967, 159, 98–103. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A 1985, 31, 1695–1697. [Google Scholar] [CrossRef]
- Mapstone, R. Measurement of corneal temperature. Exp. Eye Res. 1968, 7, 237–242. [Google Scholar] [CrossRef]
- Borsal, R.; Pecora, R. (Eds.) Soft-Matter Characterisation; Springer Science & Business Media: New York, NY, USA, 2008. [Google Scholar]
- Matsumoto, M. Relevance of hydrogen bond definitions in liquid water. J. Chem. Phys. 2007, 126, 054503. [Google Scholar] [CrossRef] [PubMed]
- Swapna, V.P.; Abhisha, V.S.; Stephen, R. Polymer/polyhedral oligomeric silsesquioxane nanocomposite membranes for pervaporation. In Polymer Nanocomposite Membranes for Pervaporation; Thomas, S., George, S.C., Jose, T., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; pp. 201–229. [Google Scholar]
- Connolly, M.L. Computation of molecular volume. J. Am. Chem. Soc. 1985, 107, 1118–1124. [Google Scholar] [CrossRef]
- Zawodzinski, T.A., Jr.; Neeman, M.; Sillerud, L.O.; Gottesfeld, S. Determination of water diffusion coefficients in perfluorosulfonate ionomeric membranes. J. Phys. Chem. 1991, 95, 6040–6044. [Google Scholar] [CrossRef]

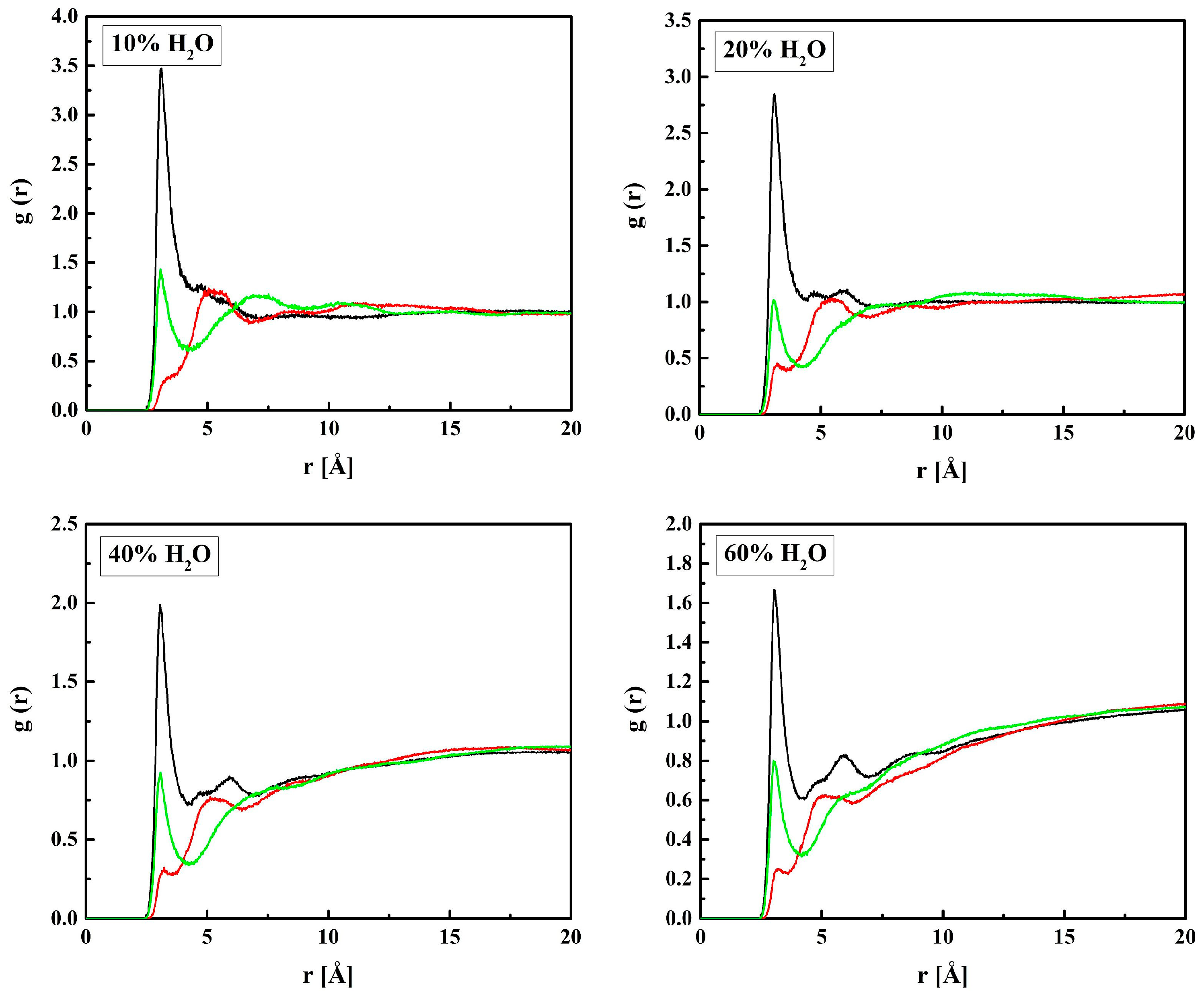
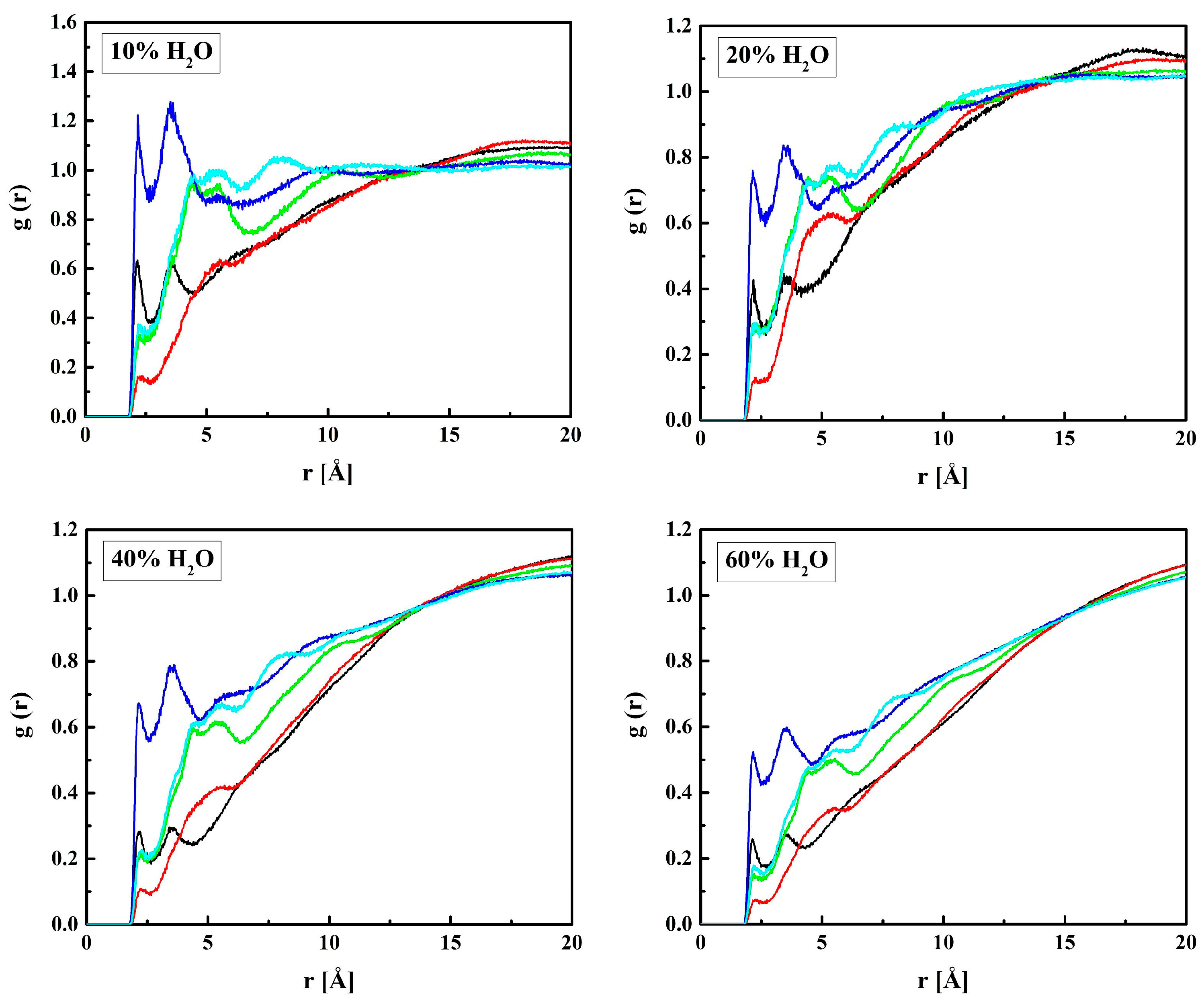
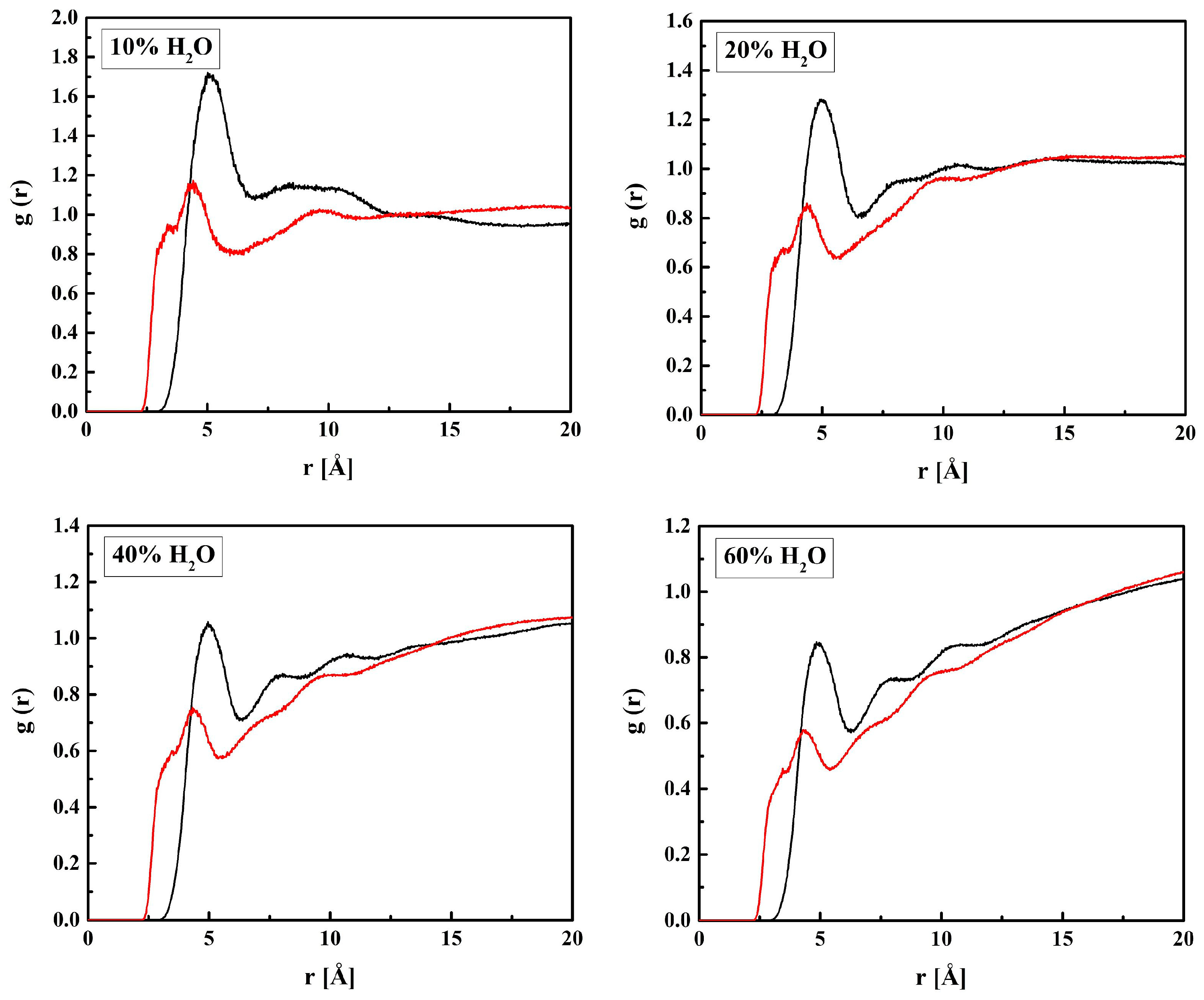
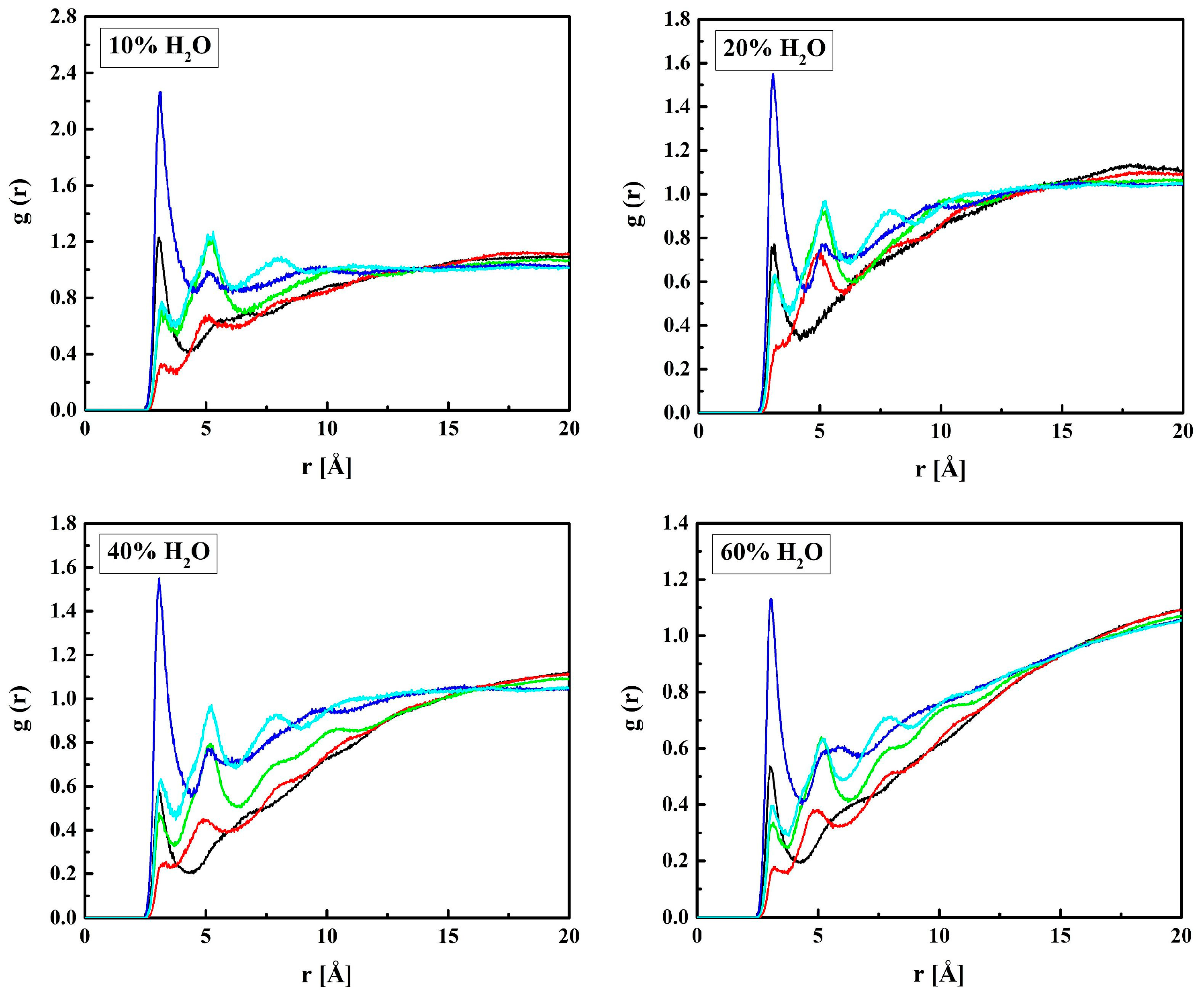



| Copolymer | Water Content by Mass (%) | Number of Water Molecules in the Cell | Unit Cell Edge (Å) |
|---|---|---|---|
| R13, B13 | 0 | 0 | 23.566 |
| 10 | 54 | 24.417 | |
| 20 | 120 | 25.381 | |
| 40 | 321 | 27.943 | |
| 60 | 721 | 31.976 | |
| R37, B37 | 0 | 0 | 26.717 |
| 10 | 78 | 27.673 | |
| 20 | 175 | 28.777 | |
| 40 | 467 | 31.672 | |
| 60 | 1050 | 36.248 |
| Copolymer | Fractional Free Volume (%) | ||||
|---|---|---|---|---|---|
| No Water | 10% H2O | 20% H2O | 40% H2O | 60% H2O | |
| B13 | 39.6 | 38.7 | 38.0 | 36.6 | 35.3 |
| R13 | 39.8 | 38.8 | 38.0 | 36.7 | 35.4 |
| B37 | 41.0 | 39.9 | 39.0 | 37.3 | 35.7 |
| R37 | 40.8 | 39.9 | 39.0 | 37.2 | 35.8 |
| Copolymer | Fractional Free Volume (%) | ||||
|---|---|---|---|---|---|
| No Water | 10% H2O | 20% H2O | 40% H2O | 60% H2O | |
| B13 | 11.6 | 22.7 | 32.6 | 51.8 | 68.7 |
| R13 | 15.7 | 23.0 | 33.5 | 51.9 | 68.4 |
| B37 | 13.9 | 25.9 | 33.5 | 51.7 | 68.2 |
| R37 | 14.6 | 24.0 | 34.5 | 51.7 | 68.1 |
| Copolymer | α | |||
|---|---|---|---|---|
| 10% H2O | 20% H2O | 40% H2O | 60% H2O | |
| B13 | 0.74 | 0.88 | 0.93 | 0.97 |
| R13 | 0.74 | 0.88 | 0.92 | 0.97 |
| B37 | 0.76 | 0.89 | 0.95 | 0.99 |
| R37 | 0.79 | 0.90 | 0.95 | 0.99 |
| Copolymer | D·10−5 (cm2 s−1) | |
|---|---|---|
| 40% H2O | 60% H2O | |
| B13 | 1.55 | 1.62 |
| R13 | 1.53 | 1.62 |
| B37 | 1.58 | 1.65 |
| R37 | 1.58 | 1.65 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Filipecka-Szymczyk, K.; Makowska-Janusik, M.; Marczak, W. Molecular Dynamics Simulation of Hydrogels Based on Phosphorylcholine-Containing Copolymers for Soft Contact Lens Applications. Molecules 2023, 28, 6562. https://doi.org/10.3390/molecules28186562
Filipecka-Szymczyk K, Makowska-Janusik M, Marczak W. Molecular Dynamics Simulation of Hydrogels Based on Phosphorylcholine-Containing Copolymers for Soft Contact Lens Applications. Molecules. 2023; 28(18):6562. https://doi.org/10.3390/molecules28186562
Chicago/Turabian StyleFilipecka-Szymczyk, Katarzyna, Malgorzata Makowska-Janusik, and Wojciech Marczak. 2023. "Molecular Dynamics Simulation of Hydrogels Based on Phosphorylcholine-Containing Copolymers for Soft Contact Lens Applications" Molecules 28, no. 18: 6562. https://doi.org/10.3390/molecules28186562
APA StyleFilipecka-Szymczyk, K., Makowska-Janusik, M., & Marczak, W. (2023). Molecular Dynamics Simulation of Hydrogels Based on Phosphorylcholine-Containing Copolymers for Soft Contact Lens Applications. Molecules, 28(18), 6562. https://doi.org/10.3390/molecules28186562