Does a Similar 3D Structure Mean a Similar Folding Pathway? The Presence of a C-Terminal α-Helical Extension in the 3D Structure of MAX60 Drastically Changes the Folding Pathway Described for Other MAX-Effectors from Magnaporthe oryzae
Abstract
1. Introduction
2. Results
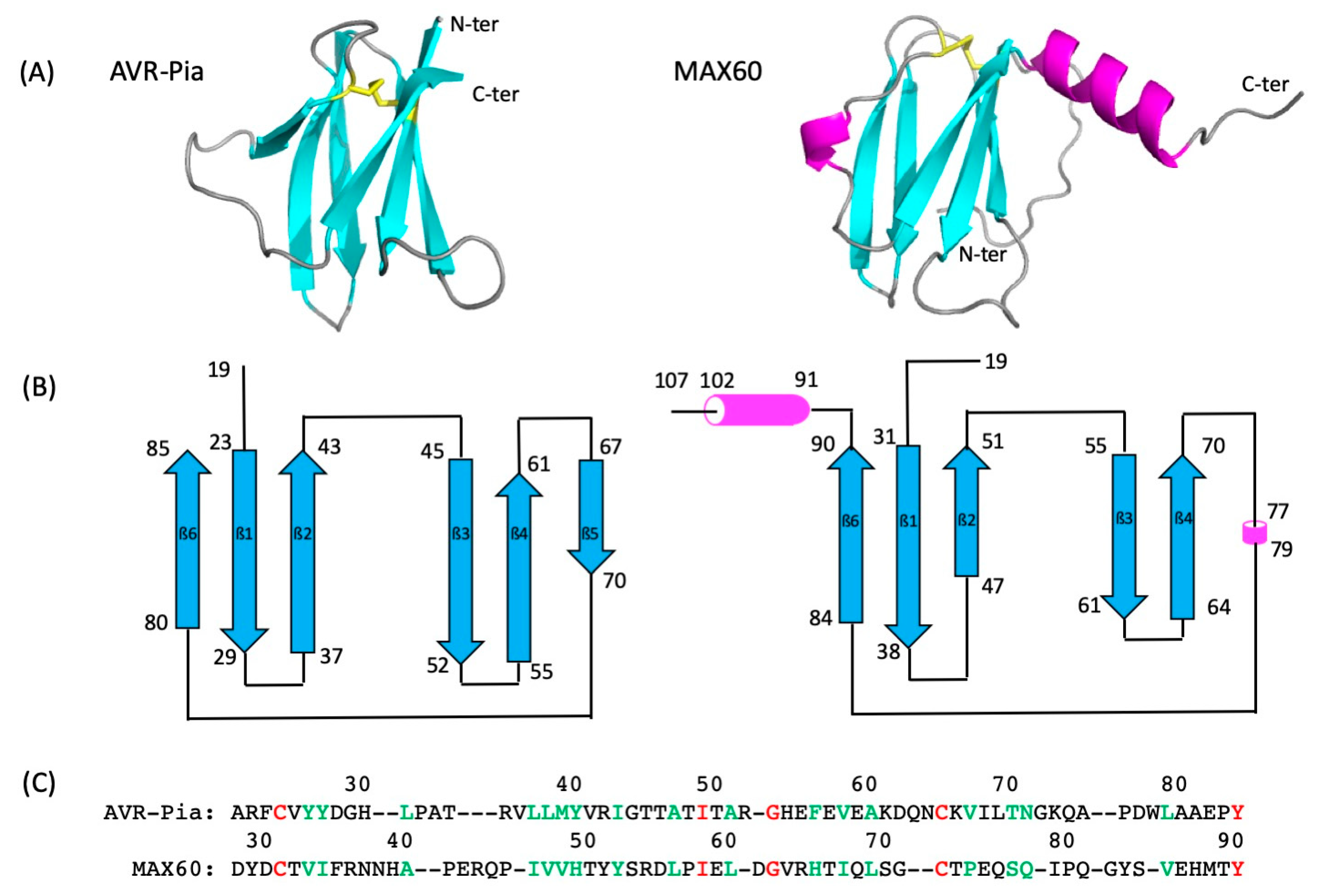
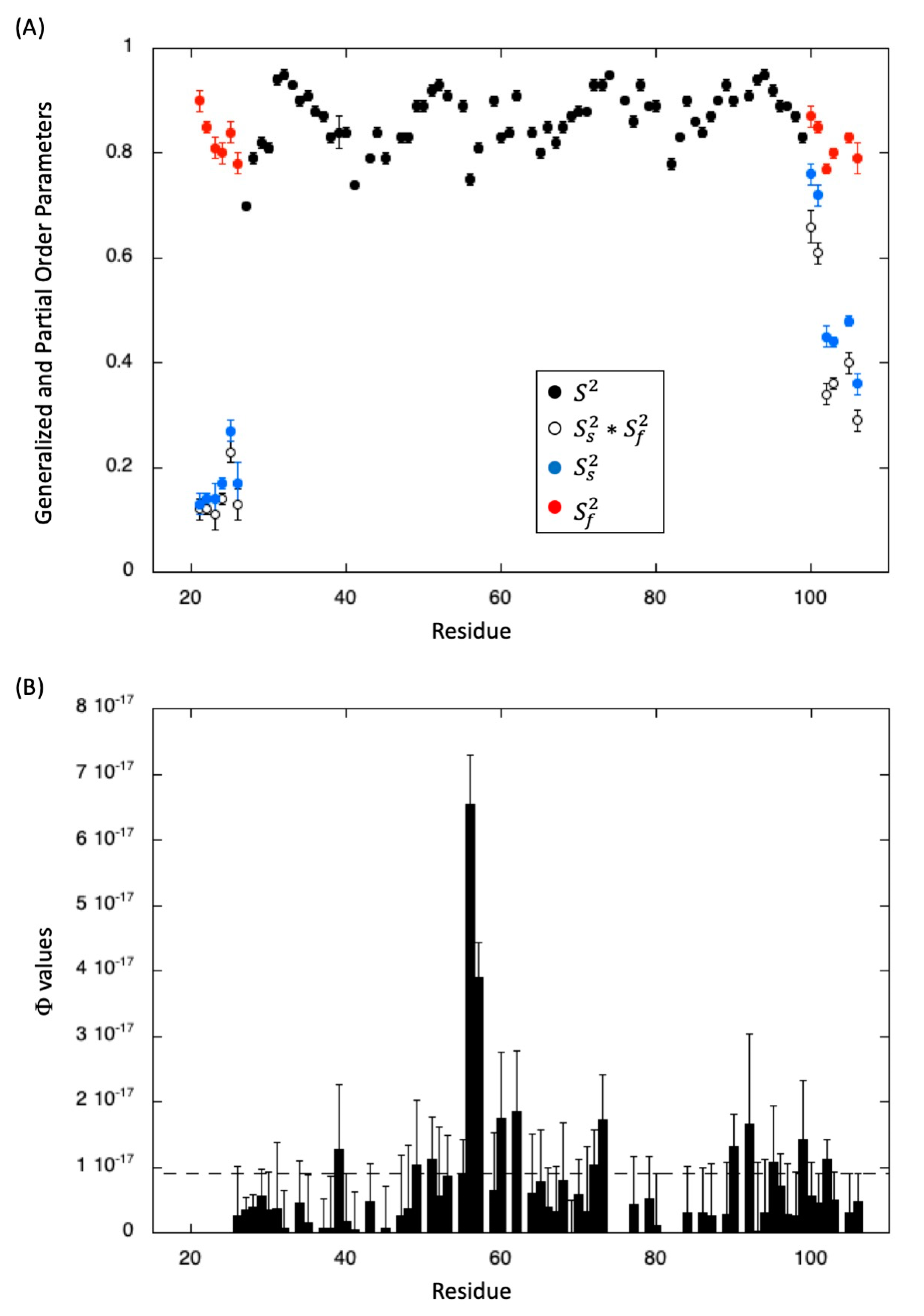
2.1. NMR Resonance Assignment, Solution Structure, and Intrinsic Dynamics
2.2. MAX60 Denaturation Studies
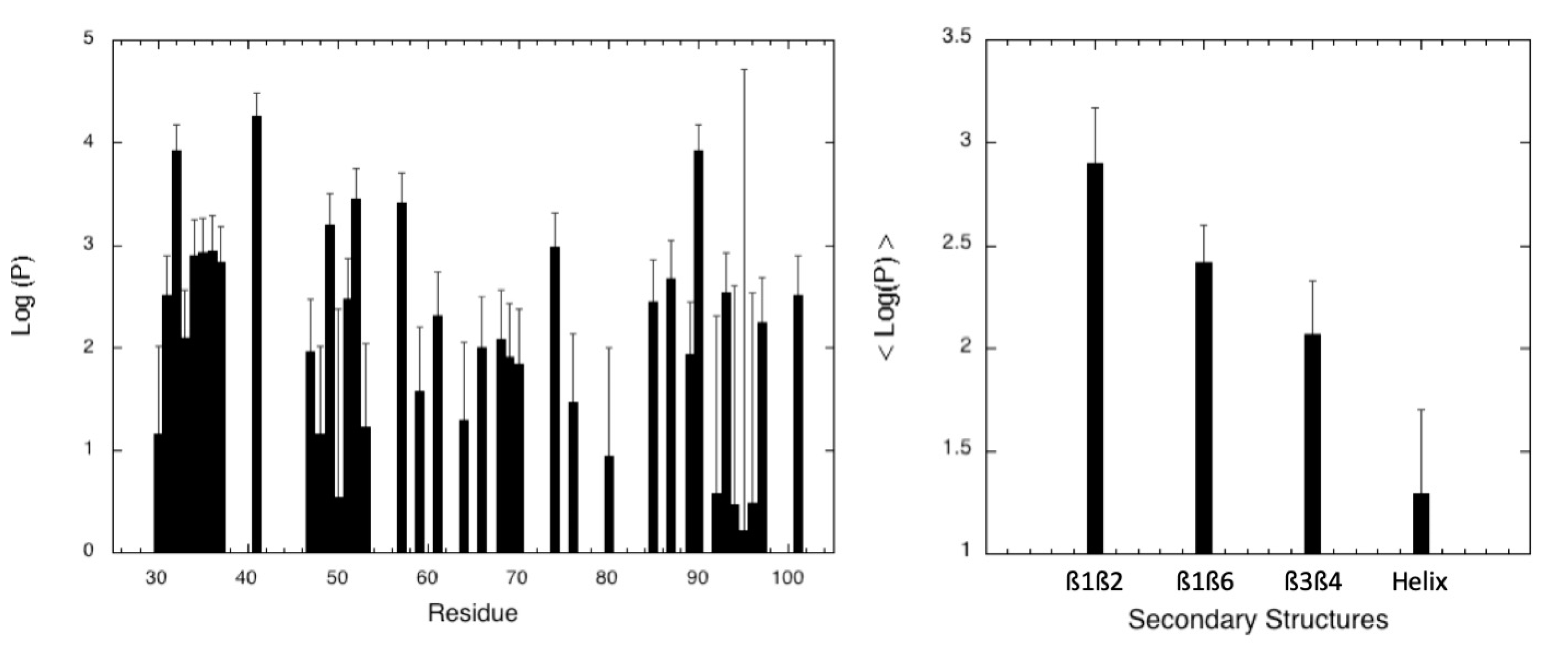
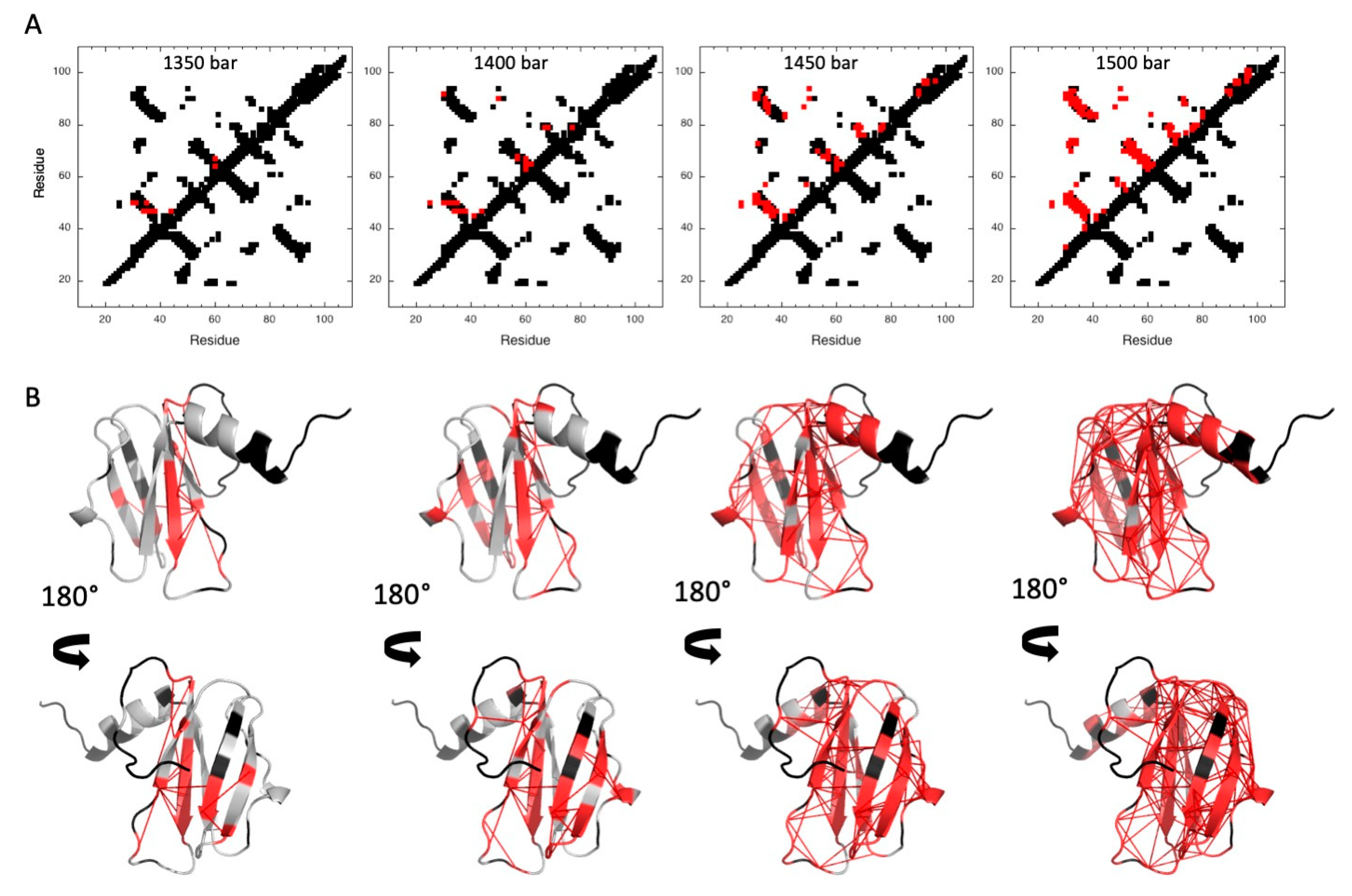
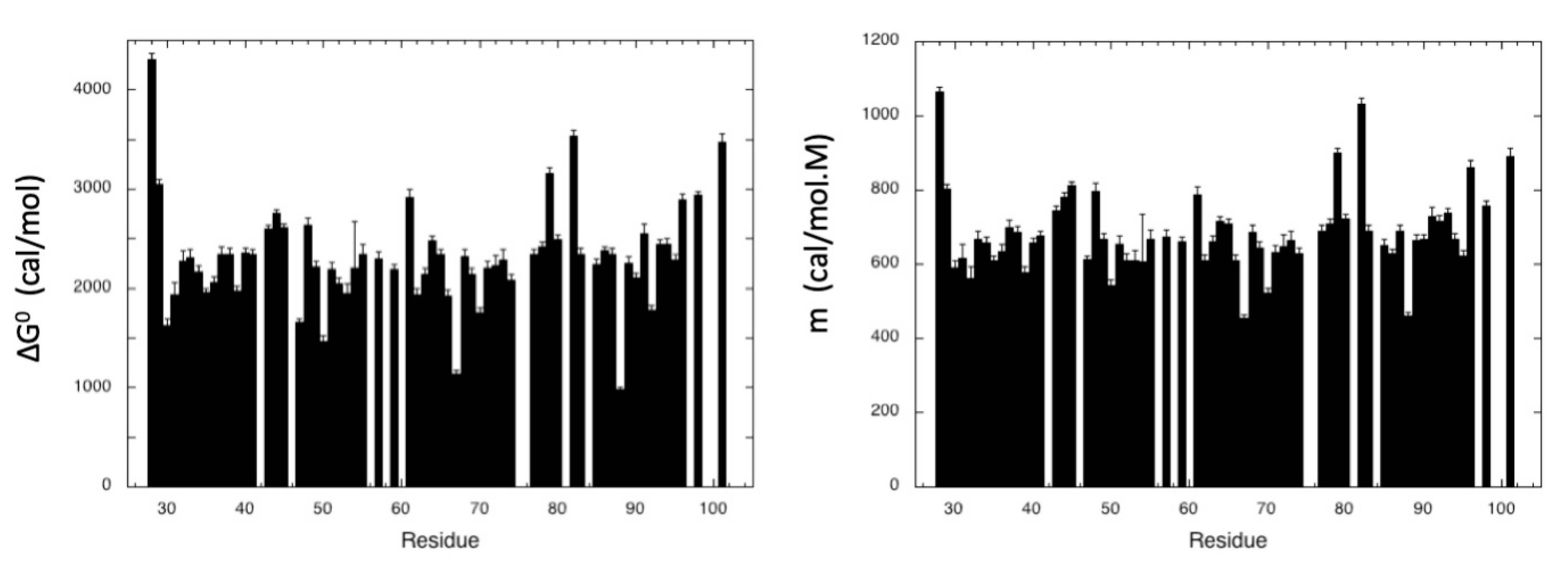
2.2.1. Pressure Denaturation
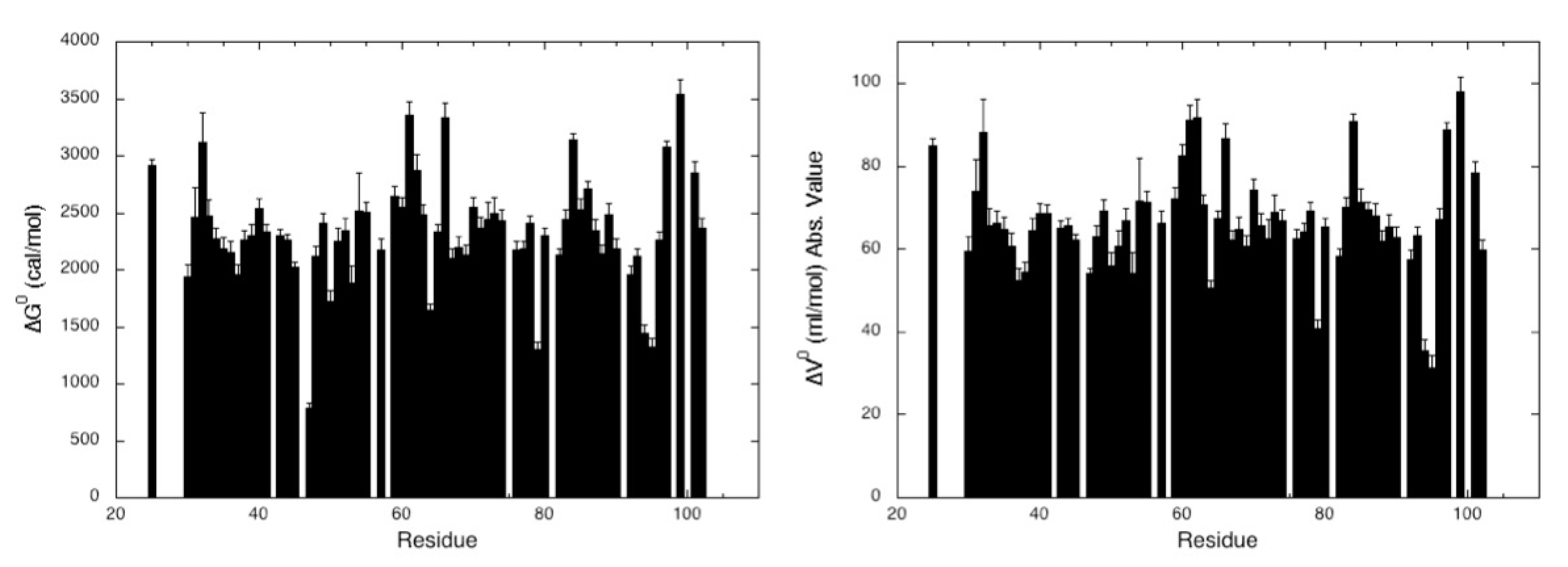
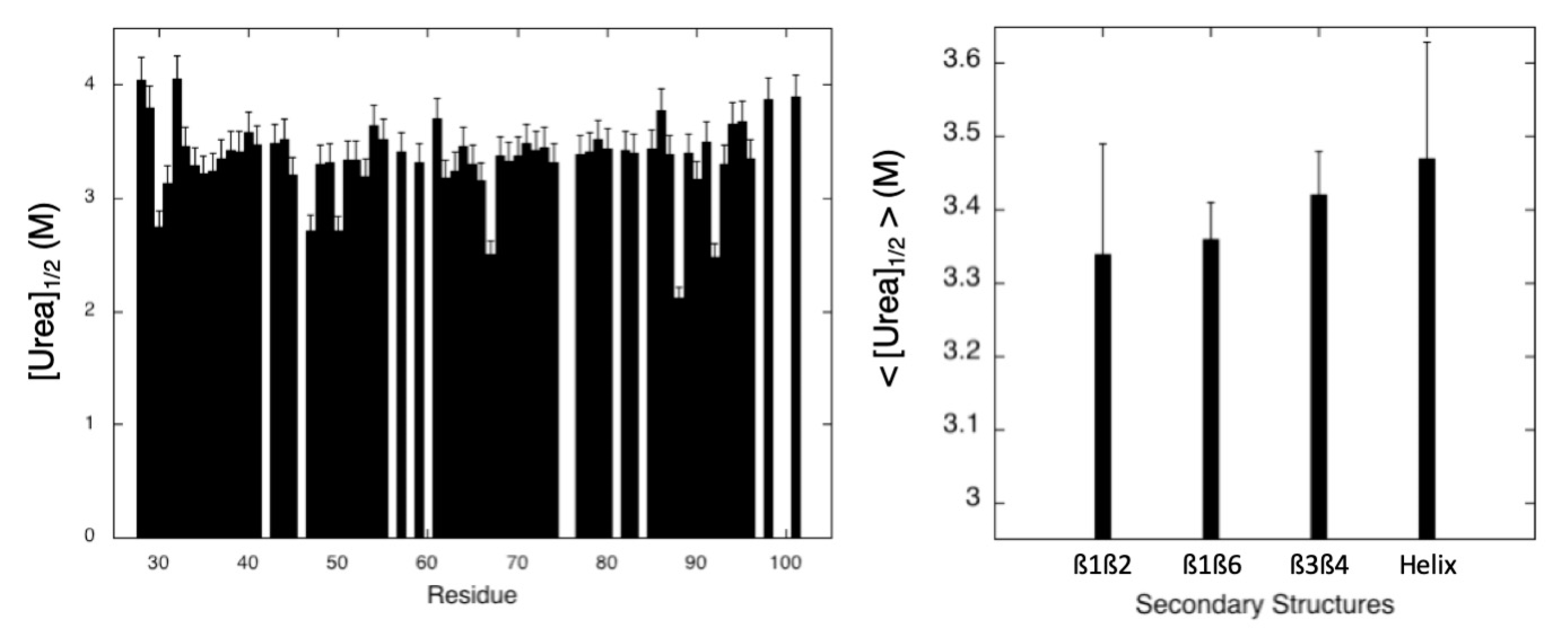
2.2.2. Chemical Denaturation
3. Discussion
4. Materials and Methods
4.1. Sample Preparation
4.2. NMR Assignments and Solution Structure
4.3. Relaxation Studies
4.4. Proton/Deuteron Exchange Measurements
4.5. Protein Unfolding
- -
- A list of Cα-Cα distance upper bounds with a cutoff of 9 Å generated by CMView from the PDB structure 7ZK0 of MAX60. In addition, lists of backbone dihedral restraints (Φ/Ψ at ± 10°) were also derived from the structures.
- -
- A list containing the probability pi to find a residue i in a folded state, obtained from the normalized experimental residue-specific denaturation curve obtained for residue i. These curves are obtained from the fit of the intensity decrease with pressure of HSQC cross-peak of residue i with Equation (5).
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
Abbreviations
References
- Anfinsen, C.B. Principles that govern the folding of protein chains. Sciences 1973, 181, 223–230. [Google Scholar] [CrossRef]
- Zhang, Z.-M.; Zhang, X.; Zhou, Z.-R.; Hu, H.Y.; Liu, M.; Zhou, B.; Zhou, J. Solution structure of the Magnaporthe oryzae avirulence protein AvrPiz-t. J. Biomol. NMR 2013, 55, 219–223. [Google Scholar] [CrossRef] [PubMed]
- Nyarko, A.; Singarapu, K.K.; Figueroa, M.; Manning, V.; Pandelova, I.; Wolpert, T.J.; Ciuffetti, L.M.; Barbar, E. Solution NMR Structures of Pyrenophora tritici-repentis ToxB and Its Inactive Homolog Reveal Potential Determinants of Toxin Activity. J. Biol. Chem. 2014, 289, 25946–25956. [Google Scholar] [CrossRef] [PubMed]
- Maqbool, A.; Saitoh, H.; Franceschetti, M.; Stevenson, C.E.; Uemura, A.; Kanzaki, H.; Kamoun, S.; Terauchi, R.; Banfield, M.J. Structural basis of pathogen recognition by an integrated HMA domain in a plant NLR immune receptor. eLife 2015, 4, e08709. [Google Scholar] [CrossRef] [PubMed]
- de Guillen, K.; Ortiz-Vallejo, D.; Gracy, J.; Fournier, E.; Kroj, T.; Padilla, A. Structure Analysis Uncovers a Highly Diverse but Structurally Conserved Effector Family in Phytopathogenic Fungi. PLoS Pathog. 2015, 11, e1005228. [Google Scholar] [CrossRef]
- Zhang, X.; He, D.; Zhao, Y.; Cheng, X.; Zhao, W.; Taylor, I.A.; Yang, J.; Liu, J.; Peng, Y.L. A positive-charged patch and stabilized hydrophobic core are essential for avirulence function of AvrPib in the rice blast fungus. Plant. J. 2018, 96, 133–146. [Google Scholar] [CrossRef] [PubMed]
- De la Concepcion, J.C.; Franceschetti, M.; Maqbool, A.; Saitoh, H.; Terauchi, R.; Kamoun, S.; Banfield, M.J. Polymorphic residues in rice NLRs expand binding and response to effectors of the blast pathogen. Nat. Plants 2018, 4, 576–585. [Google Scholar] [CrossRef]
- De la Concepcion, J.C.; Maidment, J.H.R.; Longya, A.; Xiao, G.; Franceschetti, M.; Banfield, M.J. The allelic rice immune receptor Pikh confers extended resistance to strains of the blast fungus through a single polymorphism in the effector binding interface. PLoS Pathog. 2021, 17, e1009368. [Google Scholar] [CrossRef]
- Maidment, J.H.R.; Franceschetti, M.; Maqbool, A.; Saitoh, H.; Jantasuriyarat, C.; Kamoun, S.; Terauchi, R.; Banfield, M.J. Multiple variants of the fungal effector AVR-Pik bind the HMA domain of the rice protein OsHIPP19, providing a foundation to engineer plant defense. J. Biol. Chem. 2021, 296, 100371. [Google Scholar] [CrossRef] [PubMed]
- Bentham, A.R.; Petit-Houdenot, Y.; Win, J.; Terauchi, R.; Banfield, M.J.; Kamoun, S.; Langner, T. A single amino acid polymorphism in a conserved effector of the multihost blast fungus pathogen expands host-target binding spectrum. PLoS Pathog. 2021, 17, e1009957. [Google Scholar] [CrossRef]
- Ou, S.H. Pathogen Variability and Host Resistance in Rice Blast Disease. Annu. Rev. Phytopathol. 1980, 18, 167–187. [Google Scholar] [CrossRef]
- Lo Presti, L.; Lanver, D.; Schweizer, G.; Tanaka, S.; Liang, L.; Tollot, M.; Zuccaro, A.; Reissmann, S.; Kahmann, R. Fungal Effectors and Plant Susceptibility. Annu. Rev. Plant Biol. 2015, 66, 513–545. [Google Scholar] [CrossRef] [PubMed]
- Seong, K.; Krasileva, K.V. Prediction of effector protein structures from fungal phytopathogens enables evolutionary analyses. Nat. Microbiol. 2023, 8, 174–187. [Google Scholar] [CrossRef]
- Le Naour-Vernet, M.L.; Charriat, F.; Gracy, J.; Cros-Arteil, S.; Ravel, S.; Veillet, F.; Meusnier, I.; Padilla, A.; Kroj, T.; Cesari, S.; et al. Adaptive Evolution in Virulence Effectors of the Rice Blast Fungus Pyricularia oryzae. bioRxiv 2023. [Google Scholar] [CrossRef]
- Yan, X.; Tang, B.; Ryder, L.S.; MacLean, D.; Were, V.M.; Eseola, A.B.; Cruz-Mireles, N.; Ma, W.; Forster, A.J.; Osés-Ruiz, M.; et al. The transcriptional landscape of plant infection by the rice blast fungus Magnaporthe oryzae reveals distinct families of temporally co-regulated and structurally conserved effectors. Plant Cell. 2023, 35, 1360–1385. [Google Scholar] [CrossRef]
- Seong, K.; Krasileva, K.V. Computational Structural Genomics Unravels Common Folds and Novel Families in the Secretome of Fungal Phytopathogen Magnaporthe oryzae. Mol. Plant Microbe Interact. 2021, 34, 1267–1280. [Google Scholar] [CrossRef]
- Dubois, C.; Lahfa, M.; Pissarra, J.; de Guillen, K.; Barthe, P.; Kroj, T.; Roumestand, C.; Padilla, A. Combining High-Pressure NMR and Geometrical Sampling to Obtain a Full Topological Description of Protein Folding Landscapes: Application to the Folding of Two MAX Effectors from Magnaporthe oryzae. Int. J. Mol. Sci. 2022, 23, 5461. [Google Scholar] [CrossRef]
- Güntert, P. Automated NMR structure calculation with CYANA. Methods Mol. Biol. 2004, 278, 353–378. [Google Scholar] [CrossRef] [PubMed]
- Van Deuren, V.; Yang, Y.S.; de Guillen, K.; Dubois, C.; Royer, C.A.; Roumestand, C.; Barthe, P. Comparative assessment of NMR probes for the experimental description of protein folding pathways with High-Pressure NMR. Biology 2021, 10, 656. [Google Scholar] [CrossRef] [PubMed]
- Yamada, H. Pressure-resisting glass cell for high-pressure, high resolution NMR measurements. Rev. Sci. Instrum. 1974, 45, 640–642. [Google Scholar] [CrossRef]
- Yamada, H.; Nishikawa, K.; Honda, M.; Shimura, T.; Akasaka, K.; Tabayashi, K. Pressure-resisting cell for high-pressure, high-resolution nuclear magnetic resonance measurements at very high magnetic fields. Rev. Sci. Instrum. 2001, 72, 1463–1471. [Google Scholar] [CrossRef]
- Akasaka, K. Probing conformational fluctuations of proteins by pressure perturbation. Chem. Rev. 2006, 106, 1814–1835. [Google Scholar] [CrossRef]
- Lassalle, M.W.; Akasaka, K. The use of high-pressure nuclear magnetic resonance to study protein folding. Methods Mol. Biol. 2007, 350, 21–38. [Google Scholar] [CrossRef]
- Roche, J.; Royer, C.A.; Roumestand, C. Exploring Protein Conformational Landscapes Using High-Pressure NMR. Methods Enzymol. 2019, 614, 293–320. [Google Scholar] [CrossRef]
- Le Châtelier, H. Sur un énoncé global des lois des équilibres chimiques. Comptes-Rendus L’Académie Sci. 1884, 99, 786–789. [Google Scholar]
- Royer, C.A. Revisiting volume changes in pressure-induced protein unfolding. Biochim. Biophys. Acta 2002, 1595, 201–209. [Google Scholar] [CrossRef] [PubMed]
- Royer, C.A. Why and How Does Pressure Unfold Proteins? Subcell. Biochem. 2015, 72, 59–71. [Google Scholar] [CrossRef]
- Rouget, J.; Aksel, T.; Roche, J.; Saldana, J.; Garcia, A.E.; Barrick, D.; Royer, C.A. Size and sequence and the volume change of protein folding. J. Am. Chem. Soc. 2011, 133, 6020–6027. [Google Scholar] [CrossRef] [PubMed]
- Roche, J.; Caro, J.A.; Norberto, D.R.; Barthe, P.; Roumestand, C.; Schlessman, J.L.; Garcia, A.E.; García-Moreno, B.E.; Royer, C.A. Cavities determine the pressure unfolding of proteins. Proc. Natl. Acad. Sci. USA 2012, 109, 6945–6950. [Google Scholar] [CrossRef]
- Roche, J.; Dellarole, M.J.; Caro, A.; Guca, E.; Norberto, D.R.; Yang, Y.-S.; Garcia, A.E.; Roumestand, C.; García-Moreno, B.; Royer, C.A. Remodeling of the folding free-energy landscape of staphylococcal nuclease by cavity-creating mutations. Biochemistry 2012, 51, 9535–9546. [Google Scholar] [CrossRef] [PubMed]
- Lahfa, M.; Padilla, A.; de Guillen, K.; Pissarra, J.; Raji, M.; Cesari, S.; Kroj, T.; Gladieux, P.; Roumestand, C.; Barthe, P. The NMR Structure of the MAX60 Effector from Magnaporthe oryzae. 2022. Available online: https://www.rcsb.org/structure/7ZK0 (accessed on 15 December 2022).
- Lahfa, M.; Padilla, A.; de Guillen, K.; Pissarra, J.; Raji, M.; Cesari, S.; Kroj, T.; Gladieux, P.; Roumestand, C.; Barthe, P. 1H, 13C, 15 N backbone and side-chain NMR assignments for three MAX effectors from Magnaporthe oryzae. Biomol. NMR Assign. 2022, 16, 305–309. [Google Scholar] [CrossRef] [PubMed]
- Varden, F.A.; Saitoh, H.; Yoshino, K.; Franceschetti, M.; Kamoun, S.; Terauchi, R.; Banfield, M.J. Cross-reactivity of a rice NLR immune receptor to distinct effectors from the rice blast pathogen Magnaporthe oryzae provides partial disease resistance. J. Biol. Chem. 2019, 294, 13006–13016. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Skolnick, J. TM-align: A protein structure alignment algorithm based on TM-score. Nucleic Acids Res. 2005, 33, 2302–2309. [Google Scholar] [CrossRef] [PubMed]
- Lipari, G.; Szabo, A. Model-free approach to the interpretation of nuclear magnetic resonance relaxation in macromolecules. J. Am. Chem. Soc. 1982, 104, 4546–4559. [Google Scholar] [CrossRef]
- Clore, G.M.; Driscoll, P.C.; Wingfield, P.T.; Gronenborn, A.M. Analysis of the backbone dynamics of interleukin-1 beta using two-dimensional inverse detected heteronuclear 15N-1H NMR spectroscopy. Biochemistry 1990, 29, 7387–7401. [Google Scholar] [CrossRef] [PubMed]
- Barthe, P.; Chiche, L.; Declerck, N.; Delsuc, M.A.; Lefèvre, J.F.; Malliavin, T.; Mispelter, J.; Stern, M.-H.; Lhoste, J.M.; Roumestand, C. Refined Solution Structure and Backbone Dynamics of 15N-Labeled C12A-p8MTCP1 Studied by NMR Relaxation. J. Biomol. NMR 1999, 15, 271–288. [Google Scholar] [CrossRef] [PubMed]
- Barthe, P.; Roumestand, C.; Déméné, H.; Chiche, L. Helix motion in protein C12A-p8(MTCP1): Comparison of molecular dynamics simulations and multifield NMR relaxation data. J. Comput. Chem. 2002, 16, 1577–1586. [Google Scholar] [CrossRef]
- Bai, Y.; Milne, J.S.; Mayne, L.; Englander, S.W. Primary structure effects on peptide group hydrogen exchange. Proteins 1993, 17, 75–86. [Google Scholar] [CrossRef]
- Fossat, M.J.; Dao, T.P.; Jenkins, K.; Dellarole, M.; Yang, Y.-S.; McCallum, S.A.; Garcia, A.E.; Barrick, D.; Roumestand, C.; Royer, C.A. High-Resolution Mapping of a Repeat Protein Folding Free Energy Landscape. Biophys. J. 2016, 111, 2368–2376. [Google Scholar] [CrossRef]
- Vehlow, C.; Stehr, H.; Winkelmann, M.; Duarte, J.M.; Petzold, L.; Dinse, J.; Lappe, M. CMView: Interactive contact map visualization and analysis. Bioinformatics 2011, 27, 1573–1574. [Google Scholar] [CrossRef]
- Herrada, I.; Barthe, P.; Vanheusden, M.; DeGuillen, K.; Mammri, L.; Delbecq, S.; Rico, F.; Roumestand, C. Monitoring Unfolding of Titin I27 Single and Bi Domain with High-Pressure NMR Spectroscopy. Biophys. J. 2018, 115, 341–352. [Google Scholar] [CrossRef] [PubMed]
- Saotome, T.; Doret, M.; Kulkarni, M.; Yang, Y.-S.; Barthe, P.; Kuroda, Y.; Roumestand, C. Folding of the Ig-Like Domain of the Dengue Virus Envelope Protein Analyzed by High-Hydrostatic-Pressure NMR at a Residue-Level Resolution. Biomolecules 2019, 9, 309. [Google Scholar] [CrossRef]
- Sattler, M.; Schleucher, J.; Griesinger, C. Heteronuclear multi-dimensional NMR experiments for the structure determination of proteins in solution employing pulsed field gradients. Prog. Nucl. Magn. Reson. Spectrosc. 1999, 34, 93–158. [Google Scholar] [CrossRef]
- Nederveen, A.J.; Doreleijers, J.F.; Vranken, W.; Miller, Z.; Spronk, C.A.; Nabuurs, S.B.; Güntert, P.; Livny, M.; Markley, J.L.; Nilges, M.; et al. RECOORD: A recalculated coordinate database of 500+ proteins from the PDB using restraints from the BioMagResBank. Proteins 2005, 59, 662–672. [Google Scholar] [CrossRef] [PubMed]
- Laskowski, R.A.; Moss, D.S.; Thornton, J.M. Main-chain bond lengths and bond angles in protein structures. J. Mol. Biol. 1993, 231, 1049–1067. [Google Scholar] [CrossRef] [PubMed]
- Peng, J.W.; Wagner, G. Mapping of the spectral densities of N-H bond motion in eglin c using heteronuclear relaxation experiments. Biochemistry 1992, 31, 8571–8586. [Google Scholar] [CrossRef]
- Peng, J.W.; Wagner, G. Mapping of the spectral density functions using heteronuclear NMR relaxation experiments. J. Magn. Reson. 1992, 98, 308–332. [Google Scholar] [CrossRef][Green Version]
- Kay, L.E.; Nicholson, L.K.; Delaglio, F.; Bax, A.; Torchia, D.A. Pulse sequences for removal of the effects of cross correlation between dipolar and chemical-shift anisotropy relaxation mechanisms on the measurement of heteronuclear T1 and T2 values in proteins. J. Magn. Reson. 1992, 97, 359–375. [Google Scholar] [CrossRef]
- Canet, D.; Barthe, P.; Mutzenhardt, P.; Roumestand, C. A comprehensive analysis of multifield 15N relaxation parameters in proteins: Determination of 15N chemical shift anisotropy. J. Am. Chem. Soc. 2001, 123, 4567–4576. [Google Scholar] [CrossRef] [PubMed]
- Bax, A.; Pochapsky, S.S. Optimized recording of heteronuclear multidimensional NMR spectra using pulsed field gradients. J. Magn. Reson. 1992, 99, 638–643. [Google Scholar] [CrossRef]
- Piotto, M.; Saudek, V.; Sklenar, V. Gradient-tailored excitation for single-quantum NMR spectroscopy of aqueous solutions. J. Biomol. NMR 1992, 2, 661–665. [Google Scholar] [CrossRef]
- Carr, H.Y.; Purcell, E.M. Effects of Diffusion on Free Precession in Nuclear Magnetic Resonance Experiments. Phys. Rev. 1954, 94, 630–638. [Google Scholar] [CrossRef]
- Meilboom, S.; Gill, G. Modified SpinEcho Method for Measuring Nuclear Relaxation Times. Rev. Sci. Instrum. 1958, 29, 688–691. [Google Scholar] [CrossRef]
- Kay, L.E.; Torchia, D.A.; Bax, A. Backbone dynamics of proteins as studied by 15N inverse detected heteronuclear NMR spectroscopy: Application to staphylococcal nuclease. Biochemistry 1989, 28, 8972–8973. [Google Scholar] [CrossRef] [PubMed]
- Grzesiek, S.; Bax, A. The importance of not saturating water in protein NMR. Application to sensitivity enhancement and NOE measurements. J. Am. Chem. Soc. 1995, 115, 12593–12594. [Google Scholar] [CrossRef]
- Sklenar, V.J. Suppression of Radiation Damping in Multidimensional NMR Experiments Using Magnetic Field Gradients. J. Magn. Reson. Ser. A 1995, 114, 132–135. [Google Scholar] [CrossRef]
- Pons, J.L.; Malliavin, T.E.; Delsuc, M.A. Gifa V.4: A complete package for NMR data set processing. J. Biomol. NMR 1996, 8, 445–452. [Google Scholar] [CrossRef]
- Skelton, N.J.; Palmer, A.G.; Akke, M.; Kördel, J.; Rance, M.; Chazin, W.J. Practical aspects of two-dimensional proton-detected 15N spin relaxation measurements. J. Magn. Reson. Ser. B 1993, 102, 253–264. [Google Scholar] [CrossRef]
- Press, W.H.; Flannery, B.P.; Teukolsky, S.A.; Vetterling, W.T. Numerical Recipes; Cambridge University Press: Cambridge, UK, 1986. [Google Scholar]
- Abragam, A. Principles of Nuclear Magnetism, Oxford, Science Publication; Clarendon Press: Oxford, UK, 1961. [Google Scholar]
- Farrow, N.A.; Zhang, O.; Szabo, A.; Torchia, D.A.; Kay, L.E. Spectral density function mapping using 15N relaxation data exclusively. J. Biomol. NMR 1995, 6, 153–162. [Google Scholar] [CrossRef]
- Ishima, R.; Nagayama, K. Protein backbone dynamics revealed by quasi spectral density function analysis of amide N-15 nuclei. Biochemistry 1995, 34, 3162–3171. [Google Scholar] [CrossRef]
- Ishima, R.; Nagayama, K. Quasi-spectral-density function analysis for nitrogen-15 nuclei in proteins. J. Magn. Reson. B 1995, 108, 73–76. [Google Scholar] [CrossRef]
- Lefèvre, J.-F.; Dayie, K.T.; Peng, J.W.; Wagner, G. Internal mobility in the partially folded DNA binding and dimerization domains of GAL4: NMR analysis of the N-H spectral density functions. Biochemistry 1996, 35, 2674–2686. [Google Scholar] [CrossRef] [PubMed]
- Tjandra, N.; Szabo, A.; Bax, A. Protein backbone dynamics and 15N chemical)shift anisotropy from quantitative measurement of relaxation interference effects. J. Am. Chem. Soc. 1996, 118, 6986–6991. [Google Scholar] [CrossRef]
- Habazettl, J.; Wagner, G. A new simplified method for analyzing Nitrogen-15 Nuclear Magnetic Relaxation data of proteins. J. Mag. Res. B 1995, 109, 100–104. [Google Scholar] [CrossRef]
- Vis, H.; Vorgias, C.E.; Wilson, K.S.; Kaptein, R.; Boelens, R.J. Mobility of NH bonds in DNA-binding protein HU of shape Bacillus stearothermophilus from reduced spectral density mapping analysis at multiple NMR fields. J. Biomol. NMR 1998, 11, 265–277. [Google Scholar] [CrossRef]
- Barthe, P.; Ropars, V.; Roumestand, C. DYNAMOF: A program for the dynamics analysis of relaxation data obtained at multiple magnetic fields. C. R. Chim. 2006, 9, 503–513. [Google Scholar] [CrossRef]
- Orekhov, V.Y.; Pervushin, K.V.; Korzhnev, D.M.; Arseniev, A.S. Backbone dynamics of (1-71)- and (1-36)bacterioopsin studied by two-dimensional (1)H-(15)N NMR spectroscopy. J. Biomol. NMR 1995, 6, 113–122. [Google Scholar] [CrossRef] [PubMed]
- Korzhnev, D.M.; Orekhov, V.Y.; Arseniev, A.S. Model-free approach beyond the borders of its applicability. J. Magn. Reson. 1997, 127, 184–191. [Google Scholar] [CrossRef]
- Fedyukina, D.V.; Cavagnero, S. Protein folding at the exit tunnel. Annu. Rev. Biophys. 2011, 40, 337–359. [Google Scholar] [CrossRef] [PubMed]
- Cabrita, L.D.; Dobson, C.M.; Christodoulou, J. Protein folding on the ribosome. Curr. Opin. Struct. Biol. 2010, 20, 33–45. [Google Scholar] [CrossRef]
- Gershenson, A.; Gierasch, L.M. Protein folding in the cell: Challenges and progress. Curr. Opin. Struct. Biol. 2011, 21, 32–41. [Google Scholar] [CrossRef]
- Kaiser, C.M.; Goldman, D.H.; Chodera, J.D.; Tinoco, I.; Bustamante, C. The ribosome modulates nascent protein folding. Science 2011, 334, 1723–1727. [Google Scholar] [CrossRef] [PubMed]
- Waudby, C.A.; Launay, H.; Cabrita, L.D.; Christodoulou, J. Protein folding on the ribosome studied using NMR spectroscopy. Prog. Nucl. Magn. Reson. Spectrosc. 2013, 74, 57–75. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lahfa, M.; Mouhand, A.; de Guillen, K.; Barthe, P.; Kroj, T.; Padilla, A.; Roumestand, C. Does a Similar 3D Structure Mean a Similar Folding Pathway? The Presence of a C-Terminal α-Helical Extension in the 3D Structure of MAX60 Drastically Changes the Folding Pathway Described for Other MAX-Effectors from Magnaporthe oryzae. Molecules 2023, 28, 6068. https://doi.org/10.3390/molecules28166068
Lahfa M, Mouhand A, de Guillen K, Barthe P, Kroj T, Padilla A, Roumestand C. Does a Similar 3D Structure Mean a Similar Folding Pathway? The Presence of a C-Terminal α-Helical Extension in the 3D Structure of MAX60 Drastically Changes the Folding Pathway Described for Other MAX-Effectors from Magnaporthe oryzae. Molecules. 2023; 28(16):6068. https://doi.org/10.3390/molecules28166068
Chicago/Turabian StyleLahfa, Mounia, Assia Mouhand, Karine de Guillen, Philippe Barthe, Thomas Kroj, André Padilla, and Christian Roumestand. 2023. "Does a Similar 3D Structure Mean a Similar Folding Pathway? The Presence of a C-Terminal α-Helical Extension in the 3D Structure of MAX60 Drastically Changes the Folding Pathway Described for Other MAX-Effectors from Magnaporthe oryzae" Molecules 28, no. 16: 6068. https://doi.org/10.3390/molecules28166068
APA StyleLahfa, M., Mouhand, A., de Guillen, K., Barthe, P., Kroj, T., Padilla, A., & Roumestand, C. (2023). Does a Similar 3D Structure Mean a Similar Folding Pathway? The Presence of a C-Terminal α-Helical Extension in the 3D Structure of MAX60 Drastically Changes the Folding Pathway Described for Other MAX-Effectors from Magnaporthe oryzae. Molecules, 28(16), 6068. https://doi.org/10.3390/molecules28166068






