Bragg–Williams Theory for Particles with a Size-Modulating Internal Degree of Freedom
Abstract
1. Introduction
2. Theory
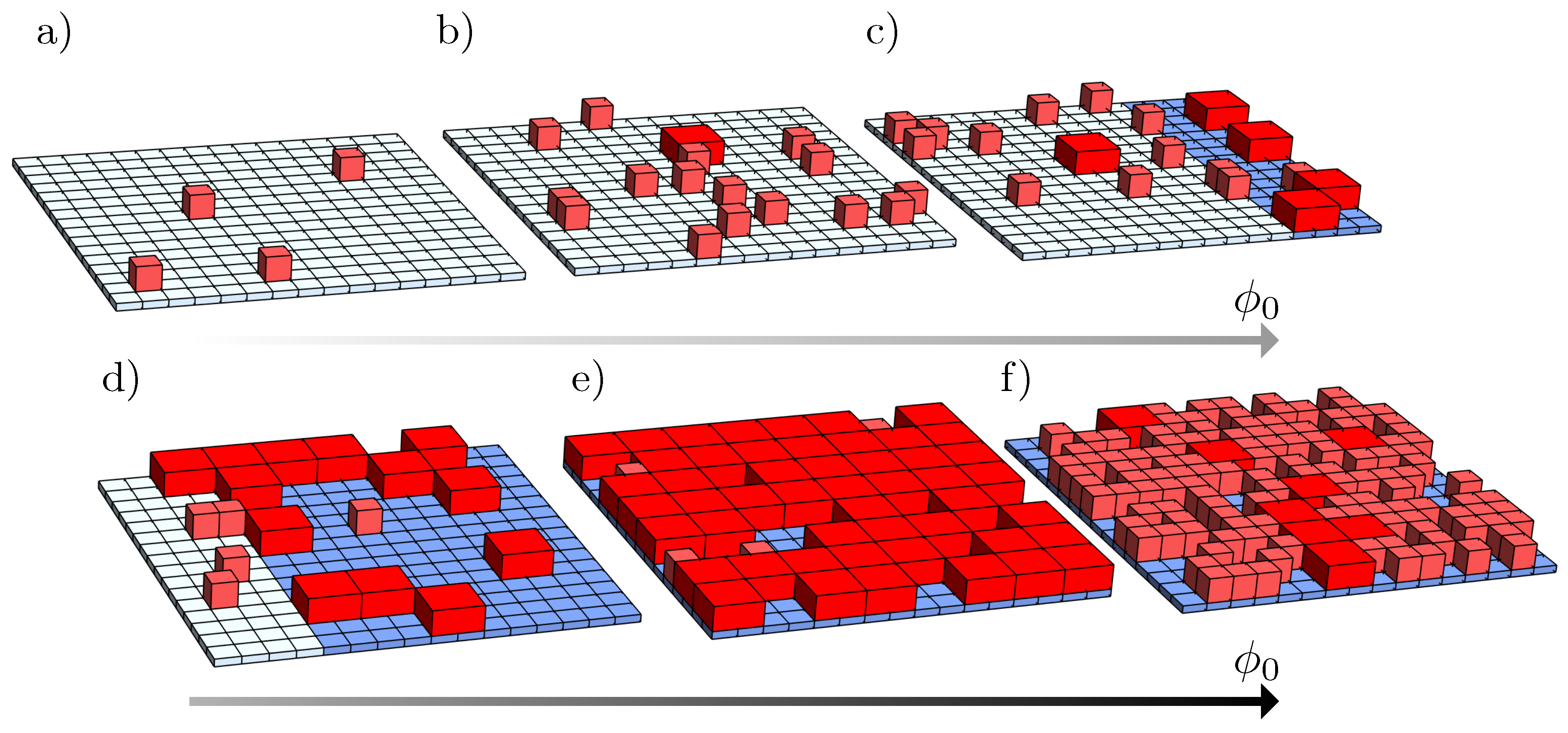
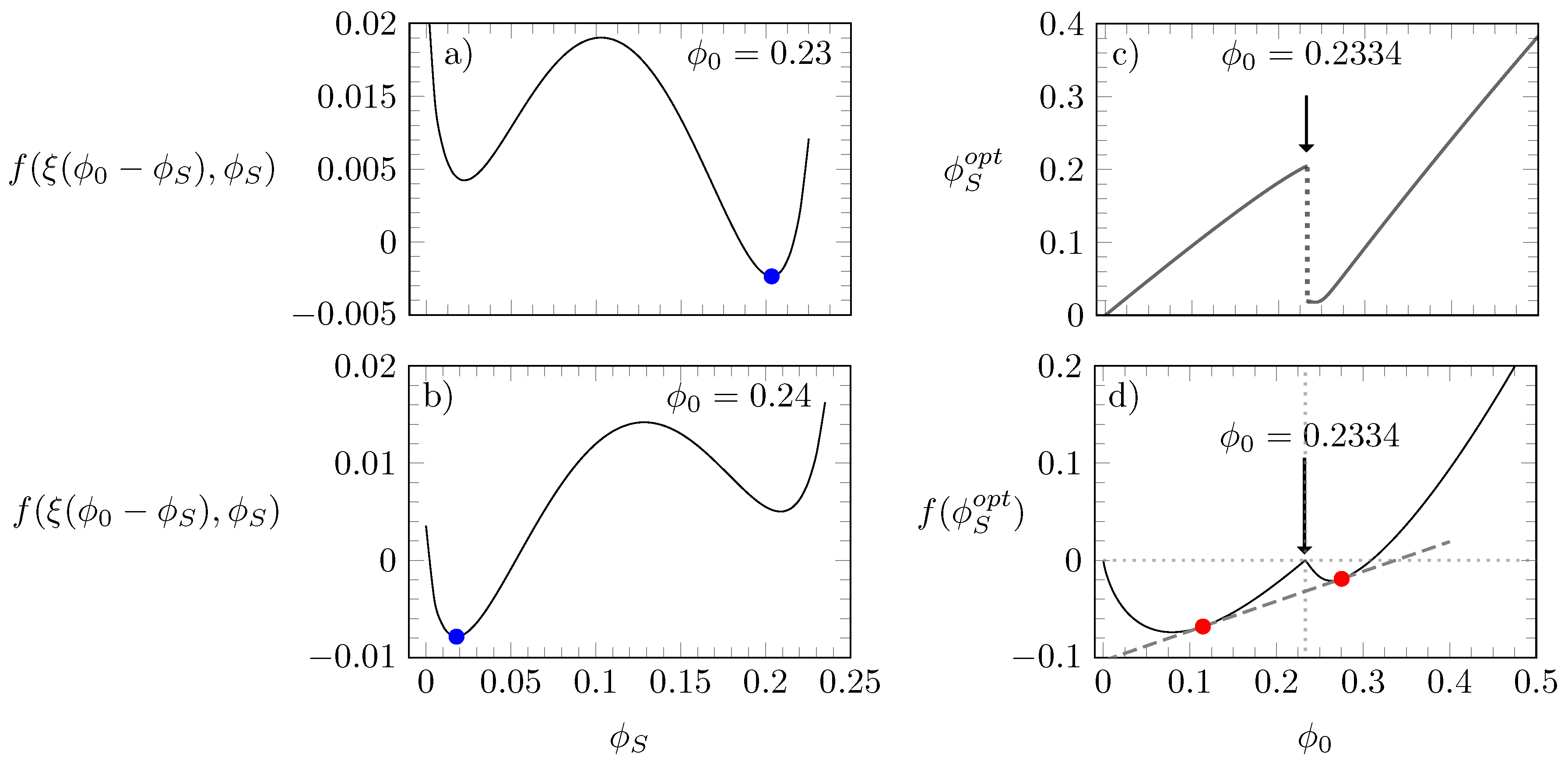
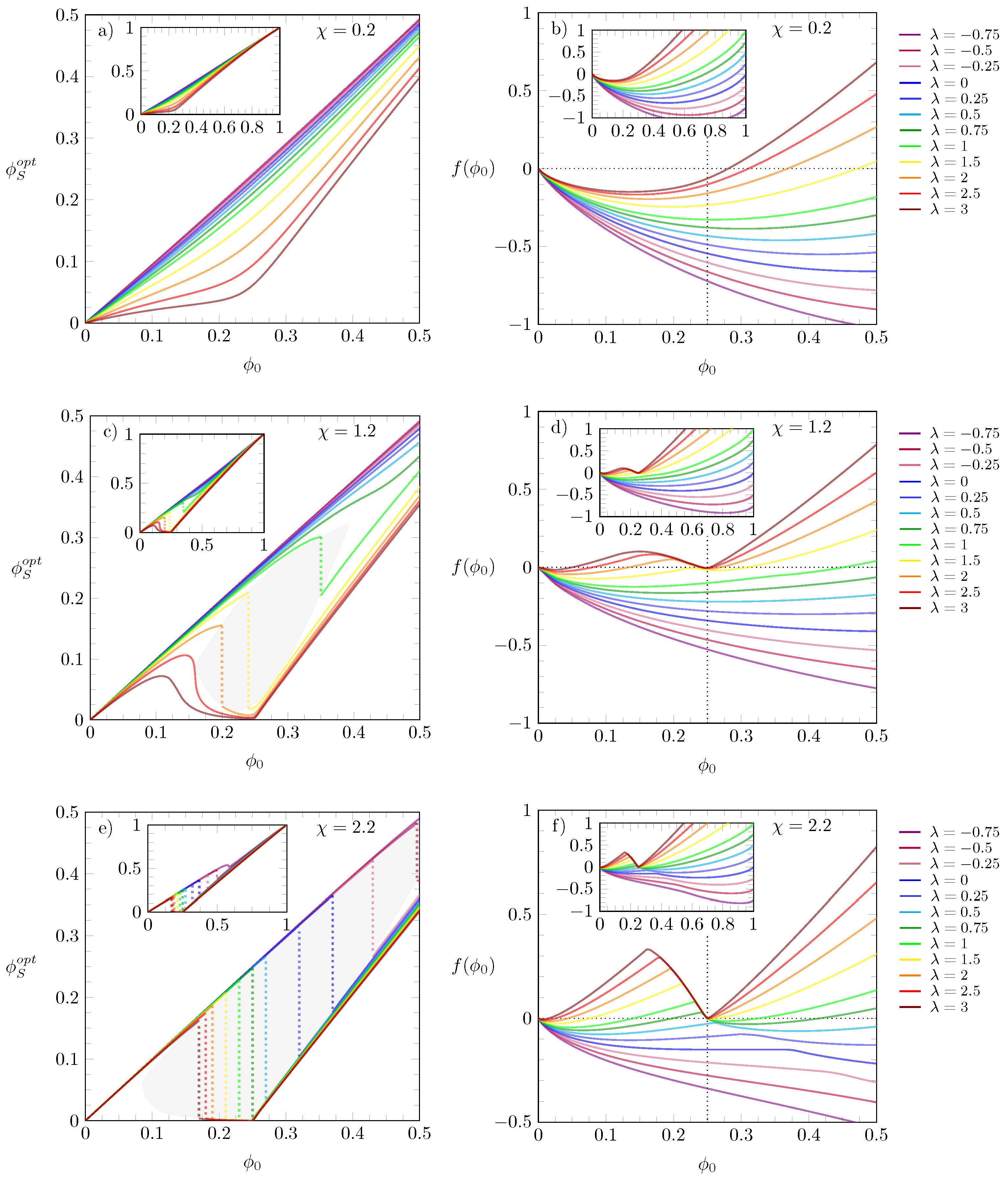
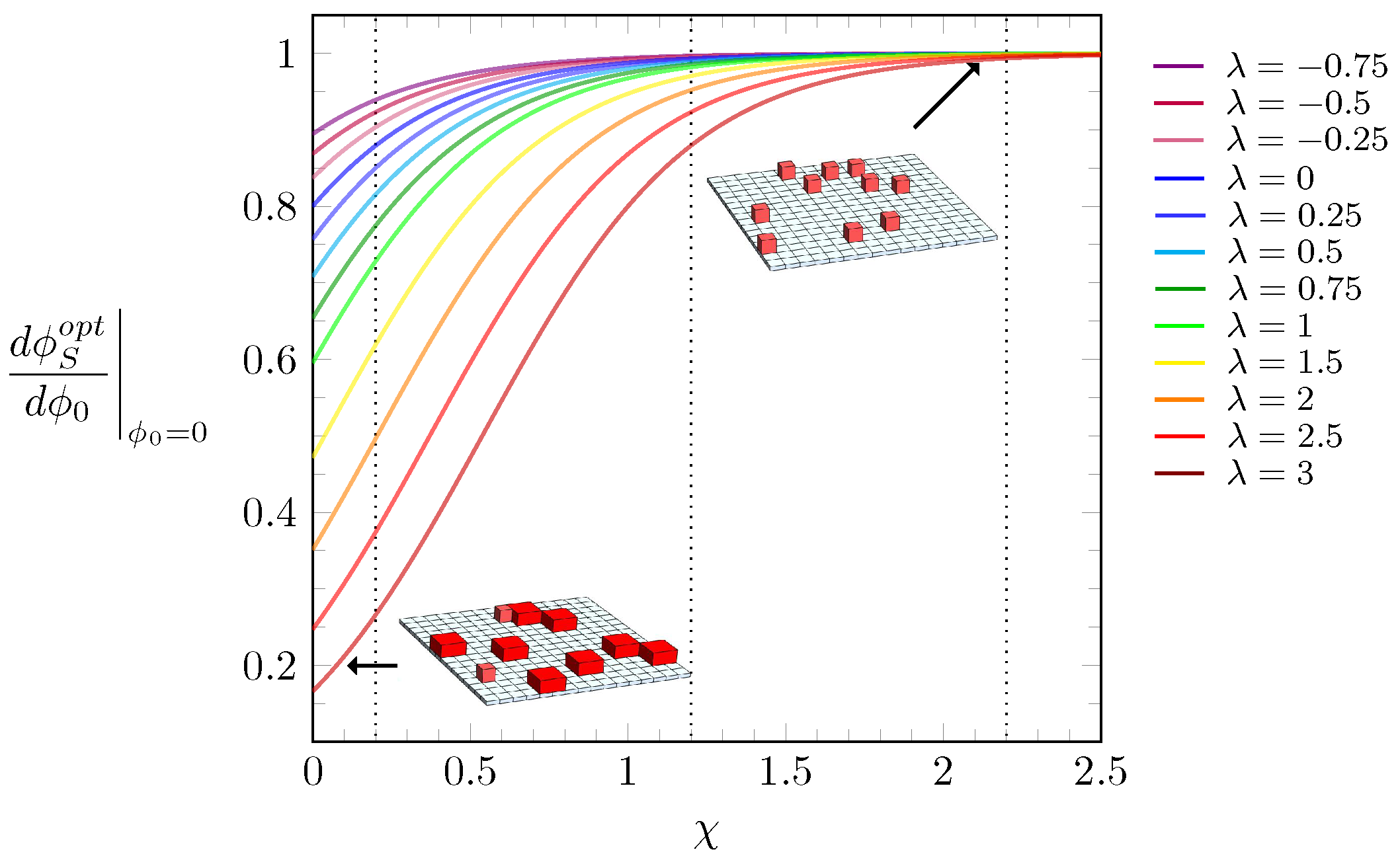
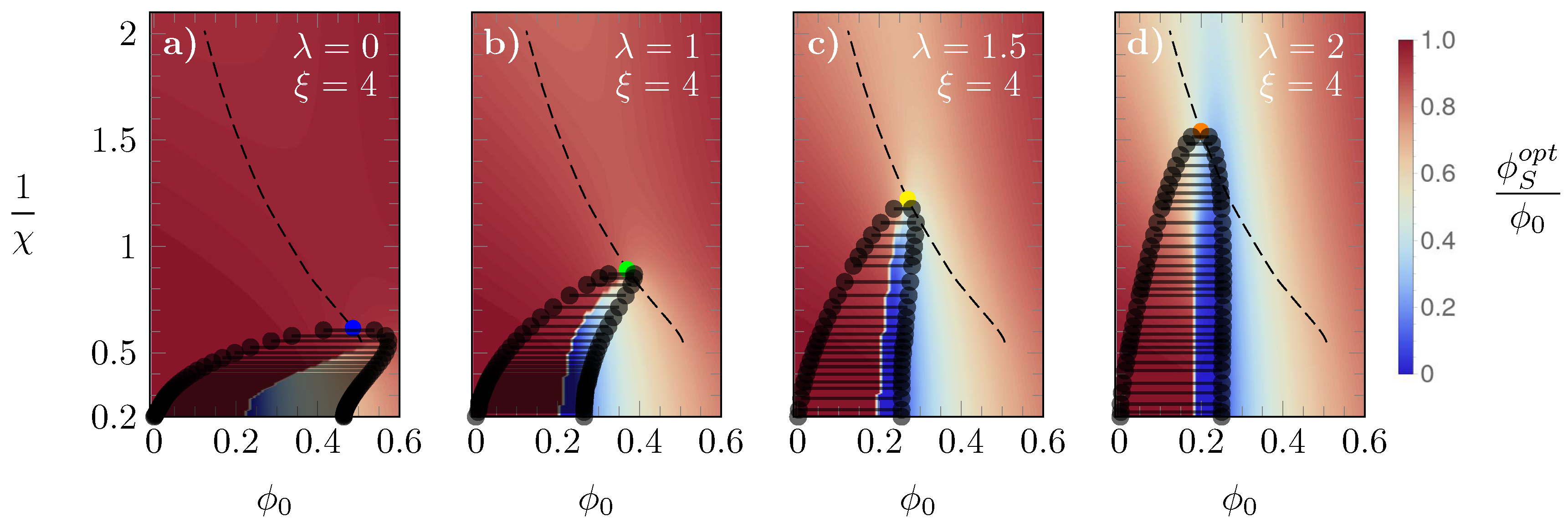
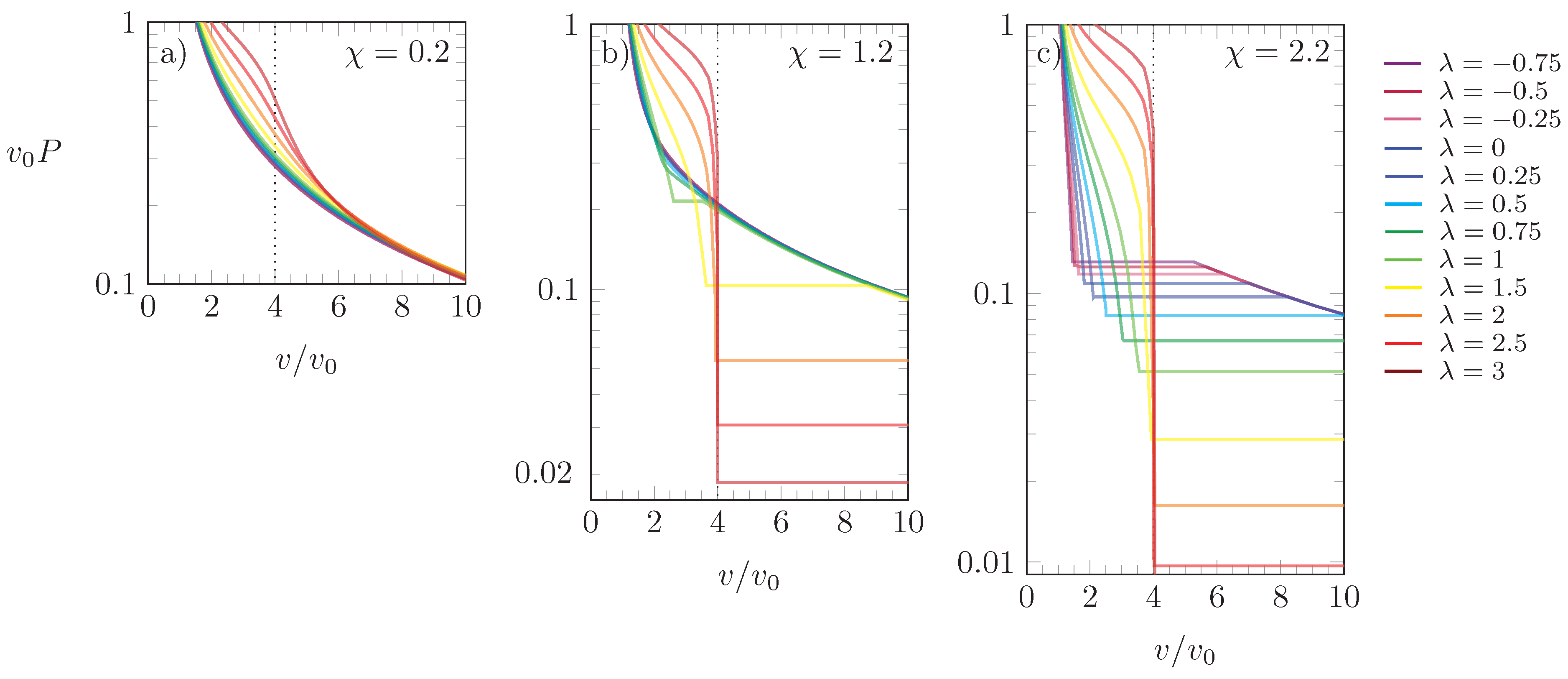
3. Results and Discussion
4. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Baoukina, S.; Monticelli, L.; Marrink, S.J.; Tieleman, D.P. Pressure- area isotherm of a lipid monolayer from molecular dynamics simulations. Langmuir 2007, 23, 12617–12623. [Google Scholar] [CrossRef]
- Blanco, E.; Pineiro, A.; Miller, R.; Ruso, J.M.; Prieto, G.; Sarmiento, F. Langmuir monolayers of a hydrogenated/fluorinated catanionic surfactant: From the macroscopic to the nanoscopic size scale. Langmuir 2009, 25, 8075–8082. [Google Scholar] [CrossRef] [PubMed]
- Javanainen, M.; Lamberg, A.; Cwiklik, L.; Vattulainen, I.; Ollila, O.S. Atomistic model for nearly quantitative simulations of Langmuir monolayers. Langmuir 2018, 34, 2565–2572. [Google Scholar] [CrossRef] [PubMed]
- Ermakov, Y.A.; Asadchikov, V.; Roschin, B.; Volkov, Y.O.; Khomich, D.; Nesterenko, A.; Tikhonov, A. Comprehensive study of the liquid expanded–liquid condensed phase transition in 1, 2-dimyristoyl-sn-glycero-3-phospho-L-serine monolayers: Surface pressure, Volta potential, X-ray reflectivity, and molecular dynamics modeling. Langmuir 2019, 35, 12326–12338. [Google Scholar] [CrossRef] [PubMed]
- Linse, P.; Bjoerling, M. Lattice theory for multicomponent mixtures of copolymers with internal degrees of freedom in heterogeneous systems. Macromolecules 1991, 24, 6700–6711. [Google Scholar] [CrossRef]
- Karnieli, A.; Markovich, T.; Andelman, D. Surface pressure of charged colloids at the air/water interface. Langmuir 2018, 34, 13322–13332. [Google Scholar] [CrossRef]
- Agudelo, J.; Bossa, G.V.; May, S. Incorporation of Molecular Reorientation into Modeling Surface Pressure-Area Isotherms of Langmuir Monolayers. Molecules 2021, 26, 4372. [Google Scholar] [CrossRef]
- Walter, J.; Sehrt, J.; Vrabec, J.; Hasse, H. Molecular dynamics and experimental study of conformation change of poly (N-isopropylacrylamide) hydrogels in mixtures of water and methanol. J. Phys. Chem. B 2012, 116, 5251–5259. [Google Scholar] [CrossRef]
- Culver, H.R.; Clegg, J.R.; Peppas, N.A. Analyte-responsive hydrogels: Intelligent materials for biosensing and drug delivery. Acc. Chem. Res. 2017, 50, 170–178. [Google Scholar] [CrossRef]
- Cilla, S.; Floría, L. Internal degrees of freedom in a thermodynamical model for intracell biological transport. Phys. D 1998, 113, 157–161. [Google Scholar] [CrossRef]
- Guigas, G.; Weiss, M. Size-dependent diffusion of membrane inclusions. Biophys. J. 2006, 91, 2393–2398. [Google Scholar] [CrossRef]
- Ilie, I.M.; den Otter, W.K.; Briels, W.J. A coarse grained protein model with internal degrees of freedom. Application to α-synuclein aggregation. J. Chem. Phys. 2016, 144, 085103. [Google Scholar] [CrossRef] [PubMed]
- Kumaki, J.; Hashimoto, T. Conformational change in an isolated single synthetic polymer chain on a mica surface observed by atomic force microscopy. J. Am. Chem. Soc. 2003, 125, 4907–4917. [Google Scholar] [CrossRef] [PubMed]
- Shen, X.; Viney, C.; Johnson, E.R.; Wang, C.; Lu, J.Q. Large negative thermal expansion of a polymer driven by a submolecular conformational change. Nat. Chem. 2013, 5, 1035–1041. [Google Scholar] [CrossRef] [PubMed]
- Yefimov, S.; Van der Giessen, E.; Onck, P.R.; Marrink, S.J. Mechanosensitive membrane channels in action. Biophys. J. 2008, 94, 2994–3002. [Google Scholar] [CrossRef] [PubMed]
- Rasmussen, T.; Flegler, V.J.; Rasmussen, A.; Böttcher, B. Structure of the mechanosensitive channel MscS embedded in the membrane bilayer. J. Mol. Biol. 2019, 431, 3081–3090. [Google Scholar] [CrossRef]
- Zhang, Y.; Daday, C.; Gu, R.X.; Cox, C.D.; Martinac, B.; de Groot, B.L.; Walz, T. Visualization of the mechanosensitive ion channel MscS under membrane tension. Nature 2021, 590, 509–514. [Google Scholar] [CrossRef]
- Thorne, J.B.; Vine, G.J.; Snowden, M.J. Microgel applications and commercial considerations. Colloid Polym. Sci. 2011, 289, 625–646. [Google Scholar] [CrossRef]
- Urich, M.; Denton, A.R. Swelling, structure, and phase stability of compressible microgels. Soft Matter 2016, 12, 9086–9094. [Google Scholar] [CrossRef]
- Marcisz, K.; Mackiewicz, M.; Romanski, J.; Stojek, Z.; Karbarz, M. Significant, reversible change in microgel size using electrochemically induced volume phase transition. Appl. Mater. Today 2018, 13, 182–189. [Google Scholar] [CrossRef]
- Butler, M.D.; Montenegro-Johnson, T.D. The swelling and shrinking of spherical thermo-responsive hydrogels. J. Fluid Mech. 2022, 947, A11. [Google Scholar] [CrossRef]
- Reese, C.E.; Mikhonin, A.V.; Kamenjicki, M.; Tikhonov, A.; Asher, S.A. Nanogel nanosecond photonic crystal optical switching. J. Am. Chem. Soc. 2004, 126, 1493–1496. [Google Scholar] [CrossRef] [PubMed]
- Giner-Casares, J.J.; Brezesinski, G.; Möhwald, H. Langmuir monolayers as unique physical models. Curr. Opin. Colloid Interface Sci. 2014, 19, 176–182. [Google Scholar] [CrossRef]
- Stefaniu, C.; Brezesinski, G.; Möhwald, H. Langmuir monolayers as models to study processes at membrane surfaces. Adv. Colloid Interface Sci. 2014, 208, 197–213. [Google Scholar] [CrossRef] [PubMed]
- Oliveira, O.N., Jr.; Caseli, L.; Ariga, K. The past and the future of Langmuir and Langmuir–Blodgett films. Chem. Rev. 2022, 122, 6459–6513. [Google Scholar] [CrossRef] [PubMed]
- McConnell, H.M.; Radhakrishnan, A. Condensed complexes of cholesterol and phospholipids. Biochim. Biophys. Acta Biomembr. 2003, 1610, 159–173. [Google Scholar] [CrossRef]
- Jurak, M. Thermodynamic aspects of cholesterol effect on properties of phospholipid monolayers: Langmuir and Langmuir–Blodgett monolayer study. J. Phys. Chem. B 2013, 117, 3496–3502. [Google Scholar] [CrossRef]
- Janich, C.; Hädicke, A.; Bakowsky, U.; Brezesinski, G.; Wölk, C. Interaction of DNA with Cationic Lipid Mixtures: Investigation at Langmuir Lipid Monolayers. Langmuir 2017, 33, 10172–10183. [Google Scholar] [CrossRef]
- Luque-Caballero, G.; Maldonado-Valderrama, J.; Quesada-Pérez, M.; Martín-Molina, A. Interaction of DNA with likely-charged lipid monolayers: An experimental study. Colloids Surf. B 2019, 178, 170–176. [Google Scholar] [CrossRef]
- Nobre, T.M.; Pavinatto, F.J.; Caseli, L.; Barros-Timmons, A.; Dynarowicz-Łątka, P.; Oliveira, O.N., Jr. Interactions of bioactive molecules & nanomaterials with Langmuir monolayers as cell membrane models. Thin Solid Films 2015, 593, 158–188. [Google Scholar]
- Rojewska, M.; Smułek, W.; Kaczorek, E.; Prochaska, K. Langmuir Monolayer Techniques for the Investigation of Model Bacterial Membranes and Antibiotic Biodegradation Mechanisms. Membranes 2021, 11, 707. [Google Scholar] [CrossRef]
- Salay, L.C.; Ferreira, M.; Oliveira, O.N., Jr.; Nakaie, C.R.; Schreier, S. Headgroup specificity for the interaction of the antimicrobial peptide tritrpticin with phospholipid Langmuir monolayers. Colloids Surf. B 2012, 100, 95–102. [Google Scholar] [CrossRef]
- Martynowycz, M.W.; Rice, A.; Andreev, K.; Nobre, T.M.; Kuzmenko, I.; Wereszczynski, J.; Gidalevitz, D. Salmonella membrane structural remodeling increases resistance to antimicrobial peptide LL-37. ACS Infect. Dis. 2019, 5, 1214–1222. [Google Scholar] [CrossRef] [PubMed]
- Fainerman, V.; Vollhardt, D. Surface pressure isotherm for the fluid state of Langmuir monolayers. J. Phys. Chem. B 2006, 110, 10436–10440. [Google Scholar] [CrossRef] [PubMed]
- Klug, J.; Masone, D.; Del Pópolo, M.G. Molecular-level insight into the binding of arginine to a zwitterionic Langmuir monolayer. RSC Adv. 2017, 7, 30862–30869. [Google Scholar] [CrossRef]
- Levental, I.; Janmey, P.; Cēbers, A. Electrostatic contribution to the surface pressure of charged monolayers containing polyphosphoinositides. Biophys. J. 2008, 95, 1199–1205. [Google Scholar] [CrossRef]
- Chachaj-Brekiesz, A.; Kobierski, J.; Wnętrzak, A.; Dynarowicz-Łatka, P. Electrical properties of membrane phospholipids in Langmuir monolayers. Membranes 2021, 11, 53. [Google Scholar] [CrossRef]
- Miñones, J.; Yebra-Pimentel, E.; Iribarnegaray, E.; Conde, O.; Casas, M. Compression—expansion curves of cyclosporin A monolayers on substrates of various ionic strengths. Colloids Surf. A 1993, 76, 227–232. [Google Scholar] [CrossRef]
- Hąc-Wydro, K.; Dynarowicz-Łątka, P. Nystatin in Langmuir monolayers at the air/water interface. Colloids Surf. B 2006, 53, 64–71. [Google Scholar] [CrossRef] [PubMed]
- Wnętrzak, A.; Chachaj-Brekiesz, A.; Janikowska-Sagan, M.; Rodriguez, J.L.F.; Conde, J.M.; Dynarowicz-Łatka, P. Crucial role of the hydroxyl group orientation in Langmuir monolayers organization–The case of 7-hydroxycholesterol epimers. Colloids Surf. A 2019, 563, 330–339. [Google Scholar] [CrossRef]
- Strzalka, J.; Chen, X.; Moser, C.C.; Dutton, P.L.; Ocko, B.M.; Blasie, J.K. X-ray scattering studies of maquette peptide monolayers. 1. Reflectivity and grazing incidence diffraction at the air/water interface. Langmuir 2000, 16, 10404–10418. [Google Scholar] [CrossRef]
- Davis, H.T. Statistical Mechanics of Phases, Interfaces, and Thin Films; Wiley: Hoboken, NJ, USA, 1996. [Google Scholar]
- Han, Y.; Huang, S.; Yan, T. A mean-field theory on the differential capacitance of asymmetric ionic liquid electrolytes. J. Phys. Condens. Matter 2014, 26, 284103. [Google Scholar] [CrossRef] [PubMed]
- Jones, R.A. Soft Condensed Matter; Oxford University Press: Oxford, UK, 2002; Volume 6. [Google Scholar]
- Andelman, D.; Brochard, F.; Knobler, C.; Rondelez, F. Structures and phase transitions in Langmuir monolayers. In Micelles, Membranes, Microemulsions and Monolayers; Springer: New York, NY, USA, 1994; pp. 559–602. [Google Scholar]
- Bossa, G.V.; Gunderson, S.; Downing, R.; May, S. Role of transmembrane proteins for phase separation and domain registration in asymmetric lipid bilayers. Biomolecules 2019, 9, 303. [Google Scholar] [CrossRef] [PubMed]
- Akasaka, R. Calculation of the critical point for mixtures using mixture models based on Helmholtz energy equations of state. Fluid Phase Equilib. 2008, 263, 102–108. [Google Scholar] [CrossRef]
- Bell, I.H.; Jäger, A. Calculation of critical points from Helmholtz-energy-explicit mixture models. Fluid Phase Equilib. 2017, 433, 159–173. [Google Scholar] [CrossRef]
- Knobler, C.M.; Desai, R.C. Phase transitions in monolayers. Annu. Rev. Phys. Chem. 1992, 43, 207–236. [Google Scholar] [CrossRef]
- Dynarowicz-Łatka, P.; Dhanabalan, A.; Oliveira, O.N. A study on two-dimensional phase transitions in langmuir monolayers of a carboxylic acid with a symmetrical triphenylbenzene ring system. J. Phys. Chem. B 1999, 103, 5992–6000. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bossa, G.V.; May, S. Bragg–Williams Theory for Particles with a Size-Modulating Internal Degree of Freedom. Molecules 2023, 28, 5060. https://doi.org/10.3390/molecules28135060
Bossa GV, May S. Bragg–Williams Theory for Particles with a Size-Modulating Internal Degree of Freedom. Molecules. 2023; 28(13):5060. https://doi.org/10.3390/molecules28135060
Chicago/Turabian StyleBossa, Guilherme Volpe, and Sylvio May. 2023. "Bragg–Williams Theory for Particles with a Size-Modulating Internal Degree of Freedom" Molecules 28, no. 13: 5060. https://doi.org/10.3390/molecules28135060
APA StyleBossa, G. V., & May, S. (2023). Bragg–Williams Theory for Particles with a Size-Modulating Internal Degree of Freedom. Molecules, 28(13), 5060. https://doi.org/10.3390/molecules28135060





