Facile Recrystallization Process for Tuning the Crystal Morphology and Thermal Safety of Industrial Grade PYX
Abstract
1. Introduction
2. Results and Discussion
2.1. Solubility Model
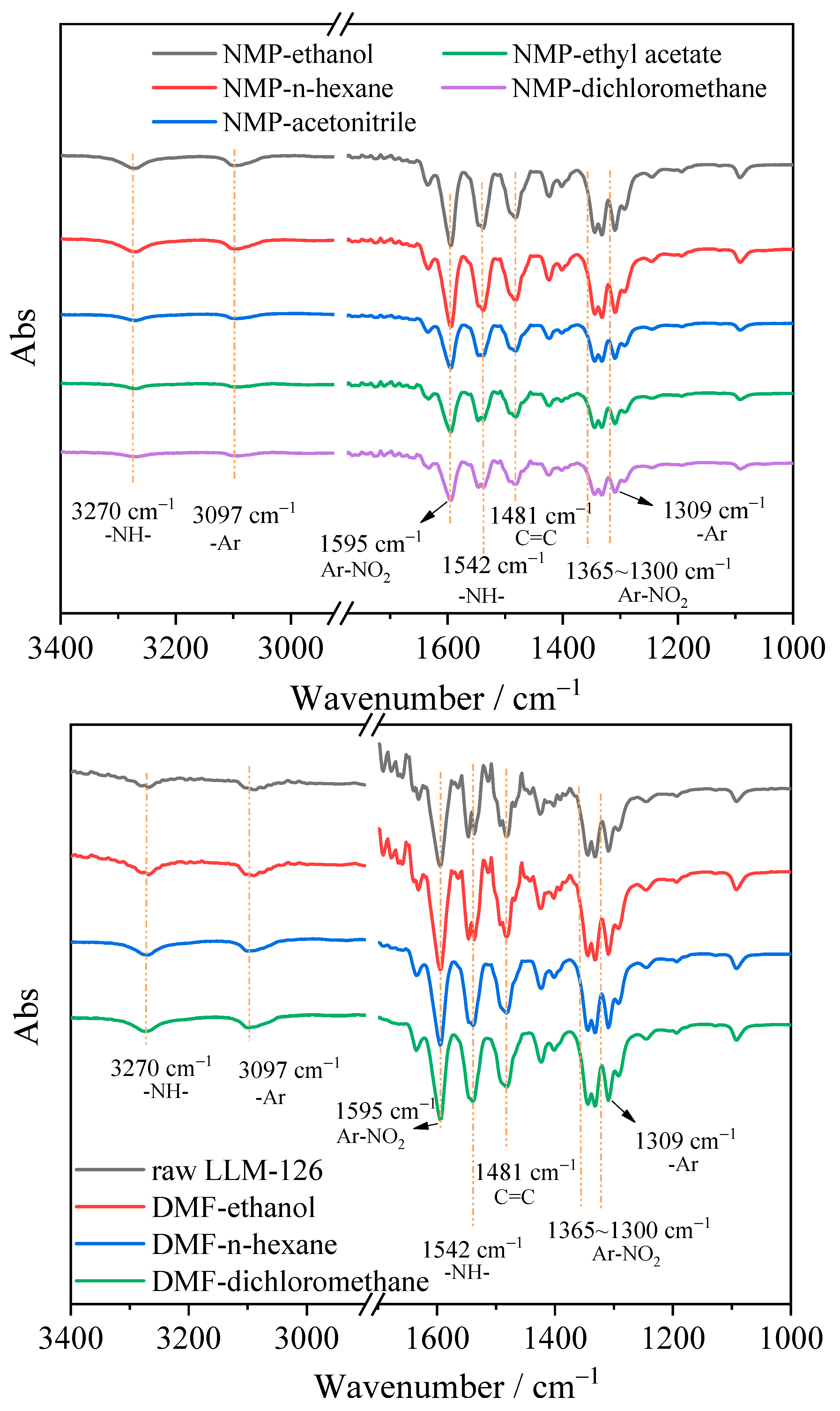
2.2. Morphology and Structure
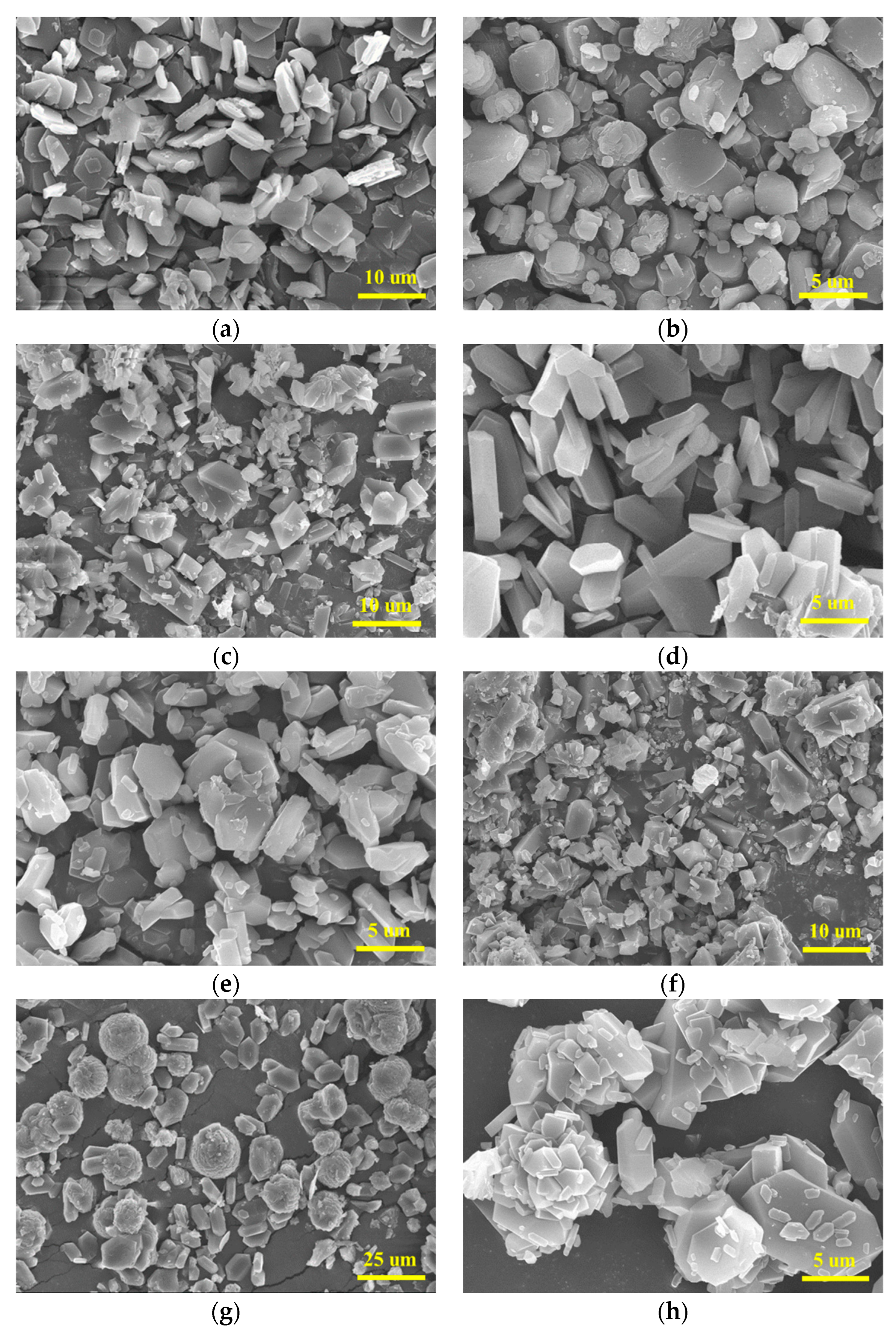
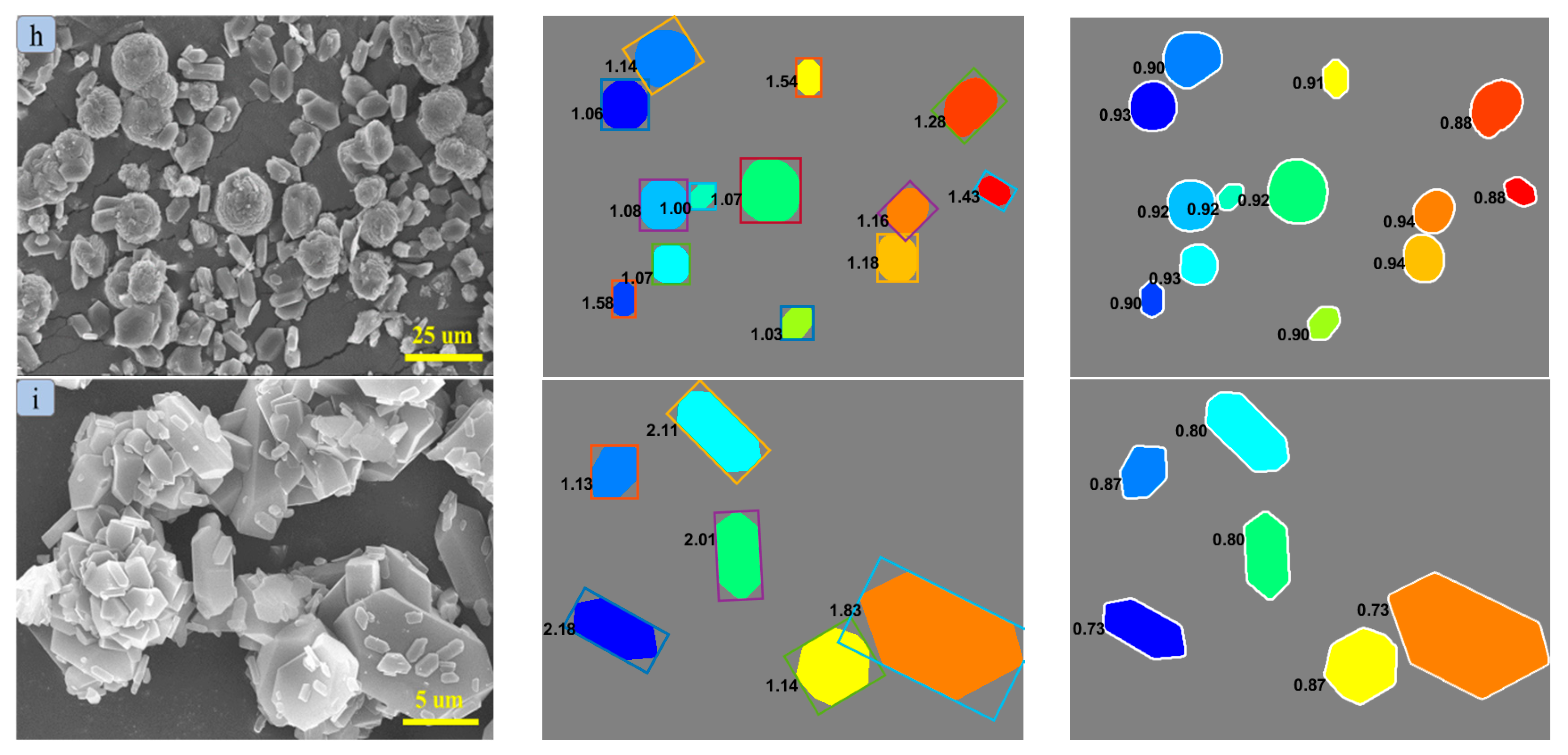
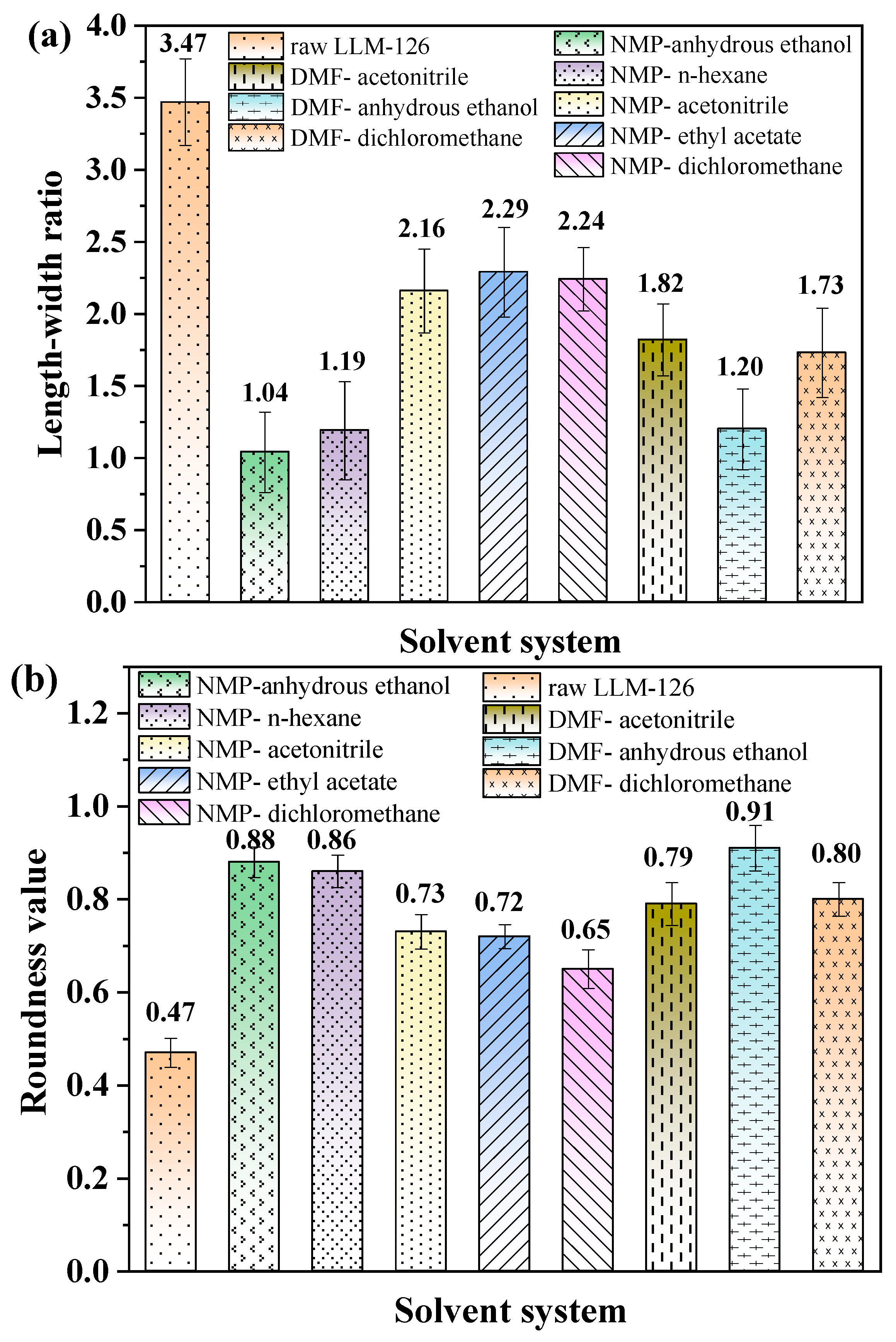
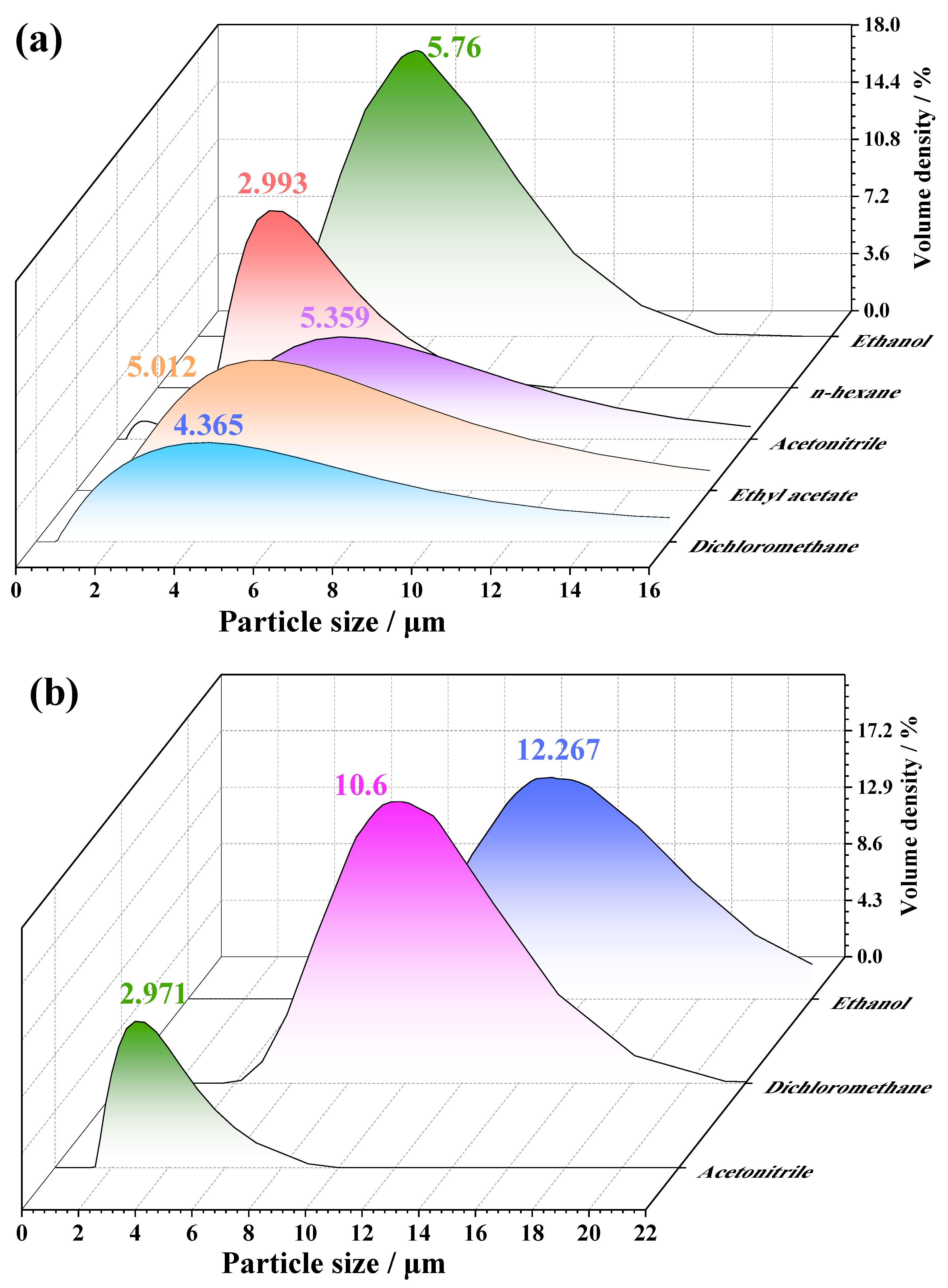
2.3. Crystal Morphology and Particle Size Distribution
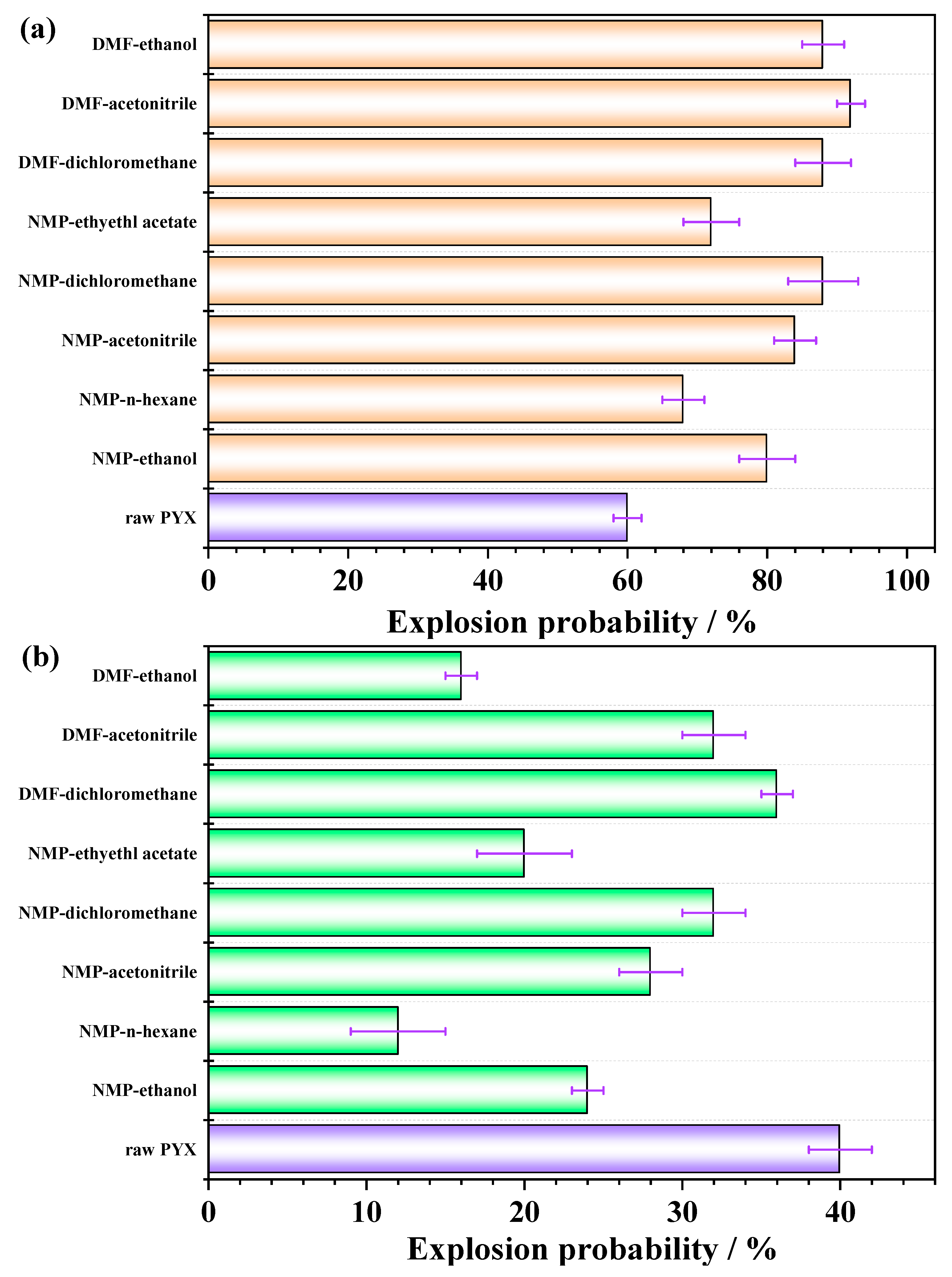
2.4. Mechanical Sensitivity
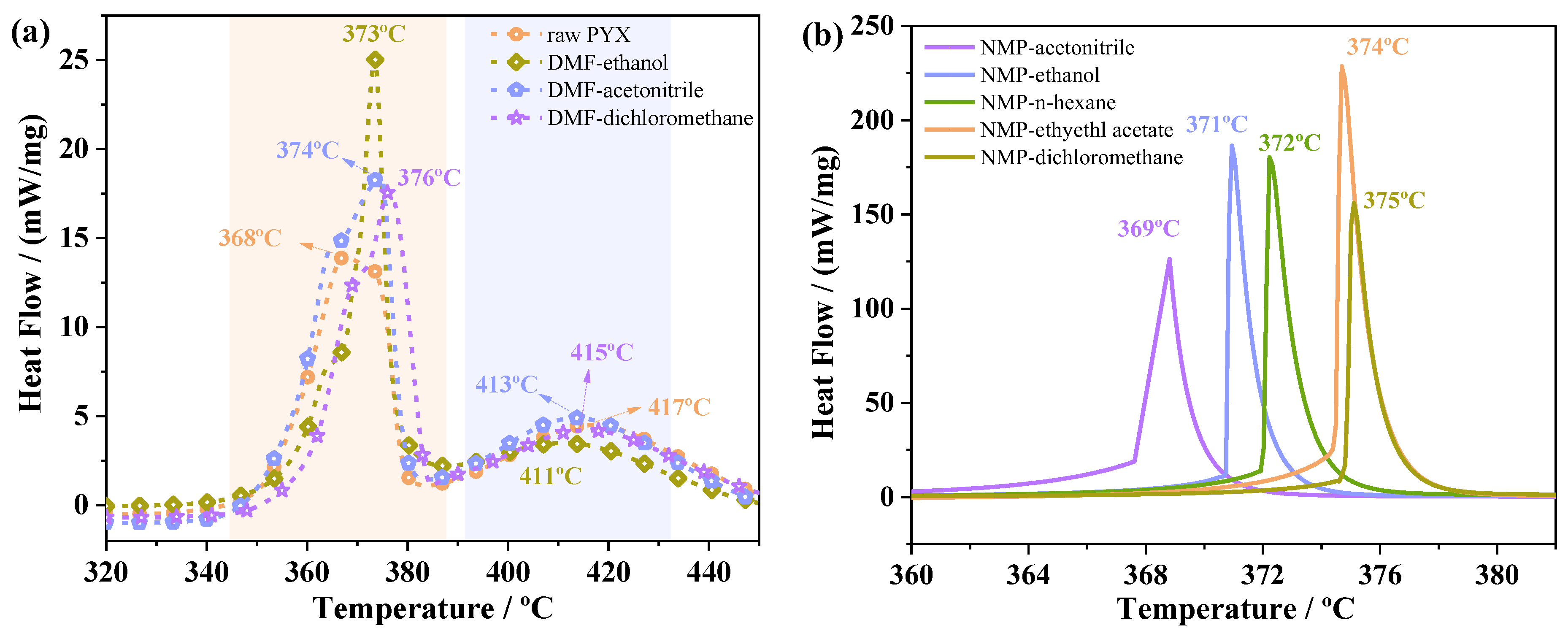
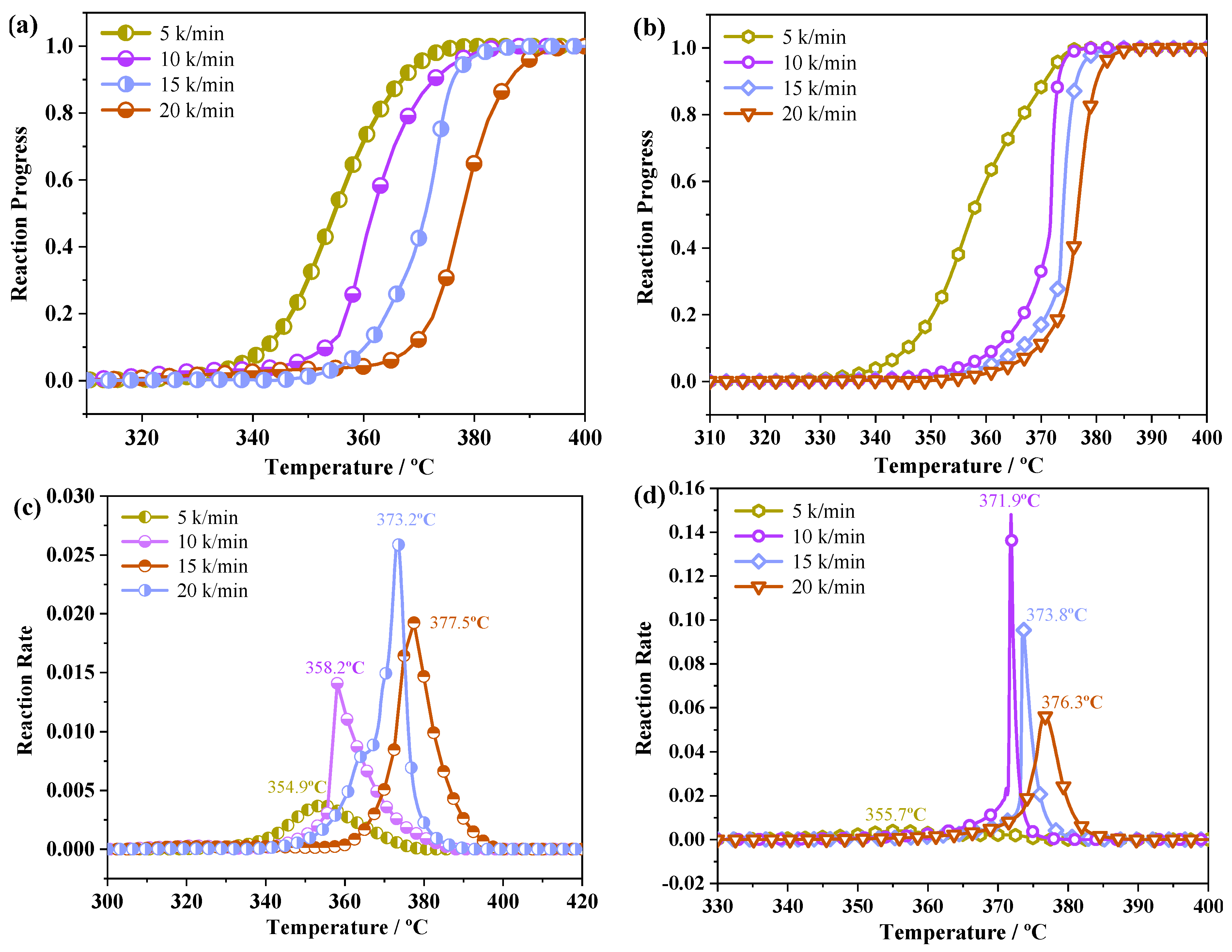
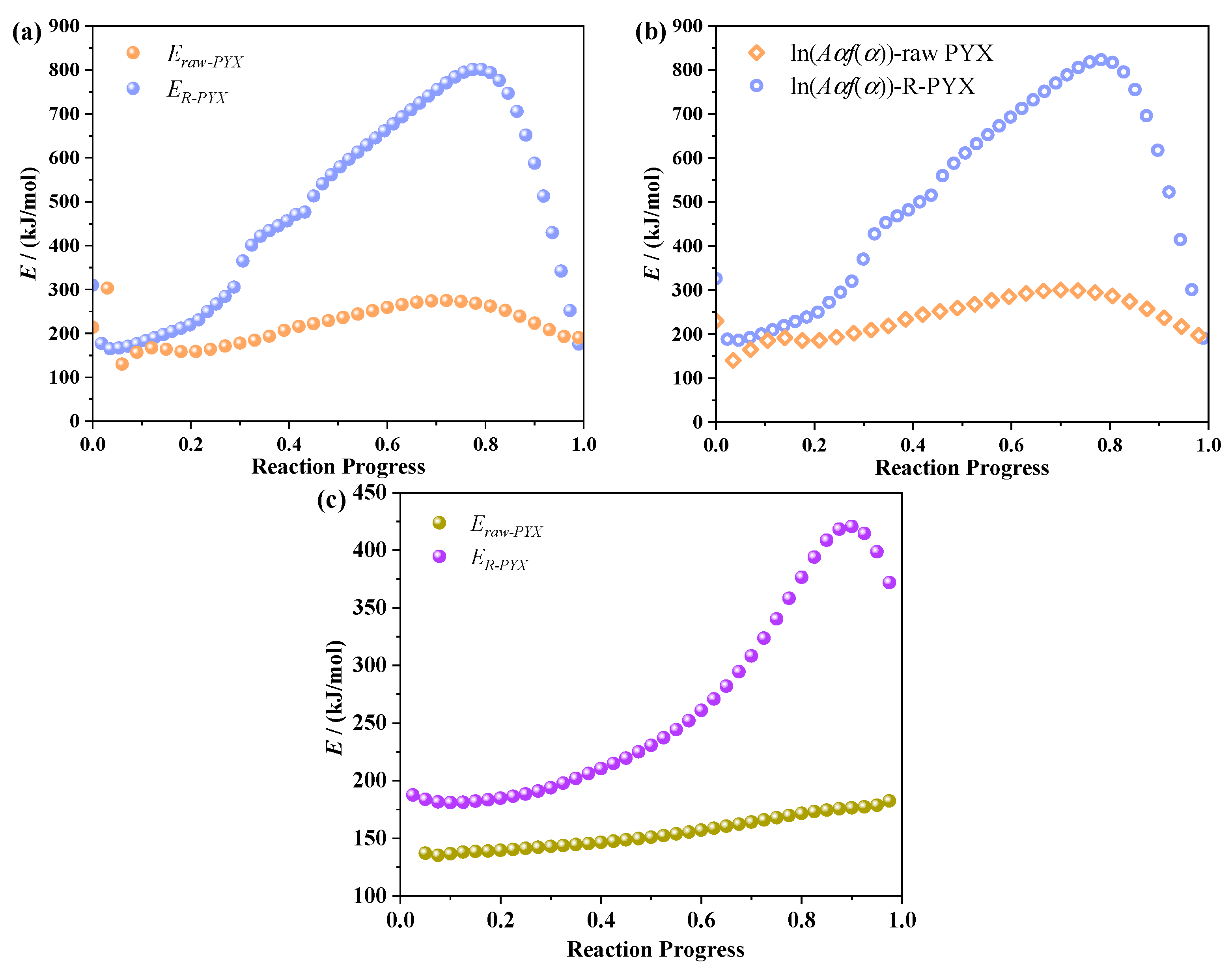
2.5. Thermal Decomposition
3. Experimental
3.1. Materials
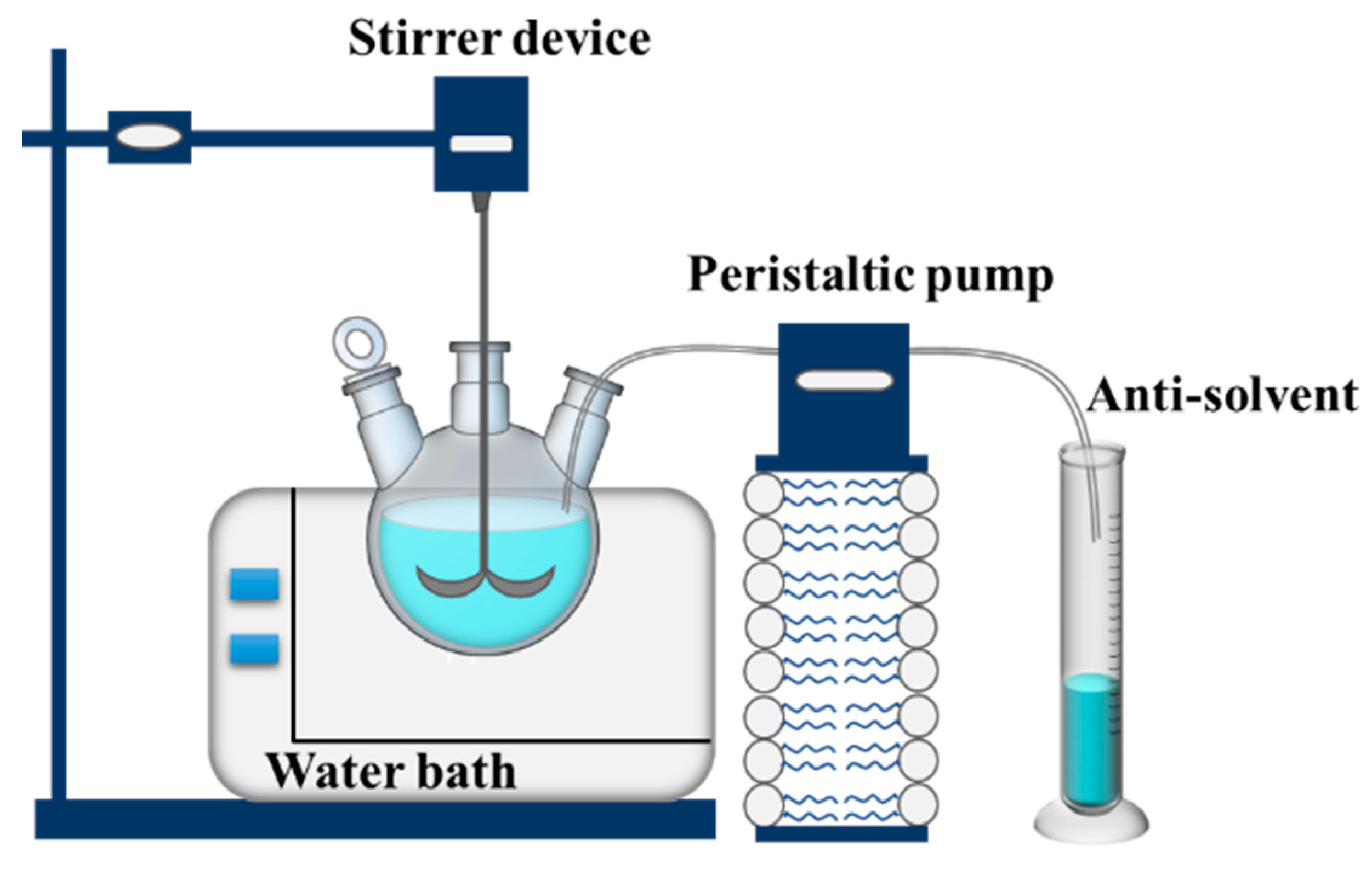
3.2. Methods
3.3. Characterizations
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Yan, T.G.; Ma, J.C.; Yang, H.W.; Cheng, G.B. Introduction of energetic bis-1,2,4-triazoles bridges: A strategy towards advanced heat resistant explosives. Chem. Eng. J. 2022, 429, 132416. [Google Scholar] [CrossRef]
- Deng, M.C.; Chen, F.; Song, S.W.; Huang, S.; Wang, Y.; Zhang, Q.H. From the sensitive primary explosive ICM-103 to insensitive heat-resistant energetic materials through a local azide-to-amino structural modification strategy. Chem. Eng. J. 2022, 429, 132172. [Google Scholar] [CrossRef]
- Pouretedal, H.R.; Damiri, S.; Karami, Z. Increasing of photostability of HNS explosive in the presence of UV photostabilizers. Def. Technol. 2021, 17, 338–342. [Google Scholar] [CrossRef]
- Han, R.S.; Lu, F.P.; Zhang, F.; Wang, Y.L.; Zhou, M.; Qin, G.S.; Chen, J.H.; Wang, H.F.; Chu, E.Y. Thermal and ignition properties of hexanitrostilbene (HNS) microspheres prepared by droplet microfluidics. Def. Technol. 2022, in press. [Google Scholar] [CrossRef]
- Han, R.S.; Chen, J.H.; Zhang, F.; Wang, Y.L.; Zhang, L.; Lu, F.P.; Wang, H.F.; Chu, E.Y. Fabrication of microspherical Hexanitrostilbene (HNS) with droplet microfluidic technology. Powder Technol. 2021, 379, 184–190. [Google Scholar] [CrossRef]
- Yan, F.H.; Zhu, P.; Zhao, S.F.; Shi, J.Y.; Mu, Y.F.; Xia, H.M.; Shen, R.Q. Microfluidic strategy for coating and modification of polymer-bonded nano-HNS explosives. Chem. Eng. J. 2022, 428, 131096. [Google Scholar] [CrossRef]
- Tun, H.; Hao, Y.F.; Haddix, M.; Chen, C.C. Thermodynamic Solubility Modeling of 2,2′,4,4′,6,6′-Hexanitrostilbene (HNS). Fluid Phase. Equil. 2022, 565, 113627. [Google Scholar] [CrossRef]
- Yang, X.M.; Lin, X.Y.; Wang, Y.N.; Wang, L.; Zhang, W.J.; Li, Z.M.; Zhang, T.L. TACOT-derived new nitrogen rich energetic compounds: Synthesis, characterization and properties. New J. Chem. 2019, 43, 19180–19185. [Google Scholar] [CrossRef]
- Gołofit, T. Thermal behaviour and safety of 1,3,7,9-tetranitrodibenzo-1,3a,4,6a-tetraazapentalen (z-TACOT). Thermochim. Acta 2018, 667, 59–64. [Google Scholar] [CrossRef]
- Liu, N.; Zhang, Q.; Duan, B.H.; Lu, X.M.; Bai, X.; Yan, Q.L. Comparative study on thermal behavior of three highly thermostable energetic materials: Z-TACOT, PYX, and TNBP. FirePhysChem 2021, 1, 61–69. [Google Scholar] [CrossRef]
- Nair, U.R.; Gore, G.M.; Sivabalan, R.; Pawar, S.J.; Asthana, S.N.; Venugopalan, S. Preparation and thermal studies on tetranitrodibenzo tetraazapentalene (TACOT): A thermally stable high explosive. J. Hazard. Mater. 2006, 143, 500–505. [Google Scholar] [CrossRef] [PubMed]
- Yang, F.; Li, Y.X.; Chai, X.X.; Dang, X. Synthesis, Crystal Structure, and Performance of 2,2′,2″,4,4′,4″,6,6′,6″-Nonanitro-1,1′:3′,1″-Terphenyl (NONA). Propellants Explos. Pyrotech. 2018, 43, 679–684. [Google Scholar] [CrossRef]
- Song, L.; Zhao, F.Q.; Xu, S.Y. Reactive molecular dynamics simulation of the high-temperature pyrolysis of 2,2′,2″,4,4′,4″,6,6′,6″-nonanitro-1,1′:3′,1″-terphenyl (NONA). RSC. Adv. 2020, 10, 5507–5515. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.; Zhan, L.W.; Zhu, G.K.; Teng, Y.Y.; Shan, Y.; Hou, J.; Li, B.D. Rapid preparation of size-tunable nano-TATB by microfluidics. Def. Technol. 2022, 18, 1139–1147. [Google Scholar] [CrossRef]
- Wang, S.; Yan, X.L.; Lu, F.Y.; Lei, S.Y.; Chen, R. Modelling of Ratchet Growth in TATB-Based Charge under Thermal Cycling. Propellants Explos. Pyrotech. 2022, 47, e202100271. [Google Scholar] [CrossRef]
- Burnham, A.K.; Stanford, V.L.; Vyazovkin, S.; Kahl, E.M. Effect of pressure on TATB and LX-17 thermal decomposition. Thermochim. Acta 2021, 699, 178908. [Google Scholar] [CrossRef]
- Zhang, Z.X.; Si, Y.T.; Yu, T.; Lai, W.P.; Ma, Y.D.; Liu, M.C.; Liu, Y.Z.; Wang, B.Z. Extra contribution to the crystal stability of insensitive explosive TATB: The cooperativity of intermolecular interactions. Def. Technol. 2022, in press. [Google Scholar] [CrossRef]
- Lewis, D.; Padfield, J.; Connors, S.; Wilson, I.; Akhavan, J. Further insights into the discoloration of TATB under ionizing radiation. J. Energ. Mater. 2020, 38, 362–376. [Google Scholar] [CrossRef]
- Chen, K.C.; Warne, L.K.; Jorgenson, R.E.; Niederhaus, J.H. TATB Sensitivity to Shocks from Electrical Arcs. Propellants Explos. Pyrotech. 2019, 44, 1000–1009. [Google Scholar] [CrossRef]
- Gołofit, T.; Szala, M. Origin of PYX thermal stability investigation with calorimetric and spectroscopic methods. J. Therm. Anal. Calorim. 2017, 130, 2047–2054. [Google Scholar] [CrossRef]
- Klapötke, T.M.; Stierstorfer, J.; Weyrauther, M.; Witkowski, T.G. Synthesis and Investigation of 2,6-Bis(picrylamino)-3,5-dinitro-pyridine (PYX) and Its Salts. Chem. Eur. J. 2016, 22, 8619–8626. [Google Scholar] [CrossRef] [PubMed]
- Ding, L.P.; Bai, Y.M.; Cao, Y.; Ren, G.J.; Blanchard, G.J.; Fang, Y. Micelle-induced versatile sensing behavior of bispyrene-based fluorescent molecular sensor for picric acid and PYX explosives. Langmuir 2014, 30, 7645–7653. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.G.; Zhang, J.L.; Chen, Y.F. Preparation and performance testing of ultra-fine PYX. Chin. J. Energ. Mater. 2007, 15, 198–200. [Google Scholar]
- Gao, X.; Lao, Y.L. Determination of the specific heat functions of PYX and KP by DSC. Thermochim. Acta 1989, 149, 123. [Google Scholar] [CrossRef]
- Liu, H.; Wang, F.; Wang, G.X.; Gong, X.D. Theoretical investigations on structure, density, detonation properties, and sensitivity of the derivatives of PYX. J. Comput. Chem. 2012, 33, 1790–1796. [Google Scholar] [CrossRef]
- Li, J.; Yang, R.; Zeng, T.; Hu, J.; Tang, W.; Liu, Z.; Gong, L. Preparation and growth mechanism of micro spherical ammonium dinitramide crystal based on ultrasound-assisted solvent-antisolvent method. Ultrason. Sonochem. 2021, 78, 105716. [Google Scholar] [CrossRef]
- James, C.; Lionel, B.; Gérard, C. Antisolvent Addition: An Effective Method of Controlled Fluid Inclusion Formation in RDX Crystals. Cryst. Growth Des. 2020, 20, 7120–7128. [Google Scholar] [CrossRef]
- Wei, X.F.; Xu, J.J.; Li, H.Z.; Long, X.P.; Zhang, C.Y. Comparative Study of Experiments and Calculations on the Polymorphisms of 2,4,6,8,10,12-Hexanitro-2,4,6,8,10,12-hexaazaisowurtzitane (CL-20) Precipitated by Solvent/Antisolvent Method. J. Phys. Chem. C 2016, 120, 5042–5051. [Google Scholar] [CrossRef]
- Rupanjali, P.; Sameer, V.D. Understanding Morphological Evolution of Griseofulvin Particles into Hierarchical Microstructures during Liquid Antisolvent Precipitation. Cryst. Growth Des. 2019, 19, 5836–5849. [Google Scholar] [CrossRef]
- Mazheva, G.; Vincent, P.; James, C.; Gérard, C.; Lionel, B.; Denis, S. Optimization of an Antisolvent Method for RDX Recrystallization: Influence on Particle Size and Internal Defects. Cryst. Growth Des. 2019, 20, 130–138. [Google Scholar] [CrossRef]
- Nadia, S.F.; Zachary, T.F.; Daniel, K.U.; Brandon, L.W. Effects of Solution Conditions on Polymorph Development in 2,4,6-Trinitrotoluene. Cryst. Growth Des. 2020, 20, 568–579. [Google Scholar] [CrossRef]
- Kumar, R.; Siril, P.F.; Soni, P. Preparation of Nano-RDX by Evaporation Assisted Solvent? Antisolvent Interaction. Propellants Explos. Pyrotech. 2014, 39, 383–389. [Google Scholar] [CrossRef]
- Dobrynin, O.S.; Zharkov, M.N.; Kuchurov, I.V. Supercritical Antisolvent Processing of Nitrocellulose: Downscaling to Nanosize, Reducing Friction Sensitivity and Introducing Burning Rate Catalyst. Nanomaterials 2019, 9, 1386. [Google Scholar] [CrossRef] [PubMed]
- Bosma, J.C.; Vonk, P.; Wesselingh, J. Which shape factor(s) best describe granules? Powder Technol. 2004, 146, 66–72. [Google Scholar] [CrossRef]
- Friedman, H.L. Kinetics of thermal degradation of char-forming plastics from thermogravimetry. Application to a phenolic plastic. J. Polym. Sci. Part C Polym. Symp. 1964, 6, 183–195. [Google Scholar] [CrossRef]
- Ozawa, T. A New Method of Analyzing Thermogravimetric Data. Bull. Chem. Soc. Jpn. 1965, 38, 1881–1886. [Google Scholar] [CrossRef]
- Kissinger, H.E. Reaction Kinetics in Differential Thermal Analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Koga, N.; Vyazovkin, S.; Burnham, A.K.; Favergeon, L. ICTAC Kinetics Committee recommendations for analysis of thermal decomposition kinetics. Thermochim. Acta 2023, 719, 179384. [Google Scholar] [CrossRef]
- Ng, K.M.; Gani, R.; Dam-Johansen, K. Chemical Product Design: Toward a Perspective through Case Studies; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
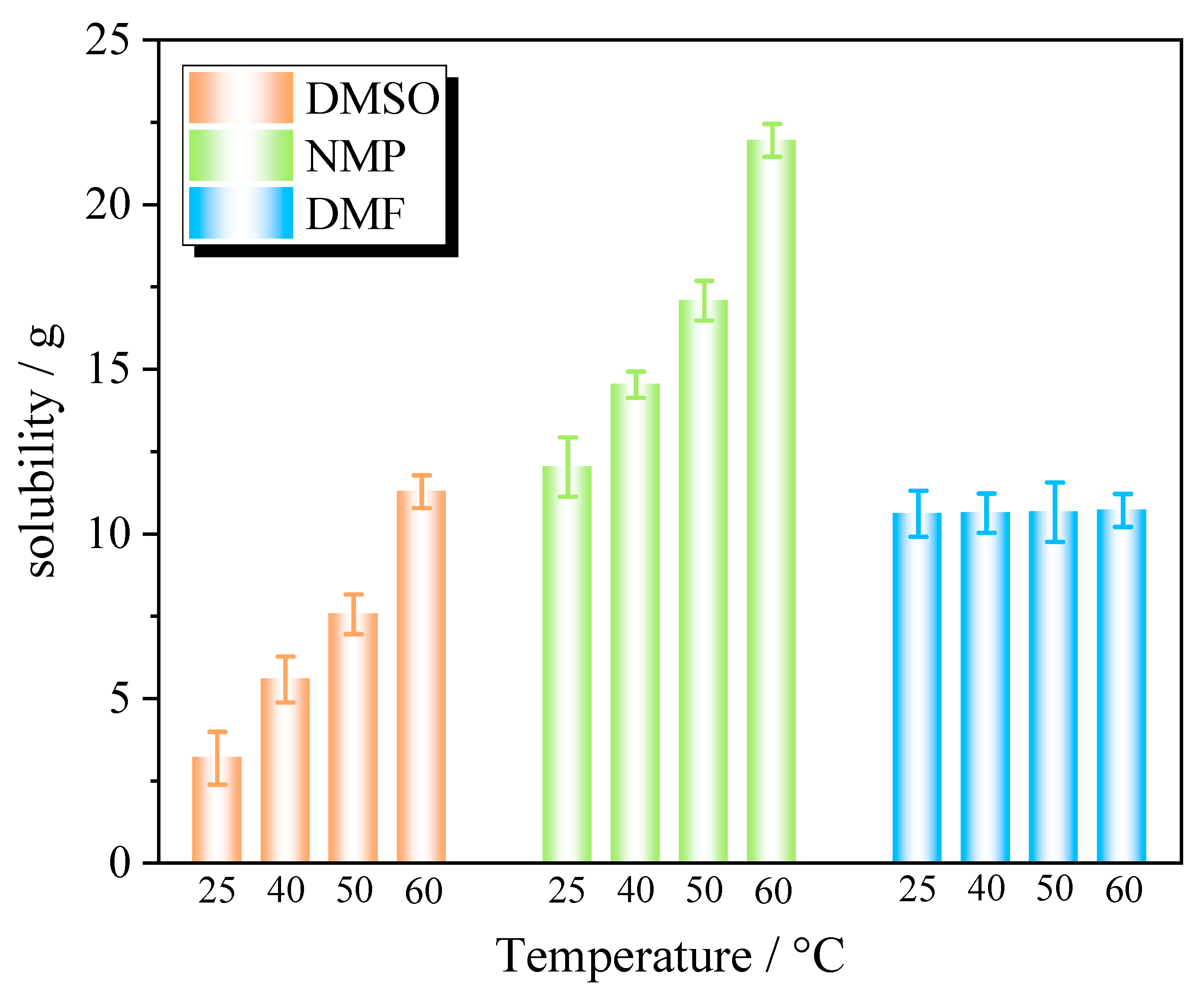



| Solvent | A | B | C | 100 RD | 100 RMSD | R2 |
|---|---|---|---|---|---|---|
| DMSO | −152.286 | 4195.838 | 24.397 | 0.127 | 2.0619 | 0.99739 |
| NMP | −163.224 | 6521.484 | 25.328 | 0.0662 | 0.4758 | 0.99936 |
| DMF | −0.27942 | 113.707 | 0.425 | 0.0073 | 0.263 | 0.99379 |
| Solvent | a | b | 100 RD | 100 RMSD | R2 |
|---|---|---|---|---|---|
| DMSO | −3489.233 | 12.472 | 0.145 | 2.4908 | 0.99617 |
| NMP | −1456.777 | 7.820 | 0.0140 | 1.5207 | 0.99188 |
| DMF | −20.159 | 2.590 | 0.0195 | 0.058 | 0.98289 |
| Solvent | T/K | lnxA,exp | Van’t Hoff Equation | Apelblat Equation | ||
|---|---|---|---|---|---|---|
| lnxA,cal | 100 RD | lnxA,cal | 100 RD | |||
| DMSO | 298.15 | 0.76733 | 0.769055 | −0.22483 | 0.791177 | −3.10775 |
| 303.15 | 0.9986 | 0.962078 | 3.657368 | 0.964812 | 3.383509 | |
| 308.15 | 1.13119 | 1.148836 | −1.55995 | 1.139343 | −0.7207 | |
| 313.15 | 1.3265 | 1.329631 | −0.236 | 1.31462 | 0.895563 | |
| 318.15 | 1.48983 | 1.504742 | −1.00095 | 1.490511 | −0.04572 | |
| 323.15 | 1.63019 | 1.674435 | −2.71413 | 1.666892 | −2.25138 | |
| 328.15 | 1.85015 | 1.838957 | 0.604968 | 1.843649 | 0.351364 | |
| 333.15 | 2.03035 | 1.998541 | 1.566696 | 2.02068 | 0.476251 | |
| NMP | 298.15 | 2.953 | 2.933946 | 0.011648 | 2.957894 | −0.14946 |
| 303.15 | 3.02098 | 3.014534 | 0.213373 | 3.01836 | 0.086733 | |
| 308.15 | 3.0842 | 3.092507 | −0.26934 | 3.083642 | 0.018096 | |
| 313.15 | 3.14229 | 3.16799 | −0.81787 | 3.153401 | −0.35361 | |
| 318.15 | 3.22672 | 3.2411 | −0.44566 | 3.227324 | −0.01872 | |
| 323.15 | 3.30399 | 3.311948 | −0.24086 | 3.305119 | −0.03416 | |
| 328.15 | 3.3887 | 3.380637 | 0.237941 | 3.386514 | 0.064519 | |
| 333.15 | 3.4663 | 3.447264 | 0.549174 | 3.471258 | −0.14303 | |
| DMF | 298.15 | 2.52313 | 2.522386 | 0.029472 | 2.523434 | −0.01204 |
| 303.15 | 2.52408 | 2.523502 | 0.022917 | 2.524212 | −0.00522 | |
| 308.15 | 2.52502 | 2.524581 | 0.017403 | 2.525078 | −0.00231 | |
| 313.15 | 2.52596 | 2.525625 | 0.013258 | 2.526027 | −0.00266 | |
| 318.15 | 2.5269 | 2.526637 | 0.010416 | 2.527053 | −0.00605 | |
| 323.15 | 2.52783 | 2.527617 | 0.008418 | 2.52815 | −0.01267 | |
| 328.15 | 2.52877 | 2.528568 | 0.007999 | 2.529314 | −0.02152 | |
| 333.15 | 2.53065 | 2.52949 | 0.045849 | 2.530541 | 0.00432 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, M.; Fu, J.; Ren, H.; Li, S.; Sun, X.; Jiao, Q. Facile Recrystallization Process for Tuning the Crystal Morphology and Thermal Safety of Industrial Grade PYX. Molecules 2023, 28, 4735. https://doi.org/10.3390/molecules28124735
Zhang M, Fu J, Ren H, Li S, Sun X, Jiao Q. Facile Recrystallization Process for Tuning the Crystal Morphology and Thermal Safety of Industrial Grade PYX. Molecules. 2023; 28(12):4735. https://doi.org/10.3390/molecules28124735
Chicago/Turabian StyleZhang, Mi, Jianbo Fu, Hui Ren, Shengfu Li, Xiaole Sun, and Qingjie Jiao. 2023. "Facile Recrystallization Process for Tuning the Crystal Morphology and Thermal Safety of Industrial Grade PYX" Molecules 28, no. 12: 4735. https://doi.org/10.3390/molecules28124735
APA StyleZhang, M., Fu, J., Ren, H., Li, S., Sun, X., & Jiao, Q. (2023). Facile Recrystallization Process for Tuning the Crystal Morphology and Thermal Safety of Industrial Grade PYX. Molecules, 28(12), 4735. https://doi.org/10.3390/molecules28124735






