On the Gas-Phase Interactions of Alkyl and Phenyl Formates with Water: Ion–Molecule Reactions with Proton-Bound Water Clusters
Abstract
1. Introduction
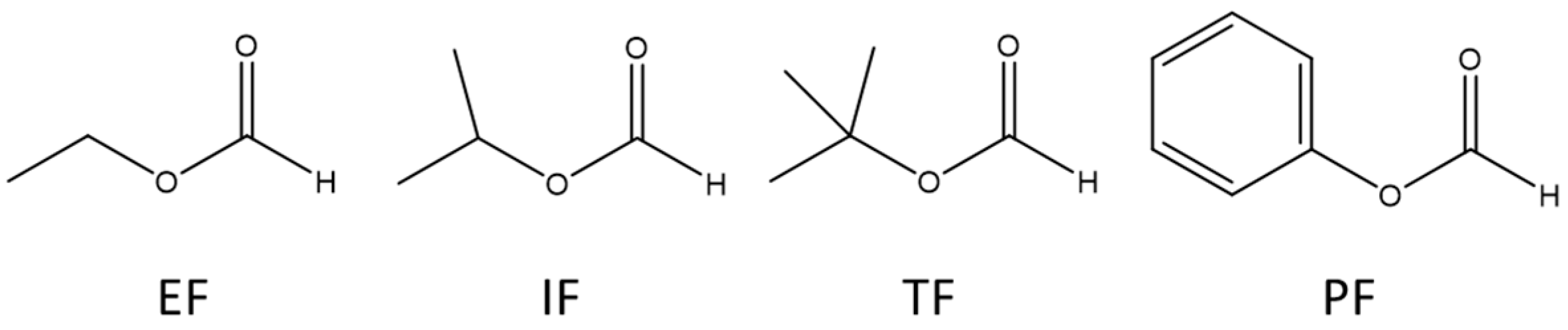
2. Results and Discussion
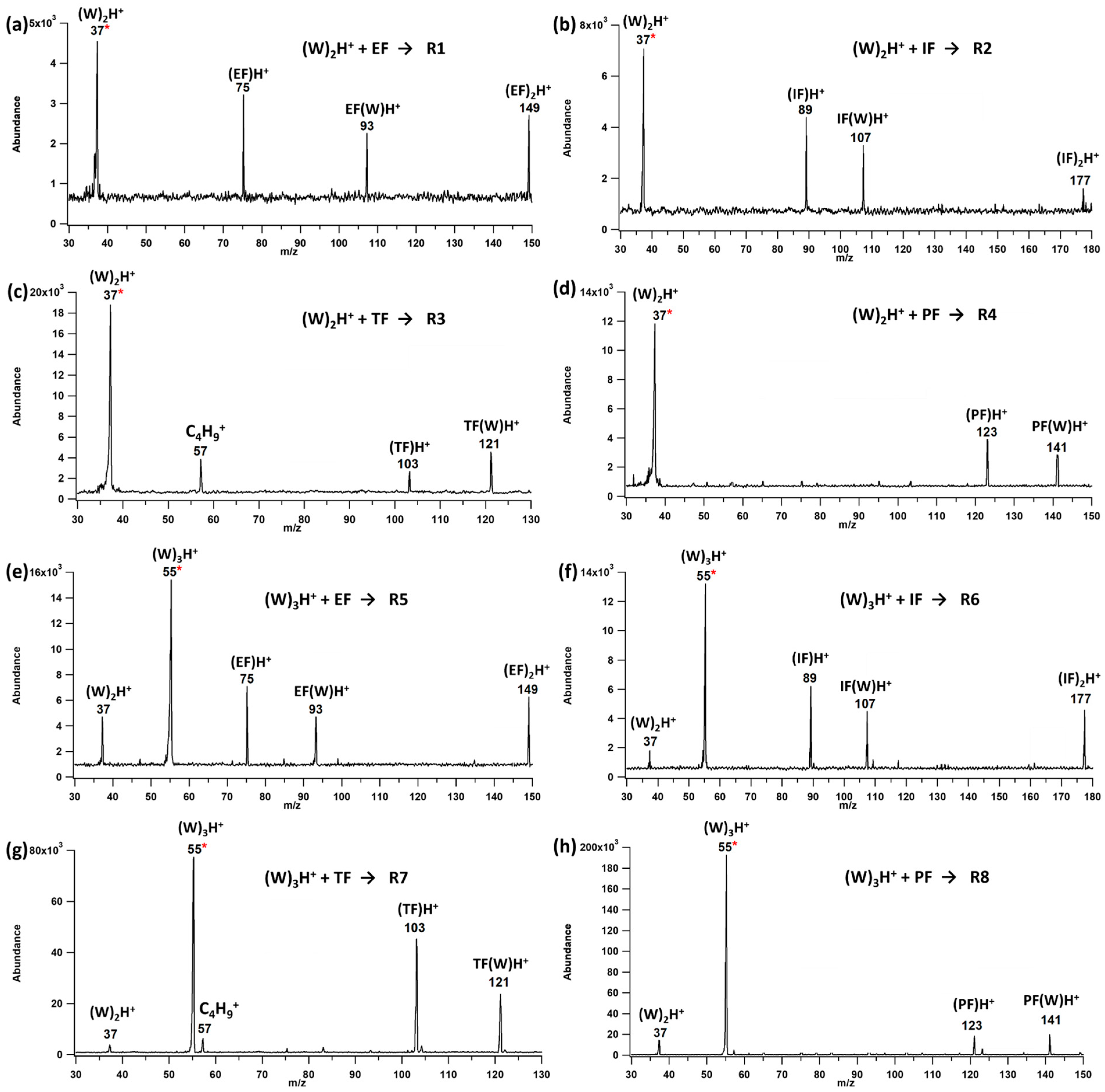
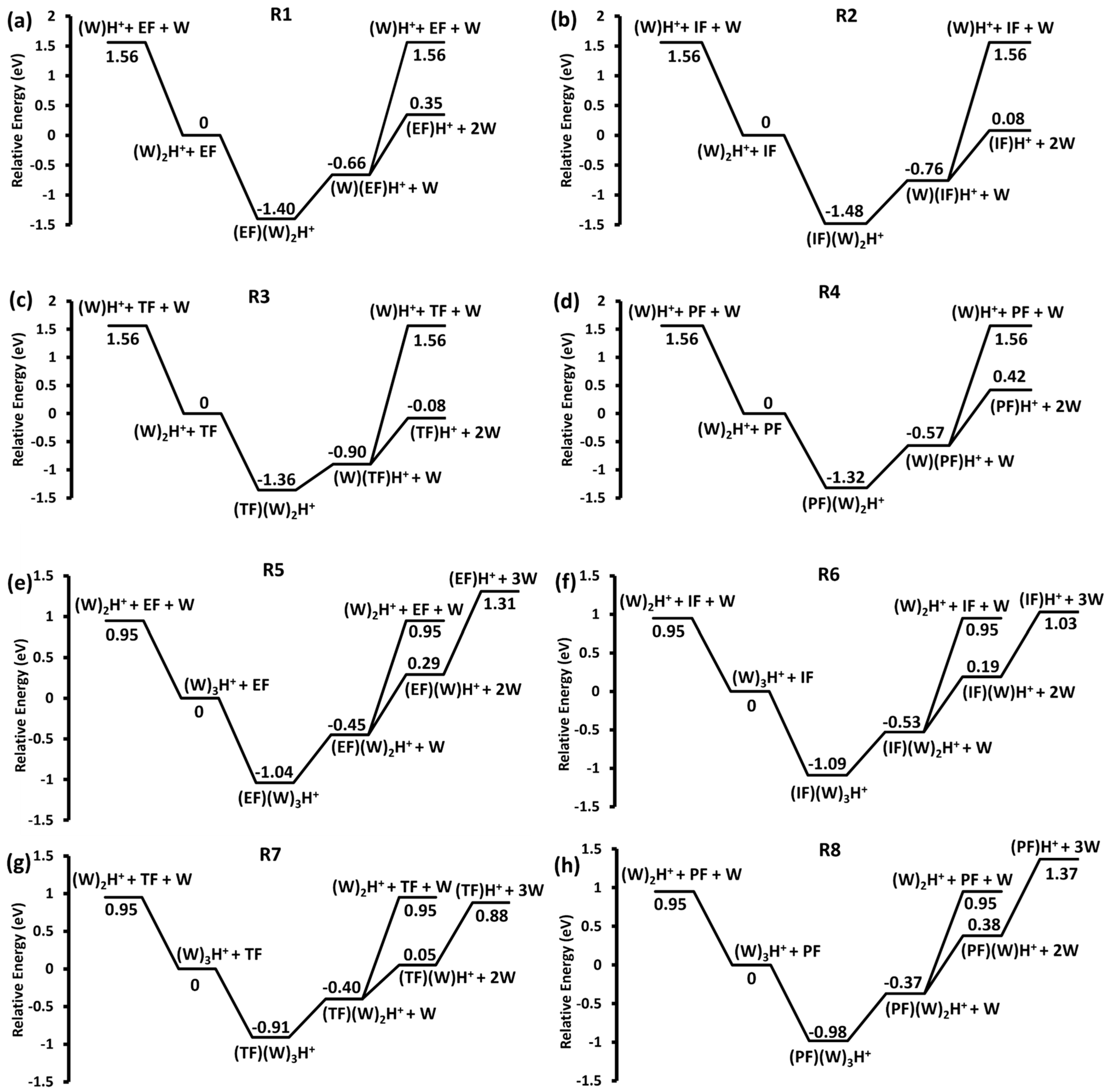
2.1. Water Cluster Ion/Formate Reactions
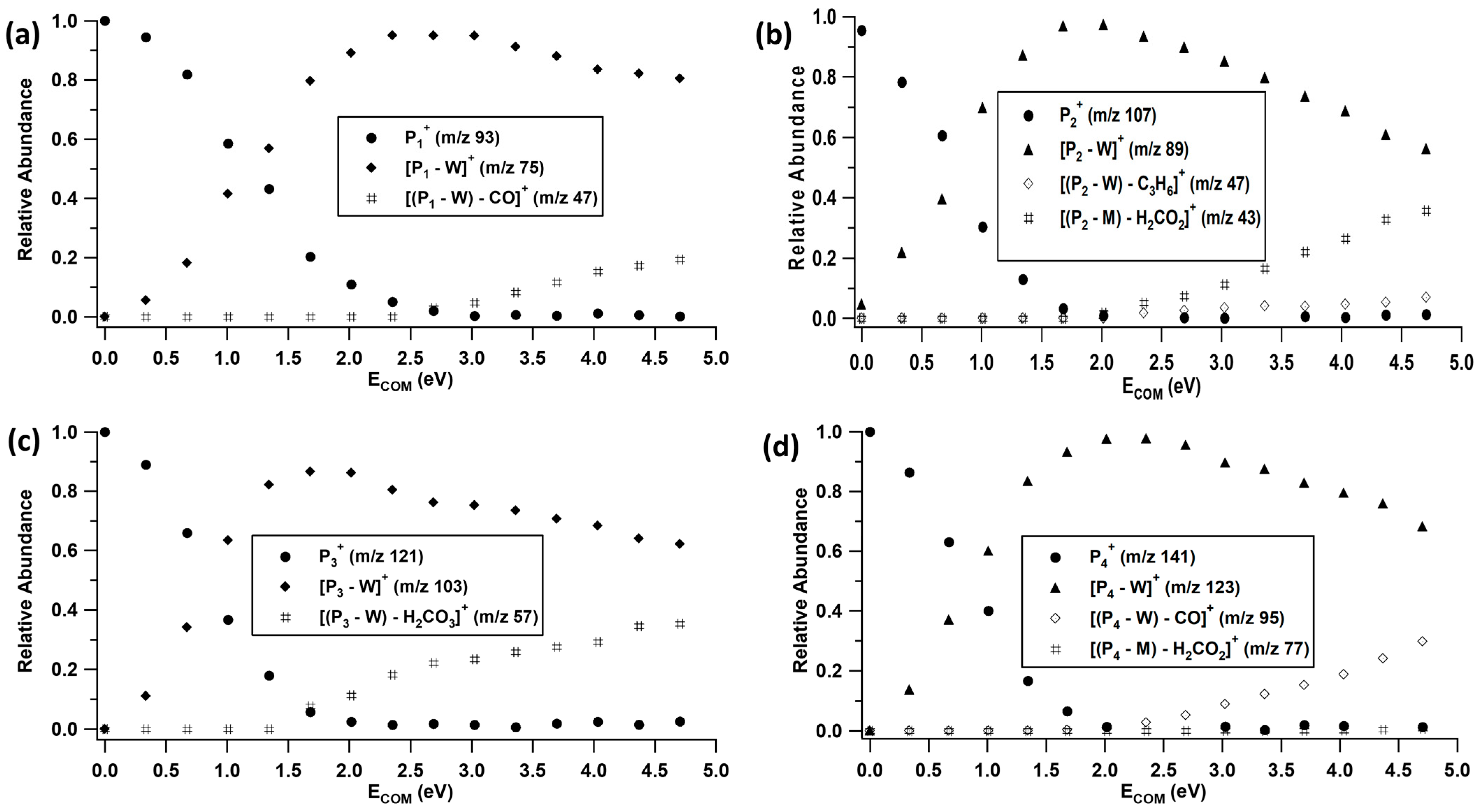
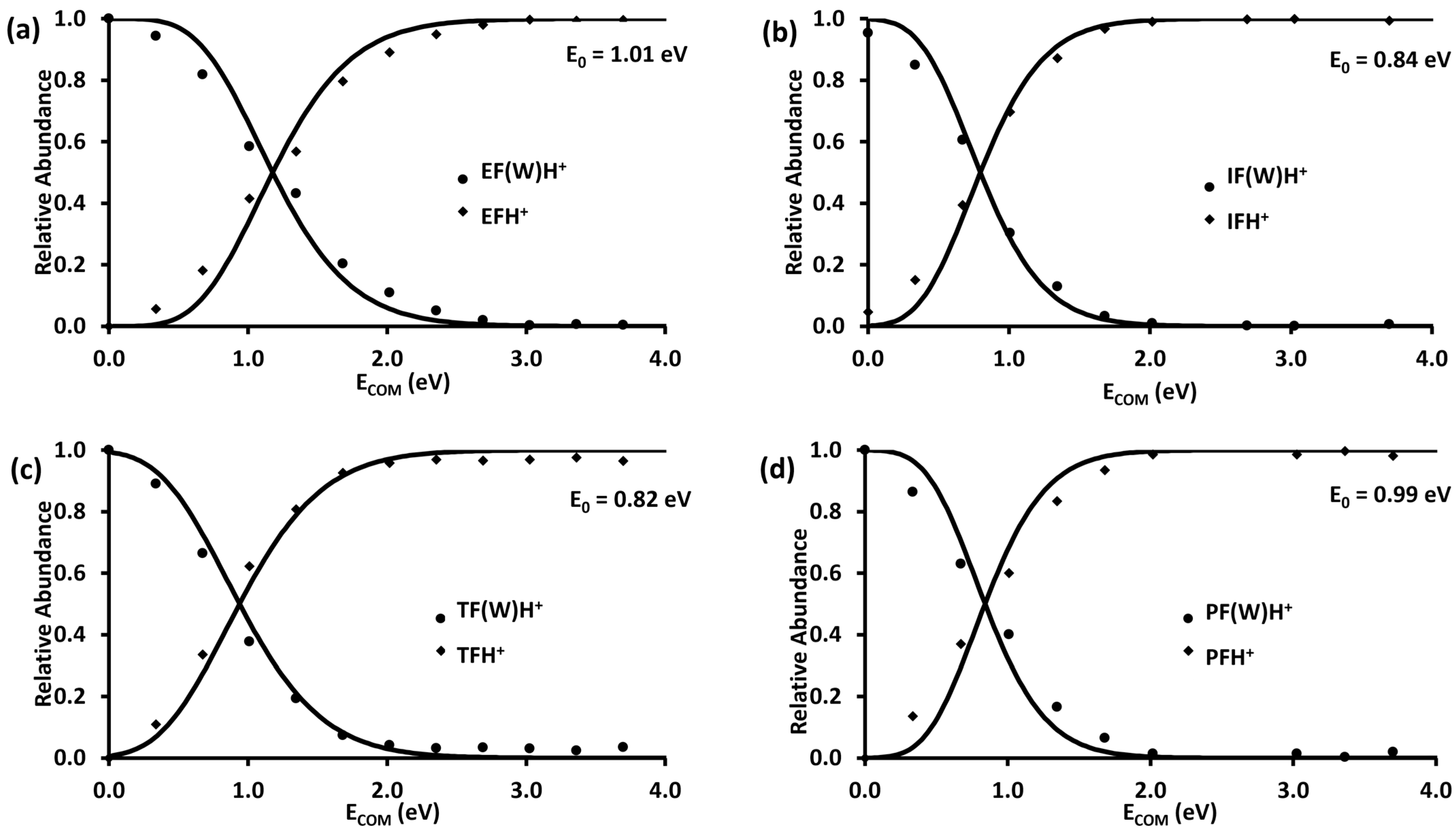
2.2. Unimolecular Reactions of Proton-Bound Water–Formate Clusters
3. Experimental Procedures
3.1. Chemicals
3.2. Tandem Mass Spectrometry
3.3. Computational Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Vaida, V. Perspective: Water cluster mediated atmospheric chemistry. Phys. Chem. Chem. Phys. 2011, 135, 020901. [Google Scholar] [CrossRef]
- Samala, N.R.; Agmon, N. Thermally Induced Hydrogen-Bond Rearrangements in Small Water Clusters and the Persistent Water Tetramer. ACS Omega 2019, 4, 22581–22590. [Google Scholar] [CrossRef]
- Gerber, R.B.; Varner, M.E.; Hammerich, A.D.; Riikonen, S.; Murdachaew, G.; Shemesh, D.; Finlayson-Pitts, B.J. Computational Studies of Atmospherically-Relevant Chemical Reactions in Water Clusters and on Liquid Water and Ice Surfaces. Acc. Chem. Res. 2015, 48, 399–406. [Google Scholar] [CrossRef] [PubMed]
- Zhang, T.; Wen, M.; Zhang, Y.; Lan, X.; Long, B.; Wang, R.; Yu, X.; Zhao, C.; Wang, W. Atmospheric chemistry of the self-reaction of HO2 radicals: Stepwise mechanism versus one-step process in the presence of (H2O)n (n = 1–3) clusters. Phys. Chem. Chem. Phys. 2019, 21, 24042–24053. [Google Scholar] [CrossRef] [PubMed]
- Zhang, T.; Yang, C.; Feng, X.; Kang, J.; Song, L.; Lu, Y.; Wang, Z.; Xu, Q.; Wang, W.; Wang, Z. The catalytic effect of water, water dimers and water trimers on H2S + 3O2 formation by the HO2 + HS reaction under tropospheric conditions. Phys. Chem. Chem. Phys. 2016, 18, 17414–17427. [Google Scholar] [CrossRef] [PubMed]
- Xiong, Y.; Bari, M.A.; Xing, Z.; Du, K. Ambient volatile organic compounds (VOCs) in two coastal cities in western Canada: Spatiotemporal variation, source apportionment, and health risk assessment. Sci. Total Environ. 2020, 706, 135970. [Google Scholar] [CrossRef]
- Heald, C.L.; Kroll, J.H. The fuel of atmospheric chemistry: Toward a complete description of reactive organic carbon. Sci. Adv. 2020, 6, eaay8967. [Google Scholar] [CrossRef]
- Huang, J.; Hartmann, H.; Hellén, H.; Wisthaler, A.; Perreca, E.; Weinhold, A.; Rücker, A.; van Dam, N.M.; Gershenzon, J.; Trumbore, S.; et al. New Perspectives on CO2, Temperature, and Light Effects on BVOC Emissions Using Online Measurements by PTR-MS and Cavity Ring-Down Spectroscopy. Environ. Sci. Technol. 2018, 52, 13811. [Google Scholar] [CrossRef]
- Inaba, S. Theoretical Study of Water Cluster Catalyzed Decomposition of Formic Acid. J. Phys. Chem. A 2014, 118, 3026–3038. [Google Scholar] [CrossRef]
- Iraqi, M.; Lifshitz, C. Studies of ion clusters of atmospheric importance by tandem mass spectrometry. Neat and mixed clusters involving methanol and water. Int. J. Mass Spectrom. 1989, 88, 45–58. [Google Scholar] [CrossRef]
- Sabbioni, C.; Ghedini, N.; Bonazza, A. Organic anions in damage layers on monuments and buildings. Atmos. Environ. 2003, 37, 1261–1269. [Google Scholar] [CrossRef]
- Legrand, M.; Preunkert, S.; Jourdain, B.; Aumont, B. Year-round records of gas and particulate formic and acetic acids in the boundary layer at Dumont d’Urville, coastal Antarctica. J. Geophys. Res. Atmos. 2004, 109, D06313. [Google Scholar] [CrossRef]
- Boring, C.B.; Genfa, Z.; Dasgupta, P.K.; Martin, M.W.; Smith, W.F. Field Measurement of Acid Gases and Soluble Anions in Atmospheric Particulate Matter Using a Parallel Plate Wet Denuder and an Alternating Filter-Based Automated Analysis System. Anal. Chem. 2002, 74, 1256–1268. [Google Scholar] [CrossRef]
- Khare, P.; Kumar, N.; Kumari, K.M.; Srivastava, S.S. Atmospheric formic and acetic acids: An overview. Rev. Geophys. 1999, 37, 227–248. [Google Scholar] [CrossRef]
- Wallington, T.J.; Hurley, M.D.; Maurer, T.; Barnes, I.; Becker, K.H.; Tyndall, G.S.; Orlando, J.J.; Pimentel, A.S.; Bilde, M. Atmospheric Oxidation Mechanism of Methyl Formate. J. Phys. Chem. A 2001, 105, 5146–5154. [Google Scholar] [CrossRef]
- Wu, J.; Ning, H.; Ma, L.; Ren, W. Pressure-dependent kinetics of methyl formate reactions with OH at combustion, atmospheric and interstellar temperatures. Phys. Chem. Chem. Phys. 2018, 20, 26190–26199. [Google Scholar] [CrossRef]
- Hendriyana; Susanto, H.; Subagjo. Atmospheric hydrogenolysis of methyl formate to bio-methanol using Cu/MgO catalyst. In AIP Conference Proceedings; Melville; AIP Publishing LLC: Melville, NY, USA, 2017. [Google Scholar]
- Bell, M.J.; Lau, K.C.; Krisch, M.J.; Bennett, D.I.G.; Butler, L.J.; Weinhold, F. Characterization of the Methoxy Carbonyl Radical Formed via Photolysis of Methyl Chloroformate at 193.3 nm. J. Phys. Chem. A 2007, 111, 1762–1770. [Google Scholar] [CrossRef]
- Pimentel, A.S.; Tyndall, G.S.; Orlando, J.J.; Hurley, M.D.; Wallington, T.J.; Sulbaek Andersen, M.P.; Marshall, P.; Dibble, T.S. Atmospheric chemistry of isopropyl formate and tert-butyl formate. Int. J. Chem. Kinet. 2010, 42, 479–498. [Google Scholar] [CrossRef][Green Version]
- Rivett, A.C.; Martin, D.; Gray, D.J.; Price, C.S.; Nickless, G.; Simmonds, P.G.; O’Doherty, S.J.; Greally, B.R.; Knights, A.; Shallcross, D.E. The role of volatile organic compounds in the polluted urban atmosphere of Bristol, UK. Atmos. Chem. Phys. Discuss. 2003, 3, 769–796. [Google Scholar]
- Atkinson, R.; Lloyd, A.C. Evaluation of kinetic and mechanistic data for modeling of photochemical smog. J. Phys. Chem. Ref. Data 1984, 13, 315–444. [Google Scholar] [CrossRef]
- Min, K.E.; Washenfelder, R.A.; Dubé, W.P.; Langford, A.O.; Edwards, P.M.; Zarzana, K.J.; Stutz, J.; Lu, K.; Rohrer, F.; Zhang, Y.; et al. A broadband cavity enhanced absorption spectrometer for aircraft measurements of glyoxal, methylglyoxal, nitrous acid, nitrogen dioxide, and water vapor. Atmos. Meas. Tech. 2016, 9, 423–440. [Google Scholar] [CrossRef]
- Diedhiou, M.; Mayer, P.M. The Interaction of Methyl Formate with Proton-Bound Solvent Clusters in the Gas Phase and the Unimolecular Chemistry of the Reaction Products. Appl. Sci. 2023, 13, 1339. [Google Scholar] [CrossRef]
- Diedhiou, M.; Mayer, P.M. Fate of Protonated Formates in the Gas Phase. J. Phys. Chem. A 2021, 125, 5096–5102. [Google Scholar] [CrossRef] [PubMed]
- Duong, C.H.; Gorlova, O.; Yang, N.; Kelleher, P.J.; Johnson, M.A.; McCoy, A.B.; Yu, Q.; Bowman, J.M. Disentangling the Complex Vibrational Spectrum of the Protonated Water Trimer, H+(H2O)3, with Two-Color IR-IR Photodissociation of the Bare Ion and Anharmonic VSCF/VCI Theory. J. Phys. Chem. Lett. 2017, 8, 3782–3789. [Google Scholar] [CrossRef]
- Hunter, E.P.L.; Lias, S.G. Evaluated Gas Phase Basicities and Proton Affinities of Molecules: An Update. J. Phys. Chem. Ref. Data 1998, 27, 413–656. [Google Scholar] [CrossRef]
- Curtis, S.; DiMuzio, J.; Mungham, A.; Roy, J.; Hassan, D.; Renaud, J.; Mayer, P.M. Reactions of Atomic Metal Anions in the Gas phase: Competition between Electron Transfer, Proton Abstraction and Bond Activation. J. Phys. Chem. A 2011, 115, 14006–14012. [Google Scholar] [CrossRef]
- Cooks, R.G. Collision-induced dissociation of polyatomic ions. In Collision Spectroscopy; Cooks, R.G., Ed.; Plenum Press: New York, NY, USA, 1978; pp. 357–446. [Google Scholar]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16 Rev. B.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Phys. Chem. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Baer, T.; Mayer, P.M. Statistical RRKM/QET Calculations in mass spectrometry. J. Am. Soc. Mass Spectrom. 1997, 8, 103–115. [Google Scholar]
- Baer, T.; Hase, W.L. Unimolecular Reaction Dynamics, Theory and Experiments; Oxford University Press: New York, NY, USA, 1996. [Google Scholar]
- Beyer, T.; Swinehart, D.R. Number of Multiply-Restricted Partitions [A1] (Algorithm 448). ACM Commun. 1973, 16, 379. [Google Scholar] [CrossRef]
- Renaud, J.B.; Martineau, E.; Mironov, G.G.; Berezovski, M.V.; Mayer, P.M. The collaborative role of molecular conformation and energetics in the binding of gas-phase non-covalent polymer/amine complexes. Phys. Chem. Chem. Phys. 2012, 14, 165–172. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Diedhiou, M.; Mayer, P.M. On the Gas-Phase Interactions of Alkyl and Phenyl Formates with Water: Ion–Molecule Reactions with Proton-Bound Water Clusters. Molecules 2023, 28, 4431. https://doi.org/10.3390/molecules28114431
Diedhiou M, Mayer PM. On the Gas-Phase Interactions of Alkyl and Phenyl Formates with Water: Ion–Molecule Reactions with Proton-Bound Water Clusters. Molecules. 2023; 28(11):4431. https://doi.org/10.3390/molecules28114431
Chicago/Turabian StyleDiedhiou, Malick, and Paul M. Mayer. 2023. "On the Gas-Phase Interactions of Alkyl and Phenyl Formates with Water: Ion–Molecule Reactions with Proton-Bound Water Clusters" Molecules 28, no. 11: 4431. https://doi.org/10.3390/molecules28114431
APA StyleDiedhiou, M., & Mayer, P. M. (2023). On the Gas-Phase Interactions of Alkyl and Phenyl Formates with Water: Ion–Molecule Reactions with Proton-Bound Water Clusters. Molecules, 28(11), 4431. https://doi.org/10.3390/molecules28114431





