Next Article in Journal
Rapid Detection of Benzo[a]pyrene in Extra Virgin Olive Oil Using Fluorescence Spectroscopy
Previous Article in Journal
Substituents Regulate the Cyclization of Conjugated Alkynes to Accurately Construct Cyclo-(E)-[3]dendralenes
Previous Article in Special Issue
Computational and Experimental Evidence for Templated Macrocyclization: The Role of a Hydrogen Bond Network in the Quantitative Dimerization of 24-Atom Macrocycles
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 28
Issue 11
10.3390/molecules28114383
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Molecular and Electronic Structures of Macrocyclic Compounds Formed at Template Synthesis in the M(II)—Thiocarbohydrazide—Diacetyl Triple Systems: A Quantum-Chemical Analysis by DFT Methods

by
Oleg V. Mikhailov
1,* and
Denis V. Chachkov
2
1
Department of Analytical Chemistry, Certificatioin and Quality Management, Kazan National Research Technological University, K. Marx Street 68, 420015 Kazan, Russia
2
Kazan Department of Joint Supercomputer Center of Russian Academy of Sciences—Branch of Federal Scientific Center “Scientific Research Institute for System Analysis of the RAS”, Lobachevskii Street 2/31, 420111 Kazan, Russia
*
Author to whom correspondence should be addressed.
Molecules 2023, 28(11), 4383; https://doi.org/10.3390/molecules28114383
Submission received: 7 May 2023 / Revised: 22 May 2023 / Accepted: 25 May 2023 / Published: 27 May 2023
(This article belongs to the Special Issue Template Synthesis (Self-Assembly) of Macrocycles: Theory and Practice)
Browse Figures
Versions Notes

Abstract

Using density functional theory (DFT) B3PW91/TZVP, M06/TZVP, and OPBE/TZVP chemistry models and the Gaussian09 program, a quantum-chemical calculation of geometric and thermodynamic parameters of Ni(II), Cu(II), and Zn(II) macrotetracyclic chelates, with (NNNN)-coordination of ligand donor centers arising during template synthesis between the indicated ions of 3d elements, thiocarbohydrazide H2N–HN–C(=S)–NH–NH2 and diacetyl Me–C(=O)–C(=O)–Me, in gelatin-immobilized matrix implants was performed. The key bond lengths and bond angles in these coordination compounds are provided, and it is noted that in all these complexes the MN4 chelate sites, the grouping of N4 atoms bonded to the M atom, and the five-membered and six-membered metal chelate rings are practically coplanar. NBO analysis of these compounds was carried out, on the basis of which it was shown that all these complexes, in full accordance with theoretical expectations, are low-spin complexes. The standard thermodynamic characteristics of the template reactions for the formation of the above complexes are also presented. Good agreement between the data obtained using the above DFT levels is noted.

1. Introduction

Previously, in [1], the template synthesis in the system Cu(II)–thiocarbohydrazide H2N–HN–C(=S)–NH–NH2–diacetyl Me–C(=O)–C(=O)–Me in copper(II)hexacyanoferrate (II) gelatin-immobilized matrix implants and the formation of a macrotetracyclic chelate of the indicated metal ion were described, in which the ligand contained in it, namely, the double-deprotonated form 3,10-dithio-6,7,13,14-tetramethyl-1,2,4,5,8,9,11,12-octaazacyclo-tetradecatetraene-1,5,7,12 (L2−), coordinated to Cu(II) via four donor nitrogen atoms (Figure 1). The question of the specificity of the molecular structure of this complex (CuL), however, has not yet been resolved, because neither in [1] nor any other researchers have been able to obtain single crystals of this compound suitable for XRD analysis. The complexes considered in the article [1] belong to the category of metal-macrocyclic compounds with a closed loop, in which the complex metal ion is located in the internal cavity of the macrocyclic organic compound. Such complexes have a number of unique properties that are not inherent in the complexes of the same metals with acyclic ligands and, for this reason alone, are of considerable interest to modern fundamental chemistry. On the other hand, these complexes and the macrocyclic ligands contained in them are quite close in their structure to metal-porphyrins and metal-porphyrazines (complexes formed by porphyrin and porphyrazine, respectively) and can be considered as their peculiar “precursors”. The field of the practical application of metal-porphyrins and metal-porphyrazines is very significant (optics, luminescence, catalysis, medicine, sensorics, etc.), as a result of which this group of coordination compounds is one of the most studied at present. In this context, it seems interesting and important to obtain objective data on the structural and geometric parameters of this chemical compound using quantum-chemical calculations using some version of the density functional theory (DFT), which is currently the most popular method for calculating the molecular and electronic structures of 3d-element complexes. On the other hand, it is very likely that in a similar way, i.e., as a result of the combination of the above ligand synthons, accompanied by intramolecular dehydration (Figure 2), the same complexes of chemical elements adjacent to copper, nickel, and zinc can be formed, although there is no information about this possibility either in [1] or in any other publications devoted to template synthesis. Since it is these two 3d elements that, in terms of the complexes they form, are closest to Cu(II) complexes compared to those for the other d-elements, it seems appropriate, first, to confirm the possibility of the existence of Ni(II) and Zn(II) metal chelates with the above ligand L2−, and secondly, in case of a positive answer to this question, compare the parameters of their molecular and electronic structures, as well as their thermodynamic characteristics, with similar parameters for the CuL complex. The presentation of these data and their discussion will be carried out further in the given article.

2. Results

We immediately note two circumstances that are of particular importance for our further narration. First, each of the three different chemistry models we used, namely, B3PW91/TZVP, M06/TZVP, and OPBE/TZVP, unambiguously predicts the possibility of the existence of each of the three NiL, CuL, and ZnL complexes mentioned above. Second, for each of these coordination compounds, all these three methods give practically the same results, both qualitatively and quantitatively.
The key parameters of the molecular structures calculated by the three different DFT methods mentioned above, namely, the bond lengths and the angles between the lines of these bonds (bond angles) for each of the three ML-type chelates (M = Ni, Cu, Zn) considered by us with the above “template” ligand 3,10-dithio-6,7,13,14-tetramethyl-1,2,4,5,8,9,11,12-octaazacyclotetradecatetraene-1,5,7,12, are presented in Table 1. All three chemistry models unambiguously predict the coplanar coordination of donor nitrogen atoms of this macrocyclic ligand with respect to the central metal ion, since in the MN4 chelate nodes for the indicated M, the sum of four bond angles (N1M1N2), (N2M1N3), (N3M1N4), and (N4M1N1), formed by donor atoms and M atoms (BAS), is exactly 360.0° (which corresponds exactly to a flat quadrilateral). The same situation also takes place for the sum of non-bonding angles (NBAS) formed by neighboring donor nitrogen atoms (Table 1); consequently, the grouping of N4 donor atoms is also coplanar. Wherein, in none of the ML (M = Ni, Cu, Zn) complexes that we considered, it is rectangular—in each of them, only pairwise equality of angles (NNN) takes place, and all these angles, of course, are not equal to 90°. This difference, as expected, is most pronounced in the case of ZnL and least strongly in the case of NiL (Table 1). As for the M–N bond lengths, taking into account the fact that they are equivalent to each other only in pairs, one should expect them to be only pairwise equal, and the calculation results for each of the three variants of the DFT chemistry models used in the work are in full agreement with this prediction. On the other hand, in the series Ni(II)–Cu(II)–Zn(II), there is an increase in ionic radii, as a result of which one can theoretically expect an increase in the lengths of these bonds; this conclusion is also in good agreement with the data presented in Table 1. Unlike the metal–nitrogen bonds, the lengths of the carbon–carbon, carbon–nitrogen, and nitrogen–nitrogen bonds show a much weaker dependence on the nature of the 3d-element M, but pairwise equality was also observed. The carbon–sulfur bond lengths in each of these complexes are the same, which also seems quite natural.
With respect to five-membered [(M1N4N5C1N1), (M1N2N6C2N3)] and six-membered [(M1N1N7C5C4N2), (M1N3N8C6C3N4)] metal chelate rings, it should be noted that all of them, as well as the MN4 chelate nodes, are also coplanar, since the sums of internal bond angles in any of them are either equal to 540° and 720°, which coincides with the sum of the internal angles in a flat pentagon and hexagon, respectively, or very slightly differs from these values (as is the case for the ZnL complex calculated by the DFT M06/TZVP method). Characteristically, these metal chelate rings are pairwise identical to each other, not only in terms of the sum of bond angles but also in their sets, which depend relatively little on the nature of M (Table 1). In view of the foregoing, the ML complexes under consideration can be considered practically planar (although, given that they also include, among other things, four methyl groups that are not a priori planar, it should be recognized that none of them). This fact is very interesting; considering that, according to quite numerous data presented in [2,3,4,5,6,7,8,9,10], macrotetracyclic chelate complexes of 3d elements with cyclic ligands containing two five-membered and two six-membered metal chelate rings, contrary to theoretical expectations, are non-coplanar, and in them, as a rule, all these four cycles are non-coplanar. Each of the NiL, CuL, and ZnL complexes under consideration has one second-order axis, a horizontal plane of symmetry, and a center of symmetry, and, consequently, they all have the C2h symmetry group. In this circumstance, it is quite understandable that according to the data of our calculation by the DFT B3PW91/TZVP as well as and by the DFT M06/TZVP and DFT OPBE/TZVP, the dipole electric moment (μ) of each of them practically does not differ from zero. Images of the molecular structures of these metal chelates are presented in Figure 3.
The key data of the NBO analysis of these compounds, namely, the effective charges on the metal atoms M1 and donor nitrogen atoms N1, N2, N3, and N4 obtained by DFT B3PW91/TZVP, DFT M06/TZVP, and DFT OPBE/TZVP chemistry models, are presented in Table 2; the full NBO analysis can be found in the Supplementary Materials. As expected, they differ quite significantly from those that would take place if all bonds between atoms were ionic. This circumstance indicates a very pronounced delocalization of the electron density within the entire molecular structure of each of the complexes under consideration. Images of higher occupied (HOMO) and lower vacant (LUMO) molecular orbitals for the considered complexes are shown in Figure 4. It should be noted that the NBO analysis data for all three NiL, CuL, and ZnL complexes obtained using the above DFT variants also agree quite well with each other (Table 2).
The values of the key thermodynamic parameters of the metal chelates considered here, namely, the standard enthalpies, entropies, and Gibbs energies of their formation ΔfH0298, Sf0298, ΔfG0298, are presented in Table 3. As can be seen from it, the values of Sf0298 obtained by different versions of the DFT are quite close to each other, while the values of ΔfH0298 and ΔfG0298 differ quite significantly from each other. Currently, it is not possible to give preference to any of these methods in relation to these parameters. However, they are all positive, and their modules are very significant. Characteristically, for each of the complexes considered here, the smallest values of ΔfH0298 and ΔfG0298 are observed in the case of using the DFT OPBE/TZVP and the largest in the case of using the DFT M06/TZVP.
The DFT B3PW91/TZVP and DFT M06/TZVP calculated standard thermodynamic parameters of the template synthesis reactions leading to the formation of these complexes (the general scheme of which is shown in Figure 2) as given in Table 4. As can be seen from it, the values of each of these parameters calculated by these two versions of the DFT are quite close to each other. That is, characteristically, for all these reactions, the relations ΔrH0298 < 0, ΔrS0298 > 0, and ΔrG0298 < 0 take place. From this, in turn, it follows that for any of the M = Ni, Cu, Zn considered by us, such reactions are thermodynamically allowed not only under standard conditions with T = 298.16 K but also at any other temperature T, since according to the Gibbs–Helmholtz equation for an isobaric process ΔrG(T) = ΔrH0 − TΔrS0 the values of ΔrG(T) for these reactions will always be negative. It should be noted, however, that the data presented in Table 4 refer to the gas phase, and therefore, our conclusion regarding the possibility of their implementation also applies to reactions occurring precisely under such conditions.
According to our data, the ground state of the NiL and ZnL chelates in each of the DFT variants used by us is a spin singlet and that of the CuL chelate is a spin doublet, so that all of them belong to the category of low-spin complexes. This is confirmed by the calculation data of the <S**2> parameter, which is equal to 0.0000 in the case of the NiL and ZnL complexes and 0.7500 in the case of CuL, which correspond to the spin multiplicities MS = 1 and MS = 2, respectively. Wherein, the difference in the energies of structures with a spin multiplicity different from that of the ground state (triplet in the case of NiL and ZnL, quartet in the case of CuL) is 132.3, 146.7, and 144.7 kJ/mol (according to DFT B3PW91/TZVP), 148.7, 157.3, and 200.1 kJ/mol (according to DFT B3PW91/TZVP), and 101.6, 120.7, and 124.3 kJ/mol (according to DFT OPBE/TZVP), respectively. As can be seen from these data, the nearest excited state in each of the complexes under study is much higher than the ground state, so that spin cross-over (spin isomerism) is impossible here in principle. That is, interestingly, the largest differences between the energies of the ground and nearest excited states with a different spin multiplicity, as well as for the parameters ΔfH0298 and ΔfG0298, take place in the case of using the DFT M06/TZVP and the smallest in the case of using the DFT OPBE/TZVP.

3. Calculation Method

When performing calculations, we used a variant of the density functional theory (DFT), which combines the standard extended valence-split basis set TZVP and the most modern hybrid functional M06, described in detail in [11], which, according to its authors, is best suited for calculations of 3d-element compounds. For comparison, we also used another version of the DFT, namely, with B3PW91 functional, which is described in detail in [12,13,14] and used by us, in particular, in recently published papers [15,16,17]. The use of this variant of the DFT, in this case, is due to the fact that, according to [12,13,14] and our experience, it allows, as a rule, to obtain the most accurate (i.e., close to experimental) values of the geometric parameters of molecular structures, as well as significantly more accurate values of thermodynamic and other physicochemical parameters compared to other variants of DFT chemistry models. In addition to them, we also carried out calculations of the molecular and electronic structures of the macrocyclic metal chelates by the OPBE/TZVP method, which combines the above TZVP basis and the non-hybrid OPBE functional [18,19], which, according to the data of works [19,20,21,22,23], in the case of 3d elements gives a fairly accurate ratio of the energy of the high-spin state with respect to the energy of the low-spin state and, at the same time, reliably characterizes the geometric parameters of the molecular structures of the metal complexes observed by us. The calculations were carried out using the Gaussian09 software package [24]. As in our previous articles, in which the above calculation methods [15,16,17] were used, the correspondence of the found stationary points to the energy minima in all cases was proved by calculating the second derivatives of the energy with respect to the atomic coordinates; in this case, all the equilibrium structures corresponding to the minimum points on the potential energy surfaces had only real (and, moreover, always positive) frequency values. From the optimized structures, the structure with the lowest total energy was chosen for further consideration. In accordance with the theory of the structure of the atoms, Ni(II), Cu(II), and Zn(II) which are the complexes under examination, should correspond to 3d8, 3d9, and 3d10 electronic configurations, respectively. In this context, spin multiplicities 1 and 3 in the case of Ni(II) and Zn(II) and spin multiplicities 2 and 4 in the case of Cu(II) are considered for the given central metal ions during the calculation. Among the structures optimized at these multiplicities, the lowest-lying structure was selected. To calculate the parameters of molecular structures with multiplicity greater than 1, we used the unrestricted method (UM06, UB3PW91, and UOPBE). The energetically most favorable structure has always been checked according to the STABLE = OPT procedure; in all cases, the wave functions corresponding to these structures were stable. Natural Bond Orbital (NBO) analysis was carried out, using the NBO 3.1 version in the framework of the Gaussian09 program [24] according to the methodology described in [25]. The standard thermodynamic parameters of a formation (ΔfH0298, Sf0298, and ΔfG0298) for the Ni(II), Cu(II), and Zn(II) macrocyclic compounds under study were calculated employing the method [26].

4. Conclusions

Interest in metal macrocyclic compounds and prediction of their physicochemical characteristics (including the calculation of molecular and electronic structures using quantum-chemical calculations of various levels) continues to be consistently high on the part of researchers, as evidenced by a number of recent works, in particular, [27,28,29,30,31,32,33,34,35,36,37,38,39,40], and this article is just one of many devoted to the above topics. In summary, we would like to emphasize that the above-mentioned data of quantum-chemical calculations performed using three different variants of density functional theory, namely, B3PW91/TZVP, M06/TZVP, and OPBE/TZVP chemistry models, unambiguously predict the possibility of the existence of macrocyclic metal complexes Ni(II), Cu(II), and Zn(II) with macrocyclic tetradentate ligand, double-deprotonated form 3,10-dithio-6,7,13,14-tetramethyl-1,2,4,5,8,9,11,12-octaazacyclotetradecatetraene-1,5,7,12 (L2−) with the ratio M(II): L2− = 1:1, resulting from template synthesis in the ternary systems M(II)–thiocarbohydrazide–diacetyl (M = Ni, Cu, Zn). Wherein, the reactions of template synthesis in each of these three ternary systems are accompanied by a decrease in enthalpy and an increase in the entropy of the reaction system; the latter circumstance is unusual for template processes, which, as a rule, are accompanied by a decrease in this thermodynamic parameter. Based on the values of ΔfH0298, Sf0298, and ΔfG0298, it can be expected that the NiL complex will be the most stable among them, the CuL complex will be the least stable, and the ZnL complex will occupy an intermediate position. Remarkably, only the least stable of these complexes is currently known [1]. In this regard, there is every reason to hope that the other two will also be obtained, and at present it is important to confirm the theoretical prediction made in the experiment.
The key structural fragments of these complexes, namely, chelate nodes, two 5-membered and two 6-membered metal chelate rings, are practically coplanar (which is also unconventional for metal chelates containing the listed atomic groups). The values of the key parameters of molecular structures (bond lengths and bond angles) in the compounds under consideration depend little on the nature of M(II) in their composition. Thus, the decisive role in the formation of these structures belongs, as expected, to the macrocyclic ligand itself.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/molecules28114383/s1, NBO Analysis Data of ML complexes.

Author Contributions

Conceptualization, O.V.M.; methodology, O.V.M. and D.V.C.; software, D.V.C.; validation, O.V.M. and D.V.C.; formal analysis, O.V.M. and D.V.C.; investigation, O.V.M. and D.V.C.; resources, D.V.C.; data curation, D.V.C.; writing—original draft preparation, O.V.M. and D.V.C.; writing—review and editing, O.V.M.; visualization, O.V.M. and D.V.C.; supervision, O.V.M.; project administration, O.V.M.; funding acquisition, D.V.C. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

This study did not require institutional approval.

Informed Consent Statement

Not applicable.

Data Availability Statement

No unpublished data were created or analyzed in this article.

Acknowledgments

All quantum-chemical calculations were performed at the Joint Supercomputer Center of the Russian Academy of Sciences—Branch of Federal Scientific Center “Scientific Research Institute for System Analysis of the RAS” which is acknowledged for technical support. The contribution of author Denis V. Chachkov was funded by the state assignment to the Federal Scientific Center “Scientific Research Institute for System Analysis of the RAS” for scientific research. Moreover, this study was carried out using the equipment of the Center for Collective Use “Nanomaterials and Nanotechnology” of the Kazan National Research Technological University with the financial support of the Ministry of Science and Higher Education of the Russian Federation under agreement No. 075-15-2021-699.

Conflicts of Interest

The authors declare that they have no conflict of interest, financial or otherwise.

Sample Availability

Not applicable.

References

  1. Mikhailov, O.V.; Kazymova, M.A.; Shumilova, T.A.; Solovieva, S.E. Template synthesis in the M(II)–thiocarbohydrazide–diacetyl triple system (M = Ni, Cu) in a metal(II)hexacyanoferrate(II) gelatin-immobilized matrix. Transit. Metal Chem. 2004, 29, 732–736. [Google Scholar] [CrossRef]
  2. Mikhailov, O.V.; Chachkov, D.V. Self-assembly of supramolecular Ni(II) and Cu(II) metalmacrocyclic compounds with tetraazamacrocyclic ligand into a gelatin-immobilized matrix. J. Coord. Chem. 2010, 63, 4309–4318. [Google Scholar] [CrossRef]
  3. Chachkov, D.V.; Mikhailov, O.V. Density Functional Theory Calculation of Molecular Structures of (5656) Macrotetracyclic 3d Metal Complexes with 4,12-Dithiooxo-1,8-dioxa-3,6,10,13-tetraazacyclotetradecanedione-5,11. Russ. J. Inorg. Chem. 2012, 57, 981–986. [Google Scholar] [CrossRef]
  4. Chachkov, D.V.; Mikhailov, O.V. Structure of (5656) Macrotetracyclic Chelates in the Ternary Systems M(II)–Ethanedithioamide–Acetone (M = Mn, Fe, Co, Ni, Cu, Zn) According to DFT Calculations. Russ. J. Inorg. Chem. 2013, 58, 1073–1078. [Google Scholar] [CrossRef]
  5. Chachkov, D.V.; Mikhailov, O.V. Quantum-Chemical Calculation of Molecular Structures of (5656) Macrotetracyclic 3d-Metal Complexes “Self-Assembled” in Quaternary Systems M(II) Ion– Ethanedithioamide–Formaldehyde– Ammonia by the Density Functional Theory Method. Russ. J. Inorg. Chem. 2014, 59, 218–223. [Google Scholar] [CrossRef]
  6. Mikhailov, O.V.; Chachkov, D.V. Macrotetracyclic Chelates of Doubly Charged 3d-Element Ions with 1,4,8,11-Tetraazacyclotetradecane-2,3,9,10-tetrathione and Their Molecular Structures according to Density Functional Theory Data. Russ. J. Inorg. Chem. 2014, 59, 1276–1282. [Google Scholar] [CrossRef]
  7. Mikhailov, O.V.; Chachkov, D.V. Molecular Structures of (5656) Macrotetracyclic Chelates Formed in the M(II) Ion– Ethanedithioamide– 2-Thiapropanediol-1,3 Systems according to Density Functional Theory Calculations. Russ. J. Inorg. Chem. 2015, 60, 1354–1359. [Google Scholar] [CrossRef]
  8. Mikhailov, O.V.; Chachkov, D.V. DFT Analysis of Molecular Structure of 14-Membered Tetraaza-, Dioxotetraaza- and Hexaazamacroheterocyclic Ligands and Their Metal ComplexesRuss. J. Gen. Chem. 2016, 86, 1102–1107. [Google Scholar] [CrossRef]
  9. Mikhailov, O.V.; Chachkov, D.V. DFT OPBE/TZVP Calculation of M olecular Structures of (5656) Macroheterocyclic Chelates of Double Charged 3d-Element Ions with 1,5,8,11-Tetraazacyclotetradecanetetrathione-2,3,9,10 and Its Dioxa- and Dithia Analogs. Macroheterocycles 2016, 9, 268–276. [Google Scholar] [CrossRef]
  10. Mikhailov, O.V.; Chachkov, D.V. Molecular Structures of (5656) Macrotetracyclic Chelates in M(II) Ion–Ethanedithioamide– Methanimine– Hydrogen Cyanide Quaternary Systems by DFT Calculations. Russ. J. Inorg. Chem. 2016, 61, 616–622. [Google Scholar] [CrossRef]
  11. Zhao, Y.; Truhlar, D.G. The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: Two new functionals and systematic testing of four M06-class functionals and 12 other functionals. Theor. Chem. Acc. 2008, 120, 215–241. [Google Scholar] [CrossRef]
  12. Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Revs. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef] [PubMed]
  13. Perdew, J.P.; Burke, K.; Wang, Y. Generalized gradient approximation for the exchange-correlation hole of a many-electron system. Phys. Revs. B 1996, 54, 16533–16539. [Google Scholar] [CrossRef] [PubMed]
  14. Medvedev, M.G.; Bushmarinov, I.S.; Sun, J.; Perdew, J.P.; Lyssenko, K.A. Density functional theory is straying from the path toward the exact functional. Science 2017, 355, 49–52. [Google Scholar] [CrossRef]
  15. Mikhailov, O.V.; Chachkov, D.V. Molecular Structure of M(N13) Compounds with 12-Membered Nitrogen-Containing Cycle and Axial Nitrogen Atom (M = Mn, Fe): Quantum-Chemical Design by DFT Method. Quantum Rep. 2023, 5, 282–293. [Google Scholar] [CrossRef]
  16. Chachkov, D.V.; Mikhailov, O.V. Heteroligand Iron(V) Complexes Containing Porphyrazine, trans-Di[benzo]porphyrazine or Tetra[benzo]porphyrazine, Oxo and Fluoro Ligands: DFT Quantum-Chemical Study. Int. J. Mol. Sci. 2023, 24, 6442. [Google Scholar] [CrossRef]
  17. Chachkov, D.V.; Mikhailov, O.V. DFT Method Used for Prediction of Molecular and Electronic Structures of Mn(VI) Macrocyclic Complexes with Porhyrazine/Phthalocyanine and Two Oxo Ligands. Materials 2023, 16, 2394. [Google Scholar] [CrossRef]
  18. Paulsen, H.; Duelund, L.; Winkler, H.; Toftlund, H.; Trautwein, A.X. Free Energy of Spin-Crossover Complexes Calculated with Density Functional Methods. Inorg. Chem. 2001, 40, 2201–2203. [Google Scholar] [CrossRef]
  19. Swart, M.; Groenhof, A.R.; Ehlers, A.W.; Lammertsma, K. Validation of Exchange−Correlation Functionals for Spin States of Iron Complexes. J. Phys. Chem. A 2004, 108, 5479–5483. [Google Scholar] [CrossRef]
  20. Swart, M.; Ehlers, A.W.; Lammertsma, K. Performance of the OPBE exchange-correlation functional. Mol. Phys. 2004, 102, 2467–2474. [Google Scholar] [CrossRef]
  21. Swart, M. Metal–ligand bonding in metallocenes: Differentiation between spin state, electrostatic and covalent bonding. Inorg. Chim. Acta 2007, 360, 179–189. [Google Scholar] [CrossRef]
  22. Møller, C.; Plesset, M.S. Note on an approximation treatment for many-electron systems. Phys. Rev. 1934, 46, 618–622. [Google Scholar] [CrossRef]
  23. Head-Gordon, M.; Pople, J.A.; Frisch, M.J. MP2 energy evaluation by direct methods. Chem. Phys. Lett. 1988, 153, 503–506. [Google Scholar] [CrossRef]
  24. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09; Revision A.01; Gaussian, Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
  25. Weinhold, F.; Landis, C.R.; Glendening, E.D. What is NBO analysis and how is it useful? Int. Revs. Phys. Chem. 2016, 35, 399–440. [Google Scholar] [CrossRef]
  26. Ochterski, J.W. Thermochemistry in Gaussian; Gaussian, Inc.: Wallingford, CT, USA, 2000. [Google Scholar]
  27. Zhabanov, Y.A.; Tverdova, N.V.; Giricheva, N.I.; Girichev, G.V.; Stuzhin, P.A. DFT Study of molecular and electronic structure of magnesium (II) tetra(1,2,5-chalcogenadiazolo) porphyrazines, [TXDPzMg] (X = O, S, Se, Te). J. Porphyr. Phthalocyanines 2017, 21, 439–452. [Google Scholar] [CrossRef]
  28. Novakova, V.; Donzello, M.P.; Ercolani, C.; Zimcik, P.; Stuzhin, P.A. Tetrapyrazinoporphyrazines and their metal derivatives. Part II: Electronic structure, electrochemical, spectral, photophysical and other application related properties. Coord. Chem. Rev. 2018, 361, 1–73. [Google Scholar] [CrossRef]
  29. Sorokin, A.B. Recent progress on exploring µ-oxo bridged binuclear porphyrinoid complexes in catalysis and material science. Coord. Chem. Rev. 2019, 389, 141–160. [Google Scholar] [CrossRef]
  30. Pritchard, B.P.; Altarawy, D.; Didier, B.; Gibson, T.D.; Windus, T.L. New Basis Set Exchange: An Open, Up-to-Date Resource for the Molecular Sciences Community. J. Chem. Inf. Model. 2019, 59, 4814–4820. [Google Scholar] [CrossRef]
  31. Onami, Y.; Koya, R.; Kawasaki, T.; Aizawa, H.; Nakagame, R.; Miyagawa, Y.; Haraguchi, T.; Akitsu, T.; Tsukiyama, K.; Palafox, M.A. Investigation by DFT Methods of the Damage of Human Serum Albumin including Amino Acid Derivative Schiff Base Zn(II) Complexes by IR-FEL Irradiation. Int. J. Mol. Sci. 2019, 20, 2846. [Google Scholar] [CrossRef]
  32. Otlyotov, A.A.; Ryzhov, I.V.; Kuzmin, I.A.; Zhabanov, Y.A.; Mikhailov, M.S.; Stuzhin, P.A. DFT Study of Molecular and Electronic Structure of Ca(II) and Zn(II) Complexes with Porphyrazine and tetrakis(1,2,5-thiadiazole)porphyrazine. Int. J. Mol. Sci. 2020, 21, 2923. [Google Scholar] [CrossRef]
  33. Martins, L.S.; Lameira, J.; Kruger, H.G.; Alves, C.N.; Silva, J.R.A. Evaluating the Performance of a Non-Bonded Cu2+ Model Including Jahn-Teller Effect into the Binding of Tyrosinase Inhibitors. Int. J. Mol. Sci. 2020, 21, 4783. [Google Scholar] [CrossRef] [PubMed]
  34. Latypov, S.K.; Kondrashova, S.A.; Polyancev, F.M.; Sinyashin, O.G. Quantum Chemical Calculations of 31P NMR Chemical Shifts in Nickel Complexes: Scope and Limitations. Organometallics 2020, 39, 1413–1422. [Google Scholar] [CrossRef]
  35. Zhou, Q.; Liu, Z.; Marks, T.J.; Darancet, P. Electronic Structure of Metallophthalocyanines, MPc (M = Fe, Co, Ni, Cu, Zn, Mg) and Fluorinated MPc. J. Phys. Chem. A 2021, 125, 4055–4061. [Google Scholar] [CrossRef] [PubMed]
  36. Zhabanov, Y.A.; Ryzhov, I.V.; Kuzmin, I.A.; Eroshin, A.V.; Stuzhin, P.A. DFT Study of Molecular and Electronic Structure of Y, La and Lu Complexes with Porphyrazine and Tetrakis(1,2,5-thiadiazole)porphyrazine. Molecules 2021, 26, 113. [Google Scholar] [CrossRef]
  37. Zhabanov, Y.A.; Eroshin, A.V.; Ryzhov, I.V.; Kuzmin, I.A.; Finogenov, D.N.; Stuzhin, P.A. Molecular Structure, Thermodynamic and Spectral Characteristics of Metal-Free and Nickel Complex of Tetrakis(1,2,5-thiadiazolo) porphyrazine. Molecules 2021, 26, 2945. [Google Scholar] [CrossRef]
  38. Eroshin, A.V.; Otlyotov, A.A.; Kuzmin, I.A.; Stuzhin, P.A.; Zhabanov, Y.A. DFT Study of the Molecular and Electronic Structure of Metal-Free Tetrabenzoporphyrin and Its Metal Complexes with Zn, Cd, Al, Ga, In. Int. J. Mol. Sci. 2022, 23, 939. [Google Scholar] [CrossRef]
  39. Ryzhov, I.V.; Eroshin, A.V.; Zhabanov, Y.A.; Finogenov, D.N.; Stuzhin, P.A. DFT Study of Molecular Structure, Electronic and Vibrational Spectra of Tetrapyrazinoporphyrazine, Its Perchlorinated Derivative and Their Al, Ga and In Complexes. Int. J. Mol. Sci. 2022, 23, 5379. [Google Scholar] [CrossRef]
  40. Eroshin, A.V.; Koptyaev, A.I.; Otlyotov, A.A.; Minenkov, Y.; Zhabanov, Y.A. Iron(II) Complexes with Porphyrin and Tetrabenzoporphyrin: CASSCF/MCQDPT2 Study of the Electronic Structures and UV–Vis Spectra by sTD-DFT. Int. J. Mol. Sci. 2023, 24, 7070. [Google Scholar] [CrossRef]
Molecules 28 04383 g001
Figure 1. The structural formula of the complex formed during template synthesis in the Cu(II)–thiocarbohydrazide–diacetyl system as described in [1].
Figure 1. The structural formula of the complex formed during template synthesis in the Cu(II)–thiocarbohydrazide–diacetyl system as described in [1].
Molecules 28 04383 g001
Molecules 28 04383 g002
Figure 2. General scheme of the key reaction of template synthesis in the M(II)–thiocarbohydrazide–diacetyl systems (M = Ni, Cu, Zns).
Figure 2. General scheme of the key reaction of template synthesis in the M(II)–thiocarbohydrazide–diacetyl systems (M = Ni, Cu, Zns).
Molecules 28 04383 g002
Molecules 28 04383 g003aMolecules 28 04383 g003b
Figure 3. Molecular structure images of NiL (a), CuL (b), and ZnL (c) metal chelates calculated using the DFT B3PW91/TZVP chemistry model.
Figure 3. Molecular structure images of NiL (a), CuL (b), and ZnL (c) metal chelates calculated using the DFT B3PW91/TZVP chemistry model.
Molecules 28 04383 g003aMolecules 28 04383 g003b
Molecules 28 04383 g004aMolecules 28 04383 g004b
Figure 4. The pictures of HOMO and LUMO in the NiL, CuL, and ZnL complexes according to the DFT B3PW91/TZVP chemistry model. The energy values of the given MOs (in brackets) are expressed in eV. The symbol “alpha” belongs to the electron having spin (+1/2), and the symbol “beta” belongs to the electron having spin (−l/2).
Figure 4. The pictures of HOMO and LUMO in the NiL, CuL, and ZnL complexes according to the DFT B3PW91/TZVP chemistry model. The energy values of the given MOs (in brackets) are expressed in eV. The symbol “alpha” belongs to the electron having spin (+1/2), and the symbol “beta” belongs to the electron having spin (−l/2).
Molecules 28 04383 g004aMolecules 28 04383 g004b
Table 1. Key parameters of the molecular structures of Ni(II), Cu(II), and Zn(II) complexes with the double-deprotonated form of the macrocyclic ligand, 3,10-dithio-6,7,13,14-tetramethyl-1,2,4,5,8,9,11,12-octaazacyclotetradecatetraene-1,5,7,12 (L2−) calculated by B3PW91/TZVP, M06/TZVP, and OPBE/TZVP chemistry models.
Table 1. Key parameters of the molecular structures of Ni(II), Cu(II), and Zn(II) complexes with the double-deprotonated form of the macrocyclic ligand, 3,10-dithio-6,7,13,14-tetramethyl-1,2,4,5,8,9,11,12-octaazacyclotetradecatetraene-1,5,7,12 (L2−) calculated by B3PW91/TZVP, M06/TZVP, and OPBE/TZVP chemistry models.
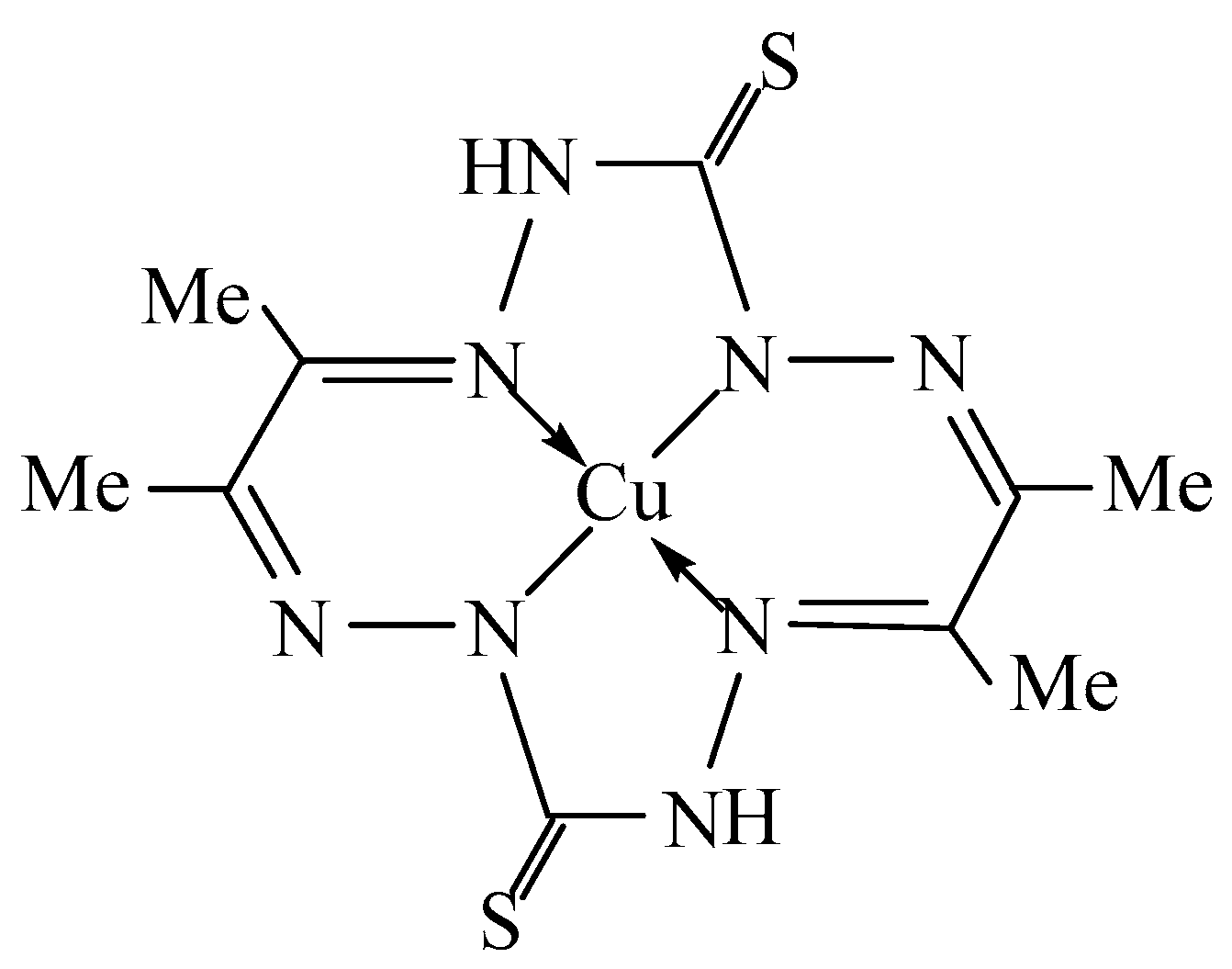
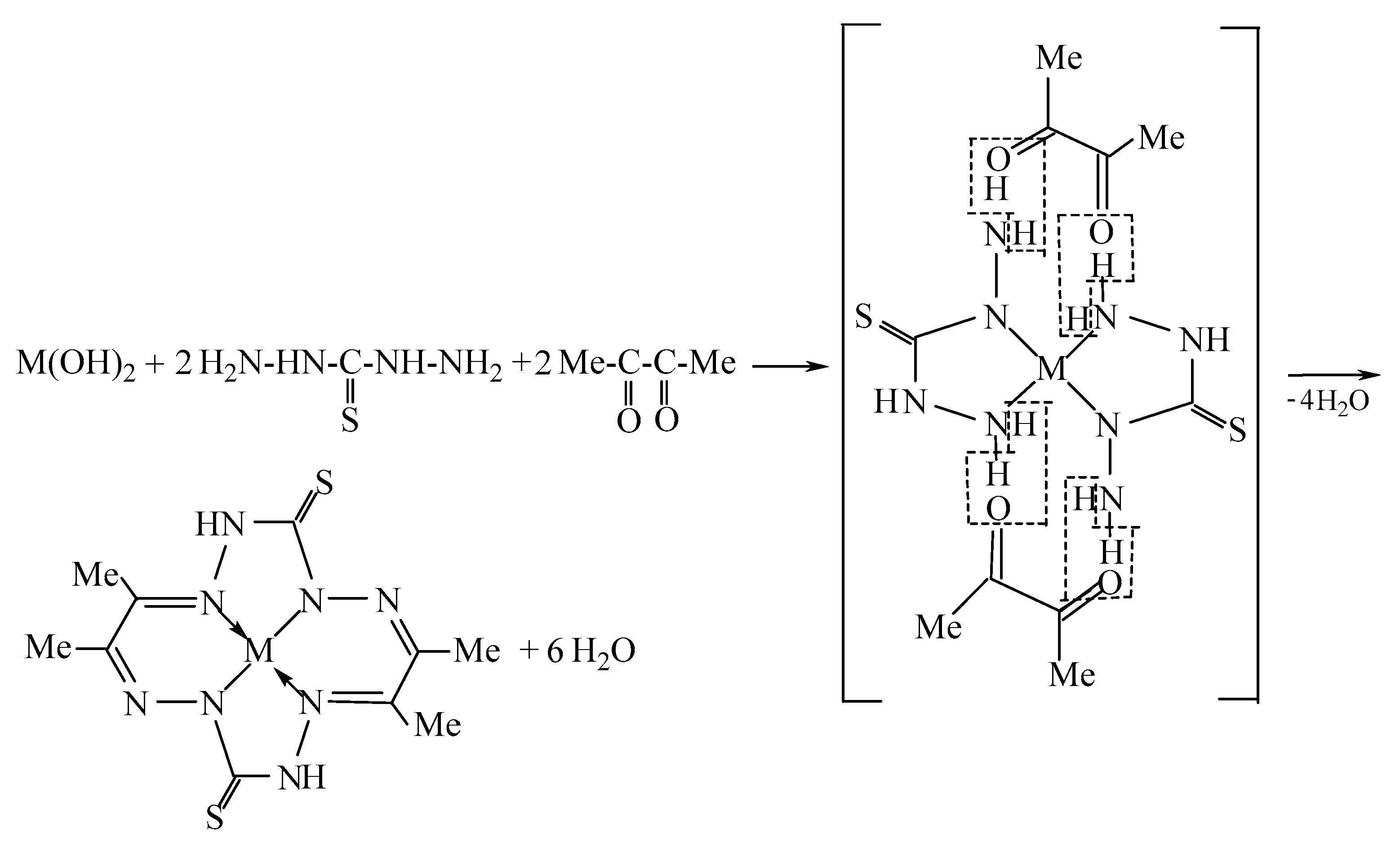
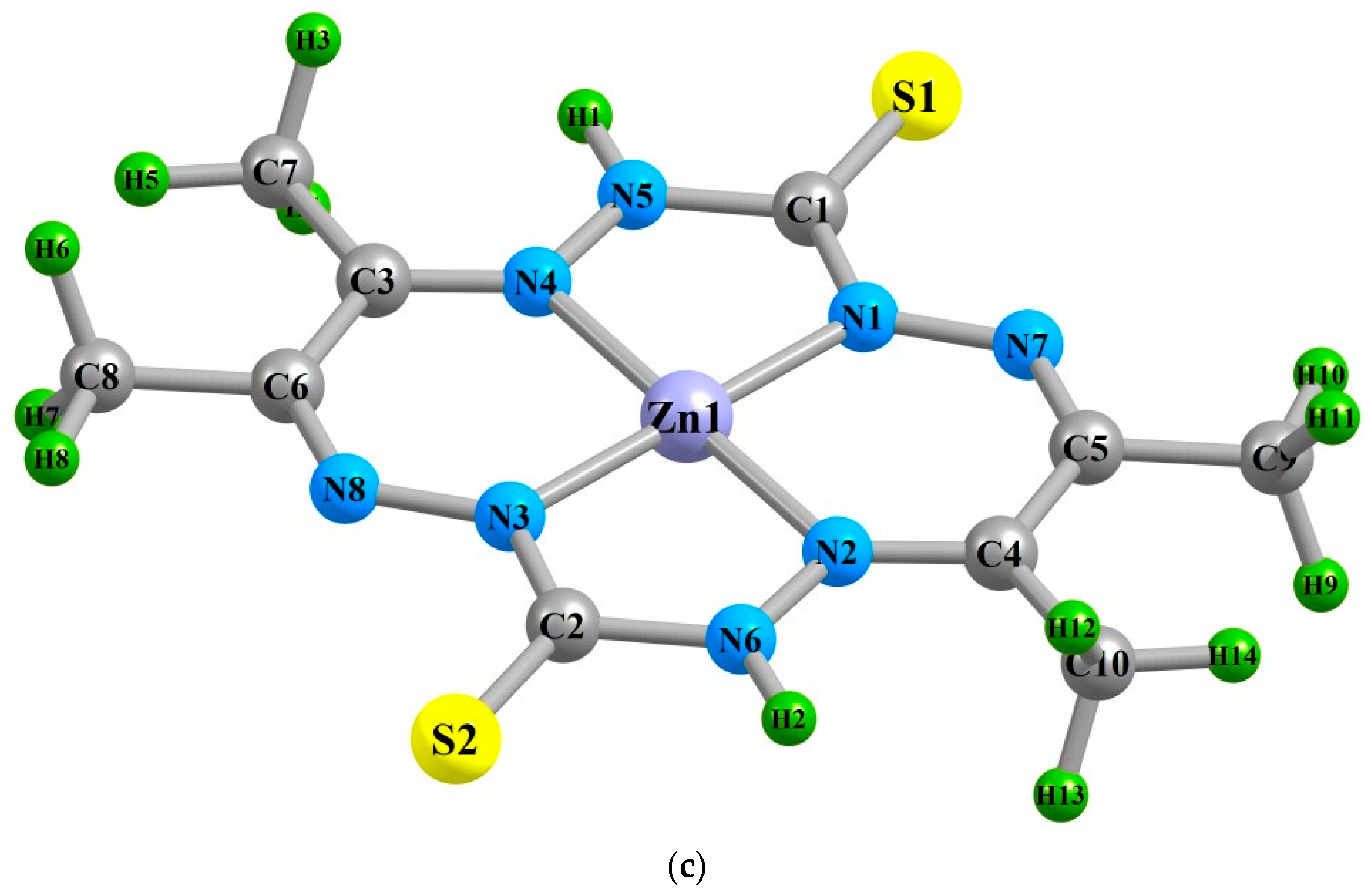
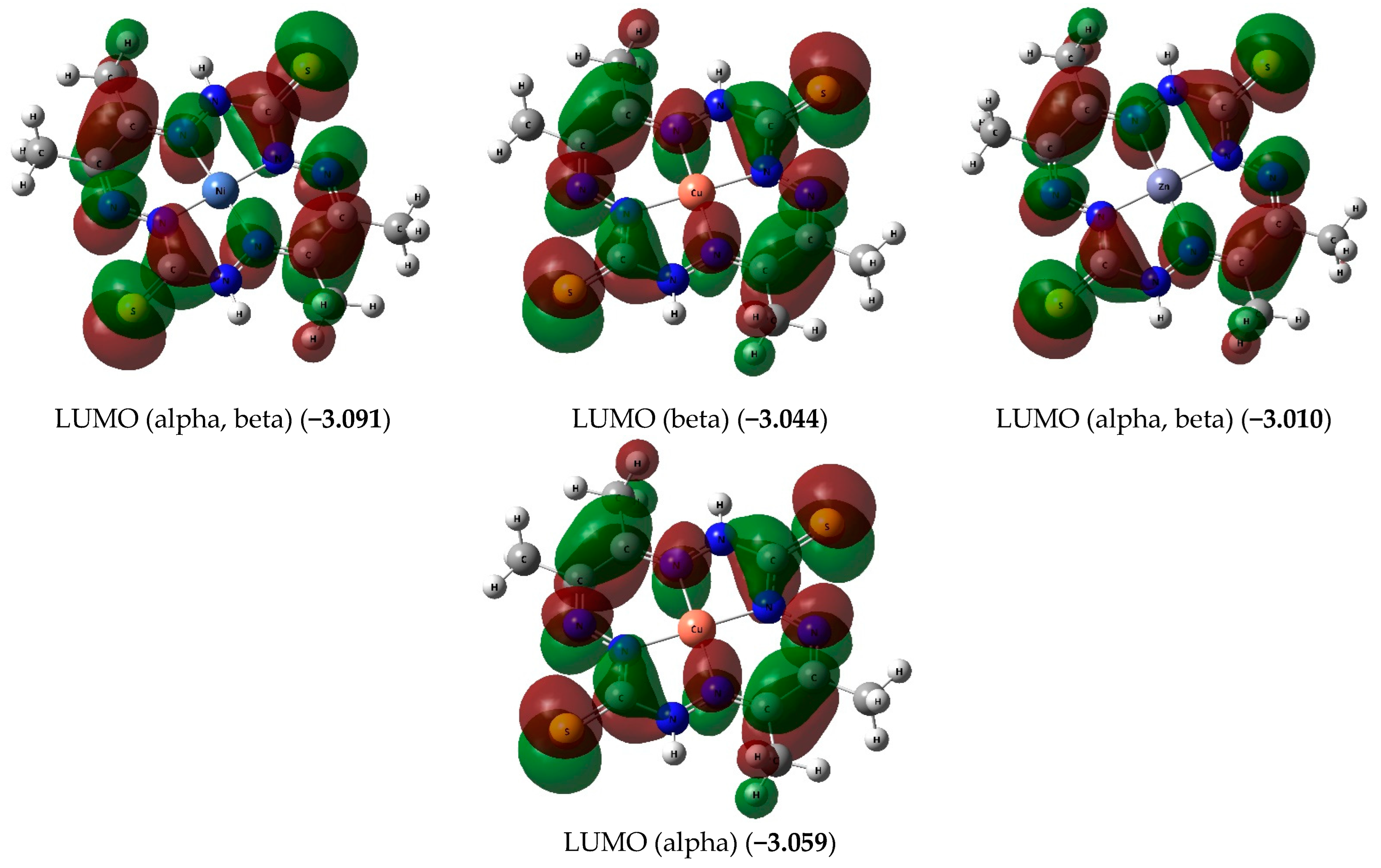
ComplexNiLCuLZnL
Chemistry ModelChemistry ModelChemistry Model
Structural ParameterB3PW91/TZVPM06/TZVPOPBE/TZVPB3PW91/TZVPM06/TZVPOPBE/TZVPB3PW91/TZVPM06/TZVPOPBE/TZVP
Bond lengths in the MN4 chelate node, pm
(M1N1)183.9184.2183.4188.9188.8189.4191.0190.5191.4
(M1N2)183.5184.2182.6190.9191.4190.9197.5198.2197.7
(M1N3)183.9184.2183.4188.9188.8189.4191.0190.5191.3
(M1N4)183.5184.2182.6190.9191.4190.9197.5198.2197.6
Separate bond lengths outside the MN4 chelate node, pm
(C1S1), (C2S2)165.9165.7165.9166.0165.7166.1166.0165.7166.0
(C1N5), (C2N6)137.8138.0137.7139.8140.0140.0141.6141.7141.8
(N2N6), (N4N5)135.6135.7135.1134.9134.9134.2134.3134.3133.8
(C4C5), (C3C6)145.7146.0145.1147.5147.7146.9148.8148.8148.2
(C5N7), (C6N8)130.1129.5131.2130.4129.8131.6130.7130.0132.0
(C6C8), (C5C9)150.6150.1150.6150.8150.3150.9151.0150.4151.1
(C4C10), (C7C8)149.9149.4149.9150.0149.5150.1150.1150.0150.2
Bond angles in the MN4 chelate node, deg
(N1M1N2)94.194.194.194.794.794.795.195.295.0
(N2M1N3)85.985.985.985.385.385.384.984.885.0
(N3M1N4)94.194.194.194.794.794.795.195.295.0
(N4M1N1)85.985.985.985.385.385.384.984.885.0
Bond angles sum (BAS)360.0360.0360.0360.0360.0360.0360.0360.0360.0
Non-bond angles in the N4 grouping, deg
(N1N2N3)90.190.090.289.489.289.588.187.788.2
(N2N3N4)89.990.089.890.690.890.591.992.391.8
(N3N4N1)90.190.090.289.489.289.588.187.788.2
(N4N1N2)89.990.089.890.690.890.591.992.391.8
Non-bond angles sum (NBAS)360.0360.0360.0360.0360.0360.0360.0360.0360.0
Bond angles in the 5-numbered (M1N4N5C1N1) chelate ring, deg
(M1N4N5)110.5110.3110.9109.0108.9109.1107.9107.6107.8
(N4N5C1)119.1119.2119.3120.5120.5120.9121.1121.0121.6
(N5C1N1)109.7109.8109.1111.1111.1110.7111.9112.0111.6
(C1N1M1)114.8114.8114.8114.1114.2114.0114.2114.5114.0
(N1M1N4)85.985.985.985.385.385.384.984.885.0
Bond angles sum (VAS51)540.0540.0540.0540.0540.0540.0540.0540.0540.0
Bond angles in the 5-numbered (M1N2N6C2N3) chelate ring, deg
(M1N2N6)110.5110.3110.9109.0108.9109.1107.9107.6107.8
(N2N6C2)119.1119.2119.3120.5120.5120.9121.1121.0121.6
(N6C2N3)109.7109.8109.1111.1111.1110.7111.9112.0111.6
(C2N3M1)114.8114.8114.8114.1114.2114.0114.2114.5114.0
(N3M1N2)85.985.985.985.385.385.384.984.885.0
Bond angles sum (VAS52)540.0540.0540.0540.0540.0540.0540.0540.0540.0
Bond angles in the 6-numbered (M1N1N7C5C4N2) chelate ring, deg
(M1N1N7)128.5128.3128.8127.0126.8127.1126.1125.9126.6
(N1N7C5)122.2122.4122.3122.3122.5122.4122.2122.3121.7
(N7C5C4)127.0127.2126.8129.5129.5129.6131.6131.6132.1
(C5C4N2)120.3120.4119.9120.4120.5120.0120.0120.1119.4
(C4N2M1)127.9127.6128.1126.1126.0126.2125.0124.4125.2
(N2M1N1)94.194.194.194.794.794.795.195.295.0
Bond angles sum (VAS61)720.0720.0720.0720.0720.0720.0720.0719.5720.0
Bond angles in the 6-numbered (M1N3N8C6C3N4) chelate ring, deg
(M1N3N8)128.5128.3128.8127.0126.8127.1126.1125.9126.6
(N3N8C6)122.2122.4122.3122.3122.5122.4122.2122.3121.7
(N8C6C3)127.0127.2126.8129.5129.5129.6131.6131.6132.1
(C6C3N4)120.3120.4119.9120.4120.5120.0120.0120.1119.4
(C3N4M1)127.9127.6128.1126.1126.0126.2125.0124.4125.2
(N4M1N3)94.194.194.194.794.794.795.195.295.0
Bond angles sum (VAS62)720.0720.0720.0720.0720.0720.0720.0719.5720.0
Bond angles outside chelate rings, deg
(N1C1S1), (N3C2S2)130.6130.6130.9130.7130.6131.1130.8130.7131.4
(N5N4C3), (N6N2C4)121.6122.1121.0124.8125.2124.6127.2127.6127.1
(N4C3C7), (N2C4C10)118.8118.7119.1118.6118.5118.9118.8119.3119.0
(C3C6C8), (C4C5C9)118.8118.4119.6117.3117.0117.9116.1115.6116.6
(C8C6N8), (C9C5N7)114.1114.4113.6113.2113.5112.4112.2112.8111.3
(C6C3C7), (C5C4C10)120.9120.8121.0121.0121.0121.1121.3120.6121.5
Table 2. Key data of NBO analysis for the NiL, CuL, and ZnL complexes in the ground state according to DFT B3PW91/TZVP, DFT M06/TZVP, and DFT OPBE/TZVP chemistry models.
Table 2. Key data of NBO analysis for the NiL, CuL, and ZnL complexes in the ground state according to DFT B3PW91/TZVP, DFT M06/TZVP, and DFT OPBE/TZVP chemistry models.
ComplexChemistry ModelThe Charges on the Atoms, in Electron Charge Units (ē)<S**2>
M1N1N2N3N4
NiLB3PW91/TZVP+0.379−0.316−0.187−0.316−0.1870.0000
M06/TZVP+0.382−0.335−0.198−0.335−0.1980.0000
OPBE/TZVP+0.314−0.265−0.173−0.265−0.1730.0000
CuLB3PW91/TZVP+0.729−0.411−0.257−0.411−0.2570.7500
M06/TZVP+0.711−0.425−0.262−0.425−0.2620.7500
OPBE/TZVP+0.672−0.364−0.245−0.364−0.2450.7500
ZnLB3PW91/TZVP+1.088−0.508−0.321−0.509−0.3210.0000
M06/TZVP+1.071−0.524−0.326−0.524−0.3260.0000
OPBE/TZVP+1.085−0.475−0.318−0.475−0.3180.0000
Table 3. Standard thermodynamic parameters of formation for NiL, CuL, and ZnL complexes calculated by B3PW91/TZVP, M06/TZVP, and OPBE/TZVP chemistry models.
Table 3. Standard thermodynamic parameters of formation for NiL, CuL, and ZnL complexes calculated by B3PW91/TZVP, M06/TZVP, and OPBE/TZVP chemistry models.
ComplexChemistry ModelΔfH0298, kJ/molSf0298, J/mol KΔfG0298, kJ/mol
NiLB3PW91/TZVP769.1745.5966.6
M06/TZVP891.0742.71089.4
OPBE/TZVP550.1753.4745.3
CuLB3PW91/TZVP916.4748.51114.1
M06/TZVP1052.2753.71248.3
OPBE/TZVP753.3760.5947.4
ZnLB3PW91/TZVP834.8772.51027.9
M06/TZVP983.9766.11178.9
OPBE/TZVP669.8771.5863.2
Table 4. Standard thermodynamic parameters of template synthesis reactions of NiL, CuL, and ZnL complexes in gaseous phase calculated by B3PW91/TZVP and M06/TZVP chemistry models.
Table 4. Standard thermodynamic parameters of template synthesis reactions of NiL, CuL, and ZnL complexes in gaseous phase calculated by B3PW91/TZVP and M06/TZVP chemistry models.
ComplexChemistry ModelΔrH0 298, kJΔrSr0298, J/KΔrG0 298, kJ
NiLB3PW91/TZVP−411.646.6−425.5
M06/TZVP−393.165.6−412.7
CuLB3PW91/TZVP−204.764.1−223.8
M06/TZVP−191.291.2−218.4
ZnLB3PW91/TZVP−77.080.5−101.0
M06/TZVP−66.796.0−95.3
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Mikhailov, O.V.; Chachkov, D.V. Molecular and Electronic Structures of Macrocyclic Compounds Formed at Template Synthesis in the M(II)—Thiocarbohydrazide—Diacetyl Triple Systems: A Quantum-Chemical Analysis by DFT Methods. Molecules 2023, 28, 4383. https://doi.org/10.3390/molecules28114383

AMA Style

Mikhailov OV, Chachkov DV. Molecular and Electronic Structures of Macrocyclic Compounds Formed at Template Synthesis in the M(II)—Thiocarbohydrazide—Diacetyl Triple Systems: A Quantum-Chemical Analysis by DFT Methods. Molecules. 2023; 28(11):4383. https://doi.org/10.3390/molecules28114383

Chicago/Turabian Style

Mikhailov, Oleg V., and Denis V. Chachkov. 2023. "Molecular and Electronic Structures of Macrocyclic Compounds Formed at Template Synthesis in the M(II)—Thiocarbohydrazide—Diacetyl Triple Systems: A Quantum-Chemical Analysis by DFT Methods" Molecules 28, no. 11: 4383. https://doi.org/10.3390/molecules28114383

APA Style

Mikhailov, O. V., & Chachkov, D. V. (2023). Molecular and Electronic Structures of Macrocyclic Compounds Formed at Template Synthesis in the M(II)—Thiocarbohydrazide—Diacetyl Triple Systems: A Quantum-Chemical Analysis by DFT Methods. Molecules, 28(11), 4383. https://doi.org/10.3390/molecules28114383

Article Metrics

Back to TopTop