Distribution of Bipartite and Tripartite Entanglement within a Spin-1/2 Heisenberg Star in a Magnetic Field
Abstract
1. Introduction
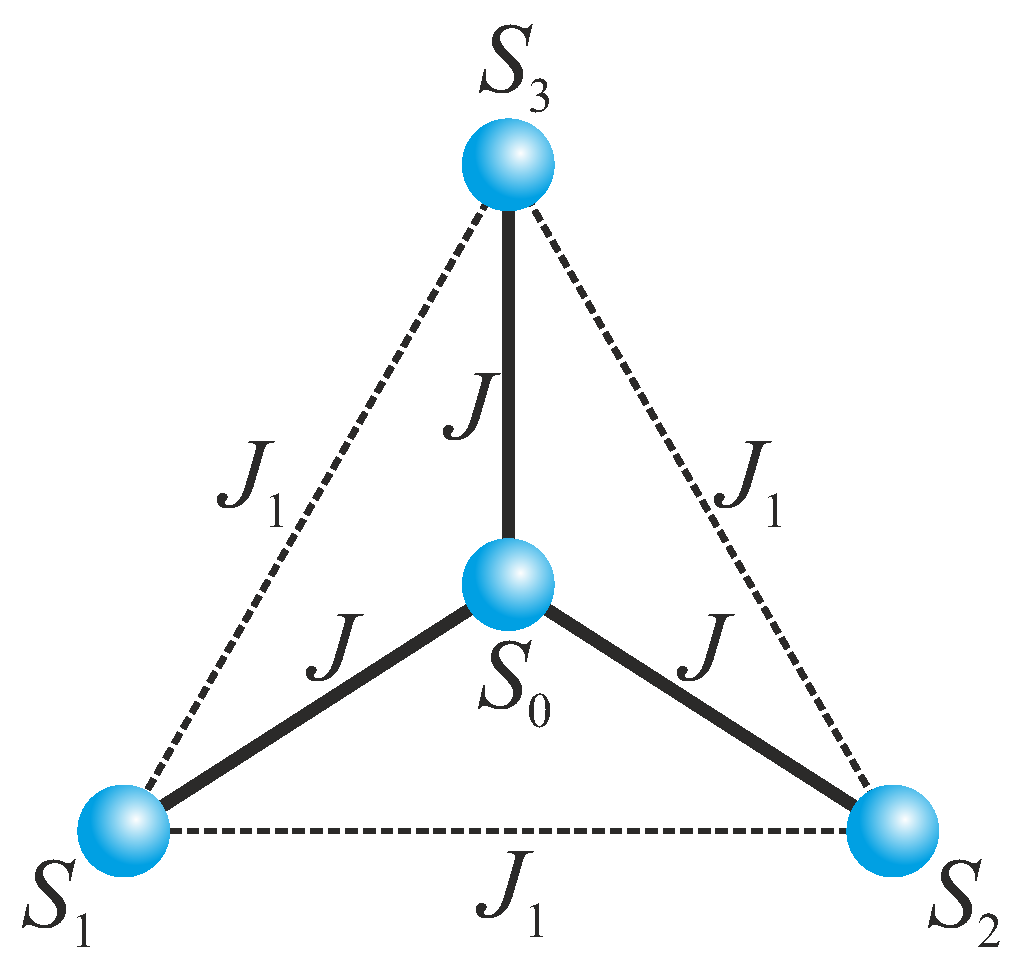
2. Model and Method
2.1. Bipartite Entanglement
2.2. Tripartite Entanglement
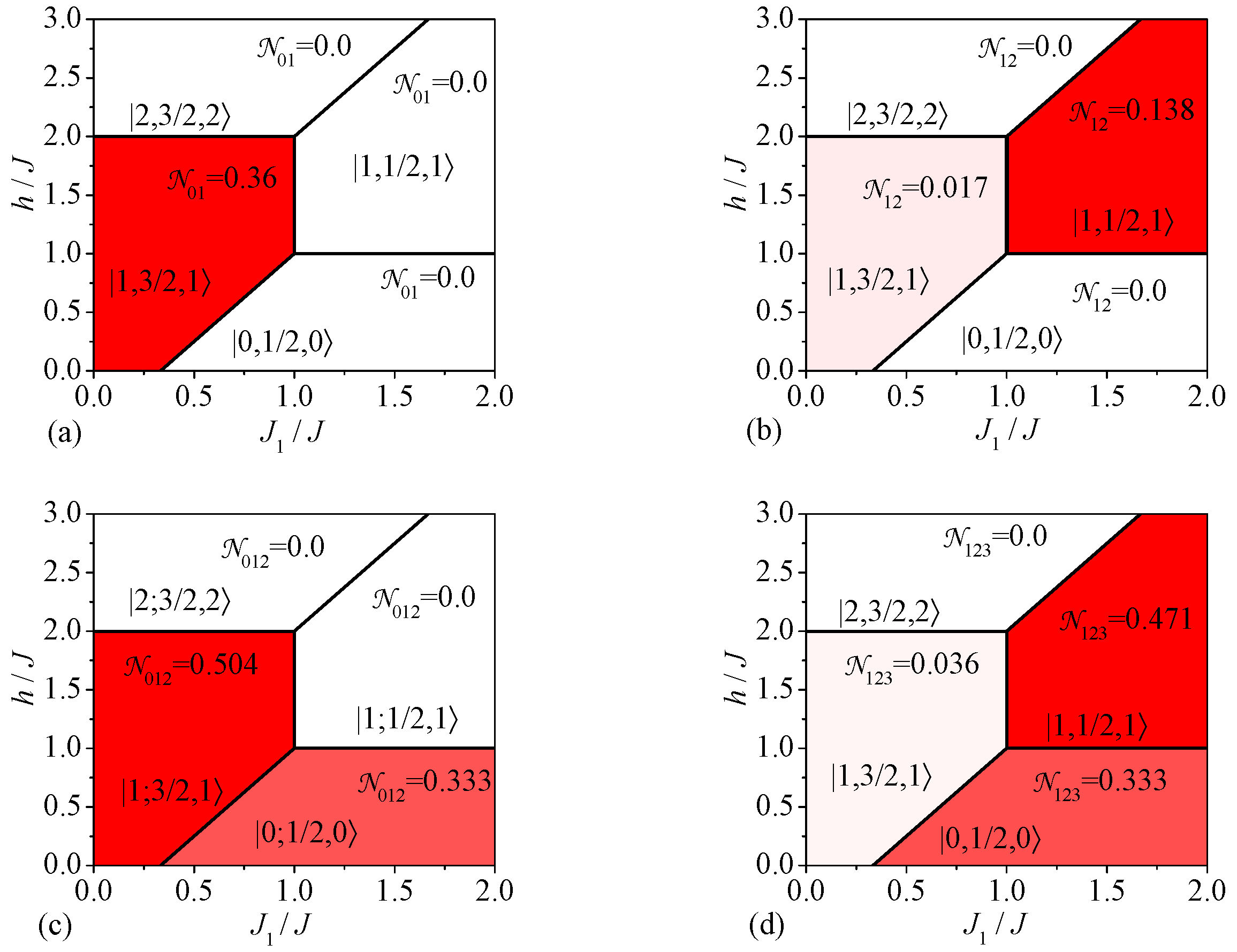
3. Results and Discussion
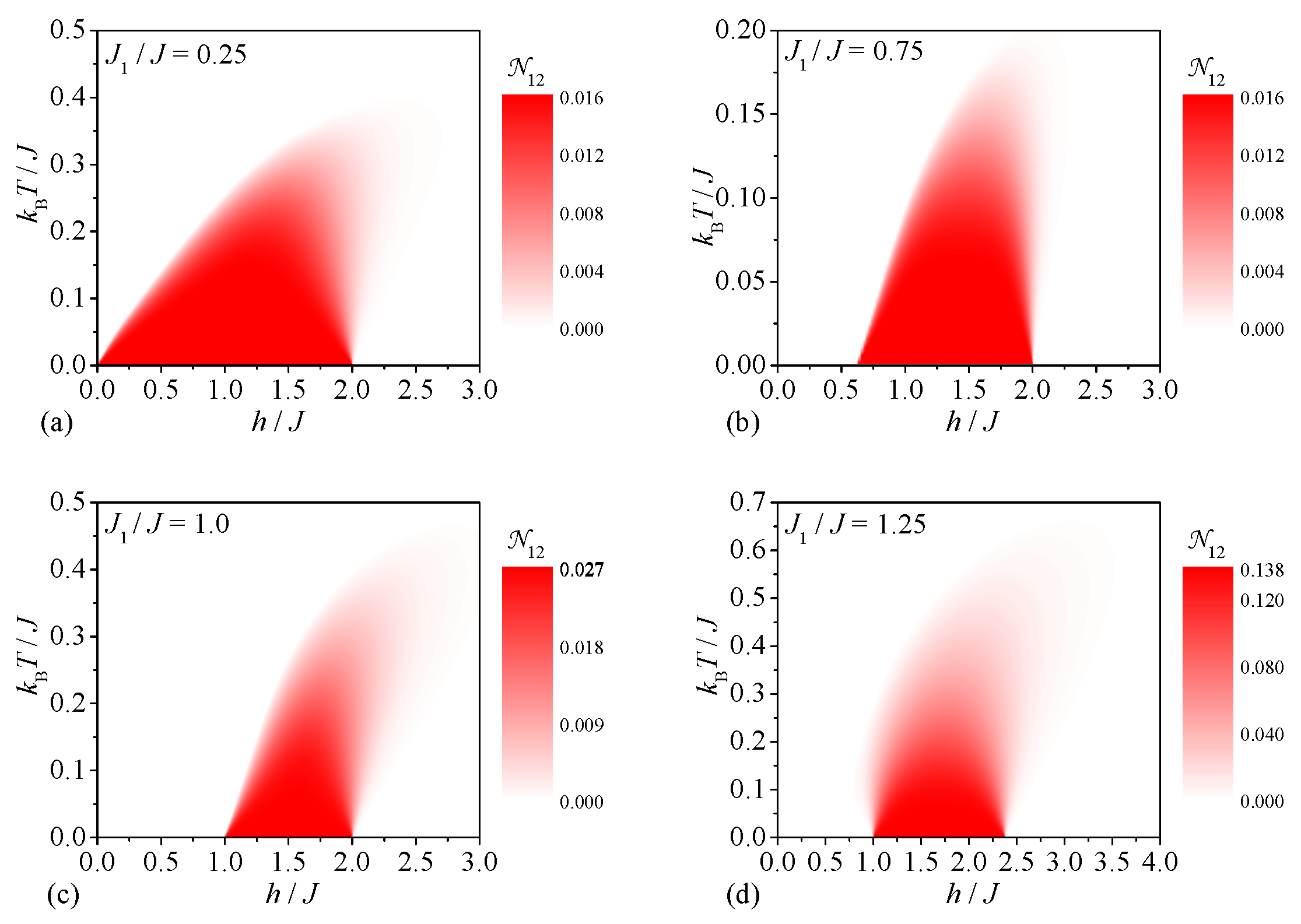
3.1. Thermal Bipartite Entanglement
3.2. Thermal Tripartite Entanglement
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
Appendix A
Appendix B
Appendix C
Appendix D
References
- Escalera-Moreno, L.; Baldoví, J.; Gaita-Arino, A.; Coronado, E. Spin states, vibrations and spin relaxation in molecular nanomagnets and spin qubits: A critical perspective. Chem. Sci. 2018, 9, 3265. [Google Scholar] [CrossRef] [PubMed]
- Ardavan, A.; Rival, O.; Morton, J.J.L.; Blundell, S.J.; Tyryshkin, A.M.; Timco, G.A.; Winpenny, R.E.P. Will Spin-Relaxation Times in Molecular Magnets Permit Quantum Information Processing? Phys. Rev. Lett. 2007, 98, 057201. [Google Scholar] [CrossRef] [PubMed]
- Ardavan, A.; Blundell, S.J. Storing quantum information in chemically engineered nanoscale magnets. J. Mater. Chem. 2009, 19, 1754. [Google Scholar] [CrossRef]
- Goodwin, C.A.P.; Ortu, F.; Reta, D.; Chilton, N.F.; Mills, D.P.; Timco, G.A. Molecular magnetic hysteresis at 60 kelvin in dysprosocenium. Nature 2017, 548, 439. [Google Scholar] [CrossRef]
- Grover, L.K. Quantum Computers Can Search Arbitrarily Large Databases by a Single Query. Phys. Rev. Lett. 1997, 79, 4709. [Google Scholar] [CrossRef]
- Leuenberger, M.N.; Loss, D. Quantum computing in molecular magnets. Nature 2001, 410, 789. [Google Scholar] [CrossRef]
- Carretta, S.; Santini, P.; Amoretti, G.; Lascialfari, A. Spin triangles as optimal units for molecule-based quantum gates. Phys. Rev. B 2007, 76, 024408. [Google Scholar] [CrossRef]
- Shor, P.W. Polynomial-time algorithms for prime factorization and discrete logarithms on a quantum computer. SIAM J. Comput. 1997, 26, 1484. [Google Scholar] [CrossRef]
- Strečka, J. A bird’s eye view of a quantum entanglement: From spooky action at a distance towards cornerstone of novel quantum technologies. Phys. B Condens. Matter 2023, 653, 414483. [Google Scholar] [CrossRef]
- Cruz, C. Quantum entanglement in low-dimensional metal complexes: An outlook. Phys. B Condens. Matter 2023, 653, 414485. [Google Scholar] [CrossRef]
- Soares-Pinto, D.O. Quantum Information Science: From foundations to new technologies. Phys. B Condens. Matter 2023, 653, 414510. [Google Scholar] [CrossRef]
- Sharma, S.; Kothari, R. Antiferromagnetic materials pave the path for quantum entanglement. Mater. Today 2021, 43, 1484. [Google Scholar] [CrossRef]
- Richter, J.; Voigt, A. The spin- 1/2 Heisenberg star with frustration: Numerical versus exact results. J. Phys. A Math. Gen. 1996, 27, 1139. [Google Scholar] [CrossRef]
- Voigt, A.; Richter, J.; Krüger, S.E. The spin 1/2 Heisenberg-star with frustration: Ground state and thermodynamics. J. Low Temp. Phys. 1995, 99, 381. [Google Scholar] [CrossRef]
- Richter, J.; Voigt, A.; Krüger, S. A solvable quantum spin model: The frustrated Heisenberg star. J. Magn. Magn. Mater. 1995, 140–144, 825. [Google Scholar] [CrossRef]
- Richter, J.; Voigt, A.; Krüger, S.E.; Gros, C. The spin-1/2 Heisenberg star with frustration: II. The influence of the embedding medium. J. Phys. A Math. Gen. 1996, 29, 825. [Google Scholar] [CrossRef]
- Pei, Y.; Journaux, Y.; Kahn, O. Ferromagnetic interactions between t2g3 and eg2 magnetic orbitals in a CrIIINi3II tetranuclear compound. Inorg. Chem. 1989, 29, 100. [Google Scholar] [CrossRef]
- Rebilly, J.-N.; Catala, L.; Riviére, E.; Guillot, R.; Wernsdorfer, W.; Mallah, T. A tetranuclear CrIIINi3II cyano-bridged complex based on M(tacn) derivative building blocks. Inorg. Chem. 2005, 44, 8194. [Google Scholar] [CrossRef]
- Prinz, M.; Kuepper, K.; Taubitz, C.H.; Raekers, M.; Khanra, S.; Biswas, B.; Weyhermüller, T.; Uhlarz, M.; Wosnitza, J.; Schnack, J.; et al. A Star-Shaped Heteronuclear CrIIIMn3II Species and Its Precise Electronic and Magnetic Structure: Spin Frustration Studied by X-Ray Spectroscopic, Magnetic, and Theoretical Methods. Inorg. Chem. 2010, 49, 2093. [Google Scholar] [CrossRef]
- Pardo, E.; Dul, M.-C.; Lescouëzec, R.; Chamoreau, L.M.; Journaux, Y.; Pasán, J.; Ruiz-Pérez, L.; Julve, M.; Lloret, F.; Ruiz-García, R.; et al. Variation of the ground spin state in homo- and hetero-octanuclear copper(ii) and nickel(ii) double-star complexes with a meso-helicate-type metallacryptand core. Dalton Trans. 2010, 39, 4786. [Google Scholar] [CrossRef]
- Motamedifar, M. Pairwise thermal entanglement and quantum discord in a three-ligand spin-star structure. Quantum Inf. Process. 2017, 16, 162. [Google Scholar] [CrossRef]
- Motamedifar, M. Dynamical pairwise entanglement and two-point correlations in the three-ligand spin-star structure. Phys. A Stat. Mech. Appl. 2017, 483, 280. [Google Scholar] [CrossRef]
- Peres, A. Separability Criterion for Density Matrices. Phys. Rev. Lett. 1996, 77, 1413. [Google Scholar] [CrossRef] [PubMed]
- Horodecki, M.; Horodecki, P.; Horodecki, R. Separability of mixed states: Necessary and sufficient conditions. Phys. Lett. A 1996, 223, 1. [Google Scholar] [CrossRef]
- Vidal, G.; Werner, R.F. Computable measure of entanglement. Phys. Rev. A 2002, 65, 032314. [Google Scholar] [CrossRef]
- Verstraete, F.; Audenaert, K.; Dehaene, J.; De Moor, B. A comparison of the entanglement measures negativity and concurrence. J. Phys. A Math. Gen. 2001, 34, 10327. [Google Scholar] [CrossRef]
- Miranowicz, A.; Grudka, A. A comparative study of relative entropy of entanglement, concurrence and negativity. J. Opt. B Quantum Semiclass. Opt. 2004, 6, 542. [Google Scholar] [CrossRef]
- Kambe, K. On the Paramagnetic Susceptibilities of Some Polynuclear Complex Salts. J. Phys. Soc. Jpn. 1950, 5, 48. [Google Scholar] [CrossRef]
- Sinn, E. Magnetic Exchange in Polynuclear Metal Complexes. Coord. Chem. Rev. 1970, 5, 313. [Google Scholar] [CrossRef]
- Sabín, C.; García-Alcaine, G. A classification of entanglement in three-qubit systems. Eur. Phys. J. D 2008, 48, 435. [Google Scholar] [CrossRef]
- Ghahi, M.G.; Akhtarshenas, S.J. Entangled graphs: A classification of four-qubit entanglement. Eur. Phys. J. D 2016, 70, 54. [Google Scholar] [CrossRef]
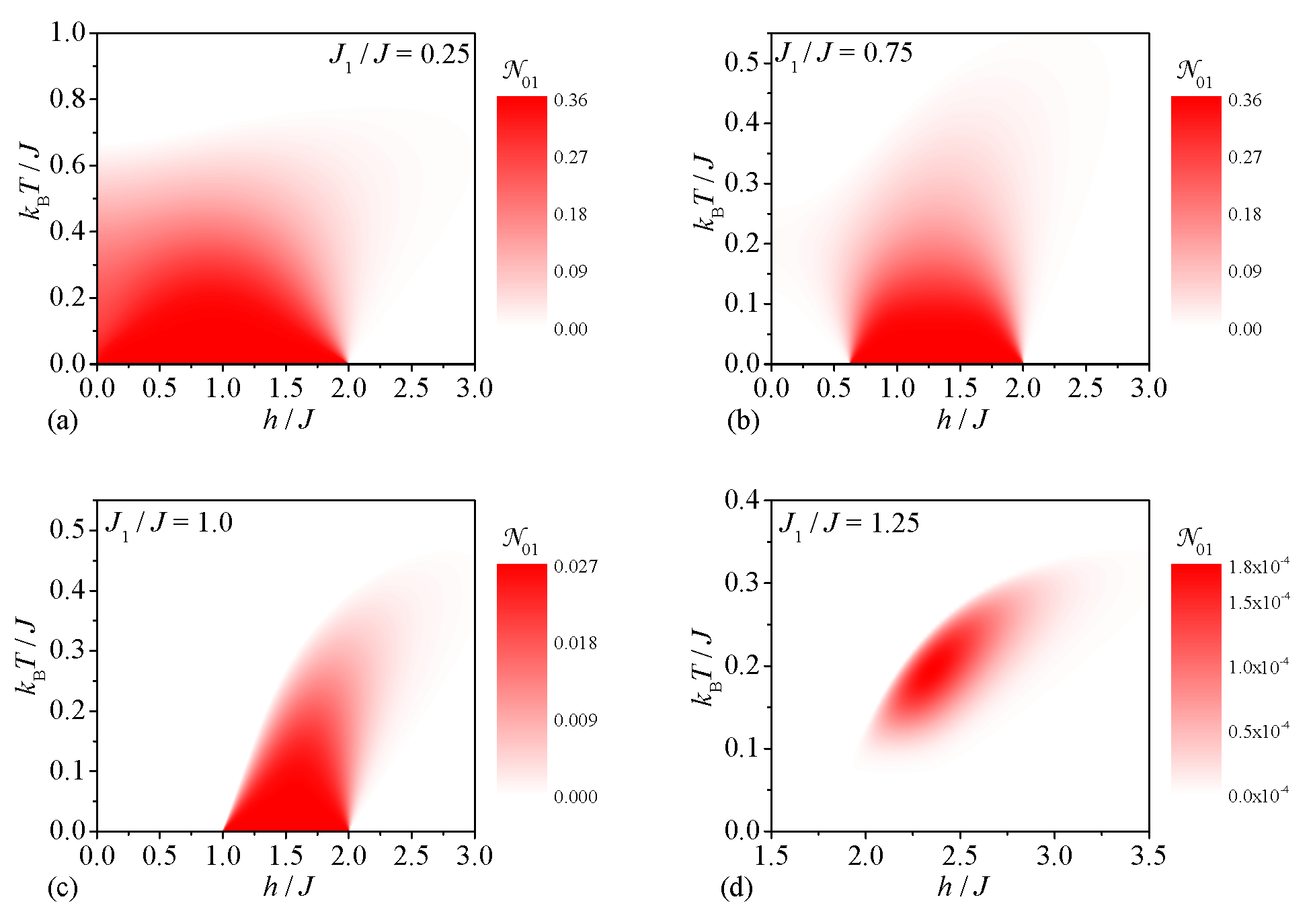
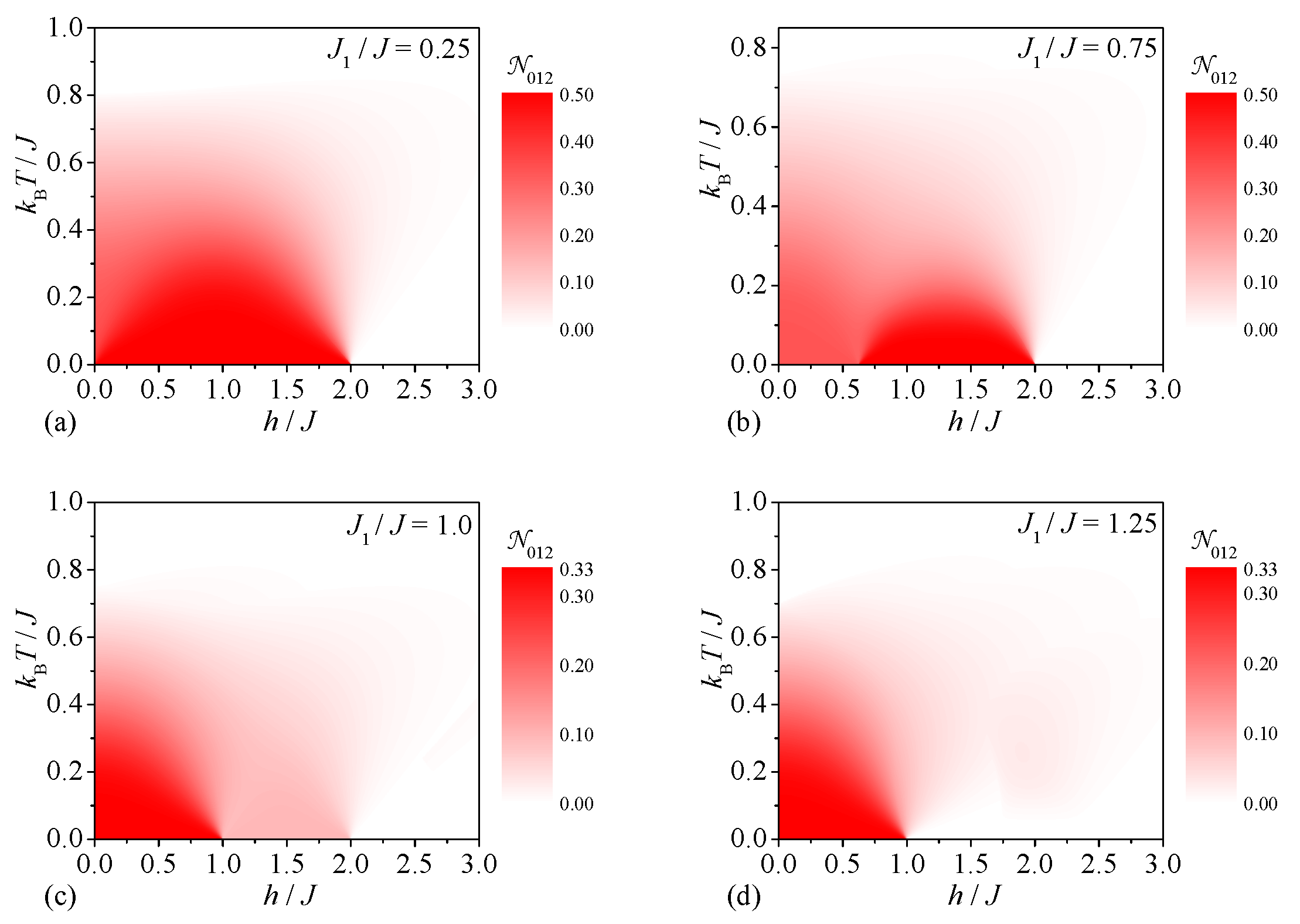
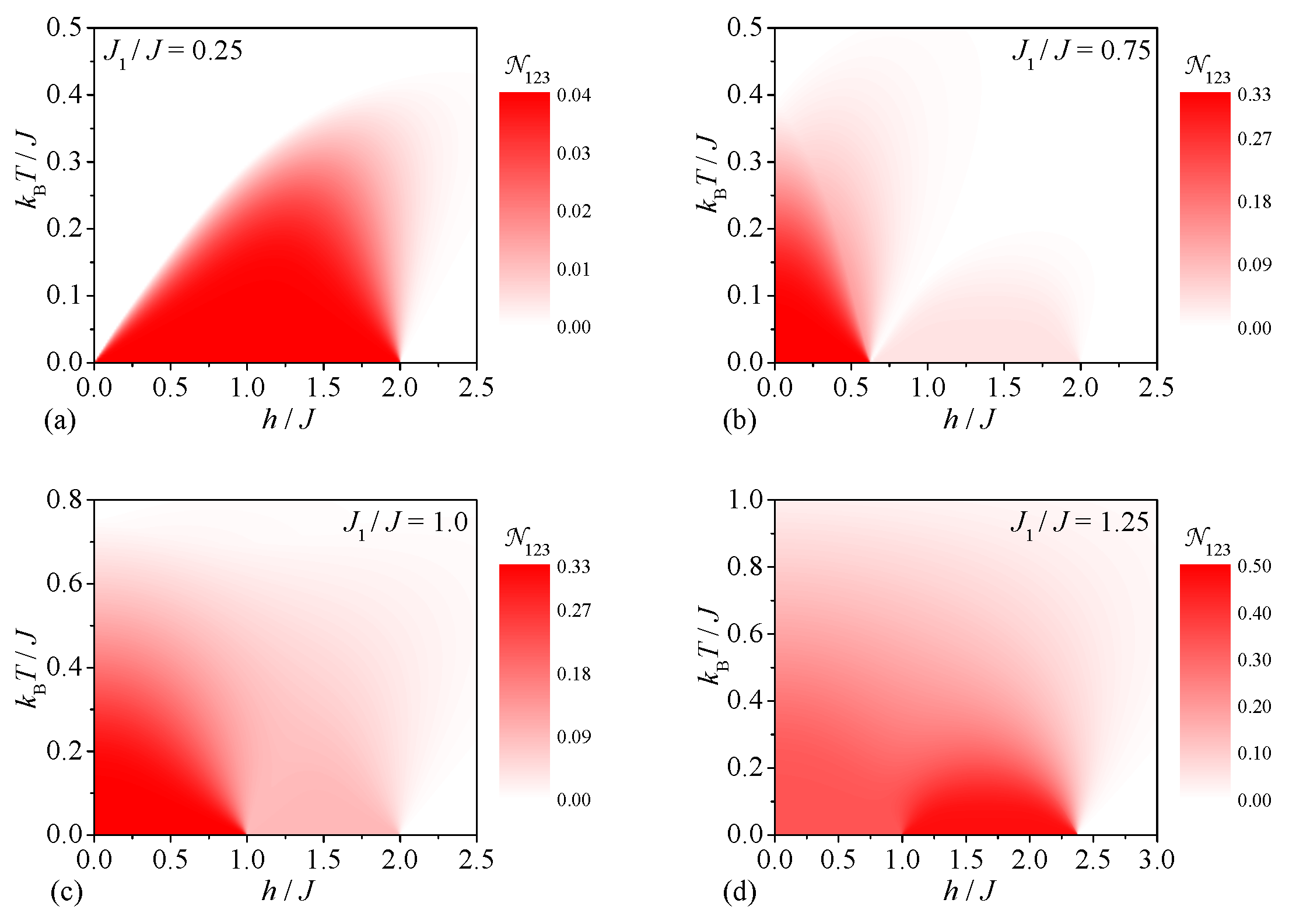
| Eigenergies | Eigenvectors | |
|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karlόvá, K.; Strečka, J. Distribution of Bipartite and Tripartite Entanglement within a Spin-1/2 Heisenberg Star in a Magnetic Field. Molecules 2023, 28, 4037. https://doi.org/10.3390/molecules28104037
Karlόvá K, Strečka J. Distribution of Bipartite and Tripartite Entanglement within a Spin-1/2 Heisenberg Star in a Magnetic Field. Molecules. 2023; 28(10):4037. https://doi.org/10.3390/molecules28104037
Chicago/Turabian StyleKarlόvá, Katarína, and Jozef Strečka. 2023. "Distribution of Bipartite and Tripartite Entanglement within a Spin-1/2 Heisenberg Star in a Magnetic Field" Molecules 28, no. 10: 4037. https://doi.org/10.3390/molecules28104037
APA StyleKarlόvá, K., & Strečka, J. (2023). Distribution of Bipartite and Tripartite Entanglement within a Spin-1/2 Heisenberg Star in a Magnetic Field. Molecules, 28(10), 4037. https://doi.org/10.3390/molecules28104037







