Heat Capacity Estimation Using a Complete Set of Homodesmotic Reactions for Organic Compounds
Abstract
1. Introduction
2. Computational Details
3. Results and Discussion
3.1. Terminology
3.2. Isobaric Molar Heat Capacities at 298 K, the KIAS16 Test Set of Organic Compounds
3.3. DFT Estimation of Gas-Phase CP at 298 K
3.4. Estimation of Gas-Phase CP (298 K) from Experimental Data by the Homodesmotic Method
3.5. Estimation of Liquid-Phase CP (298 K) by the Homodesmotic Method
EtOEt + MeOMe → 2 EtOMe
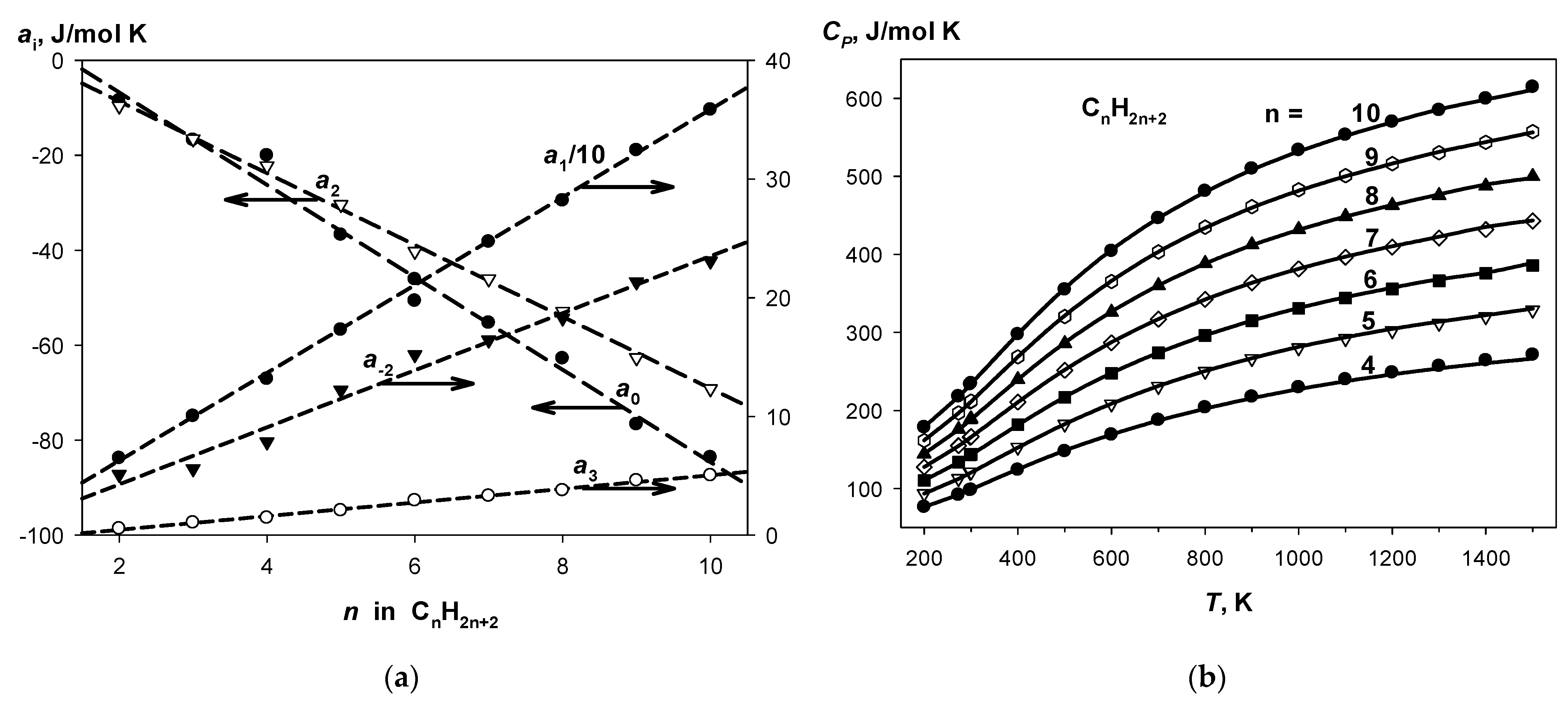
3.6. Gas-Phase Heat Capacities of n-Alkanes at 200–1500 K
- All HDRs are thermoneutral, taking into account the effect of small molecules;
- An increase in the size of a small molecule, as expected, diminishes the enthalpy correction to almost zero for n-butane;
- “Ideal” HDRs for n-alkanes should be compiled with reference compounds not less than n-butane.
4. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Benson, S.W.; Buss, J.H. Additivity rules for the estimation of molecular properties. Thermodynamic properties. J. Chem. Phys. 1958, 29, 546–572. [Google Scholar] [CrossRef]
- Benson, S.W. Thermochemical Kinetics: Methods for the Estimation of Thermochemical Data and Rate Parameters, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1976; p. 320. [Google Scholar]
- Hehre, W.J.; Ditchfield, R.; Radom, L.; Pople, J.A. Molecular orbital theory of the electronic structure of organic compounds. V. Molecular theory of bond separation. J. Am. Chem. Soc. 1970, 92, 4796–4801. [Google Scholar] [CrossRef]
- George, P.; Trachtman, M.; Bock, C.; Brett, A.M. An alternative approach to the problem of assessing stabilization energies in cyclic conjugated hydrocarbons. Theor. Chim. Acta 1975, 38, 121–129. [Google Scholar] [CrossRef]
- George, P.; Trachtman, M.; Brett, A.M.; Bock, C. Comparison of various isodesmic and homodesmotic reaction heats with values derived from published ab initio molecular orbital calculations. J. Chem. Soc. Perkin Trans. 2 1977, 8, 1036–1047. [Google Scholar] [CrossRef]
- Wheeler, S.E.; Houk, K.N.; Schleyer, P.v.R.; Allen, W.D. A hierarchy of homodesmotic reactions for thermochemistry. J. Am. Chem. Soc. 2009, 131, 2547–2560. [Google Scholar] [CrossRef] [PubMed]
- Wheeler, S.E. Homodesmotic reactions for thermochemistry. WIREs Comput. Mol. Sci. 2012, 2, 204–220. [Google Scholar] [CrossRef]
- Khursan, S.L.; Ismagilova, A.S.; Akhmerov, A.A.; Spivak, S.I. Constructing homodesmic reactions for calculating the enthalpies of formation of organic compounds. Russ. J. Phys. Chem. A 2016, 90, 796–802. [Google Scholar] [CrossRef]
- Novak, I. Computational thermochemistry of C-nitroso compounds. Struct. Chem. 2016, 27, 1395–1401. [Google Scholar] [CrossRef]
- Song, G.; Bozzelli, J.W. Structural and thermochemical studies on CH3SCH2CHO, CH3CH2SCHO, CH3SC(=O)CH3, and radicals corresponding to loss of H atom. J. Phys. Org. Chem. 2017, 30, e3688. [Google Scholar] [CrossRef]
- Song, G.; Bozzelli, J.W. Structures and thermochemistry of methyl ethyl sulfide and its hydroperoxides: HOOCH2SCH2CH3, CH3SCH(OOH)CH3, CH3SCH2CH2OOH, and radicals. J. Phys. Org. Chem. 2018, 31, e3751. [Google Scholar] [CrossRef]
- Song, G.; Bozzelli, J.W. Structural and thermochemical properties of methyl ethyl sulfide alcohols: HOCH2SCH2CH3, CH3SCH(OH)CH3, CH3SCH2CH2OH, and radicals corresponding to loss of H atom. J. Phys. Org. Chem. 2018, 31, e3836. [Google Scholar] [CrossRef]
- Zhao, Y.; Cheng, X.; Nie, K.; Han, Y.; Li, J. Structures, relative stability, bond dissociation energies, and stabilization energies of alkynes and imines from a homodesmotic reaction. Comput. Theor. Chem. 2021, 1203, 113329. [Google Scholar] [CrossRef]
- Dorofeeva, O.V.; Ryzhova, O.N. Accurate estimation of enthalpies of formation for C-, H-, O-, and N-containing compounds using DLPNO-CCSD(T1)/CBS method. Struct. Chem. 2021, 32, 553–563. [Google Scholar] [CrossRef]
- Poskrebyshev, G.A. The standard thermochemical properties of the p-benzylphenol and dimethyl phthalate, and their temperature dependencies. Comput. Theor. Chem. 2021, 1197, 113146. [Google Scholar] [CrossRef]
- Poskrebyshev, G.A. The values of ΔfHo298.15 and So298.15 of the radicals formed by the abstraction of H atom from the p-benzylphenol and dimethyl phthalate. Int. J. Chem. Kinet. 2022, 54, 619–646. [Google Scholar] [CrossRef]
- Alonso, M.; Herradón, B. A universal scale of aromaticity for π-organic compounds. J. Comput. Chem. 2010, 31, 917–928. [Google Scholar] [CrossRef] [PubMed]
- An, K.; Zhu, J. Direct energetic evaluation of aromaticity by cleaving the rings of cyclic compounds. J. Organomet. Chem. 2018, 864, 81–87. [Google Scholar] [CrossRef]
- Szatylowicz, H.; Jezuita, A.; Krygowski, T.M. On the relations between aromaticity and substituent effect. Struct. Chem. 2019, 30, 1529–1548. [Google Scholar] [CrossRef]
- Magers, D.B.; Magers, A.K.; Magers, D.H. The s-homodesmotic method for the computation of conventional strain energies of bicyclic systems and individual rings within these systems. Int. J. Quantum. Chem. 2019, 119, e25864. [Google Scholar] [CrossRef]
- Watanabe, K.; Segawa, Y.; Itami, K. A theoretical study on the strain energy of helicene-containing carbon nanobelts. Chem. Commun. 2020, 56, 15044–15047. [Google Scholar] [CrossRef]
- Akhmetshina, E.S.; Khursan, S.L. Application of group separation reaction formalism for analysis of non-valence effects of organic compounds: Three-carbon rings. Russ. Chem. Bull. 2020, 69, 76–83. [Google Scholar] [CrossRef]
- Akhmetshina, E.S.; Khursan, S.L. Complete set of homodesmotic reactions for the analysis of non-valence effects in the three-to-six-membered cyclic organic compounds. Thermochim. Acta 2020, 685, 178541. [Google Scholar] [CrossRef]
- Khursan, S.L.; Akhmetshina, E.S. Interplay of the ring and steric strains in the highly substituted cyclopropanes. J. Phys. Chem. A 2021, 125, 7607–7615. [Google Scholar] [CrossRef] [PubMed]
- Fokin, A.A.; Reshetylova, O.K.; Bakhonsky, V.V.; Pashenko, A.E.; Kivernik, A.; Zhuk, T.S.; Becker, J.; Dahl, J.E.P.; Carlson, R.M.K.; Schreiner, P.R. Synthetic doping of diamondoids through skeletal editing. Org. Lett. 2022, 24, 4845–4849. [Google Scholar] [CrossRef]
- Planells, A.R.; Ferao, A.E. Accurate ring strain energies of unsaturated three-membered heterocycles with one group 13–16 element. Inorg. Chem. 2022, 61, 6459–6468. [Google Scholar] [CrossRef]
- Planells, A.R.; Ferao, A.E. Ring strain energies of three-membered homoatomic inorganic rings El3 and diheterotetreliranes El2Tt (Tt = C, Si, Ge): Accurate versus additive approaches. Inorg. Chem. 2022, 61, 13846–13857. [Google Scholar] [CrossRef]
- Fishtik, I.; Datta, R. Group additivity vs ab initio. J. Phys. Chem. A 2003, 107, 6698–6707. [Google Scholar] [CrossRef]
- Verevkin, S.P.; Emel’yanenko, V.N.; Diky, V.; Muzny, C.D.; Chirico, R.D.; Frenkel, M. New group-contribution approach to thermochemical properties of organic compounds: Hydrocarbons and oxygen-containing compounds. J. Phys. Chem. Ref. Data 2013, 42, 033102. [Google Scholar] [CrossRef]
- Khursan, S.L.; Ismagilova, A.S.; Spivak, S.I. A graph theory method for determining the basis of homodesmic reactions for acyclic chemical compounds. Dokl. Phys. Chem. 2017, 474, 99–102. [Google Scholar] [CrossRef]
- Khursan, S.L.; Ismagilova, A.S.; Ziganshina, F.T.; Akhmet’yanova, A.I. Constructing a complete set of homodesmic reactions using the depth-first search procedure. Russ. J. Phys. Chem. A 2021, 95, 1386–1393. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Mai, T.V.-T.; Huynh, L.K. mHDFS-HoF: A generalized multilevel homodesmotic fragment-separation reaction based program for heat-of-formation calculation for acyclic hydrocarbons. J. Comput. Chem. 2019, 40, 1360–1373. [Google Scholar] [CrossRef] [PubMed]
- Minenkova, I.; Otlyotov, A.A.; Cavallo, L.; Minenkov, Y. Gas-phase thermochemistry of polycyclic aromatic hydrocarbons: An approach integrating the quantum chemistry composite scheme and reaction generator. Phys. Chem. Chem. Phys. 2022, 24, 3163–3181. [Google Scholar] [CrossRef] [PubMed]
- McQuarrie, D.A.; Simon, J.D. Molecular Thermodynamics; University Science Books: Sausalitio, CA, USA, 1999; p. 659. [Google Scholar]
- Acree, W.E., Jr.; Chickos, J.S. JPCRD: 50 years of providing the scientific community with critically evaluated thermodynamic data, predictive methods, and large thermodynamic data compilations. J. Phys. Chem. Ref. Data 2021, 50, 033101. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09; Revision C.1; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Ditchfield, R.; Hehre, W.J.; Pople, J.A. Self-consistent molecular-orbital methods. IX. An extended gaussian-type basis for molecular-orbital studies of organic molecules. J. Chem. Phys. 1971, 54, 724–728. [Google Scholar] [CrossRef]
- Zhao, Y.; Truhlar, D.G. The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: Two new functionals and systematic testing of four M06-class functionals and 12 other functionals. Theor. Chem. Acc. 2008, 120, 215–241. [Google Scholar] [CrossRef]
- Dunning, T.H. Gaussian basis sets for use in correlated molecular calculations. I. The atoms boron through neon and hydrogen. J. Chem. Phys. 1989, 90, 1007–1023. [Google Scholar] [CrossRef]
- Curtiss, L.A.; Redfern, P.C.; Raghavachari, K. Gaussian-4 theory. J. Chem. Phys. 2007, 126, 084108. [Google Scholar] [CrossRef]
- Růžička, V.; Domalski, E.S. Estimation of the heat capacities of organic liquids as a function of temperature using group additivity. I. Hydrocarbon compounds. J. Phys. Chem. Ref. Data 1993, 22, 597–618. [Google Scholar] [CrossRef]
- Zábranský, M.; Růžička, V. Estimation of the heat capacities of organic liquids as a function of temperature using group additivity: An amendment. J. Phys. Chem. Ref. Data 2004, 33, 1071–1081. [Google Scholar] [CrossRef]
- Afeefy, H.Y.; Liebman, J.F.; Stein, S.E. Neutral thermochemical data. In NIST Chemistry Webbook, NIST Standard Reference Database Number 69; Linstrom, P.J., Mallard, W.G., Eds.; National Institute of Standards and Technology: Gaithersburg, MD, USA, 20899.
- Entropy and heat capacity of organic compounds by Glushko thermocenter, Russian academy of sciences, Moscow. In NIST Chemistry Webbook, NIST Standard Reference Database Number 69; Linstrom, P.J., Mallard, W.G., Eds.; National Institute of Standards and Technology: Gaithersburg, MD, USA, 20899.
- Domalski, E.S.; Hearing, E.D. Condensed phase heat capacity data. In NIST Chemistry Webbook, NIST Standard Reference Database Number 69; Linstrom, P.J., Mallard, W.G., Eds.; National Institute of Standards and Technology: Gaithersburg, MD, USA, 20899.
- Chao, J.; Hall, K.R.; Marsh, K.N.; Wilhoit, R.C. Thermodynamic properties of key organic oxygen compounds in the carbon range C1 to C4. Part 2. Ideal gas properties. J. Phys. Chem. Ref. Data 1986, 15, 1369–1436. [Google Scholar] [CrossRef]
- Stull, D.R.; Westrum, E.F., Jr.; Sinke, G.C. The Chemical Thermodynamics of Organic Compounds; John Wiley & Sons: New York, NY, USA, 1969. [Google Scholar]
- Engineering Toolbox. Propane—Specific Heat vs. Temperature and Pressure. Available online: https://www.engineeringtoolbox.com/specific-heat-capacity-propane-Cp-Cv-isobaric-isochoric-d_2060.html (accessed on 26 October 2022).
- Engineering Toolbox. Butane—Specific Heat vs. Temperature and Pressure. Available online: https://www.engineeringtoolbox.com/butane-C4H10-specific-heat-capacity-Cp-Cv-isobaric-isochoric-d_2087.html (accessed on 26 October 2022).
- Conner, A.Z.; Elving, P.J.; Steingiser, S. Specific heats of acetaldehyde and acetaldehyde dibutyl acetal. J. Am. Chem. Soc. 1947, 69, 1532. [Google Scholar] [CrossRef]
- Acree, W.; Chickos, J.S. Phase transition enthalpy measurements of organic and organometallic compounds. Sublimation, vaporization and fusion enthalpies from 1880 to 2015. Part 1. C1—C10. J. Phys. Chem. Ref. Data 2016, 45, 033101. [Google Scholar] [CrossRef]
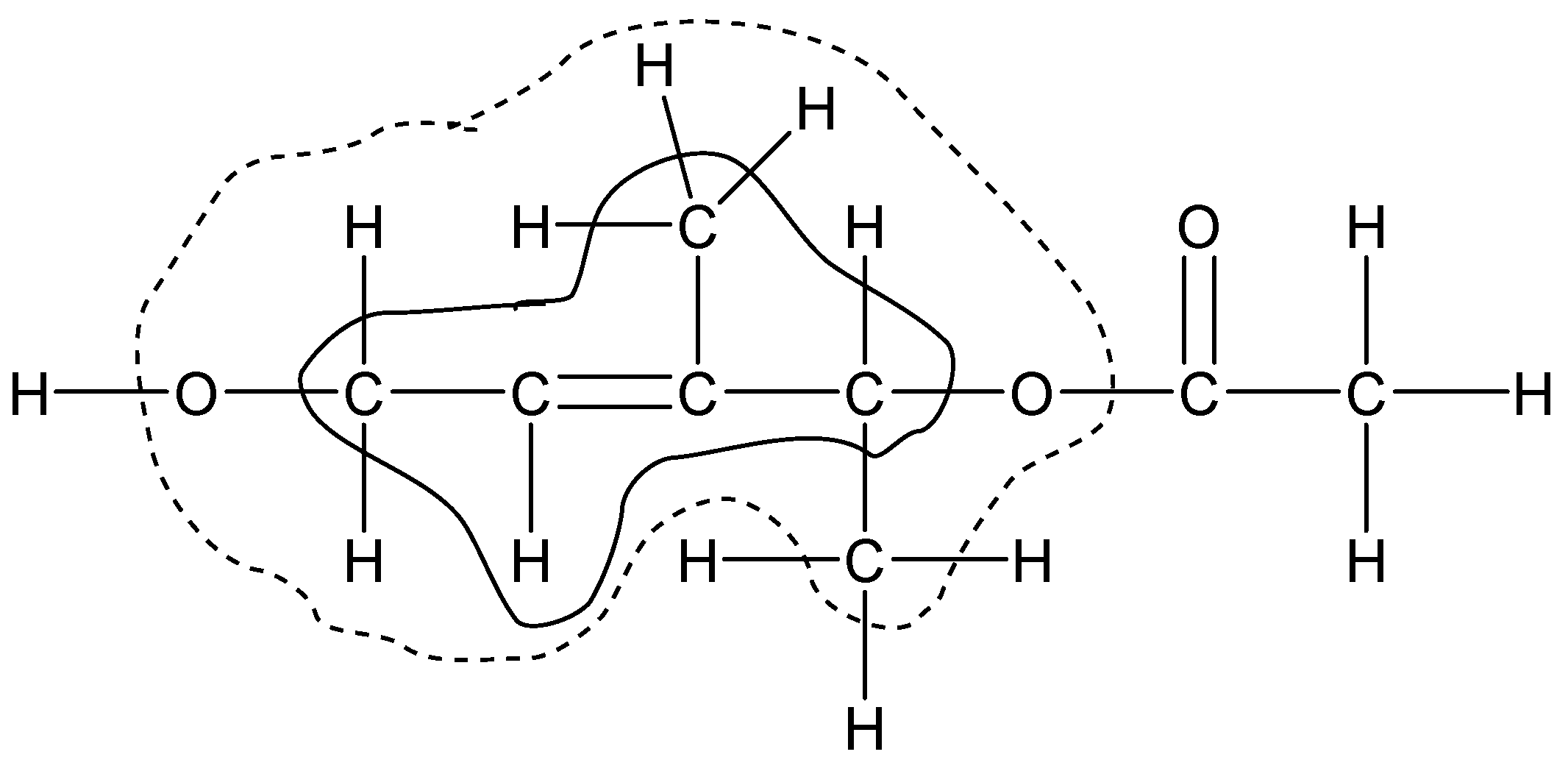
| Group of Compounds 1 | Number of HDRs | B3LYP/6-31G(d) | M06-2X/cc-pVTZ |
|---|---|---|---|
| Hydrocarbons (16) | 44 | 0.53 | 1.64 |
| Alcohols and ethers (20) | 60 | 1.04 | 2.00 |
| Carbonyl compounds (7) | 14 | 0.53 | 0.91 |
| N-containing (10) | 20 | 0.44 | 1.27 |
| KIAS16 test set (53) | 138 | 0.74 | 1.67 |
| including HDR2 and 3 | 24 | 0.25 | 0.78 |
| Level of HDR | Homodesmotic Reaction | CP |
|---|---|---|
| 1 | EtC(O)Et + MeCHO → EtC(O)Me + EtCHO | 127.1 |
| 1 | EtC(O)Et + 2 MeCHO → MeC(O)Me + 2 EtCHO | 125.8 |
| 2 | EtC(O)Et + EtCHO → EtC(O)Me + PrCHO | 124.3 |
| 2 | EtC(O)Et + 2 MeC(O)Me → 2 EtC(O)Me | 128.3 |
| Average: | CP (298 K) of pentanone-3 is | 126.4 ± 1.7 |
| No. | Compound | CP | Comment |
|---|---|---|---|
| 1 | EtCHO | 85.5 | Equation 38 |
| 2 | MeOCH2CH2OH | 100.1 ± 0.7 | Equations 20.1–20.4 |
| 3 | Me2CHCH2OH | 108.6 | Equation 22 |
| 4 | PrOMe | 108.1 ± 0.9 | Equations 24.1–24.4 |
| 5 | Me2CHOMe | 112.1 | Equation 26 |
| 6 | HO(CH2)4OH | 120.6 ± 2.0 | Equations 27.1–27.5 |
| 7 | MeOCH2CH2OMe | 121.9 ± 1.1 | Equations 28.1–28.5 |
| 8 | MeC(O)Pr | 123.7 ± 1.9 | Equations 41.1–41.5 |
| 9 | EtC(O)Et | 126.4 ± 1.7 | Equations 42.1–42.4 |
| 10 | Me(CH2)4OH | 130.3 ± 2.1 | Equations 29.1–29.6 |
| 11 | Me2CHCH2CH2OH | 132.3 ± 1.7 | Equations 30.1–30.3 |
| 12 | EtMeCHCH2OH | 132.6 ± 1.6 | Equations 31.1–31.3 |
| 13 | PrMeCHOH | 135.0 ± 1.0 | Equations 32.1–32.3 |
| 14 | Me2CHCH(Me)OH | 133.2 | Equation 33 |
| 15 | BuOMe | 130.4 ± 2.2 | Equations 34.1–34.7 |
| 16 | PrOEt | 129.7 ± 1.3 | Equations 35.1–35.6 |
| 17 | Me3COMe | 136.4 | Equation 36 |
| 18 | EtC≡CEt | 120.6 ± 0.4 | Equations 10.1–10.4 |
| 19 | BuCH=CH2 | 130.5 ± 2.0 | Equations 11.1–11.5 |
| 20 | Et2C=CH2 | 131.6 ± 0.9 | Equations 12.1–12.4 |
| Group of Compounds | Gas | Liquid | ||
|---|---|---|---|---|
| Number of HDRs | MA ΔCP | Number of HDRs | MA ΔCP | |
| Hydrocarbons | 44 (16) | 1.66 | 26 (9) | 3.18 |
| Alcohols and ethers | 56 (16) | 1.23 | 47 (11) | 2.17 |
| Carbonyl compounds | 12 (5) | 1.59 | 13 (6) | 3.80 |
| N-containing | – | – | 16 (6) | 3.37 |
| KIAS16 test set | 112 (37) | 1.44 | 102 (32) | 2.83 |
| including HDR2 and HDR3 | 20 | 1.36 | 18 | 2.68 |
| No. | Compound | CP | Comment |
|---|---|---|---|
| 1 | MeCHO | 102 | Equations 40; 41.1,3; 42.1,2 |
| 2 | MeC(O)NH2 | 94.0 | Equation 45 |
| 3 | MeOMe | 102.8 | Equations 19; 28.3 |
| 4 | C2H6 | 75.5 | Equations 17; 18 |
| 5 | EtNH2 | 129.4 ± 6.2 | Equations 44; 47.2,3,5 |
| 6 | EtC(O)NH2 | 126.7 | Equation 51 |
| 7 | EtOMe | 133.9 | Equations 19; 28.3 |
| 8 | C3H8 | 107.9 | Liquid alkanes regression |
| 9 | Et2CHCHO | 156.1 | Equation 43 |
| 10 | Me2C=CH2 | 130.2 | Equation 5 |
| 11 | PrC(O)NH2 | 158.1 ± 1.7 | Equations 50.1–50.3 |
| 12 | EtOEt | 165.4 | See text |
| 13 | n-C4H10 | 137.2 | Liquid alkanes regression |
| 14 | Me3CH | 133.5 ± 1.5 | Equations 7; 14.1,2; 15.2 |
| 15 | EtMeCHNH2 | 193.7 | Equation 49 |
| 16 | BuC≡N | 166.1 ± 2.9 | Equations 52.1–52.5 |
| 17 | EtMeCHC≡N | 185.5 | Equation 53 |
| 18 | EtMeCHCH2OH | 203.9 ± 6.0 | Equations 31.1–31.3 |
| 19 | PrMeCHOH | 225.0 ± 4.0 | Equations 32.1–32.3 |
| 20 | Me4C | 159.0 | Equation 16 |
| 21 | Et2C=CH2 | 183.9 ± 0.6 | Equations 12.1–12.4 |
| Compound | a0 | a1 | a2 | a3 | a−2 | CP (298K) 1 | CP (calc) 2 |
|---|---|---|---|---|---|---|---|
| Ethane | −8.48 | 64.96 | −9.63 | 0.56 | 5.10 | 52.49 | 52.5 |
| Propane | −16.75 | 100.42 | −16.54 | 1.07 | 5.59 | 73.60 | 73.8 |
| Butane 3 | −26.19 | 136.84 | −23.75 | 1.61 | 9.10 | 98.49 | 97.6 |
| Pentane 3 | −35.92 | 173.84 | −31.30 | 2.19 | 11.50 | 120.0 ± 0.1 | 120.3 |
| Hexane 3 | −45.66 | 210.85 | −38.86 | 2.76 | 13.90 | 142.6 ± 0.2 | 143.0 |
| Heptane 3 | −55.40 | 247.86 | −46.42 | 3.33 | 16.31 | 165.2 ± 0.3 | 165.7 |
| Octane 3 | −65.13 | 284.87 | −53.98 | 3.91 | 18.71 | 187.8 ± 0.4 | 188.4 |
| Nonane 3 | −74.87 | 321.88 | −61.53 | 4.48 | 21.12 | 210.4 ± 0.5 | 211.1 |
| Decane 3 | −84.61 | 358.89 | −69.09 | 5.06 | 23.52 | 233.1 ± 0.6 | 233.8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khursan, S.L. Heat Capacity Estimation Using a Complete Set of Homodesmotic Reactions for Organic Compounds. Molecules 2022, 27, 7814. https://doi.org/10.3390/molecules27227814
Khursan SL. Heat Capacity Estimation Using a Complete Set of Homodesmotic Reactions for Organic Compounds. Molecules. 2022; 27(22):7814. https://doi.org/10.3390/molecules27227814
Chicago/Turabian StyleKhursan, Sergey L. 2022. "Heat Capacity Estimation Using a Complete Set of Homodesmotic Reactions for Organic Compounds" Molecules 27, no. 22: 7814. https://doi.org/10.3390/molecules27227814
APA StyleKhursan, S. L. (2022). Heat Capacity Estimation Using a Complete Set of Homodesmotic Reactions for Organic Compounds. Molecules, 27(22), 7814. https://doi.org/10.3390/molecules27227814





