Neutrally Buoyant Particle Migration in Poiseuille Flow Driven by Pulsatile Velocity
Abstract
:1. Introduction
2. Method and Problem
2.1. Lattice Boltzmann Method
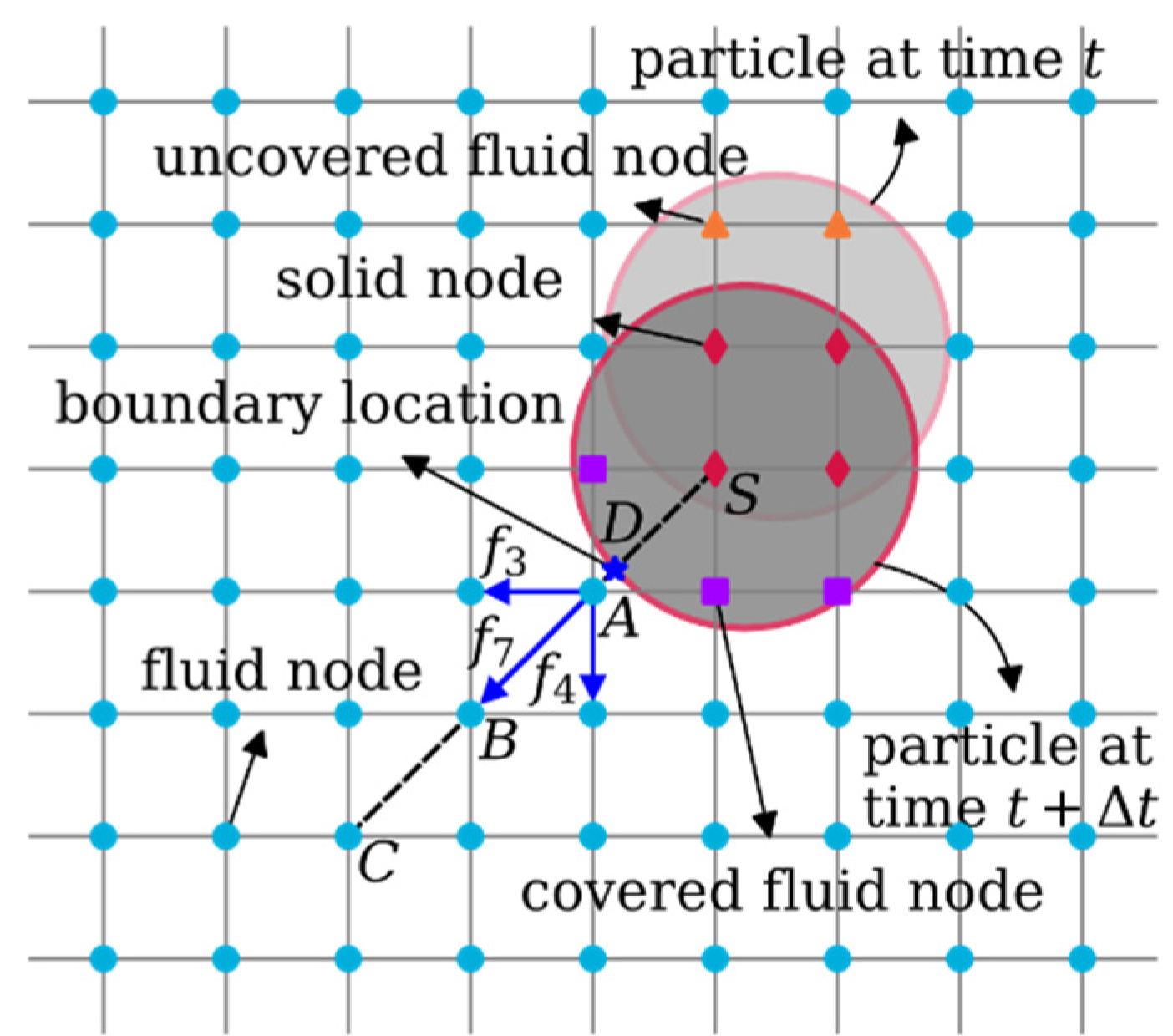
2.2. Improved Bounce-Back Scheme
2.3. Force, Torque, and Particle Motion
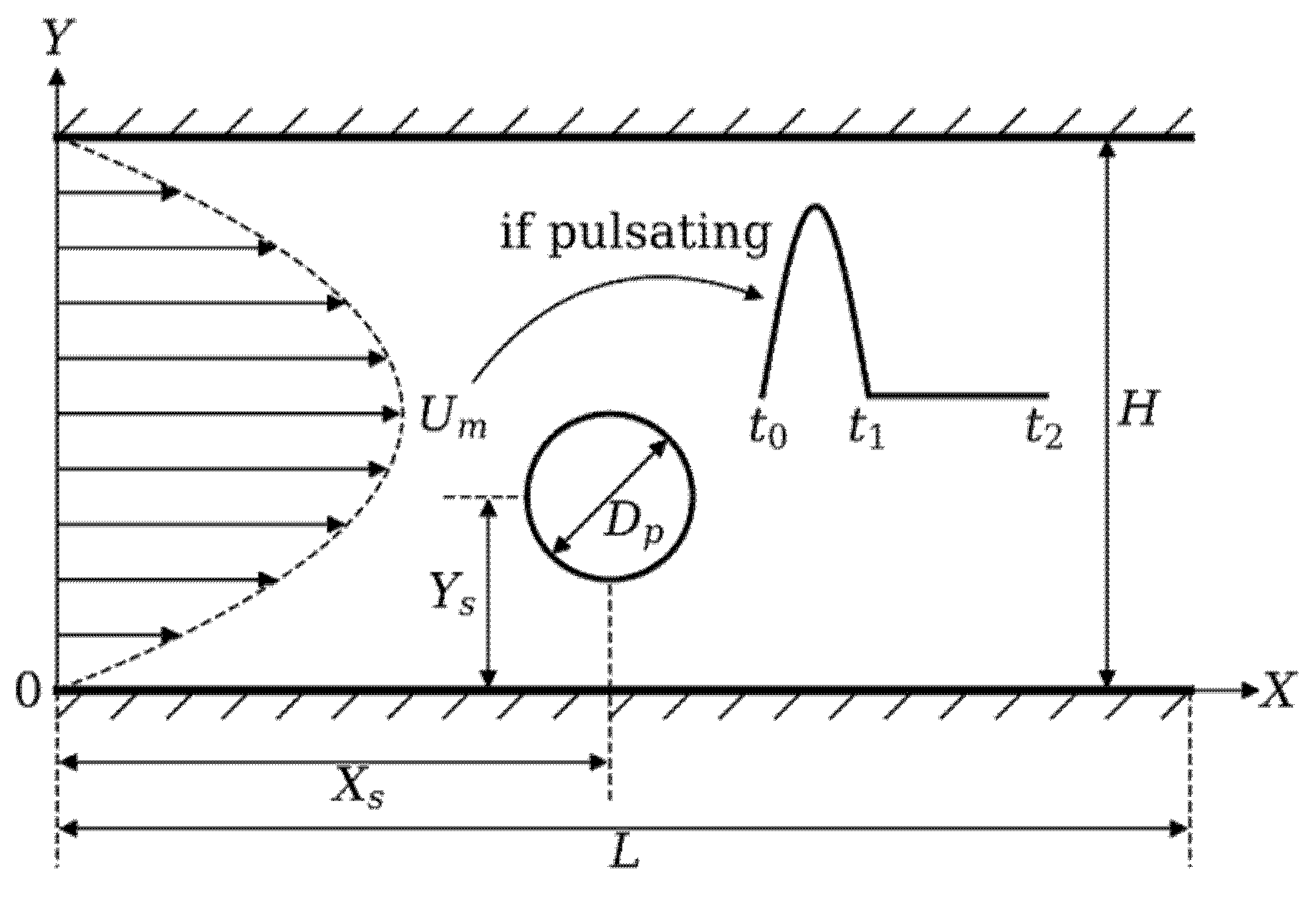
2.4. Problem
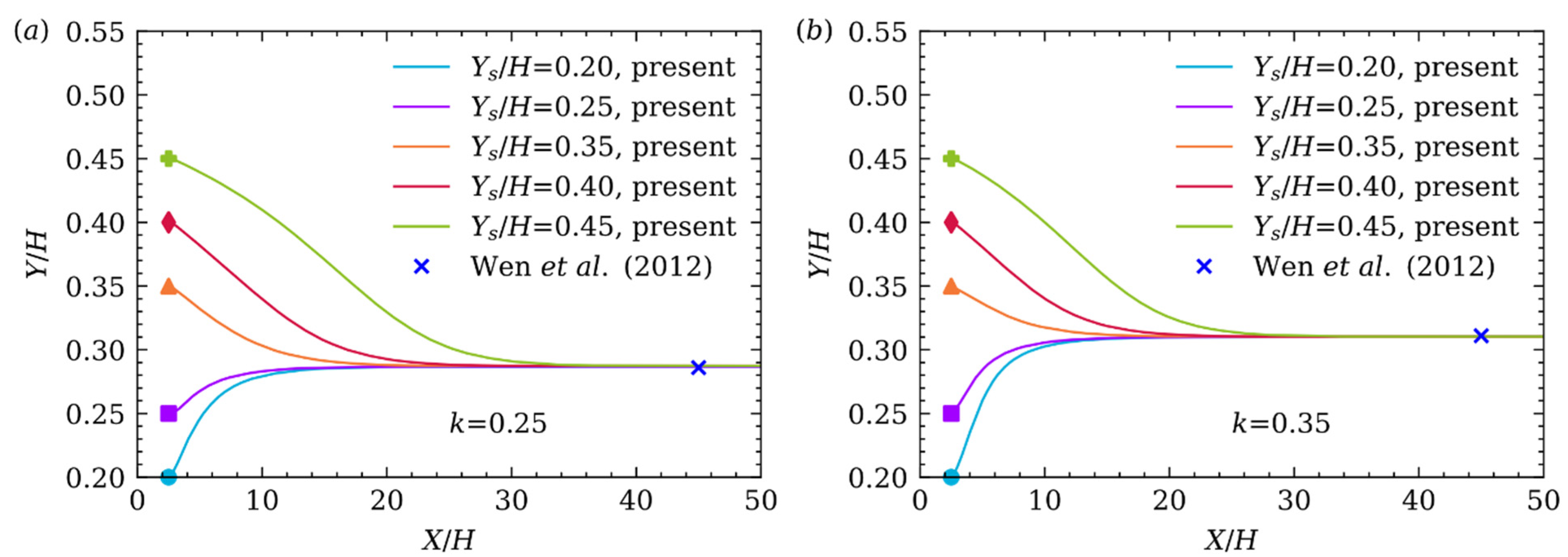
3. Validation
4. Results and Discussions
4.1. Fluid and Particle Interaction
4.2. Trajectory
4.3. Orientation
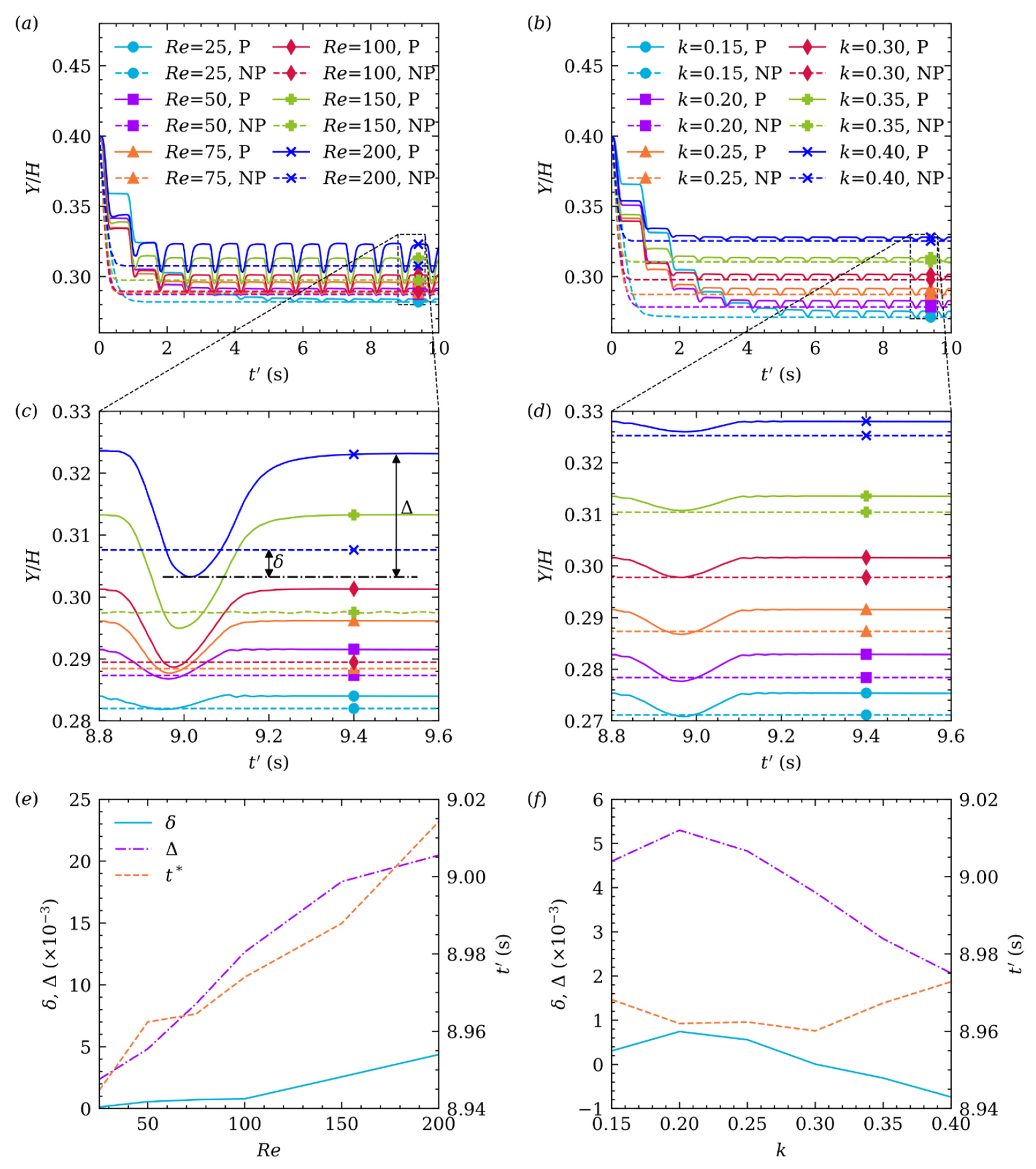
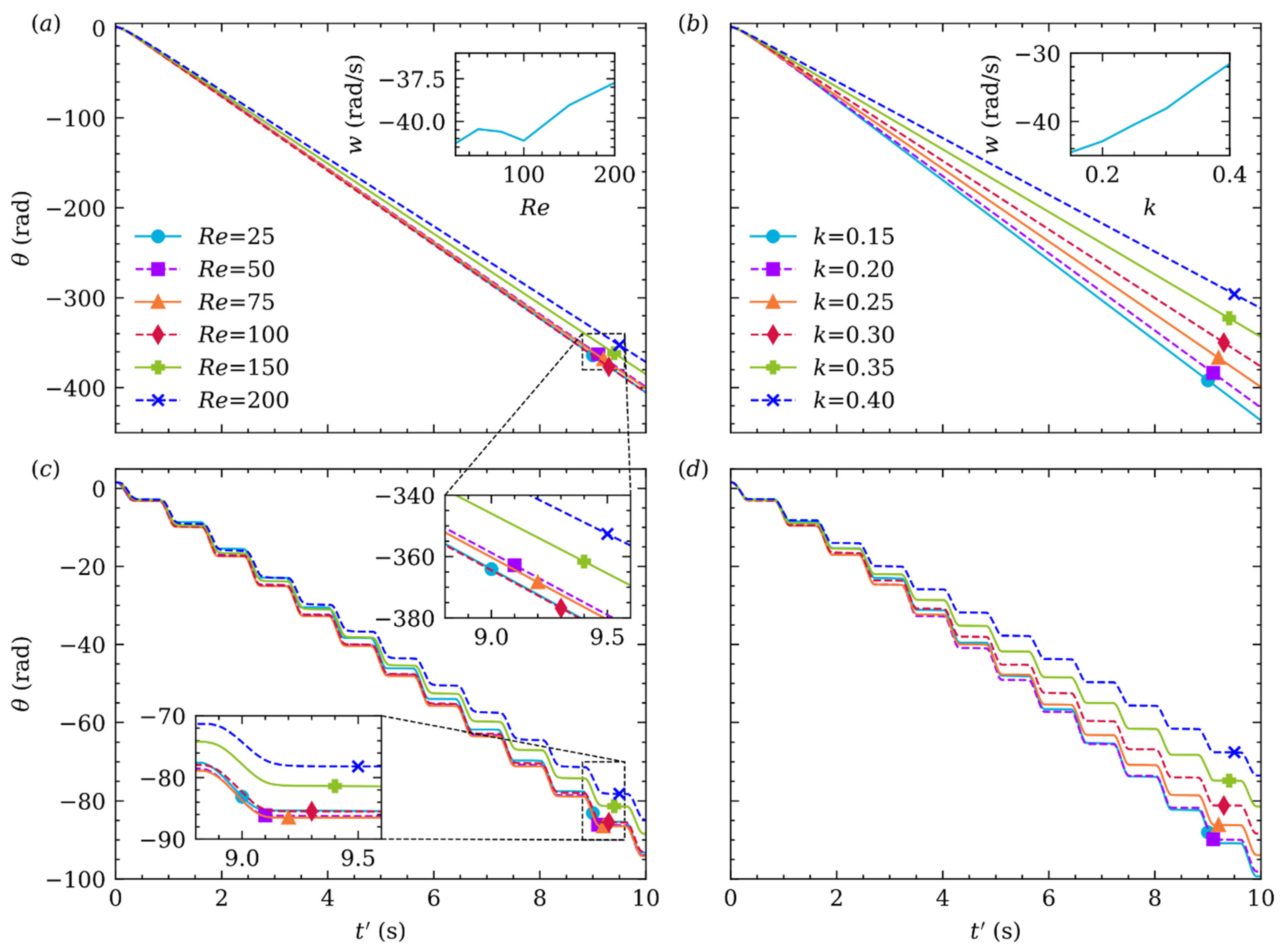
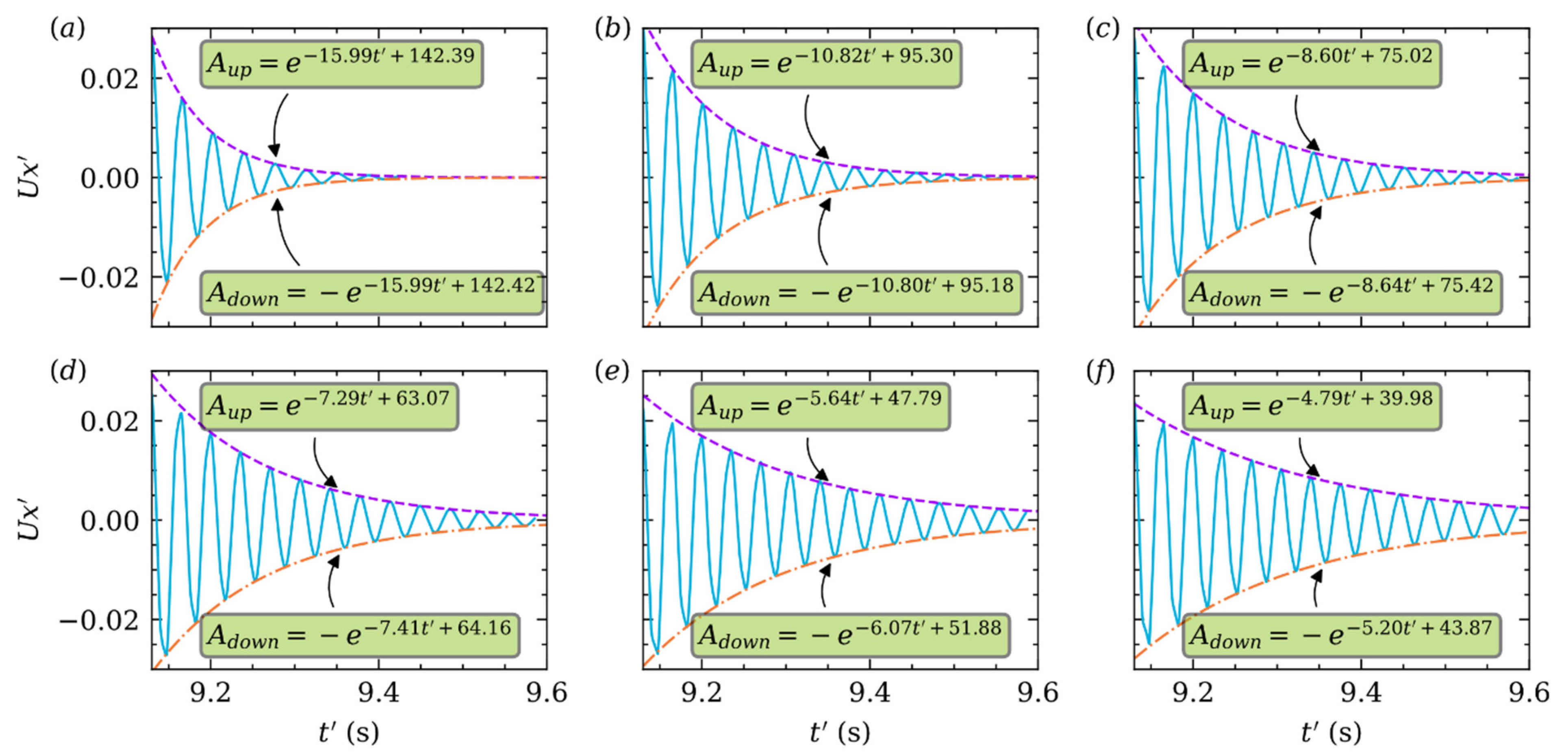
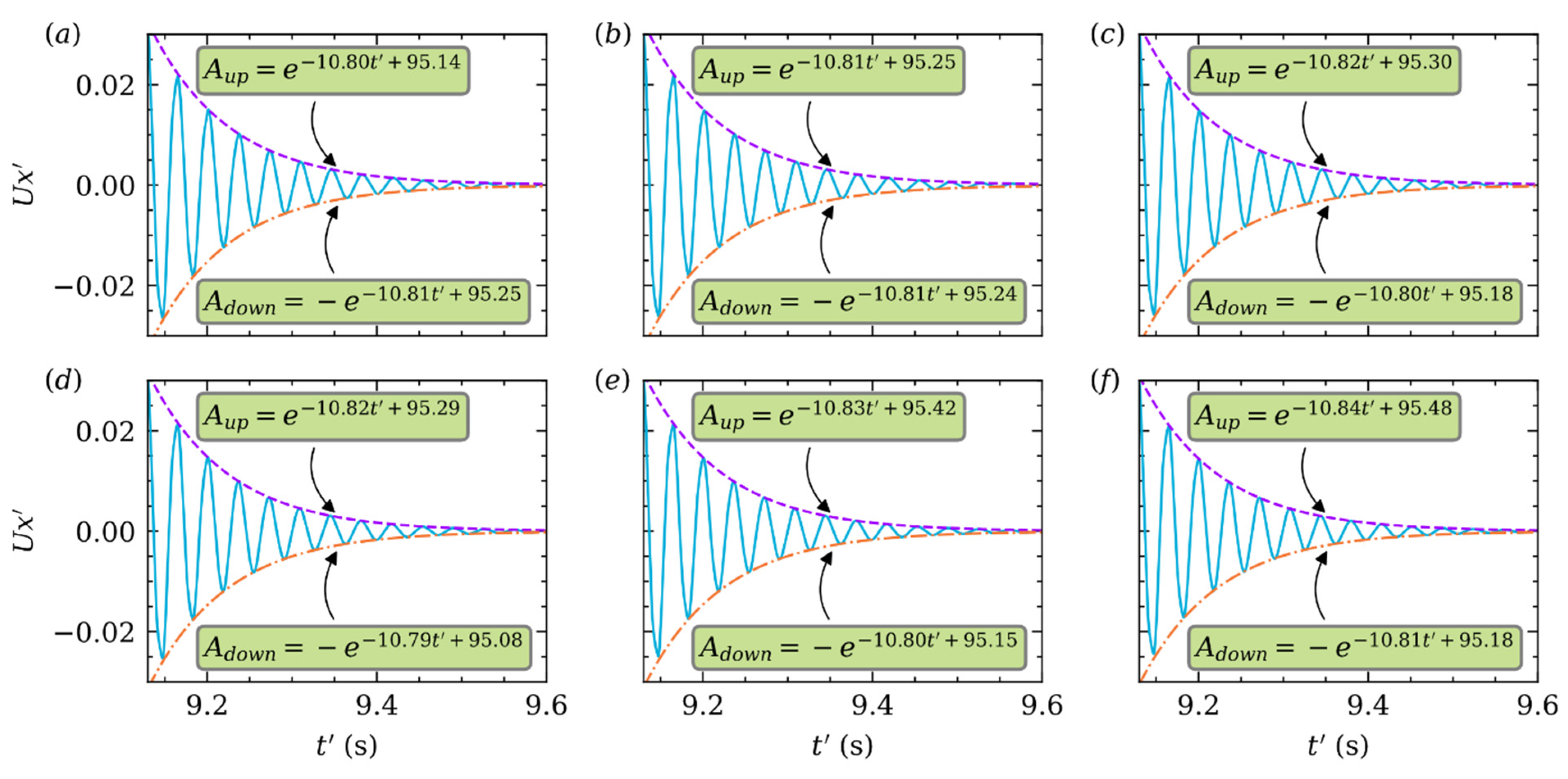
4.4. Damping
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hu, X.; Lin, J.Z.; Chen, D.M.; Ku, X.K. Influence of non-Newtonian power law rheology on inertial migration of particles in channel flow. Biomicrofluidics 2020, 14, 14105. [Google Scholar] [CrossRef]
- Hu, X.; Lin, J.Z.; Chen, D.M.; Ku, X.K. Stability condition of self-organizing staggered particle trains in channel flow. Microfluid Nanofluidics 2020, 24, 25. [Google Scholar] [CrossRef]
- Jiang, R.J.; Lin, J.Z.; Tu, C.X. Poiseuille flow-induced vibrations of a cylinder in subcritical conditions. J. Fluids Struct. 2018, 82, 272–286. [Google Scholar] [CrossRef]
- Li, G.J.; McKinley, G.H.; Ardekani, A.M. Dynamics of particle migration in channel flow of viscoelastic fluids. J. Fluid Mech. 2015, 785, 486–505. [Google Scholar] [CrossRef] [Green Version]
- Yu, Z.S.; Wang, P.; Lin, J.Z.; Hu, H.H. Equilibrium positions of the elasto-inertial particle migration in rectangular channel flow of Oldroyd-B viscoelastic fluids. J. Fluid Mech. 2019, 868, 316–340. [Google Scholar] [CrossRef]
- Zhang, J.; Yuan, D.; Zhao, Q.B.; Teo, A.J.T.; Yan, S.; Ooi, C.H.; Li, W.H.; Nguyen, N.T. Fundamentals of Differential Particle Inertial Focusing in Symmetric Sinusoidal Microchannels. Anal. Chem. 2019, 91, 4077–4084. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Segré, G.; Silberberg, A. Behaviour of macroscopic rigid spheres in Poiseuille flow Part 2. Experimental results and interpretation. J. Fluid Mech. 1962, 14, 136–157. [Google Scholar] [CrossRef]
- Ho, B.P.; Leal, L.G. Migration of rigid spheres in a two-dimensional unidirectional shear flow of a second-order fluid. J. Fluid Mech. 1976, 76, 783–799. [Google Scholar] [CrossRef] [Green Version]
- Asmolov, E.S. The inertial lift on a spherical particle in a plane Poiseuille flow at large channel Reynolds number. J. Fluid Mech. 1999, 381, 63–87. [Google Scholar] [CrossRef]
- Matas, J.P.; Morris, J.F.; Guazzelli, É. Inertial migration of rigid spherical particles in Poiseuille flow. J. Fluid Mech. 2004, 515, 171–195. [Google Scholar] [CrossRef] [Green Version]
- Matas, J.P.; Morris, J.F.; Guazzelli, É. Lateral force on a rigid sphere in large-inertia laminar pipe flow. J. Fluid Mech. 2009, 621, 59–67. [Google Scholar] [CrossRef]
- Hood, K.; Lee, S.; Roper, M. Inertial migration of a rigid sphere in three-dimensional Poiseuille flow. J. Fluid Mech. 2015, 765, 452–479. [Google Scholar] [CrossRef] [Green Version]
- Morita, Y.; Itano, T.; Sugihara Seki, M. Equilibrium radial positions of neutrally buoyant spherical particles over the circular cross-section in Poiseuille flow. J. Fluid Mech. 2017, 813, 750–767. [Google Scholar] [CrossRef]
- Nakayama, S.; Yamashita, H.; Yabu, T.; Itano, T.; Sugihara Seki, M. Three regimes of inertial focusing for spherical particles suspended in circular tube flows. J. Fluid Mech. 2019, 871, 952–969. [Google Scholar] [CrossRef]
- Feng, J.; Hu, H.H.; Joseph, D.D. Direct simulation of initial value problems for the motion of solid bodies in a Newtonian fluid. Part 2. Couette and Poiseuille flows. J. Fluid Mech. 1994, 277, 271–301. [Google Scholar] [CrossRef] [Green Version]
- Li, H.B.; Lu, X.Y.; Fang, H.P.; Qian, Y.H. Force evaluations in lattice Boltzmann simulations with moving boundaries in two dimensions. Phys. Rev. E 2004, 70, 26701. [Google Scholar] [CrossRef]
- Wen, B.H.; Li, H.B.; Zhang, C.Y.; Fang, H.P. Lattice-type-dependent momentum-exchange method for moving boundaries. Phys. Rev. E 2012, 85, 16704. [Google Scholar] [CrossRef]
- Tao, S.; Hu, J.J.; Guo, Z.L. An investigation on momentum exchange methods and refilling algorithms for lattice Boltzmann simulation of particulate flows. Comput. Fluids 2016, 133, 1–14. [Google Scholar] [CrossRef]
- Inamuro, T.; Maeba, K.; Ogino, F. Flow between parallel walls containing the lines of neutrally buoyant circular cylinders. Int. J. Multiph. Flow 2000, 26, 1981–2004. [Google Scholar] [CrossRef]
- Shao, X.M.; Yu, Z.S.; Sun, B. Inertial migration of spherical particles in circular Poiseuille flow at moderately high Reynolds numbers. Phys. Fluids 2008, 20, 103307. [Google Scholar] [CrossRef] [Green Version]
- Abbas, M.; Magaud, P.; Gao, Y.; Geoffroy, S. Migration of finite sized particles in a laminar square channel flow from low to high Reynolds numbers. Phys. Fluids 2014, 26, 123301. [Google Scholar] [CrossRef] [Green Version]
- Wang, R.J.; Sun, S.S.; Wang, W.; Zhu, Z.F. Investigation on the thermophoretic sorting for submicroparticles in a sorter with expansion-contraction microchannel. Int. J. Heat Mass Transf. 2019, 133, 912–919. [Google Scholar] [CrossRef]
- Wang, R.J.; Du, J.Y.; Guo, W.C.; Zhu, Z.F. Investigation on the Thermophoresis-Coupled Inertial Sorting of Submicrometer Particles in a Microchannel. Nanoscale Microscale Thermophys. Eng. 2016, 20, 51–65. [Google Scholar] [CrossRef]
- Du, J.Y.; Li, L.; Zhuo, Q.Y.; Wang, R.J.; Zhu, Z.F. Investigation on Inertial Sorter Coupled with Magnetophoretic Effect for Nonmagnetic Microparticles. Micromachines 2020, 11, 566. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Q.B.; Yuan, D.; Zhang, J.; Li, W.H. A Review of Secondary Flow in Inertial Microfluidics. Micromachines 2020, 11, 461. [Google Scholar] [CrossRef] [PubMed]
- Razavi Bazaz, S.; Mashhadian, A.; Ehsani, A.; Saha, S.C.; Krüger, T.; Ebrahimi Warkiani, M. Computational inertial microfluidics: A review. Lab Chip 2020, 20, 1023–1048. [Google Scholar] [CrossRef] [PubMed]
- Mutlu, B.R.; Edd, J.F.; Toner, M. Oscillatory inertial focusing in infinite microchannels. Proc. Natl. Acad. Sci. USA 2018, 115, 7682–7687. [Google Scholar] [CrossRef] [Green Version]
- Di Carlo, D. Inertial microfluidics. Lab Chip 2009, 9, 3038–3046. [Google Scholar] [CrossRef]
- Dai, Y.L.; Xu, C.; Sun, X.L.; Chen, X.Y. Nanoparticle design strategies for enhanced anticancer therapy by exploiting the tumour microenvironment. Chem. Soc. Rev. 2017, 46, 3830–3852. [Google Scholar] [CrossRef]
- Mitchell, M.J.; Billingsley, M.M.; Haley, R.M.; Wechsler, M.E.; Peppas, N.A.; Langer, R. Engineering precision nanoparticles for drug delivery. Nat. Rev. Drug Discov. 2021, 20, 101–124. [Google Scholar] [CrossRef] [PubMed]
- Aidun, C.K.; Clausen, J.R. Lattice-Boltzmann Method for Complex Flows. Annu. Rev. Fluid Mech. 2010, 42, 439–472. [Google Scholar] [CrossRef]
- Elghobashi, S. Direct Numerical Simulation of Turbulent Flows Laden with Droplets or Bubbles. Annu. Rev. Fluid Mech. 2019, 51, 217–244. [Google Scholar] [CrossRef] [Green Version]
- Ladd, A.J.C.; Verberg, R. Lattice-Boltzmann Simulations of Particle-Fluid Suspensions. J. Stat. Phys. 2001, 104, 1191–1251. [Google Scholar] [CrossRef]
- Rettinger, C.; Rüde, U. A comparative study of fluid-particle coupling methods for fully resolved lattice Boltzmann simulations. Comput. Fluids 2017, 154, 74–89. [Google Scholar] [CrossRef] [Green Version]
- Eshghinejadfard, A.; Hosseini, S.A.; Thévenin, D. Fully-resolved prolate spheroids in turbulent channel flows: A lattice Boltzmann study. AIP Adv. 2017, 7, 95007. [Google Scholar] [CrossRef] [Green Version]
- Rettinger, C.; Rüde, U. A coupled lattice Boltzmann method and discrete element method for discrete particle simulations of particulate flows. Comput. Fluids 2018, 172, 706–719. [Google Scholar] [CrossRef] [Green Version]
- Eshghinejadfard, A.; Abdelsamie, A.; Hosseini, S.A.; Thévenin, D. Immersed boundary lattice Boltzmann simulation of turbulent channel flows in the presence of spherical particles. Int. J. Multiph. Flow 2017, 96, 161–172. [Google Scholar] [CrossRef]
- Karimnejad, S.; Amiri Delouei, A.; Nazari, M.; Shahmardan, M.M.; Mohamad, A.A. Sedimentation of elliptical particles using Immersed Boundary – Lattice Boltzmann Method: A complementary repulsive force model. J. Mol. Liq. 2018, 262, 180–193. [Google Scholar] [CrossRef]
- Thorimbert, Y.; Marson, F.; Parmigiani, A.; Chopard, B.; Lätt, J. Lattice Boltzmann simulation of dense rigid spherical particle suspensions using immersed boundary method. Comput. Fluids 2018, 166, 286–294. [Google Scholar] [CrossRef]
- Jebakumar, A.S.; Magi, V.; Abraham, J. Lattice-Boltzmann simulations of particle transport in a turbulent channel flow. Int. J. Heat Mass Transf. 2018, 127, 339–348. [Google Scholar] [CrossRef]
- Peng, C.; Ayala, O.M.; Wang, L.P. A comparative study of immersed boundary method and interpolated bounce-back scheme for no-slip boundary treatment in the lattice Boltzmann method: Part I, laminar flows. Comput. Fluids 2019, 192, 104233. [Google Scholar] [CrossRef] [Green Version]
- Geneva, N.; Peng, C.; Li, X.M.; Wang, L.P. A scalable interface-resolved simulation of particle-laden flow using the lattice Boltzmann method. Parallel Comput. 2017, 67, 20–37. [Google Scholar] [CrossRef]
- Jebakumar, A.S.; Premnath, K.N.; Magi, V.; Abraham, J. Fully-resolved direct numerical simulations of particle motion in a turbulent channel flow with the lattice-Boltzmann method. Comput. Fluids 2019, 179, 238–247. [Google Scholar] [CrossRef]
- Qian, Y.H.; D’Humières, D.; Lallemand, P. Lattice BGK Models for Navier-Stokes Equation. EPL 1992, 17, 479–484. [Google Scholar] [CrossRef]
- He, X.Y.; Luo, L.S. Lattice Boltzmann Model for the Incompressible Navier–Stokes Equation. J. Stat. Phys. 1997, 88, 927–944. [Google Scholar] [CrossRef]
- Lallemand, P.; Luo, L.S. Lattice Boltzmann method for moving boundaries. J. Comput. Phys. 2003, 184, 406–421. [Google Scholar] [CrossRef]
- Mei, R.W.; Da Yu, Z.; Shyy, W.; Luo, L.S. Force evaluation in the lattice Boltzmann method involving curved geometry. Phys. Rev. E 2002, 65, 41203. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Aidun, C.K.; Lu, Y.N.; Ding, E.J. Direct analysis of particulate suspensions with inertia using the discrete Boltzmann equation. J. Fluid Mech. 1998, 373, 287–311. [Google Scholar] [CrossRef]
- Yuan, X.F.; Ball, R.C. Rheology of hydrodynamically interacting concentrated hard disks. J. Chem. Phys. 1994, 101, 9016–9021. [Google Scholar] [CrossRef]
- Pirola, S.; Cheng, Z.; Jarral, O.A.; O’Regan, D.P.; Pepper, J.R.; Athanasiou, T.; Xu, X.Y. On the choice of outlet boundary conditions for patient-specific analysis of aortic flow using computational fluid dynamics. J. Biomech. 2017, 60, 15–21. [Google Scholar] [CrossRef] [PubMed]
- Hu, X.; Lin, J.Z.; Ku, X.K. Inertial migration of circular particles in Poiseuille flow of a power-law fluid. Phys. Fluids 2019, 31, 73306. [Google Scholar]
- Nie, D.M.; Lin, J.Z. Simulation of sedimentation of two spheres with different densities in a square tube. J. Fluid Mech. 2020, 896, A12. [Google Scholar] [CrossRef]
- Di Chen, S.; Pan, T.W.; Chang, C.C. The motion of a single and multiple neutrally buoyant elliptical cylinders in plane Poiseuille flow. Phys. Fluids 2012, 24, 103302. [Google Scholar] [CrossRef]
- Qian, S.Z.; Jiang, M.Q.; Liu, Z.H. Inertial migration of aerosol particles in three-dimensional microfluidic channels. Particuology 2021, 55, 23–34. [Google Scholar] [CrossRef]
- Barkla, H.M.; Auchterlonie, L.J. The Magnus or Robins effect on rotating spheres. J. Fluid Mech. 1971, 47, 437–447. [Google Scholar] [CrossRef]
- Mahmoud, G.M.; Ahmed, M.E. Chaotic and Hyperchaotic Complex Jerk Equations. Int. J. Mod. Nonlinear Theory Appl. 2012, 01, 6–13. [Google Scholar] [CrossRef] [Green Version]
- Schaaf, C.; Stark, H. Particle pairs and trains in inertial microfluidics. Eur. Phys. J. E 2020, 43, 50. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, L.; Du, J.; Zhu, Z. Neutrally Buoyant Particle Migration in Poiseuille Flow Driven by Pulsatile Velocity. Micromachines 2021, 12, 1075. https://doi.org/10.3390/mi12091075
Huang L, Du J, Zhu Z. Neutrally Buoyant Particle Migration in Poiseuille Flow Driven by Pulsatile Velocity. Micromachines. 2021; 12(9):1075. https://doi.org/10.3390/mi12091075
Chicago/Turabian StyleHuang, Lizhong, Jiayou Du, and Zefei Zhu. 2021. "Neutrally Buoyant Particle Migration in Poiseuille Flow Driven by Pulsatile Velocity" Micromachines 12, no. 9: 1075. https://doi.org/10.3390/mi12091075





