Utility Function from Maximum Entropy Principle
Abstract
:1 Introduction
2 The Maximum Entropy Method in Economics
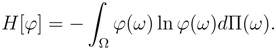
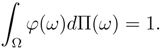




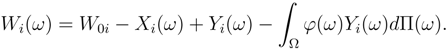
 and receives Yi(ω) upon occurrence of this event.
and receives Yi(ω) upon occurrence of this event.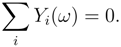

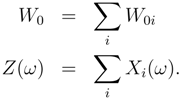
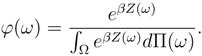
3 Utility Function
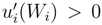 , to guarantee that the agent desires the profit and negative second derivative,
, to guarantee that the agent desires the profit and negative second derivative,  , to restrict it’s avarice (rational agent should be risk averse). The risk aversion parameter,
, to restrict it’s avarice (rational agent should be risk averse). The risk aversion parameter, 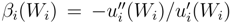 , is also involved in the utility function to scale the agent’s desire in the market with respect to it’s wealth. The market arrives at the equilibrium state upon the agents satisfaction from their trade. In this respect given the equilibrium prices, each agent maximizes utility by choosing his level of Yi(ω) in each contingency.
, is also involved in the utility function to scale the agent’s desire in the market with respect to it’s wealth. The market arrives at the equilibrium state upon the agents satisfaction from their trade. In this respect given the equilibrium prices, each agent maximizes utility by choosing his level of Yi(ω) in each contingency.
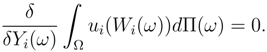

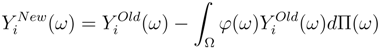
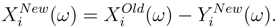
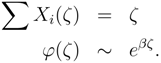
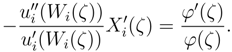
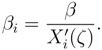
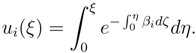
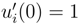 , the amount of wealth may be rescaled regarding with this assumption. The parameter ξ is used for the rescaled wealth. For constant risk aversion parameter the exponential form for utility function is obtained.
, the amount of wealth may be rescaled regarding with this assumption. The parameter ξ is used for the rescaled wealth. For constant risk aversion parameter the exponential form for utility function is obtained.4 Conclusion
References
- Burda, Z.; Jurkiewicz, J.; Nowak, M. A. Is Econophysics a Solid Science? Acta Physica Polonica 2003, B 34, 87. [Google Scholar] [PubMed]
- Acharyya, M.; Acharyya, A. B. Modelling and Computer Simulation of an Insurance Policy: A Search for Maximum Profit. Int. J. of Mod. Phys. C 2003, 14(8), 1041–1046. [Google Scholar] [CrossRef]
- Fouladvand, M. E.; Darooneh, A. H. Int. J. of Mod. Phys. C 2005, 16(3), 377–387.
- Darooneh, A. H. Non Life Insurance Pricing: Statistical Mechanics Viewpoint. Int. J. of Mod. Phys. C 2005, 16(1), 167–176. [Google Scholar] [CrossRef]
- Darooneh, H. Physical Premium Principle: A New Way for Insurance Pricing. Entropy 2005, 7[1], 97–107. [Google Scholar] [CrossRef]
- Darooneh, H. Premium Calculation Based on Physical Principles. Iranian Quarterly J. of Insurance 2005, 19(4), 72–82, In persian. [Google Scholar]
- Darooneh, H. Non Life Insurance Pricing: Multi Agent Model. Eur. Phys. J. B 2004, 42, 119–122. [Google Scholar] [CrossRef]
- Darooneh, H. Non Life Insurance Pricing: Agent- Environment Model, Will be published in Proc. of 10th IASBS Conf. in Condensed Matter, IASBS (Zanjan), 27-31 (2004). In persian.
- Jaynes, E.T. Information Theory and Statistical Mechanics. Phy. Rev. 1957, 106, 620. [Google Scholar] Jaynes, E.T. Where Do We Stand on Maximum Entropy? In Papers on Probability, Statistics and Statistical Physics; Rosenkrantz, R. D., Ed.; Klower Acad. Pub.: Dordrecht, 1989. [Google Scholar]
- Buhlmann, H. An Economic Premium Principle. ASTIN Bulletin 1980, 11, 52. [Google Scholar]
- Buhlmann, H. General Economic Premium Principle. ASTIN Bulletin 1984, 14, 13. [Google Scholar]
- Foley, D. K. A Statistical Equilibrium Theory of Markets. J. Economic Theory 1994, 62(2), 321. [Google Scholar] [CrossRef]
- Foley, D. K. Statistical Equilibrium in a Simple Labor Market. Metroeconomica 1996, 47(2), 125. [Google Scholar] [CrossRef]
- Smith, E.; Foley, D. K. SFI Working papers. 2002. [Google Scholar]
- Sergeev, V. Limit of Rationality. Fasis, Moscow, 1999; V. Sergeev, SFI Working papers. [Google Scholar]
- Milakovic’, M. LEM Working Papers. 2003. [Google Scholar]
- Drăgulescu, A.; Yakovenko, V. M. Statistical Mechanics of Money. Eur. Phys. J. B 2000, 17, 723. [Google Scholar]
- Drăgulescu, A.; Yakovenko, V. M. Evidence for the Exponential Distribution of Incomes in the USA. Eur. Phys. J. B 2001, 20, 585. [Google Scholar]
- Drăgulescu, A.; Yakovenko, V. M. Exponential and Power Law Probability Distribution of Wealth and Incomes in the United Kingdom and United States. Physica A 2001, 299, 213. [Google Scholar]
- Chatterjee, A.; Chakarbarti, B. K.; Manna, S. S. Money in Gas-Like Market: Gibbs and Pareto Laws. Physica Scripta 2003, T90, 36. [Google Scholar] —, Econophysics of the Wealth Distribution; Chatterjee, A.; Yarlagadda, S.; Chakarbarti, B. K. New Economic Windows Series; Springer Verlag: Milano, 2005. [Google Scholar]
- Debreu, G. Theory of Value; Yal Univ. Press: New Haven, 1987. [Google Scholar]
- McCauley, J. L. Thermodynamic analogies in economics and finance. Physica A 2003, 329, 199–212. [Google Scholar] [CrossRef]
- McCauley, J. L.; Kuffner, C. M. Economic System Dynamics. Discrete Dynamics in Nature and Society 2004, 1, 213–220. [Google Scholar]
- Pathria, R. K. Statistical Mechanics; Pergamon Press: Oxford, 1972. [Google Scholar]
- Prentis, J. J.; Andrus, A. E.; Stasevich, T. J. Crossover from the Exact Factor to the Boltzman Factor. Am. J. of Phys. 1999, 67(6), 508–515. [Google Scholar] [CrossRef]
© 2006 by MDPI (http://www.mdpi.org). Reproduction for noncommercial purposes permitted.
Share and Cite
Darooneh, A.H. Utility Function from Maximum Entropy Principle. Entropy 2006, 8, 18-24. https://doi.org/10.3390/e8010018
Darooneh AH. Utility Function from Maximum Entropy Principle. Entropy. 2006; 8(1):18-24. https://doi.org/10.3390/e8010018
Chicago/Turabian StyleDarooneh, Amir H. 2006. "Utility Function from Maximum Entropy Principle" Entropy 8, no. 1: 18-24. https://doi.org/10.3390/e8010018
APA StyleDarooneh, A. H. (2006). Utility Function from Maximum Entropy Principle. Entropy, 8(1), 18-24. https://doi.org/10.3390/e8010018



