Numerical Analyses of Entropy Production and Thermodynamics Exergy on a Hydrogen-Fueled Micro Combustor Featuring a Diamond-Shaped Bifurcated Inner-Tube Structure for Thermophotovoltaic Applications
Abstract
1. Introduction
2. Numerical Methodology
2.1. Geometric Model
2.2. Governing Equations
2.3. Numerical Setup and Boundary Conditions
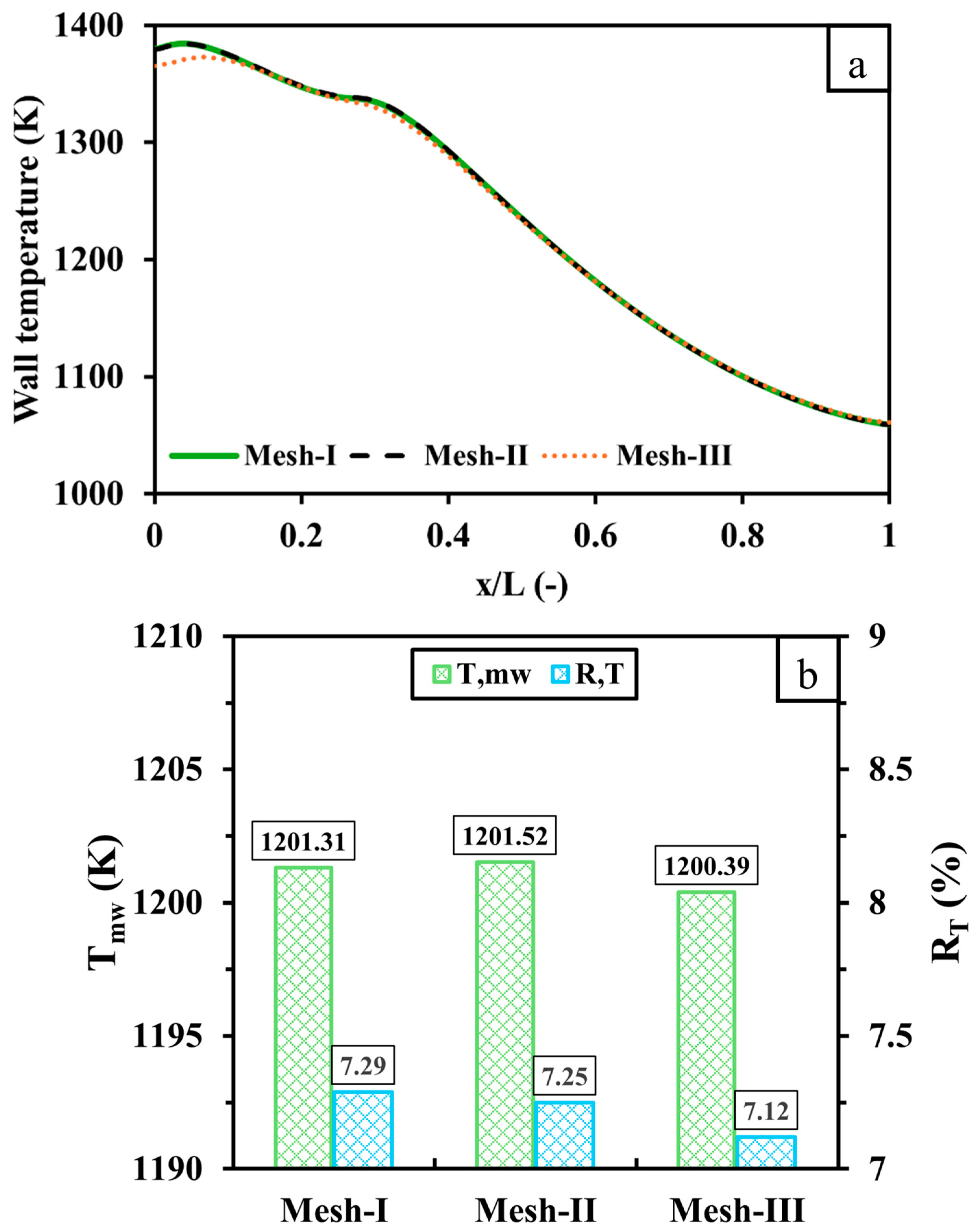
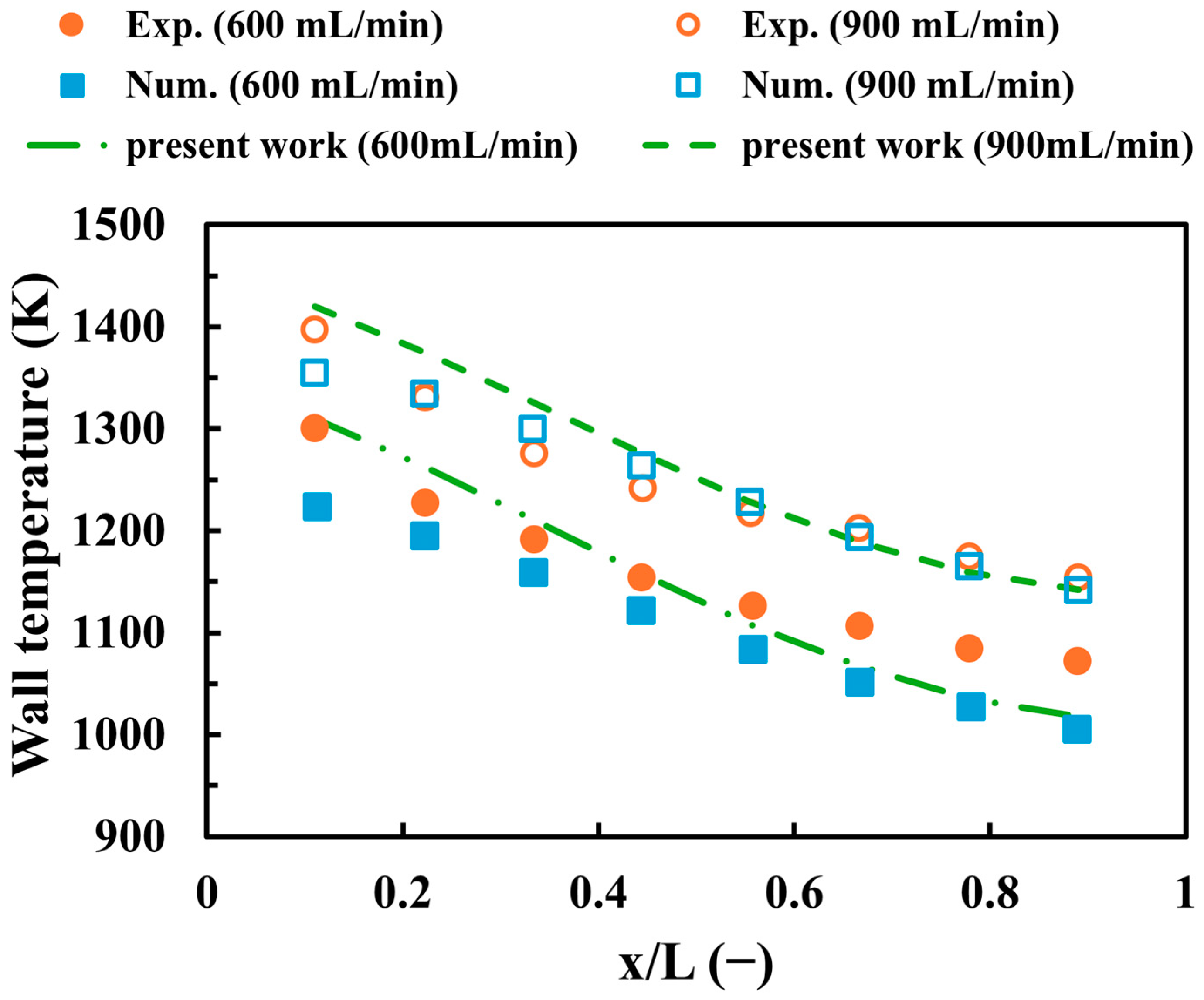
2.4. Grid Independence and Model Validation
3. Results
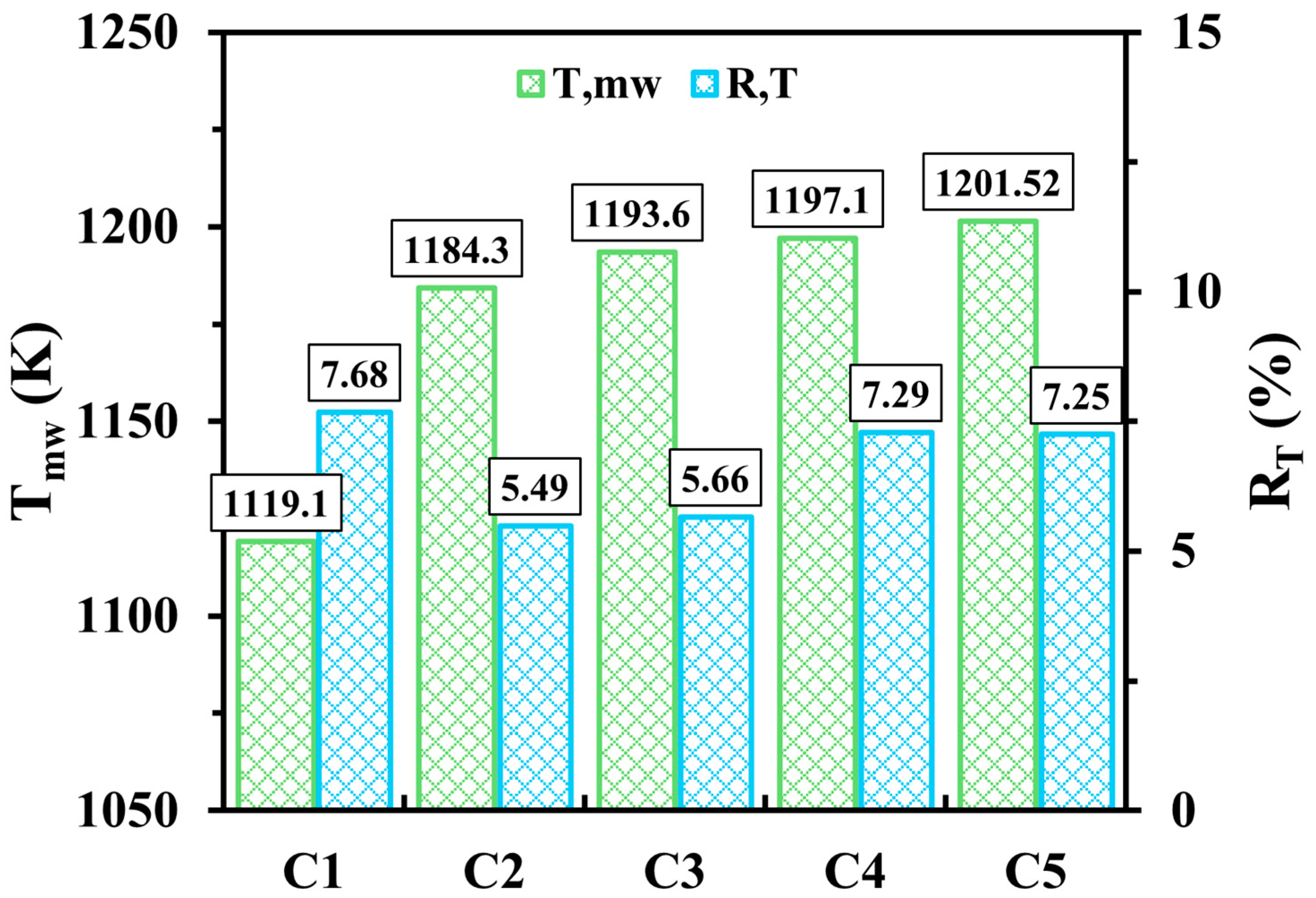
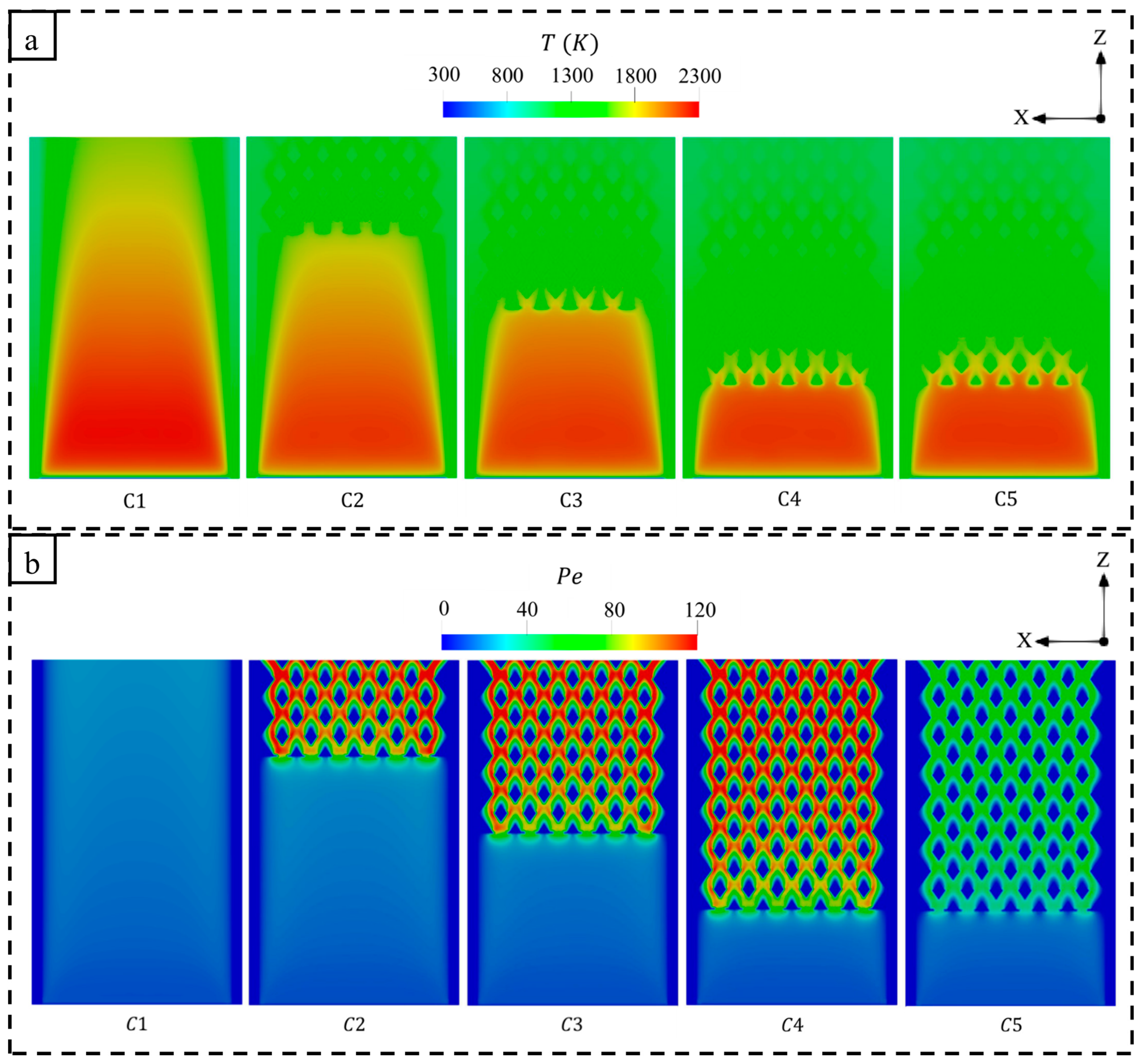
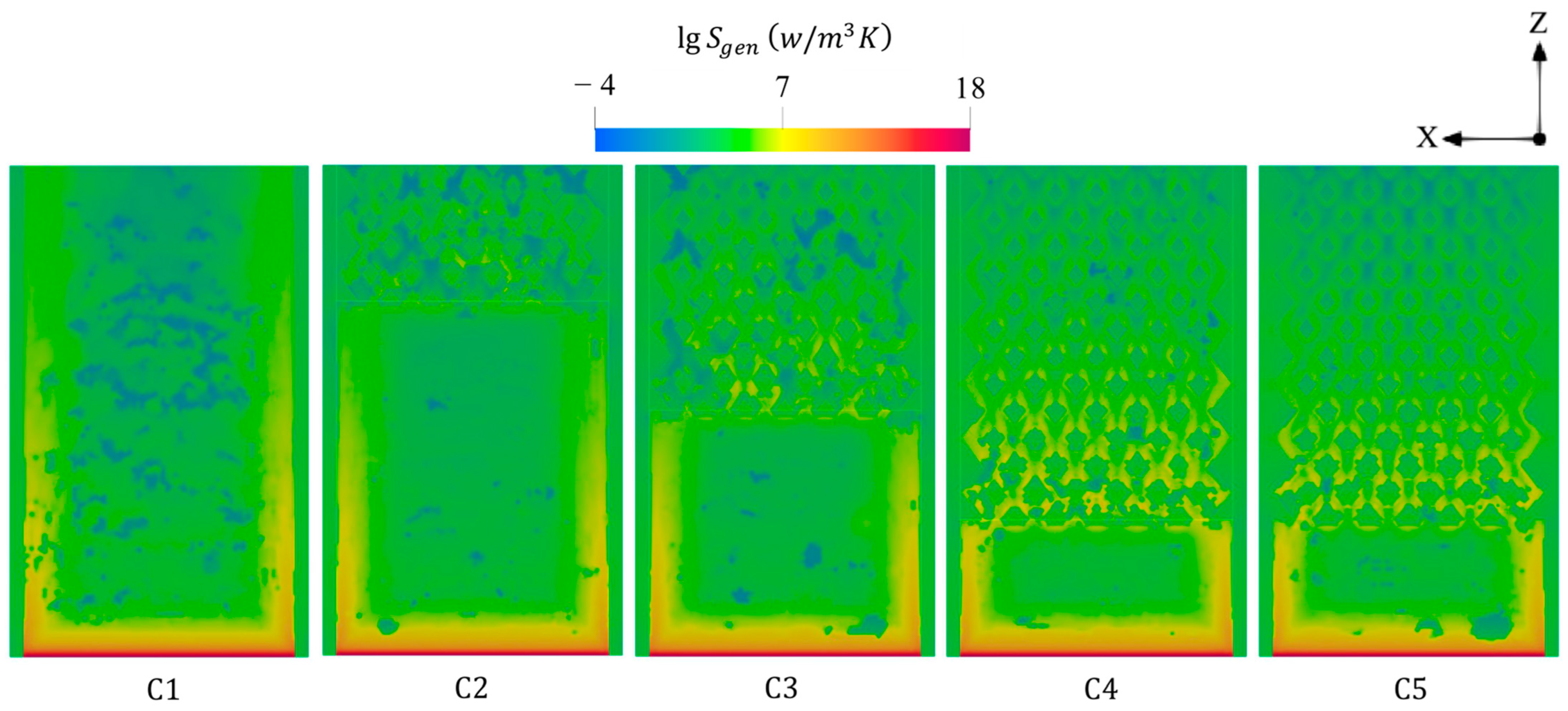
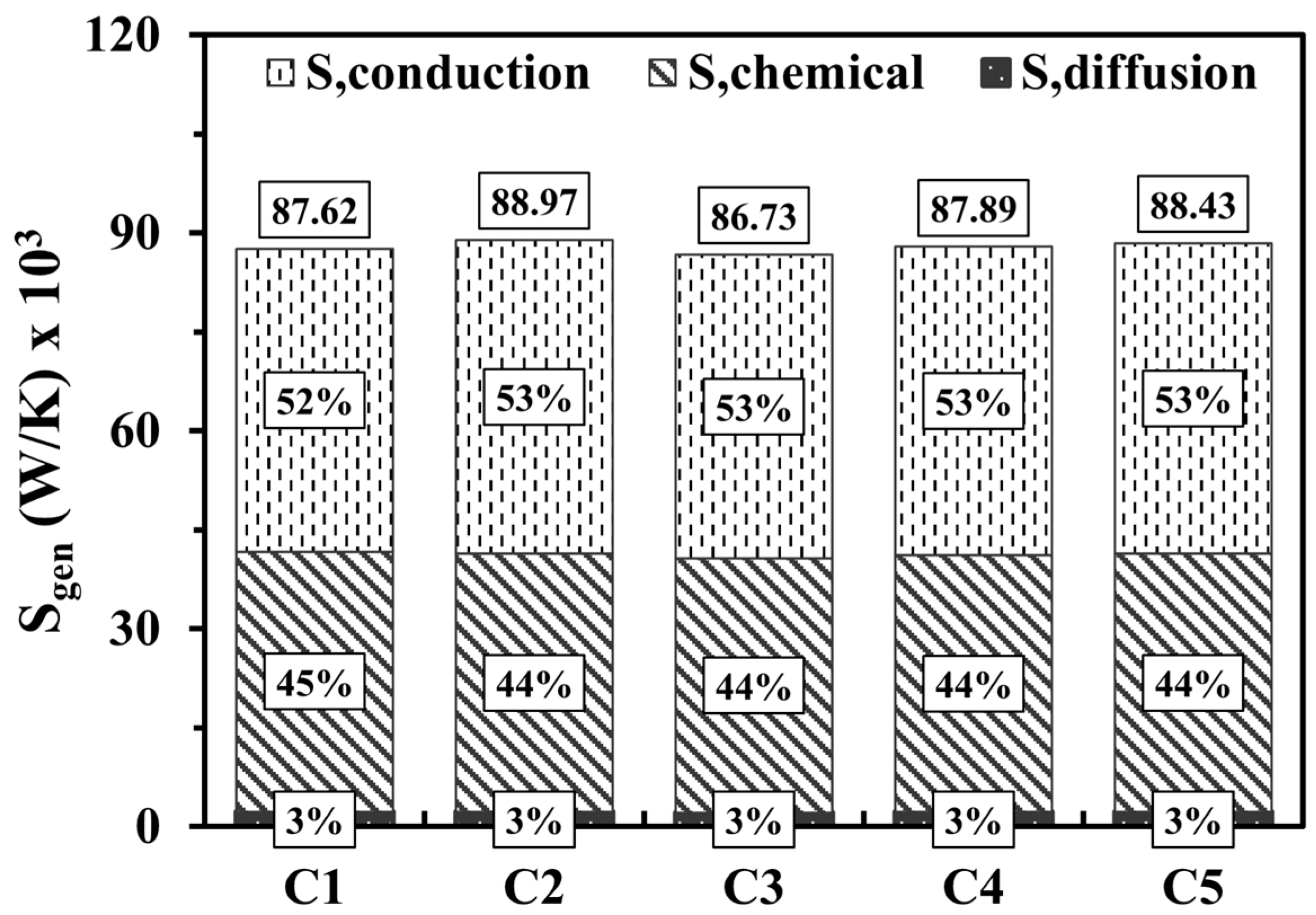
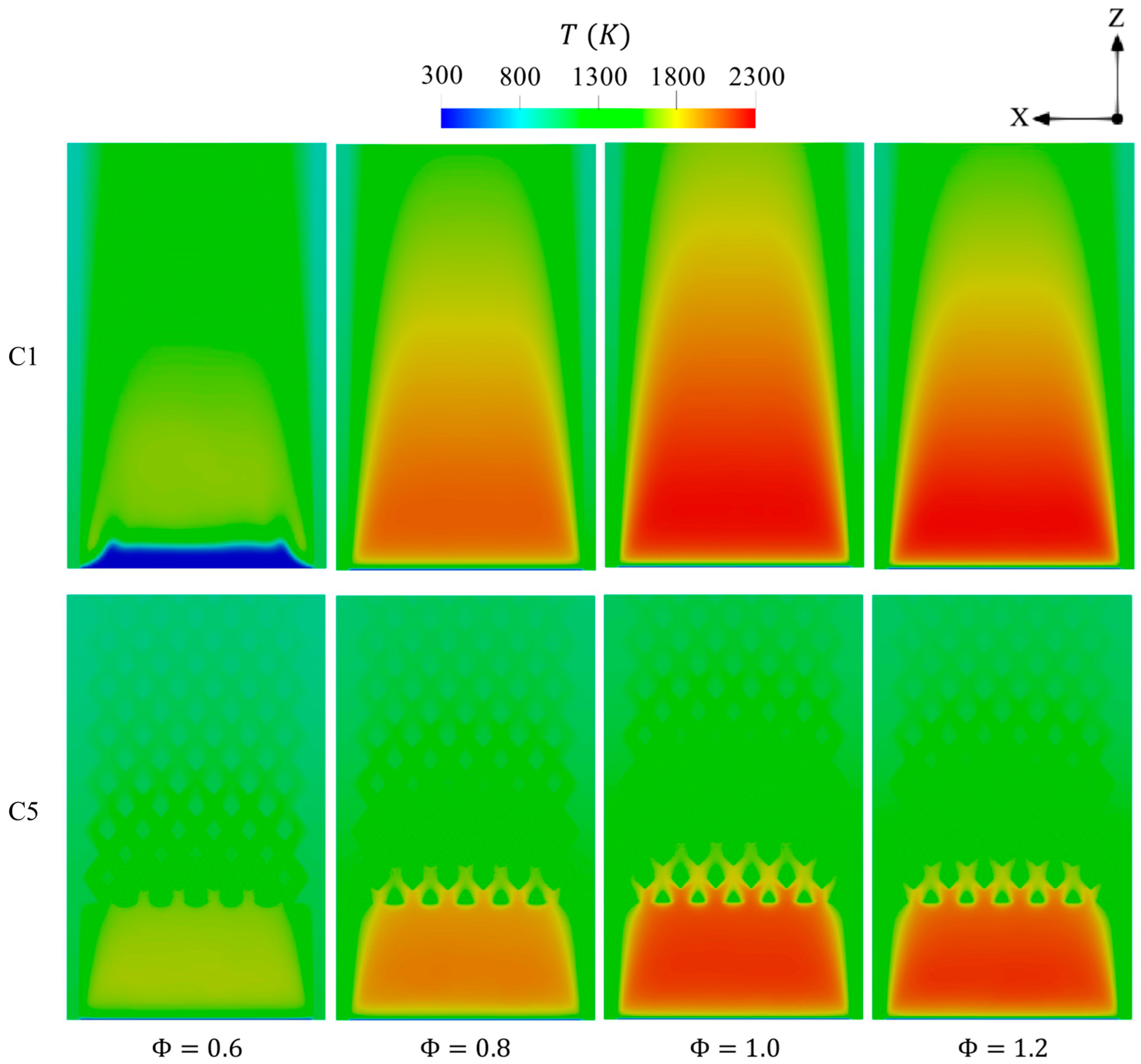
3.1. Effects of the Diamond-Shaped Bifurcated Inner-Tube Structure
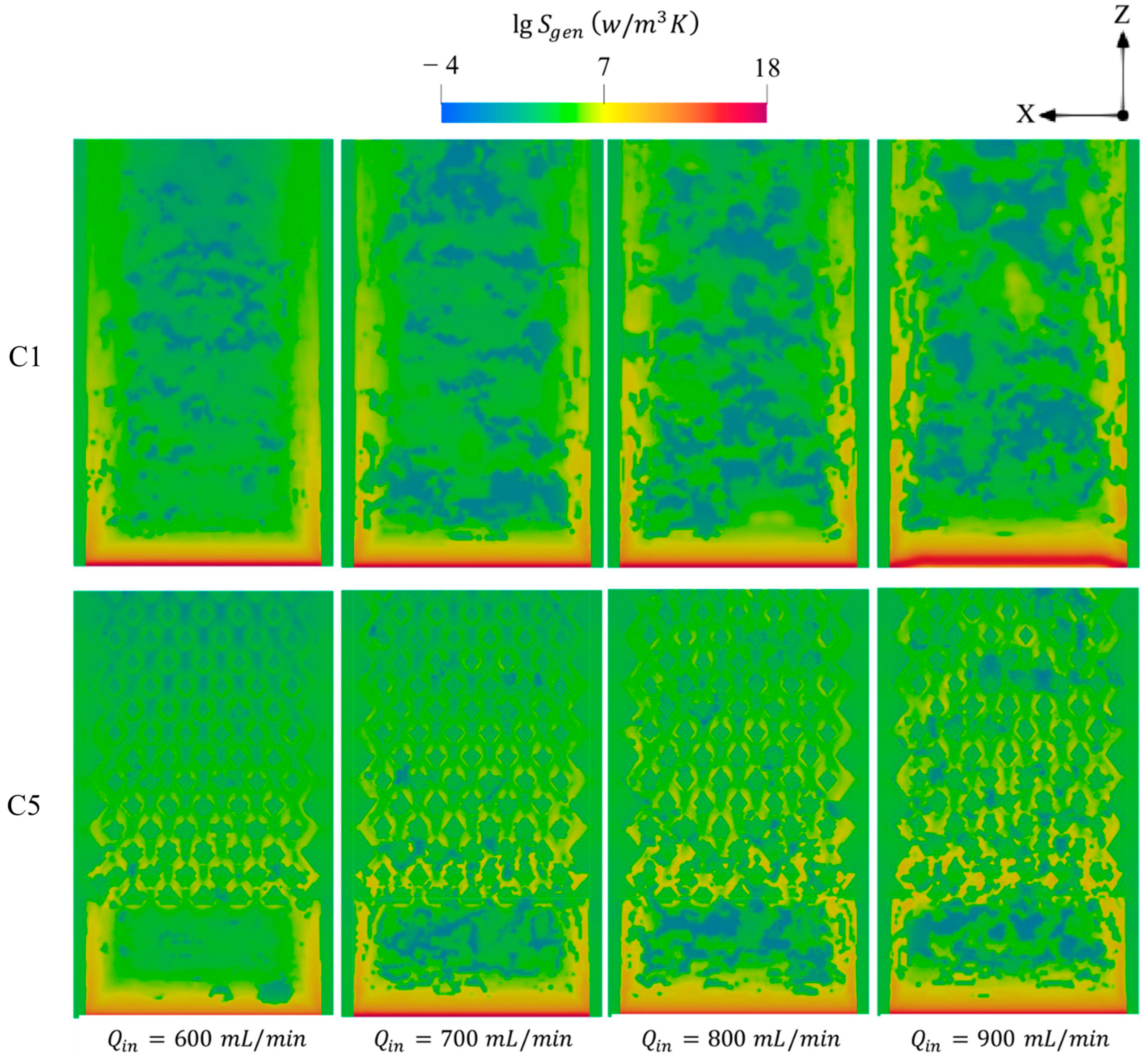
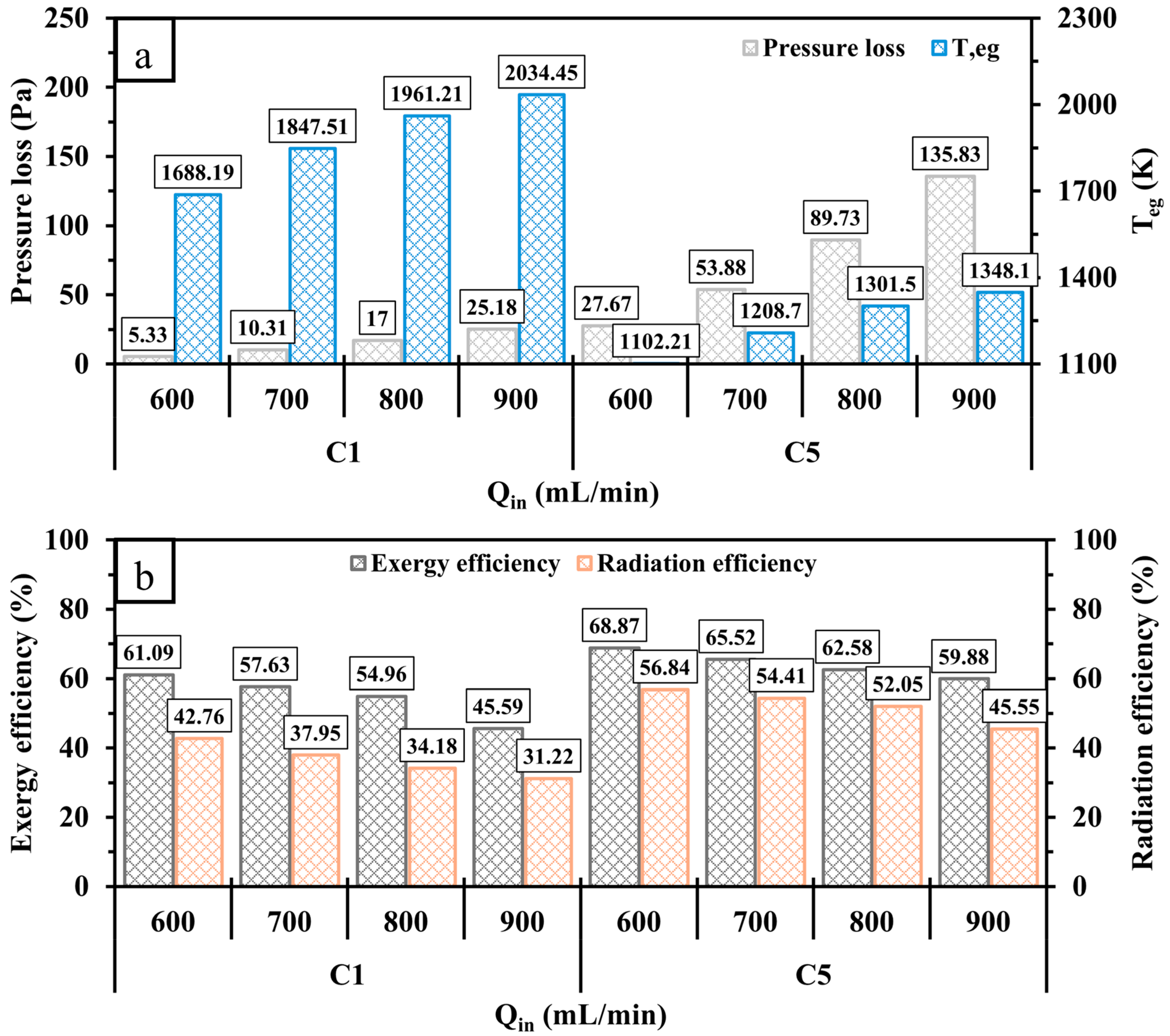
3.2. Effects of Inlet Velocity
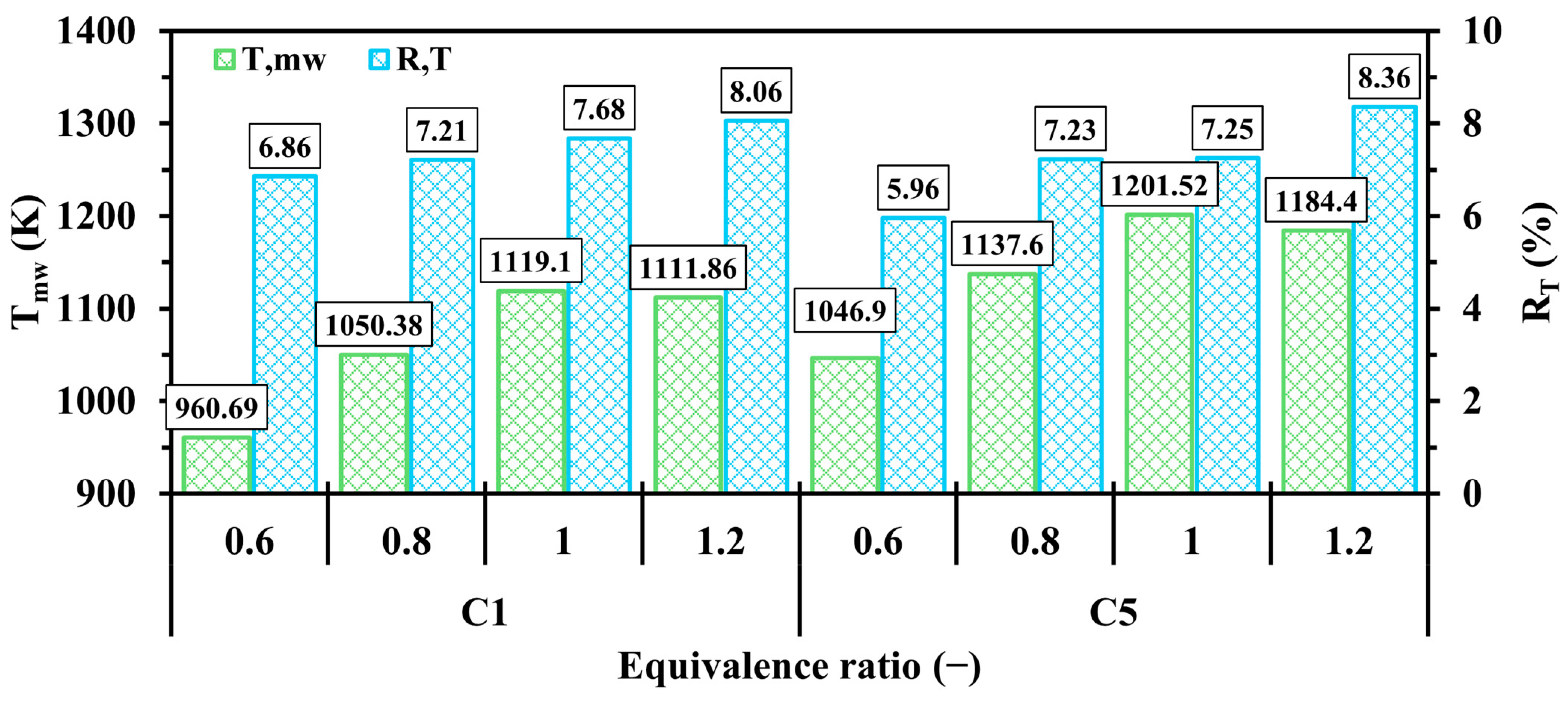
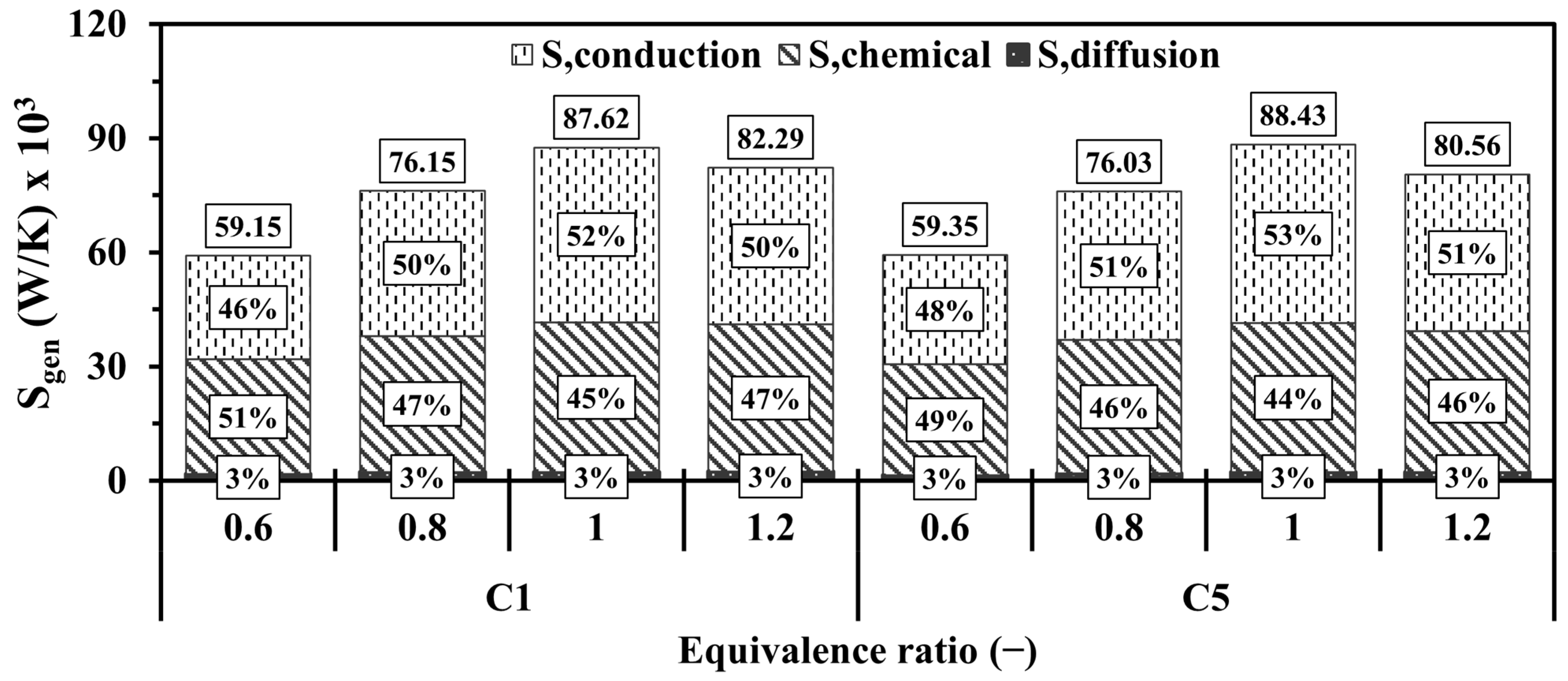
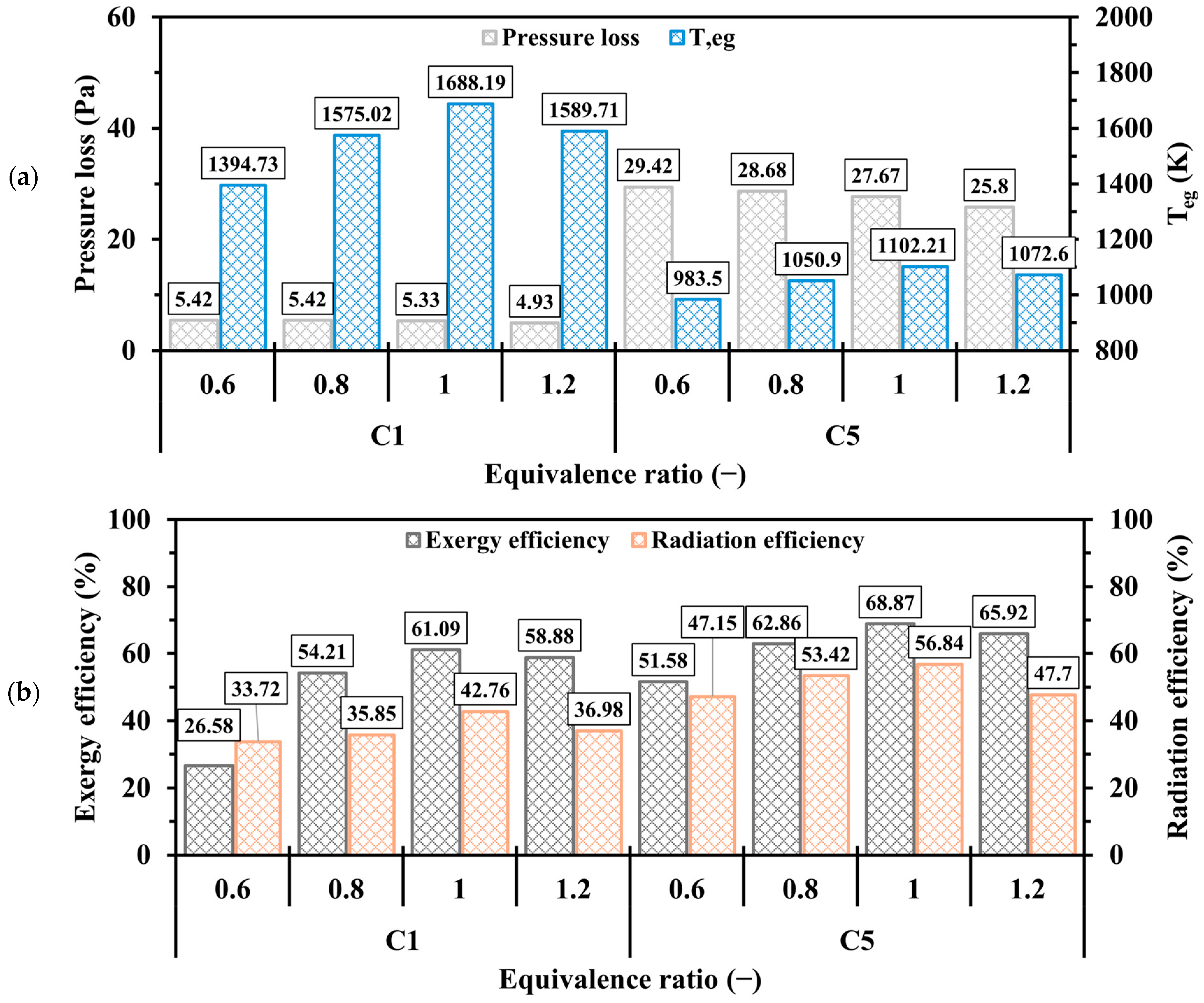
3.3. Effects of Inlet Equivalence Ratio
4. Conclusions
- The proposed configuration notably enhances mean wall temperature and its uniformity across the external surface compared to the conventional design. Furthermore, the variation in Pe numbers across the geometry implies that the new design remarkably enhances advection effects and, consequently, the heat transfer process. For this, the mean exhaust gas temperature is lowered, while both exergy and radiation efficiencies show notable improvements.
- Extending the length of the diamond-shaped inner-tube configuration enhances the mean temperature over the solid walls, although it reduces the uniformity of temperature across the walls. Moreover, the region with high Pe variations is concentrated in the diamond-shaped section; hence, its elongation shifts the thermal dynamics toward advection dominance over diffusion. This reduces pressure loss and mean exhaust gas temperature and, however, advances exergy and radiation efficiencies. Widening the flame channels in this extended structure further improves thermal performance.
- Escalating the hydrogen volume flow rate results in a greater mean wall temperature and more temperature uniformity across the walls. In addition, it amplifies the Pe number, pressure loss, and mean exhaust gas temperature because a higher energy input is supplied to the system. Nevertheless, the micro combustor’s limited capacity to effectively utilize large energy volumes causes exergy and radiation efficiencies to decrease as the flow rate increases. The entropy generation is highly increased when the inlet velocity is increased, owing essentially to the considerable enhancement of the chemical entropy mechanism.
- In contrast to the stoichiometric condition, variations in the equivalence ratio reveal that both lean and rich fuel mixtures lead to lower system performance due to insufficient hydrogen or oxygen in the premixed charge, respectively. This indicates that an equivalence ratio of unity optimizes system efficiency and output power density. However, the unity equivalence ratio case achieves the peak entropy production due to the large difference in temperature gradients (conduction entropy mechanism) between the heat and walls.
- The high auto-ignition temperature of hydrogen could be one of the practical limitations of using hydrogen in such applications. Thus, examining the effects of using catalysts to lower the ignition temperature is critical.
- The high wall temperature could damage the wall material. Thus, an investigation into employing thermal carrier coatings on the wall material is needed to ensure the sustainability of the micro combustor.
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Peclet number, | |
| Vector velocity, | |
| Heat losses by convection, | |
| Heat losses by radiation, | |
| Total total energy of the fluid, | |
| Natural convection heat transfer coefficient, | |
| Reaction net rate of production of species i | |
| Effective thermal conductivity, | |
| Specific nthalpy of species j, | |
| Source term of enthalpy, | |
| Outer wall area of cell i, | |
| Surface area of the outer wall, | |
| Diffusion flux of species , | |
| Local mass fraction of species i, | |
| Total entropy generation, | |
| Entropy generation as a result of chemical reaction, | |
| Entropy generation as a result of heat conduction, | |
| Entropy generation as a result of mass diffusion, | |
| Mixture thermal conductivity, | |
| Reference enthalpy of species i, | |
| Reference entropy of species i, | |
| Inlet exergy, | |
| Total exergy losses, | |
| Uncounted exergy destruction, | |
| Energy loss from the combustion exhaust gas, | |
| Pressure, | |
| Atmospheric pressure, | |
| Exhaust gas temperature, | |
| Temperature of external wall, | |
| Area-weighted-mean wall temperature, | |
| Outer wall temperature of cell i, | |
| Ambient temperature, | |
| Wall temperature uniformity, | |
| Mass diffusivity of species i, | |
| Mass flow rate of inlet flow, | |
| Mass flow rate of fuel, | |
| Velocity, | |
| Specific heat capacity, | |
| Gas constant, | |
| Lower heating value, | |
| Greek letters | |
| Mixture gas density, | |
| Mole fraction of species, i | |
| Viscous stress, | |
| Reynolds stress, | |
| Chemical potential of species i, | |
| Mass fraction of species, i | |
| Stephan–Boltzmann constant, | |
| Equivalence ratio, | |
| Thermal conductivity, | |
| Emissivity of the solid surface. | |
| Production rate of species i, | |
| Exergy efficiency, | |
| Radiation efficiency, |
References
- Nicoletti, G.; Arcuri, N.; Nicoletti, G.; Bruno, R. A Technical and Environmental Comparison between Hydrogen and Some Fossil Fuels. Energy Convers. Manag. 2015, 89, 205–213. [Google Scholar] [CrossRef]
- Fu, J.; Liu, J.; Feng, R.; Yang, Y.; Wang, L.; Wang, Y. Energy and Exergy Analysis on Gasoline Engine Based on Mapping Characteristics Experiment. Appl. Energy 2013, 102, 622–630. [Google Scholar] [CrossRef]
- Shindell, D.; Smith, C.J. Climate and Air-Quality Benefits of a Realistic Phase-out of Fossil Fuels. Nature 2019, 573, 408–411. [Google Scholar] [CrossRef]
- Xue, Z.; Yan, Y.; He, Z.; Shen, K.; Zhang, C. Comprehensive Numerical Insight the Thermal Performance Improvement of the Micro Combustors with Internal Bionic Y-Shaped Fins for Micro-Thermal Voltaic System Applications. Fuel 2022, 318, 123610. [Google Scholar] [CrossRef]
- Berwal, P.; Kumar, S.; Khandelwal, B. A Comprehensive Review on Synthesis, Chemical Kinetics, and Practical Application of Ammonia as Future Fuel for Combustion. J. Energy Inst. 2021, 99, 273–298. [Google Scholar] [CrossRef]
- Meher Kotay, S.; Das, D. Biohydrogen as a Renewable Energy Resource—Prospects and Potentials. Int. J. Hydrogen Energy 2008, 33, 258–263. [Google Scholar] [CrossRef]
- Hoang, A.T.; Foley, A.M.; Nižetić, S.; Huang, Z.; Ong, H.C.; Ölçer, A.I.; Pham, V.V.; Nguyen, X.P. Energy-Related Approach for Reduction of CO2 Emissions: A Critical Strategy on the Port-to-Ship Pathway. J. Clean. Prod. 2022, 355, 131772. [Google Scholar] [CrossRef]
- Saravanan, N.; Nagarajan, G.; Sanjay, G.; Dhanasekaran, C.; Kalaiselvan, K.M. Combustion Analysis on a DI Diesel Engine with Hydrogen in Dual Fuel Mode. Fuel 2008, 87, 3591–3599. [Google Scholar] [CrossRef]
- Köse, H.; Ciniviz, M. An Experimental Investigation of Effect on Diesel Engine Performance and Exhaust Emissions of Addition at Dual Fuel Mode of Hydrogen. Fuel Process. Technol. 2013, 114, 26–34. [Google Scholar] [CrossRef]
- Rong, H.; Zhao, D.; Oo, N.L.; Cai, T.; Zhao, H.; Sun, D.; Xu, J. Thermal Performances and NOx Emission Studies on an Ammonia-Hydrogen Fueled Double-Channel Outlet Micro-Combustor for Thermophotovoltaic Applications. Energy Built Environ. 2024, in press. [Google Scholar] [CrossRef]
- Zhao, H.; Zhao, D.; Becker, S. Thermal Performances Investigation on an Ammonia-Fuelled Heat-Recirculating Micro-Combustor with Reduced Chemical Mechanism. Appl. Therm. Eng. 2024, 236, 121685. [Google Scholar] [CrossRef]
- Chou, S.K.; Yang, W.M.; Chua, K.J.; Li, J.; Zhang, K.L. Development of Micro Power Generators—A Review. Appl. Energy 2011, 88, 1–16. [Google Scholar] [CrossRef]
- Yilmaz, H.; Cam, O.; Tangoz, S.; Yilmaz, I. Effect of Different Turbulence Models on Combustion and Emission Characteristics of Hydrogen/Air Flames. Int. J. Hydrogen Energy 2017, 42, 25744–25755. [Google Scholar] [CrossRef]
- Kim, N.I.; Kato, S.; Kataoka, T.; Yokomori, T.; Maruyama, S.; Fujimori, T.; Maruta, K. Flame Stabilization and Emission of Small Swiss-Roll Combustors as Heaters. Combust. Flame 2005, 141, 229–240. [Google Scholar] [CrossRef]
- Kuo, C.H.; Ronney, P.D. Numerical Modeling of Non-Adiabatic Heat-Recirculating Combustors. Proc. Combust. Inst. 2007, 31, 3277–3284. [Google Scholar] [CrossRef]
- Nauman, M.; Pan, J.; Wang, Y.; Li, F.; Oluwaleke Ojo, A.; Raza, A. A Review of Recent Advancements in Micro Combustion Techniques to Enhance Flame Stability and Fuel Residence Time. Int. J. Hydrogen Energy 2024, 49, 1165–1193. [Google Scholar] [CrossRef]
- Ni, S.; Zhao, D.; Zhu, X. Heat Transfer and Entropy Production Evaluation on Premixed Hydrogen/Air-Fuelled Micro-Combustors with Internal Threads. Fuel 2021, 303, 121325. [Google Scholar] [CrossRef]
- Zuo, W.; Li, D.; E, J.; Xia, Y.; Li, Q.; Quan, Y.; Zhang, G. Parametric Study of Cavity on the Performance of a Hydrogen-Fueled Micro Planar Combustor for Thermophotovoltaic Applications. Energy 2023, 263, 126028. [Google Scholar] [CrossRef]
- Zuo, W.; Chen, Z.; E, J.; Li, Q.; Zhang, G.; Huang, Y. Effects of Structure Parameters of Tube Outlet on the Performance of a Hydrogen-Fueled Micro Planar Combustor for Thermophotovoltaic Applications. Energy 2023, 266, 126434. [Google Scholar] [CrossRef]
- Chen, X.; Li, J.; Zhao, D.; Rashid, M.T.; Zhou, X.; Wang, N. Effects of Porous Media on Partially Premixed Combustion and Heat Transfer in Meso-Scale Burners Fuelled with Ethanol. Energy 2021, 224, 120191. [Google Scholar] [CrossRef]
- Xie, B.; Peng, Q.; Shi, Z.; Wei, J.; Kang, Z.; Wei, D.; Tian, X.; Fu, G. Investigation of CH4 and Porous Media Addition on Thermal and Working Performance in Premixed H2/Air Combustion for Micro Thermophotovoltaic. Fuel 2023, 339, 127444. [Google Scholar] [CrossRef]
- Li, Q.; Wang, J.; Li, J.; Shi, J. Fundamental Numerical Analysis of a Porous Micro-Combustor Filled with Alumina Spheres: Pore-Scale vs. Volume-Averaged Models. Appl. Sci. 2021, 11, 7496. [Google Scholar] [CrossRef]
- Peng, Q.; Yang, W.; E, J.; Li, Z.; Xu, H.; Fu, G.; Li, S. Investigation on H2/Air Combustion with C3H8 Addition in the Combustor with Part/Full Porous Medium. Energy Convers. Manag. 2021, 228, 113652. [Google Scholar] [CrossRef]
- Tang, A.; Deng, J.; Xu, Y.; Pan, J.; Cai, T. Experimental and Numerical Study of Premixed Propane/Air Combustion in the Micro-Planar Combustor with a Cross-Plate Insert. Appl. Therm. Eng. 2018, 136, 177–184. [Google Scholar] [CrossRef]
- Ma, L.; Xu, H.; Wang, X.; Fang, Q.; Zhang, C.; Chen, G. A Novel Flame-Anchorage Micro-Combustor: Effects of Flame Holder Shape and Height on Premixed CH4/Air Flame Blow-off Limit. Appl. Therm. Eng. 2019, 158, 113836. [Google Scholar] [CrossRef]
- Cai, T.; Tang, A.; Zhao, D.; Zhou, C.; Huang, Q. Experimental Observation and Numerical Study on Flame Structures, Blowout Limit and Radiant Efficiency of Premixed Methane/Air in Micro-Scale Planar Combustors. Appl. Therm. Eng. 2019, 158, 113810. [Google Scholar] [CrossRef]
- Rong, H.; Zhao, H.; Cai, T. Thermodynamic and Emission Analysis of an Ammonia Fueled Micro-Combustor with Double-Channel Reverse Flow Structure. Int. J. Hydrogen Energy 2024, 49, 1303–1314. [Google Scholar] [CrossRef]
- Cai, T.; Sun, Y.; Zhao, D. Enhancing Heat Transfer Performance Analyses of a Hydrogen-Fueled Meso-Combustor with Staggered Bluff-Bodies. Fuel Process. Technol. 2021, 218, 106867. [Google Scholar] [CrossRef]
- Zhao, H.; Zhao, D.; Becker, S.; Rong, H.; Zhao, X. Entropy Generation and Improved Thermal Performance Investigation on a Hydrogen-Fuelled Double-Channel Microcombustor with Y-Shaped Internal Fins. Energy 2023, 283, 128476. [Google Scholar] [CrossRef]
- Lachraf, A.; Si Ameur, M. Numerical Investigation of Thermal Performance of Hydrogen Fueled Micro-Combustor with Trapezoidal Ribs. Int. J. Hydrogen Energy 2023, 48, 39570–39585. [Google Scholar] [CrossRef]
- Rong, H.; Zhao, D. Thermodynamic and Entropy Generation Analyses of Telsa-Valve Structured Meso-Scale Combustors Fuelled with Hydrogen for Thermophotovoltaic Applications. Energy 2024, 307, 132788. [Google Scholar] [CrossRef]
- Zhao, T.; Yan, Y.; He, Z.; Yang, Z. Influence of Multi-Structure Optimization on the Comprehensive Performance of Micro-Cylindrical Combustor Inserting with Spiral Fin by Using Grey Relational Analysis and Analysis of Variance. Int. J. Hydrogen Energy 2021, 46, 28327–28337. [Google Scholar] [CrossRef]
- Zuo, W.; Zhang, Y.; Li, Q.; Li, J.; He, Z. Numerical Investigations on Hydrogen-Fueled Micro-Cylindrical Combustors with Cavity for Micro-Thermophotovoltaic Applications. Energy 2021, 223, 120098. [Google Scholar] [CrossRef]
- Brambati, G.; Guilizzoni, M.; Foletti, S. Convective Heat Transfer Correlations for Triply Periodic Minimal Surfaces Based Heat Exchangers. Appl. Therm. Eng. 2024, 242, 122492. [Google Scholar] [CrossRef]
- Huang, Z.; Huang, R.; Ling, J.; Aute, V.C.; Hwang, Y. Application of Bifurcated Bare-Tube Heat Exchanger on Water-Based Hybrid VRF System. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 9–12 July 2018. [Google Scholar]
- Yu, M.; Lai, X.; Xiao, H.; Liu, Z.; Liu, W. A Study on Flow and Heat Transfer Characteristics of a Constructal Bifurcation Filler in the Circular Tube. Appl. Therm. Eng. 2021, 183, 116205. [Google Scholar] [CrossRef]
- Safari, V.; Abolghasemi, H.; Kamkari, B. Experimental and Numerical Investigations of Thermal Performance Enhancement in a Latent Heat Storage Heat Exchanger Using Bifurcated and Straight Fins. Renew. Energy 2021, 174, 102–121. [Google Scholar] [CrossRef]
- Luo, X.; Gu, J.; Ma, H.; Xie, Y.; Li, A.; Wang, J.; Ding, R. Numerical Study on Enhanced Melting Heat Transfer of PCM by the Combined Fractal Fins. J. Energy Storage 2022, 45, 103780. [Google Scholar] [CrossRef]
- Sciacovelli, A.; Gagliardi, F.; Verda, V. Maximization of Performance of a PCM Latent Heat Storage System with Innovative Fins. Appl. Energy 2015, 137, 707–715. [Google Scholar] [CrossRef]
- ANSYS. ANSYS Fluent User’s Guide; Release 23.1; ANSYS, Inc.: Canonsburg, PA, USA, 2023. [Google Scholar]
- Ni, S.; Zhao, D.; Sellier, M.; Li, J.; Chen, X.; Li, X.; Cao, F.; Li, W. Thermal Performances and Emitter Efficiency Improvement Studies on Premixed Micro-Combustors with Different Geometric Shapes for Thermophotovoltaics Applications. Energy 2021, 226, 120298. [Google Scholar] [CrossRef]
- Yang, X.; Yu, B.; Peng, X.; Zhou, H. Investigation of Thermal Performance and Energy Conversion in a Novel Planar Micro-Combustor with Four-Corner Entrances for Thermo-Photovoltaic Power Generators. J. Power Sources 2021, 515, 230625. [Google Scholar] [CrossRef]
- Sun, Y.; Cai, T.; Zhao, D. Thermal Performance and NOx Emission Characteristics Studies on a Premixed Methane-Ammonia-Fueled Micro-Planar Combustor. Fuel 2021, 291, 120190. [Google Scholar] [CrossRef]
- Yang, W.M.; Chua, K.J.; Pan, J.F.; Jiang, D.Y.; An, H. Development of Micro-Thermophotovoltaic Power Generator with Heat Recuperation. Energy Convers. Manag. 2014, 78, 81–87. [Google Scholar] [CrossRef]
- Cai, T.; Zhao, D. Effects of Fuel Composition and Wall Thermal Conductivity on Thermal and NOx Emission Performances of an Ammonia/Hydrogen-Oxygen Micro-Power System. Fuel Process. Technol. 2020, 209, 106527. [Google Scholar] [CrossRef]
- Li, J.; Huang, J.; Chen, X.; Zhao, D.; Shi, B.; Wei, Z.; Wang, N. Effects of Heat Recirculation on Combustion Characteristics of N-Heptane in Micro Combustors. Appl. Therm. Eng. 2016, 109, 697–708. [Google Scholar] [CrossRef]
- Li, J.; Chou, S.K.; Li, Z.W.; Yang, W.M. Characterization of Wall Temperature and Radiation Power through Cylindrical Dump Micro-Combustors. Combust. Flame 2009, 156, 1587–1593. [Google Scholar] [CrossRef]
- Peng, Q.; E, J.; Chen, J.; Zuo, W.; Zhao, X.; Zhang, Z. Investigation on the Effects of Wall Thickness and Porous Media on the Thermal Performance of a Non-Premixed Hydrogen Fueled Cylindrical Micro Combustor. Energy Convers. Manag. 2018, 155, 276–286. [Google Scholar] [CrossRef]
- He, Z.; Yan, Y.; Zhao, T.; Feng, S.; Li, X.; Zhang, L.; Zhang, Z. Heat Transfer Enhancement and Exergy Efficiency Improvement of a Micro Combustor with Internal Spiral Fins for Thermophotovoltaic Systems. Appl. Therm. Eng. 2021, 189, 116723. [Google Scholar] [CrossRef]
- Sun, Y.; Zhao, D.; Ni, S.; David, T.; Zhang, Y. Entropy and Flame Transfer Function Analysis of a Hydrogen-Fueled Diffusion Flame in a Longitudinal Combustor. Energy 2020, 194, 116870. [Google Scholar] [CrossRef]
- Sahoo, B.B.; Saha, U.K.; Sahoo, N. Diagnosing the Effects of Pilot Fuel Quality on Exergy Terms in a Biogas Run Dual Fuel Diesel Engine. Int. J. Exergy 2012, 10, 77–93. [Google Scholar] [CrossRef]
- Stepanov, V.S. Chemical Energies and Exergies of Fuels. Energy 1995, 20, 235–242. [Google Scholar] [CrossRef]
- Tong, J.; Cai, T. Enhancing Thermal Performance, Exergy and Thermodynamics Efficiency of Premixed Methane/Air Micro-Planar Combustor in Micro-Thermophotovoltaic Systems. Energies 2023, 16, 118. [Google Scholar] [CrossRef]
- Tang, A.; Pan, J.; Yang, W.; Xu, Y.; Hou, Z. Numerical Study of Premixed Hydrogen/Air Combustion in a Micro Planar Combustor with Parallel Separating Plates. Int. J. Hydrogen Energy 2015, 40, 2396–2403. [Google Scholar] [CrossRef]
- Rong, H.; Zhao, D.; Cai, T.; Becker, S. Enhancing Thermal and Exergy Performances in a CO2-Free Micro-Combustor with Reverse Flow Double-Channel Outlet Structure. Appl. Therm. Eng. 2023, 233, 121180. [Google Scholar] [CrossRef]
- Fan, Y.; Schlick, C.P.; Umbanhowar, P.B.; Ottino, J.M.; Lueptow, R.M. Modelling Size Segregation of Granular Materials: The Roles of Segregation, Advection and Diffusion. J. Fluid Mech. 2014, 741, 252–279. [Google Scholar] [CrossRef]
- Figueroa, A.; Meunier, P.; Cuevas, S.; Villermaux, E.; Ramos, E. Chaotic Advection at Large Péclet Number: Electromagnetically Driven Experiments, Numerical Simulations, and Theoretical Predictions. Phys. Fluids 2014, 26, 013601. [Google Scholar] [CrossRef]
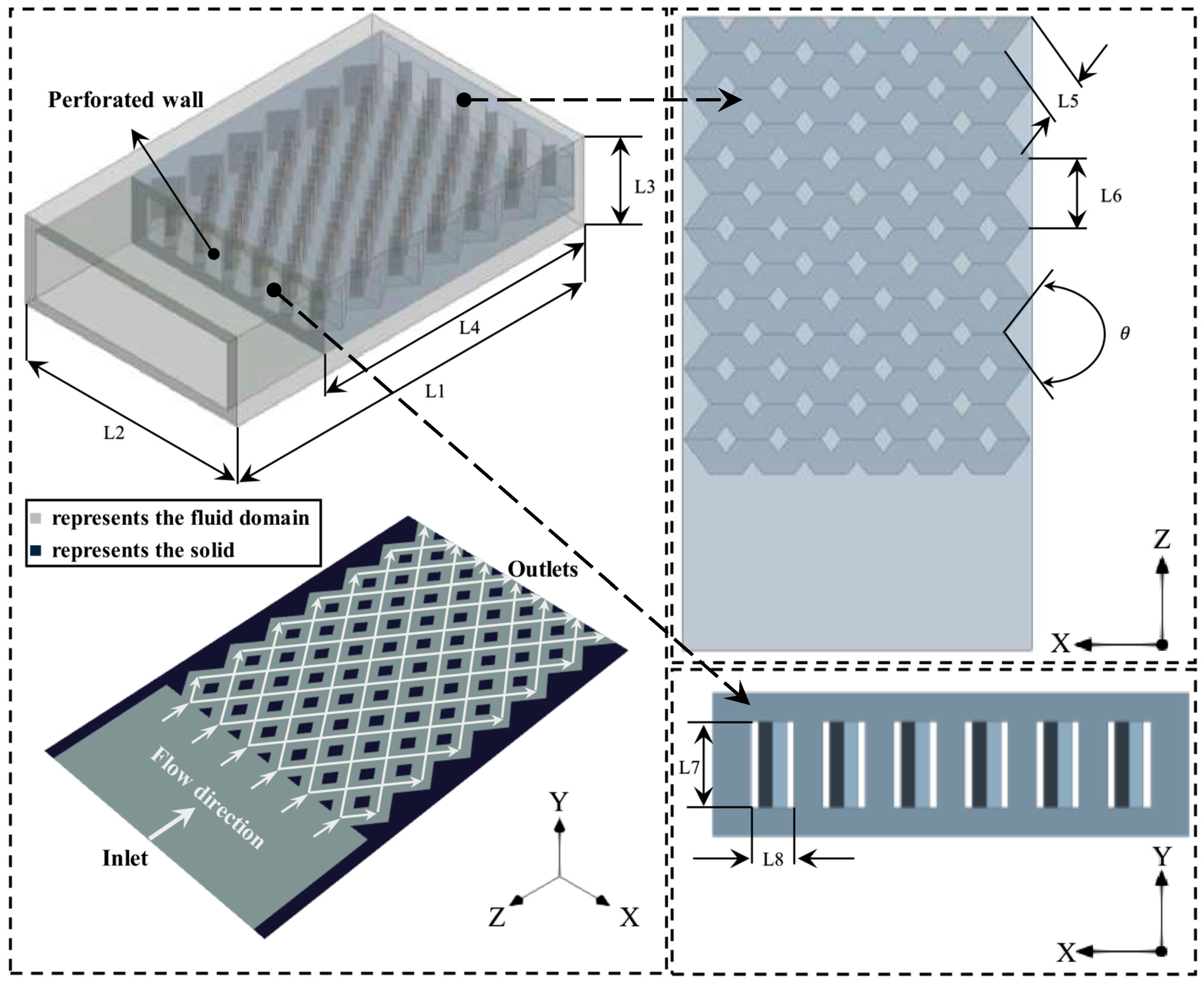







| Variables | Value (mm) | |||||
|---|---|---|---|---|---|---|
| C 1 | C 2 | C 3 | C 4 | C 5 | ||
| Length (mm) | L1 | 18 | 18 | 18 | 18 | 18 |
| L2 | 11 | 11 | 11 | 11 | 11 | |
| L3 | 4 | 4 | 4 | 4 | 4 | |
| L4 | NA | 5 | 9 | 13 | 13 | |
| L5 | NA | 1.25 | 1.25 | 1.25 | 1.25 | |
| L6 | NA | 2 | 2 | 2 | 2 | |
| L7 | NA | 0.9 | 0.9 | 0.9 | 1.8 | |
| L8 | NA | 0.9 | 0.9 | 0.9 | 0.9 | |
| Angle (degree) | NA | 106.26 | 106.26 | 106.26 | 106.26 | |
| Reactions | (m kmol s) | (J/mol) | |
|---|---|---|---|
| 5.10 1013 | −0.82 | 6.91 107 |
| 1.80 107 | 1.00 | 3.70 107 |
| 1.20 106 | 1.30 | 1.52 107 |
| 6.00 106 | 1.30 | 0.00 |
| 1.70 1010 | 0.00 | 2.0 108 |
| 7.50 1017 | −2.60 | 0.00 |
| 1.90 108 | 0.50 | 4.001 108 |
| 2.20 109 | 0.50 | 3.877 108 |
| 2.10 1012 | −1.00 | 0.00 |
| 6.70 1013 | −1.42 | 0.00 |
| 6.70 1013 | −1.42 | 0.00 |
| 2.50 1010 | 0.00 | 2.90 106 |
| 2.50 1011 | 0.00 | 7.90 106 |
| 4.80 1010 | 0.00 | 4.20 106 |
| 5.00 1010 | 0.00 | 4.20 106 |
| 2.00 109 | 0.00 | 0.00 |
| 1.30 1014 | 0.00 | 1.905 108 |
| 1.70 109 | 0.00 | 1.57 107 |
| 1.0 1010 | 0.00 | 7.50 106 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almutairi, F. Numerical Analyses of Entropy Production and Thermodynamics Exergy on a Hydrogen-Fueled Micro Combustor Featuring a Diamond-Shaped Bifurcated Inner-Tube Structure for Thermophotovoltaic Applications. Entropy 2025, 27, 114. https://doi.org/10.3390/e27020114
Almutairi F. Numerical Analyses of Entropy Production and Thermodynamics Exergy on a Hydrogen-Fueled Micro Combustor Featuring a Diamond-Shaped Bifurcated Inner-Tube Structure for Thermophotovoltaic Applications. Entropy. 2025; 27(2):114. https://doi.org/10.3390/e27020114
Chicago/Turabian StyleAlmutairi, Faisal. 2025. "Numerical Analyses of Entropy Production and Thermodynamics Exergy on a Hydrogen-Fueled Micro Combustor Featuring a Diamond-Shaped Bifurcated Inner-Tube Structure for Thermophotovoltaic Applications" Entropy 27, no. 2: 114. https://doi.org/10.3390/e27020114
APA StyleAlmutairi, F. (2025). Numerical Analyses of Entropy Production and Thermodynamics Exergy on a Hydrogen-Fueled Micro Combustor Featuring a Diamond-Shaped Bifurcated Inner-Tube Structure for Thermophotovoltaic Applications. Entropy, 27(2), 114. https://doi.org/10.3390/e27020114






