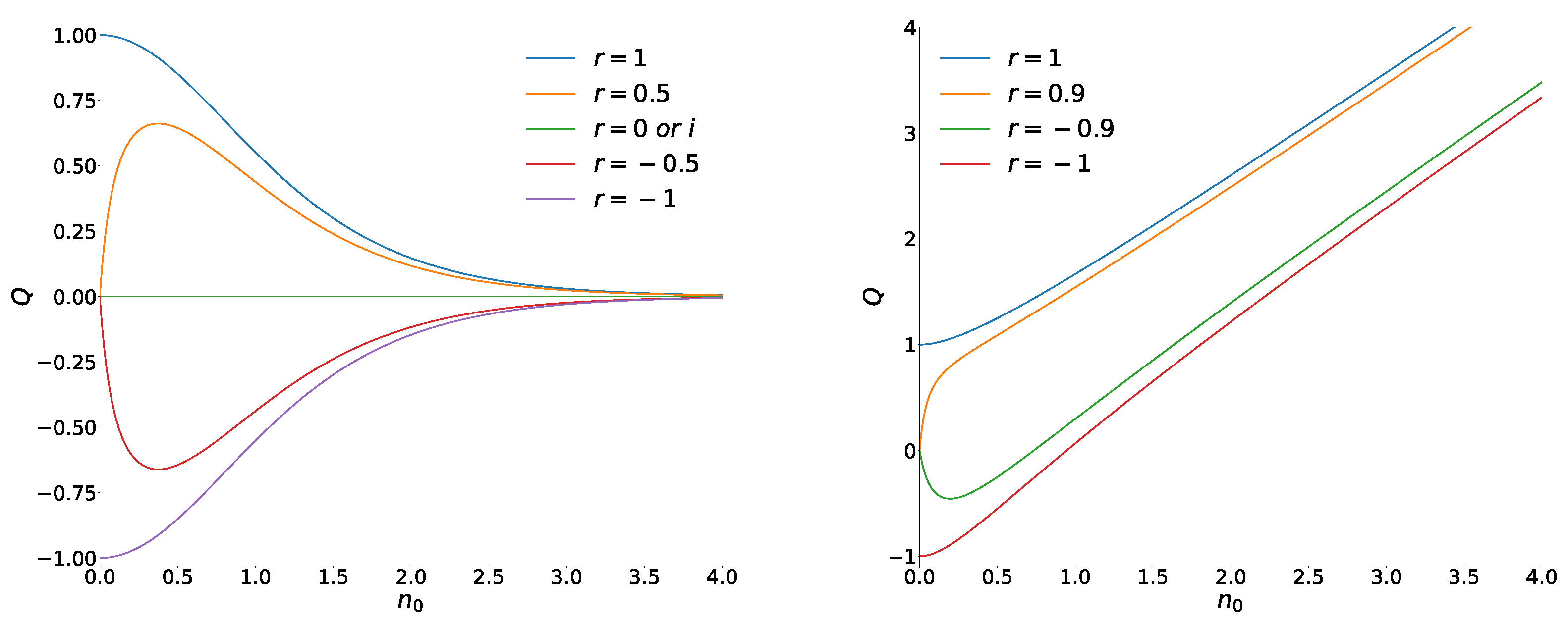Statistical Properties of Superpositions of Coherent Phase States with Opposite Arguments
Abstract
1. Introduction
2. Mean Values and Variances
Usual Coherent States
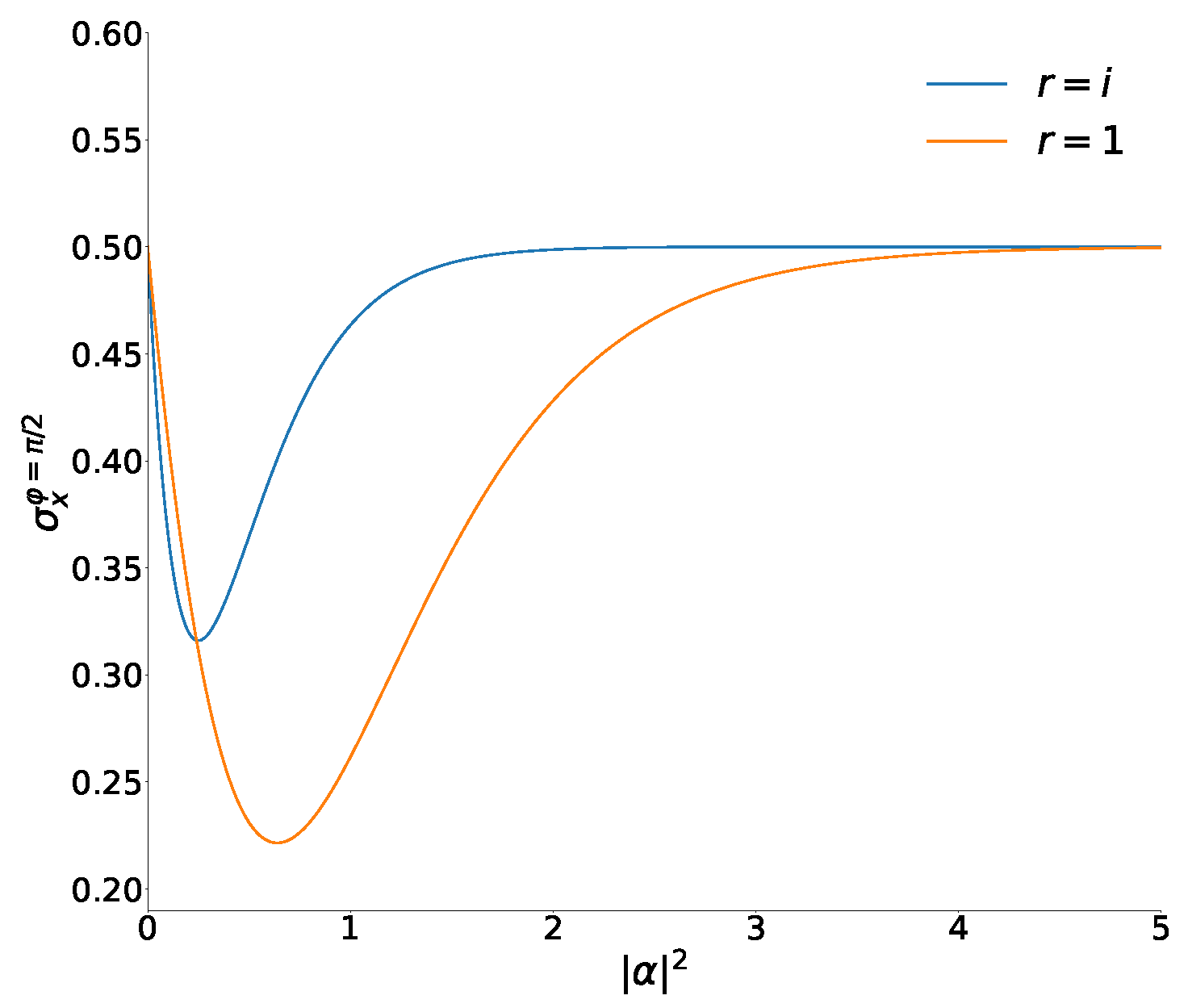
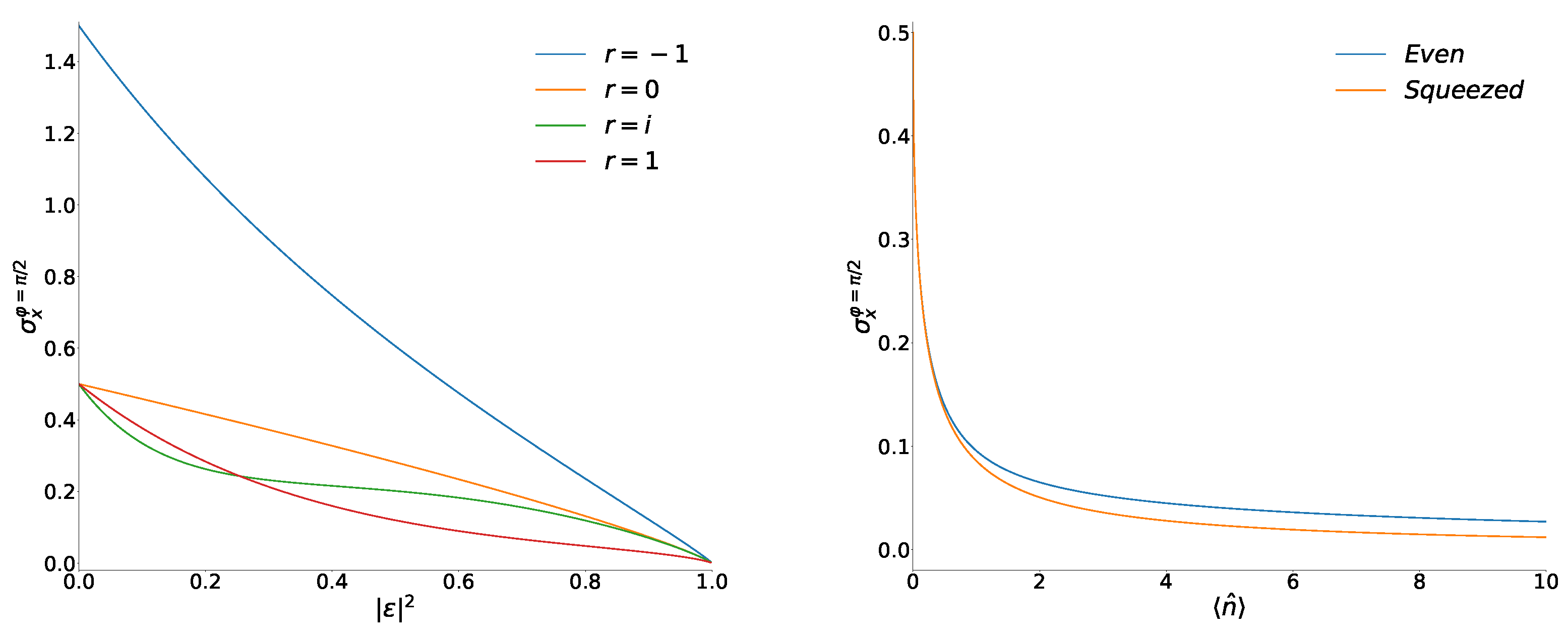
3. Squeezing
3.1. Usual Coherent States
3.2. Coherent Phase States
4. The Robertson–Schrödinger Uncertainty Products
4.1. Usual Coherent States
4.2. Coherent Phase States
5. The Mandel Factor
5.1. Usual Coherent States
5.2. Coherent Phase States
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Klauder, J.R. The action option and a Feynman quantization of spinor fields in terms of ordinary c-numbers. Ann. Phys. 1960, 11, 123–168. [Google Scholar] [CrossRef]
- Glauber, R.J. Photon correlations. Phys. Rev. Lett. 1963, 10, 84–86. [Google Scholar] [CrossRef]
- Sudarshan, E.C.G. Equivalence of semiclassical and quantum mechanical descriptions of statistical light beams. Phys. Rev. Lett. 1963, 10, 277–279. [Google Scholar] [CrossRef]
- Fock, V. Verallgemeinerung und Lösung der Diracschen statistischen Gleichung. Z. Phys. 1928, 49, 339–357. [Google Scholar] [CrossRef]
- Perelomov, A.M. Generalized coherent states and some of their applications. Sov. Phys. Uspekhi 1977, 20, 703–720. [Google Scholar] [CrossRef]
- Zhang, W.-M.; Feng, D.H.; Gilmore, R. Coherent states: Theory and some applications. Rev. Mod. Phys. 1990, 62, 867–927. [Google Scholar] [CrossRef]
- Brif, C. Two-photon algebra eigenstates. A unified approach to squeezing. Ann. Phys. 1996, 251, 180–207. [Google Scholar] [CrossRef][Green Version]
- Dodonov, V.V. ‘Nonclassical’ states in quantum optics: A ‘squeezed’ review of first 75 years. J. Opt. B Quantum Semiclassical Opt. 2002, 4, R1–R33. [Google Scholar] [CrossRef]
- Kastrup, H.A. Quantization of the optical phase space S2 = {φ mod 2π, I > 0} in terms of the group SO↑(1, 2). Fortschritte Phys. 2003, 51, 975–1134. [Google Scholar] [CrossRef]
- Vourdas, A. Analytic representations in quantum mechanics. J. Phys. A Math. Gen. 2006, 39, R65–R141. [Google Scholar] [CrossRef]
- Dell’Anno, F.; De Siena, S.; Illuminati, F. Multiphoton quantum optics and quantum state engineering. Phys. Rep. 2006, 428, 53–168. [Google Scholar] [CrossRef]
- Lerner, E.C.; Huang, H.W.; Walters, G.E. Some mathematical properties of oscillator phase operator. J. Math. Phys. 1970, 11, 1679–1684. [Google Scholar] [CrossRef]
- Aharonov, Y.; Lerner, E.C.; Huang, H.W.; Knight, J.M. Oscillator phase states, thermal equilibrium and group representations. J. Math. Phys. 1973, 14, 746–756. [Google Scholar] [CrossRef]
- Lévy-Leblond, J.-M. Who is afraid of nonhermitian operators? A quantum description of angle and phase. Ann. Phys. 1976, 101, 319–341. [Google Scholar] [CrossRef]
- Vourdas, A. SU(2) and SU(1;1) phase states. Phys. Rev. A 1990, 41, 1653–1661. [Google Scholar] [CrossRef]
- Shapiro, J.H.; Shepard, S.R. Quantum phase measurement: A system-theory perspective. Phys. Rev. A 1991, 43, 3795–3818. [Google Scholar] [CrossRef]
- Vourdas, A.; Brif, C.; Mann, A. Factorization of analytic representations in the unit disc and number-phase statistics of a quantum harmonic oscillator. J. Phys. A Math. Gen. 1996, 29, 5887–5898. [Google Scholar] [CrossRef]
- Wünsche, A. A class of phase-like states. J. Opt. B Quantum Semiclassical Opt. 2001, 3, 206–218. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Mizrahi, S.S. Creating quanta with an ‘annihilation’ operator. J. Phys. A Math. Gen. 2002, 35, 8847–8857. [Google Scholar]
- Gazeau, J.-P.; Hussin, V.; Moran, J.; Zelaya, K. Generalized Susskind–Glogower coherent states. J. Math. Phys. 2021, 62, 072104. [Google Scholar] [CrossRef]
- de Freitas, M.C.; Dodonov, V.V. Coherent phase states in the coordinate and Wigner representations. Quantum Rep. 2022, 4, 509–522. [Google Scholar] [CrossRef]
- Sudarshan, E.C.G. Diagonal harmonious state representation. Int. J. Theor. Phys. 1993, 32, 1069–1076. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Mizrahi, S.S. Uniform nonlinear evolution equations for pure and mixed quantum states. Ann. Phys. 1995, 237, 226–268. [Google Scholar] [CrossRef]
- Susskind, L.; Glogower, J. Quantum mechanical phase and time operator. Physics 1964, 1, 49–61. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Malkin, I.A.; Man‘ko, V.I. Even and odd coherent states and excitations of a singular oscillator. Physica 1974, 72, 597–615. [Google Scholar] [CrossRef]
- Yurke, B.; Stoler, D. Generating quantum mechanical superpositions of macroscopically distinguishable states via amplitude dispersion. Phys. Rev. Lett. 1986, 57, 13–16. [Google Scholar] [CrossRef] [PubMed]
- Obada, A.-S.F.; Darwish, M. Partial phase state as a nonlinear coherent state and some of its properties. J. Mod. Opt. 2004, 51, 209–222. [Google Scholar] [CrossRef]
- Obada, A.-S.F.; Yassin, O.M.; Barnett, S.M. Phase properties of coherent phase and generalized geometric states. J. Mod. Opt. 1997, 44, 149–161. [Google Scholar] [CrossRef]
- Bužek, V.; Vidiella-Barranco, A.; Knight, P.L. Superpositions of coherent states: Squeezing and dissipation. Phys. Rev. A 1992, 45, 6570–6585. [Google Scholar] [CrossRef]
- Schrödinger, E. Zum Heisenbergschen Unschärfeprinzip. Sitzungsberichte der Preussischen Akademie der Wissenschaften. Physikalisch-mathematische Klasse; Akademie der Wissenschaften: Berlin, Germany, 1930; pp. 296–303. [Google Scholar]
- Robertson, H.P. A general formulation of the uncertainty principle and its classical interpretation. Phys. Rev. 1930, 35, 667. [Google Scholar]
- Ritze, H.H.; Bandilla, A. Squeezing and first-order coherence. J. Opt. Soc. Am. B 1987, 4, 1641–1644. [Google Scholar] [CrossRef]
- Lukš, A.; Peřinová, V.; Hradil, Z. Principal squeezing. Acta Phys. Pol. A 1988, 74, 713–721. [Google Scholar]
- Dodonov, V.V. Universal integrals of motion and universal invariants of quantum systems. J. Phys. A Math. Gen. 2000, 33, 7721–7738. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Man’ko, O.V. Universal invariants of quantum-mechanical and optical systems. J. Opt. Soc. Am. A 2000, 17, 2403–2410. [Google Scholar] [CrossRef]
- Mandel, L. Sub-Poissonian photon statistics in resonance fluorescence. Opt. Lett. 1979, 4, 205–207. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
de Freitas, M.C.; Dodonov, V.V. Statistical Properties of Superpositions of Coherent Phase States with Opposite Arguments. Entropy 2024, 26, 977. https://doi.org/10.3390/e26110977
de Freitas MC, Dodonov VV. Statistical Properties of Superpositions of Coherent Phase States with Opposite Arguments. Entropy. 2024; 26(11):977. https://doi.org/10.3390/e26110977
Chicago/Turabian Stylede Freitas, Miguel Citeli, and Viktor V. Dodonov. 2024. "Statistical Properties of Superpositions of Coherent Phase States with Opposite Arguments" Entropy 26, no. 11: 977. https://doi.org/10.3390/e26110977
APA Stylede Freitas, M. C., & Dodonov, V. V. (2024). Statistical Properties of Superpositions of Coherent Phase States with Opposite Arguments. Entropy, 26(11), 977. https://doi.org/10.3390/e26110977