Dephasing Dynamics in a Non-Equilibrium Fluctuating Environment
Abstract
:1. Introduction
2. Quantum Dephasing under the Influence of Non-Equilibrium Environmental Fluctuations
2.1. Non-Equilibrium Environmental Fluctuations Described by Generalized RTN
2.2. Closed Dynamical Equation for the Decoherence Factor under Generalized RTN with a Modulatable Memory Kernel
2.3. Comparisons with Previous Work
3. Results and Discussion
3.1. Dynamical Dephasing in Weak-Coupling Weak-Memory Regime
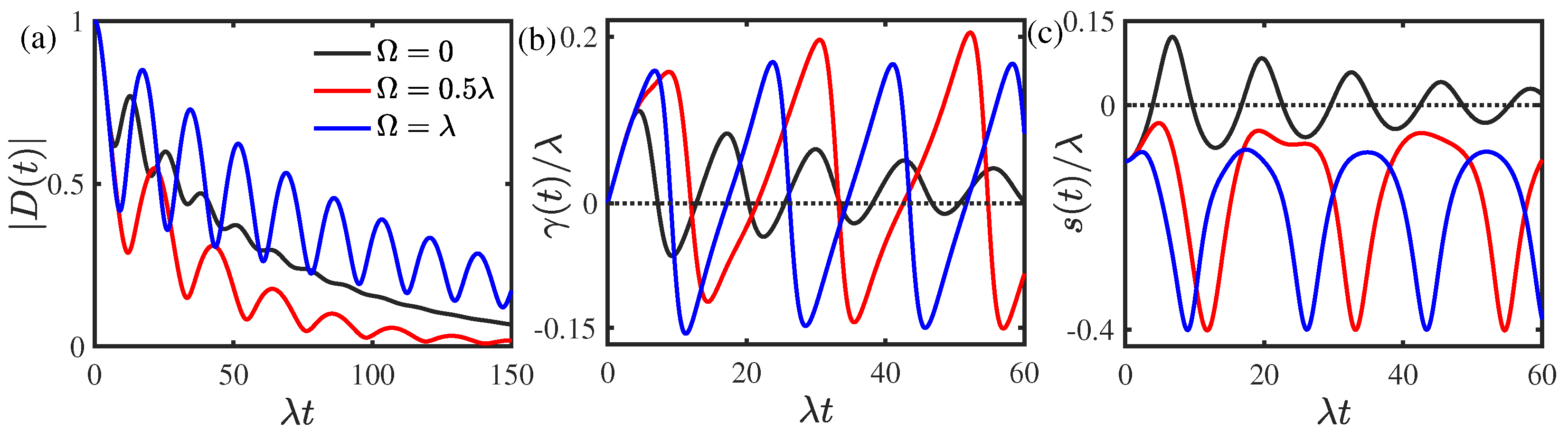
3.2. Dynamical Dephasing in Weak-Coupling Strong-Memory Regime
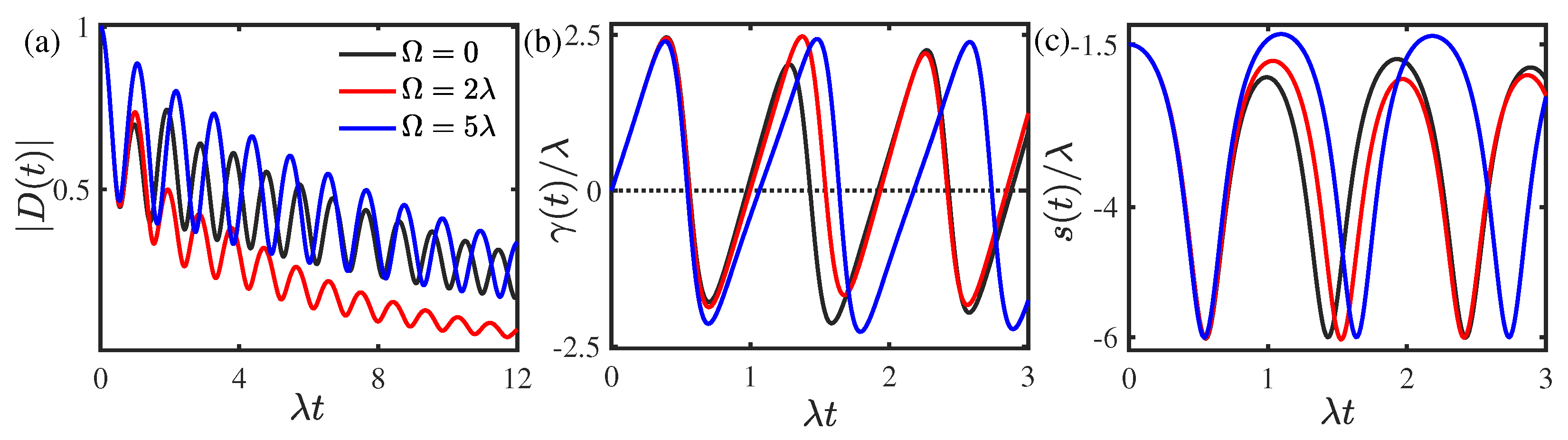
3.3. Dynamical Dephasing in Strong-Coupling Weak-Memory Regime
3.4. Dynamical Dephasing in Strong-Coupling Strong-Memory Regime
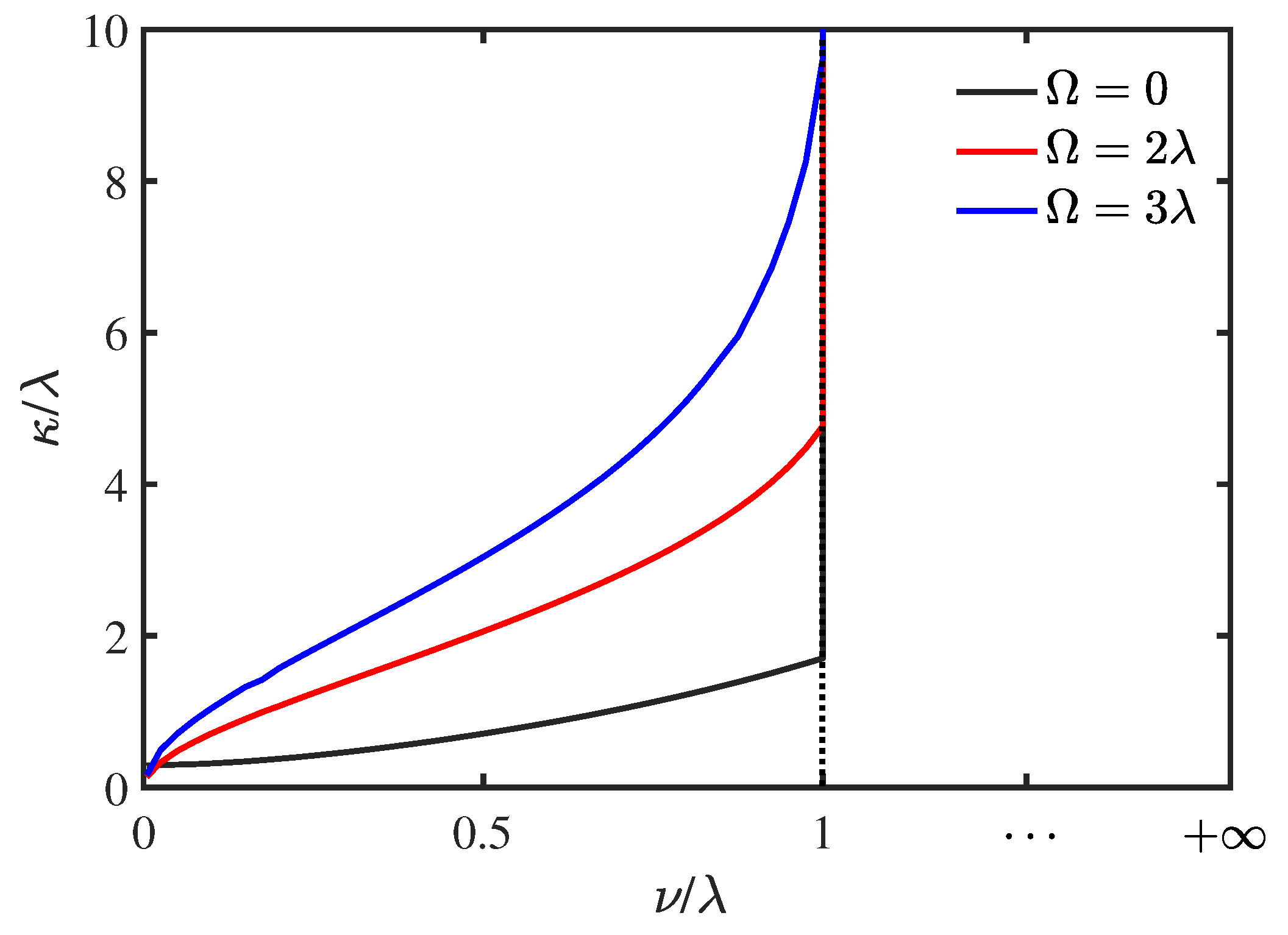
3.5. Conversion between Markovian and Non-Markovian Characters in Dephasing Dynamics
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| RTN | Random telegraph noise |
References
- Breuer, H.P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: New York, NY, USA, 2002. [Google Scholar]
- Zurek, W.H. Decoherence, einselection, and the quantum origins of the classical. Rev. Mod. Phys. 2003, 75, 715. [Google Scholar] [CrossRef] [Green Version]
- Schlosshauer, M. Decoherence, the measurement problem, and interpretations of quantum mechanics. Rev. Mod. Phys. 2005, 76, 1267. [Google Scholar] [CrossRef] [Green Version]
- Streltsov, A.; Adesso, G.; Plenio, M.B. Colloquium: Quantum coherence as a resource. Rev. Mod. Phys. 2017, 89, 041003. [Google Scholar] [CrossRef] [Green Version]
- Hu, M.L.; Hu, X.; Wang, J.; Peng, Y.; Zhang, Y.R.; Fan, H. Quantum coherence and geometric quantum discord. Phys. Rep. 2018, 762, 1. [Google Scholar] [CrossRef] [Green Version]
- Schlosshauer, M. Quantum decoherence. Phys. Rep. 2019, 831, 1. [Google Scholar] [CrossRef] [Green Version]
- Weiss, U. Quantum Dissipative Systems; World Scientific: Singapore, 1999. [Google Scholar]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Schlosshauer, M. Decoherence and the Quantum-to-Classical Transition; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Leggett, A.J.; Chakravarty, S.; Dorsey, A.T.; Fisher, M.P.A.; Garg, A.; Zwerger, W. Dynamics of the dissipative two-state system. Rev. Mod. Phys. 1987, 59, 1. [Google Scholar] [CrossRef]
- Carollo, A.; Valenti, D.; Spagnolo, B. Geometry of quantum phase transitions. Phys. Rep. 2020, 838, 1. [Google Scholar] [CrossRef] [Green Version]
- Gurvitz, S.A.; Fedichkin, L.; Mozyrsky, D.; Berman, G.P. Relaxation and the Zeno Effect in Qubit Measurements. Phys. Rev. Lett. 2003, 91, 066801. [Google Scholar] [CrossRef] [Green Version]
- Lombardo, F.C.; Villar, P.I. Decoherence induced by a composite environment. Phys. Rev. A 2005, 72, 034103. [Google Scholar] [CrossRef] [Green Version]
- Kang, L.; Zhang, Y.; Xu, X.; Tang, X. Quantum measurement of a double quantum dot coupled to two kinds of environment. Phys. Rev. B 2017, 96, 235417. [Google Scholar] [CrossRef]
- Lan, K.; Du, Q.; Kang, L.; Tang, X.; Jiang, L.; Zhang, Y.; Cai, X. Dynamics of an open double quantum dot system via quantum measurement. Phys. Rev. B 2020, 101, 174302. [Google Scholar] [CrossRef]
- Viotti, L.; Lombardo, F.C.; Villar, P.I. Boundary-induced effect encoded in the corrections to the geometric phase acquired by a bipartite two-level system. Phys. Rev. A 2020, 101, 032337. [Google Scholar] [CrossRef] [Green Version]
- Villar, P.I.; Soba, A. Geometric phase accumulated in a driven quantum system coupled to a structured environment. Phys. Rev. A 2020, 101, 052112. [Google Scholar] [CrossRef]
- Sedziak-Kacprowicz, K.; Czerwinski, A.; Kolenderski, P. Tomography of time-bin quantum states using time-resolved detection. Phys. Rev. A 2020, 102, 052420. [Google Scholar] [CrossRef]
- Czerwinski, A.; Sedziak-Kacprowicz, K.; Kolenderski, P. Phase estimation of time-bin qudits by time-resolved single-photon counting. Phys. Rev. A 2021, 103, 042402. [Google Scholar] [CrossRef]
- Viotti, L.; Lombardo, F.C.; Villar, P.I. Geometric phase in a dissipative Jaynes-Cummings model: Theoretical explanation for resonance robustness. Phys. Rev. A 2022, 105, 022218. [Google Scholar] [CrossRef]
- Czerwinski, A.; Szlachetka, J. Efficiency of photonic state tomography affected by fiber attenuation. Phys. Rev. A 2022, 105, 062437. [Google Scholar] [CrossRef]
- Rivas, A.; Huelga, S.F.; Plenio, M.B. Quantum non-Markovianity: Characterization, quantification and detection. Rep. Prog. Phys. 2014, 77, 094001. [Google Scholar] [CrossRef] [PubMed]
- Breuer, H.; Laine, E.; Piilo, J.; Vacchini, B. Colloquium: Non-Markovian dynamics in open quantum systems. Rev. Mod. Phys. 2016, 88, 021002. [Google Scholar] [CrossRef] [Green Version]
- de Vega, I.; Alonso, D. Dynamics of non-Markovian open quantum systems. Rev. Mod. Phys. 2017, 89, 015001. [Google Scholar] [CrossRef] [Green Version]
- Li, L.; Hall, M.J.W.; Wiseman, H.M. Concepts of quantum non-Markovianity: A hierarchy. Phys. Rep. 2018, 759, 1. [Google Scholar] [CrossRef] [Green Version]
- Diósi, L.; Gisin, N.; Strunz, W.T. Non-Markovian quantum state diffusion. Phys. Rev. A 1998, 58, 1699. [Google Scholar] [CrossRef] [Green Version]
- Yu, T.; Diósi, L.; Gisin, N.; Strunz, W.T. Non-Markovian quantum-state diffusion: Perturbation approach. Phys. Rev. A 1999, 60, 91. [Google Scholar] [CrossRef] [Green Version]
- Strunz, W.T.; Diósi, L.; Gisin, N. Open System Dynamics with Non-Markovian Quantum Trajectories. Phys. Rev. Lett. 1999, 82, 1801. [Google Scholar] [CrossRef] [Green Version]
- Breuer, H.P.; Kappler, B.; Petruccione, F. Stochastic wave-function method for non-Markovian quantum master equations. Phys. Rev. A 1999, 59, 1633. [Google Scholar] [CrossRef] [Green Version]
- Breuer, H.P.; Burgarth, D.; Petruccione, F. Non-Markovian dynamics in a spin star system: Exact solution and approximation techniques. Phys. Rev. B 2004, 70, 045323. [Google Scholar] [CrossRef] [Green Version]
- Budini, A.A. Random Lindblad equations from complex environments. Phys. Rev. E 2005, 72, 056106. [Google Scholar] [CrossRef] [Green Version]
- Breuer, H.P.; Gemmer, J.; Michel, M. Non-Markovian quantum dynamics: Correlated projection superoperators and Hilbert space averaging. Phys. Rev. E 2006, 73, 016139. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cai, X. Quantum Dynamics in a Fluctuating Environment. Entropy 2019, 21, 1040. [Google Scholar] [CrossRef] [Green Version]
- Piilo, J.; Härkönen, K.; Maniscalco, S.; Suominen, K.A. Open system dynamics with non-Markovian quantum jumps. Phys. Rev. A 2009, 79, 062112. [Google Scholar] [CrossRef] [Green Version]
- Tu, M.W.Y.; Zhang, W.M. Non-Markovian decoherence theory for a double-dot charge qubit. Phys. Rev. B 2008, 78, 235311. [Google Scholar] [CrossRef] [Green Version]
- Zhang, W.M.; Lo, P.Y.; Xiong, H.N.; Tu, M.W.Y.; Nori, F. General Non-Markovian Dynamics of Open Quantum Systems. Phys. Rev. Lett. 2012, 109, 170402. [Google Scholar] [CrossRef]
- Shabani, A.; Lidar, D.A. Completely positive post-Markovian master equation via a measurement approach. Phys. Rev. A 2005, 71, 020101. [Google Scholar] [CrossRef] [Green Version]
- Vacchini, B.; Breuer, H.P. Exact master equations for the non-Markovian decay of a qubit. Phys. Rev. A 2010, 81, 042103. [Google Scholar] [CrossRef] [Green Version]
- Vacchini, B. Non-Markovian master equations from piecewise dynamics. Phys. Rev. A 2013, 87, 030101. [Google Scholar] [CrossRef] [Green Version]
- Lombardo, F.C.; Villar, P.I. Corrections to the Berry phase in a solid-state qubit due to low-frequency noise. Phys. Rev. A 2014, 89, 012110. [Google Scholar] [CrossRef] [Green Version]
- Man, Z.; Xia, Y.; Lo Franco, R. Harnessing non-Markovian quantum memory by environmental coupling. Phys. Rev. A 2015, 92, 012315. [Google Scholar] [CrossRef] [Green Version]
- Vacchini, B. Generalized Master Equations Leading to Completely Positive Dynamics. Phys. Rev. Lett. 2016, 117, 230401. [Google Scholar] [CrossRef] [Green Version]
- Yan, Y.A.; Shao, J. Equivalence of stochastic formulations and master equations for open systems. Phys. Rev. A 2018, 97, 042126. [Google Scholar] [CrossRef]
- Man, Z.X.; Xia, Y.J.; Lo Franco, R. Temperature effects on quantum non-Markovianity via collision models. Phys. Rev. A 2018, 97, 062104. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Man, Z.X.; Xia, Y.J. Non-Markovianity and the Landauer principle in composite thermal environments. Phys. Rev. A 2021, 103, 032201. [Google Scholar] [CrossRef]
- Czerwinski, A. Open quantum systems integrable by partial commutativity. Phys. Rev. A 2020, 102, 062423. [Google Scholar] [CrossRef]
- Martens, C.C. Communication: Decoherence in a nonequilibrium environment: An analytically solvable model. J. Chem. Phys. 2010, 133, 241101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lombardo, F.C.; Villar, P.I. Nonunitary geometric phases: A qubit coupled to an environment with random noise. Phys. Rev. A 2013, 87, 032338. [Google Scholar] [CrossRef] [Green Version]
- Zheng, Y.; Brown, F.L.H. Single-Molecule Photon Counting Statistics via Generalized Optical Bloch Equations. Phys. Rev. Lett. 2003, 90, 238305. [Google Scholar] [CrossRef]
- Brokmann, X.; Hermier, J.P.; Messin, G.; Desbiolles, P.; Bouchaud, J.P.; Dahan, M. Statistical Aging and Nonergodicity in the Fluorescence of Single Nanocrystals. Phys. Rev. Lett. 2003, 90, 120601. [Google Scholar] [CrossRef] [Green Version]
- Burkard, G. Non-Markovian qubit dynamics in the presence of 1/f noise. Phys. Rev. B 2009, 79, 125317. [Google Scholar] [CrossRef] [Green Version]
- Rossi, M.A.C.; Paris, M.G.A. Non-Markovian dynamics of single- and two-qubit systems interacting with Gaussian and non-Gaussian fluctuating transverse environments. J. Chem. Phys. 2016, 144, 024113. [Google Scholar] [CrossRef] [Green Version]
- Benedetti, C.; Buscemi, F.; Bordone, P.; Paris, M.G.A. Dynamics of quantum correlations in colored-noise environments. Phys. Rev. A 2013, 87, 052328. [Google Scholar] [CrossRef] [Green Version]
- Benedetti, C.; Paris, M.G.A.; Maniscalco, S. Non-Markovianity of colored noisy channels. Phys. Rev. A 2014, 89, 012114. [Google Scholar] [CrossRef] [Green Version]
- Benedetti, C.; Buscemi, F.; Bordone, P.; Paris, M.G.A. Effects of classical environmental noise on entanglement and quantum discord dynamics. Int. J. Quantum Inf. 2012, 8, 1241005. [Google Scholar] [CrossRef]
- Lo Franco, R.; D’Arrigo, A.; Falci, G.; Compagno, G.; Paladino, E. Entanglement dynamics in superconducting qubits affected by local bistable impurities. Phys. Scr. 2012, T147, 014019. [Google Scholar] [CrossRef] [Green Version]
- Silveri, M.P.; Tuorila, J.A.; Thuneberg, E.V.; Paraoanu, G.S. Quantum systems under frequency modulation. Rep. Prog. Phys. 2017, 80, 056002. [Google Scholar] [CrossRef] [Green Version]
- Cialdi, S.; Rossi, M.A.C.; Benedetti, C.; Vacchini, B.; Tamascelli, D.; Olivares, S.; Paris, M.G.A. All-optical quantum simulator of qubit noisy channels. Appl. Phys. Lett. 2017, 110, 081107. [Google Scholar] [CrossRef] [Green Version]
- Cialdi, S.; Benedetti, C.; Tamascelli, D.; Olivares, S.; Paris, M.G.A.; Vacchini, B. Experimental investigation of the effect of classical noise on quantum non-Markovian dynamics. Phys. Rev. A 2019, 100, 052104. [Google Scholar] [CrossRef] [Green Version]
- Fuliński, A. Non-Markovian noise. Phys. Rev. E 1994, 50, 2668. [Google Scholar] [CrossRef] [PubMed]
- Cai, X.; Zheng, Y. Decoherence induced by non-Markovian noise in a nonequilibrium environment. Phys. Rev. A 2016, 94, 042110. [Google Scholar] [CrossRef]
- Cai, X.; Zheng, Y. Non-Markovian decoherence dynamics in nonequilibrium environments. J. Chem. Phys. 2018, 149, 094107. [Google Scholar] [CrossRef] [PubMed]
- Cai, X.; Zheng, Y. Quantum dynamical speedup in a nonequilibrium environment. Phys. Rev. A 2017, 95, 052104. [Google Scholar] [CrossRef]
- Lin, D.; Zou, H.M.; Yang, J. Based-nonequilibrium-environment non-Markovianity, quantum Fisher information and quantum coherence. Phys. Scr. 2019, 95, 015103. [Google Scholar] [CrossRef]
- Cai, X.; Meng, R.; Zhang, Y.; Wang, L. Geometry of quantum evolution in a nonequilibrium environment. Europhys. Lett. 2019, 125, 30007. [Google Scholar] [CrossRef] [Green Version]
- Basit, A.; Ali, H.; Badshah, F.; Yang, X.F.; Ge, G.Q. Controlling sudden transition from classical to quantum decoherence via non-equilibrium environments. New J. Phys. 2020, 22, 033039. [Google Scholar] [CrossRef]
- Basit, A.; Ali, H.; Badshah, F.; Yang, X.F.; Ge, G. Nonequilibrium effects on one-norm geometric correlations and the emergence of a pointer-state basis in the weak- and strong-coupling regimes. Phys. Rev. A 2021, 104, 042417. [Google Scholar] [CrossRef]
- Cai, X. Quantum dephasing induced by non-Markovian random telegraph noise. Sci. Rep. 2020, 10, 88. [Google Scholar] [CrossRef] [Green Version]
- Martens, C.C. Quantum dephasing of a two-state system by a nonequilibrium harmonic oscillator. J. Chem. Phys. 2013, 139, 024109. [Google Scholar] [CrossRef] [PubMed]
- Lombardo, F.C.; Villar, P.I. Correction to the geometric phase by structured environments: The onset of non-Markovian effects. Phys. Rev. A 2015, 91, 042111. [Google Scholar] [CrossRef] [Green Version]
- van Kampen, N.G. Stochastic Process in Physics and Chemistry; North-Holland: Amsterdam, The Netherland, 1992. [Google Scholar]
- Breuer, H.; Laine, E.; Piilo, J. Measure for the Degree of Non-Markovian Behavior of Quantum Processes in Open Systems. Phys. Rev. Lett. 2009, 103, 210401. [Google Scholar] [CrossRef] [Green Version]
- Zheng, L.; Peng, Y. Quantum decoherence of a two-level system in colored environments. Phys. Rev. A 2022, 105, 052443. [Google Scholar] [CrossRef]
- Möttönen, M.; de Sousa, R.; Zhang, J.; Whaley, K.B. High-fidelity one-qubit operations under random telegraph noise. Phys. Rev. A 2006, 73, 022332. [Google Scholar] [CrossRef] [Green Version]
- Bergli, J.; Faoro, L. Exact solution for the dynamical decoupling of a qubit with telegraph noise. Phys. Rev. B 2007, 75, 054515. [Google Scholar] [CrossRef] [Green Version]
- Cywiński, L.; Lutchyn, R.M.; Nave, C.P.; Das Sarma, S. How to enhance dephasing time in superconducting qubits. Phys. Rev. B 2008, 77, 174509. [Google Scholar] [CrossRef] [Green Version]
- Chen, M.; Chen, H.; Han, T.; Cai, X. Disentanglement Dynamics in Nonequilibrium Environments. Entropy 2022, 24, 1330. [Google Scholar] [CrossRef]
- Maniscalco, S.; Petruccione, F. Non-Markovian dynamics of a qubit. Phys. Rev. A 2006, 73, 012111. [Google Scholar] [CrossRef] [Green Version]
- Mazzola, L.; Laine, E.M.; Breuer, H.P.; Maniscalco, S.; Piilo, J. Phenomenological memory-kernel master equations and time-dependent Markovian processes. Phys. Rev. A 2010, 81, 062120. [Google Scholar] [CrossRef] [Green Version]
- Lindenberg, K.; West, B.J. Statistical properties of quantum systems: The linear oscillator. Phys. Rev. A 1984, 30, 568. [Google Scholar] [CrossRef]
- Kalandarov, S.A.; Kanokov, Z.; Adamian, G.G.; Antonenko, N.V.; Scheid, W. Non-Markovian dynamics of an open quantum system with nonstationary coupling. Phys. Rev. E 2011, 83, 041104. [Google Scholar] [CrossRef] [PubMed]
- Min, W.; Luo, G.; Cherayil, B.J.; Kou, S.C.; Xie, X.S. Observation of a Power-Law Memory Kernel for Fluctuations within a Single Protein Molecule. Phys. Rev. Lett. 2005, 94, 198302. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, X.; Sun, Y.; Wang, Q.; Ren, J.; Cai, X.; Czerwinski, A. Dephasing Dynamics in a Non-Equilibrium Fluctuating Environment. Entropy 2023, 25, 634. https://doi.org/10.3390/e25040634
Meng X, Sun Y, Wang Q, Ren J, Cai X, Czerwinski A. Dephasing Dynamics in a Non-Equilibrium Fluctuating Environment. Entropy. 2023; 25(4):634. https://doi.org/10.3390/e25040634
Chicago/Turabian StyleMeng, Xiangjia, Yaxin Sun, Qinglong Wang, Jing Ren, Xiangji Cai, and Artur Czerwinski. 2023. "Dephasing Dynamics in a Non-Equilibrium Fluctuating Environment" Entropy 25, no. 4: 634. https://doi.org/10.3390/e25040634
APA StyleMeng, X., Sun, Y., Wang, Q., Ren, J., Cai, X., & Czerwinski, A. (2023). Dephasing Dynamics in a Non-Equilibrium Fluctuating Environment. Entropy, 25(4), 634. https://doi.org/10.3390/e25040634








