Thermoelectric Rectification and Amplification in Interacting Quantum-Dot Circuit-Quantum-Electrodynamics Systems
Abstract
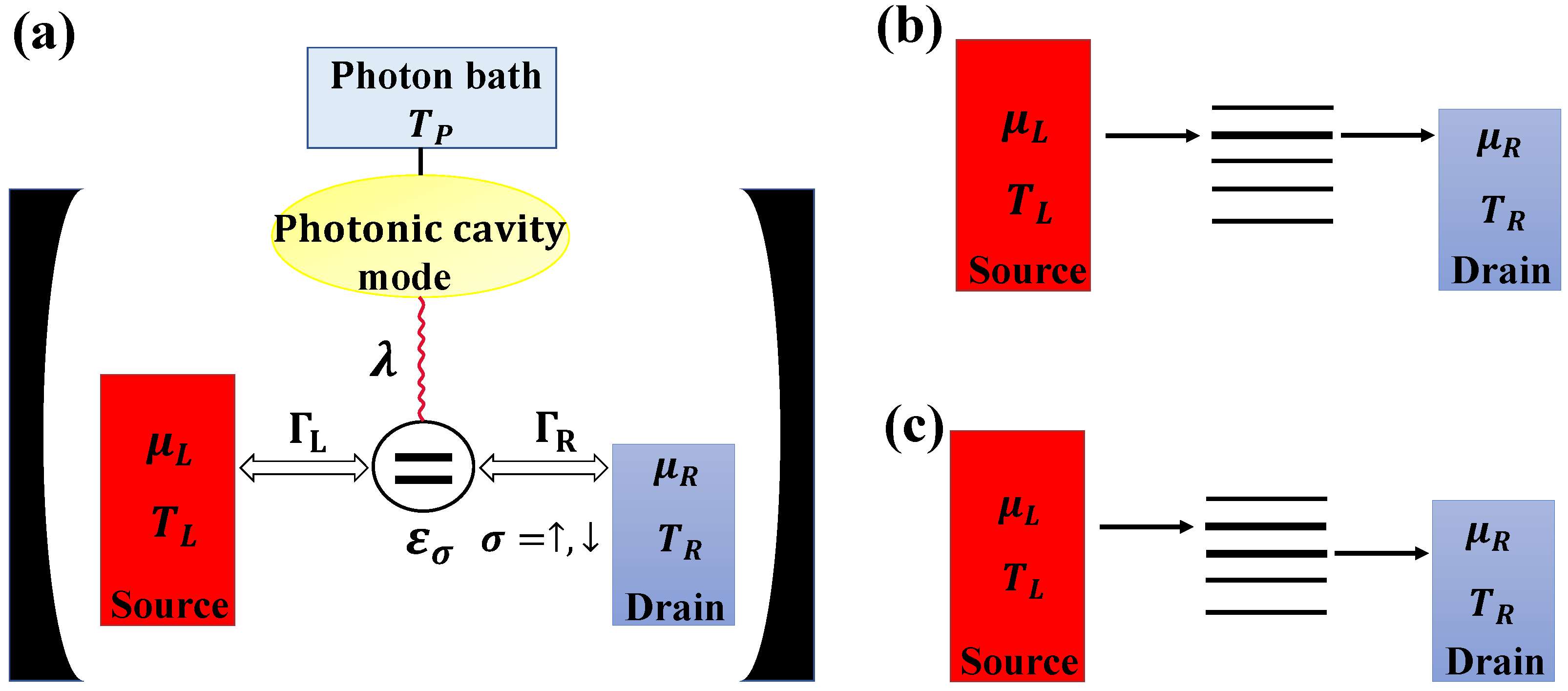
1. Introduction
2. Method and Approximation
3. Non-Perturbative Hybridized Dot Green’s Function
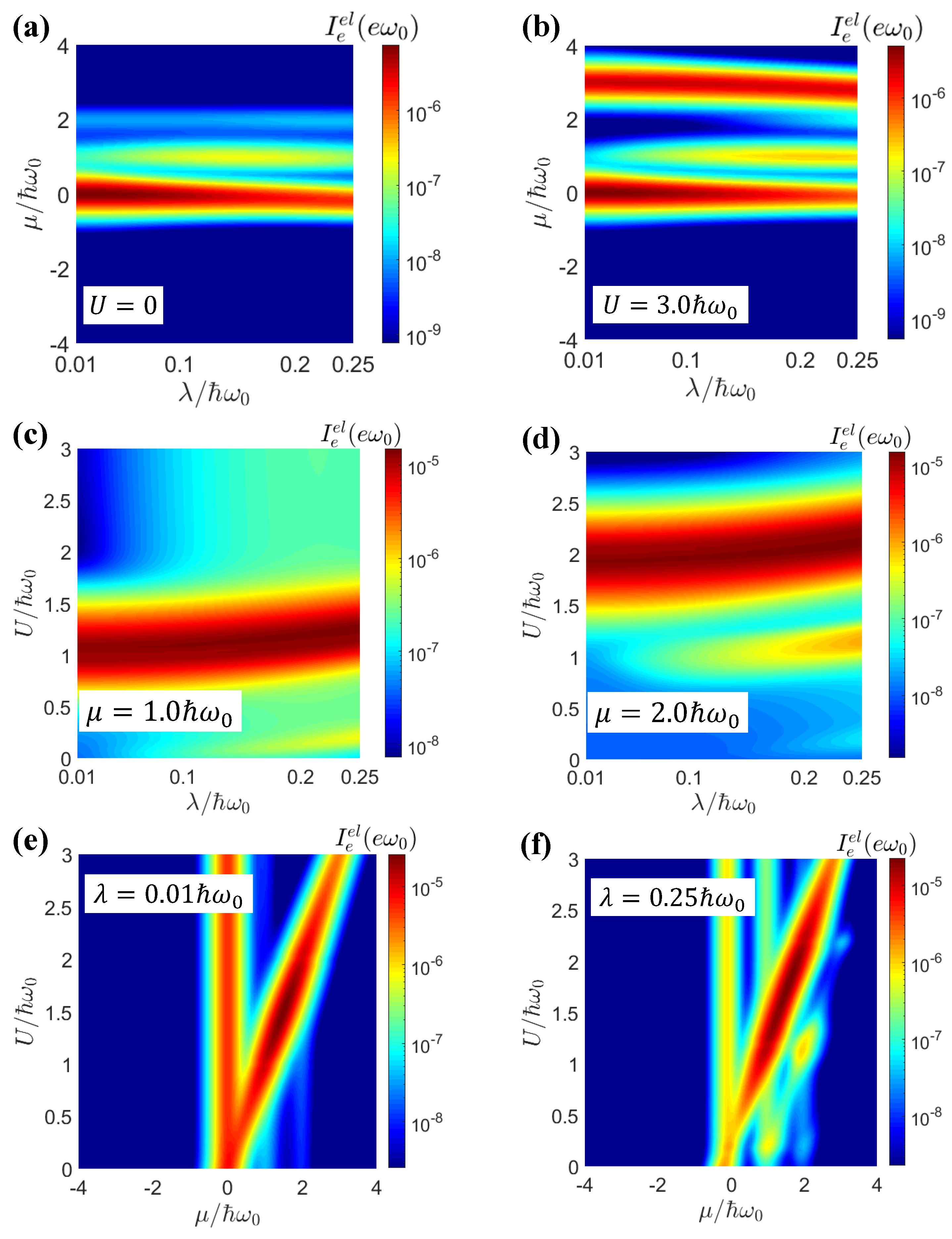
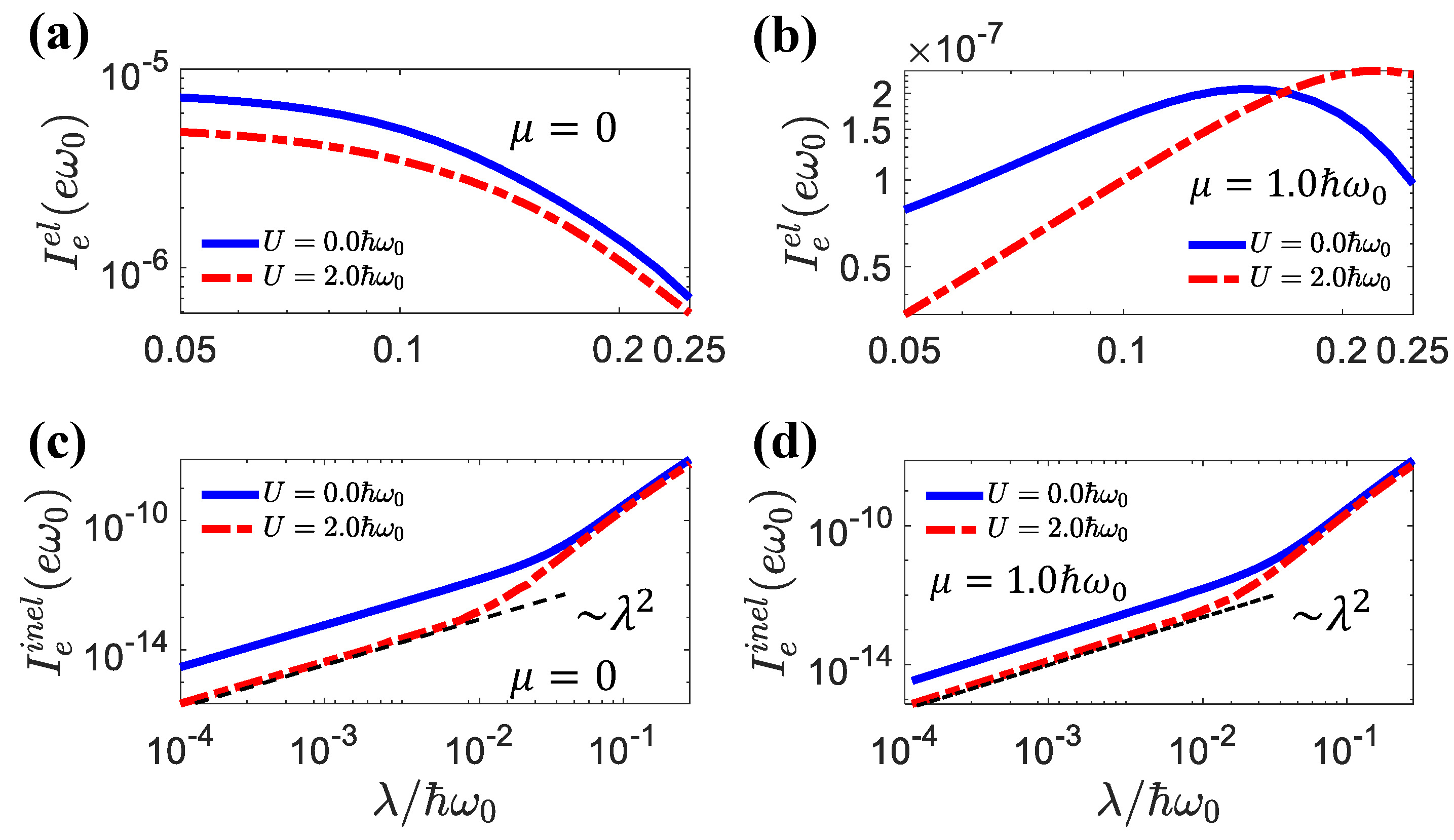
4. Inelastic and Elastic Currents
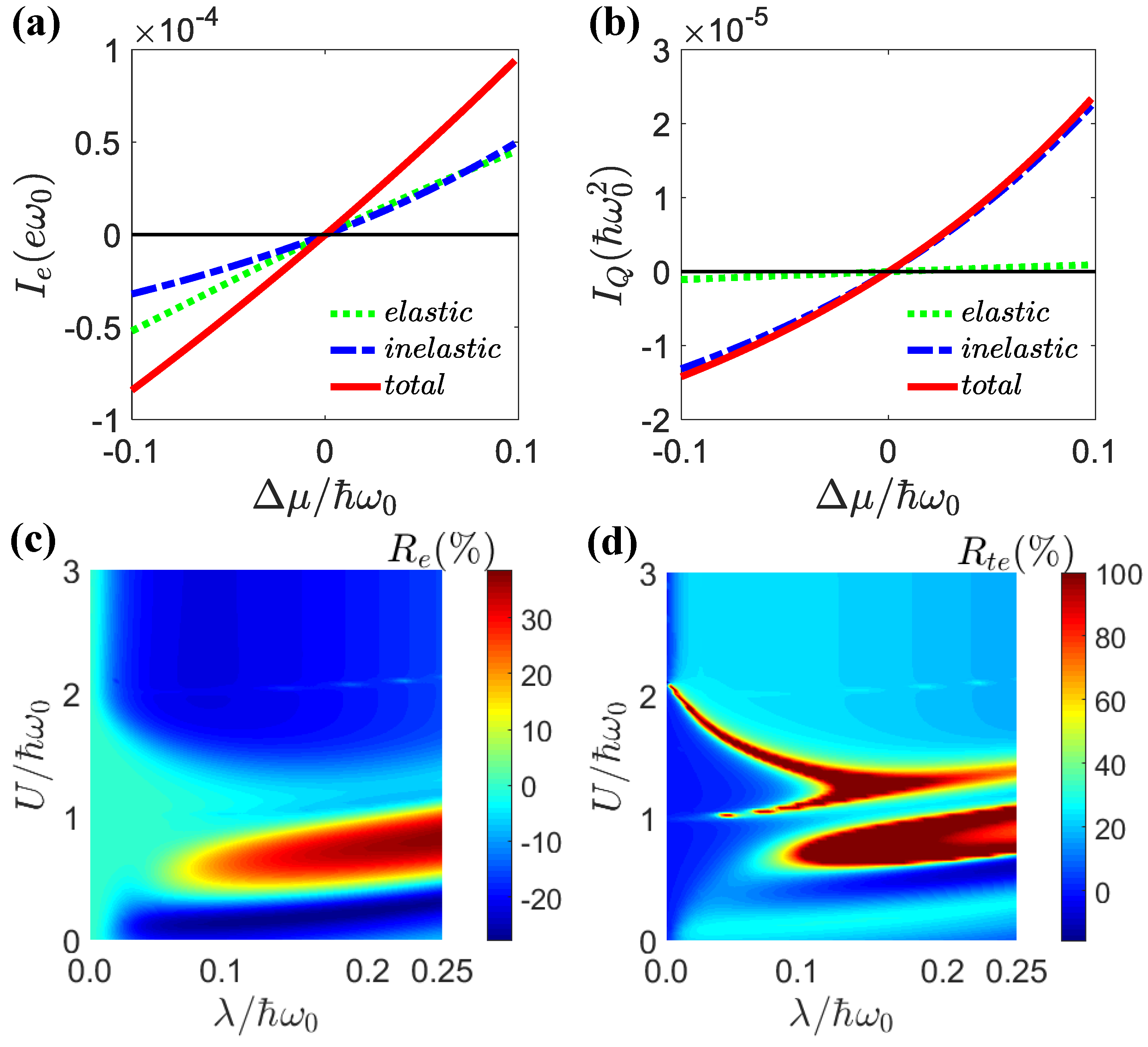
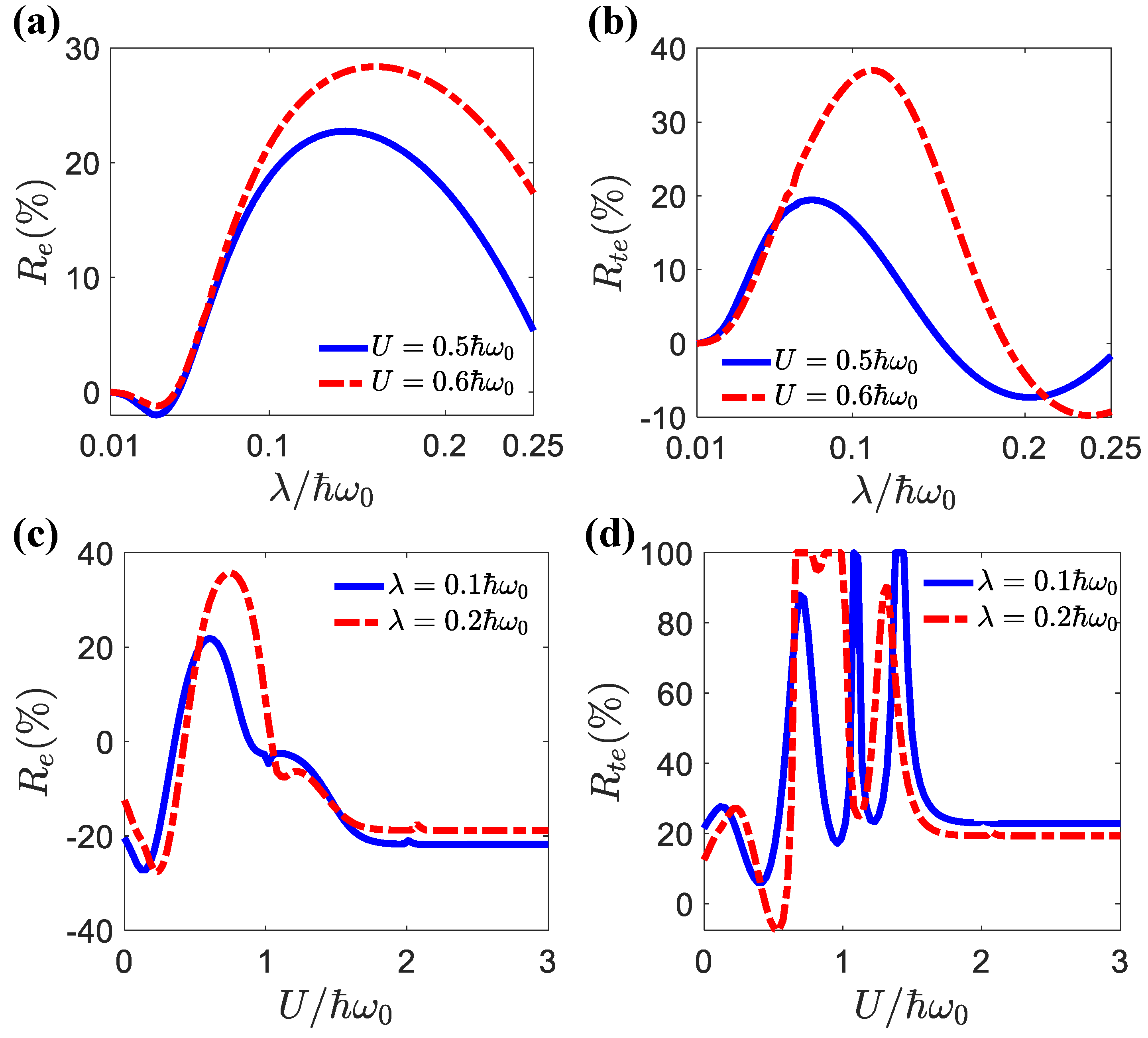
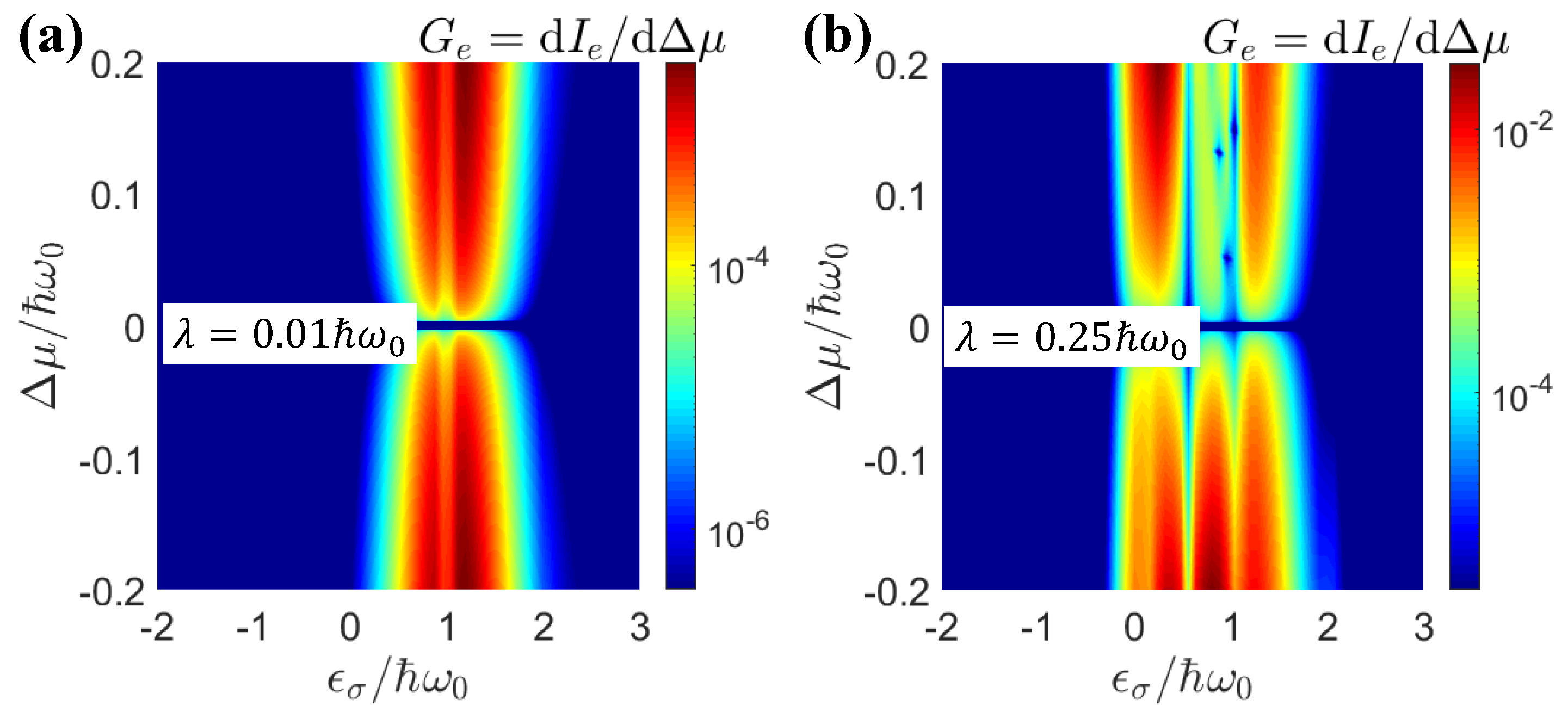
5. Rectification Effects
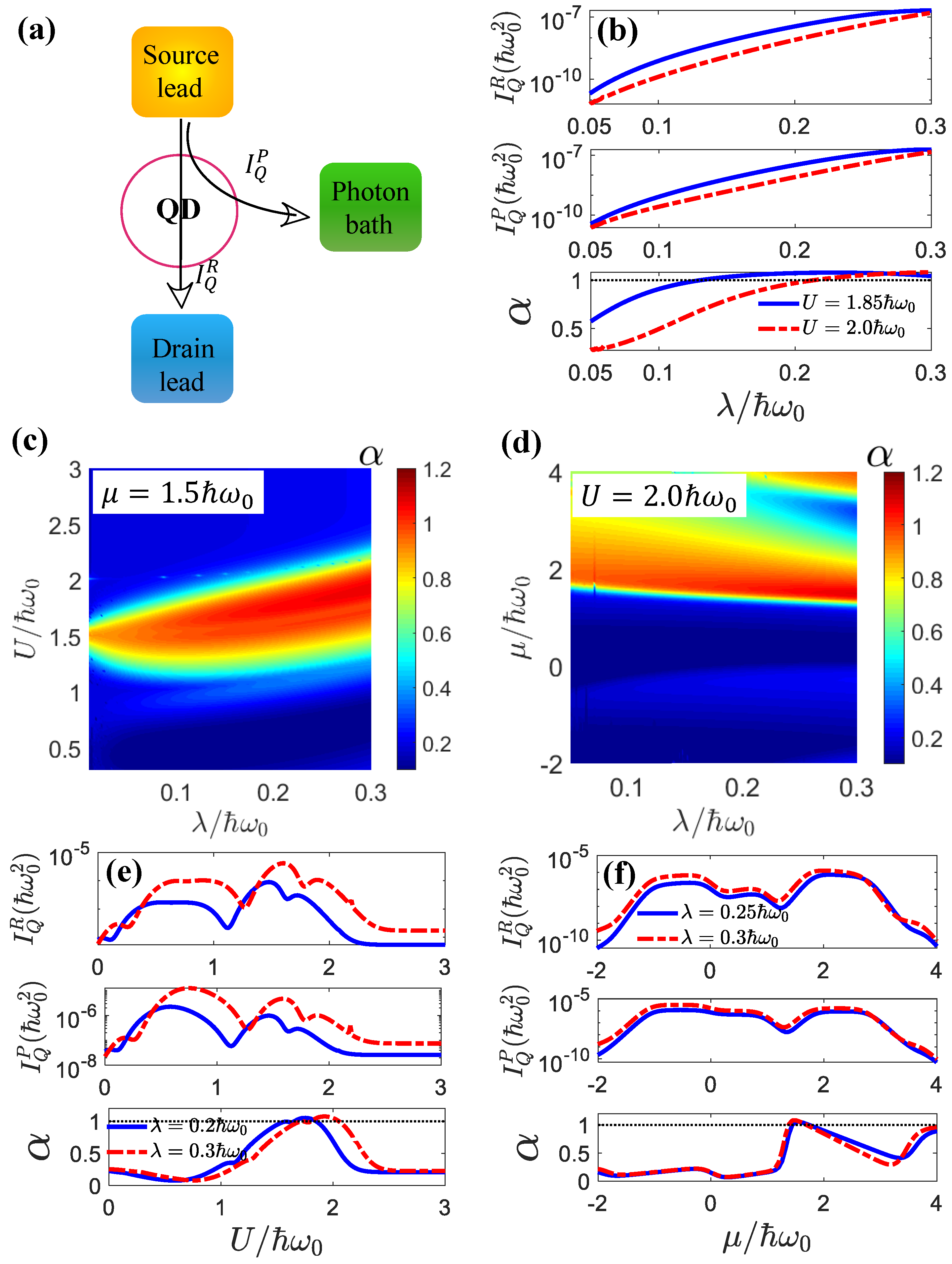
6. Thermal Transistor Effects in the Linear Transport Regime
7. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, N.; Ren, J.; Wang, L.; Zhang, G.; Hänggi, P.; Li, B. Colloquium: Phononics: Manipulating heat flow with electronic analogs and beyond. Rev. Mod. Phys. 2012, 84, 1045–1066. [Google Scholar] [CrossRef]
- Jiang, J.H.; Imry, Y. Linear and nonlinear mesoscopic thermoelectric transport with coupling with heat baths. C. R. Phys. 2016, 17, 1047–1059. [Google Scholar] [CrossRef]
- Benenti, G.; Casati, G.; Saito, K.; Whitney, R. Fundamental aspects of steady-state conversion of heat to work at the nanoscale. Phys. Rep. 2017, 694, 1–124. [Google Scholar] [CrossRef]
- Cottet, A.; Dartiailh, M.C.; Desjardins, M.M.; Cubaynes, T.; Contamin, L.C.; Delbecq, M.; Viennot, J.J.; Bruhat, L.E.; Douçot, B.; Kontos, T. Cavity QED with hybrid nanocircuits: From atomic-like physics to condensed matter phenomena. J. Phys. Condens. Matter 2017, 29, 433002. [Google Scholar] [CrossRef]
- Pekola, J.P.; Karimi, B. Colloquium: Quantum heat transport in condensed matter systems. Rev. Mod. Phys. 2021, 93, 041001. [Google Scholar] [CrossRef]
- Myers, N.M.; Abah, O.; Deffner, S. Quantum thermodynamic devices: From theoretical proposals to experimental reality. AVS Quantum Sci. 2022, 4, 027101. [Google Scholar] [CrossRef]
- Wang, R.; Wang, C.; Lu, J.; Jiang, J.H. Inelastic thermoelectric transport and fluctuations in mesoscopic systems. Adv. Phys. X 2022, 7, 2082317. [Google Scholar] [CrossRef]
- Burkard, G.; Gullans, M.J.; Mi, X.; Petta, J.R. Superconductor-semiconductor hybrid-circuit quantum electrodynamics. Nat. Rev. Phys. 2020, 2, 129–140. [Google Scholar] [CrossRef]
- Cottet, A.; Kontos, T.; Douçot, B. Electron-photon coupling in mesoscopic quantum electrodynamics. Phys. Rev. B 2015, 91, 205417. [Google Scholar] [CrossRef]
- Hagenmüller, D.; Schachenmayer, J.; Schütz, S.; Genes, C.; Pupillo, G. Cavity-Enhanced Transport of Charge. Phys. Rev. Lett. 2017, 119, 223601. [Google Scholar] [CrossRef]
- Buffoni, L.; Solfanelli, A.; Verrucchi, P.; Cuccoli, A.; Campisi, M. Quantum Measurement Cooling. Phys. Rev. Lett. 2019, 122, 070603. [Google Scholar] [CrossRef] [PubMed]
- Chen, Z.H.; Che, H.X.; Chen, Z.K.; Wang, C.; Ren, J. Tuning nonequilibrium heat current and two-photon statistics via composite qubit-resonator interaction. Phys. Rev. Res. 2022, 4, 013152. [Google Scholar] [CrossRef]
- Li, B.; Calarco, T.; Motzoi, F. Nonperturbative Analytical Diagonalization of Hamiltonians with Application to Circuit QED. PRX Quantum 2022, 3, 030313. [Google Scholar] [CrossRef]
- Wang, F.Y.; Lu, J.C.; Wang, Z.; Duan, L.W.; Wang, C.; Ren, J. Nonequilibrium thermal transport in the two-mode qubit-resonator system. Front. Phys. 2022, 10, 964858. [Google Scholar] [CrossRef]
- Kulkarni, M.; Cotlet, O.; Türeci, H.E. Cavity-coupled double-quantum dot at finite bias: Analogy with lasers and beyond. Phys. Rev. B 2014, 90, 125402. [Google Scholar] [CrossRef]
- Härtle, R.; Kulkarni, M. Effect of broadening in the weak-coupling limit of vibrationally coupled electron transport through molecular junctions and the analogy to quantum dot circuit QED systems. Phys. Rev. B 2015, 91, 245429. [Google Scholar] [CrossRef]
- Bruhat, L.E.; Cubaynes, T.; Viennot, J.J.; Dartiailh, M.C.; Desjardins, M.M.; Cottet, A.; Kontos, T. Circuit QED with a quantum-dot charge qubit dressed by Cooper pairs. Phys. Rev. B 2018, 98, 155313. [Google Scholar] [CrossRef]
- Purkayastha, A.; Kulkarni, M.; Joglekar, Y.N. Emergent PT symmetry in a double-quantum-dot circuit QED setup. Phys. Rev. Research 2020, 2, 043075. [Google Scholar] [CrossRef]
- Thierschmann, H.; Sánchez, R.; Sothmann, B.; Arnold, F.; Heyn, C.; Hansen, W.; Buhmann, H.; Molenkamp, L.W. Three-terminal energy harvester with coupled quantum dots. Nat. Nanotech. 2015, 10, 854. [Google Scholar] [CrossRef]
- Hartke, T.R.; Liu, Y.Y.; Gullans, M.J.; Petta, J.R. Microwave Detection of Electron-Phonon Interactions in a Cavity-Coupled Double Quantum Dot. Phys. Rev. Lett. 2018, 120, 097701. [Google Scholar] [CrossRef]
- Wang, C.; Wang, L.Q.; Ren, J. Impact of counter-rotating-wave term on quantum heat transfer and phonon statistics in nonequilibrium qubit-phonon hybrid system. Chin. Phys. B 2021, 30, 030506. [Google Scholar] [CrossRef]
- Wang, C.; Wang, L.Q.; Ren, J. Managing Quantum Heat Transfer in a Nonequilibrium Qubit-Phonon Hybrid System with Coherent Phonon States. Chin. Phys. Lett. 2021, 38, 010501. [Google Scholar] [CrossRef]
- Liu, Y.Y.; Petersson, K.D.; Stehlik, J.; Taylor, J.M.; Petta, J.R. Photon Emission from a Cavity-Coupled Double Quantum Dot. Phys. Rev. Lett. 2014, 113, 036801. [Google Scholar] [CrossRef] [PubMed]
- Agarwalla, B.K.; Kulkarni, M.; Mukamel, S.; Segal, D. Tunable photonic cavity coupled to a voltage-biased double quantum dot system: Diagrammatic nonequilibrium Green’s function approach. Phys. Rev. B 2016, 94, 035434. [Google Scholar] [CrossRef]
- Agarwalla, B.K.; Kulkarni, M.; Mukamel, S.; Segal, D. Giant photon gain in large-scale quantum dot-circuit QED systems. Phys. Rev. B 2016, 94, 121305. [Google Scholar] [CrossRef]
- Agarwalla, B.K.; Kulkarni, M.; Segal, D. Photon statistics of a double quantum dot micromaser: Quantum treatment. arXiv 2019, arXiv:1903.08209. [Google Scholar] [CrossRef]
- Lambert, N.; Nori, F.; Flindt, C. Bistable Photon Emission from a Solid-State Single-Atom Laser. Phys. Rev. Lett. 2015, 115, 216803. [Google Scholar] [CrossRef]
- Sánchez, R.; Büttiker, M. Optimal energy quanta to current conversion. Phys. Rev. B 2011, 83, 085428. [Google Scholar] [CrossRef]
- Simine, L.; Segal, D. Vibrational cooling, heating, and instability in molecular conducting junctions: Full counting statistics analysis. Phys. Chem. Chem. Phys. 2012, 14, 13820–13834. [Google Scholar] [CrossRef]
- Jiang, J.H. Enhancing efficiency and power of quantum-dots resonant tunneling thermoelectrics in three-terminal geometry by cooperative effects. J. Appl. Phys. 2014, 116, 194303. [Google Scholar] [CrossRef]
- Zhang, Y.; Lin, G.; Chen, J. Three-terminal quantum-dot refrigerators. Phys. Rev. E 2015, 91, 052118. [Google Scholar] [CrossRef]
- Schiró, M.; Le Hur, K. Tunable hybrid quantum electrodynamics from nonlinear electron transport. Phys. Rev. B 2014, 89, 195127. [Google Scholar] [CrossRef]
- Entin-Wohlman, O.; Jiang, J.H.; Imry, Y. Efficiency and dissipation in a two-terminal thermoelectric junction, emphasizing small dissipation. Phys. Rev. E 2014, 89, 012123. [Google Scholar] [CrossRef] [PubMed]
- Lu, J.; Wang, R.; Liu, Y.; Jiang, J.H. Thermoelectric cooperative effect in three-terminal elastic transport through a quantum dot. J. Appl. Phys. 2017, 122, 044301. [Google Scholar] [CrossRef]
- Wang, R.; Lu, J.; Wang, C.; Jiang, J.H. Nonlinear effects for three-terminal heat engine and refrigerator. Sci. Rep. 2018, 8, 2607. [Google Scholar] [CrossRef]
- Yamamoto, K.; Entin-Wohlman, O.; Aharony, A.; Hatano, N. Efficiency bounds on thermoelectric transport in magnetic fields: The role of inelastic processes. Phys. Rev. B 2016, 94, 121402. [Google Scholar] [CrossRef]
- Ludovico, M.F.; Capone, M. Enhanced performance of a quantum-dot-based nanomotor due to Coulomb interactions. Phys. Rev. B 2018, 98, 235409. [Google Scholar] [CrossRef]
- Erdman, P.A.; Mazza, F.; Bosisio, R.; Benenti, G.; Fazio, R.; Taddei, F. Thermoelectric properties of an interacting quantum dot based heat engine. Phys. Rev. B 2017, 95, 245432. [Google Scholar] [CrossRef]
- Jiang, J.H.; Imry, Y. Near-field three-terminal thermoelectric heat engine. Phys. Rev. B 2018, 97, 125422. [Google Scholar] [CrossRef]
- Ma, Y.H.; Zhai, R.X.; Chen, J.; Sun, C.P.; Dong, H. Experimental Test of the 1/τ-Scaling Entropy Generation in Finite-Time Thermodynamics. Phys. Rev. Lett. 2020, 125, 210601. [Google Scholar] [CrossRef]
- Cao, X.; Wang, C.; Zheng, H.; He, D. Quantum thermal transport via a canonically transformed Redfield approach. Phys. Rev. B 2021, 103, 075407. [Google Scholar] [CrossRef]
- Xi, M.; Wang, R.; Lu, J.; Jiang, J.H. Coulomb Thermoelectric Drag in Four-Terminal Mesoscopic Quantum Transport. Chin. Phys. Lett. 2021, 38, 088801. [Google Scholar] [CrossRef]
- Tu, Z.C. Abstract models for heat engines. Front. Phys. 2021, 16, 1–12. [Google Scholar] [CrossRef]
- McConnell, C.; Nazir, A. Strong coupling in thermoelectric nanojunctions: A reaction coordinate framework. New J. Phys. 2022, 24, 025002. [Google Scholar] [CrossRef]
- Zhai, R.X.; Cui, F.M.; Ma, Y.H.; Sun, C.P.; Dong, H. Experimental implementation of finite-time Carnot cycle. arXiv 2022, arXiv:2206.10153. [Google Scholar]
- Lu, J.; Wang, Z.; Wang, R.; Peng, J.; Wang, C.; Jiang, J.H. Multitask quantum thermal machines and cooperative effects. Phys. Rev. B 2023, 107, 075428. [Google Scholar] [CrossRef]
- Jiang, J.H.; Entin-Wohlman, O.; Imry, Y. Thermoelectric three-terminal hopping transport through one-dimensional nanosystems. Phys. Rev. B 2012, 85, 075412. [Google Scholar] [CrossRef]
- Jiang, J.H.; Imry, Y. Enhancing Thermoelectric Performance Using Nonlinear Transport Effects. Phys. Rev. Applied 2017, 7, 064001. [Google Scholar] [CrossRef]
- Lu, J.; Wang, R.; Wang, C.; Jiang, J.H. Brownian thermal transistors and refrigerators in mesoscopic systems. Phys. Rev. B 2020, 102, 125405. [Google Scholar] [CrossRef]
- Murphy, P.; Mukerjee, S.; Moore, J. Optimal thermoelectric figure of merit of a molecular junction. Phys. Rev. B 2008, 78, 161406. [Google Scholar] [CrossRef]
- Wysokiński, K.I. Thermal transport of molecular junctions in the pair tunneling regime. Phys. Rev. B 2010, 82, 115423. [Google Scholar] [CrossRef]
- Liu, J.; Sun, Q.f.; Xie, X.C. Enhancement of the thermoelectric figure of merit in a quantum dot due to the Coulomb blockade effect. Phys. Rev. B 2010, 81, 245323. [Google Scholar] [CrossRef]
- Costi, T.A.; Zlatić, V. Thermoelectric transport through strongly correlated quantum dots. Phys. Rev. B 2010, 81, 235127. [Google Scholar] [CrossRef]
- Jordan, A.N.; Sothmann, B.; Sánchez, R.; Büttiker, M. Powerful and efficient energy harvester with resonant-tunneling quantum dots. Phys. Rev. B 2013, 87, 075312. [Google Scholar] [CrossRef]
- Jiang, J.H.; Entin-Wohlman, O.; Imry, Y. Hopping thermoelectric transport in finite systems: Boundary effects. Phys. Rev. B 2013, 87, 205420. [Google Scholar] [CrossRef]
- Jiang, J.H.; Entin-Wohlman, O.; Imry, Y. Three-terminal semiconductor junction thermoelectric devices: Improving performance. New J. Phys. 2013, 15, 075021. [Google Scholar] [CrossRef]
- Sánchez, R.; Sothmann, B.; Jordan, A.N. Chiral Thermoelectrics with Quantum Hall Edge States. Phys. Rev. Lett. 2015, 114, 146801. [Google Scholar] [CrossRef]
- Whitney, R.S. Quantum Coherent Three-Terminal Thermoelectrics: Maximum Efficiency at Given Power Output. Entropy 2016, 18, 208. [Google Scholar] [CrossRef]
- Lu, J.; Liu, Y.; Wang, R.; Wang, C.; Jiang, J.H. Optimal efficiency and power, and their trade-off in three-terminal quantum thermoelectric engines with two output electric currents. Phys. Rev. B 2019, 100, 115438. [Google Scholar] [CrossRef]
- Wang, R.; Lu, J.; Jiang, J.H. Enhancing Thermophotovoltaic Performance Using Graphene-BN-InSb Near-Field Heterostructures. Phys. Rev. Appl. 2019, 12, 044038. [Google Scholar] [CrossRef]
- Lu, J.C.; Zhuo, F.J.; Sun, Z.Z.; Jiang, J.H. Cooperative Spin Caloritronic Devices. ES Energy. Environ. 2019, 7, 17–28. [Google Scholar] [CrossRef]
- Liu, Y.; Lu, J.; Wang, R.; Wang, C.; Jiang, J.H. Energy cooperation in quantum thermoelectric systems withmultiple electric currents. Chin. Phys. B 2020, 29, 40504. [Google Scholar] [CrossRef]
- Wang, L.; Wang, Z.; Wang, C.; Ren, J. Cycle Flux Ranking of Network Analysis in Quantum Thermal Devices. Phys. Rev. Lett. 2022, 128, 067701. [Google Scholar] [CrossRef]
- Lu, J.; Jiang, J.H.; Imry, Y. Unconventional four-terminal thermoelectric transport due to inelastic transport: Cooling by transverse heat current, transverse thermoelectric effect, and Maxwell demon. Phys. Rev. B 2021, 103, 085429. [Google Scholar] [CrossRef]
- Jiang, J.H.; Wu, S. Spin susceptibility and helical magnetic order at the edges/surfaces of topological insulators due to Fermi surface nesting. Phys. Rev. B 2011, 83, 205124. [Google Scholar] [CrossRef]
- Haug, H.; Jauho, A.P. Quantum Kinetics in Transport and Optics of Semiconductors; Springer: Heidelberg/Berlin, Germany, 2008. [Google Scholar]
- Yu, Z.Z.; Xiong, G.H.; Zhang, L.F. A brief review of thermal transport in mesoscopic systems from nonequilibrium Green’s function approach. Front. Phys. 2021, 16, 1–18. [Google Scholar] [CrossRef]
- Kulkarni, M.; Öztop, B.; Türeci, H.E. Cavity-Mediated Near-Critical Dissipative Dynamics of a Driven Condensate. Phys. Rev. Lett. 2013, 111, 220408. [Google Scholar] [CrossRef]
- Delbecq, M.R.; Schmitt, V.; Parmentier, F.D.; Roch, N.; Viennot, J.J.; Fève, G.; Huard, B.; Mora, C.; Cottet, A.; Kontos, T. Coupling a Quantum Dot, Fermionic Leads, and a Microwave Cavity on a Chip. Phys. Rev. Lett. 2011, 107, 256804. [Google Scholar] [CrossRef]
- Orgiu, E.; George, J.; Hutchison, J.A.; Devaux, E.; Dayen, J.F.; Doudin, B.; Stellacci, F.; Genet, C.; Schachenmayer, J.; Genes, C. Conductivity in organic semiconductors hybridized with the vacuum field. Nat. Mater. 2015, 14, 1123–1129. [Google Scholar] [CrossRef]
- Bruhat, L.E.; Viennot, J.J.; Dartiailh, M.C.; Desjardins, M.M.; Kontos, T.; Cottet, A. Cavity Photons as a Probe for Charge Relaxation Resistance and Photon Emission in a Quantum Dot Coupled to Normal and Superconducting Continua. Phys. Rev. X 2016, 6, 021014. [Google Scholar] [CrossRef]
- Jaliel, G.; Puddy, R.K.; Sánchez, R.; Jordan, A.N.; Sothmann, B.; Farrer, I.; Griffiths, J.P.; Ritchie, D.A.; Smith, C.G. Experimental Realization of a Quantum Dot Energy Harvester. Phys. Rev. Lett. 2019, 123, 117701. [Google Scholar] [CrossRef] [PubMed]
- Ren, J.; Zhu, J.X.; Gubernatis, J.E.; Wang, C.; Li, B. Thermoelectric transport with electron-phonon coupling and electron-electron interaction in molecular junctions. Phys. Rev. B 2012, 85, 155443. [Google Scholar] [CrossRef]
- Entin-Wohlman, O.; Imry, Y.; Aharony, A. Three-terminal thermoelectric transport through a molecular junction. Phys. Rev. B 2010, 82, 115314. [Google Scholar] [CrossRef]
- Hubbard, J.; Flowers, B.H. Electron correlations in narrow energy bands. Proc. Math. Phys. Eng. Sci. 1963, 276, 238–257. [Google Scholar] [CrossRef]
- Lu, J.; Wang, R.; Ren, J.; Kulkarni, M.; Jiang, J.H. Quantum-dot circuit-QED thermoelectric diodes and transistors. Phys. Rev. B 2019, 99, 035129. [Google Scholar] [CrossRef]
- Datta, S. Electronic Transport in Mesoscopic Systems; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Simine, L.; Segal, D. Path-integral simulations with fermionic and bosonic reservoirs: Transport and dissipation in molecular electronic junctions. J. Chem. Phys. 2013, 138, 214111. [Google Scholar] [CrossRef]
- Martínez-Pérez, M.J.; Fornieri, A.; Giazotto, F. Rectification of electronic heat current by a hybrid thermal diode. Nat. Nanotechnol. 2015, 10, 303. [Google Scholar] [CrossRef]
- Ren, J.; Zhu, J.X. Heat diode effect and negative differential thermal conductance across nanoscale metal-dielectric interfaces. Phys. Rev. B 2013, 87, 241412. [Google Scholar] [CrossRef]
- Jiang, J.H.; Kulkarni, M.; Segal, D.; Imry, Y. Phonon thermoelectric transistors and rectifiers. Phys. Rev. B 2015, 92, 045309. [Google Scholar] [CrossRef]
- Bergenfeldt, C.; Samuelsson, P.; Sothmann, B.; Flindt, C.; Büttiker, M. Hybrid Microwave-Cavity Heat Engine. Phys. Rev. Lett. 2014, 112, 076803. [Google Scholar] [CrossRef]
- Sánchez, R.; Sothmann, B.; Jordan, A.N. Heat diode and engine based on quantum Hall edge states. New J. Phys. 2015, 17, 075006. [Google Scholar] [CrossRef]
- Thierschmann, H.; Arnold, F.; Mittermüller, M.; Maier, L.; Heyn, C.; Hansen, W.; Buhmann, H.; Molenkamp, L.W. Thermal gating of charge currents with Coulomb coupled quantum dots. New J. Phys. 2015, 17, 113003. [Google Scholar] [CrossRef]
- Marcos-Vicioso, A.; López-Jurado, C.; Ruiz-Garcia, M.; Sánchez, R. Thermal rectification with interacting electronic channels: Exploiting degeneracy, quantum superpositions, and interference. Phys. Rev. B 2018, 98, 035414. [Google Scholar] [CrossRef]
- Tesser, L.; Bhandari, B.; Erdman, P.A.; Paladino, E.; Fazio, R.; Taddei, F. Heat rectification through single and coupled quantum dots. New J. Phys. 2022, 24, 035001. [Google Scholar] [CrossRef]
- Ren, J.; Zhu, J.X. Theory of asymmetric and negative differential magnon tunneling under temperature bias: Towards a spin Seebeck diode and transistor. Phys. Rev. B 2013, 88, 094427. [Google Scholar] [CrossRef]
- Jiang, J.H. Thermodynamic bounds and general properties of optimal efficiency and power in linear responses. Phys. Rev. E 2014, 90, 042126. [Google Scholar] [CrossRef]
- Joulain, K.; Drevillon, J.; Ezzahri, Y.; Ordonez-Miranda, J. Quantum Thermal Transistor. Phys. Rev. Lett. 2016, 116, 200601. [Google Scholar] [CrossRef]
- Sánchez, R.; Thierschmann, H.; Molenkamp, L.W. All-thermal transistor based on stochastic switching. Phys. Rev. B 2017, 95, 241401. [Google Scholar] [CrossRef]
- Wang, C.; Chen, X.M.; Sun, K.W.; Ren, J. Heat amplification and negative differential thermal conductance in a strongly coupled nonequilibrium spin-boson system. Phys. Rev. A 2018, 97, 052112. [Google Scholar] [CrossRef]
- Wang, C.; Xu, D.; Liu, H.; Gao, X. Thermal rectification and heat amplification in a nonequilibrium V-type three-level system. Phys. Rev. E 2019, 99, 042102. [Google Scholar] [CrossRef]
- Liu, H.; Wang, C.; Wang, L.Q.; Ren, J. Strong system-bath coupling induces negative differential thermal conductance and heat amplification in nonequilibrium two-qubit systems. Phys. Rev. E 2019, 99, 032114. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.Q.; Yu, D.H.; Yu, C.S. Common Environmental Effects on Quantum Thermal Transistor. Entropy 2022, 24, 32. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Wang, L.; Chen, J.; Wang, C.; Ren, J. Geometric heat pump: Controlling thermal transport with time-dependent modulations. Front. Phys. 2022, 17, 1–14. [Google Scholar] [CrossRef]
- Ren, J.; Hänggi, P.; Li, B. Berry-Phase-Induced Heat Pumping and Its Impact on the Fluctuation Theorem. Phys. Rev. Lett. 2010, 104, 170601. [Google Scholar] [CrossRef] [PubMed]
- Terrén Alonso, P.; Abiuso, P.; Perarnau-Llobet, M.; Arrachea, L. Geometric Optimization of Nonequilibrium Adiabatic Thermal Machines and Implementation in a Qubit System. PRX Quantum 2022, 3, 010326. [Google Scholar] [CrossRef]
- Lu, J.; Wang, Z.; Peng, J.; Wang, C.; Jiang, J.H.; Ren, J. Geometric thermodynamic uncertainty relation in a periodically driven thermoelectric heat engine. Phys. Rev. B 2022, 105, 115428. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, J.; Wang, R.; Wang, C.; Jiang, J.-H. Thermoelectric Rectification and Amplification in Interacting Quantum-Dot Circuit-Quantum-Electrodynamics Systems. Entropy 2023, 25, 498. https://doi.org/10.3390/e25030498
Lu J, Wang R, Wang C, Jiang J-H. Thermoelectric Rectification and Amplification in Interacting Quantum-Dot Circuit-Quantum-Electrodynamics Systems. Entropy. 2023; 25(3):498. https://doi.org/10.3390/e25030498
Chicago/Turabian StyleLu, Jincheng, Rongqian Wang, Chen Wang, and Jian-Hua Jiang. 2023. "Thermoelectric Rectification and Amplification in Interacting Quantum-Dot Circuit-Quantum-Electrodynamics Systems" Entropy 25, no. 3: 498. https://doi.org/10.3390/e25030498
APA StyleLu, J., Wang, R., Wang, C., & Jiang, J.-H. (2023). Thermoelectric Rectification and Amplification in Interacting Quantum-Dot Circuit-Quantum-Electrodynamics Systems. Entropy, 25(3), 498. https://doi.org/10.3390/e25030498





