Quantum Machine Learning for Distributed Quantum Protocols with Local Operations and Noisy Classical Communications
Abstract
1. Introduction
1.1. Motivation
1.2. Entanglement Distillation
1.3. Quantum State Discrimination
1.4. Main Contributions
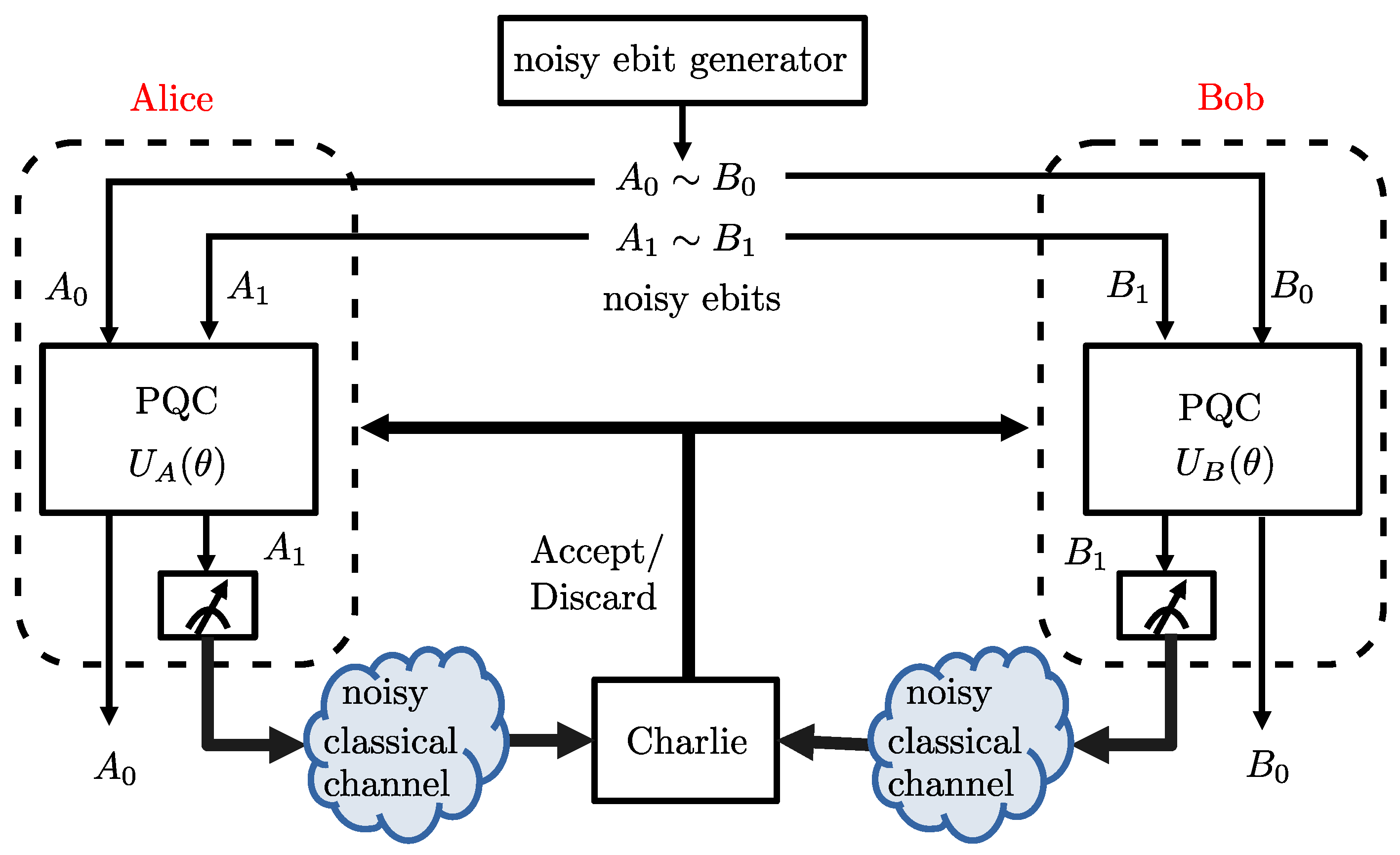
- As observed in Figure 1, we first introduce NA-LOCCNet as a novel PQC-based architecture for the distributed entanglement distillation (see Figure 4) that is designed with the goal of maximizing the average fidelity while accounting for the randomness caused by communication errors.
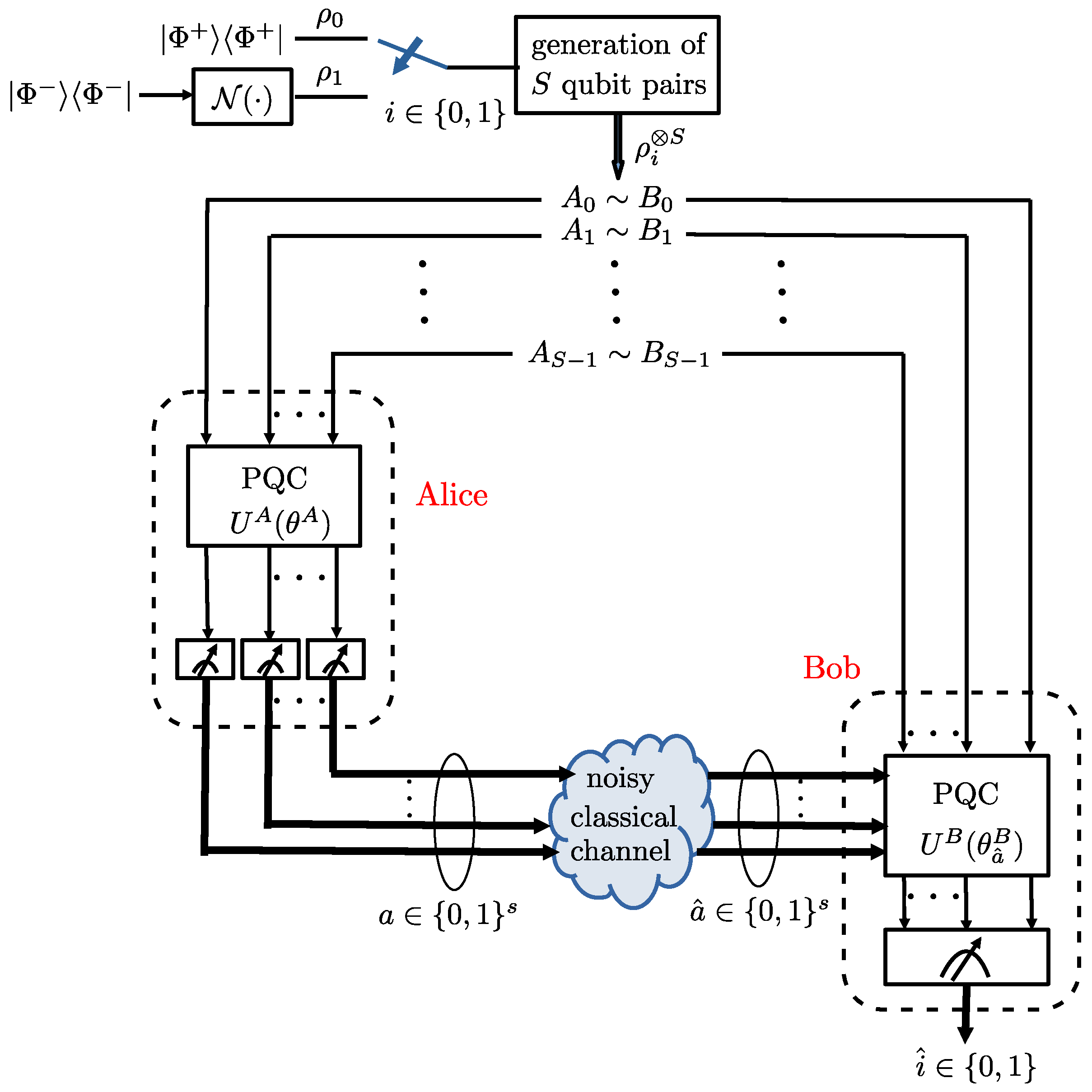
- Then, we adapt the NA-LOCCNet framework for the problem of the distributed quantum state discrimination (see Figure 9), with the goal of maximizing the average probability of successful detection for quantum state discrimination.
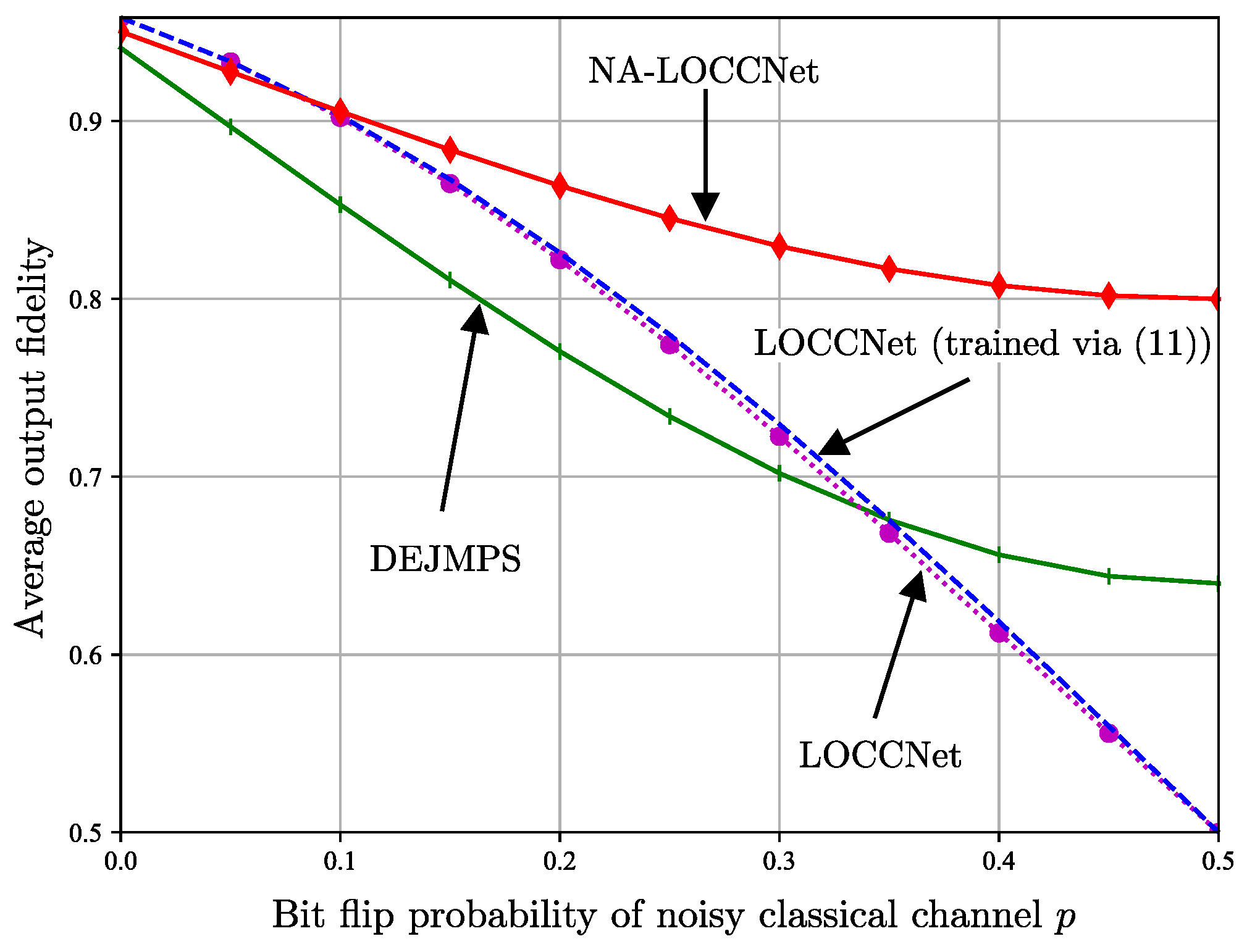
- The introduced NA-LOCCNet is shown via experiments to have significant advantages over existing protocols designed for noiseless communications. Furthermore, in quantum state discrimination, we make the important observation that, depending on the level of classical noise, a larger level of entanglement-breaking noise can be advantageous to facilitate successful distributed discrimination.
1.5. Organization
1.6. Notations and Definitions
2. Learning Entanglement Distillation with Noisy Classical Communication
2.1. Problem Formulation
2.1.1. Setting
2.1.2. Performance Metrics
2.2. Existing Distillation Protocols
2.2.1. DEJMPS Protocol
2.2.2. LOCCNet
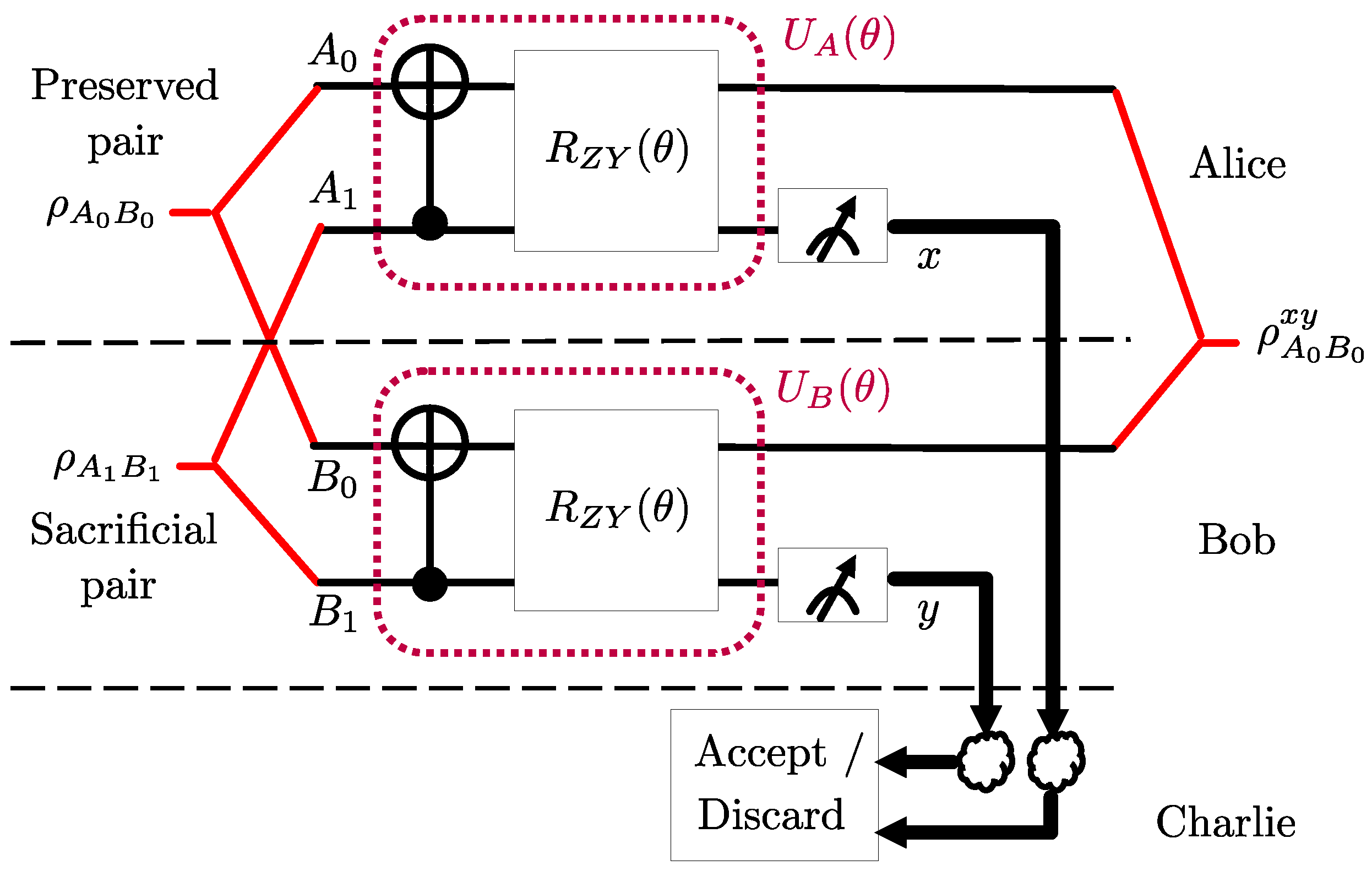
2.3. Noise Aware-LOCCNet
2.3.1. Design Objective
2.3.2. Architecture of the PQCs
2.3.3. Optimization
2.4. Experiments
3. Learning Quantum State Discrimination with Noisy Classical Communication
3.1. Setting and Performance Metrics
3.1.1. Setting
3.1.2. Performance Metrics
Helstrom Bound
Positive Partial Transpose (PPT) Bound
3.2. LOCCNet
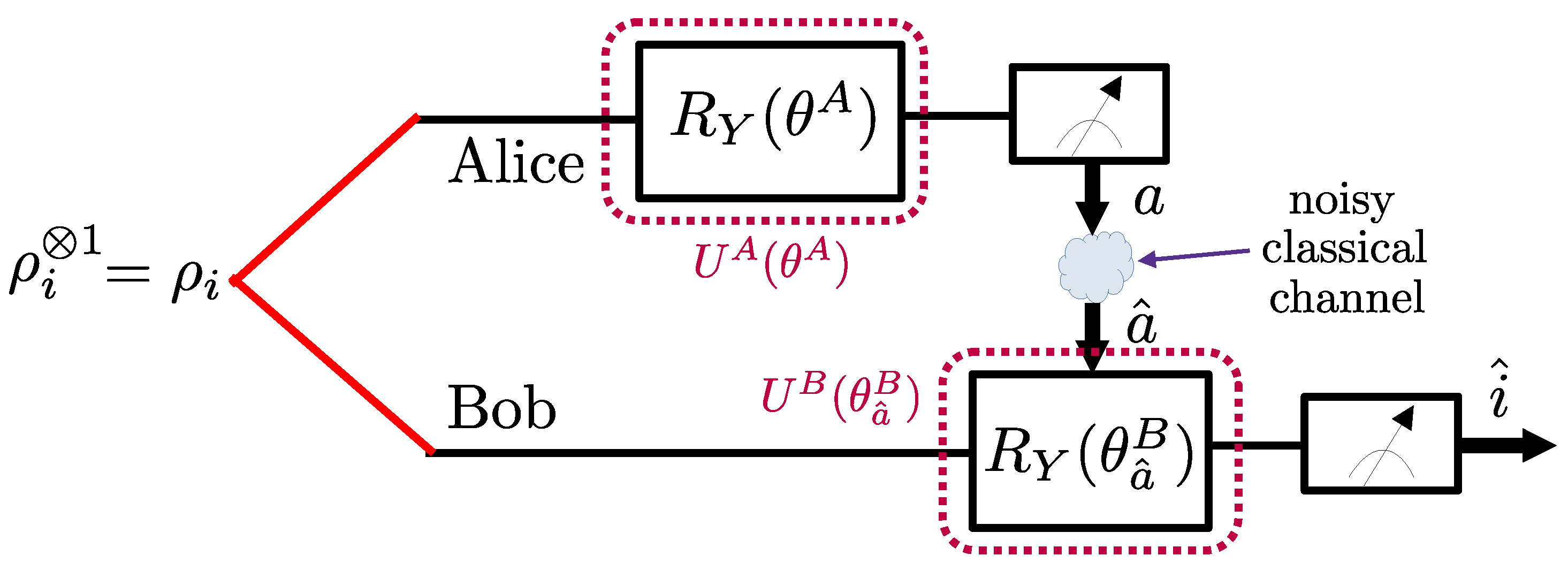
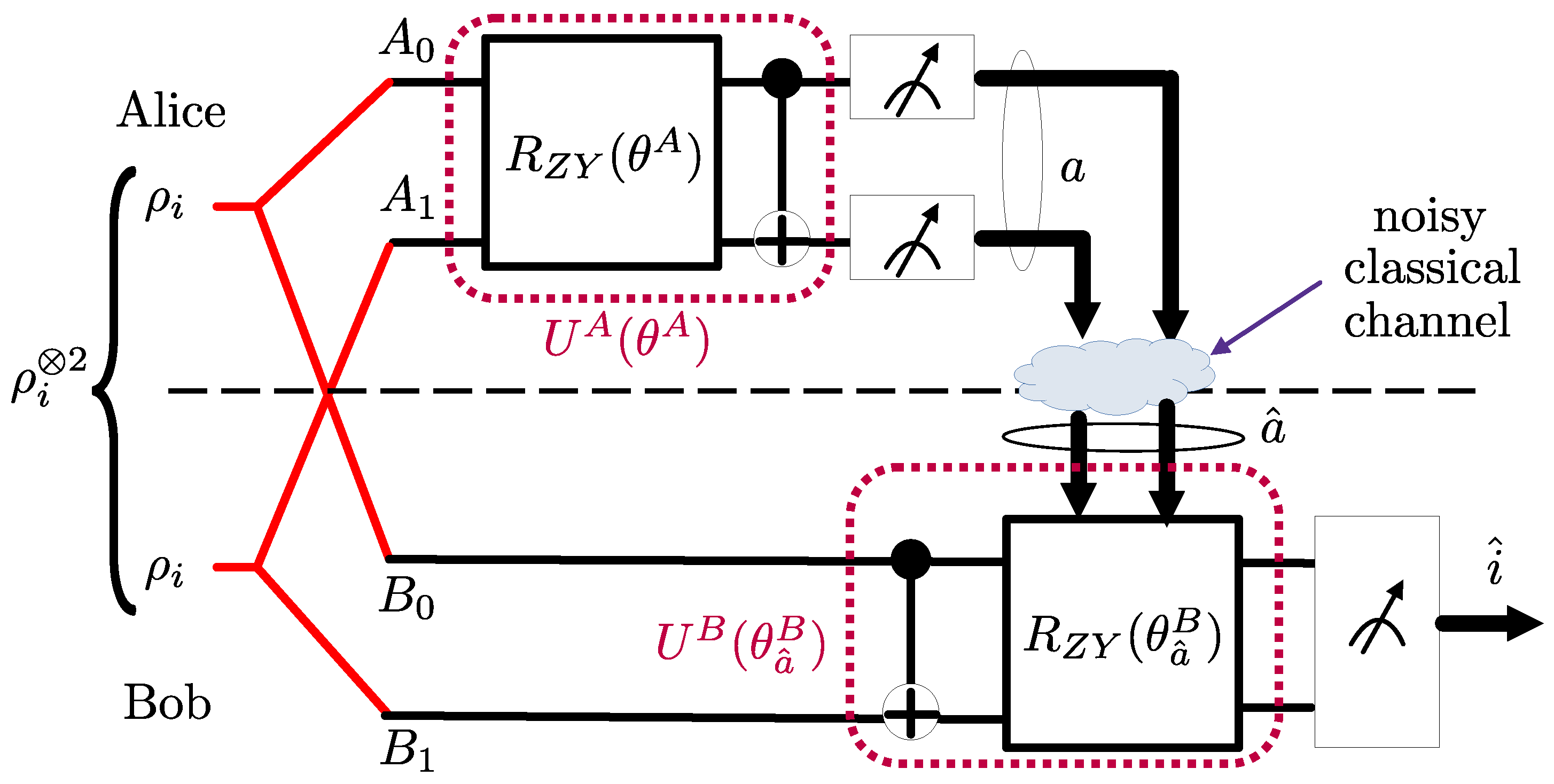
3.3. Noise Aware-LOCCNet
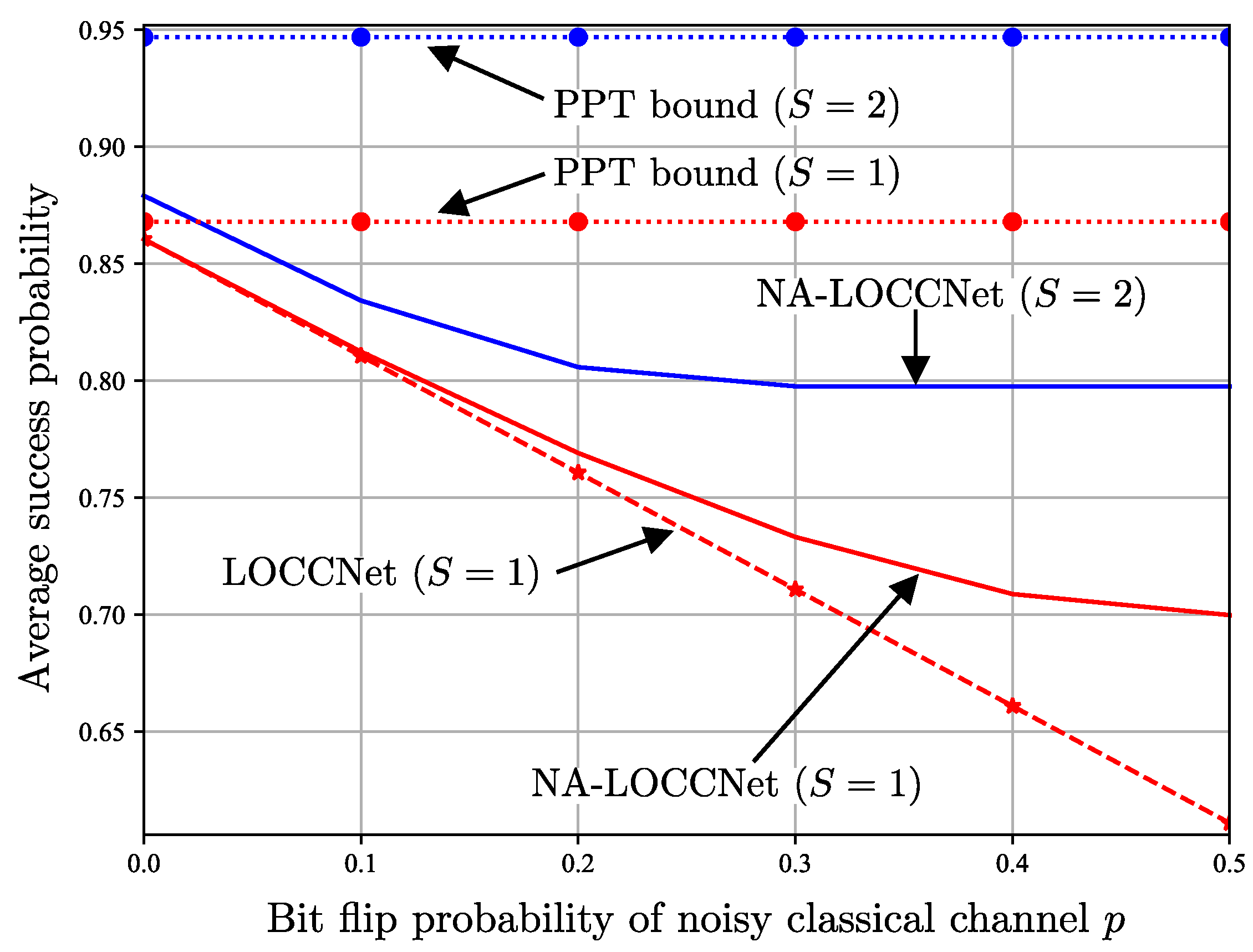
3.4. Experiments
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cacciapuoti, A.S.; Caleffi, M.; Tafuri, F.; Cataliotti, F.S.; Gherardini, S.; Bianchi, G. Quantum Internet: Networking Challenges in Distributed Quantum Computing. IEEE Netw. 2020, 34, 137–143. [Google Scholar] [CrossRef]
- Bennett, C.H.; Brassard, G.; Crépeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1993, 70, 1895–1899. [Google Scholar] [CrossRef] [PubMed]
- Chitambar, E.; Leung, D.; Mančinska, L.; Ozols, M.; Winter, A. Everything you always wanted to know about LOCC (but were afraid to ask). Commun. Math. Phys. 2014, 328, 303–326. [Google Scholar] [CrossRef]
- Bennett, C.H.; Brassard, G.; Popescu, S.; Schumacher, B.; Smolin, J.A.; Wootters, W.K. Purification of Noisy Entanglement and Faithful Teleportation via Noisy Channels. Phys. Rev. Lett. 1996, 76, 722–725. [Google Scholar] [CrossRef] [PubMed]
- Walgate, J.; Short, A.J.; Hardy, L.; Vedral, V. Local Distinguishability of Multipartite Orthogonal Quantum States. Phys. Rev. Lett. 2000, 85, 4972–4975. [Google Scholar] [CrossRef]
- Pirandola, S.; Laurenza, R.; Ottaviani, C.; Banchi, L. Fundamental limits of repeaterless quantum communications. Nat. Commun. 2017, 8, 15043. [Google Scholar] [CrossRef]
- Cope, T.P.W.; Hetzel, L.; Banchi, L.; Pirandola, S. Simulation of non-Pauli channels. Phys. Rev. A 2017, 96, 022323. [Google Scholar] [CrossRef]
- Zhao, X.; Zhao, B.; Wang, Z.; Song, Z.; Wang, X. Practical distributed quantum information processing with LOCCNet. NPJ Quantum Inf. 2021, 7, 159. [Google Scholar] [CrossRef]
- Schuld, M.; Petruccione, F. Machine Learning with Quantum Computers; Springer: Cham, Switzerland, 2021. [Google Scholar] [CrossRef]
- Simeone, O. An Introduction to Quantum Machine Learning for Engineers. Found. Trends Signal Process. 2022, 16, 1–223. [Google Scholar] [CrossRef]
- Rohde, P. The Quantum Internet: The Second Quantum Revolution; Cambridge University Press: Cambridge, UK, 2021. [Google Scholar]
- Van Meter, R. Quantum Networking; Wiley-IEEE Press: London, UK, 2014. [Google Scholar]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar] [CrossRef]
- Wilde, M.M. Quantum Information Theory; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar] [CrossRef]
- Eisaman, M.D.; Fan, J.; Migdall, A.; Polyakov, S.V. Invited review article: Single-photon sources and detectors. Rev. Sci. Instrum. 2011, 82, 071101. [Google Scholar] [CrossRef]
- Campbell, E.T.; Benjamin, S.C. Measurement-Based Entanglement under Conditions of Extreme Photon Loss. Phys. Rev. Lett. 2008, 101, 130502. [Google Scholar] [CrossRef] [PubMed]
- Deutsch, D.; Ekert, A.; Jozsa, R.; Macchiavello, C.; Popescu, S.; Sanpera, A. Quantum Privacy Amplification and the Security of Quantum Cryptography over Noisy Channels. Phys. Rev. Lett. 1996, 77, 2818–2821. [Google Scholar] [CrossRef] [PubMed]
- Bae, J.; Kwek, L.C. Quantum state discrimination and its applications. J. Phys. Math. Theor. 2015, 48, 083001. [Google Scholar] [CrossRef]
- Lloyd, S. Enhanced Sensitivity of Photodetection via Quantum Illumination. Science 2008, 321, 1463–1465. [Google Scholar] [CrossRef]
- Bennett, C.H. Quantum cryptography using any two nonorthogonal states. Phys. Rev. Lett. 1992, 68, 3121–3124. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Zhuang, Q. Distributed quantum sensing. Quantum Sci. Technol. 2021, 6, 043001. [Google Scholar] [CrossRef]
- Ghosh, S.; Kar, G.; Roy, A.; Sarkar, D. Distinguishability of maximally entangled states. Phys. Rev. A 2004, 70, 022304. [Google Scholar] [CrossRef]
- Chittoor, H.H.S.; Simeone, O. Learning Quantum Entanglement Distillation with Noisy Classical Communications. arXiv 2022, arXiv:2205.08561. [Google Scholar]
- Rozpedek, F.; Schiet, T.; Thinh, L.P.; Elkouss, D.; Doherty, A.C.; Wehner, S. Optimizing practical entanglement distillation. Phys. Rev. A 2018, 97, 062333. [Google Scholar] [CrossRef]
- Nickerson, N.H.; Fitzsimons, J.F.; Benjamin, S.C. Freely Scalable Quantum Technologies Using Cells of 5-to-50 Qubits with Very Lossy and Noisy Photonic Links. Phys. Rev. X 2014, 4, 041041. [Google Scholar] [CrossRef]
- Dür, W.; Briegel, H.J. Entanglement purification and quantum error correction. Rep. Prog. Phys. 2007, 70, 1381–1424. [Google Scholar] [CrossRef]
- You, J.B.; Koh, D.E.; Kong, J.F.; Ding, W.J.; Png, C.E.; Wu, L. Exploring variational quantum eigensolver ansatzes for the long-range XY model. arXiv 2021, arXiv:2109.00288. [Google Scholar]
- Kingma, D.P.; Ba, J. Adam: A method for stochastic optimization. arXiv 2014, arXiv:1412.6980. [Google Scholar]
- Benedetti, M.; Lloyd, E.; Sack, S.; Fiorentini, M. Parameterized quantum circuits as machine learning models. Quantum Sci. Technol. 2019, 4, 043001. [Google Scholar] [CrossRef]
- Kusyk, J.; Saeed, S.M.; Uyar, M.U. Survey on Quantum Circuit Compilation for Noisy Intermediate-Scale Quantum Computers: Artificial Intelligence to Heuristics. IEEE Trans. Quantum Eng. 2021, 2, 1–16. [Google Scholar] [CrossRef]
- Huggins, W.J.; McClean, J.R.; Rubin, N.C.; Jiang, Z.; Wiebe, N.; Whaley, K.B.; Babbush, R. Efficient and noise resilient measurements for quantum chemistry on near-term quantum computers. NPJ Quantum Inf. 2021, 7, 1–9. [Google Scholar] [CrossRef]
- Sharma, K.; Khatri, S.; Cerezo, M.; Coles, P.J. Noise resilience of variational quantum compiling. New J. Phys. 2020, 22, 043006. [Google Scholar] [CrossRef]
- Helstrom, C.W. Quantum detection and estimation theory. J. Stat. Phys. 1969, 1, 231–252. [Google Scholar] [CrossRef]
- Holevo, A. Statistical decision theory for quantum systems. J. Multivar. Anal. 1973, 3, 337–394. [Google Scholar] [CrossRef]
- Yu, N.; Duan, R.; Ying, M. Distinguishability of Quantum States by Positive Operator-Valued Measures With Positive Partial Transpose. IEEE Trans. Inf. Theory 2014, 60, 2069–2079. [Google Scholar] [CrossRef]
- Cosentino, A. Positive-partial-transpose-indistinguishable states via semidefinite programming. Phys. Rev. A 2013, 87, 012321. [Google Scholar] [CrossRef]
- Matthews, W.; Winter, A. Pure-state transformations and catalysis under operations that completely preserve positivity of partial transpose. Phys. Rev. A 2008, 78, 012317. [Google Scholar] [CrossRef]
- Illiano, J.; Caleffi, M.; Manzalini, A.; Cacciapuoti, A.S. Quantum Internet protocol stack: A comprehensive survey. Comput. Netw. 2022, 213, 109092. [Google Scholar] [CrossRef]
- Endo, S.; Benjamin, S.C.; Li, Y. Practical Quantum Error Mitigation for Near-Future Applications. Phys. Rev. X 2018, 8, 031027. [Google Scholar] [CrossRef]
- Steane, A.M. Error Correcting Codes in Quantum Theory. Phys. Rev. Lett. 1996, 77, 793–797. [Google Scholar] [CrossRef]
- Wood, C.J. Special Session: Noise Characterization and Error Mitigation in Near-Term Quantum Computers. In Proceedings of the 2020 IEEE 38th International Conference on Computer Design (ICCD), Hartford, CT, USA, 18–21 October 2020; pp. 13–16. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chittoor, H.H.S.; Simeone, O. Quantum Machine Learning for Distributed Quantum Protocols with Local Operations and Noisy Classical Communications. Entropy 2023, 25, 352. https://doi.org/10.3390/e25020352
Chittoor HHS, Simeone O. Quantum Machine Learning for Distributed Quantum Protocols with Local Operations and Noisy Classical Communications. Entropy. 2023; 25(2):352. https://doi.org/10.3390/e25020352
Chicago/Turabian StyleChittoor, Hari Hara Suthan, and Osvaldo Simeone. 2023. "Quantum Machine Learning for Distributed Quantum Protocols with Local Operations and Noisy Classical Communications" Entropy 25, no. 2: 352. https://doi.org/10.3390/e25020352
APA StyleChittoor, H. H. S., & Simeone, O. (2023). Quantum Machine Learning for Distributed Quantum Protocols with Local Operations and Noisy Classical Communications. Entropy, 25(2), 352. https://doi.org/10.3390/e25020352





