Fisher and Shannon Functionals for Hyperbolic Diffusion
Abstract
1. Introduction
- The wave limit
- The diffusion limit
2. Hyperbolic Diffusion
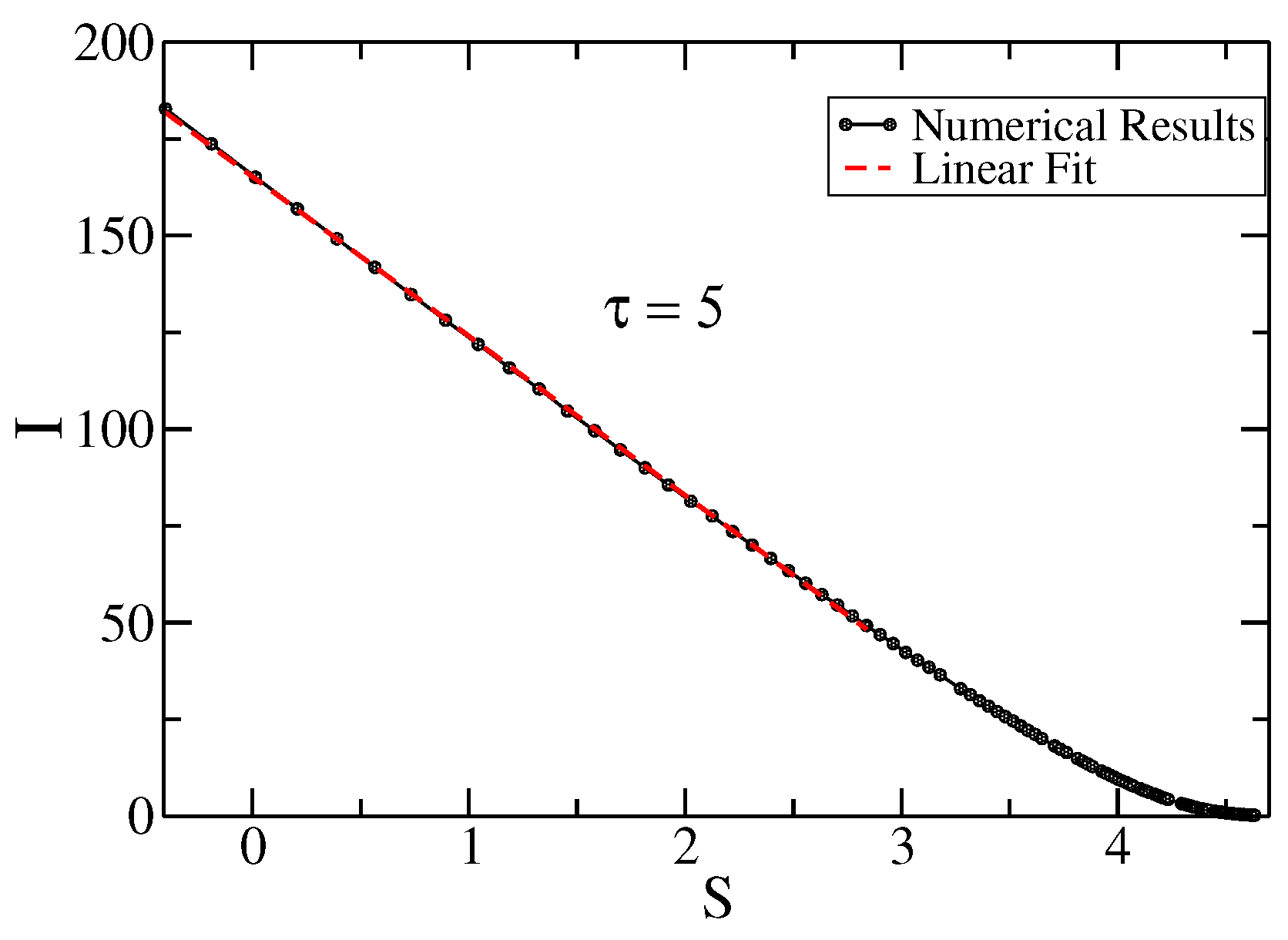
3. Fisher and Shannon Functionals
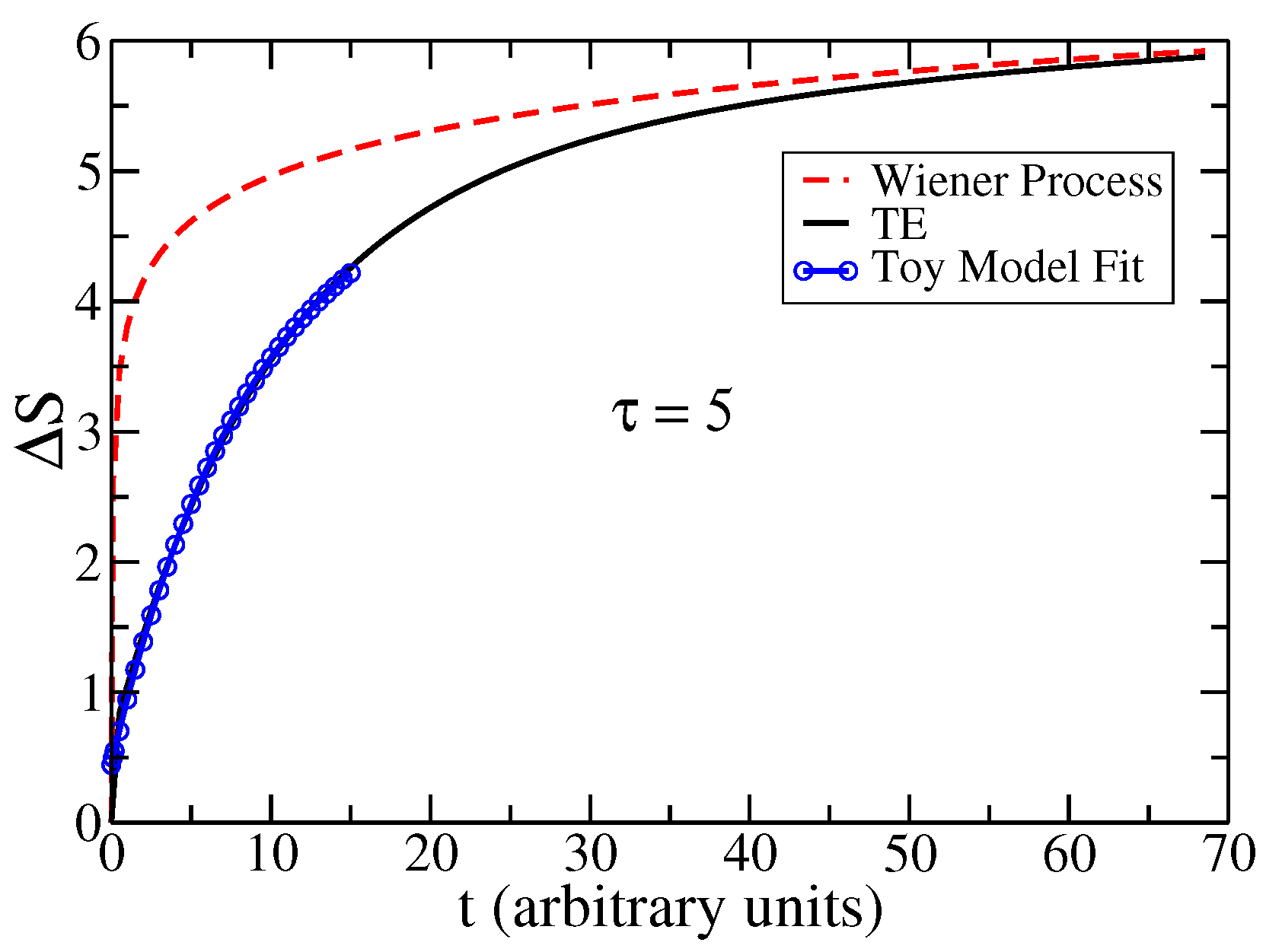
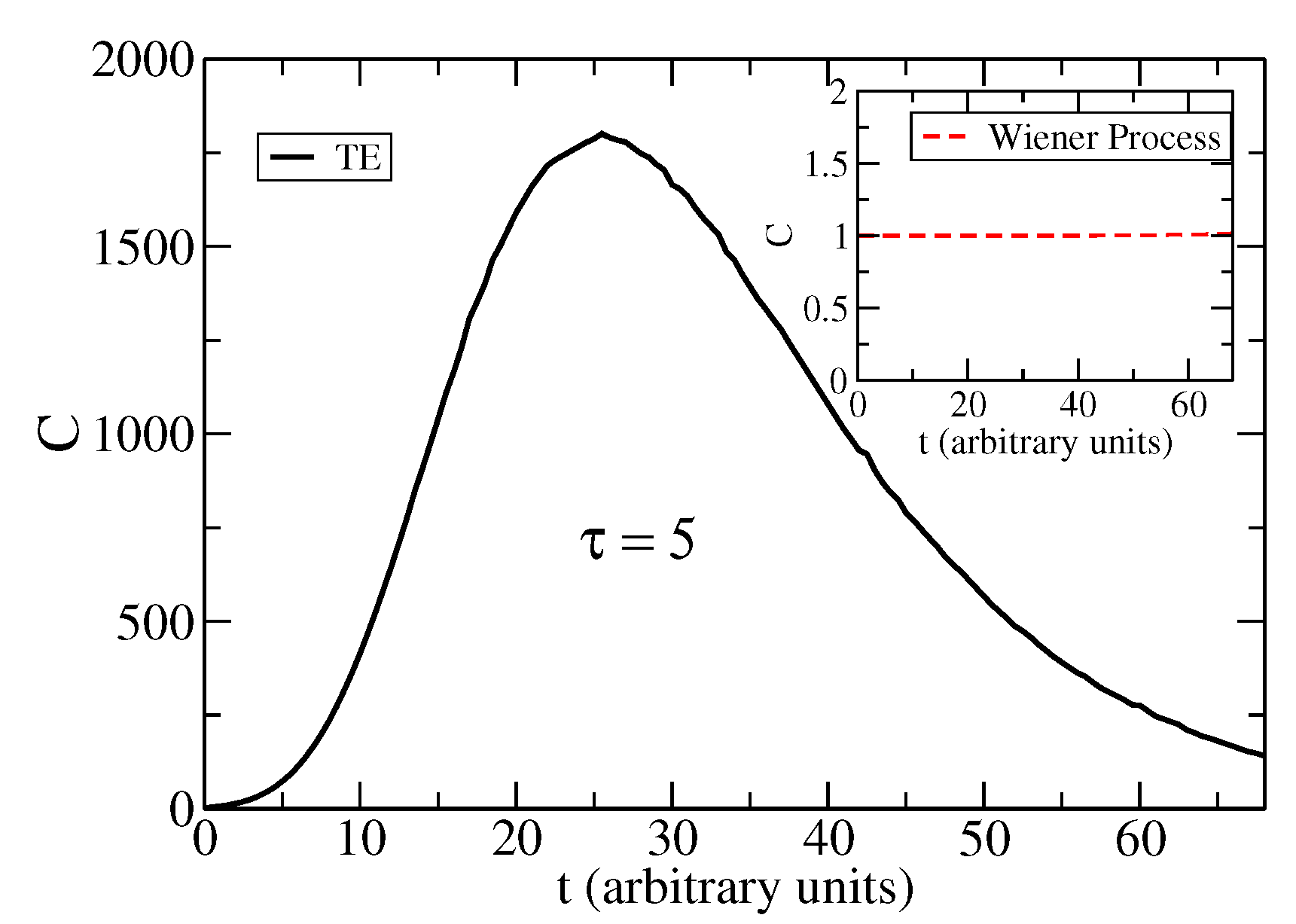
3.1. Wiener Diffusion Case
3.2. Hyperbolic Diffusion Case
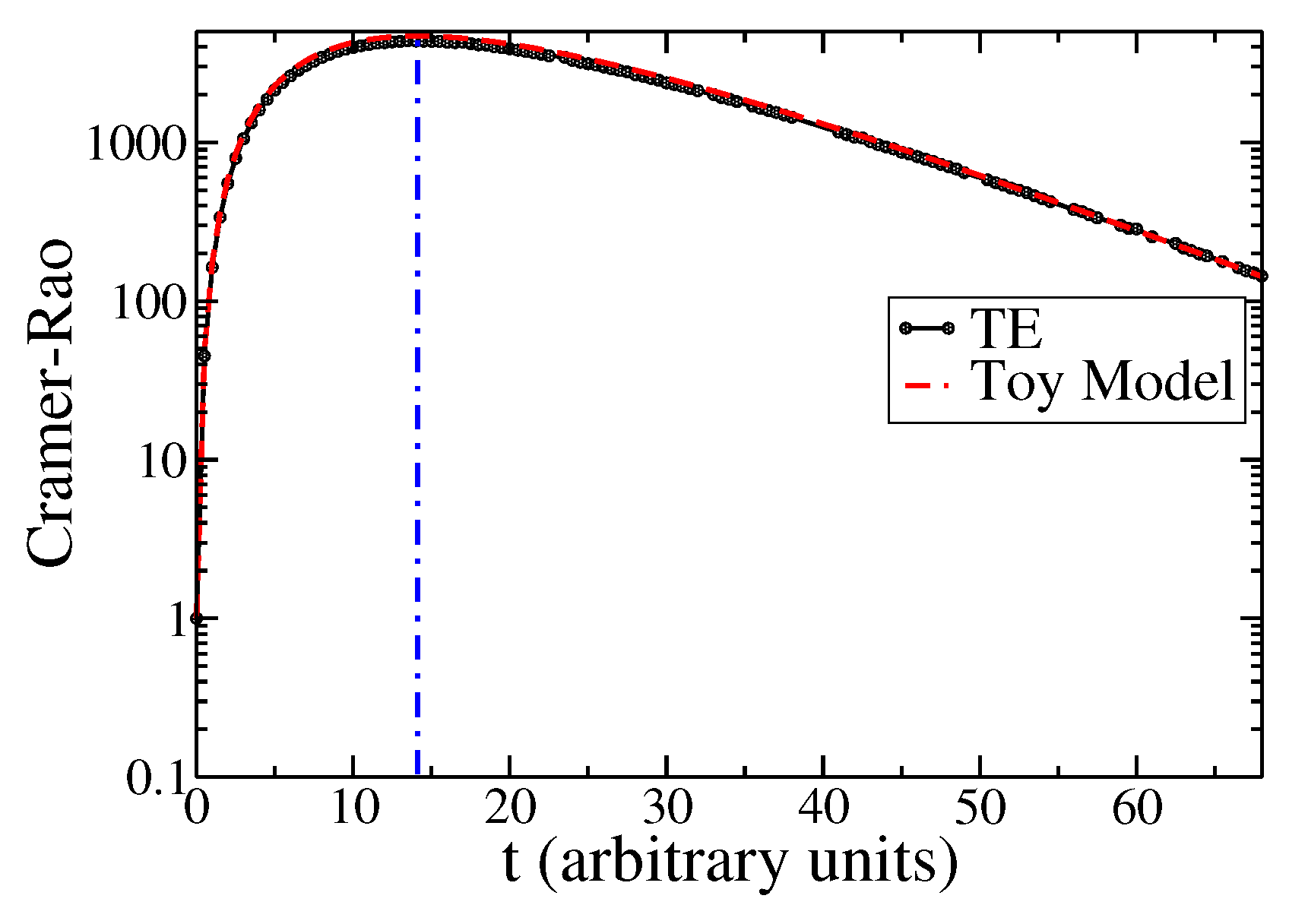
3.3. Estimation Theory
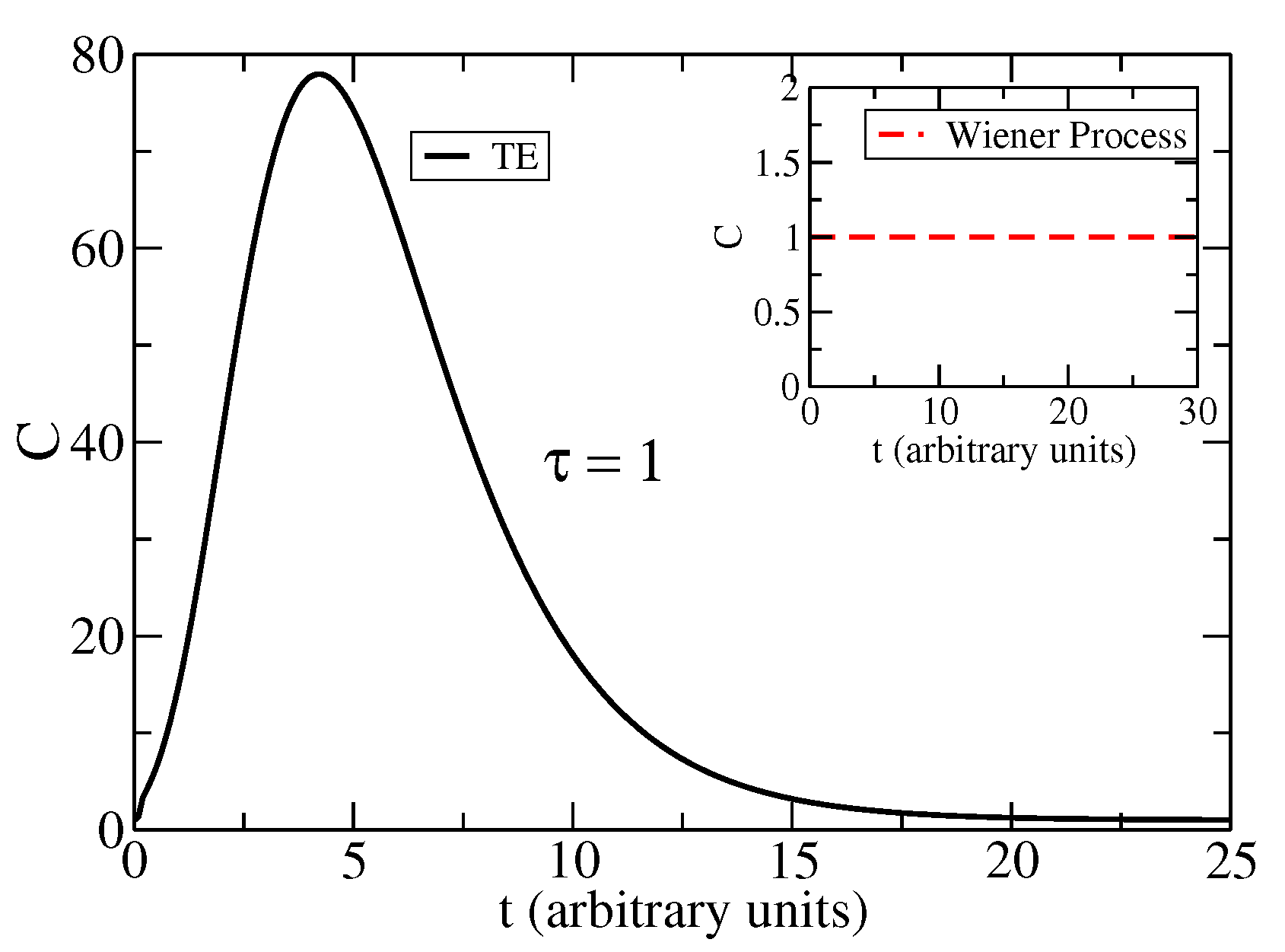
4. Numerical Results for the Telegrapher’s Equation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Solution to the Telegrapher’s Equation
Appendix A.1. The Toy Model for τ ≫ 1
Appendix A.2. The Exact Solution in Real Space-Time
Appendix A.3. Convergence to the Gaussian Diffusion for t/τ ≫ 1
Appendix B. On Non-Local Fisher’s Information ITE(t,t′)
Appendix B.1. Relative Entropy from the Toy Model
Appendix C. Perturbation for Shannon’s Entropy of the Telegrapher’s Equation
References
- Pearson, J.M. A Theory of Waves; Allyn and Bacon, Inc.: Boston, MA, USA, 1966; Chapter 1.7. [Google Scholar]
- Landau, L.D.; Bell, J.S.; Kearsley, M.J. Electrodynamics of Continuous Media; Elsevier Science: Amsterdam, The Netherlands, 2013; ISBN 9781483293752. Chapter 71. [Google Scholar]
- Heaviside, O. Electrical Papers of Oliver Heaviside; Chelsea: New York, NY, USA, 1970; Volume 1, p. 307. [Google Scholar]
- Nagy, G.B.; Ortiz, O.E.; Reula, O.A. The behavior of hyperbolic heat equations’ solutions near their parabolic limits. J. Math. Phys. 1994, 35, 4334. [Google Scholar] [CrossRef][Green Version]
- Compte, A.; Metzlerz, R. The generalized Cattaneo equation for the description of anomalous transport processes. J. Phys. A Math. Gen. 1997, 30, 7277–7289. [Google Scholar] [CrossRef]
- Górska, K.; Horzela, A.; Lenzi, E.K.; Pagnini, G.; Sandev, T. Generalized Cattaneo (telegrapher’s) equations in modeling anomalous diffusion phenomena. Phys. Rev. E 2020, 102, 022128. [Google Scholar] [CrossRef]
- Bear, M.F.; Connors, B.W.; Paradiso, M.A. Neuroscience: Exploring the Brain, 4th ed.; Wolters Kluwer: New York, NY, USA, 2016. [Google Scholar]
- Pettersen, K.H.; Einevoll, G.T. Neurophysics: What the telegrapher’s equation has taught us about the brain. In An Anthology of Developments in Clinical Engineering and Bioimpedance: Festschrift for Sverre Grimnes; Martinsen, ø., Jensen, ø., Eds.; Unipub Forlag: Oslo, Norway, 2009. [Google Scholar]
- Graaff, R.; Hoenders, B.J. Telegrapher’s Equation for Light Transport in Tissue with Substantial Absorption. In Proceedings of the Biomedical Optics 2008, St. Petersburg, FL, USA, 16–19 March 2008. [Google Scholar] [CrossRef]
- Shlepnev, Y. Coupled 2D telegrapher’s equations for PDN analysis. In Proceedings of the 2012 IEEE 21st Conference on Electrical Performance of Electronic Packaging and Systems, Tempe, AZ, USA, 21–24 October 2012; pp. 171–174. [Google Scholar] [CrossRef]
- Nizama, M.; Caceres, M.O. Penetration of waves in global stochastic conducting media. Phys. Rev. E 2023, 107, 054107. [Google Scholar] [CrossRef]
- Heizler, S.I. Asymptotic telegrapher’s equation (P1) approximation for the transport equation. Nucl. Sci. Eng. 2010, 166, 17–35. [Google Scholar] [CrossRef]
- Cáceres, M.O.; Wio, H.S. Non-Markovian diffusion-like equation for transport processes with anisotropic scattering. Physica A 1987, 142, 563. [Google Scholar] [CrossRef]
- Cáceres, M.O.; Nizama, M. Stochastic telegrapher’s approach for solving the random Boltzmann-Lorentz gas. Phys. Rev. E 2022, 2022 105, 044131. [Google Scholar] [CrossRef]
- Ureña, F.; Gavete, L.; Benito, J.J.; García, A.; Vargas, A.M. Solving the telegraph equation in 2-D and 3-D using generalized finite difference method (GFDM). Eng. Anal. Boundary Elem. V. 2020, 112, 13–24. [Google Scholar] [CrossRef]
- Broadbridge, P.; Kolesnik, A.D.; Leonenko, N.; Olenko, A. Random spherical hyperbolic diffusion. J. Stat. Phys. 2019, 177, 889–916. [Google Scholar] [CrossRef]
- Broadbridge, P.; Kolesnik, A.D.; Leonenko, N.; Olenko, A.; Omari, A.D. Spherically restricted random hyperbolic diffusion. Entropy 2020, 22, 217. [Google Scholar] [CrossRef] [PubMed]
- Cáceres, M.O. Finite-velocity diffusion in random media. J. Stat. Phys. 2020, 179, 729–747. [Google Scholar] [CrossRef]
- Sandev, T.; Iomin, A. Finite-velocity diffusion on a comb. Europhys. Lett. 2018, 124, 20005. [Google Scholar] [CrossRef]
- Keller, J.B. Diffusion at finite speed and random walks. Proc. Nac. Acd. Sci. USA 2004, 101, 1120–1122. [Google Scholar] [CrossRef] [PubMed]
- Cáceres, M.O. Comments on wave-like propagation with binary disorder. J. Stat. Phys. 2021, 182, 36. [Google Scholar] [CrossRef]
- Cáceres, M.O. Surface gravity waves on randomly irregular floor and the telegrapher’s equation. AIP Adv. 2021, 11, 045218. [Google Scholar] [CrossRef]
- Marín, E.; Vaca-Oyola, L.S.; Delgado-Vasallo, O. On thermal waves’ velocity: Some open questions in thermal waves’ physics. Rev. Mex. Fis. E 2016, 62, 1–4. [Google Scholar]
- Marin, E. On thermal waves. Eur. J. Phys. 2013, 34, L83–L85. [Google Scholar] [CrossRef]
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Fisher, R.A. Statistical Methods and Scientific Inference, 2nd ed.; Oliver and Boyd: London, UK, 1959; Chapter IV. [Google Scholar]
- Szabó, J.B.; Sen, K.D.; Nagy, Á. The Fisher-Shannon information plane for atoms. Phys. Lett. A 2008, 372, 2428. [Google Scholar] [CrossRef]
- Dehesa, J.S.; López-Rosaa, S.; Manzano, D. Configuration complexities of hydrogenic atoms. Eur. Phys. J. D 2009, 55, 539. [Google Scholar] [CrossRef]
- Cáceres, M.O. Non-Equilibrium Statistical Physics with Application to Disordered Systems; Springer: Berlin, Germany, 2017; ISBN 978-3-319-51552-6. [Google Scholar]
- Cramér, H. Mathematical Methods of Statistics; Princeton University Press: Princeton, NJ, USA, 1946; ISBN 0-691-08004-6. Chapter 32. [Google Scholar]
- Rao, C.R.; Das Gupta, S. (Eds.) Selected Papers of C. R. Rao; Wiley: New York, NY, USA, 1994; ISBN 978-0-470-22091-7. [Google Scholar]
- Radaelli, M.; Landi, G.T.; Modi, K.; Binder, F.C. Fisher information of correlated stochastic processes. New J. Phys. 2023, 25, 053037. [Google Scholar] [CrossRef]
- Yoshida, N. Statistical inference for stochastic processes: Concepts and developments in asymptotic theory. In Stochastic Processes and Applications to Mathematical Finance, Proceedings of the March 3rd-7th 2004 Symposium at Ritsumeikan University BKC; Biwako Kusatsu Campus, Ritsumeikan University: Kyoto, Japan, 2004. [Google Scholar]
- Morse, P.M.; Feshbach, H. Methods of Theoretical Physics; McGraw-Hill Book Company, Inc.: New York, NY, USA, 1953; p. 865. [Google Scholar]
- Kac, M. A Stochastic model related to the telegraphers equation. Rocky Mt. J. Math. 1974, 4, 497. [Google Scholar] [CrossRef]
- Masoliver, J.; Weiss, G.H. Finite-velocity diffusion. Eur. J. Phys. 1996, 17, 190. [Google Scholar] [CrossRef]
- Sonnenschein, E. Wave packets and group velocity in absorbing media: Solutions of the telegrapher’s equation. Prog. Electromagn. Res. PIER 2000, 27, 129–158. [Google Scholar] [CrossRef]
- Spanier, J.; Oldham, K. An Atlas of Functions; Springer: Berlin, Germany, 1987. [Google Scholar]
- Marcinkiewicz, J. Sur une propriété de la loi de Gauβ. Math. Z. 1939, 44, 612. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cáceres, M.O.; Nizama, M.; Pennini, F. Fisher and Shannon Functionals for Hyperbolic Diffusion. Entropy 2023, 25, 1627. https://doi.org/10.3390/e25121627
Cáceres MO, Nizama M, Pennini F. Fisher and Shannon Functionals for Hyperbolic Diffusion. Entropy. 2023; 25(12):1627. https://doi.org/10.3390/e25121627
Chicago/Turabian StyleCáceres, Manuel O., Marco Nizama, and Flavia Pennini. 2023. "Fisher and Shannon Functionals for Hyperbolic Diffusion" Entropy 25, no. 12: 1627. https://doi.org/10.3390/e25121627
APA StyleCáceres, M. O., Nizama, M., & Pennini, F. (2023). Fisher and Shannon Functionals for Hyperbolic Diffusion. Entropy, 25(12), 1627. https://doi.org/10.3390/e25121627