Evaluation of Transport Properties and Energy Conversion of Bacterial Cellulose Membrane Using Peusner Network Thermodynamics
Abstract
1. Introduction
2. Materials and Methods
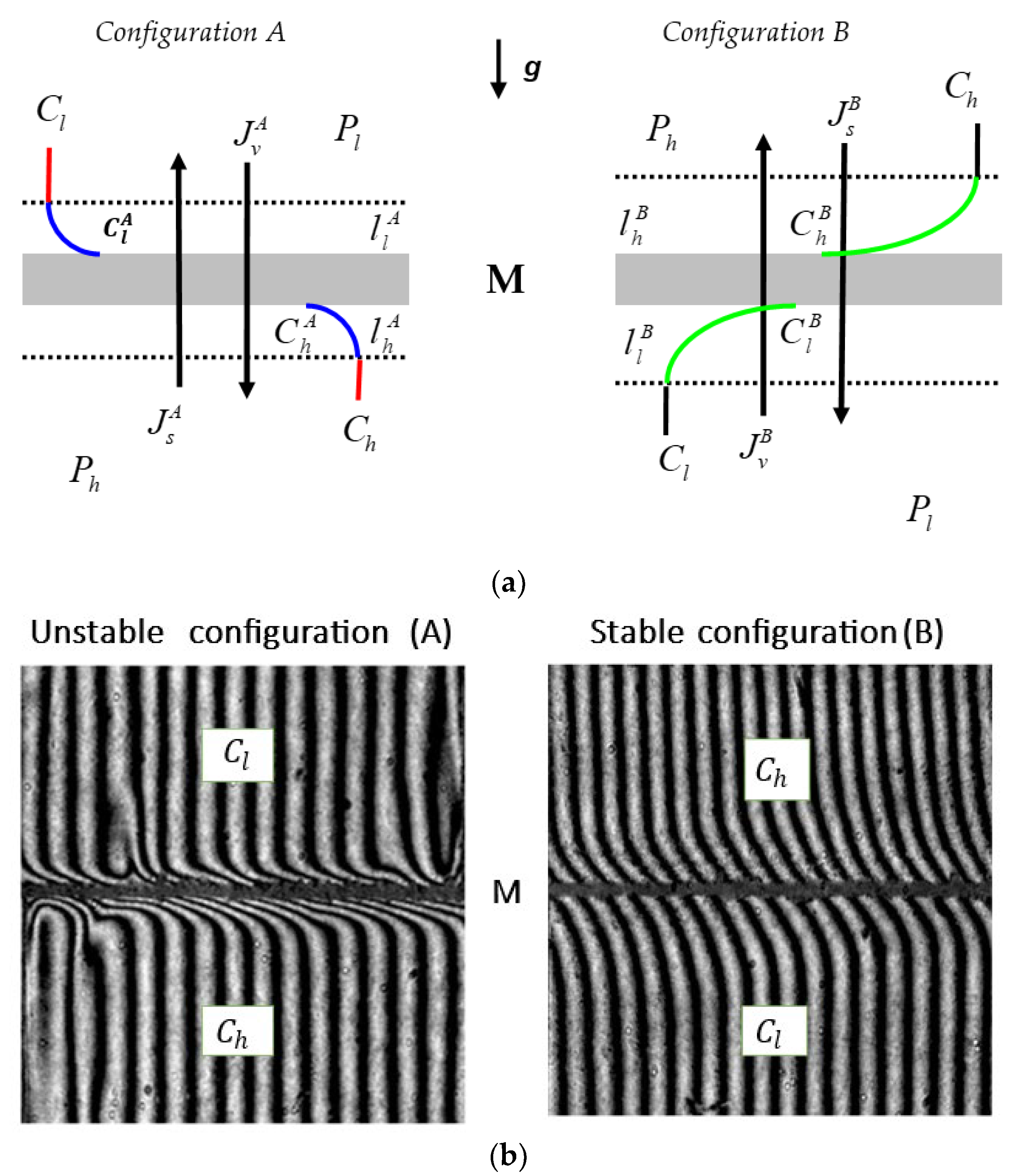
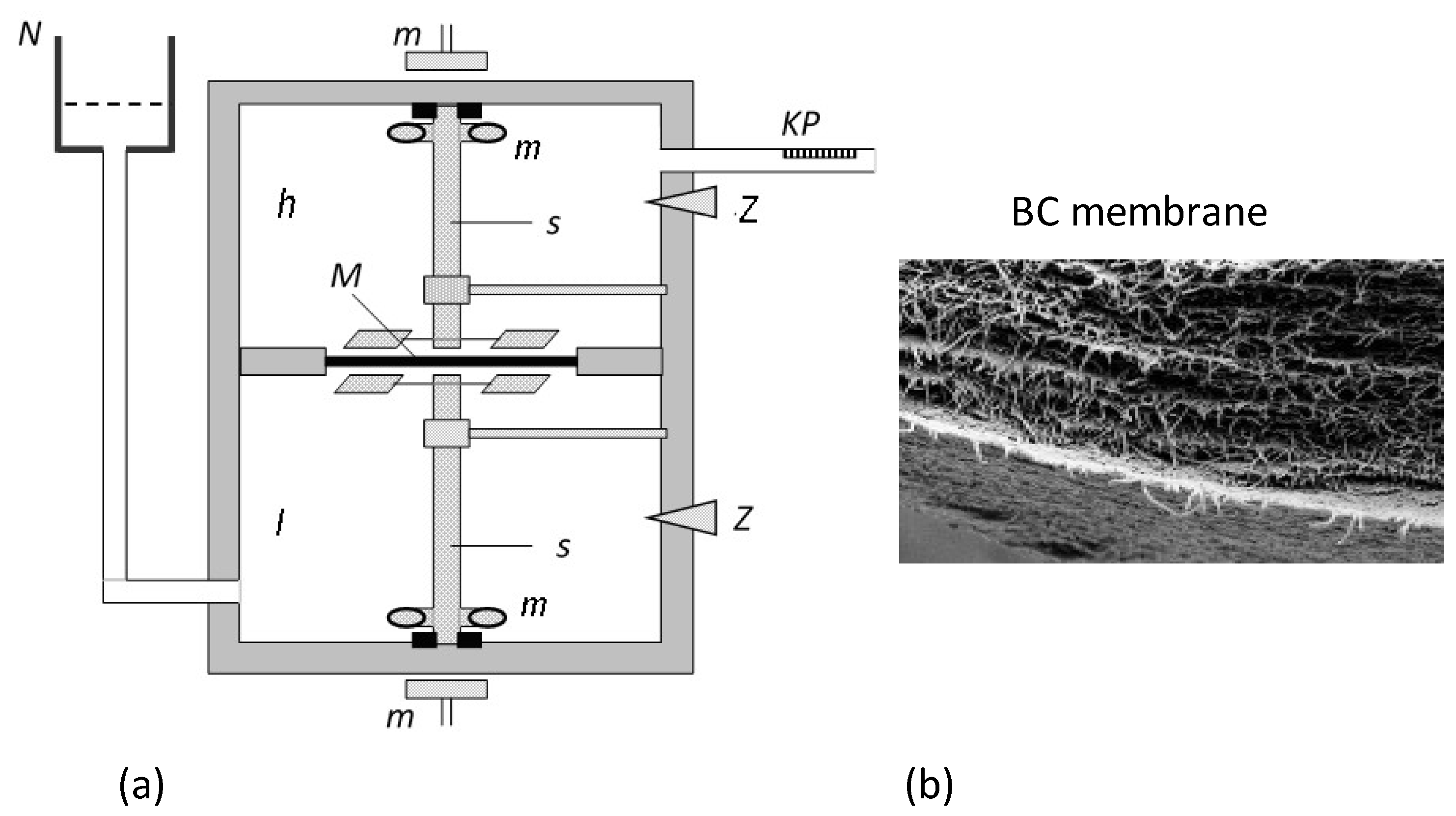
2.1. Membrane System
2.2. The Rr Form of Kedem–Katchalsky Equations for Binary Nonelectrolyte Solutions
3. Results and Discussion
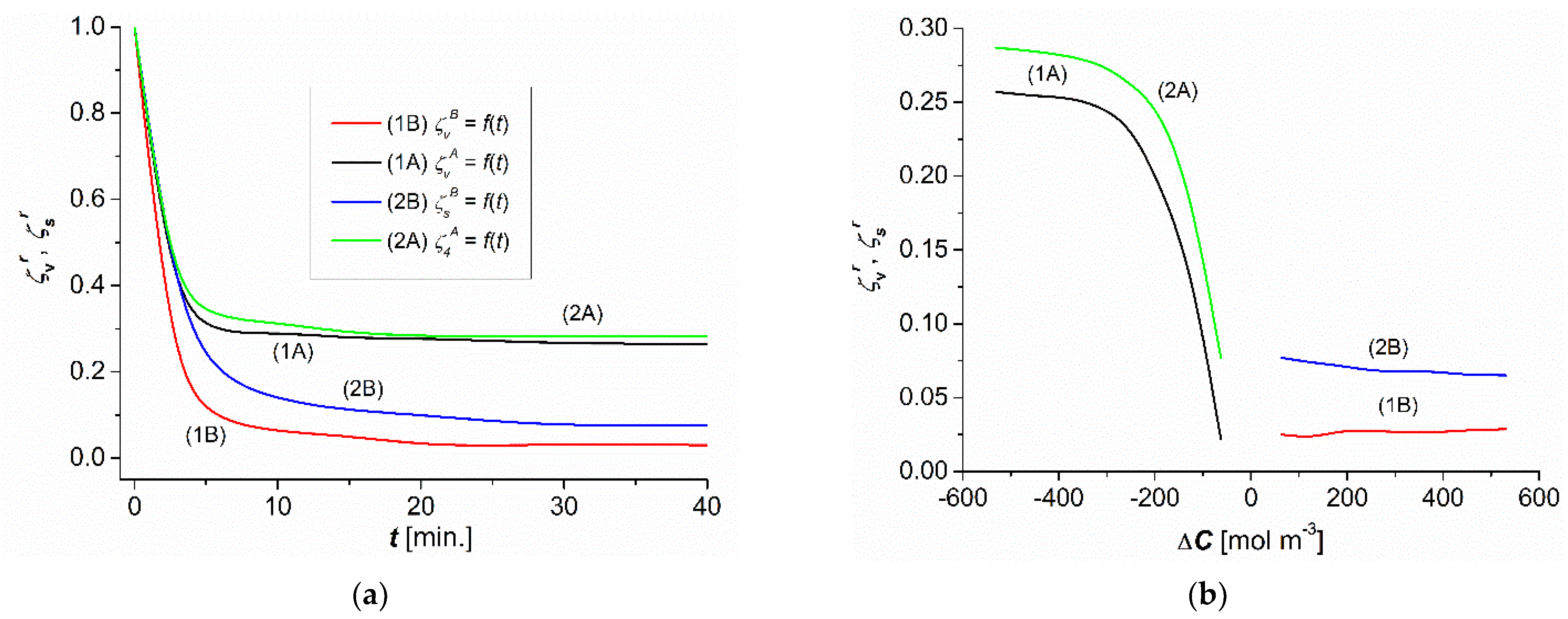
3.1. The Time and Concentration Dependencies of and
3.2. The Time and Concentration Dependencies of and
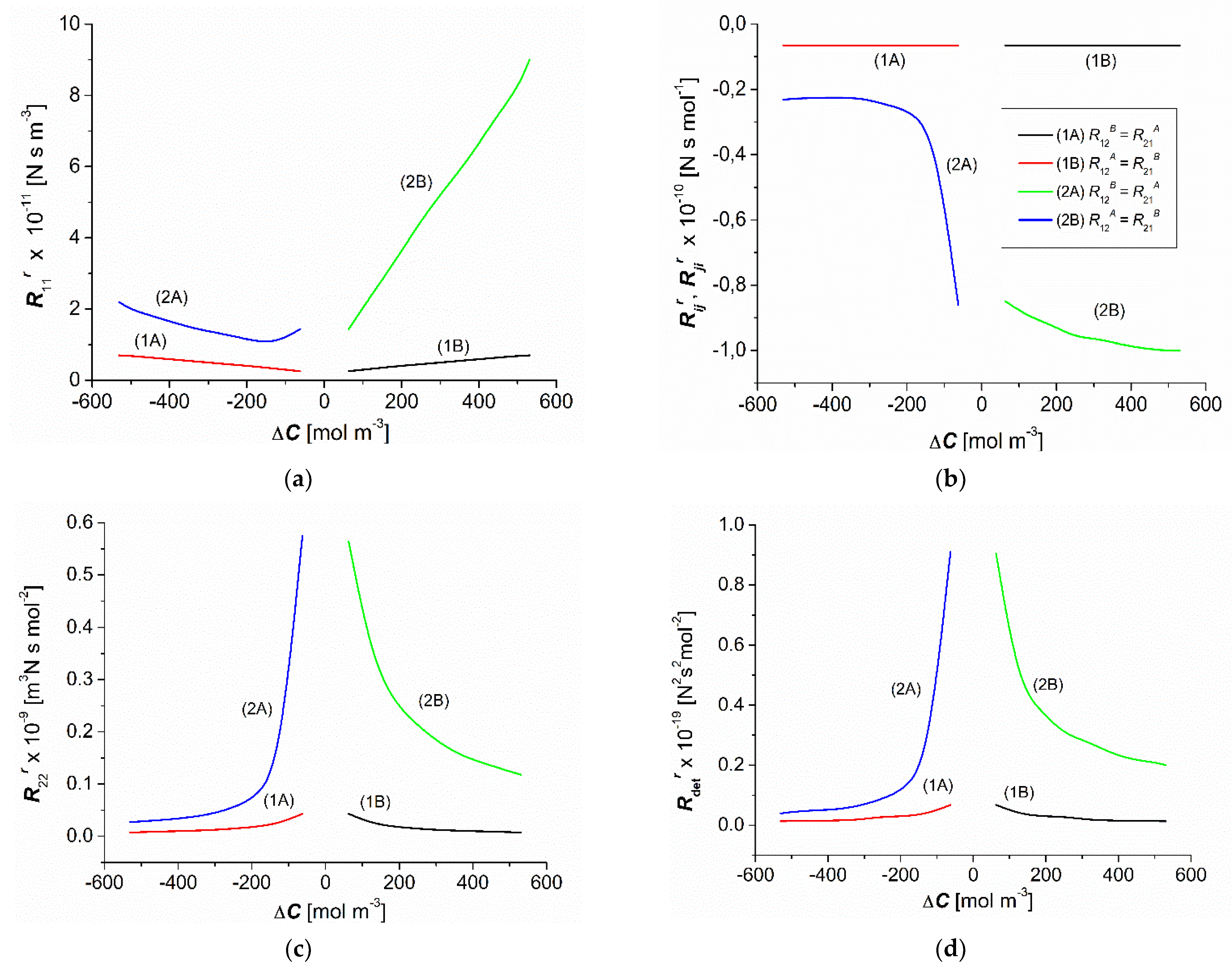
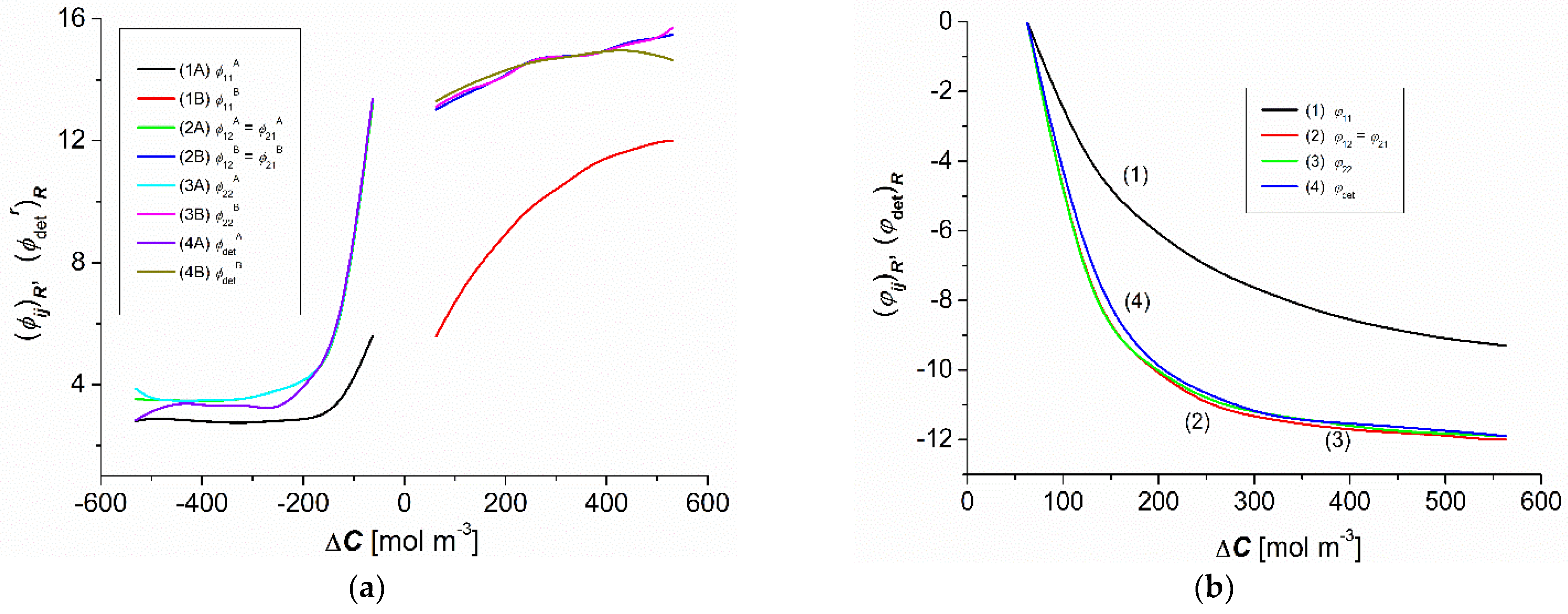
3.3. Concentration Dependencies of the Resistance Coefficients and
3.4. Concentration Dependencies and
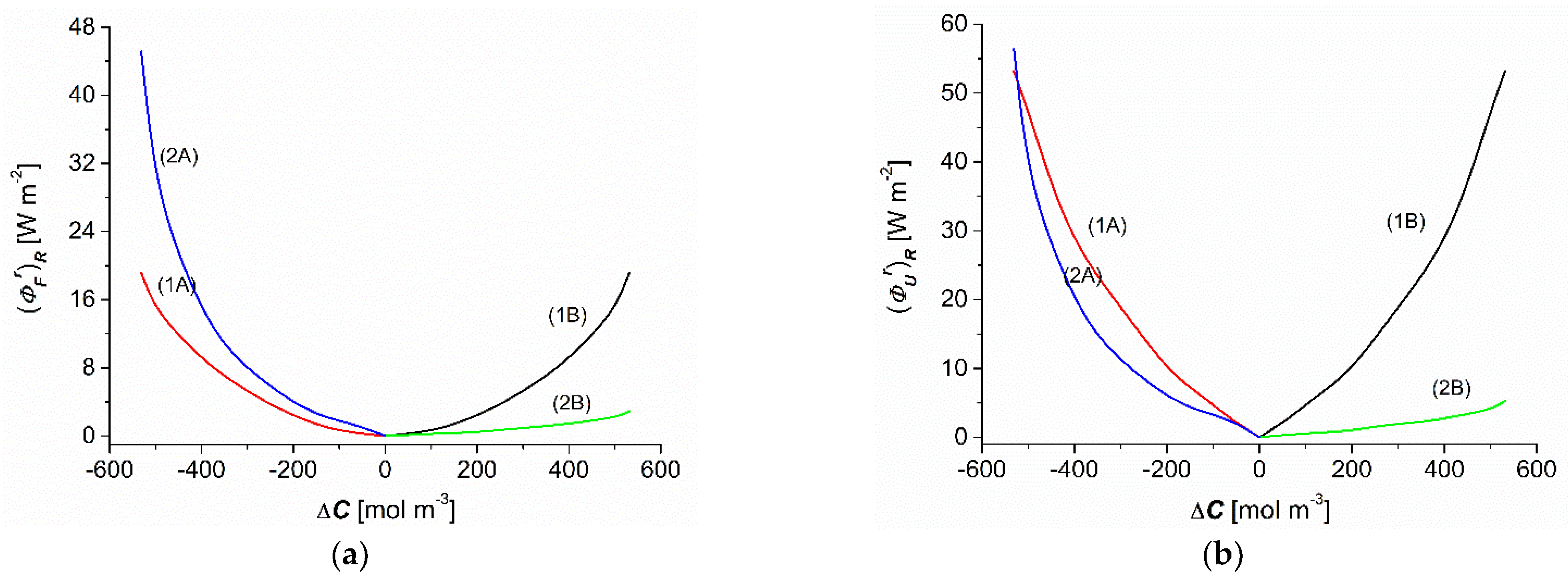
3.5. Concentration Dependencies of , , , and
4. Conclusions
- Developed within the framework of the Kedem–Katchalsky–Peusner formalism, the procedure using the Peusner coefficients (i = j ∈ {1, 2}, r = A, B) and is suitable for evaluating the transport properties of polymer membranes and assessing the conversion of internal energy (U-energy) to useful energy (F-energy) and degraded energy (S-energy).
- Peusner coefficients and are related to the membrane Peclét coefficients and .
- The procedure developed in this paper to evaluate the conversion of internal energy (U-energy) to useful energy (F-energy) and degraded energy (S-energy) requires the calculation of the value of the flux of S-energy and efficiency factors and , followed by the fluxes of F-energy and U-energy ().
- The procedure proposed in the paper can be applied to membranes for which the coefficients , , , and can be determined experimentally.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
List of Symbols
| hydraulic permeability coefficient (m3N−1s−1) | |
| average concentration of solutes (mol m−3) | |
| hydraulic, osmotic, diffusive, and advective coefficients of CP | |
| i, j ∈ {1, 2}, r = A, B | (N2s2mol−2)) |
| thicknesses of the concentration boundary layers (CBLs) (m) | |
| energy conversion efficiency coefficients | |
| matrix of the Peusner coefficients | |
| flux of S-energy (W m−2) | |
| flux of F-energy (W m−2) | |
| flux of U-energy (W m−2) | |
| mass density (kg m−3) | |
| coupling coefficient | |
| concentration Rayleigh number | |
| concentration polarization effects | |
| effects of gravitational convection in osmotic and diffusive transport | |
| Peclét number | |
| (s m−1)) | |
| (mol m−2s−1)) | |
| A and B | configurations of membrane system |
| M | membrane |
| CP | concentration polarization |
| BC | bacterial cellulose |
| the concentration boundary layers (CBLs) | |
| complex of CBLs and membrane | |
| KKP equations | Kedem–Katchalsky–Peusner equations |
References
- Baker, R. Membrane Technology and Application; John Wiley & Sons: New York, NY, USA, 2012; ISBN 978-0-470-74372-0. [Google Scholar]
- Savencu, I.; Iurian, S.; Porfire, A.; Bogdan, C.; Tomuță, I. Review of advances in polymeric wound dressing films. React. Funct. Polym. 2021, 168, 105059. [Google Scholar] [CrossRef]
- Demirel, Y. Nonequilibrium Thermodynamics: Transport and Rate Processes in Physical, Chemical and Biological Systems; Elsevier: Amsterdam, The Netherlands, 2014; ISBN 978-0-444-53079-0. [Google Scholar]
- Lipton, B. The Biology of Belief: Unleashing the Power of Consciousness; Hay House: Carlsbad, CA, USA, 2018; ISBN-10: 1401923127. [Google Scholar]
- Batko, K.M.; Ślęzak, A. Evaluation of the global S-entropy production in membrane transport of aqueous solutions of hydrochloric acid and ammonia. Entropy 2020, 22, 1021. [Google Scholar] [CrossRef] [PubMed]
- Delmotte, M.; Chanu, J. Non-equilibrium Thermodynamics and Membrane Potential Measurement in Biology. In Topics Bioelectrochemistry and Bioenergetics; Millazzo, G., Ed.; John Wiley Publish & Sons: Chichester, UK, 1979; pp. 307–359. [Google Scholar]
- Gerke, K.M.; Vasilyev, R.V.; Khirevich, S.; Collins, D.; Karsanina, M.V.; Sizonenko, T.O.; Korost, D.V.; Lamontagne, S.; Mallants, D. Finite-difference method Stokes solver (FDMSS) for 3D pore geometries: Software development, validation and case studies. Comput. Geosci. 2018, 114, 41–58. [Google Scholar] [CrossRef]
- Blunt, M.J. Flow in porous media—Pore-network models and multiphase flow. Curr. Opin. Colloid Interface Sci. 2001, 6, 197–207. [Google Scholar] [CrossRef]
- Koroteev, D.; Dinariev, O.; Evseev, N.; Klemin, D.; Nadeev, A.; Safonov, S.; Gurpinar, O.; Berg, S.; van Kruijsdijk, C.; Armstrong, R.; et al. Direct hydrodynamic simulation of multiphase flow in porous rock. Petrophysics 2014, 55, 294–303. [Google Scholar]
- Veselý, M.; Bultreys, T.; Peksa, M.; Lang, J.; Cnudde, V.; Van Hoorebeke, L.; Kočiřík, M.; Hejtmánek, V.; Šolcová, O.; Soukup, K.; et al. Prediction and evaluation of time-dependent effective self-diffusivity of water and other effective transport properties associated with reconstructed porous solids. Transp. Porous Media 2015, 110, 81–111. [Google Scholar] [CrossRef]
- Jarzyńska, M.; Pietruszka, M. The application of the Kedem-Katchalsky equations to membrane transport of ethyl alcohol and glucose. Desalination 2011, 280, 14–19. [Google Scholar] [CrossRef]
- Jarzyńska, M.; Staryga, E.; Kluza, F.; Spiess, W.E.L.; Góral, D. Diffusion characteristics in ethyl alcohol and glucose solutions using Kedem-Katchalsky equations. Chem. Eng. Technol. 2020, 43, 248–252. [Google Scholar] [CrossRef]
- Ślęzak, A. Irreversible thermodynamic model equations of the transport across a horizontally mounted membrane. Biophys. Chem. 1989, 34, 91–102. [Google Scholar] [CrossRef]
- Dworecki, K.; Ślęzak, A.; Ornal-Wąsik, B.; Wąsik, S. Effect of hydrodynamic instabilities on solute transport in a membrane system. J. Membr. Sci. 2005, 265, 94–100. [Google Scholar] [CrossRef]
- Ślęzak, A. A model equation for the gravielectric effect in electrochemical cells. Biophys. Chem. 1990, 38, 189–199. [Google Scholar] [CrossRef] [PubMed]
- Kargol, A. Modified Kedem-Katchalsky equations and their application. J. Membr. Sci. 2000, 174, 43–53. [Google Scholar] [CrossRef]
- Ślęzak, A.; Dworecki, K.; Anderson, J.E. Gravitational effects on transmembrane flux: The Rayleigh-Taylor convective instability. J. Membr. Sci. 1985, 23, 71–81. [Google Scholar] [CrossRef]
- Ślęzak, A.; Dworecki, K.; Jasik-Ślęzak, J.; Wąsik, J. Method to determine the practical concentration Rayleigh number in isothermal passive membrane transport processes. Desalination 2004, 168, 397–412. [Google Scholar] [CrossRef]
- Puthenveettil, B.A.; Arakeri, J.H. Plum structure in high-Rayleigh-Number convection. J. Fluid Mech. 2005, 542, 217–249. [Google Scholar] [CrossRef]
- Slezak-Prochazka, I.; Batko, K.M.; Ślęzak, A.; Bajdur, W.M.; Włodarczyk-Makuła, M. Non-linear effects in osmotic membrane transport: Evaluation of the S-entropy production by volume flux of aqueous ammonia and sulfuric acid solutions under concentration polarization conditions. Desal. Water Treat. 2022, 260, 23–36. [Google Scholar] [CrossRef]
- Katchalsky, A.; Curran, P.F. Nonequilibrium Thermodynamics in Biophysics; Harvard: Cambridge, UK, 1965; ISBN 9780674494121. [Google Scholar]
- Friedman, M.H.; Meyer, R.A. Transport across homoporous and heteroporous membranes in nonideal nondilute solutions. I. Inequality of reflection coefficients for volume flow and solute flow. Biophys. J. 1981, 34, 535–544. [Google Scholar] [CrossRef] [PubMed]
- Kargol, M.; Kargol, A. Mechanistic formalism for membrane transport generated by osmotic and mechanical pressure. Gen. Physiol. Biophys. 2003, 22, 51–68. [Google Scholar]
- Batko, K.M.; Ślęzak, A.; Grzegorczyn, S.; Bajdur, W.M. The Rr form of the Kedem–Katchalsky–Peusner model equations for description of the membrane transport in concentration polarization conditions. Entropy 2020, 22, 857. [Google Scholar] [CrossRef]
- Richter, T.; Keipert, S. In vitro permeation studies comparing bovine nasal mucosa, porcine cornea and artificial membrane: Androstendedione in microemulsions and their components. Eur. J. Pharma Biopharm. 2004, 58, 137–143. [Google Scholar] [CrossRef]
- Twardowski, Z. Scholarly Review: History of hemodialyzers’ designs. Hemodial. Inter. 2008, 12, 173–210. [Google Scholar] [CrossRef] [PubMed]
- Zholkovskiy, E.; Koter, I.; Koter, S.; Kujawski, W.; Yaroshchuk, A. Analysis of membrane transport equations for reverse electrodialysis (RED) using irreversible thermodynamics. Int. J. Mol. Sci. 2020, 21, 6325. [Google Scholar] [CrossRef]
- Auclair, B.; Nikonenko, V.; Larchet, C.; Métayer, M.; Dammak, L. Correlation between transport parameters of ion-exchange membranes. J. Membr. Sci. 2002, 195, 89–102. [Google Scholar] [CrossRef]
- Batko, K.M.; Ślęzak-Prochazka, I.; Grzegorczyn, S.M.; Pilis, A.; Dolibog, P.; Ślęzak, A. Energy conversion in Textus Bioactiv Ag membrane dressings using Peusner’s network thermodynamic descriptions. [published on line as ahead of print November 10, 2022]. Polym. Med. 2022, 12. [Google Scholar] [CrossRef]
- Peusner, L. Studies in Network Thermodynamics; Elsevier: Amsterdam, The Netherlands, 1986; ISBN 0444425802. [Google Scholar]
- Ślęzak, A.; Grzegorczyn, S.; Batko, K.M. Resistance coefficients of polymer membrane with concentration polarization. Transp. Porous Media 2012, 95, 151–170. [Google Scholar] [CrossRef]
- Ślęzak, A.; Grzegorczyn, S.; Jasik-Ślęzak, J.; Michalska-Małecka, K. Natural convection as an asymmetrical factor of the transport through porous membrane. Transp. Porous. Media 2010, 84, 685–698. [Google Scholar] [CrossRef]
- Ślęzak, A.; Kucharzewski, M.; Franek, A.; Twardokęs, W. Evaluation of the efficiency of venous leg ulcer treatment with a membrane dressing. Med. Eng. Phys. 2004, 26, 53–60. [Google Scholar] [CrossRef]
- Batko, K.M.; Ślęzak, A.; Pilis, W. Evaluation of transport properties of biomembranes by means of Peusner network thermodynamics. Acta Bioeng. Biomech. 2021, 23, 63–72. [Google Scholar] [CrossRef]
- Hussain, Z.; Sajjad, W.; Khan, T.; Wahid, F. Production of bacterial cellulose from industrial wastes: A review. Cellulose 2019, 26, 2895–2911. [Google Scholar] [CrossRef]
- Tayeb, A.H.; Amini, E.; Ghasemi, M.; Tajvidi, S. Cellulose nanomaterials—Binding properties and applications: A review. Molecules 2018, 23, 2683. [Google Scholar] [CrossRef]
- Grzegorczyn, S.; Ślęzak, A. Kinetics of concentration boundary layers buildup in the system consisted of microbial cellulose biomembrane and electrolyte solutions. J. Membr. Sci. 2007, 304, 148–155. [Google Scholar] [CrossRef]
- Ewing, G.W. Instrumental Methods of Chemical Analysis; McGraw-Hill Book, Co.: New York, NY, USA, 1985; ISBN 0-07-019857-8. [Google Scholar]
- Dworecki, K. Interferometric investigation of near-membrane diffusion layers. J. Biol. Phys. 1995, 21, 37–49. [Google Scholar] [CrossRef]
- Gałczyńska, K.; Rachuna, J.; Ciepluch, K.; Kowalska, M.; Wąsik, S.; Kosztołowicz, T.; Lewandowska, K.D.; Semaniak, J.; Kurdziel, K.; Arabski, M. Experimental and theoretical analysis of metal complex diffusion through cell monolayer. Entropy 2021, 23, 360. [Google Scholar] [CrossRef] [PubMed]
- Arabski, M.; Wąsik, S.; Dworecki, K.; Kaca, W. Laser interferometric determination of ampicillin and colistin transfer through cellulose biomembrane in the presence of Proteus vulgaris O25 lipopolysaccharide. J. Membr. Sci. 2007, 299, 268–275. [Google Scholar] [CrossRef]
- Bason, S.; Kedem, O.; Freger, V. Determination of concentration-dependent transport coefficient s in nanofiltration: Experimental evaluation of coefficients. J. Membr. Sci. 2008, 310, 197–204. [Google Scholar] [CrossRef]
- Kedem, O.; Caplan, S.R. Degree of coupling and its relations to efficiency of energy conversion. Trans. Faraday Soc. 1965, 61, 1897–1911. [Google Scholar] [CrossRef]
- Lebon, G.; Jou, D.; Casas-Vasquez, J. Understanding Non-Equilibrium Thermodynamics. Foundations, Applications, Frontiers; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Batko, K.M.; Ślęzak-Prochazka, I.; Ślęzak, A.; Bajdur, W.M.; Włodarczyk-Makuła, M. Management of energy conversion processes in membrane systems. Energies 2022, 15, 1661. [Google Scholar] [CrossRef]
- Mohammad, A.W.; Teow, Y.H.; Ang, W.L.; Chung, Y.T.; Oatley-Radcliffe, D.L.; Hilal, N. Nanofiltration membranes review: Recent advances and future prospects. Desalination 2015, 356, 226–354. [Google Scholar] [CrossRef]
- Han, Y.; Xu, Z.; Gao, C. Ultrathin Graphene nanofiltration membrane for water purification. Adv. Funct. Mat. 2013, 23, 3693–3700. [Google Scholar] [CrossRef]
- Weinstein, A.M. An equation for flow in the renal proximal tubule. Bull. Math. Biol. 1986, 48, 29–57. [Google Scholar] [CrossRef]
- Huang, Y.; Chen, W.; Lei, Y. Outer membrane vesicles (OMVs) enabled bio-applications: A critical review. Biotech. Bioeng. 2022, 119, 34–47. [Google Scholar] [CrossRef] [PubMed]
- Stamatialis, D.F.; Papenburg, B.J.; Gironés, M.; Saiful, S.; Bettahalli, S.N.M.; Schmitmeier, S.; Wessling, M. Medical applications of membranes: Drug delivery, artificial organs and tissue engineering. J. Membr. Sci. 2008, 308, 1–34. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ślęzak-Prochazka, I.; Batko, K.M.; Ślęzak, A. Evaluation of Transport Properties and Energy Conversion of Bacterial Cellulose Membrane Using Peusner Network Thermodynamics. Entropy 2023, 25, 3. https://doi.org/10.3390/e25010003
Ślęzak-Prochazka I, Batko KM, Ślęzak A. Evaluation of Transport Properties and Energy Conversion of Bacterial Cellulose Membrane Using Peusner Network Thermodynamics. Entropy. 2023; 25(1):3. https://doi.org/10.3390/e25010003
Chicago/Turabian StyleŚlęzak-Prochazka, Izabella, Kornelia M. Batko, and Andrzej Ślęzak. 2023. "Evaluation of Transport Properties and Energy Conversion of Bacterial Cellulose Membrane Using Peusner Network Thermodynamics" Entropy 25, no. 1: 3. https://doi.org/10.3390/e25010003
APA StyleŚlęzak-Prochazka, I., Batko, K. M., & Ślęzak, A. (2023). Evaluation of Transport Properties and Energy Conversion of Bacterial Cellulose Membrane Using Peusner Network Thermodynamics. Entropy, 25(1), 3. https://doi.org/10.3390/e25010003






