Temperature Dependence of Structural Relaxation in Glass-Forming Liquids and Polymers
Abstract
1. Introduction
2. Theoretical Concepts Proposed for Describing the Temperature Dependence of Structural Relaxation in Supercooled Liquids
2.1. Semi-Empirical Equations Describing τα(T)
2.2. Free Volume Approach
2.3. Elastic Models
2.4. Entropic Models
2.5. Other Model Approaches
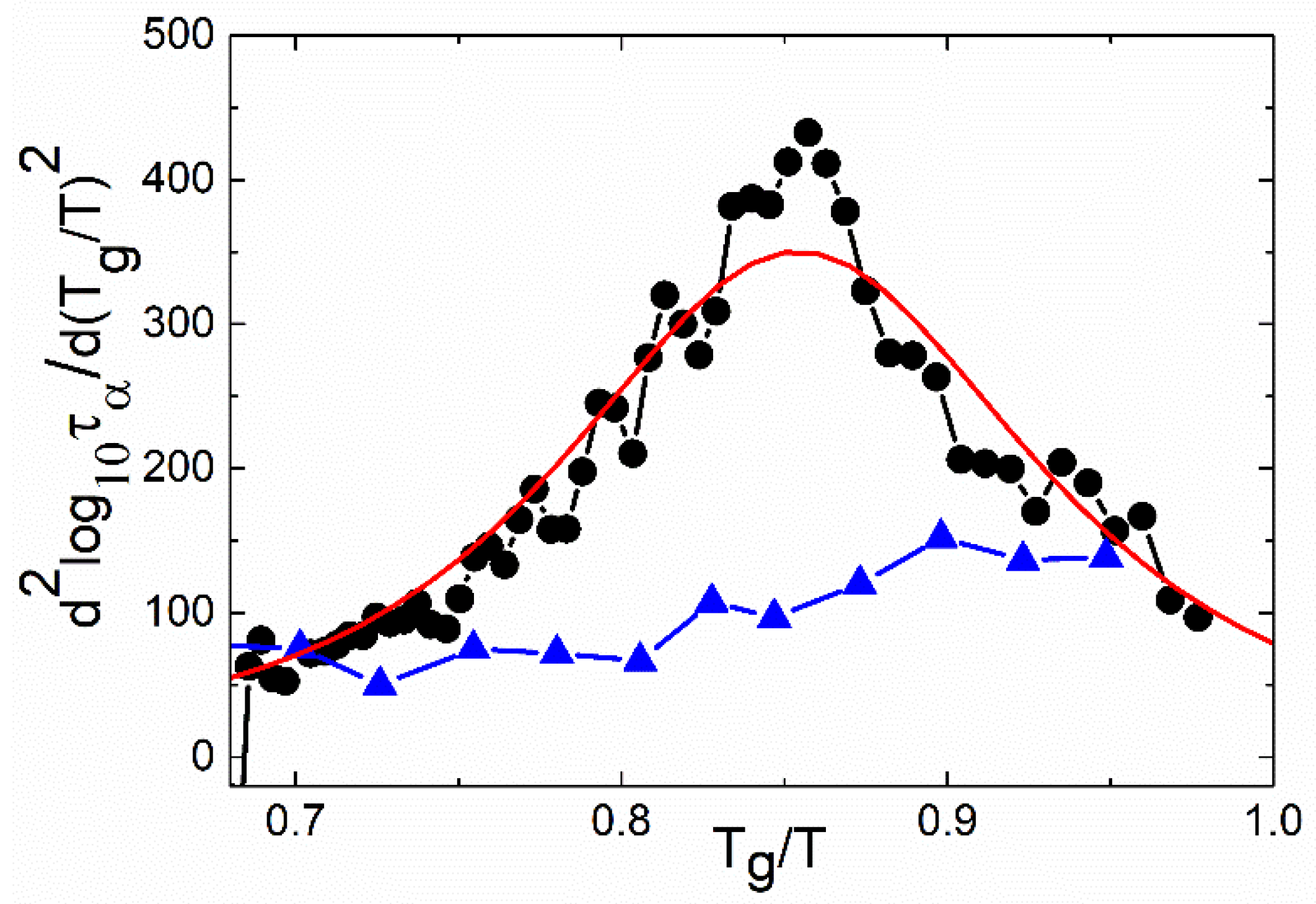
3. Experimental Tests of Different Models: Search for Cooperativity
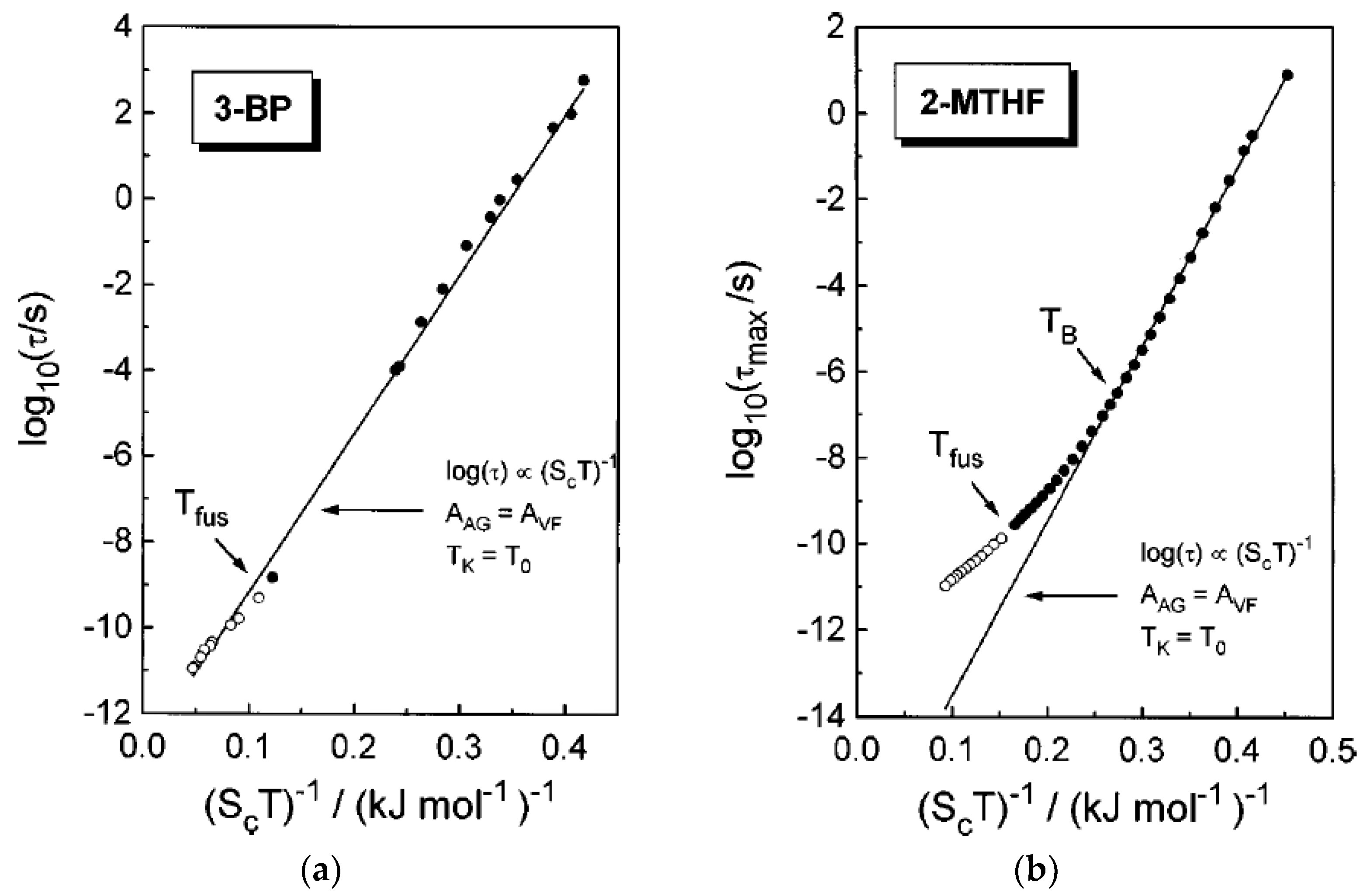
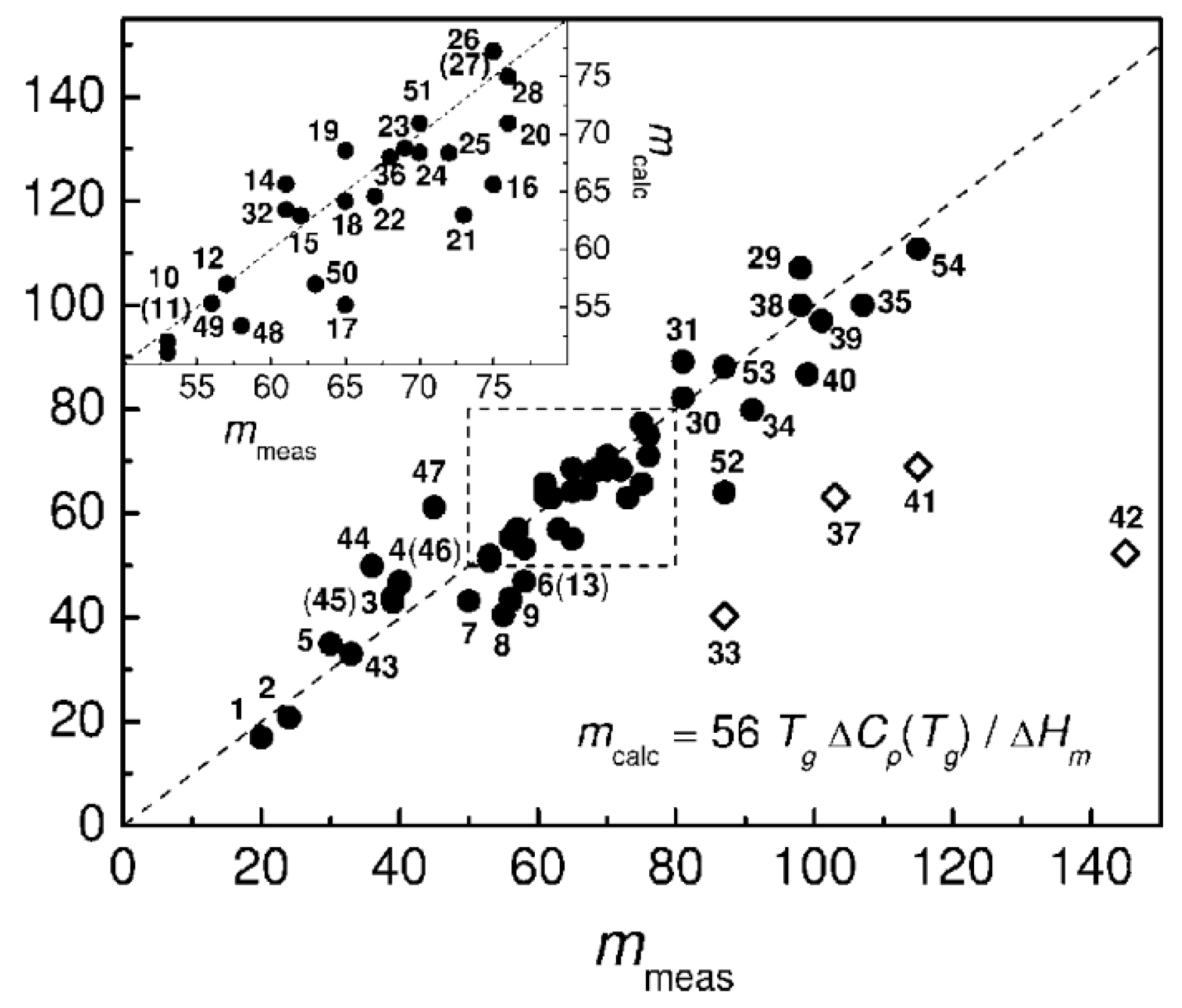
3.1. Thermodynamic Properties
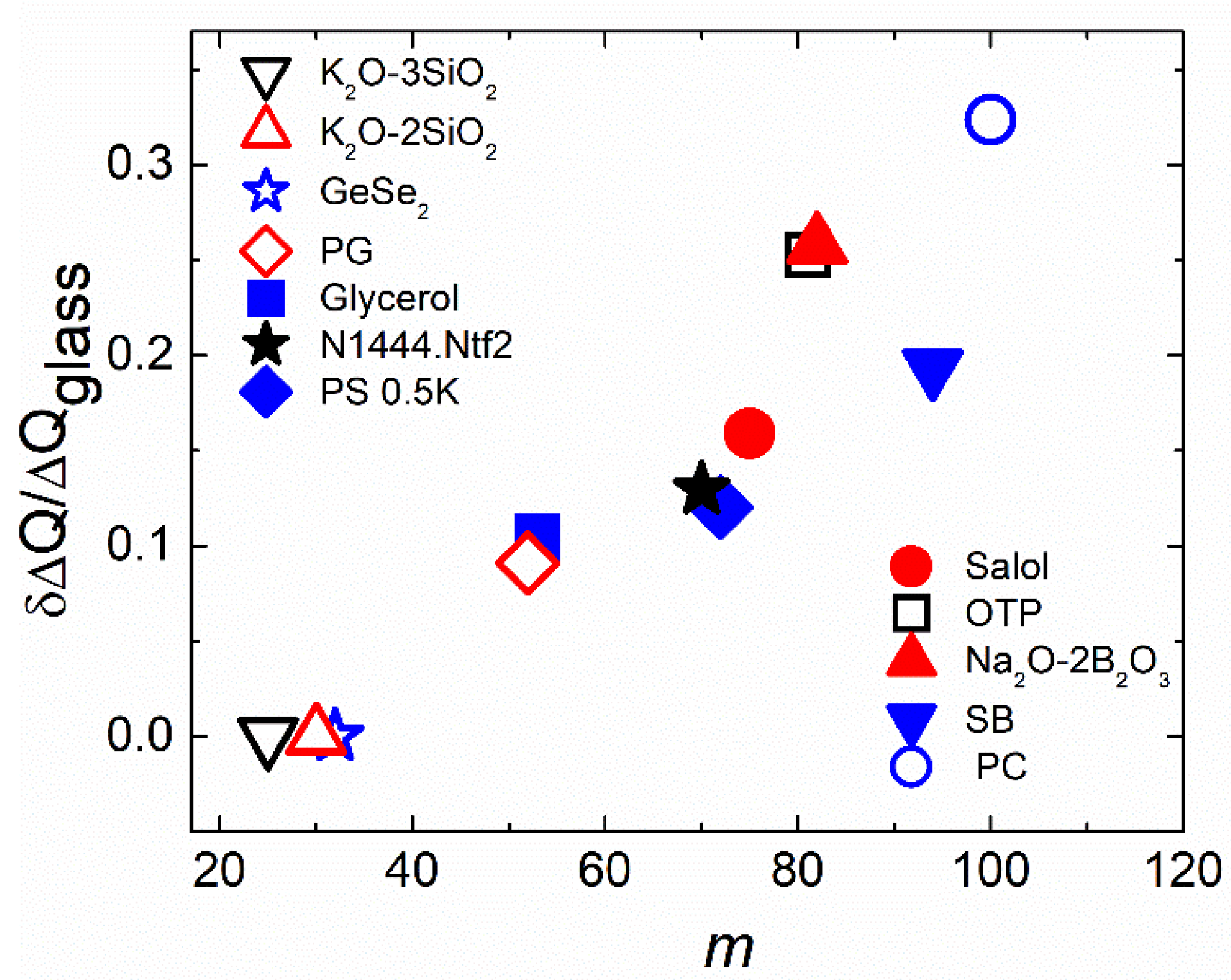
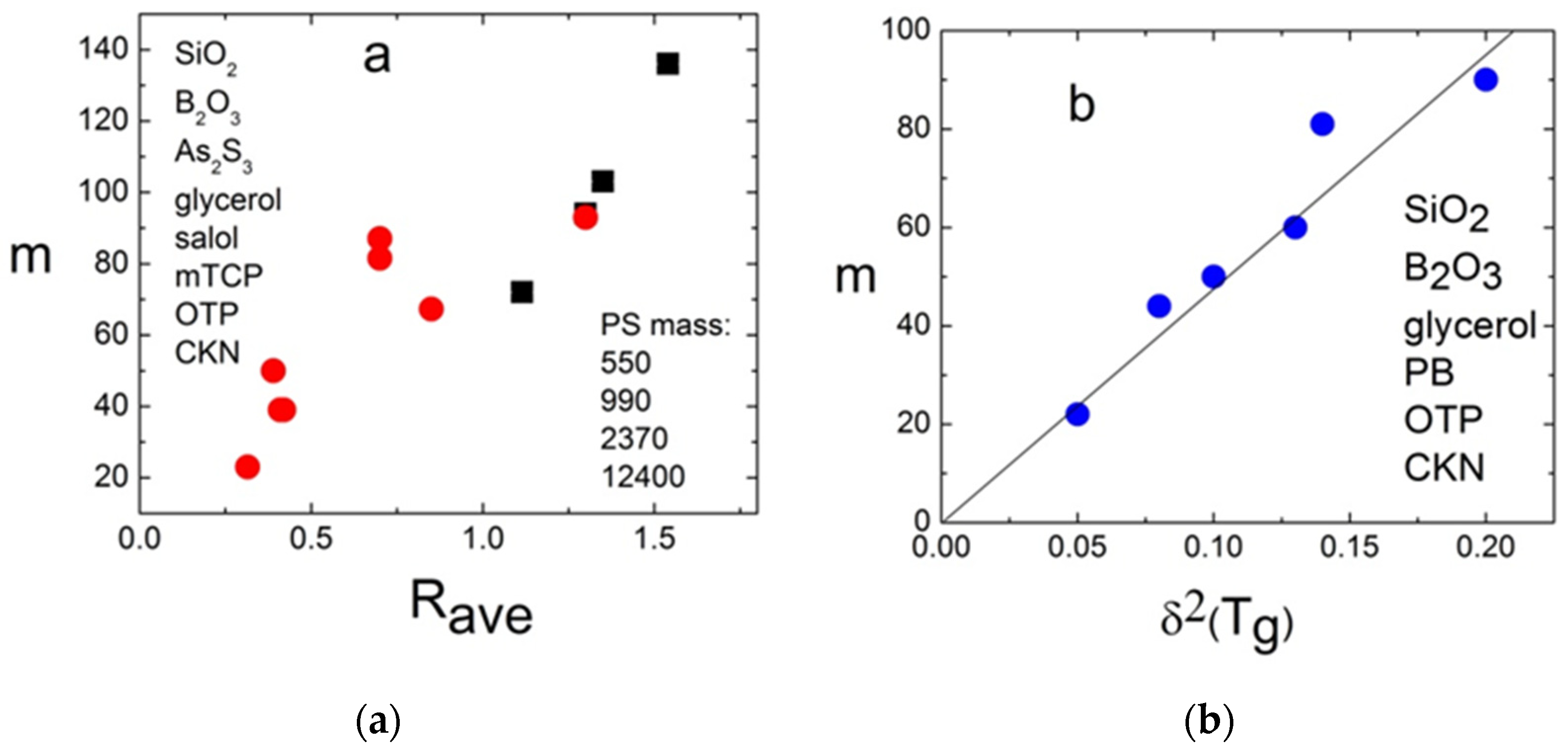
3.2. Role of Cooperativity/Heterogeneity
3.3. Role of Density (Free Volume) and Thermal Energy
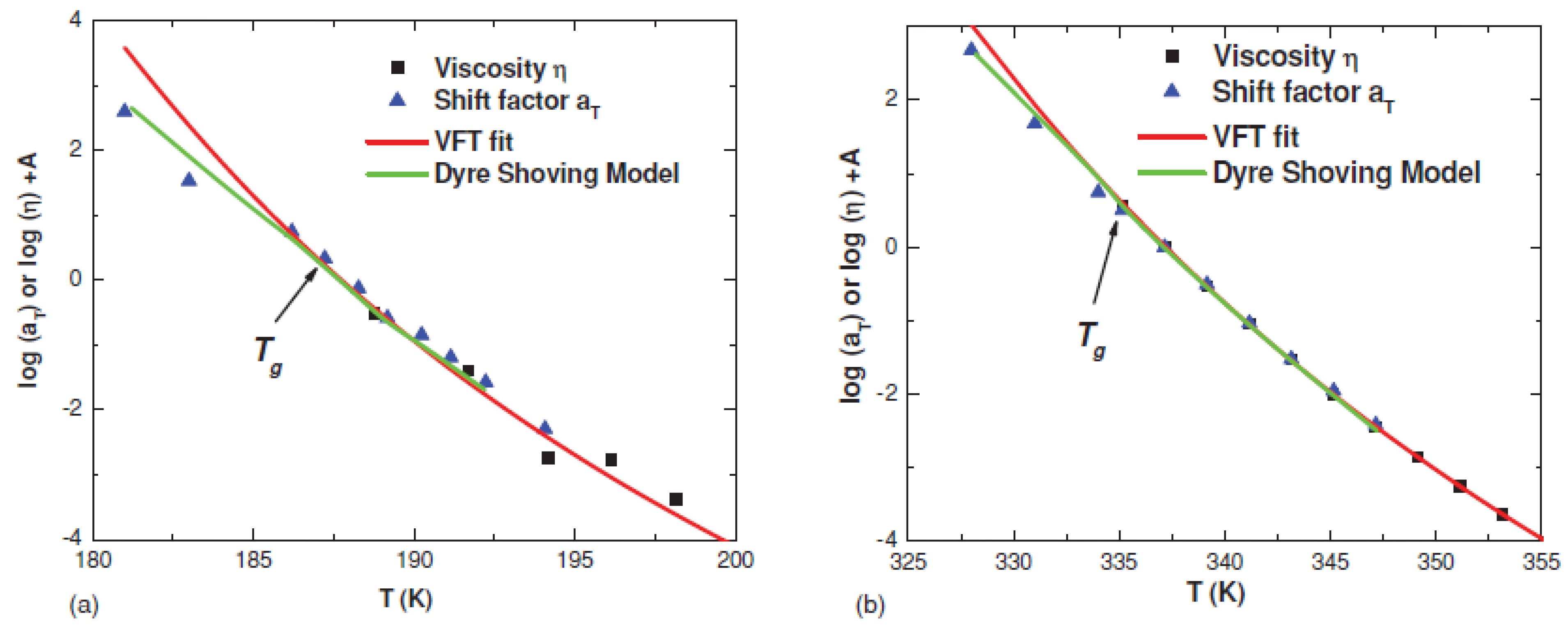
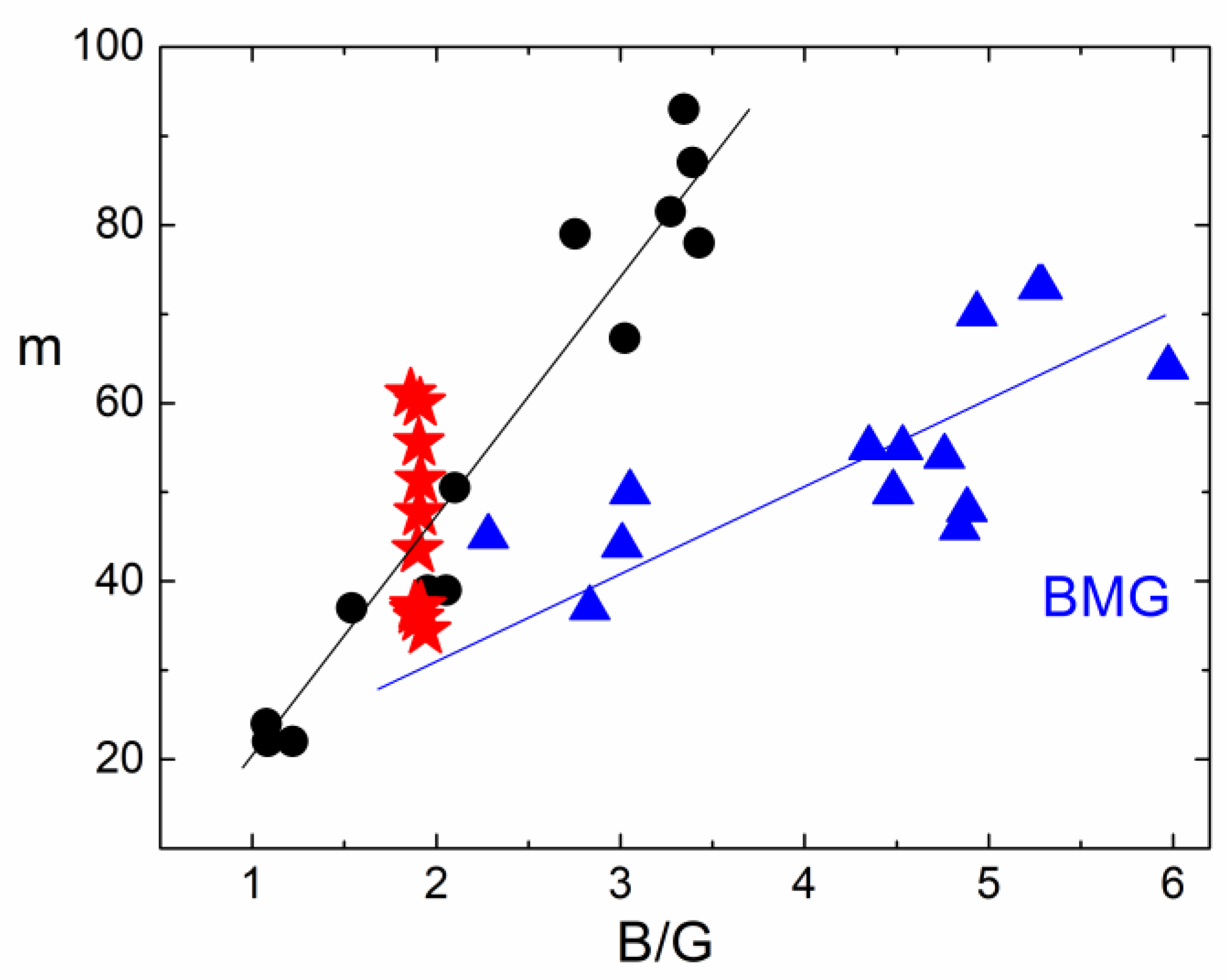
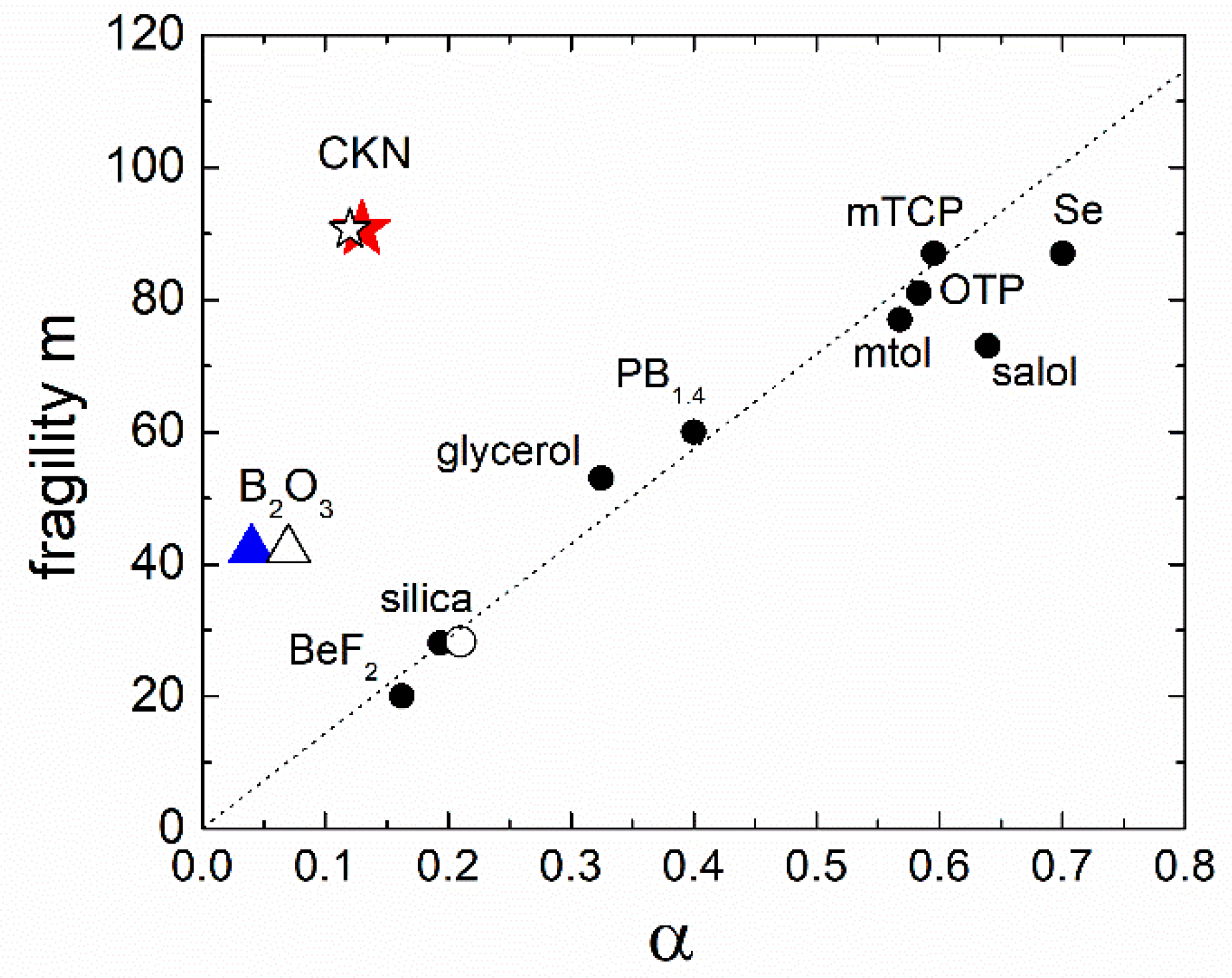
3.4. Tests of Elasticity Model
4. Connection between Fast and Slow Dynamics
4.1. Relationship of Fragility and Short Time 〈u2〉
4.2. Fragility and Fast Dynamics
4.3. Fragility and Nonergodicity Parameter in Glassy State
4.4. A General Picture Connecting Fast and Slow Dynamics
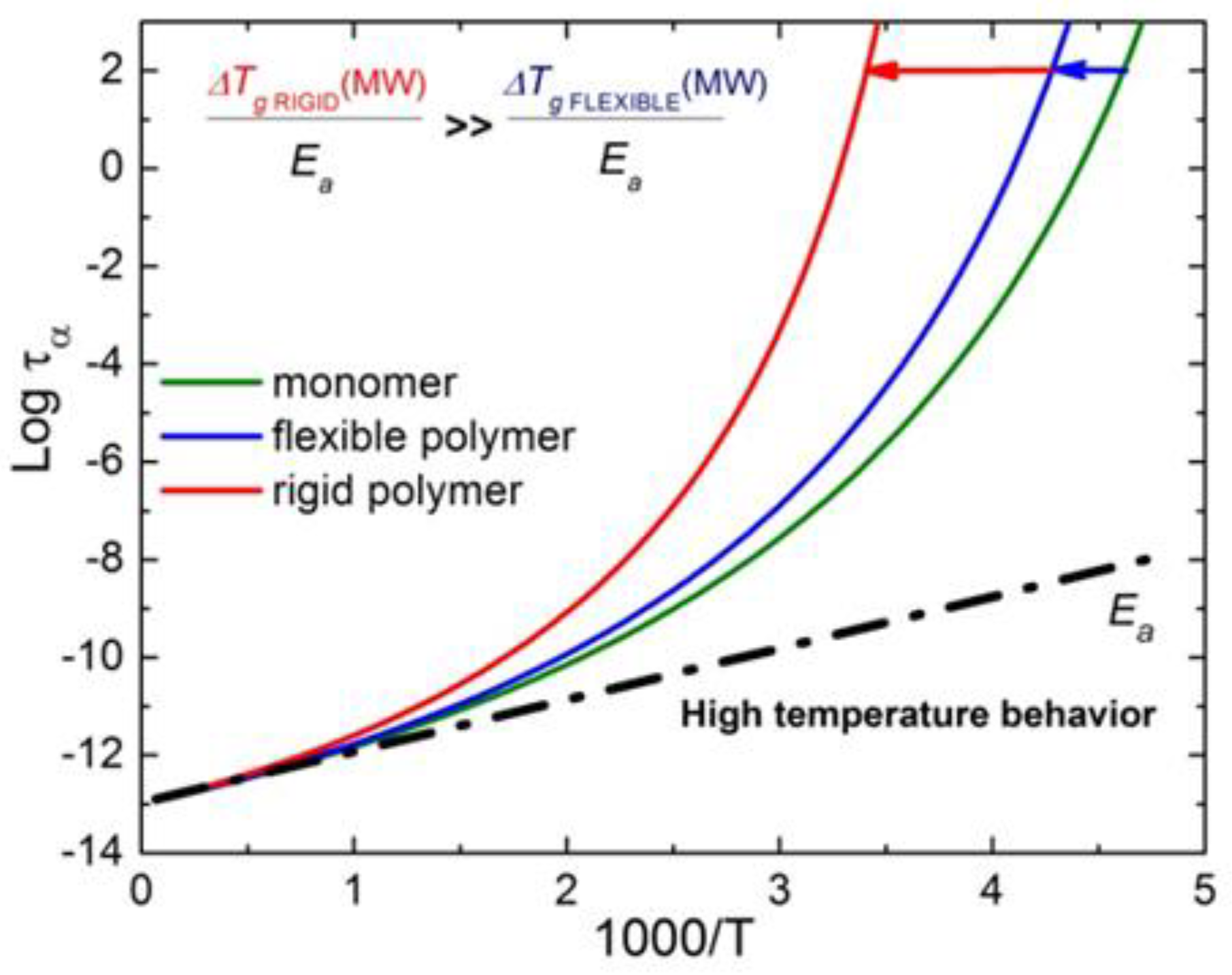
5. Extreme Fragility in Polymers
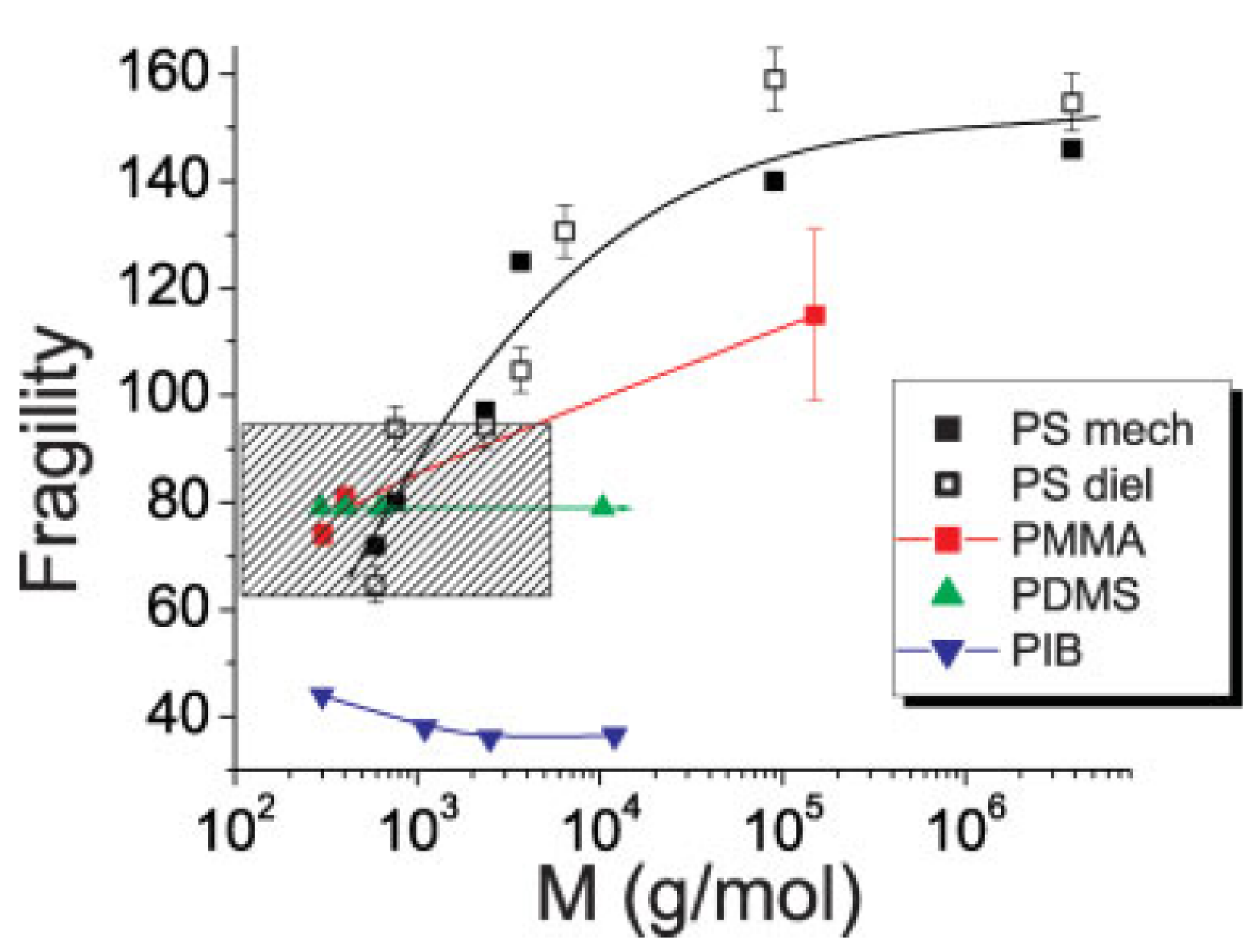
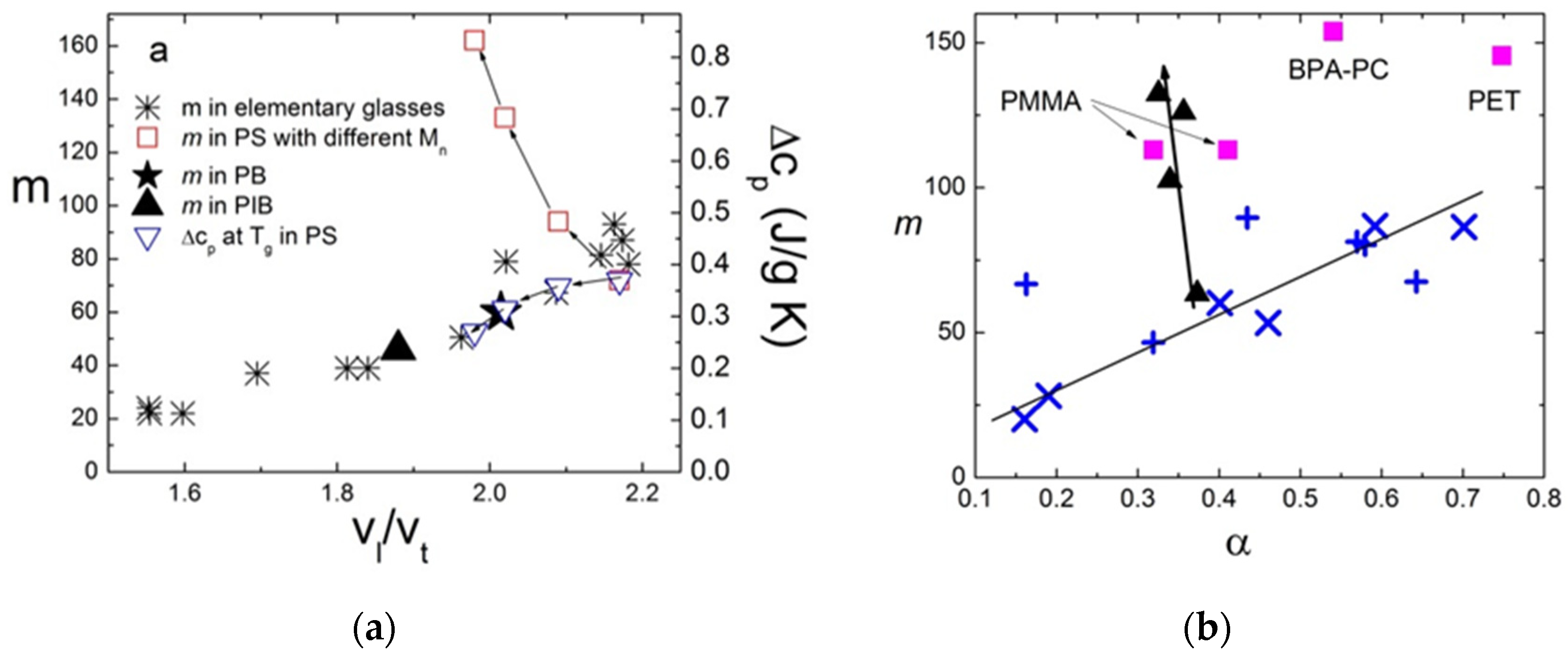
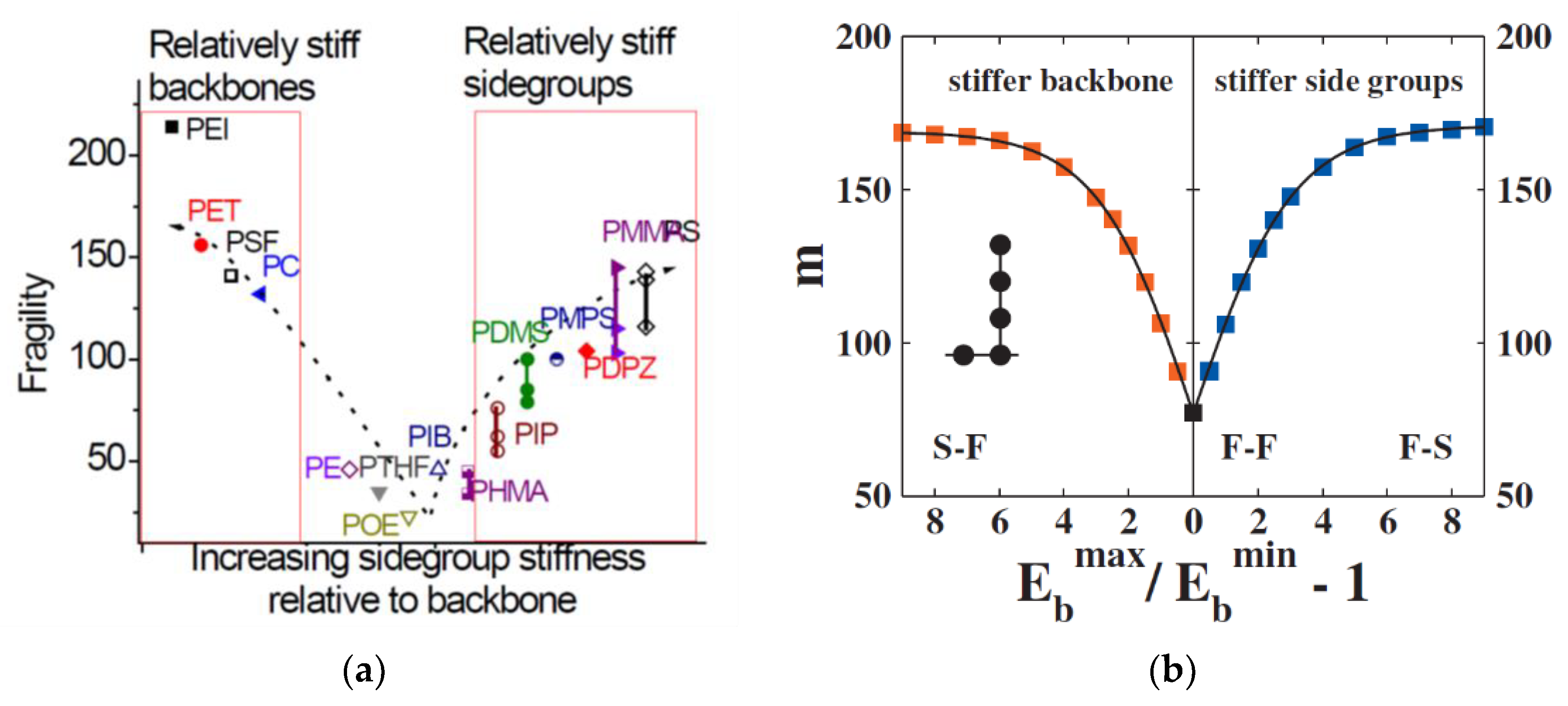
5.1. Failure of Many Correlations in Polymers
5.2. Polymer Specific Contribution to Fragility
6. Quantum Effects and ‘Super-Strong’ Behavior of Water
7. Conclusions and Perspectives
Author Contributions
Funding
Conflicts of Interest
References
- Angell, C.A. Formation of glasses from liquids and biopolymers. Science 1995, 267, 1924–1935. [Google Scholar] [CrossRef] [PubMed]
- Angell, C.A. Liquid fragility and the glass transition in water and aqueous solutions. Chem. Rev. 2002, 102, 2627–2650. [Google Scholar] [CrossRef] [PubMed]
- Greer, A.L.; Kelton, K.; Sastry, S. (Eds.) Fragility of Glass-Forming Liquids; Hindustan Book Agency: Gurgaon, India, 2014. [Google Scholar]
- Ngai, K.L. Relaxation and Diffusion in Complex Systems; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Böhmer, R.; Ngai, K.L.; Angell, C.A.; Plazek, J. Nonexponential relaxations in strong and fragile glass formers. J. Chem. Phys. 1993, 99, 4201–4209. [Google Scholar] [CrossRef]
- Huang, D.; McKenna, G.B. New insights into the fragility dilemma in liquids. J. Chem. Phys. 2001, 114, 5621–5630. [Google Scholar] [CrossRef]
- Angell, C.A.; Tucker, J.C. Physical Chemistry of Process Metallurgy: Papers Presented at the July 1973 Richardson Conference; Jeffes, J.H.E., Tait, R.J., Eds.; Institution of Mining and Metallurgy Publication: London, UK, 1974. [Google Scholar]
- Angell, C.A. Spectroscopy simulation and scattering, and the medium range order problem in glass. J. Non-Cryst. Solids 1985, 73, 1–17. [Google Scholar] [CrossRef]
- Oldekop, W. Theoretical considerations on the viscosity of glass. Glastechn. Ber. 1957, 30, 8–14. [Google Scholar]
- Nemilov, S.V. On the interrelation of the entropy of the activation of a viscous flow, heat capacity and valence structure of glass. Zhurnal Pikladnoi Khimii 1964, 37, 293–300. [Google Scholar]
- Laughlin, W.T.; Uhlmann, D.R. Viscous flow in simple organic liquids. J. Phys. Chem. 1972, 76, 2317–2325. [Google Scholar] [CrossRef]
- Zheng, Q.; Mauro, J.C.; Ellison, A.J.; Potuzak, M.; Yue, Y. Universality of the high-temperature viscosity limit of silicate liquids. Phys. Rev. B 2011, 83, 212202. [Google Scholar] [CrossRef]
- Nemilov, S.V. Thermodynamic and Kinetic Aspects of the Vitreous State; CRC Press: Boca Raton, FL, USA, 1994. [Google Scholar]
- Novikov, V.N.; Ding, Y.; Sokolov, A.P. Correlation of fragility of supercooled liquids with elastic properties of glasses. Phys. Rev. E 2005, 71, 061501. [Google Scholar] [CrossRef]
- Götze, W. Complex Dynamics of Glass-Forming Liquids: A Mode-Coupling Theory; Oxford University Press: Oxford, UK, 2012. [Google Scholar]
- Vogel, H. Das Temperaturabhängigkeitsgesestz der Viskosität von Flüssigkeiten. Phys. Z. 1921, 22, 645–646. [Google Scholar]
- Fulcher, G.S. Analysis of recent measurements of the viscosity of glasses. J. Am. Ceram. Soc. 1925, 8, 339–355. [Google Scholar] [CrossRef]
- Tammann, G. Glasses as supercooled liquids. J. Soc. Glass Technol. 1925, 9, 166–185. [Google Scholar]
- Williams, M.L.; Landel, R.F.; Ferry, J.D. The temperature dependence of relaxation mechanisms in amorphous polymers and other glass-forming liquids. J. Am. Chem. Soc. 1955, 77, 3701–3707. [Google Scholar] [CrossRef]
- Stickel, F.; Fischer, E.W.; Richert, R. Dynamics of glass-forming liquids. II. Detailed comparison of dielectric relaxation, dc-conductivity, and viscosity data. J. Chem. Phys. 1996, 104, 2043–2055. [Google Scholar] [CrossRef]
- Avramov, I.; Milchev, A. Effect of disorder on diffusion and viscosity in condensed systems. J. Non-Cryst. Solids 1988, 104, 253–260. [Google Scholar] [CrossRef]
- Hutton, J.F.; Phillips, M.C. Amorphous Materials; Douglas, R.W., Ellis, B., Eds.; Wiley: New York, NY, USA, 1972. [Google Scholar]
- Utracki, L.A. Temperature dependence of liquid viscosity. J. Macromol. Sci. B 1974, 10, 477–505. [Google Scholar] [CrossRef]
- Sturm, V.K.G. Zur Temperaturabhaengigkeit der Viskositaet von Fluessigkeiten. Glastechn. Ber. 1980, 53, 63–76. [Google Scholar]
- Mauro, J.C.; Yue, Y.; Ellison, A.J.; Gupta, P.K.; Allan, D.C. Viscosity of glass-forming liquids. Proc. Natl. Acad. Sci. USA 2009, 106, 19780–19784. [Google Scholar] [CrossRef]
- Waterton, S.C. The viscosity-temperature relationship and some inferences on the nature of molten and of plastic glass. J. Soc. Glass Technol. 1932, 16, 244–249. [Google Scholar]
- Schmidtke, B.; Petzold, N.; Kahlau, R.; Hofmann, V.; Rössler, E.A. From boiling point to glass transition temperature: Transport coefficients in molecular liquids follow three parameter scaling. Phys. Rev. E 2012, 86, 041507. [Google Scholar] [CrossRef]
- Hecksher, T.; Nielsen, A.I.; Olsen, N.B.; Dyre, J.C. Little evidence for dynamic divergences in ultraviscous molecular liquids. Nature Phys. 2008, 4, 737–741. [Google Scholar] [CrossRef]
- Zhao, J.; Simon, S.L.; McKenna, G.B. Using 20-million-year-old amber to test the super-Arrhenius behaviour of glass-forming systems. Nat. Commun. 2013, 4, 1783. [Google Scholar] [CrossRef]
- Elmatad, Y.; Garrahan, J.P.; Chandler, D. Corresponding states of structural glass formers. J. Phys. Chem. B 2009, 113, 5563–5567. [Google Scholar] [CrossRef]
- Novikov, V.N.; Sokolov, A.P. Qualitative change in structural dynamics of some glass-forming systems. Phys. Rev. E 2015, 92, 062304. [Google Scholar] [CrossRef]
- Angell, C.A.; Ngai, K.L.; McKenna, G.B.; McMillan, P.F.; Martin, S.W. Relaxation in glass-forming liquids and amorphous solids. J. Appl. Phys. 2000, 88, 3113–3157. [Google Scholar] [CrossRef]
- Doolittle, A.K. Studies in newtonian flow. II. The dependence of the viscosity of liquids on free-space. J. Appl. Phys. 1951, 22, 1471–1475. [Google Scholar] [CrossRef]
- Cohen, M.H.; Turnbull, D. Molecular transport in liquids and glasses. J. Chem. Phys. 1959, 31, 1164–1169. [Google Scholar] [CrossRef]
- Turnbull, D.; Cohen, M.H. Free-volume model of the amorphous phase: Glass transition. J. Chem. Phys. 1961, 34, 120–125. [Google Scholar] [CrossRef]
- Turnbull, D.; Cohen, M.H.J. On the Free-Volume Model of the Liquid-Glass Transition. J. Chem. Phys. 1970, 52, 3038–3041. [Google Scholar]
- Fox, T.G.; Flory, P.J. Second-order transition temperatures and related properties of polystyrene. I. Influence of molecular weight. J. Appl. Phys. 1950, 21, 581–591. [Google Scholar] [CrossRef]
- Corezzi, S.; Capaccioli, S.; Casalini, R.; Fioretto, D.; Paluch, M.; Rolla, P.A. Check of the temperature- and pressure-dependent Cohen–Grest equation. Chem. Phys. Lett. 2000, 320, 113–117. [Google Scholar] [CrossRef]
- Cohen, M.H.; Grest, G.S. Liquid-glass transition, a free-volume approach. Phys. Rev. B 1979, 20, 1077–1098. [Google Scholar] [CrossRef]
- Nemilov, S.V. Interrelation between shear modulus and the molecular parameters of viscous flow for glass forming liquids. J. Non-Cryst. Sol. 2006, 352, 2715–2725. [Google Scholar] [CrossRef]
- Dyre, J.C.; Olsen, N.B.; Christensen, T. Local elastic expansion model for viscous-flow activation energies of glass-forming molecular liquids. Phys. Rev. B 1996, 53, 2171–2174. [Google Scholar] [CrossRef]
- Dyre, J.C.; Christensen, T.; Olsen, N.B. Elastic models for the non-Arrhenius viscosity of glass-forming liquids. J. Non-Cryst. Solids 2006, 352, 4632–4635. [Google Scholar] [CrossRef]
- Dyre, J.C. Colloquium: The glass transition and elastic models of glassforming liquids. Rev. Mod. Phys. 2006, 78, 953–972. [Google Scholar] [CrossRef]
- Tobolsky, A.; Powell, R.E.; Eyring, R.E. Elastic-viscous properties of matter. In Frontiers in Chemistry; Burk, R.E., Grummit, O., Eds.; Interscience: New York, NY, USA, 1943. [Google Scholar]
- Dudowicz, J.; Freed, K.F.; Douglas, J.F. Entropy theory of polymer glass formation revisited. I. General formulation. J. Chem. Phys. 2006, 124, 064901. [Google Scholar] [CrossRef]
- van Zanten, J.H.; Rufener, K.P. Brownian motion in a single relaxation time Maxwell fluid. Phys. Rev. E 2000, 62, 5389–5396. [Google Scholar] [CrossRef]
- Hall, R.W.; Wolynes, P.G. The aperiodic crystal picture and free energy barriers in glasses. J. Chem. Phys. 1987, 86, 2943–2948. [Google Scholar] [CrossRef]
- Buchenau, U.; Zorn, R. A relation between fast and slow motions in glassy and liquid selenium. Europhys. Lett. 1992, 18, 523–528. [Google Scholar] [CrossRef]
- Novikov, V.N.; Sokolov, A.P. Universality of the dynamic crossover in glass-forming liquids: A ‘‘magic’’ relaxation time. Phys. Rev. E 2003, 67, 031507. [Google Scholar] [CrossRef]
- Roland, C.M.; Ngai, K.L. The anomalous Debye—Waller factor and the fragility of glasses. J. Chem. Phys. 1996, 104, 2967–2970. [Google Scholar] [CrossRef]
- Teeter, M.M.; Yamano, A.; Stec, B.; Mohanty, U. On the nature of a glassy state of matter in a hydrated protein: Relation to protein function. Proc. Natl. Acad. Sci. USA 2001, 98, 11242–11247. [Google Scholar] [CrossRef]
- Bordat, P.F.; Affouard, F.; Descamps, M.; Ngai, K.L. Does the interaction potential determine both the fragility of a liquid and the vibrational properties of its glassy state? Phys. Rev. Lett. 2004, 93, 105502. [Google Scholar] [CrossRef]
- Ngai, K.L. Why the fast relaxation in the picosecond to nanosecond time range can sense the glass transition. Philos. Mag. 2004, 84, 1341–1353. [Google Scholar] [CrossRef]
- Adam, G.; Gibbs, J.H. On the temperature dependence of cooperative relaxation properties in glass-forming liquids. J. Chem. Phys. 1965, 43, 139–146. [Google Scholar] [CrossRef]
- Magill, J.H. Physical Properties of aromatic hydrocarbons. iii. A test of the adam—gibbs relaxation model for glass formers based on the heat-capacity data of 1,3,5-tri-α-naphthylbenzene. J. Chem. Phys. 1967, 47, 2802–2807. [Google Scholar] [CrossRef]
- Richert, R.; Angell, C.A. Dynamics of glass-forming liquids. V. On the link between molecular dynamics and configurational entropy. J. Chem. Phys. 1998, 108, 9016–9026. [Google Scholar] [CrossRef]
- Roland, C.M.; Capaccioli, S.; Lucchesi, M.; Casalini, R. Adam–Gibbs model for the supercooled dynamics in the ortho-terphenyl ortho-phenylphenol mixture. J. Chem. Phys. 2004, 120, 10640–10646. [Google Scholar] [CrossRef]
- Mohanty, U.; Craig, N.; Fourkas, J.T. Relationship between kinetics and thermodynamics of supercooled liquids. J. Chem. Phys. 2001, 114, 10577–10578. [Google Scholar] [CrossRef]
- Scala, A.; Starr, F.W.; La Nave, E.; Sciortino, F.; Stanley, H.E. Configurational entropy and diffusivity of supercooled water. Nature 2000, 406, 166–169. [Google Scholar] [CrossRef]
- Sastry, S. The relationship between fragility, configurational entropy and the potential energy landscape of glass-forming liquids. Nature 2001, 409, 164–167. [Google Scholar] [CrossRef]
- Sengupta, S.; Karmakar, S.; Dasgupta, C.; Sastry, S. Adam-Gibbs relation for glass-forming liquids in two, three, and four dimensions. Phys. Rev. Lett. 2012, 109, 095705. [Google Scholar] [CrossRef]
- Dyre, J.; Hecksher, T.; Niss, K. A brief critique of the Adam-Gibbs entropy model. J. Non-Cryst. Solids 2009, 355, 624–627. [Google Scholar]
- Tanaka, H. Relation between thermodynamics and kinetics of glass-forming liquids. Phys. Rev. Lett. 2003, 90, 055701. [Google Scholar] [CrossRef]
- Kirkpatrick, T.R.; Thirumalai, D.; Wolynes, P.G. Scaling concepts for the dynamics of viscous liquids near an ideal glassy state. Phys. Rev. A 1989, 40, 1045–1054. [Google Scholar] [CrossRef]
- Xia, X.; Wolynes, P.G. Fragilities of liquids predicted from the random first order transition theory of glasses. Proc. Natl. Acad. Sci. USA 2000, 97, 2990–2994. [Google Scholar] [CrossRef]
- Lubchenko, V.; Wolynes, P.G. Barrier softening near the onset of nonactivated transport in supercooled liquids: Implications for establishing detailed connection between thermodynamic and kinetic anomalies in supercooled liquids. J. Chem. Phys. 2003, 119, 9088–9105. [Google Scholar] [CrossRef]
- Stevenson, J.D.; Wolynes, P.G. Thermodynamic-kinetic correlations in supercooled liquids: A critical survey of experimental data and predictions of the random first-order transition theory of glasses. J. Phys. Chem. B 2005, 109, 15093–15097. [Google Scholar] [CrossRef]
- Bouchaud, J.-P.; Biroli, G. On the Adam-Gibbs-Kirkpatrick-Thirumalai-Wolynes scenario for the viscosity increase in glasses. J. Chem. Phys. 2004, 121, 7347–7354. [Google Scholar] [CrossRef]
- Wolynes, P.G. Randomness and complexity in chemical physics. Acc. Chem. Res. 1992, 25, 513–519. [Google Scholar] [CrossRef]
- Freed, K.F. Influence of monomer molecular structure on the glass transition in polymers I. Lattice cluster theory for the configurational entropy. J. Chem. Phys. 2003, 119, 5730–5739. [Google Scholar] [CrossRef]
- Dudowicz, J.; Freed, K.F.; Douglas, J.F. Generalized entropy theory of glass formation. Adv. Chem. Phys. 2008, 137, 125–222. [Google Scholar]
- Gibbs, J.H.; Dimarzio, E.A. Nature of the Glass Transition and the Glassy State. J. Chem. Phys. 1958, 28, 373–383. [Google Scholar] [CrossRef]
- Rössler, E.; Hess, K.-U.; Novikov, V.N. Universal representation of viscosity in glass forming liquids. J. Non-Cryst. Solids 1998, 223, 207–222. [Google Scholar] [CrossRef]
- Schweizer, K.S.; Saltzman, E.J. Theory of dynamic barriers, activated hopping, and the glass transition in polymer melts. J. Chem. Phys. 2004, 121, 1984–2000. [Google Scholar] [CrossRef]
- Saltzman, E.J.; Schweizer, K.S. Universal scaling, dynamic fragility, segmental relaxation, and vitrification in polymer melts. J. Chem. Phys. 2004, 121, 2001–2009. [Google Scholar] [CrossRef]
- Schweizer, K.S.; Saltzman, E.J. Activated hopping, barrier fluctuations, and heterogeneity in glassy suspensions and liquids. J. Phys. Chem. B 2004, 108, 19729–19741. [Google Scholar] [CrossRef]
- Starr, F.W.; Sastry, S.; Douglas, J.F.; Glotzer, S.C. What do we learn from the local geometry of glass-forming liquids? Phys. Rev. Lett. 2002, 89, 125501. [Google Scholar] [CrossRef]
- Wyart, M. Correlations between vibrational entropy and dynamics in liquids. Phys. Rev. Lett. 2010, 104, 095901. [Google Scholar] [CrossRef]
- Yan, L.; Düring, G.; Wyart, M. Why glass elasticity affects the thermodynamics and fragility of supercooled liquids. Proc. Natl. Acad. Sci. USA 2013, 110, 6307–6312. [Google Scholar] [CrossRef]
- Novikov, V.N.; Rössler, E.; Malinovsky, V.K.; Surovtsev, N.V. Strong and fragile liquids in percolation approach to the glass transition. Europhys. Lett. 1996, 35, 289–294. [Google Scholar] [CrossRef]
- Mirigian, S.; Schweizer, K.S. Unified theory of activated relaxation in liquids over 14 decades in time. J. Phys. Chem. Lett. 2013, 4, 3648–3653. [Google Scholar] [CrossRef]
- Mirigian, S.; Schweizer, K.S. Elastically cooperative activated barrier hopping theory of relaxation in viscous fluids. I. General formulation and application to hard sphere fluids. J. Chem. Phys. 2014, 140, 194506. [Google Scholar] [CrossRef]
- Mirigian, S.; Schweizer, K.S. Elastically cooperative activated barrier hopping theory of relaxation in viscous fluids. II. Thermal liquids. J. Chem. Phys. 2014, 140, 194507. [Google Scholar] [CrossRef]
- Mirigian, S.; Schweizer, K.S. Dynamical Theory of segmental relaxation and emergent elasticity in supercooled polymer melts. Macromolecules 2015, 48, 1901–1913. [Google Scholar] [CrossRef]
- Xie, S.J.; Schweizer, K.S. Nonuniversal coupling of cage scale hopping and collective elastic distortion as the origin of dynamic fragility diversity in glass forming polymer liquids. Macromolecules 2016, 49, 9655–9664. [Google Scholar] [CrossRef]
- Mei, B.; Zhou, Y.; Schweizer, K.S. Experimental tests of a theoretically predicted noncausal correlation between dynamics and thermodynamics in glassforming polymer melts. Macromolecules 2021, 54, 10086–10099. [Google Scholar] [CrossRef]
- Mei, B.; Zhou, Y.; Schweizer, K.S. Thermodynamics-structure-dynamics correlations and nonuniversal effects in the elastically collective activated hopping theory of glass-forming liquids. J. Phys. Chem. B 2020, 124, 6121–6131. [Google Scholar] [CrossRef]
- Mei, B.; Zhou, Y.; Schweizer, K.S. Experimental test of a predicted dynamics-structure-thermodynamics connection in molecularly complex glass-forming liquids. Proc. Natl. Acad. Sci. USA 2021, 118, e2025341118. [Google Scholar] [CrossRef]
- Kivelson, D.; Kivelson, S.A.; Zhao, X.-L.; Nussinov, Z.; Tarjus, G. A thermodynamic theory of supercooled liquids. Phys. A 1995, 219, 27–38. [Google Scholar] [CrossRef]
- Tarjus, G.; Kivelson, S.A.; Nussinov, Z.; Viot, P. The frustration-based approach of supercooled liquids and the glass transition: A review and critical assessment. J. Phys. Condens. Matter 2005, 17, R1143–R1182. [Google Scholar] [CrossRef]
- Chandler, D.; Garrahan, J.P. Dynamics on the way to forming glass: Bubbles in space-time. Annu. Rev. Phys. Chem. 2010, 61, 191–217. [Google Scholar] [CrossRef] [PubMed]
- Merolle, M.; Garrahan, J.P.; Chandler, D. Space-time thermodynamics of the glass transition. Proc. Natl. Acad. Sci. USA 2005, 102, 10837–10840. [Google Scholar] [CrossRef]
- Keys, A.S.; Hedges, L.O.; Garrahan, J.P.; Glotzer, S.C.; Chandler, D. Excitations are localized and relaxation is hierarchical in glass-forming liquids. Phys. Rev. X 2011, 1, 021013. [Google Scholar] [CrossRef]
- Cangialosi, D.; Algeria, A.; Colmenero, J. A thermodynamic approach to the fragility of glass-forming polymers. J. Chem. Phys. 2006, 124, 024906. [Google Scholar] [CrossRef]
- Ruta, B.; Monaco, G.; Giordano, V.M.; Scarponi, F.; Fioretto, D.; Ruocco, G.; Andrikopoulos, K.S.; Yannopoulos, S.N. Nonergodicity factor, fragility, and elastic properties of polymeric glassy sulfur. J. Phys. Chem. B 2011, 115, 14052–14063. [Google Scholar] [CrossRef]
- Paluch, M.; Pawlus, S.; Hensel-Bielowka, S.; Kaminska, E.; Prevosto, D.; Capaccioli, S.; Rolla, P.A.; Ngai, K.L. Two secondary modes in decahydroisoquinoline: Which one is the true Johari-Goldstein process? J. Chem. Phys. 2005, 122, 234506. [Google Scholar] [CrossRef]
- Martinez, L.-M.; Angell, C.A. A thermodynamic connection to the fragility of glass-forming liquids. Nature 2001, 410, 663–667. [Google Scholar] [CrossRef]
- Wang, L.-M.; Angell, C.A. Response to “Comment on ‘Direct determination of the fragility indices of glassforming liquids by differential scanning calorimetry: Kinetic versus thermodynamic fragilities’”. J. Chem. Phys. 2003, 118, 10353–10355. [Google Scholar] [CrossRef]
- Wang, L.-M.; Angell, C.A.; Richert, R. Fragility and thermodynamics in nonpolymeric glass-forming liquids. J. Chem. Phys. 2006, 125, 074505. [Google Scholar] [CrossRef]
- Wang, L.-M.; Mauro, J.C. An upper limit to kinetic fragility in glass-forming liquids. J. Chem. Phys. 2011, 134, 044522. [Google Scholar] [CrossRef]
- Wang, L.-M.; Richert, R. Primary and secondary relaxation time dispersions in fragile supercooled liquids. Phys. Rev. B 2007, 76, 064201. [Google Scholar] [CrossRef]
- Wang, L.-M.; Richert, R. Measuring the configurational heat capacity of liquids. Phys. Rev. Lett. 2007, 99, 185701. [Google Scholar] [CrossRef]
- Karmakar, S.; Dasgupta, C.; Sastry, S. Growing length and time scales in glass-forming liquids. Proc. Natl. Acad. Sci. USA 2009, 106, 3675–3679. [Google Scholar] [CrossRef]
- Dasgupta, C.; Indrani, A.V.; Ramaswamy, S.; Phani, M.K. Is There a growing correlation length near the glass transition? Europhys. Lett. 1991, 15, 307–312. [Google Scholar] [CrossRef]
- Berthier, L.; Biroli, G.; Bouchaud, J.-P.; Cipelletti, L.; van Saarloos, W. (Eds.) Dynamical Heterogeneities in Glasses, Colloids, and Granular Media; Oxford University Press: Oxford, UK, 2011. [Google Scholar]
- Berthier, L.; Biroli, G.; Bouchaud, J.-P.; Jack, R.L. Overview of different characterizations of dynamic heterogeneity. In Dynamical Heterogeneities in Glasses, Colloids, and Granular Media; Berthier, L., Biroli, G., Bouchaud, J.-P., Cipelletti, L., van Saarloos, W., Eds.; Oxford University Press: Oxford, UK, 2011. [Google Scholar]
- Glotzer, S.G.; Novikov, V.N.; Schroeder, T.B. Time-dependent, four-point density correlation function description of dynamical heterogeneity and decoupling in supercooled liquids. J. Chem. Phys. 2000, 112, 509–512. [Google Scholar] [CrossRef]
- Tracht, U.; Wilhelm, M.; Heuer, A.; Feng, H.; Schmidt-Rohr, K.; Spiess, H.W. Length scale of dynamic heterogeneities at the glass transition determined by multidimensional nuclear magnetic resonance. Phys. Rev. Lett. 1998, 81, 2727–2730. [Google Scholar] [CrossRef]
- Qiu, X.H.; Ediger, M.D. Length scale of dynamic heterogeneity in supercooled d-sorbitol: Comparison to model predictions. J. Phys. Chem. B 2003, 107, 459–464. [Google Scholar]
- Berthier, L.; Biroli, G.; Bouchaud, J.-P.; Cipelletti, L.; El Masri, D.; L’Hôte, D.; Ladieu, F.; Pierno, M. Direct experimental evidence of a growing length scale accompanying the glass transition. Science 2005, 310, 1797–1800. [Google Scholar] [CrossRef]
- Dalle-Ferrie, C.; Thibierge, C.; Alba-Simionesco, C.; Berthier, L.; Biroli, G.; Bouchaud, J.-P.; Ladieu, F.; L’Hôte, D.; Tarjus, G. Spatial correlations in the dynamics of glassforming liquids: Experimental determination of their temperature dependence. Phys. Rev. E 2007, 76, 041510. [Google Scholar] [CrossRef] [PubMed]
- Hong, L.; Novikov, V.N.; Sokolov, A.P. Is there a connection between fragility of glass-forming systems and dynamic heterogeneity/cooperativity? J. Non-Cryst. Solids 2011, 357, 351–356. [Google Scholar] [CrossRef]
- Hong, L.; Gujrati, P.D.; Novikov, V.N.; Sokolov, A.P. Molecular cooperativity in the dynamics of glass forming systems: A new insight. J. Chem. Phys. 2009, 131, 194511. [Google Scholar] [CrossRef] [PubMed]
- Jäckle, J. Low frequency Raman scattering in glasses. In Amorphous Solids: Low-Temperature Properties; Phillips, W.A., Ed.; Springer: Berlin/Heidelberg, Germany, 1981; pp. 135–160. [Google Scholar]
- Buchenau, U.; Prager, M.; Nücker, N.; Dianoux, A.J.; Ahmad, N.; Phillips, W.A. Low-frequency modes in vitreous silica. Phys. Rev. B 1986, 34, 5665–5673. [Google Scholar] [CrossRef] [PubMed]
- Courtens, E.; Foret, M.; Hehlen, B.; Vacher, R. The vibrational modes of glasses. Solid State Commun. 2001, 117, 187–200. [Google Scholar] [CrossRef]
- Marruzzo, A.; Schirmacher, W.; Fratalocchi, A.; Ruocco, G. Heterogeneous shear elasticity of glasses: The origin of the boson peak. Sci. Rep. 2013, 3, 1407. [Google Scholar] [CrossRef]
- Parisi, G. On the origin of the boson peak. J. Phys. Condens. Matter 2003, 15, S765–S774. [Google Scholar] [CrossRef]
- Duval, E.; Boukenter, A.; Achibat, T. Vibrational dynamics and the structure of glasses. J. Phys. Condens. Matter 1990, 2, 10227–10234. [Google Scholar] [CrossRef]
- Sokolov, A.P.; Kisliuk, A.; Soltwisch, M.; Quitmann, D. Medium-range order in glasses: Comparison of raman and diffraction measurements. Phys. Rev. Lett. 1992, 69, 1540–1543. [Google Scholar] [CrossRef]
- Schirmacher, W.; Schmid, B.; Tomaras, C.; Viliani, G.; Baldi, G.; Ruocco, G.; Scopigno, T. Vibrational excitations in systems with correlated disorder. Phys. Stat. Solidi C 2008, 5, 862–866. [Google Scholar] [CrossRef]
- Fragiadakis, D.; Casalini, R.; Roland, C.M. Comparing dynamic correlation lengths from an approximation to the four-point dynamic susceptibility and from the picosecond vibrational dynamics. Phys. Rev. E 2011, 84, 042501. [Google Scholar] [CrossRef]
- Crauste-Thibierge, C.; Brun, C.; Ladieu, F.; L’Hôte, D.; Biroli, G.; Bouchaud, J.-P. Evidence of growing spatial correlations at the glass transition from nonlinear response experiments. Phys. Rev. Lett. 2010, 104, 165703. [Google Scholar] [CrossRef]
- Crauste-Thibierge, C.; Brun, C.; Ladieu, F.; L’Hôte, D.; Biroli, G.; Bouchaud, J.-P. Nonlinear susceptibility measurements in a supercooled liquid close to Tg: Growth of the correlation length and possible critical behavior. J. Non-Cryst. Solids 2011, 357, 279–281. [Google Scholar] [CrossRef]
- Brun, C.; Ladieu, F.; L’Hôte, D.; Tarzia, M.; Biroli, G.; Bouchaud, J.-P. Nonlinear dielectric susceptibilities: Accurate determination of the growing correlation volume in a supercooled liquid. Phys. Rev. B 2011, 84, 104204. [Google Scholar] [CrossRef]
- Brun, C.; Ladieu, F.; L’Hôte, D.; Biroli, G.; Bouchaud, J.-P. Evidence of growing spatial correlations during the aging of glassy glycerol. Phys. Rev. Lett. 2012, 109, 175702. [Google Scholar] [CrossRef]
- Bauer, T.; Lunkenheimer, P.; Loidl, A. Cooperativity and the freezing of molecular motion at the glass transition. Phys. Rev. Lett. 2013, 111, 225702. [Google Scholar] [CrossRef]
- Bauer, T.; Lunkenheimer, P.; Kastner, S.; Loidl, A. Nonlinear dielectric response at the excesswing of glass-forming liquids. Phys. Rev. Lett. 2013, 110, 107603. [Google Scholar] [CrossRef]
- Casalini, R.; Fragiadakis, D.; Roland, C.M. Dynamic correlation length scales under isochronal conditions. J. Chem. Phys. 2015, 142, 064504. [Google Scholar] [CrossRef]
- Michl, M.; Bauer, T.; Lunkenheimer, P.; Loidl, A. Cooperativity and heterogeneity in plastic crystals studied by nonlinear dielectric spectroscopy. Phys. Rev. Lett. 2015, 114, 067601. [Google Scholar] [CrossRef]
- Bauer, T.; Michl, M.; Lunkenheimer, P.; Loidl, A. Nonlinear dielectric response of Debye, α, and β relaxation in 1-propanol. J. Non-Cryst. Solids 2015, 407, 66–71. [Google Scholar] [CrossRef][Green Version]
- Samanta, S.; Richert, R. Limitations of heterogeneous models of liquid dynamics: Very slow rate exchange in the excess wing. J. Chem. Phys. 2014, 140, 054508. [Google Scholar] [CrossRef]
- Novikov, V.N.; Schweizer, K.S.; Sokolov, A.P. Coherent neutron scattering and collective dynamics on mesoscale. J. Chem. Phys. 2013, 138, 164508. [Google Scholar] [CrossRef]
- Voylov, D.N.; Griffin, P.J.; Mercado, B.; Keum, J.K.; Nakanishi, M.; Novikov, V.N.; Sokolov, A.P. Correlation between temperature variations of static and dynamic properties in glass forming liquids. Phys. Rev. E 2016, 94, 060603(R). [Google Scholar] [CrossRef]
- Roland, C.M.; Hensel-Bielowka, S.; Paluch, M.; Casalini, R. Supercooled dynamics of glass-forming liquids and polymers under hydrostatic pressure. Rep. Progr. Phys. 2005, 68, 1405–1478. [Google Scholar] [CrossRef]
- Floudas, G.; Paluch, M.; Grzybowski, A.; Ngai, K.L. Molecular dynamics of glass-forming systems: Effects of pressure. In Advances in Dielectrics; Kremer, F., Ed.; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Grzybowski, A.; Paluch, M. Universality of density scaling. In The Scaling of Relaxation Processes; Kremer, F., Loidl, A., Eds.; Springer International Publishing AG: Berlin/Heidelberg, Germany, 2018; pp. 77–120. [Google Scholar]
- Roland, C.M.; Casalini, R. Comment on: “Disentangling density and temperature effects in the viscous slowing down of glass forming liquids”. J. Chem. Phys. 2004, 121, 11503–11504. [Google Scholar] [CrossRef]
- Alba-Simionseco, C.; Cailliaux, A.; Alegria, A.; Tarjus, G. Scaling out the density dependence of the α relaxation in glass-forming polymers. Europhys. Lett. 2004, 68, 58–64. [Google Scholar] [CrossRef]
- Hong, L.; Novikov, V.N.; Sokolov, A.P. Dynamic heterogeneities, boson peak and activation volume in glass-forming liquids Phys. Rev. E 2011, 83, 061508. [Google Scholar]
- Casalini, R.; Roland, C.M. Thermodynamical scaling of the glass transition dynamics. Phys. Rev. E 2004, 69, 062501. [Google Scholar] [CrossRef]
- Casalini, R.; Roland, C.M. Excess wing in the dielectric loss spectra of propylene glycol oligomers at elevated pressure. Phys. Rev. B 2004, 69, 094202. [Google Scholar] [CrossRef]
- Dreyfus, C.; Le Grand, A.; Gapinski, J.; Steffen, W.; Patkowski, A. Scaling the α-relaxation time of supercooled fragile organic liquids. Eur. J. Phys. 2004, 42, 309–319. [Google Scholar] [CrossRef]
- Longuet-Higgins, H.C.; Widom, B. A rigid sphere model for the melting of argon. Mol. Phys. 1964, 8, 549–556. [Google Scholar] [CrossRef]
- Hoover, W.H.; Ross, M. Statistical theories of melting. Contemp. Phys. 1971, 12, 339–356. [Google Scholar] [CrossRef]
- Speedy, R.J. The energy–density landscape for soft spheres. J. Phys. Condens. Matter 2003, 15, S1243–S1251. [Google Scholar] [CrossRef]
- Roland, C.M.; Feldman, J.L.; Casalini, R. Scaling of the local dynamics and the intermolecular potential. J. Non-Cryst. Solids 2006, 352, 4895–4899. [Google Scholar] [CrossRef]
- Casalini, R.; Roland, C.M. Determination of the thermodynamic scaling exponent for relaxation in liquids from static ambient-pressure quantities. Phys. Rev. Lett. 2014, 113, 085701. [Google Scholar] [CrossRef]
- Casalini, R.; Roland, C.M. Scaling of the segmental relaxation times of polymers and its relation to the thermal expansivity. Colloid Polym. Sci. 2004, 283, 107–110. [Google Scholar] [CrossRef]
- Boyer, R.F.; Spencer, R.S. Thermal expansion and second-order transition effects in high polymers: Part I. Experimental results. J. Appl. Phys. 1944, 15, 398–405. [Google Scholar] [CrossRef]
- Puosi, F.; Leporini, D. Communication: Correlation of the instantaneous and the intermediate-time elasticity with the structural relaxation in glassforming systems. J. Chem. Phys. 2012, 136, 041104. [Google Scholar] [CrossRef]
- Dyre, J.C.; Wang, W.H. The instantaneous shear modulus in the shoving model. J. Chem. Phys. 2012, 136, 224108. [Google Scholar] [CrossRef]
- Xu, B.; McKenna, G.B. Evaluation of the Dyre shoving model using dynamic data near the glass temperature. J. Chem. Phys. 2011, 134, 124902. [Google Scholar]
- Hecksher, T.; Dyre, J.C. A review of experiments testing the shoving model. J. Non-Cryst. Solids 2015, 407, 14–22. [Google Scholar] [CrossRef]
- Novikov, V.N.; Sokolov, A.P. Poisson’s ratio and fragility of glass-forming liquids. Nature 2004, 431, 961–963. [Google Scholar] [CrossRef]
- Novikov, V.N.; Sokolov, A.P. Correlation of fragility and Poisson’s ratio: Difference between metallic and non-metallic glassformers. Phys. Rev. B 2006, 74, 064203. [Google Scholar] [CrossRef]
- Yannopoulos, S.N.; Johari, G.P. Poisson’s ratio and liquid’s fragility. Nature 2006, 442, E7–E8. [Google Scholar] [CrossRef]
- Ahmed, M.S.; Chao, J.; Crossley, J.; Hossain, M.S.; Walker, S. Cooperative motion in some simple hydrocarbons. J. Chem. Soc. Faraday Trans. II 1984, 280, 1047–1054. [Google Scholar] [CrossRef]
- Köplinger, J.; Kasper, G.; Hunklinger, S. Glass transition in chlorobenzene–decalin under pressure. J. Chem. Phys. 2000, 113, 4701–4706. [Google Scholar] [CrossRef]
- Whitaker, K.R.; Scifo, D.J.; Ediger, M.D.; Ahrenberg, M.; Schick, C. Highly stable glasses of cis-decalin and cis/trans-decalin mixtures. J. Phys. Chem. B 2013, 117, 12724–12733. [Google Scholar] [CrossRef]
- Sokolov, A.P.; Rössler, E.; Kisliuk, A.; Quitmann, D. Dynamics of strong and fragile glass formers: Differences and correlation with low-temperature properties. Phys. Rev. Lett. 1993, 71, 2062–2065. [Google Scholar] [CrossRef]
- Sokolov, A.P.; Calemczuk, R.; Salce, B.; Kisliuk, A.; Quitmann, D.; Duval, E. Low-Temperature Anomalies in Strong and Fragile Glass Formers. Phys. Rev. Lett. 1997, 78, 2405–2408. [Google Scholar] [CrossRef]
- Scopigno, T.; Ruocco, G.; Sette, F.; Monako, G. Is the fragility of a liquid embedded in the properties of its glass? Science 2003, 302, 849–852. [Google Scholar] [CrossRef]
- Dyre, J.C. Heirs of liquid treasures. Nat. Mat. 2004, 3, 749–750. [Google Scholar] [CrossRef]
- Ngai, K.L.; Bao, L.-R.; Yee, A.F.; Soles, C.L. Correlation of positron annihilation and other dynamic properties in small molecule glass-forming substances. Phys. Rev. Lett. 2001, 87, 215901. [Google Scholar] [CrossRef]
- Ottochian, A.; Leporini, D. Universal scaling between structural relaxation and caged dynamics in lass-forming systems: Free volume and time scales. J. Non-Cryst. Solids 2011, 357, 298–301. [Google Scholar] [CrossRef]
- Pazmiño Betancourt, B.A.; Hanakata, P.Z.; Starr, F.W.; Douglas, J.F. Quantitative relations between cooperative motion, emergent elasticity, and free volume in model glass-forming polymer materials. Proc. Natl. Acad. Sci. USA 2015, 112, 2966–2971. [Google Scholar] [CrossRef]
- Larini, L.; Ottochian, A.; De Michele, C.; Leporini, D. Universal scaling between structural relaxation and vibrational dynamics in glass-forming liquids and polymers. Nat. Phys. 2008, 4, 42–45. [Google Scholar] [CrossRef]
- Ottochian, A.; De Michele, C.; Leporini, D. Universal divergenceless scaling between structural relaxation and caged dynamics in glass-forming systems. J. Chem. Phys. 2009, 131, 224517. [Google Scholar] [CrossRef]
- Simmons, D.S.; Cicerone, M.T.; Zhong, Q.; Tyagi, M.; Douglas, J.F. Generalized localization model of relaxation in glass-forming liquids. Soft Matter 2012, 8, 11455–11461. [Google Scholar] [CrossRef]
- Novikov, V.N. Vibration anharmonicity and fast relaxation in the region of glass transition. Phys. Rev. B 1998, 58, 8367–8378. [Google Scholar] [CrossRef]
- Gilroy, K.S.; Phillips, W.A. An asymmetric double-well potential model for structural relaxation processes in amorphous materials. Philos. Mag. B 1981, 43, 735–746. [Google Scholar] [CrossRef]
- Surovtsev, N.V.; Wiedersich, J.; Novikov, V.N.; Sokolov, A.P.; Rössler, E. Light-scattering spectra of fast relaxation in glasses. Phys. Rev. B 1998, 58, 14888–14891. [Google Scholar] [CrossRef]
- Kojima, S.; Novikov, V.N. Correlation of temperature dependence of quasielastic-light-scattering intensity and α-relaxation time. Phys. Rev. B 1996, 54, 222–227. [Google Scholar] [CrossRef] [PubMed]
- Novikov, V.N.; Sokolov, A.P.; Strube, B.; Surovtsev, N.V.; Duval, E.; Mermet, A. Connection between quasielastic Raman scattering and free volume in polymeric glasses and supercooled liquids. J. Chem. Phys. 1997, 107, 1057–1065. [Google Scholar] [CrossRef]
- Novikov, V.N. Phonon-density fluctuations and fast relaxation in glasses. Phys. Rev. B 1997, 55, R14685–R14688. [Google Scholar] [CrossRef]
- Vacher, R.; Curtens, E.; Foret, M. Anharmonic versus relaxational sound damping in glasses. II. Vitreous silica. Phys. Rev. B 2005, 72, 214205. [Google Scholar] [CrossRef]
- Buchenau, U.; Wischnewski, A. Fragility and compressibility at the glass transition. Phys. Rev. B 2004, 70, 092201. [Google Scholar] [CrossRef]
- Scopigno, T.; Cangialosi, D.; Ruocco, G. Universal relation between viscous flow and fast dynamics in glass-forming materials. Phys. Rev. B 2010, 81, 100202(R). [Google Scholar] [CrossRef]
- Brodin, A.; Börjesson, L.; Engberg, D.; Torell, L.M.; Sokolov, A.P. Relaxational and vibrational dynamics in the glass-transition range of a strong glass former B2O3. Phys. Rev. B 1996, 53, 11511–11520. [Google Scholar] [CrossRef]
- Ferry, J.D. Viscoelastic Properties of Polymers; Wiley: New York, NY, USA, 1980. [Google Scholar]
- Ngai, K.L.; Plazek, D.J. Identification of different modes of molecular motion in polymers that cause thermorheological complexity. Rubber Chem. Technol. 1995, 68, 376–434. [Google Scholar] [CrossRef]
- Ding, Y.; Novikov, V.N.; Sokolov, A.P.; Cailliaux, A.; Dalle-Ferrier, C.; Alba-Simionesco, C.; Frick, B. Influence of molecular weight on fast dynamics and fragility of polymers. Macromolecules 2004, 37, 9264–9272. [Google Scholar] [CrossRef]
- Kunal, K.; Robertson, C.G.; Pawlus, S.; Hahn, S.F.; Sokolov, A.P. Role of chemical structure in fragility of polymers: A qualitative picture. Macromolecules 2008, 41, 7232–7238. [Google Scholar] [CrossRef]
- Roland, C.M.; McGrath, K.J.; Casalini, R. Volume effects on the glass transition dynamics. J. Non-Cryst. Solids 2006, 352, 4910–4914. [Google Scholar] [CrossRef][Green Version]
- Casalini, R.; Roland, C.M.; Capaccioli, S. Effect of chain length on fragility and thermodynamic scaling of the local segmental dynamics in polymethylmethacrylate. J. Chem. Phys. 2007, 126, 184903. [Google Scholar] [CrossRef]
- Kunal, K.; Paluch, M.; Roland, C.M.; Puskas, J.E.; Chen, Y.; Sokolov, A.P. Polyisobutylene: A most unusual polymer. J. Pol. Sci. B Pol. Phys. 2008, 46, 1390–1399. [Google Scholar] [CrossRef]
- Andreozzi, L.; Autiero, C.; Faetti, M.; Giordano, M.; Zulli, F. Dynamics, fragility, and glass transition of low-molecular-weight linear homopolymers. Phil. Mag. 2008, 88, 4151–4159. [Google Scholar] [CrossRef]
- Cangialosi, D.; Alegrıa, A.; Colmenero, J. Relationship between dynamics and thermodynamics in glass-forming polymers. Europhys. Lett. 2005, 70, 614–620. [Google Scholar] [CrossRef]
- Sokolov, A.P.; Novikov, V.N.; Ding, Y. Why many polymers are so fragile. J. Phys. Condens. Matter 2007, 19, 205116. [Google Scholar] [CrossRef]
- Abou Elfadl, A.; Herrmann, A.; Hintermeyer, J.; Petzold, N.; Novikov, V.N.; Rössler, E.A. Molecular weight dependence of fragility in polymers. Macromolecules 2009, 42, 6816. [Google Scholar] [CrossRef]
- Roland, C.M.; Casalini, R. Temperature dependence of local segmental motion in polystyrene and its variation with molecular weight. J. Chem. Phys. 2003, 119, 1838–1842. [Google Scholar] [CrossRef]
- Plazek, D.J.; O’Rourke, V.M. Viscoelastic behavior of low molecular weight polystyrene. J. Polym. Sci. B 1971, 9, 209–243. [Google Scholar] [CrossRef]
- Lin, Y.-H. Glass transition-related thermorheological complexity in polystyrene melts. J. Phys. Condens. Matter 2007, 19, 466101. [Google Scholar] [CrossRef]
- Qin, Q.; McKenna, G.B. Correlation between dynamic fragility and glass transition temperature for different classes of glass forming liquids. J. Non-Cryst. Solids 2006, 352, 2977–2985. [Google Scholar] [CrossRef]
- Ngai, K.L.; Roland, C.M. Chemical structure and intermolecular cooperativity: Dielectric relaxation results. Macromolecules 1993, 26, 6824–6830. [Google Scholar] [CrossRef]
- Agapov, A.L.; Sokolov, A.P. Decoupling ionic conductivity from structural relaxation: A way to solid polymer electrolytes? Macromolecules 2011, 44, 4410–4414. [Google Scholar] [CrossRef]
- Kunal, K. Influence of Chemical Structure and Molecular Weight on Fragility in Polymers. Ph.D. Thesis, The University of Akron, Akron, OH, USA, August 2009. [Google Scholar]
- Stukalin, E.B.; Douglas, J.F.; Freed, K.F. Application of the entropy theory of glass formation to poly (α-olefins). J. Chem. Phys. 2009, 131, 114905. [Google Scholar] [CrossRef]
- Colmenero, J. Are polymers standard glass-forming systems? The role of intramolecular barriers on the glass-transition phenomena of glass-forming polymers. J. Phys. Condens. Matter 2015, 27, 103101. [Google Scholar] [CrossRef]
- Kumar, R.; Goswami, M.; Sumpter, B.G.; Novikov, V.N.; Sokolov, A.P. Effects of backbone rigidity on the local structure and dynamics in polymer melts and glasses. Phys. Chem. Chem. Phys. 2013, 15, 4604–4609. [Google Scholar] [CrossRef]
- Agapov, A.L.; Novikov, V.N.; Hong, T.; Fan, F.; Sokolov, A.P. Surprising temperature scaling of viscoelastic properties in polymers. Macromolecules 2018, 51, 4874–4881. [Google Scholar] [CrossRef]
- Guo, J.; Simon, S. Thermodynamic scaling of polymer dynamics versus T−Tg scaling. J. Chem. Phys. 2011, 135, 074901. [Google Scholar] [CrossRef]
- Ngai, K.L.; Plazek, D.J.; Roland, C.M. On the universality of chain dynamics. Macromolecules 2008, 41, 3925–3929. [Google Scholar] [CrossRef][Green Version]
- Markland, T.E.; Morrone, J.A.; Berne, B.J.; Miyazaki, K.; Rabani, E.; Reichman, D.R. Quantum fluctuations can promote or inhibit glass formation. Nat. Phys. 2011, 7, 134–137. [Google Scholar] [CrossRef]
- Novikov, V.N.; Sokolov, A.P. Role of quantum effects in the glass transition. Phys. Rev. Lett. 2013, 110, 065701. [Google Scholar] [CrossRef] [PubMed]
- Gainaru, C.; Agapov, A.L.; Fuentes-Landete, V.; Amann-Winkel, K.; Nelson, H.; Köster, K.; Kolesnikov, A.I.; Novikov, V.N.; Richert, R.; Böhmer, R.; et al. Anomalously large isotope effect in the glass transition of water. Pros. Natl. Acad. Sci. USA 2014, 111, 17402–17407. [Google Scholar] [CrossRef] [PubMed]
- Agapov, A.L.; Kolesnikov, A.I.; Novikov, V.N.; Richert, R.; Sokolov, A.P. Quantum effects in the dynamics of deeply supercooled water. Phys. Rev. E 2015, 91, 022312. [Google Scholar] [CrossRef] [PubMed]
- Habershon, S.; Markland, T.E.; Manolopoulos, D.E. Competing quantum effects in the dynamics of a flexible water model. J. Chem. Phys. 2009, 131, 024501. [Google Scholar] [CrossRef]
- Miller, T.F.; Manolopoulos, D.E.J. Quantum diffusion in liquid water from ring polymer molecular dynamics. Chem. Phys. 2005, 123, 154504. [Google Scholar]
- Kaatze, U. Dielectric relaxation of H2O/D2O mixtures. Chem. Phys. Lett. 1993, 203, 1–4. [Google Scholar] [CrossRef]
- Okada, K.; Yao, M.; Hiejima, Y.; Kohno, H.; Kajihara, Y. Dielectric relaxation of water and heavy water in the whole fluid phase. J. Chem. Phys. 1999, 110, 3026–3036. [Google Scholar] [CrossRef]
- Buchner, R.; Barthel, J.; Stauber, J. The dielectric relaxation of water between 0 °C and 35 °C. Chem. Phys. Lett. 1999, 306, 57–63. [Google Scholar] [CrossRef]
- Angell, C.A. Water II is a “strong” liquid. J. Phys. Chem. 1993, 97, 6339–6341. [Google Scholar] [CrossRef]
- Richert, R. Dynamics of nanoconfined supercooled liquids. Ann. Rev. Phys. Chem. 2011, 62, 65–84. [Google Scholar] [CrossRef]





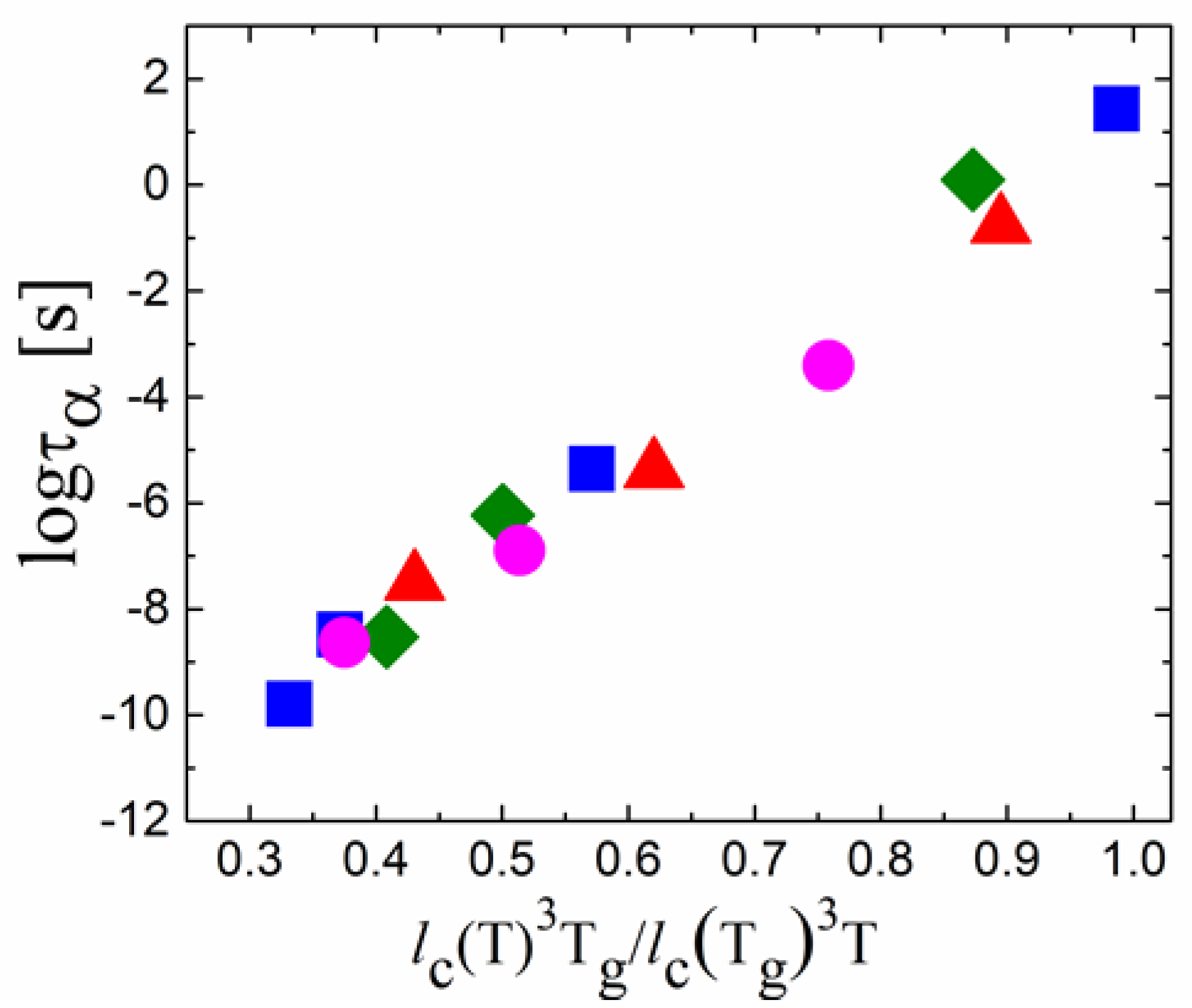

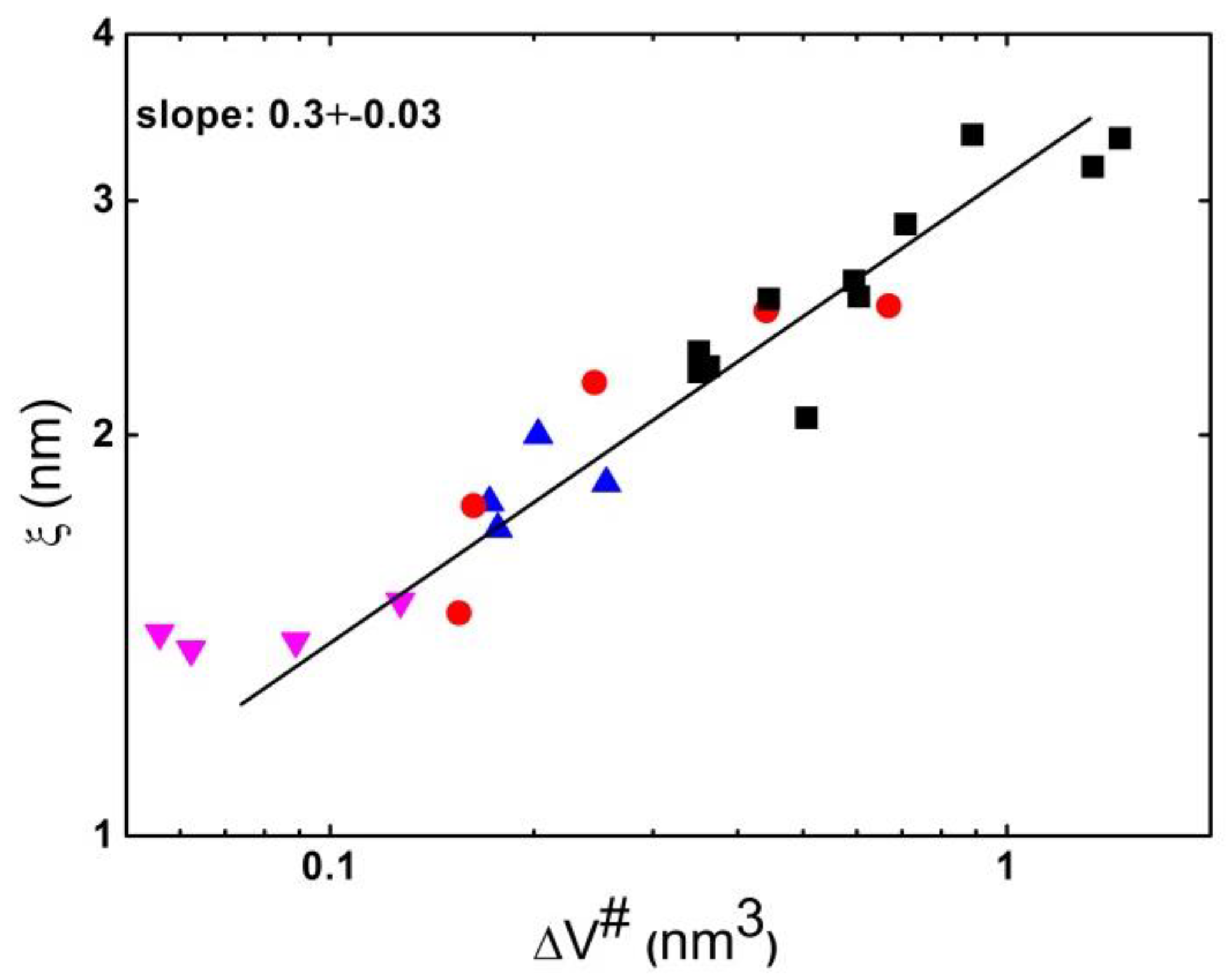

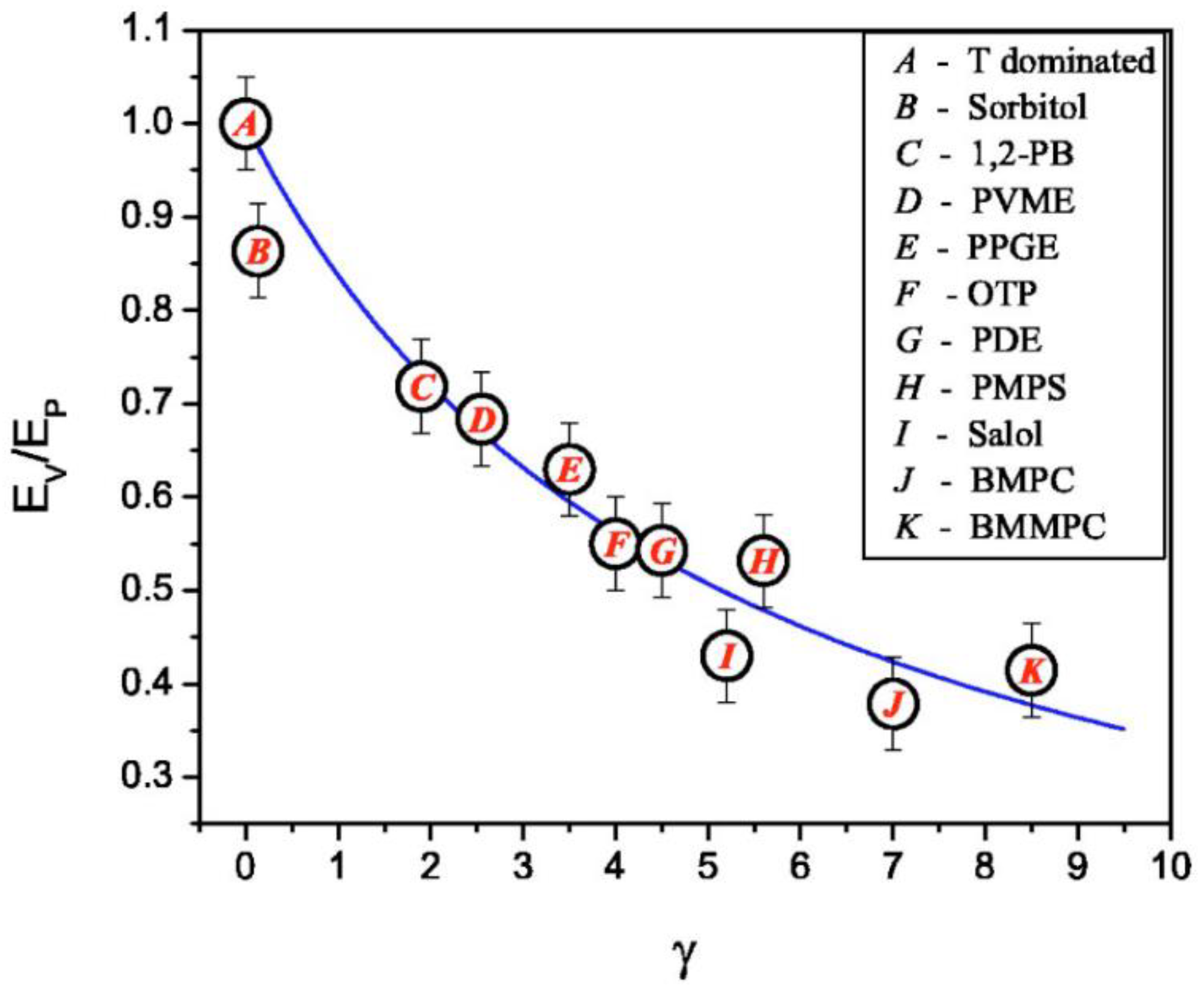
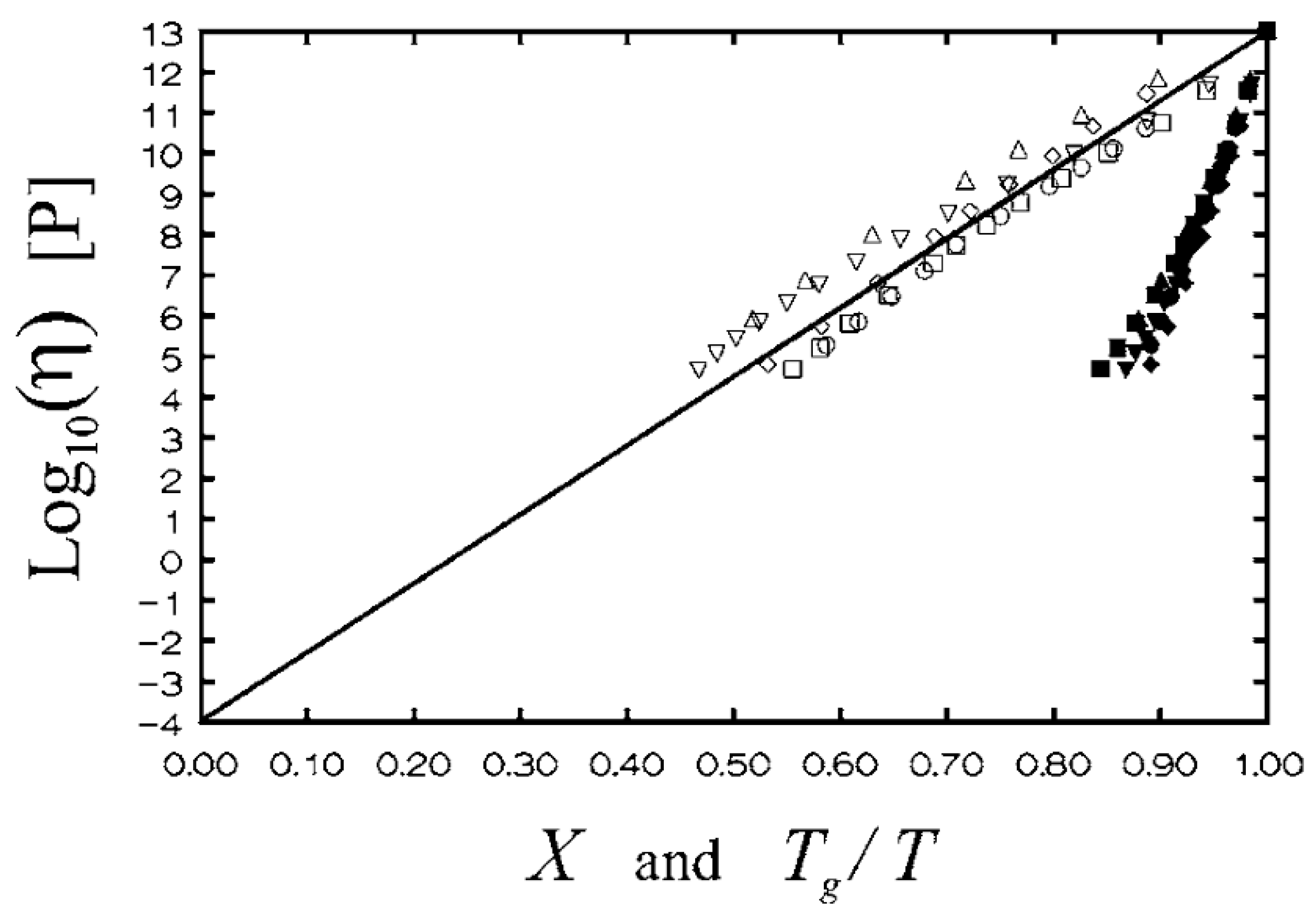


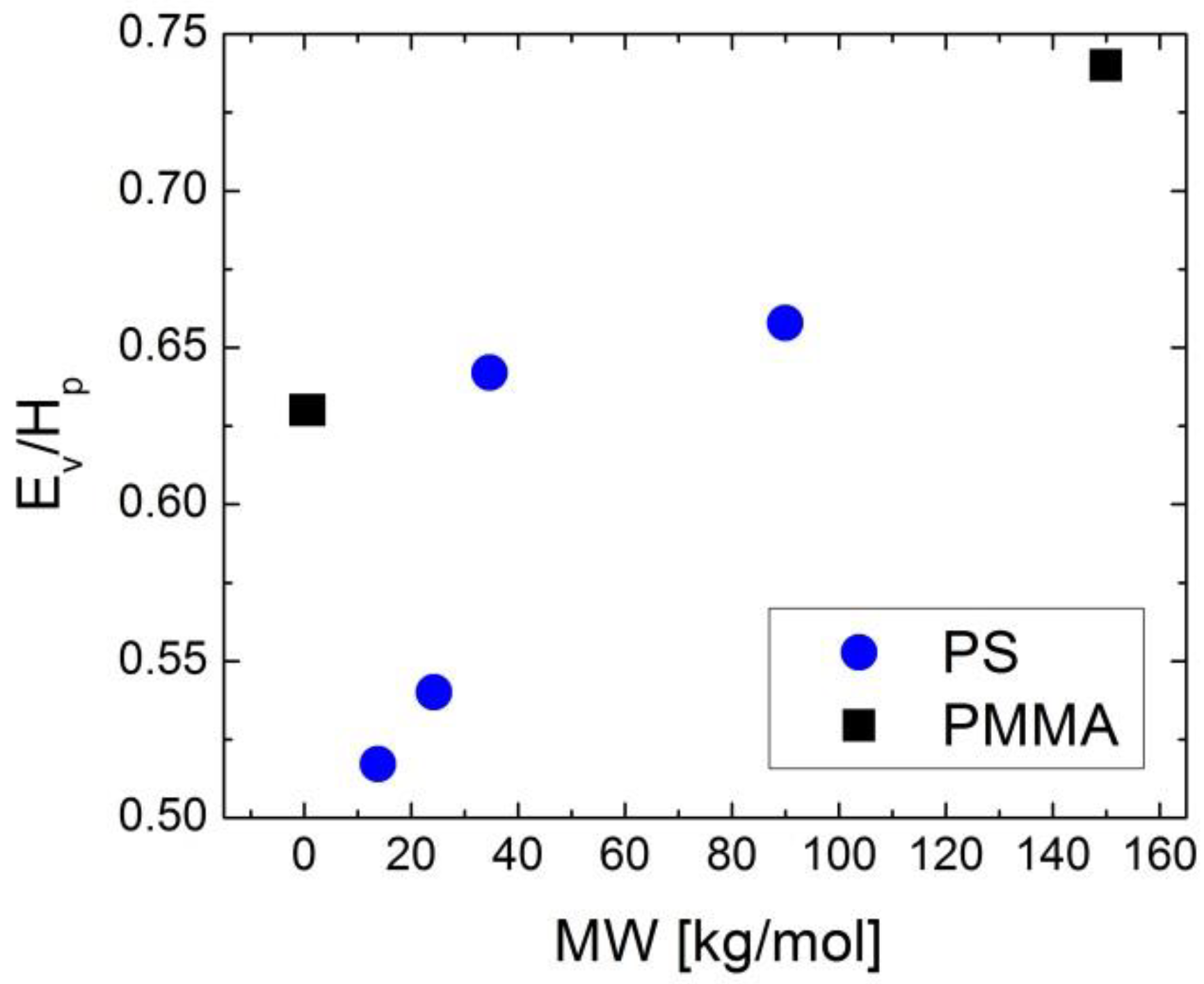
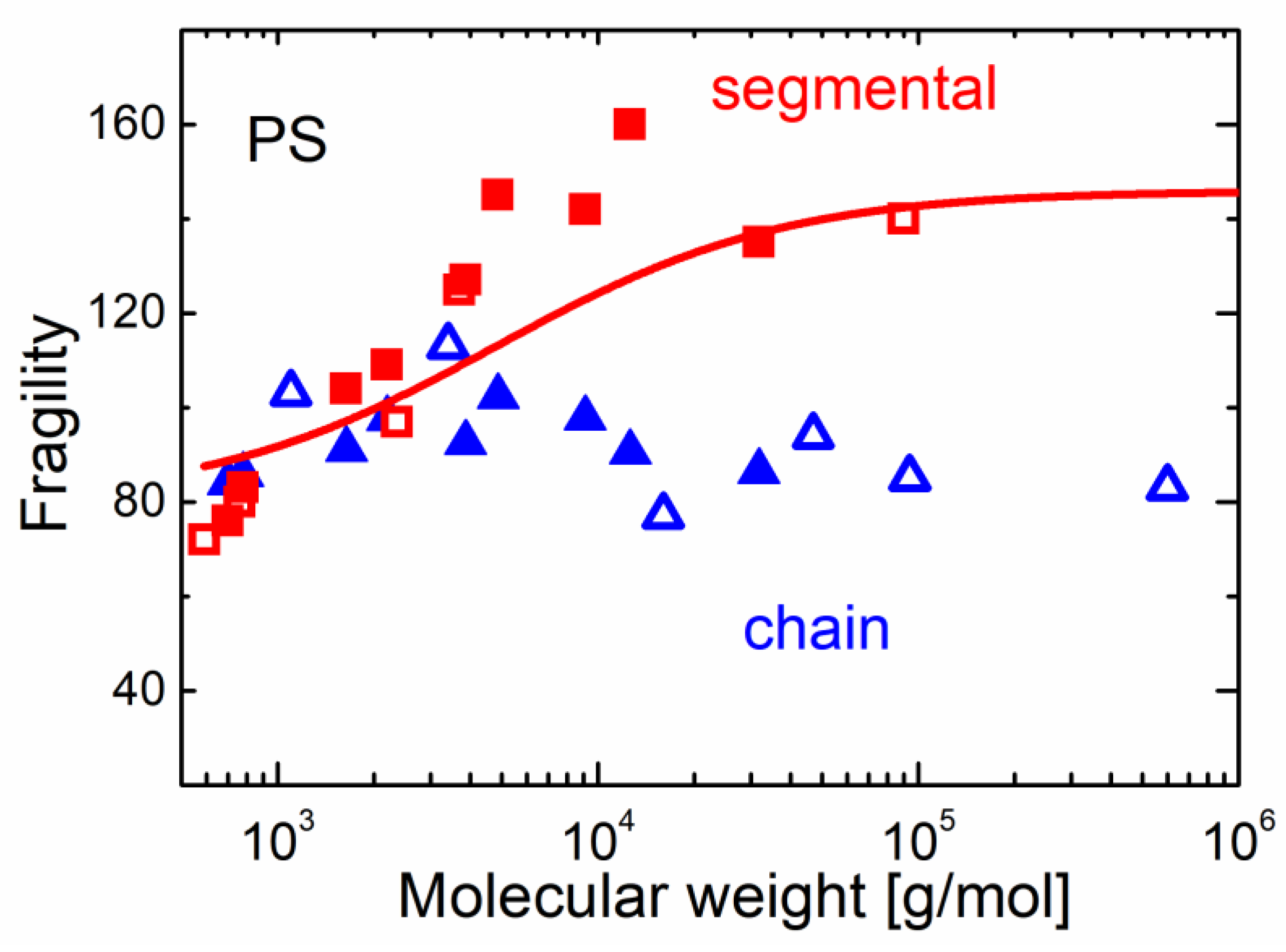
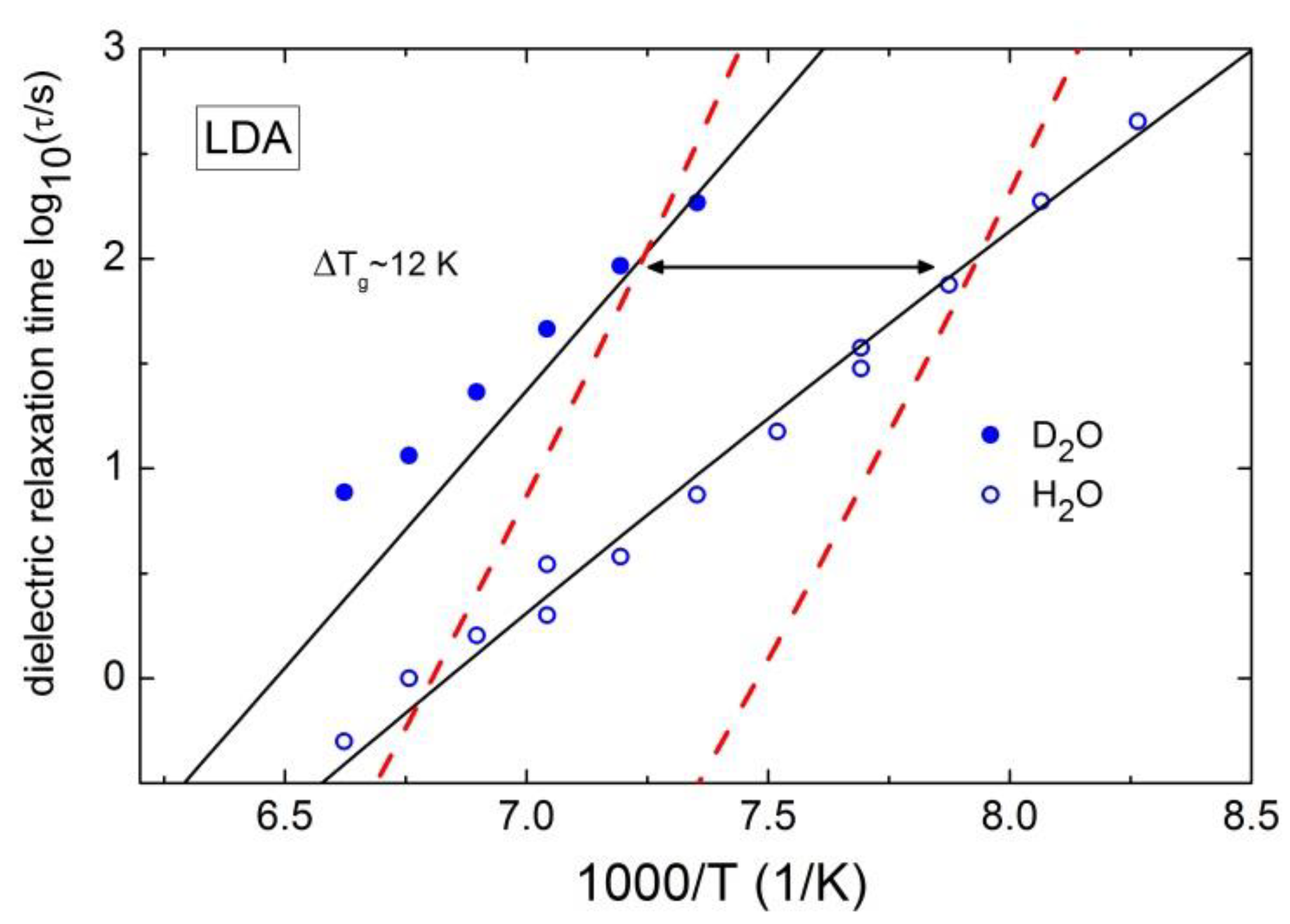
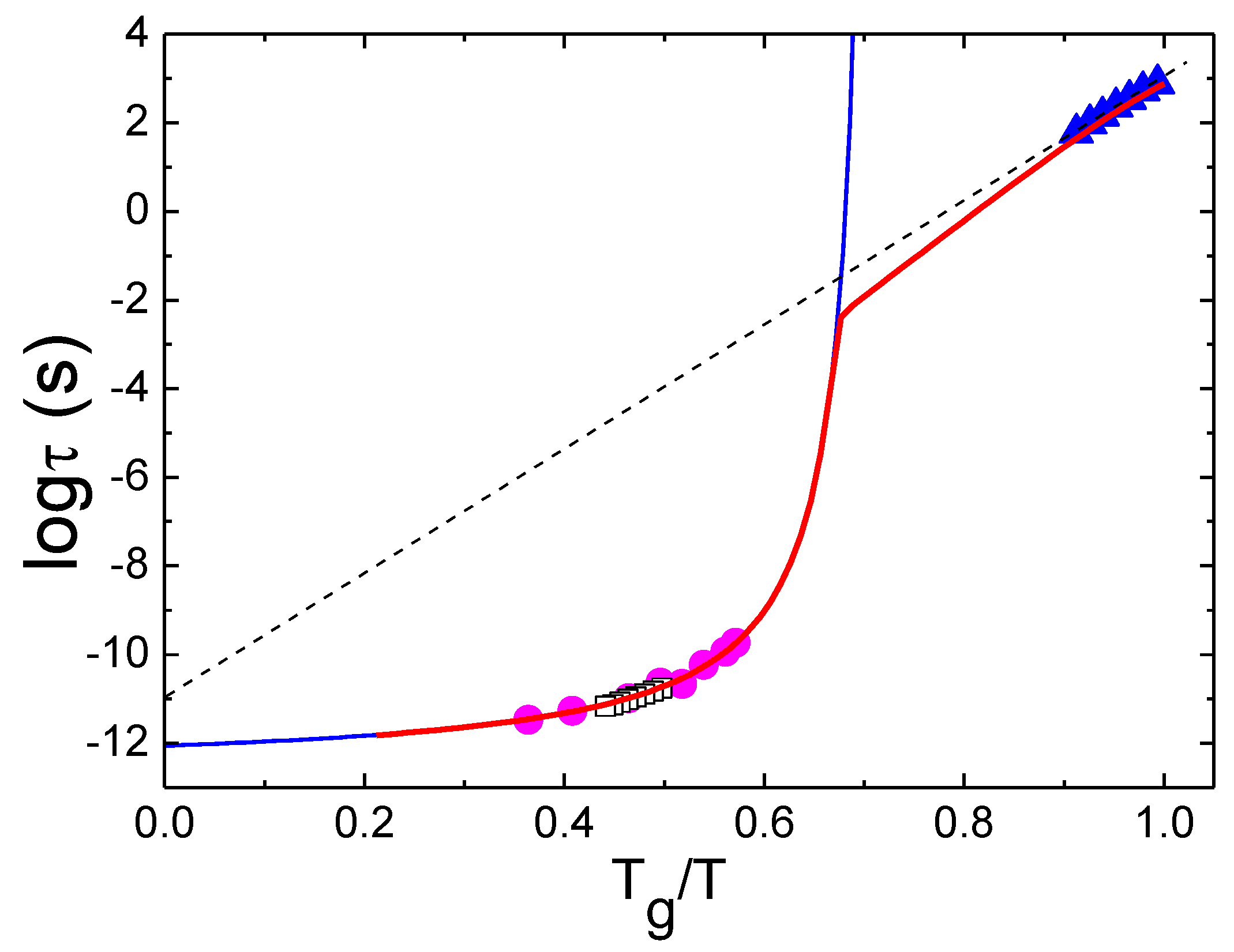
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Novikov, V.N.; Sokolov, A.P. Temperature Dependence of Structural Relaxation in Glass-Forming Liquids and Polymers. Entropy 2022, 24, 1101. https://doi.org/10.3390/e24081101
Novikov VN, Sokolov AP. Temperature Dependence of Structural Relaxation in Glass-Forming Liquids and Polymers. Entropy. 2022; 24(8):1101. https://doi.org/10.3390/e24081101
Chicago/Turabian StyleNovikov, Vladimir N., and Alexei P. Sokolov. 2022. "Temperature Dependence of Structural Relaxation in Glass-Forming Liquids and Polymers" Entropy 24, no. 8: 1101. https://doi.org/10.3390/e24081101
APA StyleNovikov, V. N., & Sokolov, A. P. (2022). Temperature Dependence of Structural Relaxation in Glass-Forming Liquids and Polymers. Entropy, 24(8), 1101. https://doi.org/10.3390/e24081101





