Quantum Information of the Aharanov–Bohm Ring with Yukawa Interaction in the Presence of Disclination
Abstract
:1. Introduction
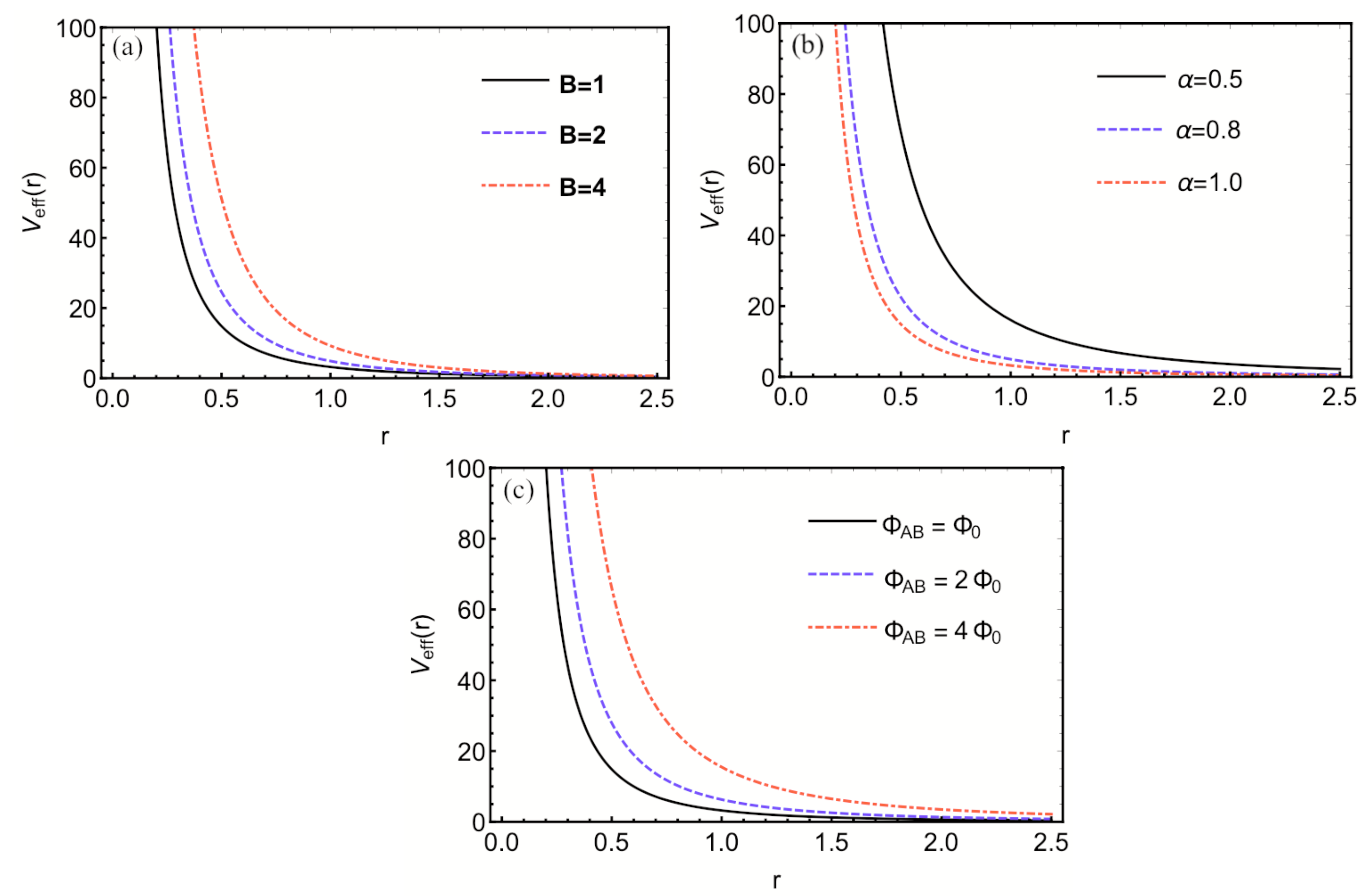
2. Theory and Solutions
3. Shannon’s Entropy
4. Final Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kuroda, T.; Mano, T.; Ochiai, T.; Sanguinetti, S.; Kido, K.S.G.; Koguchi, N. Optical transitions in quantum ring complexes. Phys. Rev. B 2005, 72, 205301. [Google Scholar] [CrossRef]
- Chakraborty, T.; Pietiläinen, P. Electron-electron interaction and the persistent current in a quantum ring. Phys. Rev. B 1994, 50, 8460. [Google Scholar] [CrossRef] [PubMed]
- Ahn, J.C.; Kwak, K.S.; Park, B.H.; Kang, H.Y.; Kim, J.Y.; Kwon, O. Photonic quantum ring. Phys. Rev. Lett. 1999, 82, 536. [Google Scholar] [CrossRef]
- Netto, A.S.; Chesman, C.; Furtado, C. Influence of topology in a quantum ring. Phys. Lett. A 2008, 372, 3894. [Google Scholar] [CrossRef]
- Fomin, V.M. (Ed.) Physics of Quantum Rings, in NanoScience and Technology; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Nowozin, T. Self-Organized Quantum Dots for Memories: Electronic Properties and Carrier Dynamics; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Michler, P. Single Quantum Dots: Fundamentals, Applications, and New Concepts; Springer: Berlin, Germany, 2003; Volume 90. [Google Scholar]
- Cheung, H.F.; Gefen, Y.; Riedel, E.K.; Shih, W.H. Persistent currents in small one-dimensional metal rings. Phys. Rev. B 1988, 37, 6050. [Google Scholar] [CrossRef] [PubMed]
- Meijer, F.E.; Morpurgo, A.F.; Klapwijk, T.M. One-dimensional ring in the presence of Rashba spin-orbit interaction: Derivation of the correct Hamiltonian. Phys. Rev. B 2002, 66, 033107. [Google Scholar] [CrossRef]
- Frustaglia, D.; Richter, K. Spin interference effects in ring conductors subject to Rashba coupling. Phys. Rev. B 2004, 69, 235310. [Google Scholar] [CrossRef]
- Lorke, A.; Luyken, R.J.; Govorov, A.O.; Kotthaus, J.P.; Garcia, J.M.; Petroff, P.M. Spectroscopy of nanoscopic semiconductor rings. Phys. Rev. Lett. 2000, 84, 2223. [Google Scholar] [CrossRef]
- Kettemann, S.; Fulde, P.; Strehlow, P. Correlated persistent tunneling currents in glasses. Phys. Rev. Lett. 1999, 83, 4325. [Google Scholar] [CrossRef]
- Tan, W.C.; Inkson, J.C. Magnetization, persistent currents, and their relation in quantum rings and dots. Phys. Rev. B 1999, 60, 5626. [Google Scholar] [CrossRef]
- Bulaev, D.V.; Geyler, V.A.; Margulis, V.A. Effect of surface curvature on magnetic moment and persistent currents in two-dimensional quantum rings and dots. Phys. Rev. B 2004, 69, 195313. [Google Scholar] [CrossRef]
- Duque, C.M.; Morales, A.L.; Mora-Ramos, M.E.; Duque, C.A. Optical nonlinearities associated to applied electric fields in parabolic two-dimensional quantum rings. J. Lumin. 2013, 143, 81. [Google Scholar] [CrossRef]
- Nowak, M.P.; Szafran, B. Spin-orbit coupling effects in two-dimensional circular quantum rings: Elliptical deformation of confined electron density. Phys. Rev. B 2009, 80, 195319. [Google Scholar] [CrossRef]
- Oliveira, R.R.S.; Filho, A.A.A.; Lima, F.C.E.; Maluf, R.V.; Almeida, C.A.S. Thermodynamic properties of an Aharonov-Bohm quantum ring. Eur. Phys. J. Plus 2019, 134, 495. [Google Scholar] [CrossRef]
- Russo, S.; Oostinga, J.B.; Wehenkel, D.; Heersche, H.B.; Sobhani, S.S.; Vandersypen, L.M.K.; Morpurgo, A.F. Observation of Aharonov-Bohm conductance oscillations in a graphene ring. Phys. Rev. B 2008, 77, 085413. [Google Scholar] [CrossRef]
- Yeyati, A.L.; Büttiker, M. Aharonov-Bohm oscillations in a mesoscopic ring with a quantum dot. Phys. Rev. B 1995, 52, R14360(R). [Google Scholar] [CrossRef]
- Hansen, A.E.; Kristensen, A.; Pedersen, S.; Sorensen, C.B.; Lindelof, P.E. Mesoscopic decoherence in Aharonov-Bohm rings. Phys. Rev. B 2001, 64, 045327. [Google Scholar] [CrossRef]
- Reulet, B.; Ramin, M.; Bouchiat, H.; Mailly, D. Dynamic response of isolated aharonov-bohm rings coupled to an electromagnetic resonator. Phys. Rev. Lett. 1995, 75, 124. [Google Scholar] [CrossRef]
- Aeberhard, U.; Wakabayashi, K.; Sigrist, M. Effect of spin-orbit coupling on zero-conductance resonances in asymmetrically coupled one-dimensional rings. Phys. Rev. B 2005, 72, 075328. [Google Scholar] [CrossRef]
- Shelykh, I.A.; Bagraev, N.T.; Galkin, N.G.; Klyachkin, L.E. Interplay of h/e and h/2e oscillations in gate-controlled Aharonov-Bohm rings. Phys. Rev. B 2005, 71, 113311. [Google Scholar] [CrossRef]
- Ahmed, F. Relativistic motions of spin-zero quantum oscillator field in a global monopole space-time with external potential and AB-effect. Sci. Rep. 2022, 12, 8794. [Google Scholar] [CrossRef] [PubMed]
- Zare, S.; Hassanabadi, H.; Guvendi, A.; Chung, W.S. On the interaction of a Cornell-type nonminimal coupling with the scalar field under the background of topological defects. Int. J. Mod. Phys. A 2022, 37, 2250033. [Google Scholar] [CrossRef]
- Shikakhwa, M.S.; Chair, N. Constructing Hermitian Hamiltonians for spin zero neutral and charged particles on a curved surface: Physical approach. Eur. Phys. J. Plus 2022, 137, 560. [Google Scholar] [CrossRef]
- Yukawa, H. On the interaction of elementary particles. I. Proc. Phys. Math. Soc. Jpn. 1935, 17, 48. [Google Scholar]
- Rowlinson, J.S. The Yukawa potential. Phys. Stat. Mech. Its Appl. 1989, 156, 15. [Google Scholar] [CrossRef]
- Okorie, U.S.; Ibekwe, E.E.; Ikot, A.N.; Onyeaju, M.C.; Chukwuocha, E.O. Thermodynamic properties of the modified Yukawa potential. J. Kor. Phys. Soc. 2018, 73, 1211. [Google Scholar] [CrossRef]
- Edet, C.O.; Okoi, P.O.; Chima, S.O. Persistent Current, Magnetic Susceptibility, and Thermal Properties for a Class of Yukawa Potential in the Presence of External Magnetic and Aharanov–Bohm Fields. Int. J. Thermophys. 2020, 42, e20190083. [Google Scholar] [CrossRef]
- Khrapak, S.A.; Ivlev, A.V.; Morfill, G.E.; Zhdanov, S.K. Scattering in the Attractive Yukawa Potential in the Limit of Strong Interaction. Phys. Rev. Lett. 2020, 90, 225002. [Google Scholar] [CrossRef]
- Loeb, A.; Weiner, N. Cores in dwarf galaxies from dark matter with a Yukawa potential. Phys. Rev. Lett. 2011, 106, 171302. [Google Scholar] [CrossRef]
- Hamzavi, M.; Movahedi, M.; Thylwe, K.-E.; Rajabi, A.A. Approximate analytical solution of the Yukawa potential with arbitrary angular momenta. Chin. Phys. Lett. 2012, 29, 080302. [Google Scholar] [CrossRef]
- Martin, B.R.; Shaw, G. Particle Physics, 3rd ed.; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar]
- Carvalho, J.C.N.; Ferreira, W.P.; Farias, G.A.; Peeters, F.M. Yukawa particles confined in a channel and subject to a periodic potential: Ground state and normal modes. Phys. Rev. B 2011, 83, 094109. [Google Scholar] [CrossRef]
- Bahlouli, H.; Abdelmonem, M.S.; Nasser, I.M. Analytical treatment of the Yukawa potential. Phys. Scr. 2010, 82, 065005. [Google Scholar] [CrossRef]
- Imbo, T.; Pagnamenta, A.; Sukhatme, U. Bound states of the Yukawa potential via the shifted 1/N expansion technique. Phys. Lett. A 1984, 105, 183. [Google Scholar] [CrossRef]
- Maireche, A. A model of modified Klein-Gordon equation with modified scalar-vector Yukawa potential. Afr. Rev. Phys. 2020, 15, 0001. [Google Scholar]
- Maireche, A. Nonrelativistic treatment of Hydrogen-like and neutral atoms subjected to the generalized perturbed Yukawa potential with centrifugal barrier in the symmetries of noncommutative Quantum mechanics. Int. J. Geo. Met. Mod. Phys. 2020, 17, 2050067. [Google Scholar] [CrossRef]
- Maireche, A. Approximate Arbitrary k State Solutions of Dirac Equation with Improved Inversely Quadratic Yukawa Potential within Improved Coulomb-like Tensor Interaction in Deformation Quantum Mechanics Symmetries. Few-Body Syst. 2022, 63, 54. [Google Scholar] [CrossRef]
- Edet, C.O.; Ettah, E.B.; Aljunid, S.A.; Endut, R.; Ali, N.; Ikot, A.N.; Asjad, M. Global Quantum Information-Theoretic Measures in the Presence of Magnetic and Aharanov-Bohm (AB) Fields. Symmetry 2022, 14, 976. [Google Scholar] [CrossRef]
- Jiao, L.G.; Zan, L.R.; Zhang, Y.Z.; Ho, Y.K. Benchmark values of S hannon entropy for spherically confined hydrogen atom. Int. J. Quantum Chem. 2017, 117, e25375. [Google Scholar] [CrossRef]
- Amadi, P.O.; Ikot, A.N.; Ngiangia, A.T.; Okorie, U.S.; Rampho, G.J.; Abdullah, H.Y. Shannon entropy and Fisher information for screened Kratzer potential. Int. J. Quantum Chem. 2020, 120, e26246. [Google Scholar] [CrossRef]
- Dehesa, J.G.; Belega, E.D.; Toranzo, I.V.; Aptekarev, A.I. The Shannon entropy of high-dimensional hydrogenic and harmonic systems. Int. J. Quantum Chem. 2019, 119, e25977. [Google Scholar] [CrossRef]
- Martínez-Flores, C. Shannon entropy and Fisher information for endohedral confined one- and two-electron atoms. Phys. Lett. A 2021, 386, 126988. [Google Scholar] [CrossRef]
- Sekh, G.A.; Saha, A.; Talukdar, B. Shannon entropies and Fisher information of K-shell electrons of neutral atoms. Phys. Lett. A 2018, 382, 315. [Google Scholar] [CrossRef]
- Shannon, C.E. A mathematical theory of communication. Bell Syst. Tecn. J. 1948, 27, 379. [Google Scholar] [CrossRef]
- Grasselli, F. Quantum Cryptography; Springer: Cham, Switzerland, 2021. [Google Scholar]
- Amigó, J.M.; Dale, R.; Tempesta, P. A generalized permutation entropy for noisy dynamics and random processes. Chaos 2021, 31, 013115. [Google Scholar] [CrossRef] [PubMed]
- Hassanabadi, H.; Hosseinpour, M. Thermodynamic properties of neutral particle in the presence of topological defects in magnetic cosmic string background. Eur. Phys. J. C 2016, 76, 553. [Google Scholar] [CrossRef]
- Bakke, K.; Ribeiro, L.R.; Furtado, C.; Nascimento, J.R. Landau quantization for a neutral particle in the presence of topological defects. Phys. Rev. D 2009, 79, 024008. [Google Scholar] [CrossRef]
- Nwabuzor, P.; Edet, C.; Ikot, A.N.; Okorie, U.; Ramantswana, M.; Horchani, R. Analyzing the Effects of Topological Defect (TD) on the Energy Spectra and Thermal Properties of LiH, TiC and I2 Diatomic Molecules. Entropy 2021, 198, 1060. [Google Scholar] [CrossRef] [PubMed]
- Edet, C.O.; Ikot, A.N. Effects of Topological Defect on the Energy Spectra and Thermo-magnetic Properties of CO Diatomic Molecule. J. Low Temp. Phys. 2021, 203, 84. [Google Scholar] [CrossRef]
- Greene, R.L.; Aldrich, C. Variational wave functions for a screened Coulomb potential. Phys. Rev. A 1976, 14, 2363. [Google Scholar] [CrossRef]
- Qiang, W.-C.; Dong, S.-H. Analytical approximations to the solutions of the Manning–Rosen potential with centrifugal term. Phys. Lett. A 2007, 368, 13. [Google Scholar] [CrossRef]
- Dong, S.; García-Ravelo, J.; Dong, S.-H. Analytical approximations to the l-wave solutions of the Schrödinger equation with an exponential-type potential. Phys. Scr. 2007, 76, 393. [Google Scholar] [CrossRef]
- Dong, S.; Sun, G.-H.; Dong, S.-H.; Draayer, J.P. Quantum information entropies for a squared tangent potential well. Phys. Lett. A 2014, 378, 124. [Google Scholar] [CrossRef]
- Sun, G.-H.; Aoki, M.A.; Dong, S.-H. Quantum information entropies of the eigenstates for the Pöschl—Teller-like potential. Chin. Phys. B 2013, 22, 050302. [Google Scholar] [CrossRef]
- Song, X.-D.; Sun, G.-H.; Dong, S.-H. Shannon information entropy for an infinite circular well. Phys. Lett. A 2015, 379, 1402. [Google Scholar] [CrossRef]
- Sun, G.-H.; Dong, S.-H. Quantum information entropies of the eigenstates for a symmetrically trigonometric Rosen–Morse potential. Phys. Scr. 2013, 87, 045003. [Google Scholar] [CrossRef]
- Sun, G.-H.; Dong, S.-H.; Saad, N. Quantum information entropies for an asymmetric trigonometric Rosen-Morse potential. Ann. Phys. 2013, 525, 943. [Google Scholar] [CrossRef]
- Serrano, F.A.; Falaye, B.J.; Dong, S.-H. Information-theoretic measures for a solitonic profile mass Schrödinger equation with a squared hyperbolic cosecant potential. Physica A 2016, 446, 152. [Google Scholar] [CrossRef]
- Gil-Barrera, C.A.; Carrillo, R.S.; Sun, G.-H.; Dong, S.-H. Quantum Information Entropies on Hyperbolic Single Potential Wells. Entropy 2022, 24, 604. [Google Scholar] [CrossRef]
- Pathria, R.K. Statistical Mechanics, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 1996. [Google Scholar]
- Lima, F.C.E.; Moreira, A.R.P.; Machado, L.E.S.; Almeida, C.A.S. Statistical properties of linear Majorana fermions. Int. J. Quantum Chem. 2021, 121, e26749. [Google Scholar] [CrossRef]
- Lima, F.C.E.; Moreira, A.R.P.; Almeida, C.A.S. Information and thermodynamic properties of a non-Hermitian particle ensemble. Int. J. Quantum Chem. 2021, 121, e26645. [Google Scholar] [CrossRef]
- Yanez-Navarro, G.; Sun, G.-H.; Dytrych, T.; Launey, K.D.; Dong, S.-H.; Draayer, J.P. Quantum information entropies for position-dependent mass Schrödinger problem. Ann. Phys. 2014, 348, 153. [Google Scholar] [CrossRef]
- Lima, F.C.E. Quantum information entropies for a soliton at hyperbolic well. Ann. Phys. 2022, 442, 168906. [Google Scholar] [CrossRef]
- Sun, G.-H.; Dong, S.-H.; Launey, K.D.; Dytrych, T.; Draayer, J.P. Shannon information entropy for a hyperbolic double-well potential. Int. J. Quantum Chem. 2015, 115, 891. [Google Scholar] [CrossRef]
- Hirschmann, I.I., Jr. American Journal of Mathematics; The Johns Hopkins University Press: Baltimore, MD, USA, 1957; Volume 79, p. 152. [Google Scholar]
- Beckner, W. Inequalities in Fourier analysis. Ann. Math. 1975, 102, 159. [Google Scholar] [CrossRef]
- Born, M. Statistical interpretation of quantum mechanics. Science 1955, 122, 675. [Google Scholar] [CrossRef] [PubMed]
- Bialynicki-Birula, I.; Mycielski, J. Uncertainty relations for information entropy in wave mechanics. Comm. Math. Phys. 1975, 44, 129. [Google Scholar] [CrossRef]
- Fisher, R.A. Theory of statistical estimation. Math. Proc. Cambridge Philos. Soc. 1925, 22, 700. [Google Scholar] [CrossRef]
- Falaye, B.J.; Serrano, F.A.; Dong, S.-H. Fisher information for the position-dependent mass Schrödinger system. Phys. Lett. A 2016, 380, 267. [Google Scholar] [CrossRef]

| n | m | B | ||||
|---|---|---|---|---|---|---|
| 0 | 0 | 1 | 1 | 1.32078 | 2.91721 | 4.23799 |
| 2 | 1 | 0.69776 | 3.53949 | 4.23725 | ||
| 4 | 1 | 0.20082 | 4.37062 | 4.57144 | ||
| 1 | 2 | 0.68816 | 3.24081 | 3.92897 | ||
| 1 | 4 | 0.20081 | 5.93350 | 6.13431 | ||
| 1 | 0 | 1 | 1 | 4.41786 | 7.14836 | 11.56622 |
| 2 | 1 | 0.53510 | 10.14113 | 10.67623 | ||
| 4 | 1 | 0.41849 | 10.44393 | 10.86242 | ||
| 1 | 2 | 0.83093 | 4.83668 | 5.66761 | ||
| 1 | 4 | 0.19793 | 6.30831 | 6.50624 | ||
| 1 | 1 | 1 | 1 | 6.52497 | 8.45892 | 14.98389 |
| 2 | 1 | 0.36401 | 11.48506 | 11.84907 | ||
| 4 | 1 | 0.31416 | 11.78786 | 12.10202 | ||
| 1 | 2 | 0.35912 | 6.29333 | 6.65245 | ||
| 1 | 4 | 0.03149 | 6.36141 | 6.39290 |
| n | m | ||||
|---|---|---|---|---|---|
| 0 | 0 | 0.1 | −1.43473 | 5.30857 | 3.87384 |
| 0.2 | −0.73813 | 3.14145 | 2.40332 | ||
| 0.4 | 1.24625 | 1.96739 | 3.21364 | ||
| 1 | 0 | 0.1 | −1.52895 | 8.04484 | 6.51589 |
| 0.2 | −0.91699 | 6.30221 | 5.38522 | ||
| 0.4 | −0.05982 | 4.30012 | 4.24030 | ||
| 1 | 1 | 0.1 | −1.60572 | 9.35928 | 7.75356 |
| 0.2 | −0.96676 | 9.14016 | 8.17340 | ||
| 0.4 | −0.31977 | 5.52721 | 5.20744 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Edet, C.O.; Lima, F.C.E.; Almeida, C.A.S.; Ali, N.; Asjad, M. Quantum Information of the Aharanov–Bohm Ring with Yukawa Interaction in the Presence of Disclination. Entropy 2022, 24, 1059. https://doi.org/10.3390/e24081059
Edet CO, Lima FCE, Almeida CAS, Ali N, Asjad M. Quantum Information of the Aharanov–Bohm Ring with Yukawa Interaction in the Presence of Disclination. Entropy. 2022; 24(8):1059. https://doi.org/10.3390/e24081059
Chicago/Turabian StyleEdet, Collins Okon, Francisco Cleiton E. Lima, Carlos Alberto S. Almeida, Norshamsuri Ali, and Muhammad Asjad. 2022. "Quantum Information of the Aharanov–Bohm Ring with Yukawa Interaction in the Presence of Disclination" Entropy 24, no. 8: 1059. https://doi.org/10.3390/e24081059
APA StyleEdet, C. O., Lima, F. C. E., Almeida, C. A. S., Ali, N., & Asjad, M. (2022). Quantum Information of the Aharanov–Bohm Ring with Yukawa Interaction in the Presence of Disclination. Entropy, 24(8), 1059. https://doi.org/10.3390/e24081059








