Quantum Thermal Amplifiers with Engineered Dissipation
Abstract
:1. Introduction
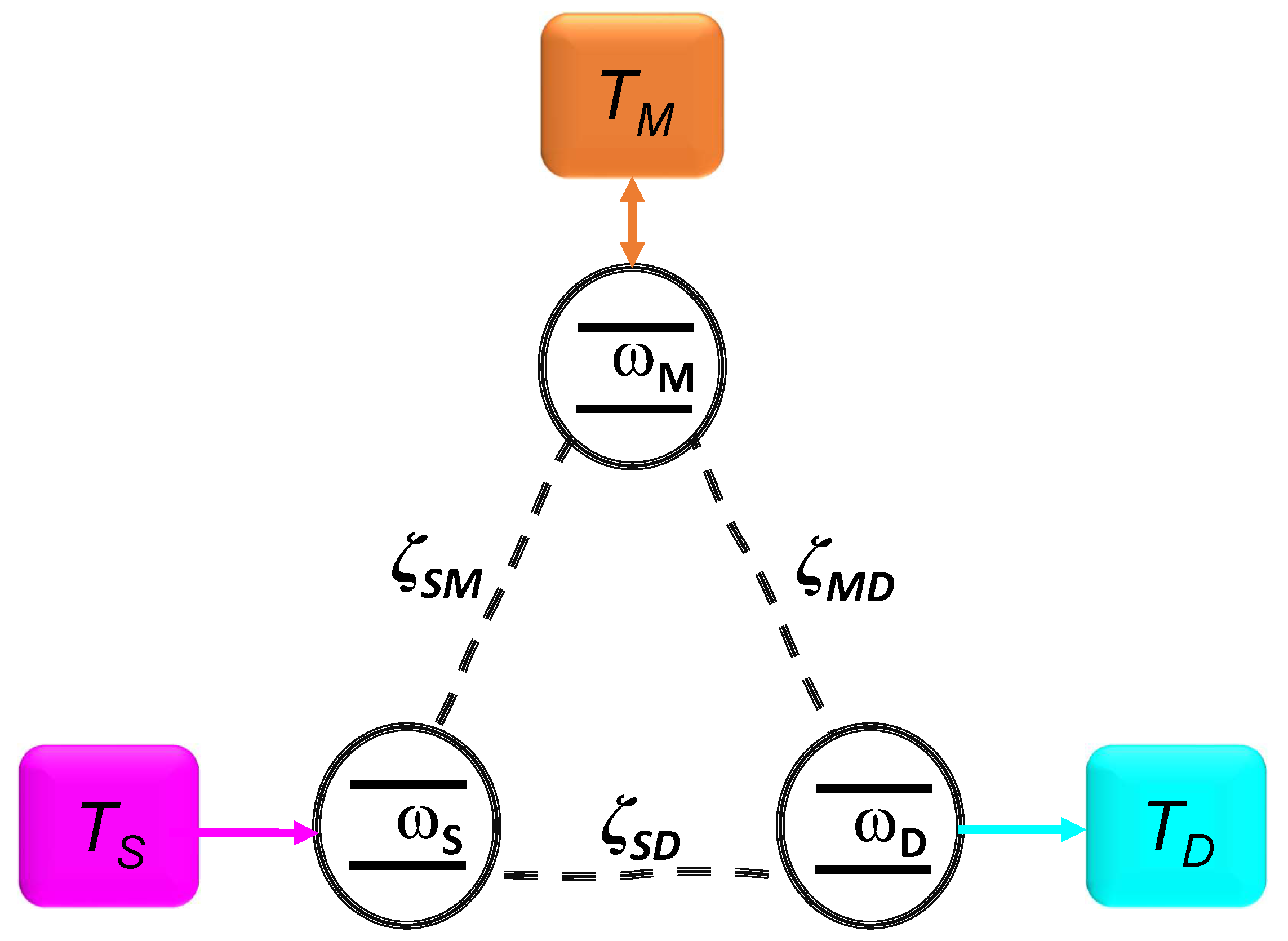
2. The Model
3. Non-Equilibrium Dynamics
Definition of Heat Currents
4. Quantum Thermal Transistor
4.1. Amplification of Heat Currents
4.2. Amplification Factor
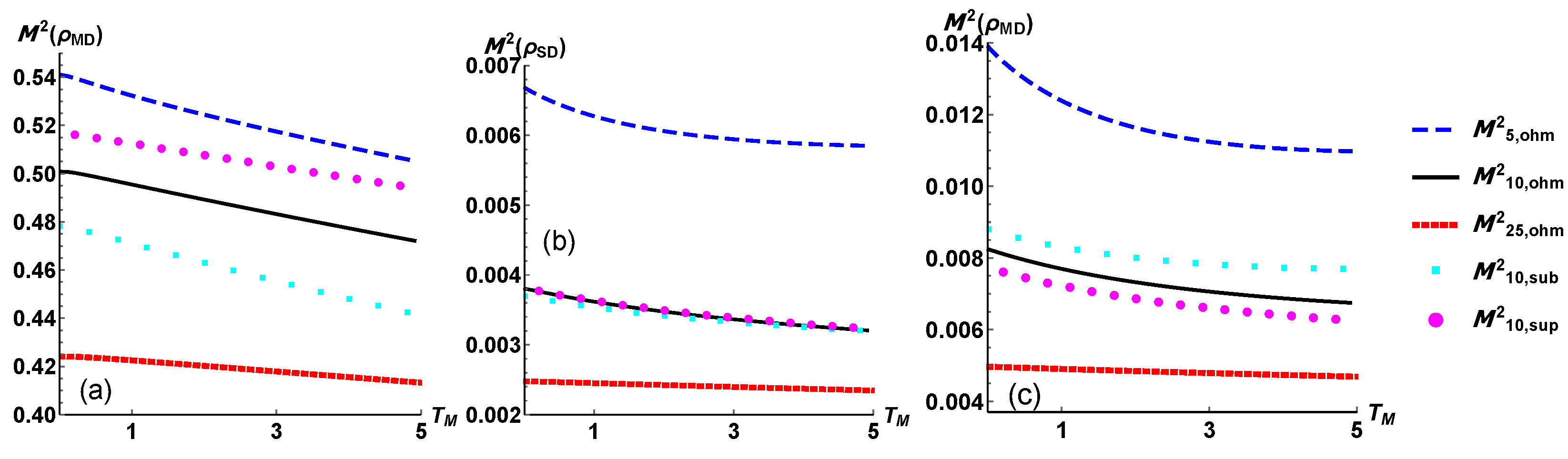
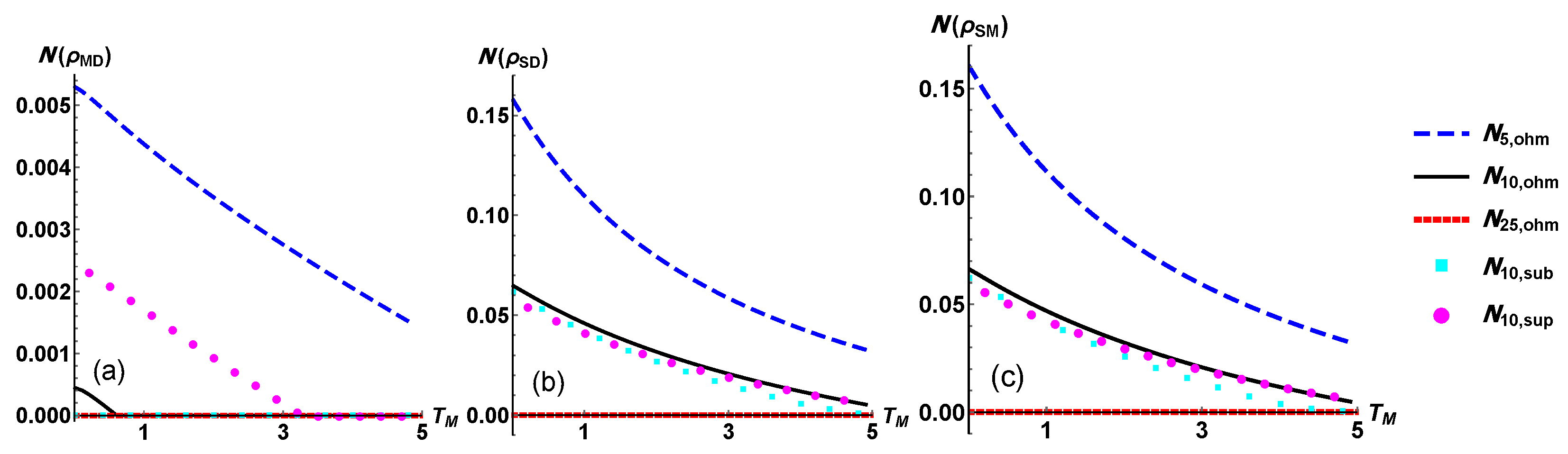
5. Insights into the Transistor Effect via Entropic Measures of Correlations
6. Outro and Future Perspectives
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| GKSL | Gorini Kossakowski Sudarshan Lindblad |
| MME | Markovian Master Equation |
| NESS | Non-Equilibrium Steady State |
| QTT | Quantum Thermal Transistor |
Appendix A

References
- Wang, L.; Li, B. Phononics gets hot. Phys. World 2008, 21, 27. [Google Scholar] [CrossRef]
- Li, N.; Ren, J.; Wang, L.; Zhang, G.; Hänggi, P.; Li, B. Colloquium: Phononics: Manipulating heat flow with electronic analogs and beyond. Rev. Mod. Phys. 2012, 84, 1045–1066. [Google Scholar] [CrossRef] [Green Version]
- Dutta, B.; Majidi, D.; Talarico, N.W.; Lo Gullo, N.; Courtois, H.; Winkelmann, C.B. Single-Quantum-Dot Heat Valve. Phys. Rev. Lett. 2020, 125, 237701. [Google Scholar] [CrossRef]
- Li, B.; Wang, L.; Casati, G. Thermal Diode: Rectification of Heat Flux. Phys. Rev. Lett. 2004, 93, 184301. [Google Scholar] [CrossRef] [Green Version]
- Pereira, E. Graded anharmonic crystals as genuine thermal diodes: Analytical description of rectification and negative differential thermal resistance. Phys. Rev. E 2010, 82, 040101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Werlang, T.; Marchiori, M.A.; Cornelio, M.F.; Valente, D. Optimal rectification in the ultrastrong coupling regime. Phys. Rev. E 2014, 89, 062109. [Google Scholar] [CrossRef] [Green Version]
- Ordonez-Miranda, J.; Ezzahri, Y.; Joulain, K. Quantum thermal diode based on two interacting spinlike systems under different excitations. Phys. Rev. E 2017, 95, 022128. [Google Scholar] [CrossRef]
- Li, B.; Wang, L.; Casati, G. Negative differential thermal resistance and thermal transistor. Appl. Phys. Lett. 2006, 88, 143501. [Google Scholar] [CrossRef] [Green Version]
- Joulain, K.; Drevillon, J.; Ezzahri, Y.; Ordonez-Miranda, J. Quantum Thermal Transistor. Phys. Rev. Lett. 2016, 116, 200601. [Google Scholar] [CrossRef] [Green Version]
- Mandarino, A.; Joulain, K.; Gómez, M.D.; Bellomo, B. Thermal Transistor Effect in Quantum Systems. Phys. Rev. Appl. 2021, 16, 034026. [Google Scholar] [CrossRef]
- Guo, B.Q.; Liu, T.; Yu, C.S. Quantum thermal transistor based on qubit-qutrit coupling. Phys. Rev. E 2018, 98, 022118. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Majland, M.; Christensen, K.S.; Zinner, N.T. Quantum thermal transistor in superconducting circuits. Phys. Rev. B 2020, 101, 184510. [Google Scholar] [CrossRef]
- Wang, L.; Li, B. Thermal Logic Gates: Computation with Phonons. Phys. Rev. Lett. 2007, 99, 177208. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.Q.; Yu, D.H.; Yu, C.S. Common environmental effects on quantum thermal transistor. Entropy 2021, 24, 32. [Google Scholar] [CrossRef] [PubMed]
- Wijesekara, R.T.; Gunapala, S.D.; Premaratne, M. Darlington pair of quantum thermal transistors. Phys. Rev. B 2021, 104, 045405. [Google Scholar] [CrossRef]
- Wijesekara, R.T.; Gunapala, S.D.; Premaratne, M. Towards quantum thermal multi-transistor systems: Energy divider formalism. Phys. Rev. B 2022, 105, 235412. [Google Scholar] [CrossRef]
- Salvatori, G.; Mandarino, A.; Paris, M.G.A. Quantum metrology in Lipkin-Meshkov-Glick critical systems. Phys. Rev. A 2014, 90, 022111. [Google Scholar] [CrossRef] [Green Version]
- Opatrný, T.C.V.; Kolář, M.; Das, K.K. Spin squeezing by tensor twisting and Lipkin-Meshkov-Glick dynamics in a toroidal Bose-Einstein condensate with spatially modulated nonlinearity. Phys. Rev. A 2015, 91, 053612. [Google Scholar] [CrossRef] [Green Version]
- Campbell, S.; De Chiara, G.; Paternostro, M.; Palma, G.M.; Fazio, R. Shortcut to Adiabaticity in the Lipkin-Meshkov-Glick Model. Phys. Rev. Lett. 2015, 114, 177206. [Google Scholar] [CrossRef] [Green Version]
- Linden, N.; Popescu, S.; Skrzypczyk, P. How Small Can Thermal Machines Be? The Smallest Possible Refrigerator. Phys. Rev. Lett. 2010, 105, 130401. [Google Scholar] [CrossRef]
- Brunner, N.; Huber, M.; Linden, N.; Silva, R.; Popescu, S.; Skrzypczyk, P. Entanglement enhances cooling in microscopic quantum refrigerators. Phys. Rev. E 2014, 89, 032115. [Google Scholar] [CrossRef] [Green Version]
- Correa, L.A.; Palao, J.P.; Alonso, D.; Adesso, G. Quantum-enhanced absorption refrigerators. Sci. Rep. 2014, 4, 3949. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Correa, L.A.; Palao, J.P.; Adesso, G.; Alonso, D. Performance bound for quantum absorption refrigerators. Phys. Rev. E 2013, 87, 042131. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Correa, L.A.; Palao, J.P.; Adesso, G.; Alonso, D. Optimal performance of endoreversible quantum refrigerators. Phys. Rev. E 2014, 90, 062124. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Galve, F.; Mandarino, A.; Paris, M.G.; Benedetti, C.; Zambrini, R. Microscopic description for the emergence of collective dissipation in extended quantum systems. Sci. Rep. 2017, 7, 42050. [Google Scholar] [CrossRef]
- Davies, E.B. Markovian master equations. Comm. Math. Phys. 1974, 39, 91–110. [Google Scholar] [CrossRef]
- Alicki, R.; Lendi, K. Quantum Dynamical Semigroups and Applications; Springer: Berlin/Heidelberg, Germany, 2007; Volume 717. [Google Scholar]
- Gorini, V.; Kossakowski, A.; Sudarshan, E.C.G. Completely positive dynamical semigroups of N-level systems. J. Math. Phys. 1976, 17, 821–825. [Google Scholar] [CrossRef]
- Lindblad, G. On the generators of quantum dynamical semigroups. Commun. Math. Phys. 1976, 48, 119–130. [Google Scholar] [CrossRef]
- Leggett, A.J.; Chakravarty, S.; Dorsey, A.T.; Fisher, M.P.A.; Garg, A.; Zwerger, W. Dynamics of the dissipative two-state system. Rev. Mod. Phys. 1987, 59, 1–85. [Google Scholar] [CrossRef]
- Caldeira, A.O.; Leggett, A.J. Influence of Dissipation on Quantum Tunneling in Macroscopic Systems. Phys. Rev. Lett. 1981, 46, 211–214. [Google Scholar] [CrossRef]
- Caldeira, A.O.; Leggett, A.J. Quantum tunnelling in a dissipative system. Ann. Phys. 1983, 149, 374–456. [Google Scholar] [CrossRef]
- Wilner, E.Y.; Wang, H.; Thoss, M.; Rabani, E. Sub-Ohmic to super-Ohmic crossover behavior in nonequilibrium quantum systems with electron-phonon interactions. Phys. Rev. B 2015, 92, 195143. [Google Scholar] [CrossRef] [Green Version]
- Weiss, U. Quantum Dissipative Systems, 4th ed.; World Scientific: Singapore, 2012. [Google Scholar] [CrossRef]
- Kosloff, R. Quantum thermodynamics: A dynamical viewpoint. Entropy 2013, 15, 2100–2128. [Google Scholar] [CrossRef] [Green Version]
- Rivas, Á. Quantum thermodynamics in the refined weak coupling limit. Entropy 2019, 21, 725. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Seshadri, A.; Madhok, V.; Lakshminarayan, A. Tripartite mutual information, entanglement, and scrambling in permutation symmetric systems with an application to quantum chaos. Phys. Rev. E 2018, 98, 052205. [Google Scholar] [CrossRef] [Green Version]
- Rota, M. Tripartite information of highly entangled states. J. High Energy Phys. 2016, 2016, 75. [Google Scholar] [CrossRef] [Green Version]
- Rangamani, M.; Rota, M. Entanglement structures in qubit systems. J. Phys. A Math. Theor. 2015, 48, 385301. [Google Scholar] [CrossRef] [Green Version]
- Kalaga, J.K.; Leoński, W.; Szczęśniak, R.; Peřina Jr, J. Mixedness, Coherence and Entanglement in a Family of Three-Qubit States. Entropy 2022, 24, 324. [Google Scholar] [CrossRef]
- Peres, A. Separability Criterion for Density Matrices. Phys. Rev. Lett. 1996, 77, 1413–1415. [Google Scholar] [CrossRef] [Green Version]
- Horodecki, M.; Horodecki, P.; Horodecki, R. Separability of mixed states: Necessary and sufficient conditions. Phys. Lett. A 1996, 223, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Vidal, G.; Werner, R.F. Computable measure of entanglement. Phys. Rev. A 2002, 65, 032314. [Google Scholar] [CrossRef] [Green Version]
- Yu, T.; Eberly, J.H. Sudden Death of Entanglement. Science 2009, 323, 598–601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, J.S.; Wang, J.; Zeng, N. Nonequilibrium Green’s function approach to mesoscopic thermal transport. Phys. Rev. B 2006, 74, 033408. [Google Scholar] [CrossRef] [Green Version]
- Bina, M.; Mandarino, A.; Olivares, S.; Paris, M.G.A. Drawbacks of the use of fidelity to assess quantum resources. Phys. Rev. A 2014, 89, 012305. [Google Scholar] [CrossRef] [Green Version]
- Mandarino, A.; Bina, M.; Olivares, S.; Paris, M.G. About the use of fidelity in continuous variable systems. Int. J. Quantum Inf. 2014, 12, 1461015. [Google Scholar] [CrossRef] [Green Version]
- Mandarino, A.; Bina, M.; Porto, C.; Cialdi, S.; Olivares, S.; Paris, M.G.A. Assessing the significance of fidelity as a figure of merit in quantum state reconstruction of discrete and continuous-variable systems. Phys. Rev. A 2016, 93, 062118. [Google Scholar] [CrossRef] [Green Version]
- Enríquez, M.; Delgado, F.; Życzkowski, K. Entanglement of three-qubit random pure states. Entropy 2018, 20, 745. [Google Scholar] [CrossRef] [Green Version]
- Dür, W.; Vidal, G.; Cirac, J.I. Three qubits can be entangled in two inequivalent ways. Phys. Rev. A 2000, 62, 062314. [Google Scholar] [CrossRef] [Green Version]
- Acín, A.; Bruß, D.; Lewenstein, M.; Sanpera, A. Classification of Mixed Three-Qubit States. Phys. Rev. Lett. 2001, 87, 040401. [Google Scholar] [CrossRef] [Green Version]

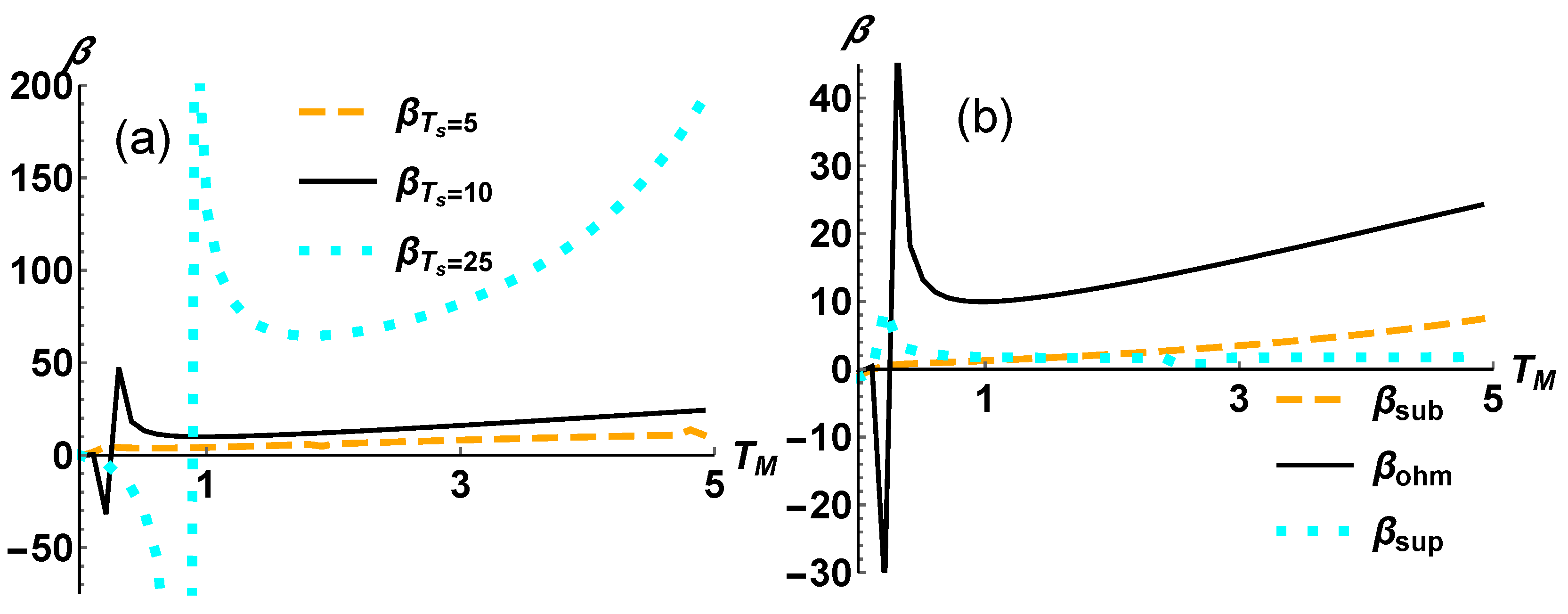

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mandarino, A. Quantum Thermal Amplifiers with Engineered Dissipation. Entropy 2022, 24, 1031. https://doi.org/10.3390/e24081031
Mandarino A. Quantum Thermal Amplifiers with Engineered Dissipation. Entropy. 2022; 24(8):1031. https://doi.org/10.3390/e24081031
Chicago/Turabian StyleMandarino, Antonio. 2022. "Quantum Thermal Amplifiers with Engineered Dissipation" Entropy 24, no. 8: 1031. https://doi.org/10.3390/e24081031
APA StyleMandarino, A. (2022). Quantum Thermal Amplifiers with Engineered Dissipation. Entropy, 24(8), 1031. https://doi.org/10.3390/e24081031





