Robust Finite-Time Stability for Uncertain Discrete-Time Stochastic Nonlinear Systems with Time-Varying Delay
Abstract
:1. Introduction
2. Problem Statement and Preliminaries
3. Main Results
3.1. FTS for Stochastic Systems with Nonlinear Disturbances and Uncertain Parameters
3.2. FTS for Nominal Systems
3.3. FTS for Stochastic Systems with Nonlinear Disturbances
3.4. FTS for Stochastic Systems with Uncertain Parameters
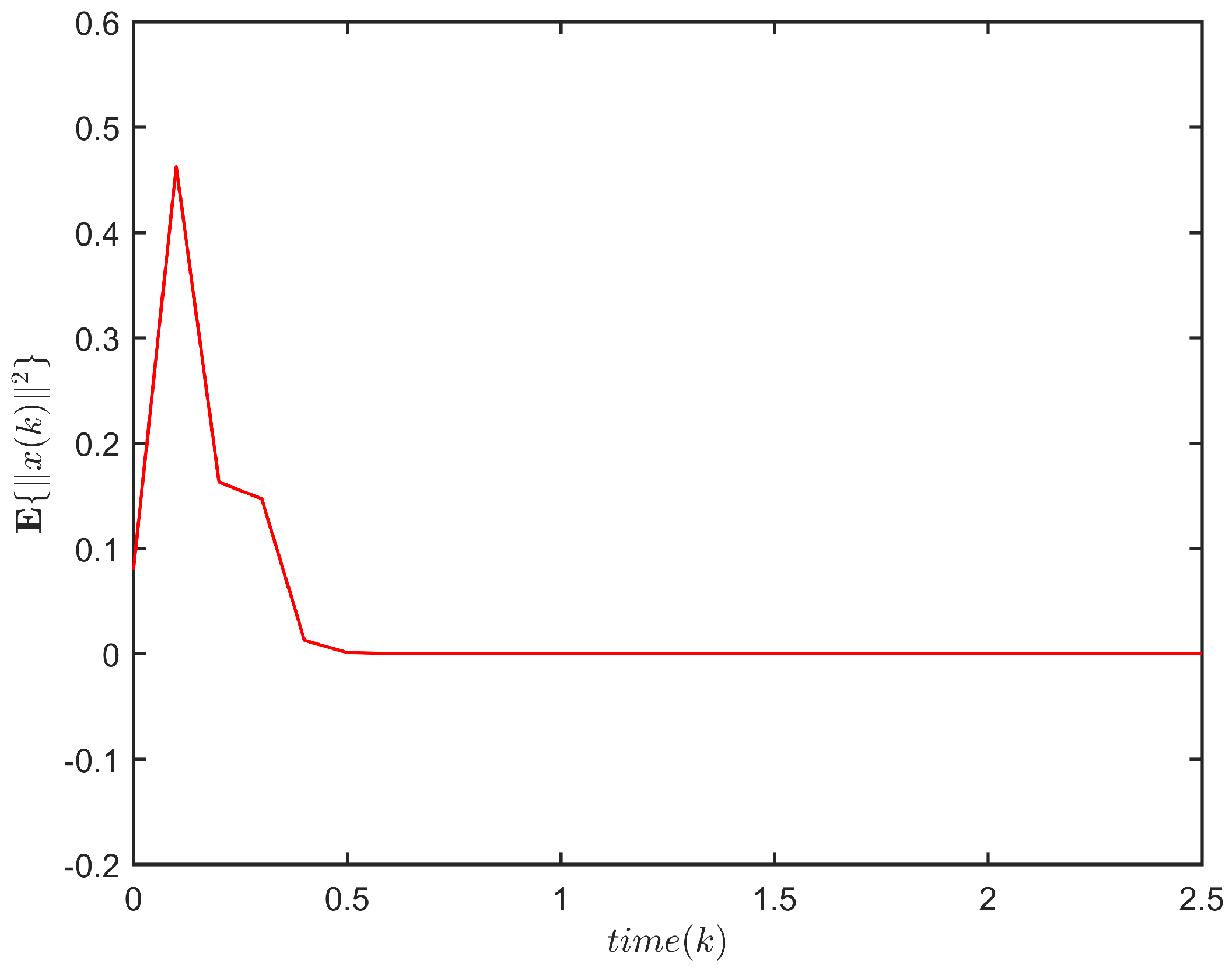
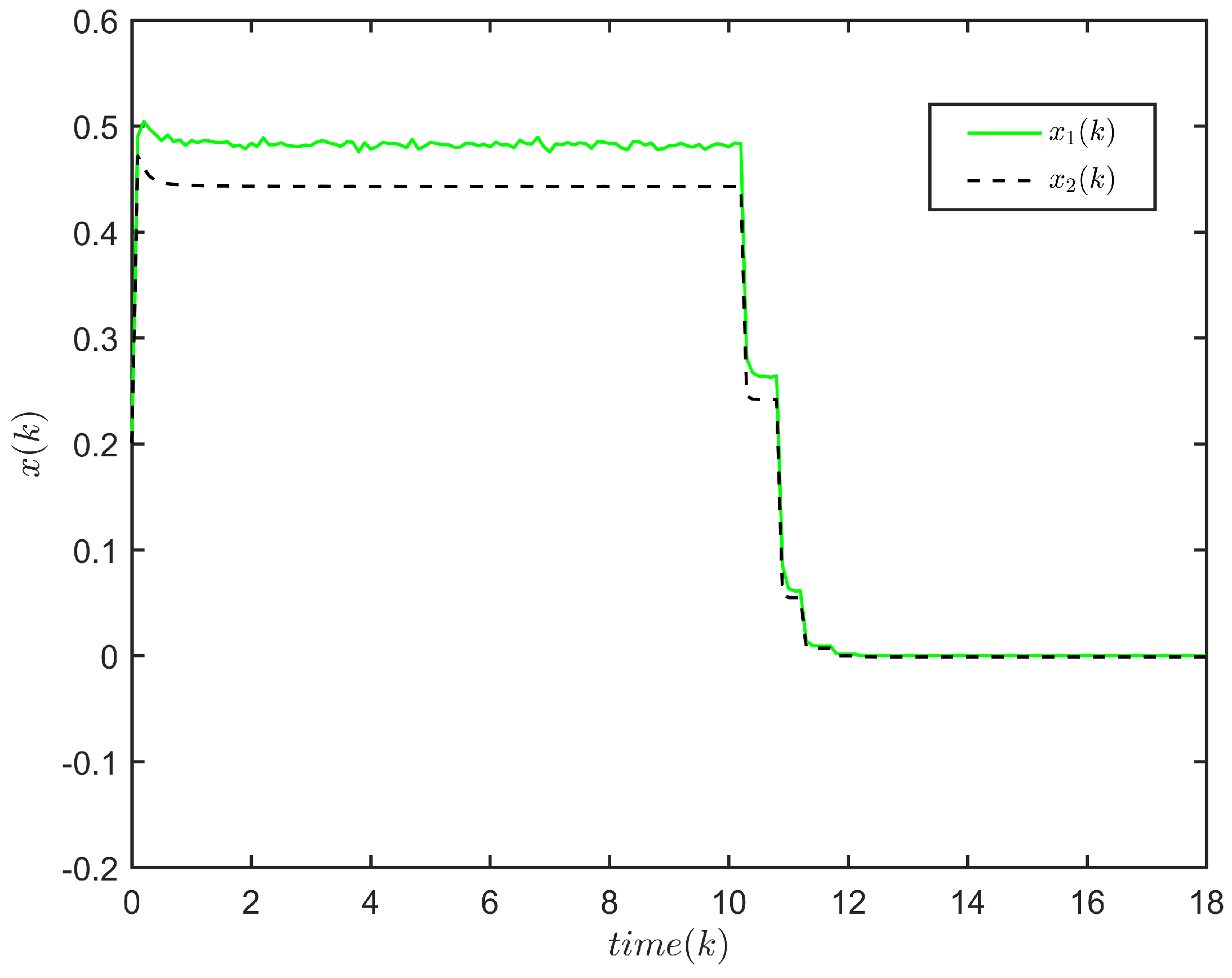
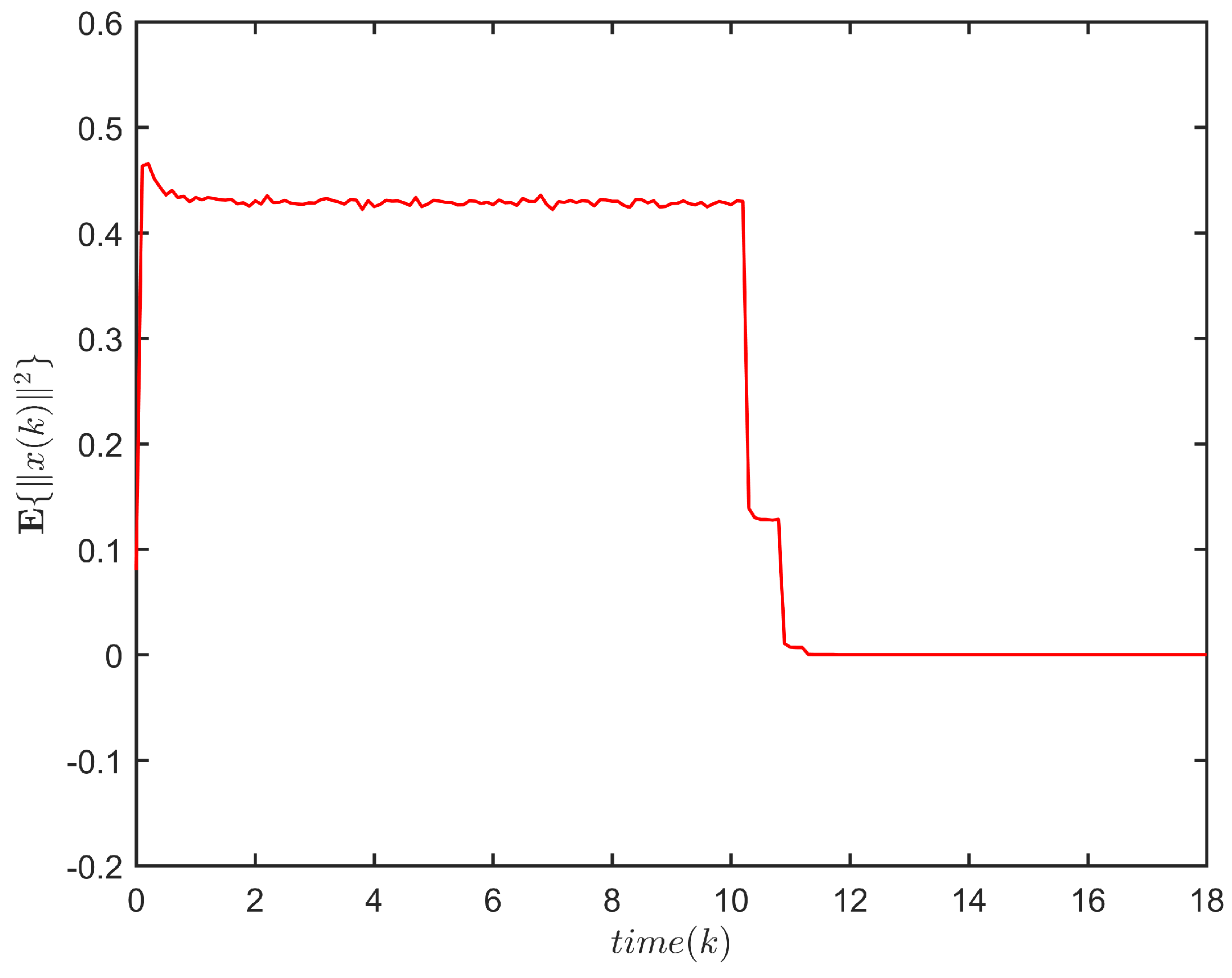
4. Numerical Examples
- (a)
- (b)
- (c)
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Aouiti, C.; Hui, Q.; Jallouli, H.; Moulay, E. Fixed-time stabilization of fuzzy neutral-type inertial neural networks with time-varying delay. Fuzzy Sets Syst. 2021, 411, 48–67. [Google Scholar] [CrossRef]
- Zhou, B.; Luo, W. Improved Razumikhin and Krasovskii stability criteria for time-varying stochastic time-delay systems. Automatica 2018, 89, 382–391. [Google Scholar] [CrossRef] [Green Version]
- Li, H.; Liu, Q.; Feng, G.; Zhang, X. Leader-follower consensus of nonlinear time-delay multiagent systems: A time-varying gain approach. Automatica 2021, 126, 109444. [Google Scholar] [CrossRef]
- Chen, L.; Bai, S.; Li, G.; Li, Z.; Xiao, Q.; Bai, L.; Li, C.; Xian, L.; Hu, Z.; Dai, G.; et al. Accuracy of real-time respiratory motion tracking and time delay of gating radiotherapy based on optical surface imaging technique. Radiat. Oncol. 2020, 15, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Yang, X.; Song, S. Lyapunov conditions for finite-time stability of time-varying time-delay systems. Automatica 2019, 103, 135–140. [Google Scholar] [CrossRef]
- Zhang, C.K.; He, Y.; Jiang, L.; Wu, M.; Wang, Q.G. An extended reciprocally convex matrix inequality for stability analysis of systems with time-varying delay. Automatica 2017, 85, 481–485. [Google Scholar] [CrossRef]
- Zhou, B. Finite-time stability analysis and stabilization by bounded linear time-varying feedback. Automatica 2020, 121, 109191. [Google Scholar] [CrossRef]
- Li, R.; Zhao, P. Practical stability of time-varying positive systems with time delay. IET Control. Theory Appl. 2021, 15, 1082–1090. [Google Scholar] [CrossRef]
- Long, F.; Lin, W.J.; He, Y.; Jiang, L.; Wu, M. Stability analysis of linear systems with time-varying delay via a quadratic function negative-definiteness determination method. IET Control. Theory Appl. 2020, 14, 1478–1485. [Google Scholar] [CrossRef]
- Wu, B.; Wang, C.L.; Hu, Y.J.; Ma, X.L. Stability analysis for time-delay systems with nonlinear disturbances via new generalized integral inequalities. Int. J. Control. Autom. Syst. 2018, 16, 2772–2780. [Google Scholar] [CrossRef]
- Dong, Y.; Liang, S.; Wang, H. Robust stability and H∞ control for nonlinear discrete-time switched systems with interval time-varying delay. Math. Methods Appl. Sci. 2019, 42, 1999–2015. [Google Scholar] [CrossRef]
- Ruan, Y.; Huang, T. Finite-Time control for nonlinear systems with time-varying delay and exogenous disturbance. Symmetry 2020, 12, 447. [Google Scholar] [CrossRef] [Green Version]
- Kang, W.; Zhong, S.; Shi, K.; Cheng, J. Finite-time stability for discrete-time system with time-varying delay and nonlinear perturbations. ISA Trans. 2016, 60, 67–73. [Google Scholar] [CrossRef] [PubMed]
- Stojanovic, S. Robust finite-time stability of discrete time systems with interval time-varying delay and nonlinear perturbations. J. Frankl. Inst. 2017, 354, 4549–4572. [Google Scholar] [CrossRef]
- Yang, X.; Li, X.; Cao, J. Robust finite-time stability of singular nonlinear systems with interval time-varying delay. J. Frankl. Inst. 2018, 355, 1241–1258. [Google Scholar] [CrossRef]
- Haddad, W.; Lee, J. Finite-time stability of discrete autonomous systems. Automatica 2020, 122, 109282. [Google Scholar] [CrossRef]
- Xi, Q.; Liang, Z.; Li, X. Uniform finite-time stability of nonlinear impulsive time-varying systems. Appl. Math. Model. 2021, 91, 913–922. [Google Scholar] [CrossRef]
- Alzabut, J.; Selvam, A.G.M.; El-Nabulsi, R.A.; Dhakshinamoorthy, V.; Samei, M.E. Asymptotic stability of nonlinear discrete fractional pantograph equations with non-local initial conditions. Symmetry 2021, 13, 473. [Google Scholar] [CrossRef]
- Rubbioni, P. Asymptotic stability of solutions for some classes of impulsive differential equations with distributed delay. Nonlinear Anal. Real World Appl. 2021, 61, 103324. [Google Scholar] [CrossRef]
- Dorato, P. Short time stability in linear time-varying systems. In Proceedings of the IRE International Convention Record Part 4, New York, NY, USA, 9 May 1961; Volume 4, pp. 83–87. [Google Scholar]
- Shi, C.; Vong, S. Finite-time stability for discrete-time systems with time-varying delay and nonlinear perturbations by weighted inequalities. J. Frankl. Inst. 2020, 357, 294–313. [Google Scholar] [CrossRef]
- Lin, X.; Liang, K.; Li, H.; Jiao, Y.; Nie, J. Finite-time stability and stabilization for continuous systems with additive time-varying delays. Circuits Syst. Signal Process. 2017, 36, 2971–2990. [Google Scholar] [CrossRef]
- Jia, R.; Wang, J.; Zhou, J. Fault diagnosis of industrial process based on the optimal parametric t-distributed stochastic neighbor embedding. Sci. China Inf. Sci. 2020, 64, 541–553. [Google Scholar] [CrossRef]
- Yu, X.; Yin, J.; Khoo, S. New Lyapunov conditions of stochastic finite-time stability and instability of nonlinear time-varying SDEs. Int. J. Control 2021, 94, 1674–1681. [Google Scholar] [CrossRef]
- Wang, M.; Liu, Y.; Cao, G.; Owens, D.H. Energy-based finite-time stabilization and H∞ control of stochastic nonlinear systems. Int. J. Robust Nonlinear Control 2020, 30, 7169–7184. [Google Scholar] [CrossRef]
- Cheng, G.; Ju, Y.; Mu, X. Stochastic finite-time stability and stabilisation of semi-Markovian jump linear systems with generally uncertain transition rates. Int. J. Syst. Sci. 2021, 52, 185–195. [Google Scholar] [CrossRef]
- Yan, Z.; Song, Y.; Liu, X. Finite-time stability and stabilization for Itô-type stochastic Markovian jump systems with generally uncertain transition rates. Appl. Math. Comput. 2018, 321, 512–525. [Google Scholar] [CrossRef]
- Zhang, T.; Deng, F.; Zhang, W. Finite-time stability and stabilization of linear discrete time-varying stochastic systems. J. Frankl. Inst. 2019, 356, 1247–1267. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Liu, Q.; Li, Y. Finite-time guaranteed cost control for uncertain mean-field stochastic systems. J. Frankl. Inst. 2020, 357, 2813–2829. [Google Scholar] [CrossRef]
- Niamsup, P.; Phat, V. Robust finite-time H∞ control of linear time-varying delay systems with bounded control via Riccati equations. Int. J. Autom. Comput. 2018, 15, 355–363. [Google Scholar] [CrossRef]
- Wang, G.; Li, Z.; Zhang, Q.; Yang, C. Robust finite-time stability and stabilization of uncertain Markovian jump systems with time-varying delay. Appl. Math. Comput. 2017, 293, 377–393. [Google Scholar] [CrossRef]
- Liu, H.; Lin, X. Finite-time H∞ control for a class of nonlinear system with time-varying delay. Neurocomputing 2015, 149, 1481–1489. [Google Scholar] [CrossRef]
- Zhou, M.; Fu, Y. Stability and stabilization for discrete-time Markovian jump stochastic systems with piecewise homogeneous transition probabilities. Int. J. Control. Autom. Syst. 2019, 17, 2165–2173. [Google Scholar] [CrossRef]
- Gao, M.; Zhao, J.; Sun, W. Stochastic H2/H∞ control for discrete-time mean-field systems with Poisson jump. J. Frankl. Inst. 2021, 358, 2933–2947. [Google Scholar] [CrossRef]
- Rui, H.; Liu, J.; Qu, Y.; Cong, S.; Song, H. Global asymptotic stability analysis of discrete-time stochastic coupled systems with time-varying delay. Int. J. Control 2021, 94, 757–766. [Google Scholar] [CrossRef]
- Stojanovic, S. New results for finite-time stability of discrete-time linear systems with interval time-varying delay. Discret. Dyn. Nat. Soc. 2015, 2015, 480816. [Google Scholar] [CrossRef]
- Ouellette, D. Schur complements and statistics. Linear Algebra Its Appl. 1981, 36, 187–295. [Google Scholar] [CrossRef] [Green Version]
- Zuo, Z.; Li, H.; Wang, Y. New criterion for finite-time stability of linear discrete-time systems with time-varying delay. J. Frankl. Inst. 2013, 350, 2745–2756. [Google Scholar] [CrossRef]
- Seuret, A.; Gouaisbaut, F.; Fridman, E. Stability of discrete-time systems with time-varying delays via a novel summation inequality. IEEE Trans. Autom. Control 2015, 60, 2740–2745. [Google Scholar] [CrossRef] [Green Version]
- He, Y.; Wang, Q.; Xie, L.; Lin, C. Further improvement of free-weighting matrices technique for systems with time-varying delay. IEEE Trans. Autom. Control 2007, 52, 293–299. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, Z.; Zhang, H.; Zheng, B.; Karimi, H.R. Finite-time stability analysis and stabilization for linear discrete-time system with time-varying delay. J. Frankl. Inst. 2014, 351, 3457–3476. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, X.; Li, W.; Wang, J.; Li, Y. Robust Finite-Time Stability for Uncertain Discrete-Time Stochastic Nonlinear Systems with Time-Varying Delay. Entropy 2022, 24, 828. https://doi.org/10.3390/e24060828
Liu X, Li W, Wang J, Li Y. Robust Finite-Time Stability for Uncertain Discrete-Time Stochastic Nonlinear Systems with Time-Varying Delay. Entropy. 2022; 24(6):828. https://doi.org/10.3390/e24060828
Chicago/Turabian StyleLiu, Xikui, Wencong Li, Jiqiu Wang, and Yan Li. 2022. "Robust Finite-Time Stability for Uncertain Discrete-Time Stochastic Nonlinear Systems with Time-Varying Delay" Entropy 24, no. 6: 828. https://doi.org/10.3390/e24060828
APA StyleLiu, X., Li, W., Wang, J., & Li, Y. (2022). Robust Finite-Time Stability for Uncertain Discrete-Time Stochastic Nonlinear Systems with Time-Varying Delay. Entropy, 24(6), 828. https://doi.org/10.3390/e24060828






