Quantum Information Entropies on Hyperbolic Single Potential Wells
Abstract
:1. Introduction
2. Formalism
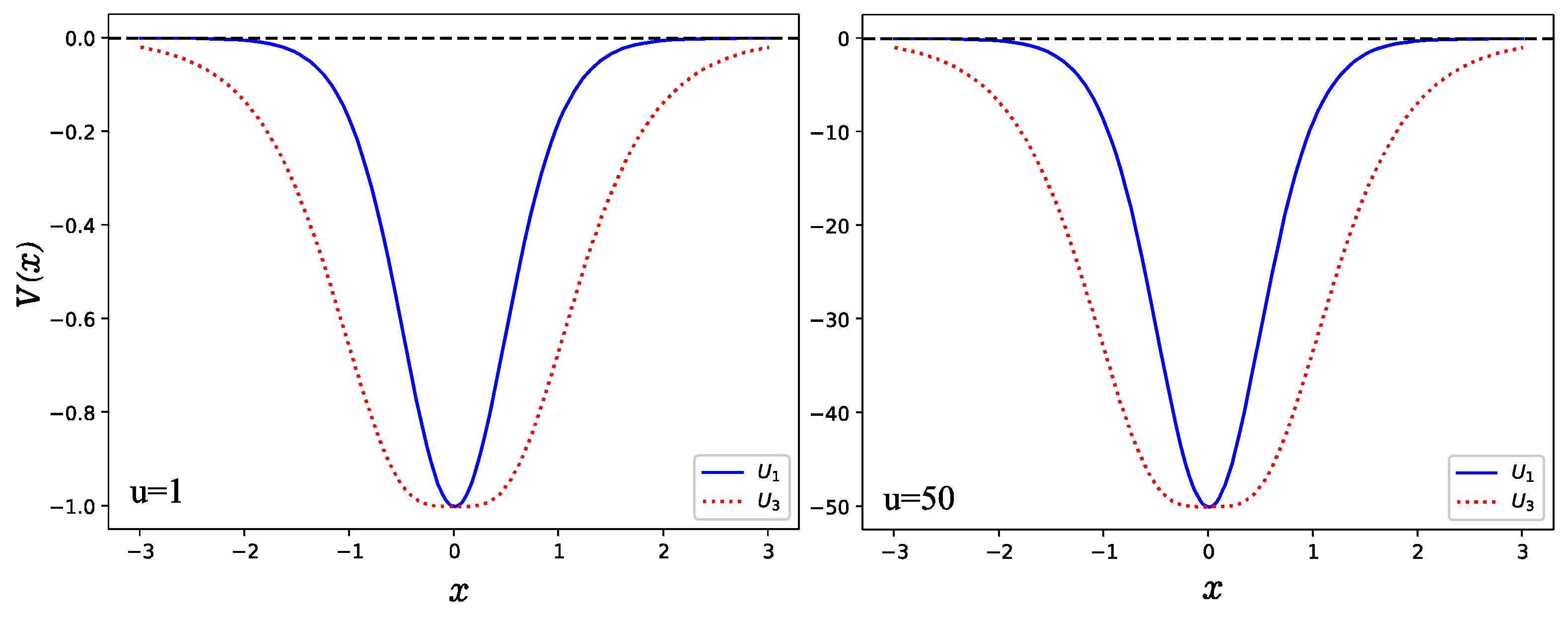
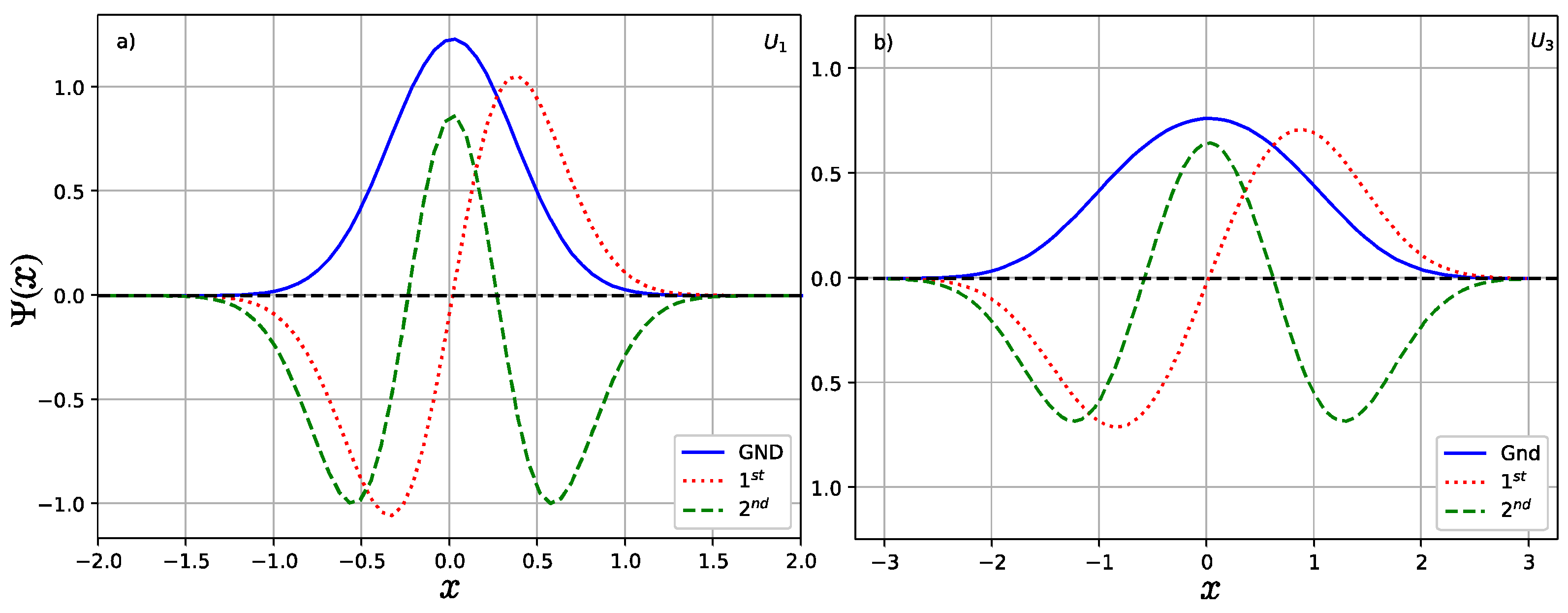
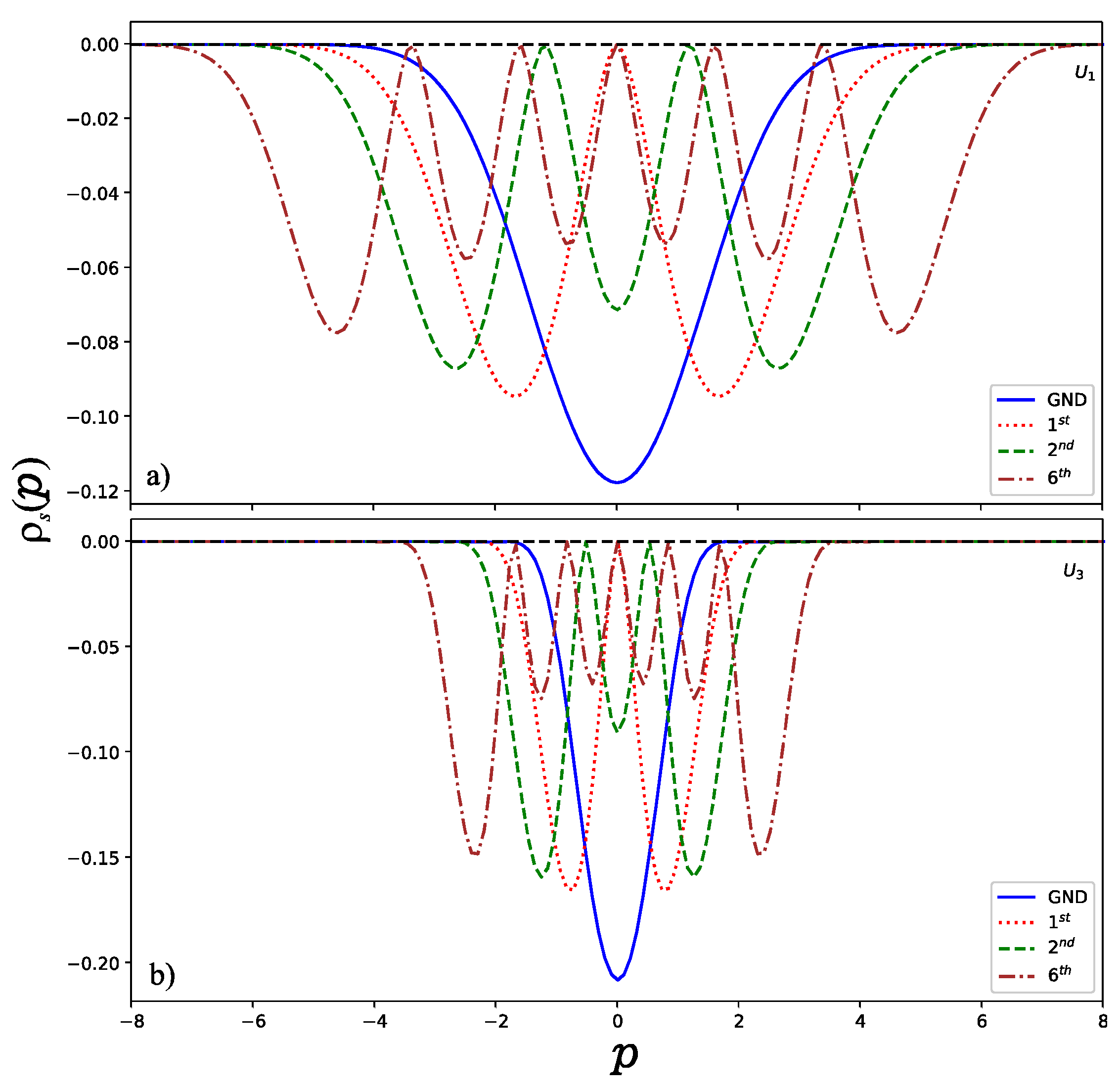
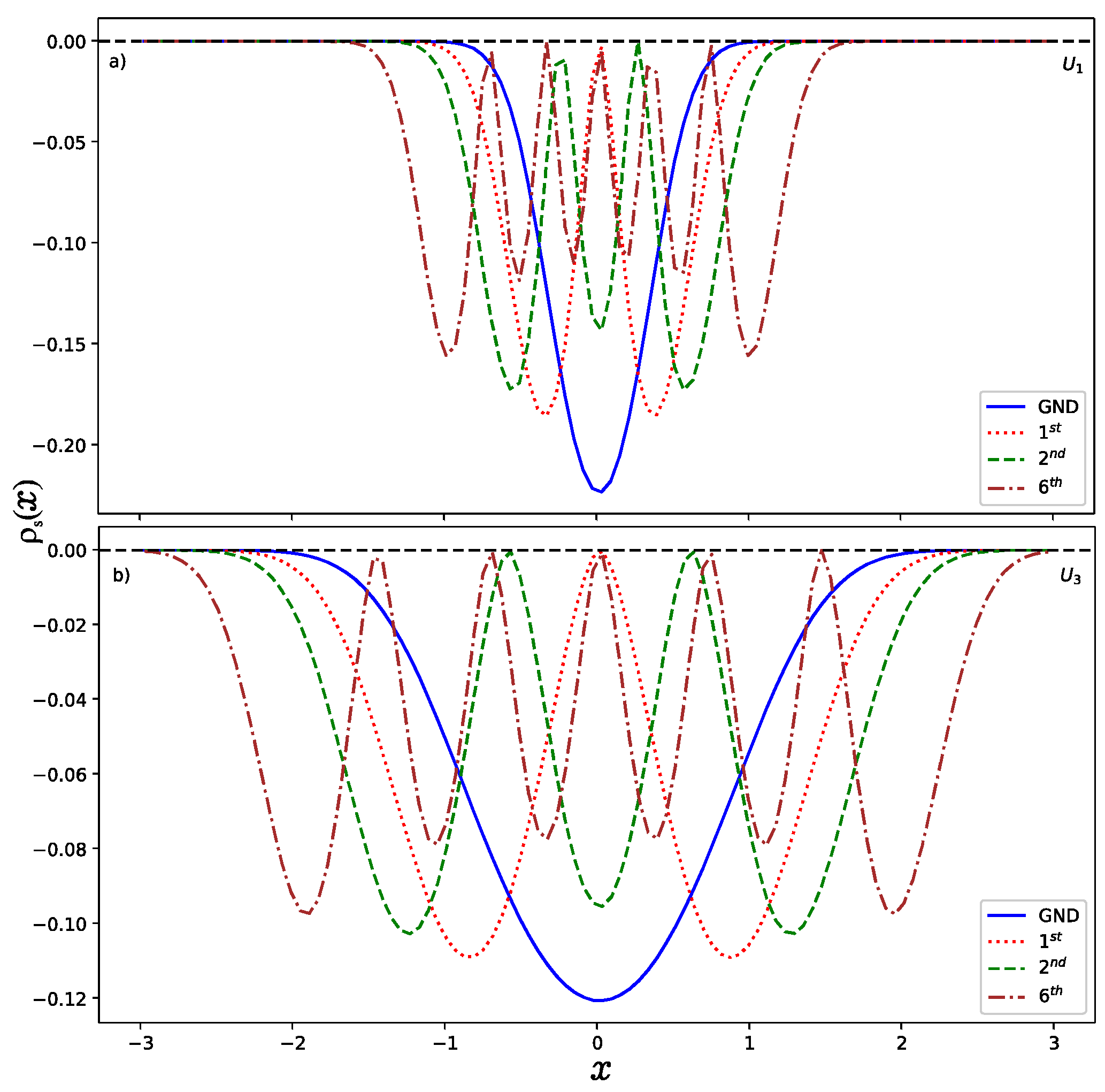
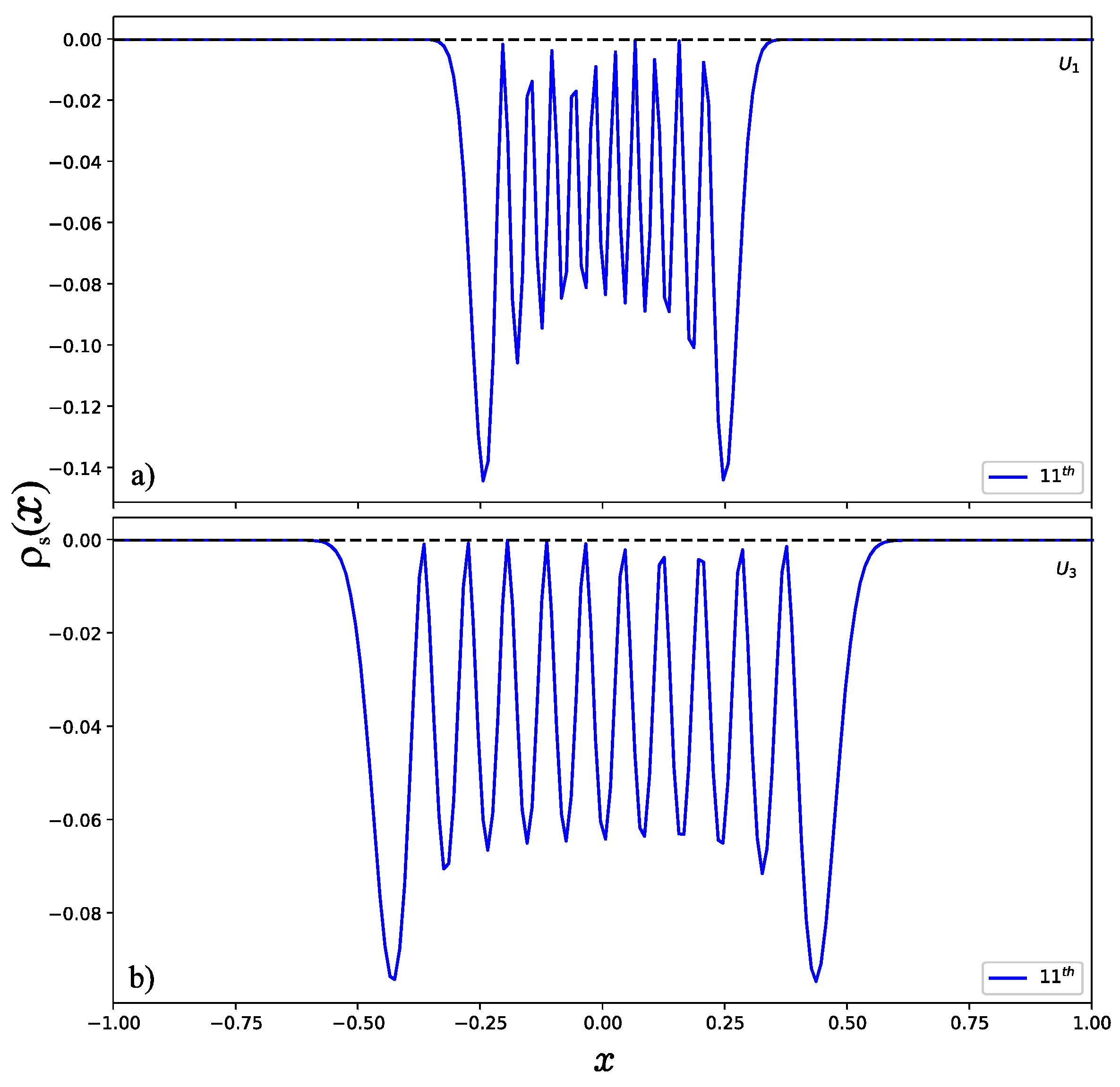
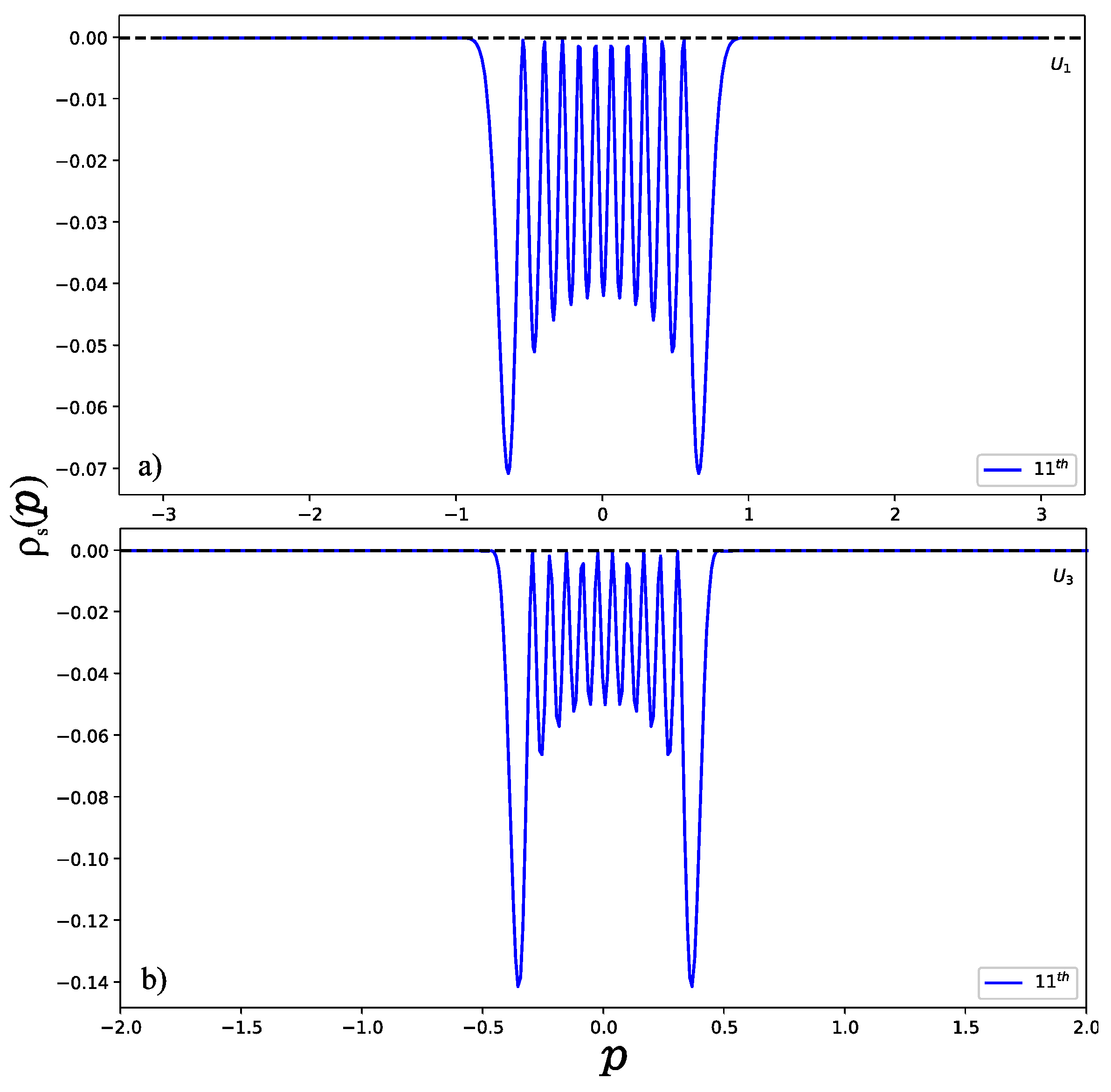
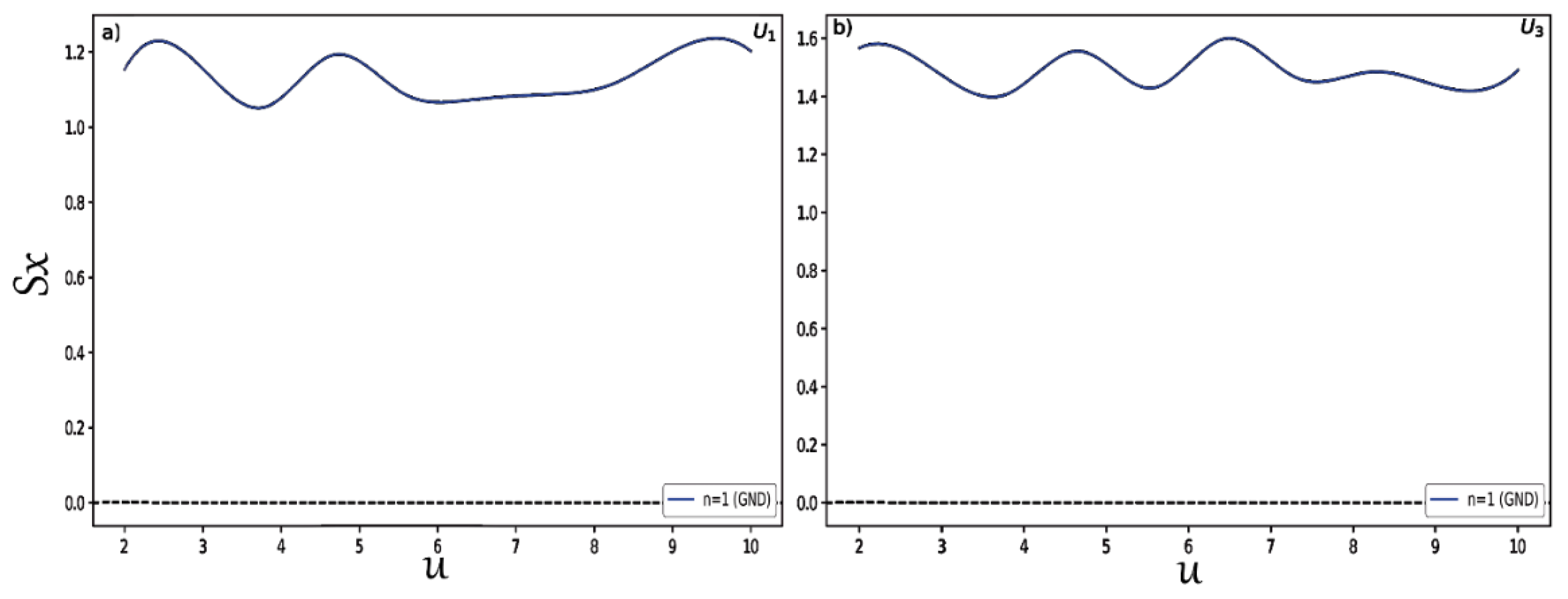
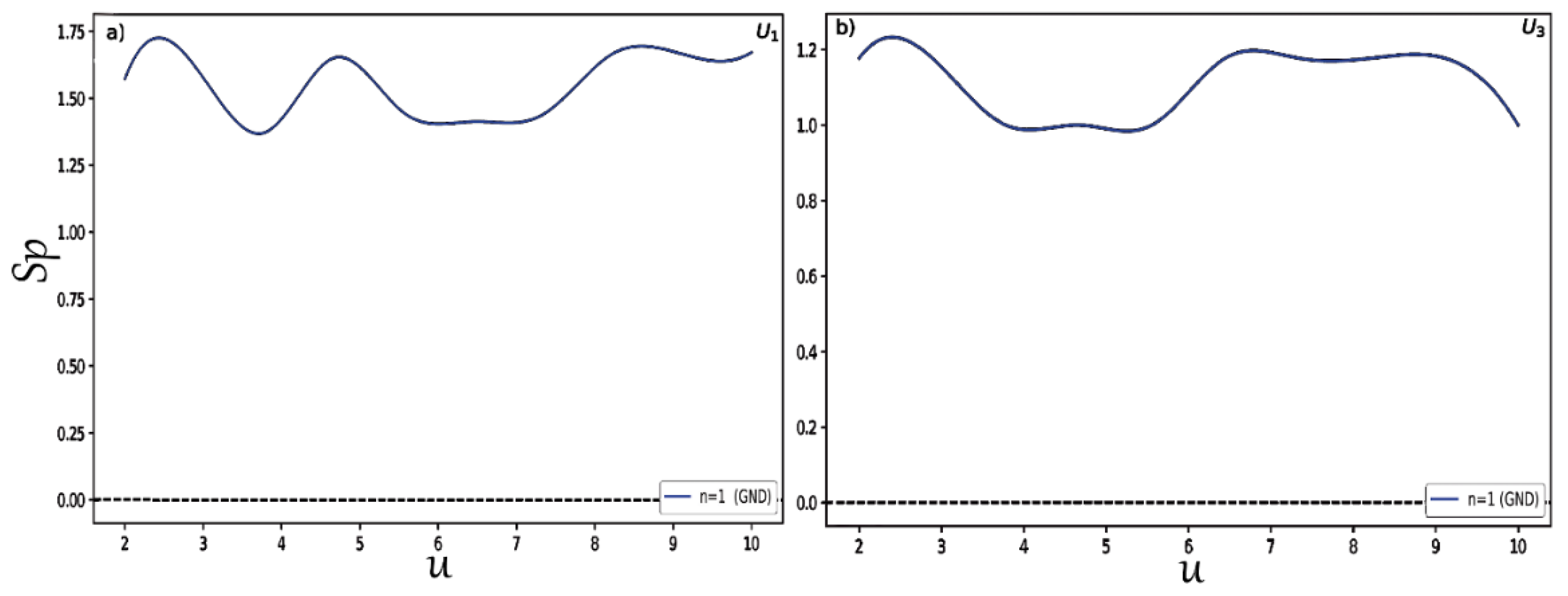
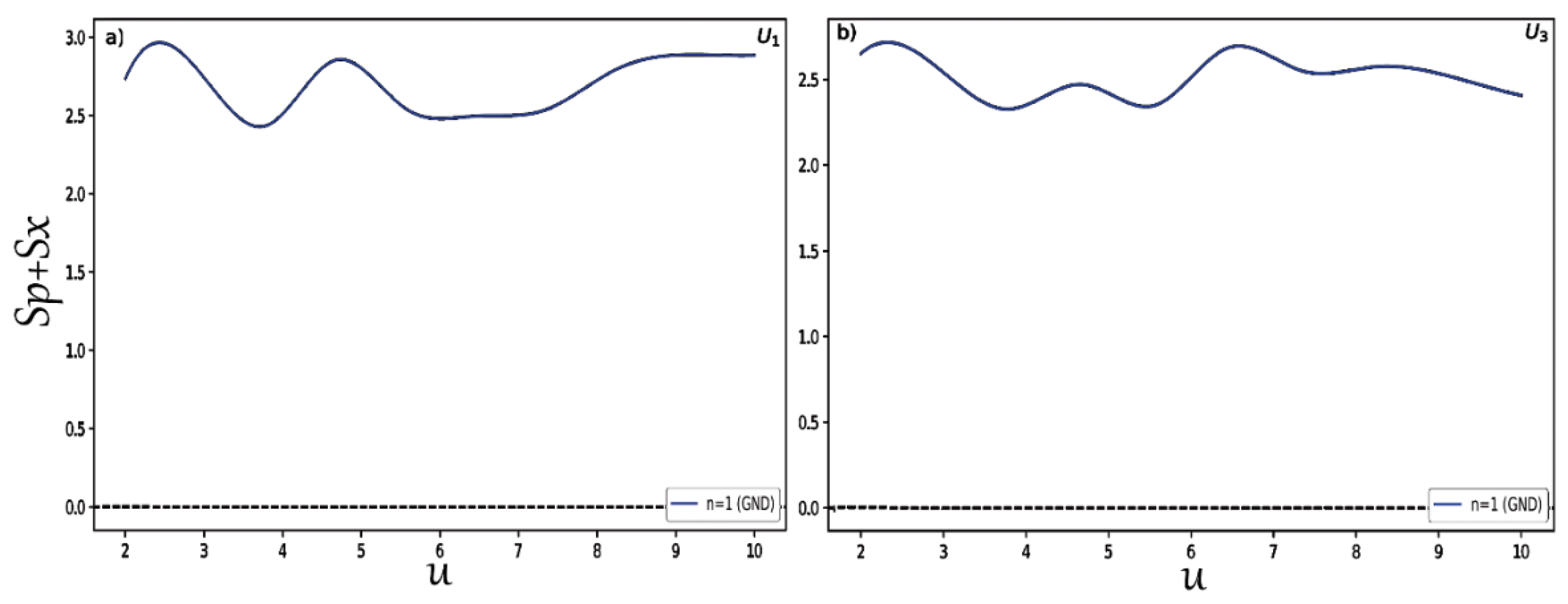
3. Results and Discussions
4. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Simos, T.E.; Williams, P.S. A finite-difference method for the numerical solution of the Schrödinger equation. J. Comput. Appl. Math. 1997, 79, 189. [Google Scholar] [CrossRef] [Green Version]
- Sanz-Serna, J.M. Methods for the numerical solution of the nonlinear Schrödinger equation. Math. Comput. 1984, 43, 21. [Google Scholar] [CrossRef]
- Dehesa, J.S.; Van Assche, W.; Yáñez, R.J. Information entropy of classical orthogonal polynomials and their application to the harmonic oscillator and Coulomb potentials. Methods Appl. Math. 1997, 4, 91. [Google Scholar] [CrossRef] [Green Version]
- Bialynicki-Birula, I.; Mycielski, J. Uncertainty relations for information entropy in wave mechanics. Commun. Math. Phys. 1975, 44, 129. [Google Scholar] [CrossRef]
- Wilde, M.M. From classical to quantum Shannon theory. arXiv 2011, arXiv:1106.1445. [Google Scholar]
- Merhav, N. Physics of the Shannon limits. IEEE Trans. Inf. Theory 2010, 56, 4274. [Google Scholar] [CrossRef]
- Hellman, M. An extension of the Shannon theory approach to cryptography. IEEE Trans. Inf. Theory 1977, 23, 289. [Google Scholar] [CrossRef]
- Alipour, M.; Mohajeri, A. Onicescu information energy in terms of Shannon entropy and Fisher information densities. Mol. Phys. 2012, 110, 403. [Google Scholar] [CrossRef]
- Xu, Q. Measuring information content from observations for data assimilation: Relative entropy versus Shannon entropy difference. Tellus A: Dyn. Meteorol. Oceanogr. 2007, 59, 198. [Google Scholar] [CrossRef]
- Akçakaya, M.; Tarokh, V. Shannon-theoretic limits on noisy compressive sampling. IEEE Trans. Inf. Theory 2009, 56, 492. [Google Scholar] [CrossRef]
- Bruhn, J.; Lehmann, L.E.; Röpcke, H.; Bouillon, T.W.; Hoeft, A. Shannon entropy applied to the measurement of the electroencephalographic effects of desflurane. J. Am. Soc. Anesthesiol. 2001, 95, 30. [Google Scholar] [CrossRef] [PubMed]
- Sun, G.H.; Dong, S.H. Quantum information entropies of the eigenstates for a symmetrically trigonometric Rosen–Morse potential. Phys. Scr. 2013, 87, 045003. [Google Scholar] [CrossRef]
- Sun, G.H.; Dong, S.H.; Launey, K.D.; Dytrych, T.; Draayer, J.P. Shannon information entropy for a hyperbolic double-well potential. Int. J. Quant. Chem. 2015, 115, 891. [Google Scholar] [CrossRef]
- Sun, G.H.; Aoki, M.A.; Dong, S.H. Quantum information entropies of the eigenstates for the Pöschl—Teller-like potential. Chin. Phys. B 2013, 22, 050302. [Google Scholar] [CrossRef]
- Song, X.D.; Sun, G.H.; Dong, S.H. Shannon information entropy for an infinite circular well. Phys. Lett. A 2015, 379, 1402. [Google Scholar] [CrossRef]
- Majerník, V.; Opatrný, T.J. Entropic uncertainty relations for a quantum oscillator. Phys. A 1996, 29, 2187. [Google Scholar] [CrossRef]
- Hazra, R.K.; Ghosh, M.; Bhattacharyya, S.P. Information entropy and level-spacing distribution based signatures of quantum chaos in electron doped 2D single carrier quantum dots. Chem. Phys. Lett. 2008, 460, 209. [Google Scholar] [CrossRef]
- Majerník, V.; Majerníková, E. Entropic uncertainty relations for a quantum oscillator. J. Phys. A 2002, 35, 5751. [Google Scholar] [CrossRef]
- Yáñez, R.J.; Van Assche, W.; Dehesa, J.S. Position and momentum information entropies of the D-dimensional harmonic oscillator and hydrogen atom. Phys. Rev. A 1994, 50, 3065. [Google Scholar] [CrossRef]
- Van Assche, W.; Yáñez, R.J.; Dehesa, J.S. Entropy of orthogonal polynomials with Freud weights and information entropies of the harmonic oscillator potential. J. Math. Phys. 1995, 36, 4106. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.H.; Chen, C.Y.; You, Y.; Lu, F.L.; Sun, D.S.; Dong, S.H. Exact solutions of the Schrödinger equation for a class of hyperbolic potential well. Chin. Phys. B 2022, 31, 040301. [Google Scholar] [CrossRef]
- Santana, R.; Carrillo, C.; Gil-Barrera, A.; Sun, G.H.; Solaimani, M.; Dong, S.H. Shannon entropies of asymmetric multiple quantum well systems with a constant total length. Eur. Phys. J. Plus 2021, 136, 1060. [Google Scholar]
- Solaimani, M.; Sun, G.H.; Dong, S.H. Shannon information entropies for rectangular multiple quantum well systems with constant total lengths. Chin. Phys. B 2018, 27, 040301. [Google Scholar] [CrossRef]
- Solaimani, M.; Dong, S.H. Quantum information entropies of multiple quantum well systems in fractional Schrödinger equations. Int. J. Quan. Chem. 2020, 120, e26113. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gil-Barrera, C.A.; Santana Carrillo, R.; Sun, G.-H.; Dong, S.-H. Quantum Information Entropies on Hyperbolic Single Potential Wells. Entropy 2022, 24, 604. https://doi.org/10.3390/e24050604
Gil-Barrera CA, Santana Carrillo R, Sun G-H, Dong S-H. Quantum Information Entropies on Hyperbolic Single Potential Wells. Entropy. 2022; 24(5):604. https://doi.org/10.3390/e24050604
Chicago/Turabian StyleGil-Barrera, Carlos Ariel, Raymundo Santana Carrillo, Guo-Hua Sun, and Shi-Hai Dong. 2022. "Quantum Information Entropies on Hyperbolic Single Potential Wells" Entropy 24, no. 5: 604. https://doi.org/10.3390/e24050604
APA StyleGil-Barrera, C. A., Santana Carrillo, R., Sun, G.-H., & Dong, S.-H. (2022). Quantum Information Entropies on Hyperbolic Single Potential Wells. Entropy, 24(5), 604. https://doi.org/10.3390/e24050604







