Chaotic Time-Delay Signature Suppression and Entropy Growth Enhancement Using Frequency-Band Extractor
Abstract
:1. Introduction
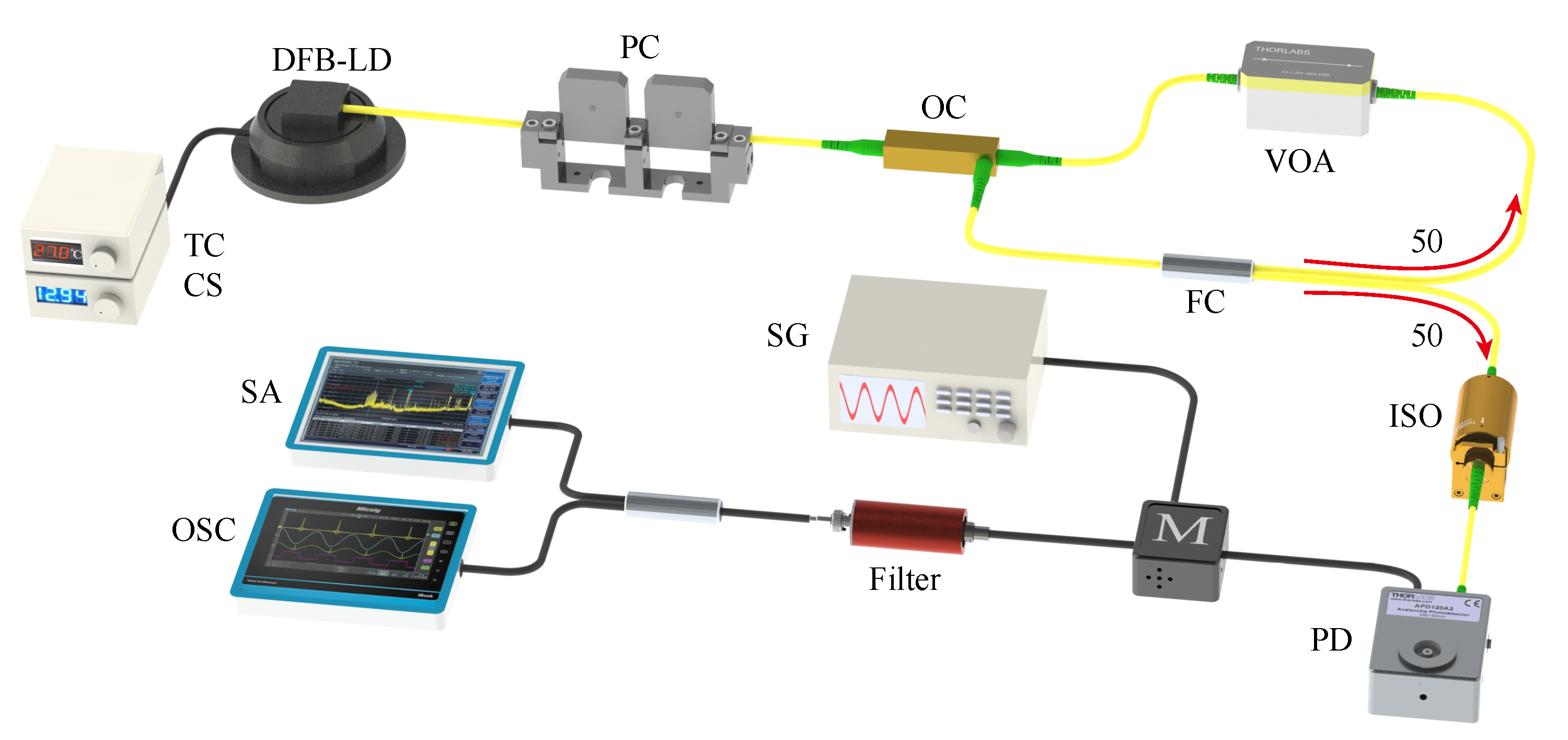
2. Experimental Setup
3. Theoretical Model and Methods
3.1. Lang–Kobayashi and Frequency-Band Extraction Model
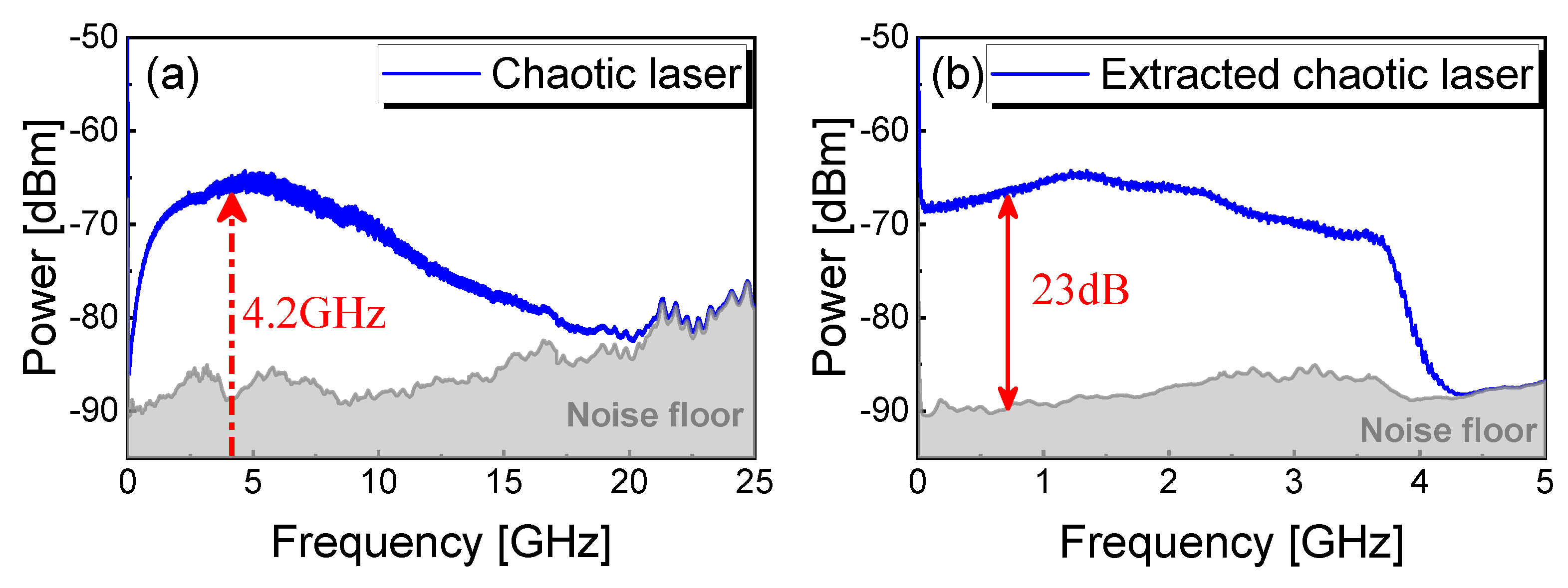
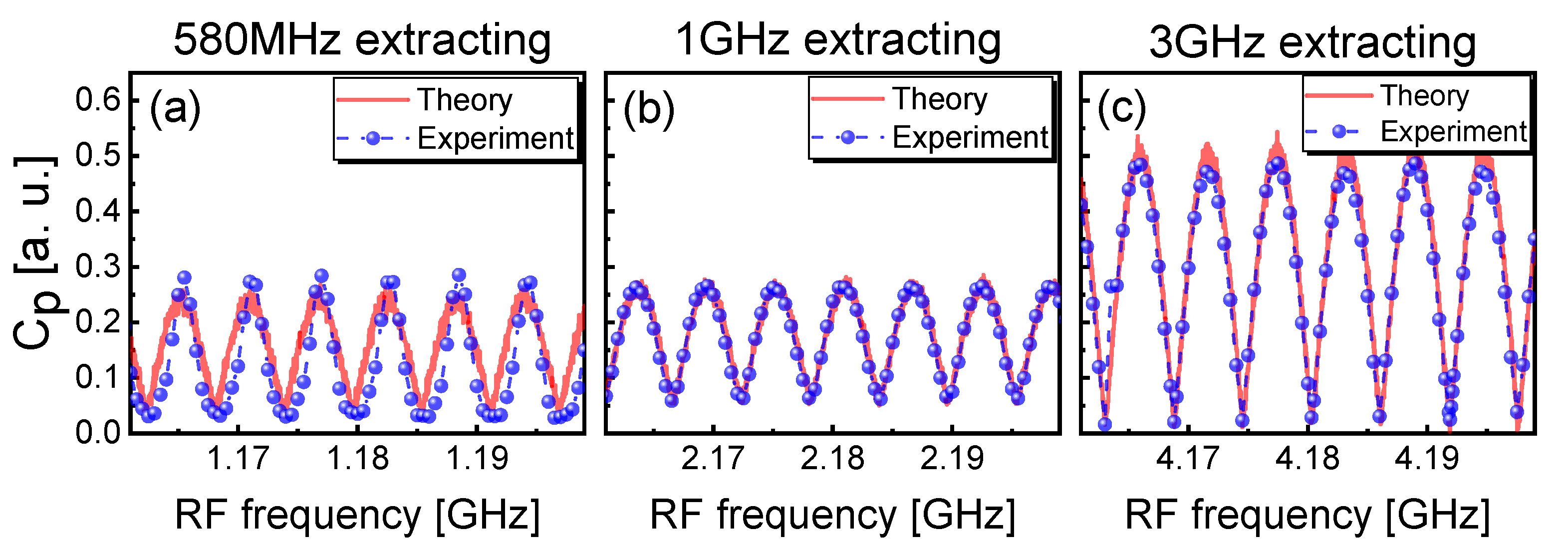
3.2. Time Delay Signature
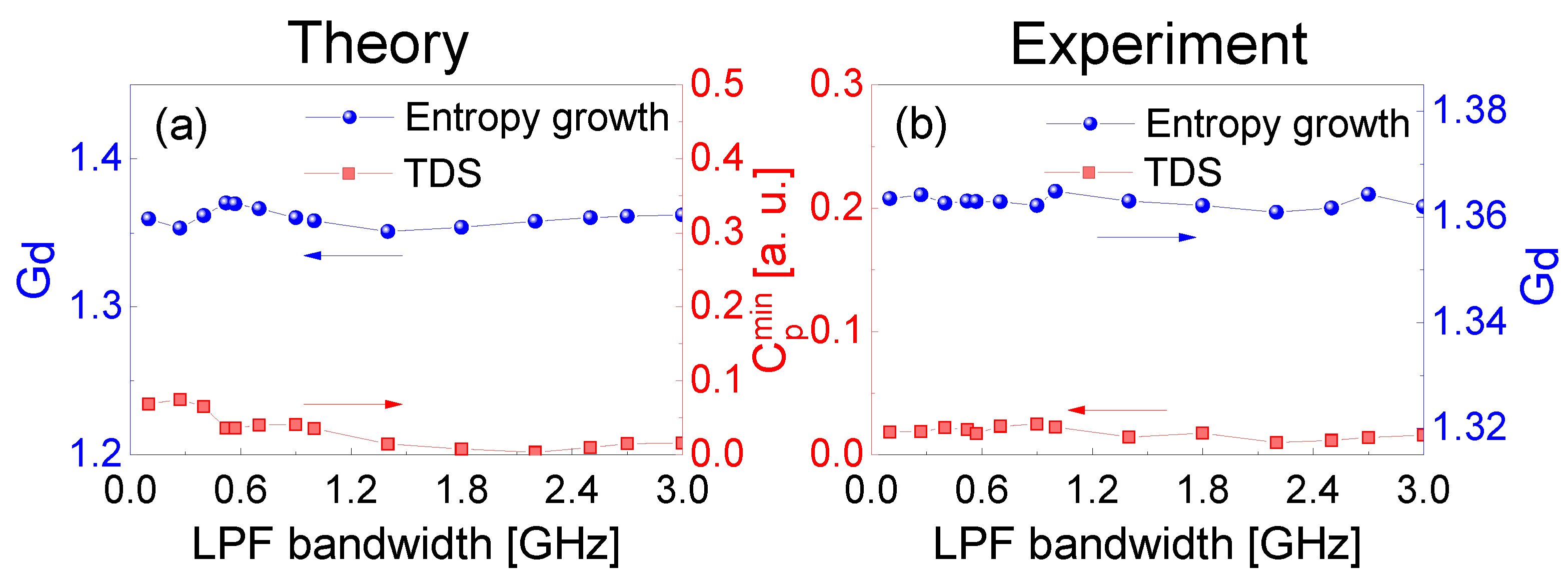
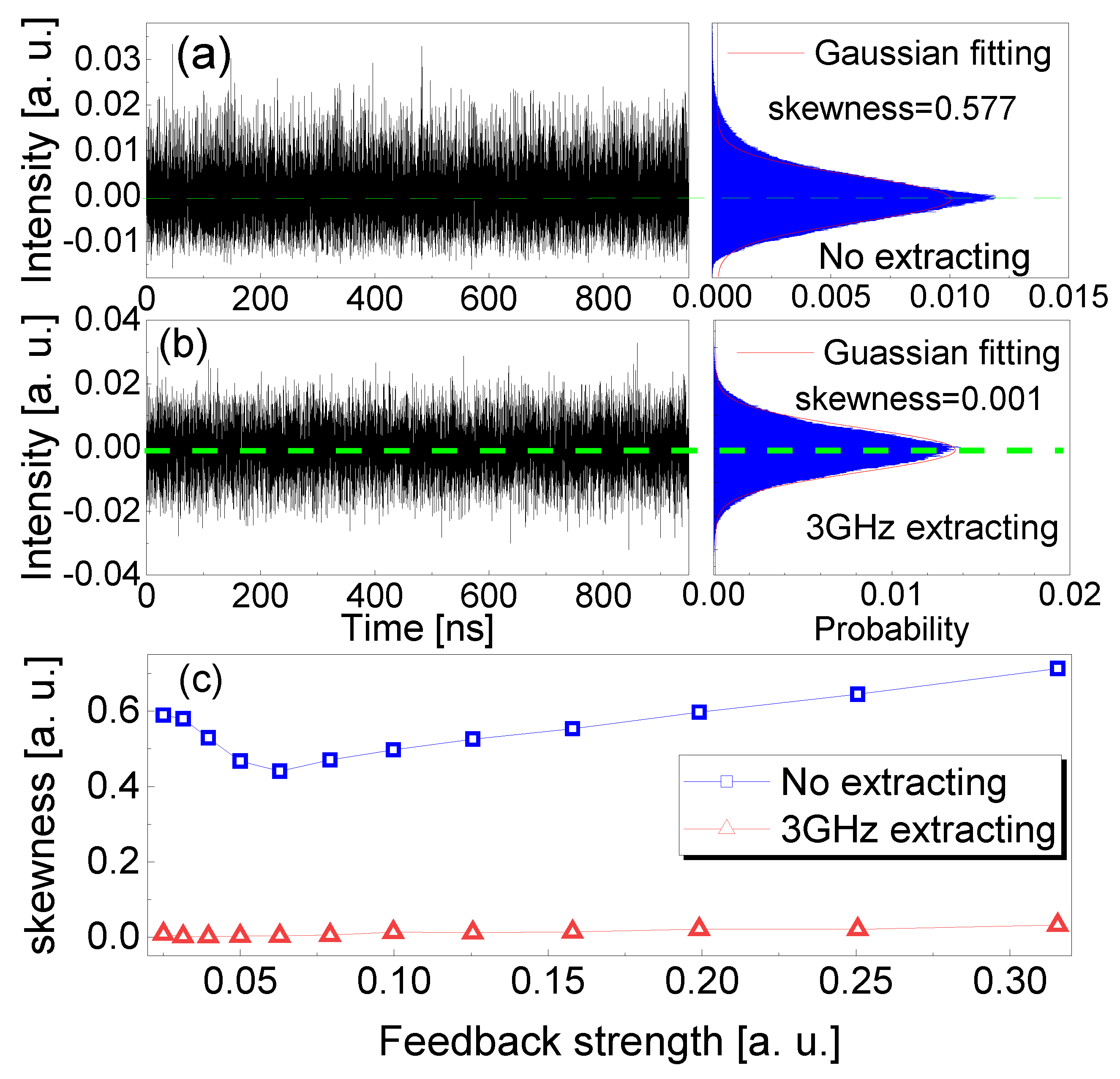
3.3. Entropy Growth
4. Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Soriano, M.C.; García-Ojalvo, J.; Mirasso, C.R.; Fischer, I. Complex photonics: Dynamics and applications of delay-coupled semiconductors lasers. Rev. Mod. Phys. 2013, 85, 421–470. [Google Scholar] [CrossRef] [Green Version]
- Sciamanna, M.; Shore, K.A. Physics and applications of laser diode chaos. Nat. Photonics 2015, 9, 151–162. [Google Scholar] [CrossRef] [Green Version]
- Chembo, Y.K.; Brunner, D.; Jacquot, M.; Larger, L. Optoelectronic oscillators with time-delayed feedback. Rev. Mod. Phys. 2019, 91, 035006. [Google Scholar] [CrossRef]
- Sunada, S.; Harayama, T.; Davis, P.; Tsuzuki, K.; Arai, K.; Yoshimura, K.; Uchida, A. Noise amplification by chaotic dynamics in a delayed feedback laser system and its application to nondeterministic random bit generation. Chaos 2012, 22, 047513. [Google Scholar] [CrossRef] [PubMed]
- Hart, J.D.; Terashima, Y.; Uchida, A.; Baumgartner, G.B.; Murphy, T.E.; Roy, R. Recommendations and illustrations for the evaluation of photonic random number generators. APL Photonics 2017, 2, 090901. [Google Scholar] [CrossRef]
- Argyris, A.; Syvridis, D.; Larger, L.; Annovazzi-Lodi, V.; Colet, P.; Fischer, I.; García-Ojalvo, J.; Mirasso, C.R.; Pesquera, L.; Shore, K.A. Chaos-based communications at high bit rates using commercial fibre-optic links. Nature 2005, 438, 343–346. [Google Scholar] [CrossRef] [PubMed]
- Kusumoto, K.; Ohtsubo, J. 1.5-GHz message transmission based on synchronization of chaos in semiconductor lasers. Opt. Lett. 2002, 27, 989–991. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jiang, N.; Pan, W.; Yan, L.; Luo, B.; Zhang, W.; Xiang, S.; Yang, L.; Zheng, D. Chaos synchronization and communication in mutually coupled semiconductor lasers driven by a third laser. J. Lightw. Technol. 2010, 28, 1978–1986. [Google Scholar] [CrossRef]
- Lin, F.Y.; Liu, J.M. Chaotic lidar. IEEE J. Sel. Top. Quantum Electron. 2004, 10, 991–997. [Google Scholar] [CrossRef]
- Wang, Y.C.; Wang, B.J.; Wang, A.B. Chaotic correlation optical time domain reflectometer utilizing laser diode. IEEE Photonics Technol. Lett. 2008, 20, 1636–1638. [Google Scholar] [CrossRef]
- Ma, Z.; Zhang, M.; Liu, Y.; Bao, X.; Liu, H.; Zhang, Y.; Wang, Y. Incoherent brillouin optical time-domain reflectometry with random state correlated brillouin spectrum. IEEE Photonics J. 2015, 7, 1–7. [Google Scholar] [CrossRef]
- Uchida, A.; Amano, K.; Inoue, M.; Hirano, K.; Naito, S.; Someya, H.; Oowada, I.; Kurashige, T.; Shiki, M.; Yoshimori, S.; et al. Fast physical random bit generation with chaotic semiconductor lasers. Nat. Photonics 2008, 2, 728–732. [Google Scholar] [CrossRef]
- Wang, A.; Wang, L.; Li, P.; Wang, Y. Minimal-post-processing 320-Gbps true random bit generation using physical white chaos. Opt. Express 2017, 25, 3153–3164. [Google Scholar] [CrossRef]
- Virte, M.; Mercier, E.; Thienpont, H.; Panajotov, K.; Sciamanna, M. Physical random bit generation from chaotic solitary laser diode. Opt. Express 2014, 22, 17271–17280. [Google Scholar] [CrossRef] [Green Version]
- Wu, J.G.; Wu, Z.M.; Xia, G.Q.; Feng, G.Y. Evolution of time delay signature of chaos generated in a mutually delay-coupled semiconductor lasers system. Opt. Express 2012, 20, 1741–1753. [Google Scholar] [CrossRef] [PubMed]
- Hong, Y. Experimental study of time-delay signature of chaos in mutually coupled vertical-cavity surface-emitting lasers subject to polarization optical injection. Opt. Express 2013, 21, 17894–17903. [Google Scholar] [CrossRef]
- Mu, P.; He, P.; Li, N. Simultaneous Chaos Time-Delay Signature Cancellation and Bandwidth Enhancement in Cascade-Coupled Semiconductor Ring Lasers. IEEE Access 2019, 7, 11041–11048. [Google Scholar] [CrossRef]
- Li, S.S.; Chan, S.C. Chaotic time-delay signature suppression in a semiconductor laser with frequency-detuned grating feedback. IEEE J. Sel. Top. Quantum Electron. 2015, 21, 547–552. [Google Scholar]
- Zhong, Z.Q.; Wu, Z.M.; Xia, G.Q. Experimental investigation on the time-delay signature of chaotic output from a 1550 nm VCSEL subject to FBG feedback. Photonics Res. 2017, 5, 6–10. [Google Scholar] [CrossRef]
- Xu, Y.; Zhang, M.; Zhang, L.; Lu, P.; Bao, X. Time-delay signature suppression in a chaotic semiconductor laser by fiber random grating induced random distributed feedback. Opt. Lett. 2017, 42, 1–16. [Google Scholar] [CrossRef] [Green Version]
- Cheng, M.; Luo, C.; Jiang, X.; Deng, L.; Zhang, M.; Ke, C.; Fu, S.; Tang, M.; Shum, P.; Liu, D. An electro-optic chaotic system based on a hybrid feedback loop. J. Lightw. Technol. 2018, 36, 4259–4266. [Google Scholar] [CrossRef]
- Xiang, S.; Pan, W.; Zhang, L.; Wen, A.; Shang, L.; Zhang, H.; Lin, L. Phase-modulated dual-path feedback for time delay signature suppression from intensity and phase chaos in semiconductor laser. Opt. Commun. 2014, 324, 38–46. [Google Scholar] [CrossRef]
- Mu, P.; Pan, W.; Yan, L.; Luo, B.; Zou, X. Concealment of Chaos Time-Delay Signature Through Phase-Conjugate Feedback and Chaos Optical Injection. IEEE Photonics J. 2017, 9, 1505608. [Google Scholar] [CrossRef]
- Zhao, A.; Jiang, N.; Liu, S.; Xue, C.; Qiu, K. Wideband Time Delay Signature-Suppressed Chaos Generation Using Self-Phase-Modulated Feedback Semiconductor Laser Cascaded With Dispersive Component. J. Lightw. Technol. 2019, 37, 5132–5139. [Google Scholar] [CrossRef]
- Zhang, R.; Zhou, P.; Yang, Y.; Fang, Q.; Mu, P.; Li, N. Enhancing time-delay suppression in a semiconductor laser with chaotic optical injection via parameter mismatch. Opt. Express 2020, 28, 7197–7206. [Google Scholar] [CrossRef] [PubMed]
- Kanter, I.; Aviad, Y.; Reidler, I.; Cohen, E.; Rosenbluh, M. An optical ultrafast random bit generator. Nat. Photonics 2010, 4, 58–61. [Google Scholar] [CrossRef]
- Cheng, C.H.; Chen, Y.C.; Lin, F.Y. Chaos time delay signature suppression and bandwidth enhancement by electrical heterodyning. Opt. Express 2015, 23, 2308–2319. [Google Scholar] [CrossRef]
- Ji, S.; Hong, Y. Effect of Bias Current on Complexity and Time Delay Signature of Chaos in Semiconductor Laser with Time-Delayed Optical Feedback. IEEE J. Sel. Top. Quantum Electron. 2017, 23, 1–6. [Google Scholar] [CrossRef] [Green Version]
- Bandt, C.; Pompe, B. Permutation entropy: A natural complexity measure for time series. Phys. Rev. Lett. 2002, 88, 174102. [Google Scholar] [CrossRef] [PubMed]
- Toomey, J.P.; Kane, D.M. Mapping the dynamic complexity of a semiconductor laser with optical feedback using permutation entropy. Opt. Express 2014, 22, 1713–1725. [Google Scholar] [CrossRef]
- Li, N.; Pan, W.; Locquet, A.; Chizhevsky, V.N.; Citrin, D.S. Statistical Properties of an External-Cavity Semiconductor Laser: Experiment and Theory. IEEE J. Sel. Top. Quantum Electron. 2015, 21, 553–560. [Google Scholar]
- Guo, X.; Liu, T.; Wang, L.; Fang, X.; Zhao, T.; Virte, M.; Guo, Y. Evaluating entropy rate of laser chaos and shot noise. Opt. Express 2020, 28, 1238–1248. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Quintero-Quiroz, C.; Pigolotti, S.; Torrent, M.C.; Masoller, C. Numerical and experimental study of the effects of noise on the permutation entropy. New J. Phys. 2015, 17, 093002. [Google Scholar] [CrossRef]
- Lang, R.; Kobayashi, K. External optical feedback effects on semiconductor injection laser properties. IEEE J. Quantum Electron. 1980, 16, 347–355. [Google Scholar] [CrossRef]
- Guo, Y.; Peng, C.; Ji, Y.; Li, P.; Guo, Y.; Guo, X. Photon statistics and bunching of a chaotic semiconductor laser. Opt. Express 2018, 26, 5991–6000. [Google Scholar] [CrossRef] [PubMed] [Green Version]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Y.; Liu, T.; Zhao, T.; Zhang, H.; Guo, X. Chaotic Time-Delay Signature Suppression and Entropy Growth Enhancement Using Frequency-Band Extractor. Entropy 2021, 23, 516. https://doi.org/10.3390/e23050516
Guo Y, Liu T, Zhao T, Zhang H, Guo X. Chaotic Time-Delay Signature Suppression and Entropy Growth Enhancement Using Frequency-Band Extractor. Entropy. 2021; 23(5):516. https://doi.org/10.3390/e23050516
Chicago/Turabian StyleGuo, Yanqiang, Tong Liu, Tong Zhao, Haojie Zhang, and Xiaomin Guo. 2021. "Chaotic Time-Delay Signature Suppression and Entropy Growth Enhancement Using Frequency-Band Extractor" Entropy 23, no. 5: 516. https://doi.org/10.3390/e23050516
APA StyleGuo, Y., Liu, T., Zhao, T., Zhang, H., & Guo, X. (2021). Chaotic Time-Delay Signature Suppression and Entropy Growth Enhancement Using Frequency-Band Extractor. Entropy, 23(5), 516. https://doi.org/10.3390/e23050516








