Auto- versus Cross-Correlation Noise in Periodically Driven Quantum Coherent Conductors
Abstract
1. Introduction
2. Electrical Noise and Electron Correlation Function
2.1. Frequency-Dependent Noise
2 × 2 Circuit
2.2. First-Order Correlation Function
2.3. Noise Power in Terms of
3. Examples
3.1. Energy-Independent Source
3.2. Injection from a Quantum Level Raising at a Constant Rapidity
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Auto-Correlation Noise from Equation (9)

Appendix B. Relation between an Electrical Noise and an Electron Correlation Function
Appendix B.1. Auto-Correlation Nose
Appendix B.1.1. Equilibrium Noise,
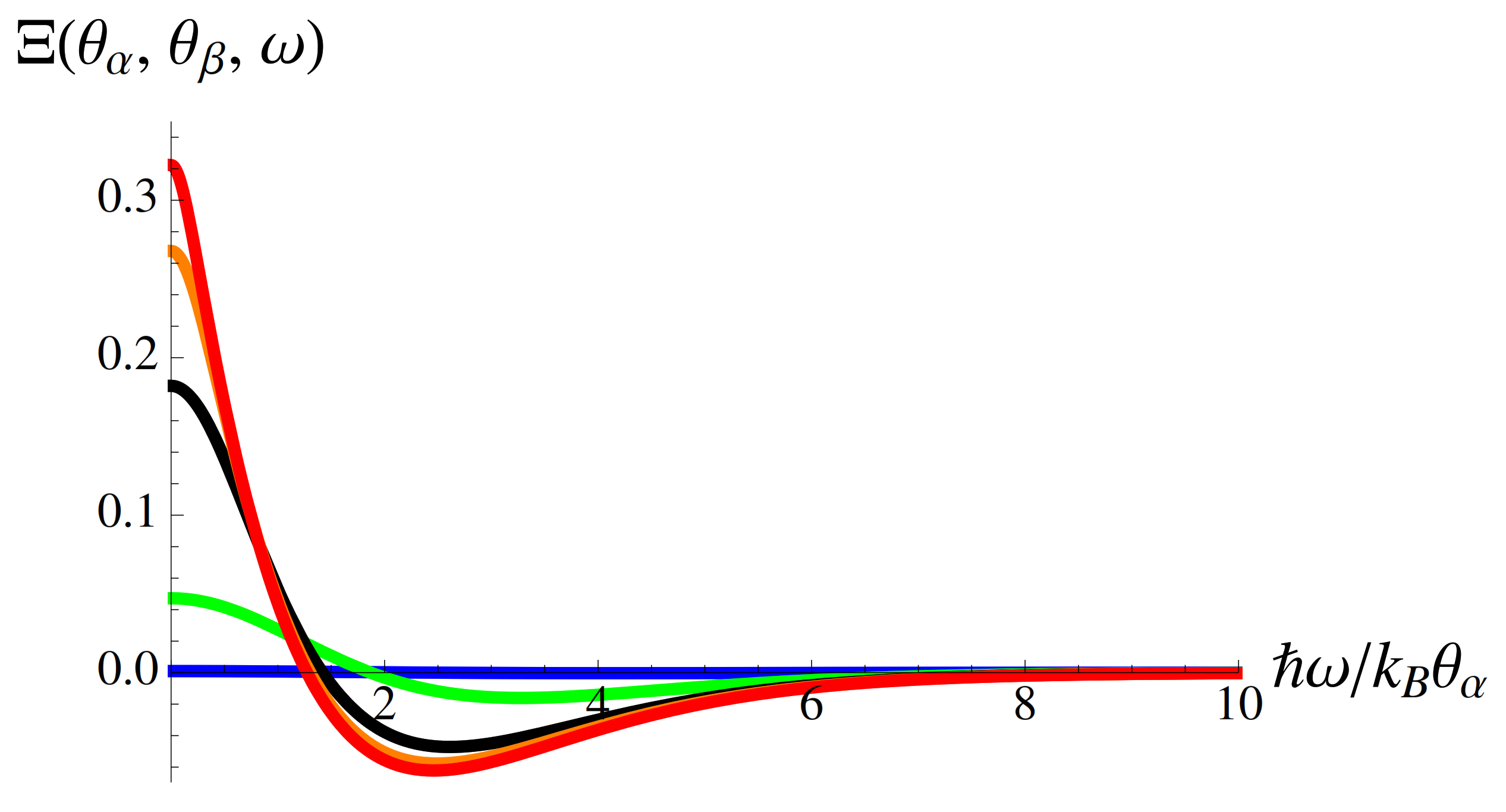
Appendix B.1.2. Excess Noise,
Appendix B.2. Cross-Correlation Nose
Appendix B.3. The Excess Noise Conservation at Zero Frequency
Appendix B.4. Noise and the Outgoing Correlation Matrix of a Linear Electronic Circuit
Appendix C. Noise Caused by an Electron Injected from a Quantum Level Raising at a Constant Rapidity at Zero Temperature
Appendix C.1. The Term with
Appendix C.2. The Term with
Appendix C.3. Excess Noise Power
Appendix D. Noise Caused by an Electron Injected from a Quantum Level Raising at a Constant Rapidity at Non-Zero Temperature
Appendix D.1. The Term with
Appendix D.2. The Term with
Appendix D.3. Excess Noise Power
References
- Jullien, T.; Roulleau, P.; Roche, B.; Cavanna, A.; Jin, Y.; Glattli, D.C. Quantum tomography of an electron. Nature 2014, 514, 603–607. [Google Scholar] [CrossRef] [PubMed]
- Bisognin, R.; Marguerite, A.; Roussel, B.; Kumar, M.; Cabart, C.; Chapdelaine, C.; Mohammad-Djafari, A.; Berroir, J.M.; Bocquillon, E.; Plaçais, B.; et al. Quantum tomography of electrical currents. Nat. Commun. 2019, 10, 3379. [Google Scholar] [CrossRef] [PubMed]
- Bocquillon, E.; Parmentier, F.D.; Grenier, C.; Berroir, J.M.; Degiovanni, P.; Glattli, D.C.; Plaçais, B.; Cavanna, A.; Jin, Y.; Fève, G. Electron Quantum Optics: Partitioning Electrons One by One. Phys. Rev. Lett. 2012, 108, 196803. [Google Scholar] [CrossRef]
- Dubois, J.; Jullien, T.; Portier, F.; Roche, P.; Cavanna, A.; Jin, Y.; Wegscheider, W.; Roulleau, P.; Glattli, D.C. Minimal-excitation states for electron quantum optics using levitons. Nature 2013, 502, 659–663. [Google Scholar] [CrossRef] [PubMed]
- Bocquillon, E.; Freulon, V.; Berroir, J.M.; Degiovanni, P.; Plaçais, B.; Cavanna, A.; Jin, Y.; Fève, G. Coherence and Indistinguishability of Single Electrons Emitted by Independent Sources. Science 2013, 339, 1054–1057. [Google Scholar] [CrossRef]
- Marguerite, A.; Bocquillon, E.; Berroir, J.M.; Plaçais, B.; Cavanna, A.; Jin, Y.; Degiovanni, P.; Fève, G. Two-particle interferometry in quantum Hall edge channels. Phys. Status Solidi 2016, 254, 1600618. [Google Scholar] [CrossRef]
- Glattli, D.C.; Roulleau, P.S. Levitons for electron quantum optics. Phys. Status Solidi 2016, 254, 1600650. [Google Scholar] [CrossRef]
- Mahé, A.; Parmentier, F.D.; Bocquillon, E.; Berroir, J.M.; Glattli, D.C.; Kontos, T.; Plaçais, B.; Fève, G.; Cavanna, A.; Jin, Y. Current correlations of an on-demand single-electron emitter. Phys. Rev. B 2010, 82, 201309. [Google Scholar] [CrossRef]
- Parmentier, F.D.; Bocquillon, E.; Berroir, J.M.; Glattli, D.C.; Plaçais, B.; Fève, G.; Albert, M.; Flindt, C.; Büttiker, M. Current noise spectrum of a single-particle emitter: Theory and experiment. Phys. Rev. B 2012, 85, 165438. [Google Scholar] [CrossRef]
- Maire, N.; Hohls, F.; Kaestner, B.; Pierz, K.; Schumacher, H.W.; Haug, R.J. Noise measurement of a quantized charge pump. Appl. Phys. Lett. 2008, 92, 082112. [Google Scholar] [CrossRef]
- Gabelli, J.; Thibault, K.; Gasse, G.; Lupien, C.; Reulet, B. Characterization and control of charge transfer in a tunnel junction. Phys. Status Solidi 2017, 254, 1600619. [Google Scholar] [CrossRef]
- Landauer, R. The noise is the signal. Nature 1998, 392, 658–659. [Google Scholar] [CrossRef]
- Reznikov, M.; de Picciotto, R.; Heiblum, M.; Glattli, D.C.; Kumar, A.; Saminadayar, L. Quantum shot noise. Superlattices Microstruct. 1998, 23, 901–915. [Google Scholar] [CrossRef][Green Version]
- Blanter, Y.; Büttiker, M. Shot noise in mesoscopic conductors. Phys. Rep. 2000, 336, 1–166. [Google Scholar] [CrossRef]
- Fletcher, J.D.; Johnson, N.; Locane, E.; See, P.; Griffiths, J.P.; Farrer, I.; Ritchie, D.A.; Brouwer, P.W.; Kashcheyevs, V.; Kataoka, M. Continuous-variable tomography of solitary electrons. Nat. Commun. 2019, 10, 1355. [Google Scholar] [CrossRef] [PubMed]
- Albert, M.; Flindt, C.; Büttiker, M. Accuracy of the quantum capacitor as a single-electron source. Phys. Rev. B 2010, 82, 041407. [Google Scholar] [CrossRef]
- Grenier, C.; Hervé, R.; Fève, G.; Degiovanni, P. Electron quantum optics in quantum hall edge channels. Mod. Phys. Lett. B 2011, 25, 1053–1073. [Google Scholar] [CrossRef]
- Grenier, C.; Hervé, R.; Bocquillon, E.; Parmentier, F.D.; Plaçais, B.; Berroir, J.M.; Fève, G.; Degiovanni, P. Single-electron quantum tomography in quantum Hall edge channels. New J. Phys. 2011, 13, 093007. [Google Scholar] [CrossRef]
- Dubois, J.; Jullien, T.; Grenier, C.; Degiovanni, P.; Roulleau, P.; Glattli, D.C. Integer and fractional charge Lorentzian voltage pulses analyzed in the framework of photon-assisted shot noise. Phys. Rev. B 2013, 88, 085301. [Google Scholar] [CrossRef]
- Grenier, C.; Dubois, J.; Jullien, T.; Roulleau, P.; Glattli, D.C.; Degiovanni, P. Fractionalization of minimal excitations in integer quantum Hall edge channels. Phys. Rev. B 2013, 88, 085302. [Google Scholar] [CrossRef]
- Ferraro, D.; Feller, A.; Ghibaudo, A.; Thibierge, E.; Bocquillon, E.; Fève, G.; Grenier, C.; Degiovanni, P. Wigner function approach to single electron coherence in quantum Hall edge channels. Phys. Rev. B 2013, 88, 205303. [Google Scholar] [CrossRef]
- Thibierge, E.; Ferraro, D.; Roussel, B.; Cabart, C.; Marguerite, A.; Fève, G.; Degiovanni, P. Two-electron coherence and its measurement in electron quantum optics. Phys. Rev. B 2016, 93, 081302. [Google Scholar] [CrossRef]
- Gaury, B.; Waintal, X. A computational approach to quantum noise in time-dependent nanoelectronic devices. Phys. E Low-Dimens. Syst. Nanostructures 2016, 75, 72–76. [Google Scholar] [CrossRef]
- Rech, J.; Ferraro, D.; Jonckheere, T.; Vannucci, L.; Sassetti, M.; Martin, T. Minimal Excitations in the Fractional Quantum Hall Regime. Phys. Rev. Lett. 2017, 118, 076801. [Google Scholar] [CrossRef] [PubMed]
- Roussel, B.; Cabart, C.; Fève, G.; Thibierge, E.; Degiovanni, P. Electron quantum optics as quantum signal processing. Phys. Status Solidi 2017, 254, 1600621. [Google Scholar] [CrossRef]
- Ferraro, D.; Ronetti, F.; Vannucci, L.; Acciai, M.; Rech, J.; Jockheere, T.; Martin, T.; Sassetti, M. Hong-Ou-Mandel characterization of multiply charged Levitons. Eur. Phys. J. Spec. Top. 2018, 227, 1345–1359. [Google Scholar] [CrossRef]
- Misiorny, M.; Fève, G.; Splettstoesser, J. Shaping charge excitations in chiral edge states with a time-dependent gate voltage. Phys. Rev. B 2018, 97, 075426. [Google Scholar] [CrossRef]
- Glattli, D.C.; Roulleau, P. Pseudorandom binary injection of levitons for electron quantum optics. Phys. Rev. B 2018, 97, 125407. [Google Scholar] [CrossRef]
- Dittmann, N.; Splettstoesser, J. Finite-frequency noise of interacting single-electron emitters: Spectroscopy with higher noise harmonics. Phys. Rev. B 2018, 98, 115414. [Google Scholar] [CrossRef]
- Rebora, G.; Acciai, M.; Ferraro, D.; Sassetti, M. Collisional interferometry of levitons in quantum Hall edge channels at ν=2. Phys. Rev. B 2020, 101, 245310. [Google Scholar] [CrossRef]
- Yue, X.K.; Yin, Y. Quasiparticles states for integer- and fractional-charged electron wave packets. arXiv 2020, arXiv:2004.00743. [Google Scholar]
- Roussel, B.; Cabart, C.; Fève, G.; Degiovanni, P. Processing quantum signals carried by electrical currents. arXiv 2020, arXiv:2008.01580v1. [Google Scholar]
- Bäuerle, C.; Glattli, D.C.; Meunier, T.; Portier, F.; Roche, P.; Roulleau, P.; Takada, S.; Waintal, X. Coherent control of single electrons: A review of current progress. Rep. Prog. Phys. 2018, 81, 056503. [Google Scholar] [CrossRef] [PubMed]
- Haack, G.; Moskalets, M.; Splettstoesser, J.; Büttiker, M. Coherence of single-electron sources from Mach-Zehnder interferometry. Phys. Rev. B 2011, 84, 081303. [Google Scholar] [CrossRef]
- Haack, G.; Moskalets, M.; Büttiker, M. Glauber coherence of single-electron sources. Phys. Rev. B 2013, 87, 201302. [Google Scholar] [CrossRef]
- Levitov, L.S.; Lee, H.; Lesovik, G.B. Electron counting statistics and coherent states of electric current. J. Math. Phys. 1996, 37, 4845–4866. [Google Scholar] [CrossRef]
- Ivanov, D.A.; Lee, H.W.; Levitov, L.S. Coherent states of alternating current. Phys. Rev. B 1997, 56, 6839–6850. [Google Scholar] [CrossRef]
- Flindt, C. Quantum physics: Single electrons pop out of the Fermi sea. Nature 2013, 502, 630–632. [Google Scholar] [CrossRef] [PubMed]
- Keeling, J.; Klich, I.; Levitov, L.S. Minimal Excitation States of Electrons in One-Dimensional Wires. Phys. Rev. Lett. 2006, 97, 116403. [Google Scholar] [CrossRef] [PubMed]
- Büttiker, M.; Thomas, H.; Prêtre, A. Mesoscopic capacitors. Phys. Lett. A 1993, 180, 364–369. [Google Scholar] [CrossRef]
- Gabelli, J.; Fève, G.; Berroir, J.M.; Plaçais, B.; Cavanna, A.; Etienne, B.; Jin, Y.; Glattli, D.C. Violation of Kirchhoff’s laws for a coherent RC circuit. Science 2006, 313, 499–502. [Google Scholar] [CrossRef] [PubMed]
- Fève, G.; Mahé, A.; Berroir, J.M.; Kontos, T.; Plaçais, B.; Glattli, D.C.; Cavanna, A.; Etienne, B.; Jin, Y. An on-demand coherent single-electron source. Science 2007, 316, 1169–1172. [Google Scholar] [CrossRef] [PubMed]
- Keeling, J.; Shytov, A.V.; Levitov, L.S. Coherent Particle Transfer in an On-Demand Single-Electron Source. Phys. Rev. Lett. 2008, 101, 196404. [Google Scholar] [CrossRef] [PubMed]
- Moskalets, M.; Haack, G. Heat and charge transport measurements to access single-electron quantum characteristics. Phys. Status Solidi 2016, 254, 1600616. [Google Scholar] [CrossRef]
- Filippone, M.; Marguerite, A.; Le Hur, K.; Fève, G.; Mora, C. Phase-Coherent Dynamics of Quantum Devices with Local Interactions. Entropy 2020, 22, 847. [Google Scholar] [CrossRef]
- Moskalets, M.; Haack, G.; Büttiker, M. Single-electron source: Adiabatic versus nonadiabatic emission. Phys. Rev. B 2013, 87, 125429. [Google Scholar] [CrossRef]
- Beenakker, C.W.J. Search for Majorana Fermions in Superconductors. Annu. Rev. Condens. Matter Phys. 2013, 4, 113–136. [Google Scholar] [CrossRef]
- Aguado, R. Majorana quasiparticles in condensed matter. La Rivista del Nuovo Cimento 2017, 40, 523–593. [Google Scholar] [CrossRef]
- Hassler, F.; Grabsch, A.; Pacholski, M.J.; Oriekhov, D.O.; Ovdat, O.; Adagideli, I.; Beenakker, C.W.J. Half-integer charge injection by a Josephson junction without excess noise. Phys. Rev. B 2020, 102, 045431. [Google Scholar] [CrossRef]
- Moskalets, M. Fractionally Charged Zero-Energy Single-Particle Excitations in a Driven Fermi Sea. Phys. Rev. Lett. 2016, 117, 046801. [Google Scholar] [CrossRef] [PubMed]
- Moskalets, M.; Haack, G. Single-electron coherence: Finite temperature versus pure dephasing. Phys. E Low-Dimens. Syst. Nanostructures 2016, 75, 358–369. [Google Scholar] [CrossRef][Green Version]
- Moskalets, M.; Kotilahti, J.; Burset, P.; Flindt, C. Composite two-particle sources. Eur. Phys. J. Spec. Top. 2020, 229, 647–662. [Google Scholar] [CrossRef]
- Büttiker, M. Edge-State Physics Without Magnetic Fields. Science 2009, 325, 278–279. [Google Scholar] [CrossRef]
- Büttiker, M. Scattering theory of thermal and excess noise in open conductors. Phys. Rev. Lett. 1990, 65, 2901–2904. [Google Scholar] [CrossRef] [PubMed]
- Büttiker, M. Scattering theory of current and intensity noise correlations in conductors and wave guides. Phys. Rev. B 1992, 46, 12485–12507. [Google Scholar] [CrossRef] [PubMed]
- Moskalets, M.; Büttiker, M. Floquet scattering theory of quantum pumps. Phys. Rev. B 2002, 66, 205320. [Google Scholar] [CrossRef]
- Moskalets, M.; Büttiker, M. Time-resolved noise of adiabatic quantum pumps. Phys. Rev. B 2007, 75, 035315. [Google Scholar] [CrossRef]
- Moskalets, M.; Büttiker, M. Spectroscopy of electron flows with single- and two-particle emitters. Phys. Rev. B 2011, 83, 035316. [Google Scholar] [CrossRef]
- Burset, P.; Kotilahti, J.; Moskalets, M.; Flindt, C. Time-Domain Spectroscopy of Mesoscopic Conductors Using Voltage Pulses (Adv. Quantum Technol. 3-4/2019). Adv. Quantum Technol. 2019, 2, 1970023. [Google Scholar] [CrossRef]
- Moskalets, M. Single-particle emission at finite temperatures. Low Temp. Phys. 2017, 43, 865–876. [Google Scholar] [CrossRef][Green Version]
- Moskalets, M. Single-particle shot noise at nonzero temperature. Phys. Rev. B 2017, 96, 165423. [Google Scholar] [CrossRef]
- Bocquillon, E.; Freulon, V.; Parmentier, F.D.; Berroir, J.M.; Plaçais, B.; Wahl, C.; Rech, J.; Jonckheere, T.; Martin, T.; Grenier, C.; et al. Electron quantum optics in ballistic chiral conductors. Ann. Der Phys. 2014, 526, 1–30. [Google Scholar] [CrossRef]
- Glattli, D.C.; Roulleau, P. Hanbury-Brown Twiss noise correlation with time controlled quasi-particles in ballistic quantum conductors. Phys. E Low-Dimens. Syst. Nanostructures 2016, 76, 216–222. [Google Scholar] [CrossRef]
- Moskalets, M. Noise of a single-electron emitter. Phys. Rev. B 2013, 88, 035433. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moskalets, M. Auto- versus Cross-Correlation Noise in Periodically Driven Quantum Coherent Conductors. Entropy 2021, 23, 393. https://doi.org/10.3390/e23040393
Moskalets M. Auto- versus Cross-Correlation Noise in Periodically Driven Quantum Coherent Conductors. Entropy. 2021; 23(4):393. https://doi.org/10.3390/e23040393
Chicago/Turabian StyleMoskalets, Michael. 2021. "Auto- versus Cross-Correlation Noise in Periodically Driven Quantum Coherent Conductors" Entropy 23, no. 4: 393. https://doi.org/10.3390/e23040393
APA StyleMoskalets, M. (2021). Auto- versus Cross-Correlation Noise in Periodically Driven Quantum Coherent Conductors. Entropy, 23(4), 393. https://doi.org/10.3390/e23040393





