Analysis of the Properties of Fractional Heat Conduction in Porous Electrodes of Lithium-Ion Batteries
Abstract
1. Introduction
2. Fractional Heat Conduction Model
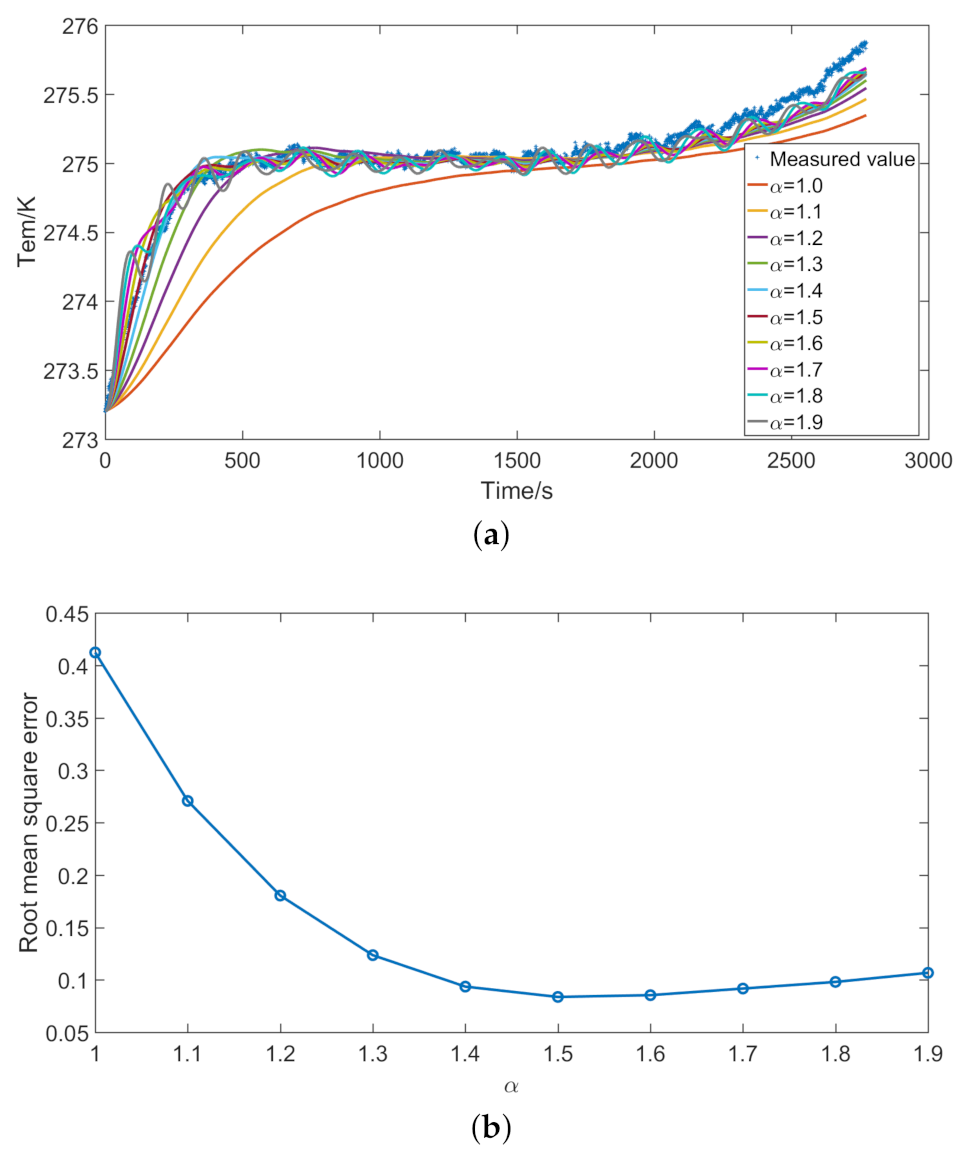
2.1. Numerical Solution of the Fractional Heat Conduction Equation
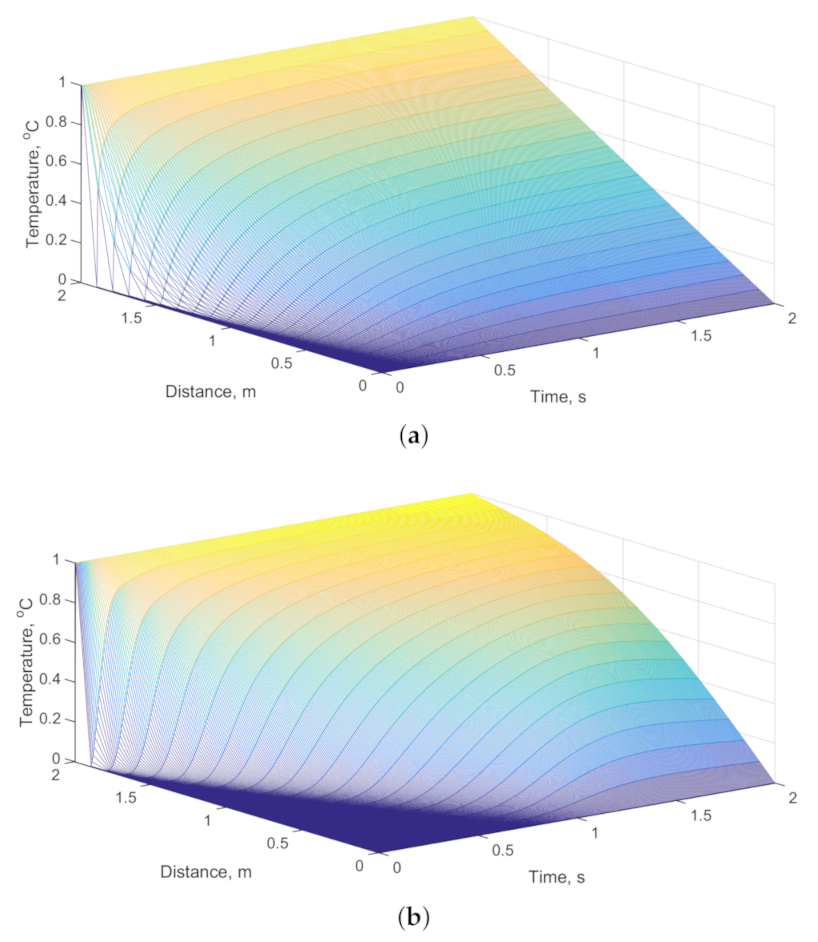
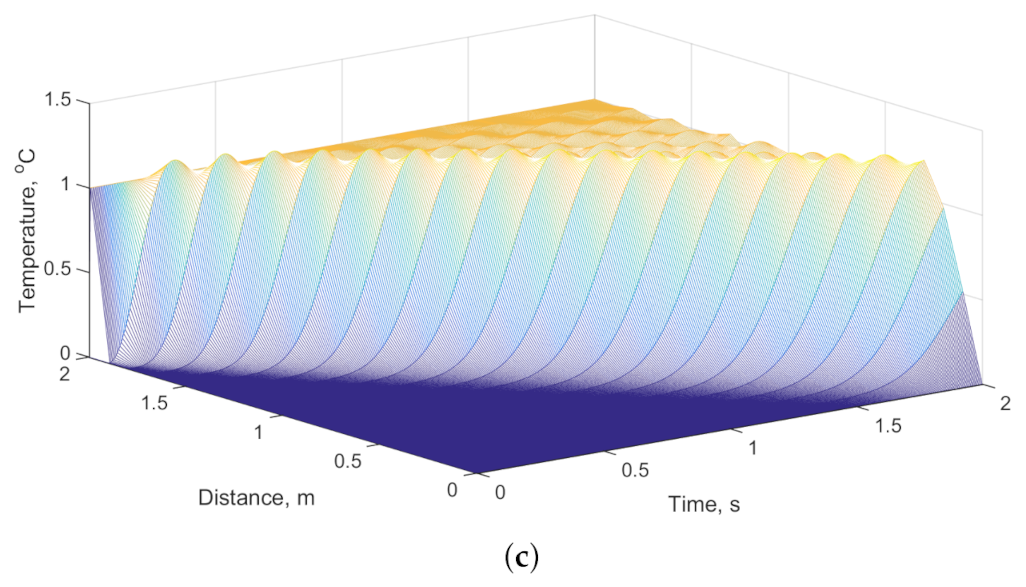
2.2. Characteristics of the Fractional Heat Conduction Model
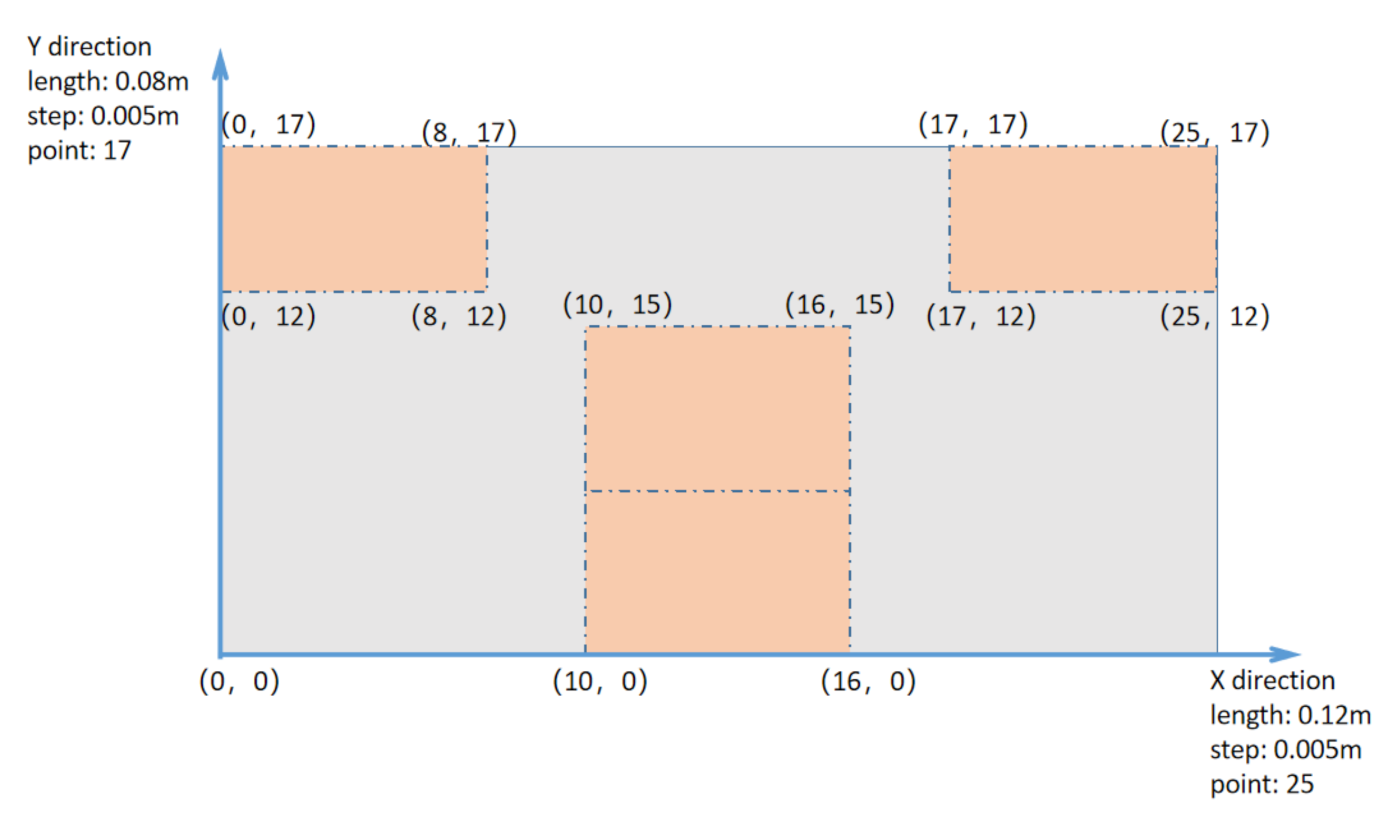
3. Heat Conduction Model and Temperature Test of the Battery
3.1. Fractional Heat Conduction Model of the Lithium-Ion Battery
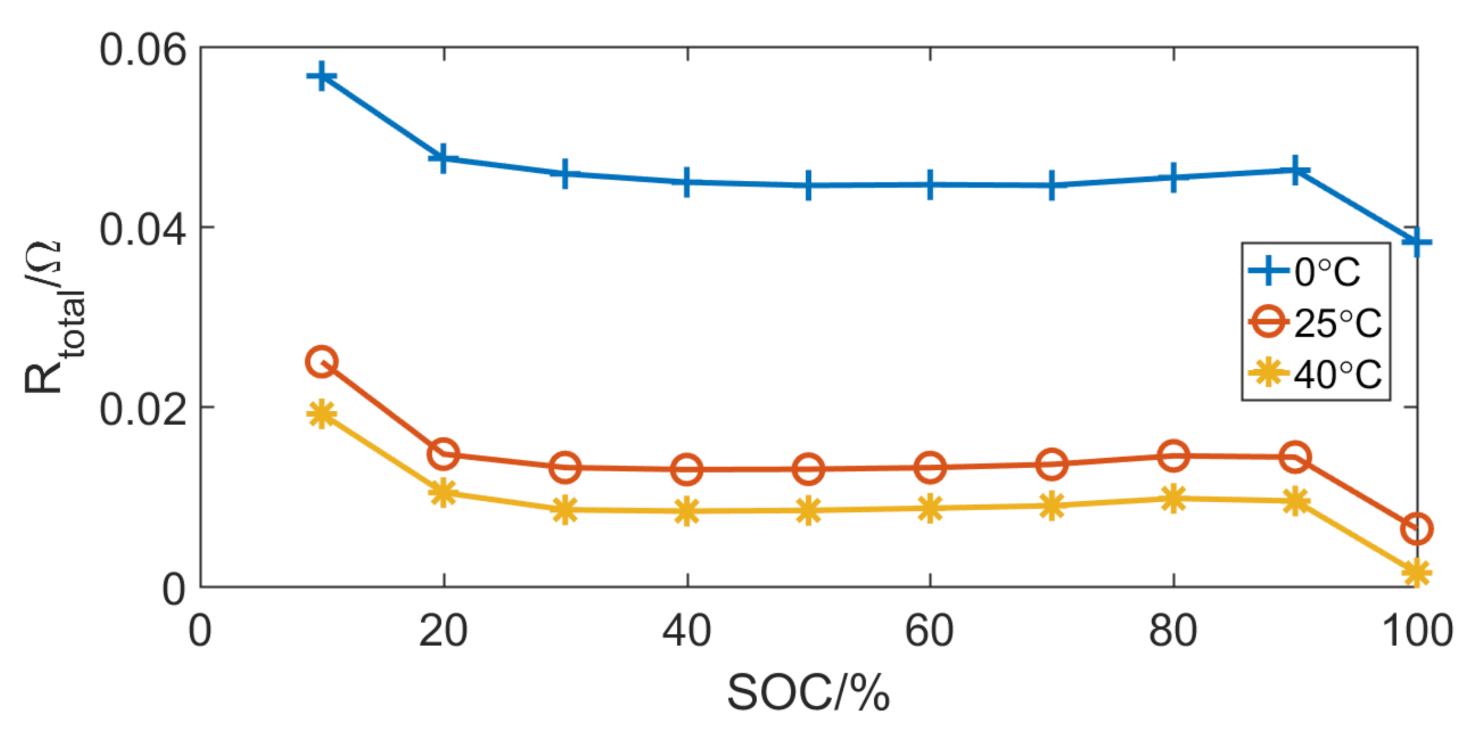
3.2. Thermal Properties Parameters of the Lithium-Ion Battery
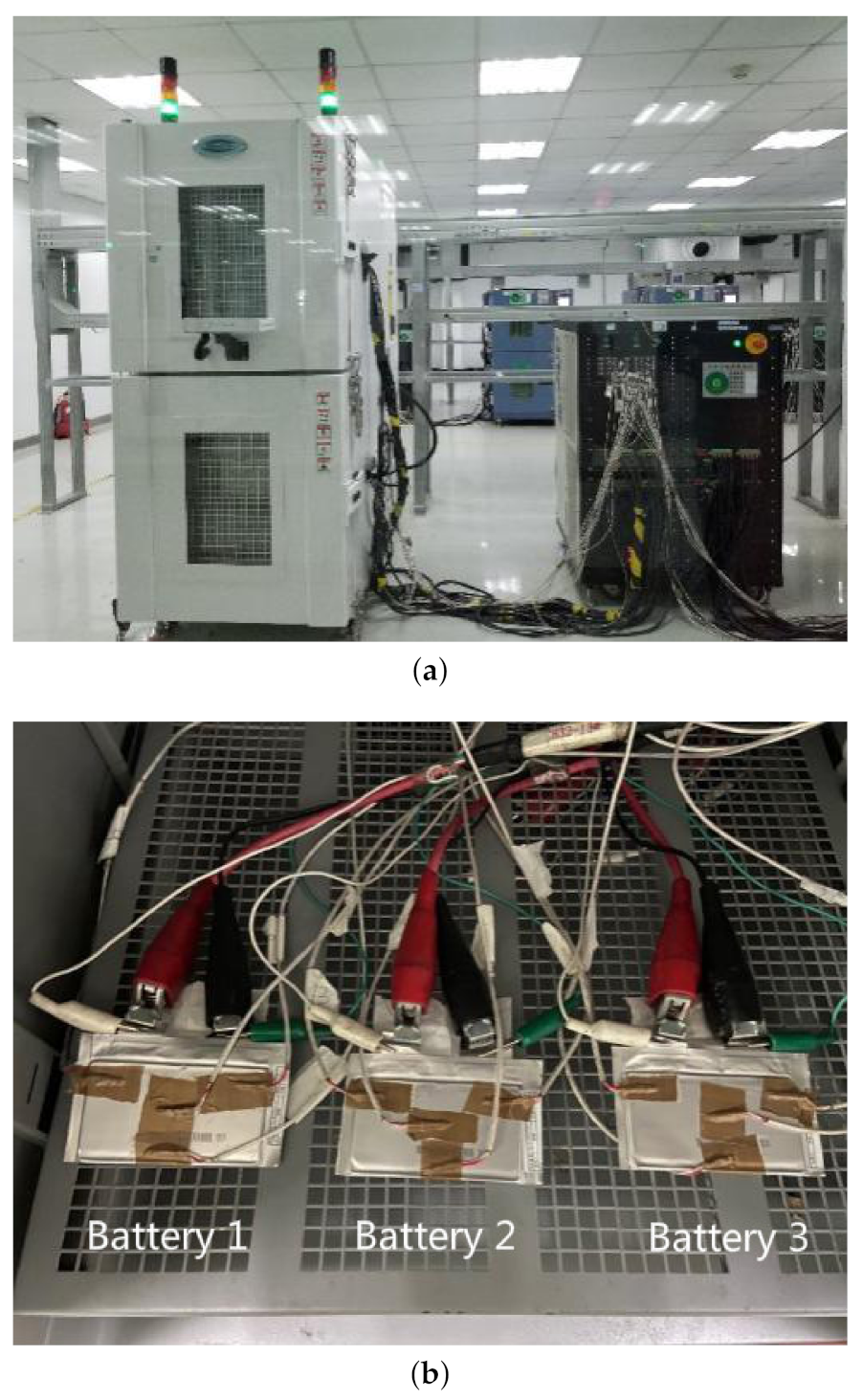
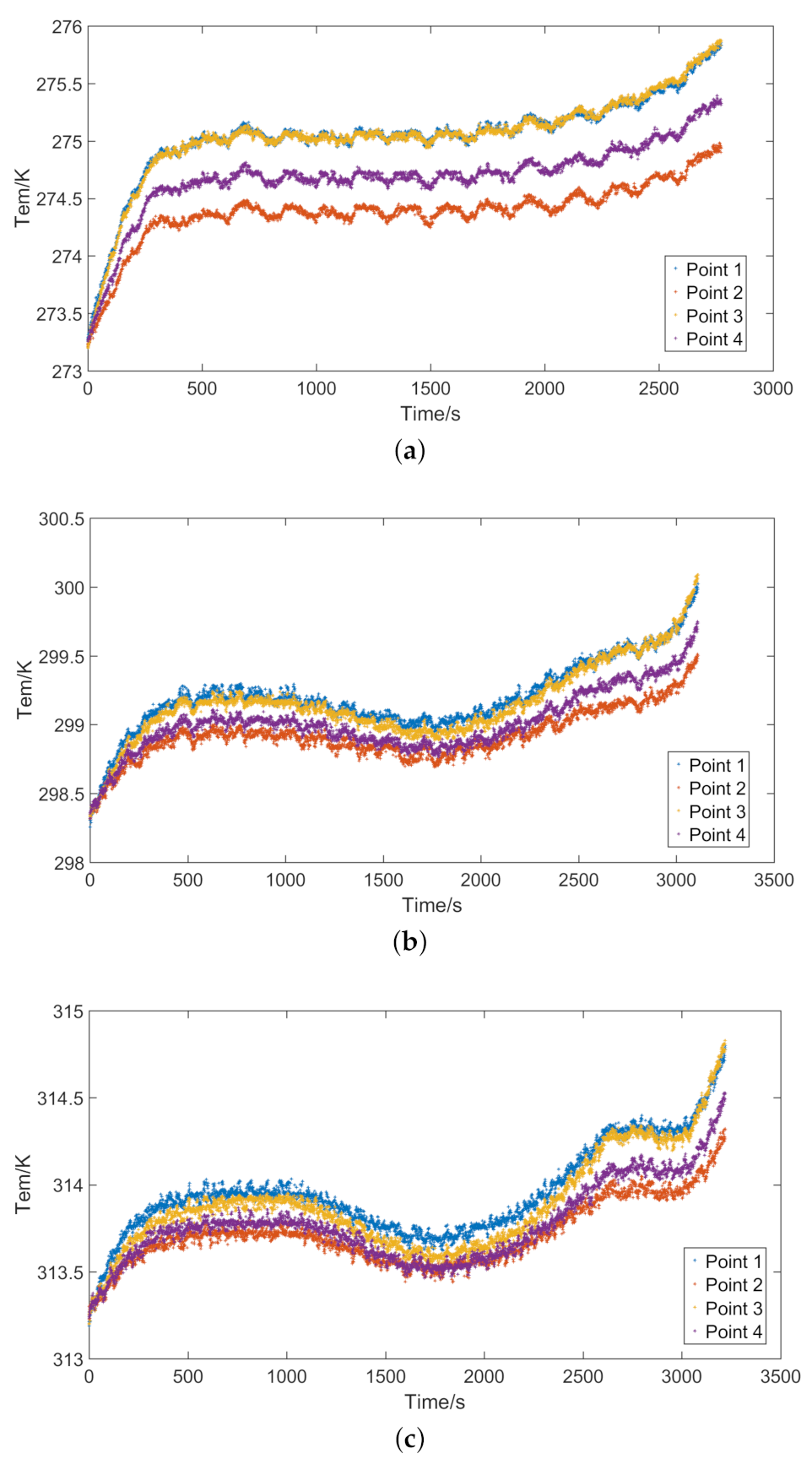
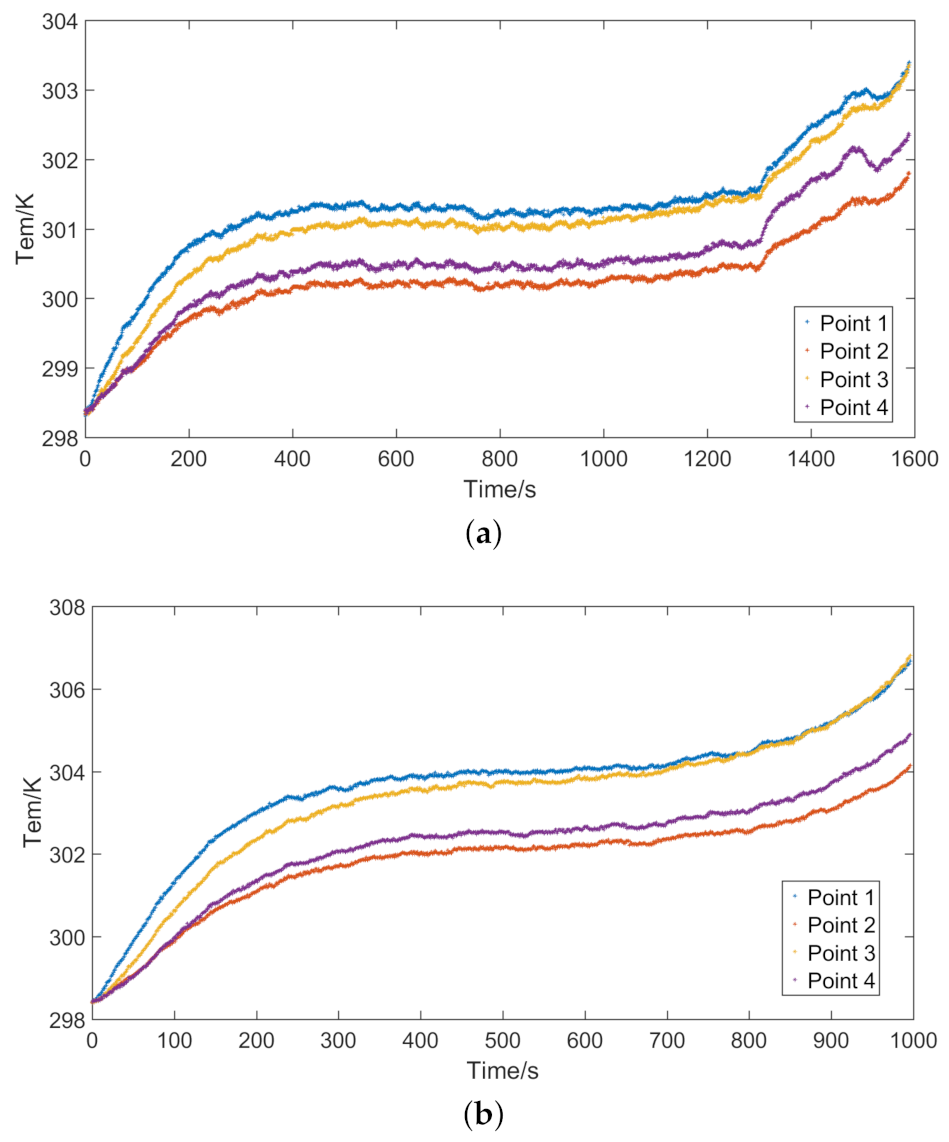
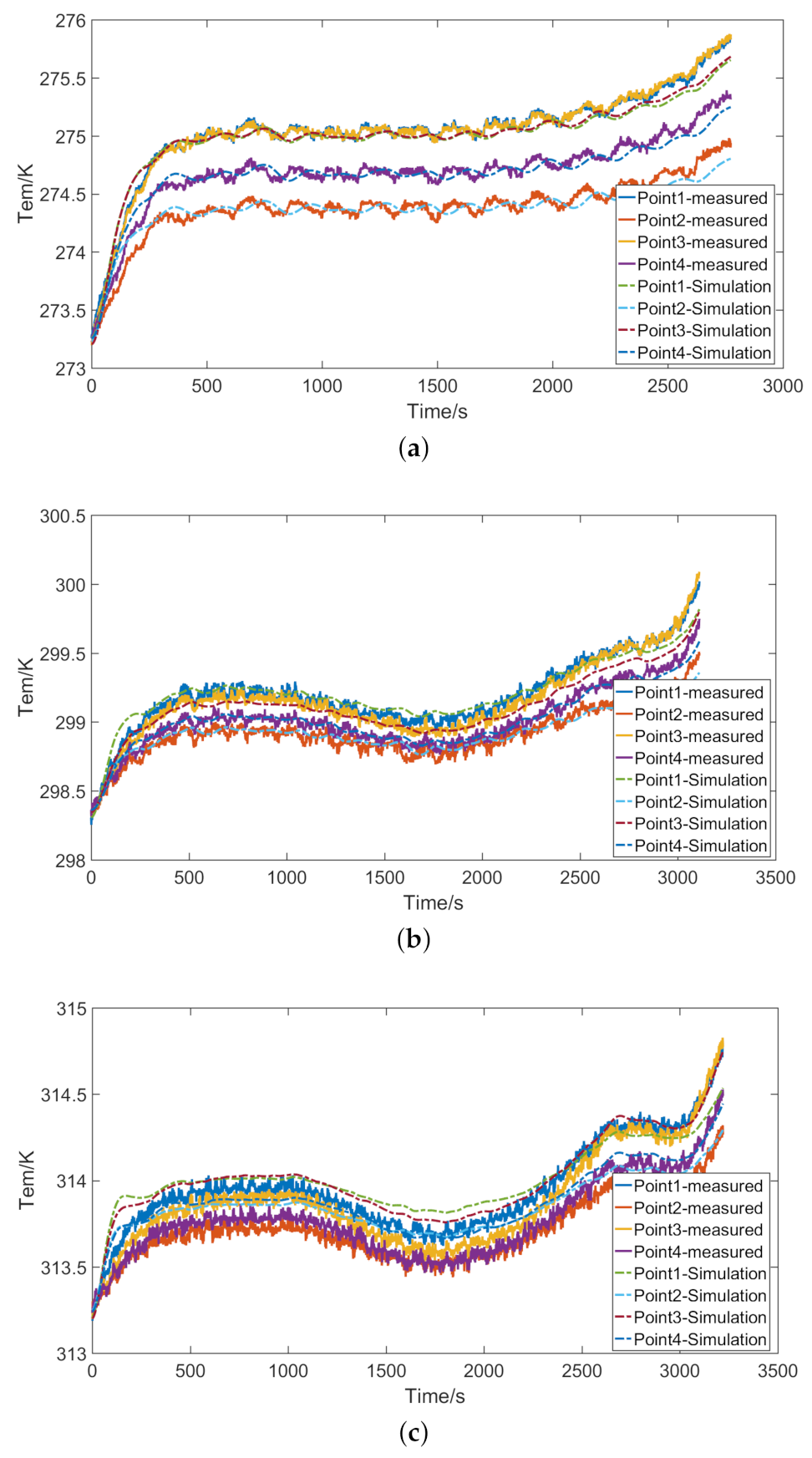
3.3. Temperature Characteristic Test of the Lithium-Ion Battery
- The thermostat was set to a constant temperature (T). The battery was charged to the upper voltage limit by a constant current (1 C rate). Then, the battery was continuously charged until the current was less than 0.05 C (constant current and constant voltage mode). Finally, the load current of the battery was cut off and the battery was allowed to stand for a certain period of time (1 h).
- The battery was discharged to the cut-off voltage by a current of 1 C.
- The thermostat was set to different ambient temperatures (T = 0, 25, and 40 C). The battery was preheated in the incubator for 5 h. Then, the above steps were repeated.
- The thermostat was set to a constant temperature (25 C). The battery was charged to the upper voltage limit by a constant current (0.5 C rate). Then, the battery was continuously charged until the current is less than 0.05 C (constant current and constant voltage mode). Finally, the load current of the battery was cut off and the battery was allowed to stand for a certain period of time(1 h).
- The battery was discharged to the cut-off voltage by a current of 2 C.
- The discharge rate of the battery is set to 3 C. Then, the above steps were repeated.
- The thermostat was set to a constant temperature (T). The battery was charged to the upper voltage limit by a constant current (1 C rate). Then, the battery was continuously charged until the current is less than 0.05 C (constant current and constant voltage mode). Finally, the load current of the battery was cut off and the battery was allowed to stand for a certain period of time (1 h).
- The battery was discharged by 1C rate current until the state of charge drops by 10%. The battery was then allowed to stand for 1 h.
- The battery was discharged by 1C rate current for 10 s, and then the battery was allowed to stand for 40 s. The battery was charged by 0.75 C current for 10 s and then allowed to stand for 1 h.
- Steps 2 and 3 were repeated until the voltage reached the cut-off voltage of the battery.
- The thermostat was set to different ambient temperatures (T = 0, 25, and 40 C). The battery as preheated in the incubator for 5 h. Then, the above steps were repeated.
4. Simulation of the Temperature Field of Lithium-Ion Batteries
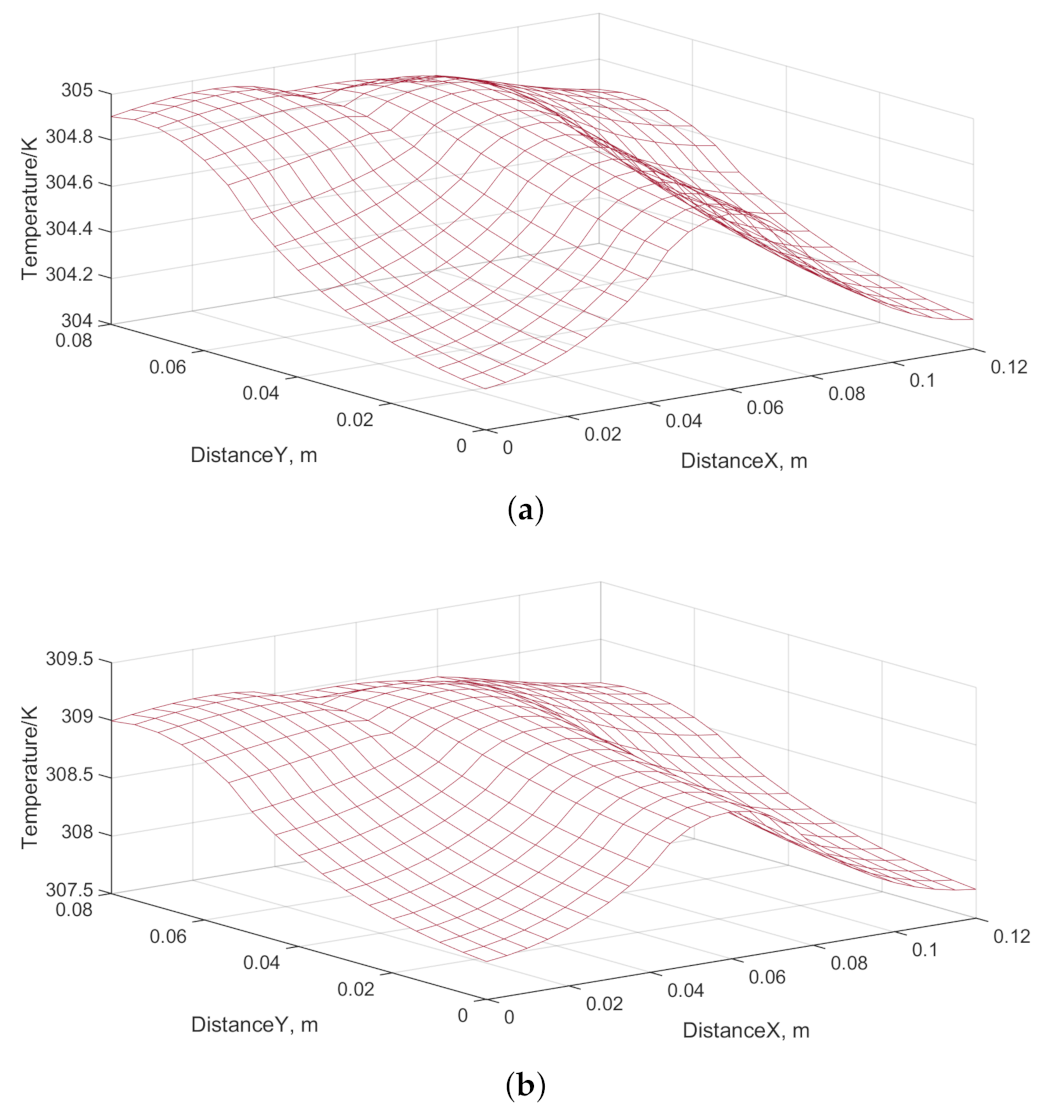
4.1. Temperature Simulation
4.2. Transient Temperature Field of the Battery
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kawahara, Y.; Sakabe, K.; Nakao, R.; Tsuru, K.; Okawa, K.; Aoshima, Y.; Kudo, A.; Emori, A. Development of status detection method of lithium-ion rechargeable battery for hybrid electric vehicles. J. Power Sources 2021, 481, 228760. [Google Scholar] [CrossRef]
- Malik, M.S.S.; Li, G.; Chen, Z. An optimal charging algorithm to minimise solid electrolyte interface layer in lithium-ion battery. J. Power Sources 2021, 482, 228895. [Google Scholar] [CrossRef]
- Ng, K.S.; Moo, C.S.; Chen, Y.P.; Hsieh, Y.C. Enhanced coulomb counting method for estimating state-of-charge and state-of-health of lithium-ion batteries. Appl. Energy 2009, 86, 1506–1511. [Google Scholar] [CrossRef]
- Kang, L.W.; Zhao, X.; Ma, J. A new neural network model for the state-of-charge estimation in the battery degradation process. Appl. Energy 2014, 121, 20–27. [Google Scholar] [CrossRef]
- Yang, F.; Xing, Y.; Wang, D.; Tsui, K.L. A comparative study of three model-based algorithms for estimating state-of-charge of lithium-ion batteries under a new combined dynamic loading profile. Appl. Energy 2016, 164, 387–399. [Google Scholar] [CrossRef]
- Lee, D.C.; Kim, C.W. Two-way nonlinear mechanical-electrochemical-thermal coupled analysis method to predict thermal runaway of lithium-ion battery cells caused by quasi-static indentation. J. Power Sources 2020, 475, 228678. [Google Scholar] [CrossRef]
- Peng, W.; Ayala, J.G.; Su, S.; Chen, J.; Hernández, A.C. A two-stage sodium thermal electrochemical converter: Parametric optimization and performance enhancement. J. Power Sources 2020, 480, 229147. [Google Scholar] [CrossRef]
- Basu, S.; Hariharan, K.S.; Kolake, S.M.; Song, T.; Sohn, D.K.; Yeo, T. Coupled electrochemical thermal modelling of a novel Li-ion battery pack thermal management system. Appl. Energy 2016, 181, 1–13. [Google Scholar] [CrossRef]
- Pan, D.; Guo, H.; Tang, S.; Li, X.; Wang, Z.; Peng, W.; Wang, J.; Yan, G. Evaluating the accuracy of electro-thermal coupling model in lithium-ion battery via altering internal resistance acquisition methods. J. Power Sources 2020, 463, 228174. [Google Scholar] [CrossRef]
- Huang, J.; Li, Z.; Liaw, B.Y.; Zhang, J. Graphical analysis of electrochemical impedance spectroscopy data in Bode and Nyquist representations. J. Power Sources 2016, 309, 82–98. [Google Scholar] [CrossRef]
- Pan, Y.W.; Hua, Y.; Zhou, S.; He, R.; Zhang, Y.; Yang, S.; Liu, X.; Lian, Y.; Yan, X.; Wu, B. A computational multi-node electro-thermal model for large prismatic lithium-ion batteries. J. Power Sources 2020, 459, 228070. [Google Scholar] [CrossRef]
- Ye, Y.; Shi, Y.; Saw, L.H.; Tay, A.A. An electro-thermal model and its application on a spiral-wound lithium ion battery with porous current collectors. Electrochim. Acta 2014, 121, 143–153. [Google Scholar] [CrossRef]
- Zhu, J.; Knapp, M.; Darma, M.S.; Fang, Q.; Wang, X.; Dai, H.; Wei, X.; Ehrenberg, H. An improved electro-thermal battery model complemented by current dependent parameters for vehicular low temperature application. Appl. Energy 2019, 248, 149–161. [Google Scholar] [CrossRef]
- Liu, C.; Liu, W.; Wang, L.; Hu, G.; Ma, L.; Ren, B. A new method of modeling and state of charge estimation of the battery. J. Power Sources 2016, 320, 1–12. [Google Scholar] [CrossRef]
- Xu, J.; Mi, C.C.; Cao, B.; Cao, J. A new method to estimate the state of charge of lithium-ion batteries based on the battery impedance model. J. Power Sources 2013, 233, 277–284. [Google Scholar] [CrossRef]
- Zhou, D.; Zhang, K.; Ravey, A.; Gao, F.; Miraoui, A. Parameter sensitivity analysis for fractional-order modeling of lithium-ion batteries. Energies 2016, 9, 123. [Google Scholar] [CrossRef]
- Oustaloup, A.; Sabatier, J.; Lanusse, P. From fractal robustness to the CRONE control. Fract. Calc. Appl. Anal. 2007, 2, 1–30. [Google Scholar]
- Zou, Y.; Li, S.E.; Shao, B.; Wang, B. State-space model with non-integer order derivatives for lithium-ion battery. Appl. Energy 2016, 161, 330–336. [Google Scholar] [CrossRef]
- Mastali, M.; Samadani, E.; Farhad, S.; Fraser, R.; Fowler, M. Three-dimensional multi-particle electrochemical model of LiFePO4 Cells based on a resistor network methodology. Electrochim. Acta 2016, 190, 574–587. [Google Scholar] [CrossRef]
- Wang, B.; Li, S.E.; Peng, H.; Liu, Z. Fractional-order modeling and parameter identification for lithium-ion batteries. J. Power Sources 2015, 293, 151–161. [Google Scholar] [CrossRef]
- He, H.; Xiong, R.; Guo, H. Online estimation of model parameters and state-of-charge of LiFePO4 batteries in electric vehicles. Appl. Energy 2012, 89, 413–420. [Google Scholar] [CrossRef]
- Eichi, H.R.; Baronti, F.; Chow, M.Y. Online adaptive parameter identification and state-of-charge coestimation for lithium-polymer battery cells. IEEE Trans. Ind. Electron. 2014, 61, 2053–2061. [Google Scholar] [CrossRef]
- Li, X.; Song, K.; Wei, G.; Lu, R.; Zhu, C. A novel grouping method for lithium iron phosphate batteries based on a fractional joint Kalman filter and a new modified K-means clustering algorithm. Energies 2015, 8, 7703–7728. [Google Scholar] [CrossRef]
- Zhong, F.; Li, H.; Zhong, S.; Zhong, Q.; Yin, C. An SOC estimation approach based on adaptive sliding mode observer and fractional order equivalent circuit model for lithium-ion batteries. Commun. Nonlinear Sci. Numer. Simul. 2015, 24, 127–144. [Google Scholar] [CrossRef]
- Xiao, R.; Shen, J.; Li, X.; Yan, W.; Pan, E.; Chen, Z. Comparisons of modeling and state of charge estimation for lithium-ion battery based on fractional order and integral order methods. Energies 2016, 9, 184. [Google Scholar] [CrossRef]
- Riu, D.; Montaru, M.; Bultel, Y. Time domain simulation of Li-ion batteries using non-integer order equivalent electrical circuit. Nonlinear Sci. Numer. Simul. 2013, 18, 1454–1462. [Google Scholar] [CrossRef]
- Deng, Z.; Cao, H.; Li, X.; Jiang, J.; Yang, J.; Qin, Y. Generalized predictive control for fractional order dynamic model of solid oxide fuel cell output power. J. Power Sources 2010, 195, 8097–8103. [Google Scholar] [CrossRef]
- Wang, Y.; Yu, Y.; Jing, Z.; Wang, C.; Zhou, G.; Zhao, W. Thermal performance of lithium-ion batteries applying forced air cooling with an improved aluminium foam heat sink design. Int. J. Heat Mass Transf. 2021, 167, 120827. [Google Scholar] [CrossRef]
- Ma, Q.; Xu, Q.; Chen, Q.; Chen, Z.; Su, H.; Zhang, W. Lattice Boltzmann model for complex transfer behaviors in porous electrode of all copper redox flow battery with deep eutectic solvent electrolyte. Appl. Therm. Eng. 2019, 160, 114015. [Google Scholar] [CrossRef]
- Choudhari, V.G.; Dhoble, A.S.; Satyam, P. Numerical analysis of different fin structures in phase change material module for battery thermal management system and its optimization. Int. J. Heat Mass Transf. 2020, 163, 120434. [Google Scholar] [CrossRef]
- Lin, J.; Liu, X.; Li, S.; Zhang, C.; Yang, S. A review on recent progress, challenges and perspective of battery thermal management system. Int. J. Heat Mass Transf. 2021, 167, 120834. [Google Scholar] [CrossRef]
- Wang, Q.; Sun, Q.; Ping, P.; Zhao, X.; Sun, J.; Lin, Z. Heat transfer in the dynamic cycling of lithium–titanate batteries. Int. J. Heat Mass Transf. 2016, 93, 896–905. [Google Scholar] [CrossRef]
- Wang, H.; He, F.; Ma, L. Experimental and modeling study of controller-based thermal management of battery modules under dynamic loads. Int. J. Heat Mass Transf. 2016, 103, 154–164. [Google Scholar] [CrossRef]
- Li, J.; Zhu, Z.; Zhao, L.; Peng, H. Experimental investigation of the heat transfer and flow characteristics of microchannels with microribs. Int. J. Heat Mass Transf. 2019, 143, 118482. [Google Scholar] [CrossRef]
- Zhang, X.; Klein, R.; Subbaraman, A.; Chumakov, S.; Li, X.; Christensen, J.; Linder, C.; Kim, S.U. Evaluation of convective heat transfer coefficient and specific heat capacity of a lithium-ion battery using infrared camera and lumped capacitance method. J. Power Sources 2019, 412, 552–558. [Google Scholar] [CrossRef]
- Loges, A.; Herberger, S.; Seegert, P.; Wetzel, T. A study on specific heat capacities of Li-ion cell components and their influence on thermal management. J. Power Sources 2016, 336, 341–350. [Google Scholar] [CrossRef]
- Bryden, T.S.; Dimitrov, B.; Hilton, G.; León, C.P.; Bugryniec, P.; Brown, S.; Cumming, D.; Cruden, A. Methodology to determine the heat capacity of lithium-ion cells. J. Power Sources 2018, 395, 369–378. [Google Scholar] [CrossRef]
- Richter, F.; Kjelstrup, S.; Vie, P.J.S.; Burheim, O.S. Thermal conductivity and internal temperature profiles of Li-ion secondary batteries. J. Power Sources 2017, 359, 592–600. [Google Scholar] [CrossRef]
- Werner, D.; Loges, A.; Becker, D.J.; Wetzel, T. Thermal conductivity of Li-ion batteries and their electrode configurations—A novel combination of modelling and experimental approach. J. Power Sources 2017, 364, 72–83. [Google Scholar] [CrossRef]
- Jagannadham, K. Thermal conductivity and interface thermal conductance of thin films in Li ion batteries. J. Power Sources 2016, 327, 565–572. [Google Scholar] [CrossRef]
- Kleiner, J.; Komsiyska, L.; Elger, G.; Endisch, C. Thermal Modelling of a Prismatic Lithium-Ion Cell in a Battery Electric Vehicle Environment: Influences of the Experimental Validation Setup. Energies 2020, 13, 62. [Google Scholar] [CrossRef]








| Battery | Nominal Capacity (Ah) | Operating Voltage (V) | Length, Width and Height (mm) | Weight (g) | Volume (cm) |
|---|---|---|---|---|---|
| LiNiMnCoO | 5 | 2.8–4.2 | 125, 86, and 5.6 | 82 | 36.3 |
| Composition | Material | Specific Heat Capacity (J/(kg·K)) | Thermal Conductivity (W/m·K) | Density (kg/cm) | Thickness (um) |
|---|---|---|---|---|---|
| Shell | Aluminum plastic film | 1376.947 | 0.427 | 1636.0 | 145 |
| Separator | Polypropylene | 1978.16 | 0.3344 | 648.773 | 40 |
| Cathode | Ternary materials | 1067.6 | 2.7 | 2584.25 | 40 |
| Anode | Graphite | 1064.0 | 1671.24 | 3.3 | 35 |
| Electrode conductor (+) | Aluminum | 903.0 | 238.0 | 2702.0 | - |
| Electrode conductor (−) | Copper | 385.0 | 398.0 | 8933.0 | - |
| Equivalent Specific Heat Capacity (J/(kg·K)) | Equivalent Thermal Conductivity (W/m·K) | Equivalent Density (kg/cm) | Temperature Influence Coefficient (mV·/K) |
|---|---|---|---|
| 944.24 | 27.242 (x,y) − 3.583 (z) | 2.1339 | 0.279 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, X.; Li, H.; Chen, N. Analysis of the Properties of Fractional Heat Conduction in Porous Electrodes of Lithium-Ion Batteries. Entropy 2021, 23, 195. https://doi.org/10.3390/e23020195
Lu X, Li H, Chen N. Analysis of the Properties of Fractional Heat Conduction in Porous Electrodes of Lithium-Ion Batteries. Entropy. 2021; 23(2):195. https://doi.org/10.3390/e23020195
Chicago/Turabian StyleLu, Xin, Hui Li, and Ning Chen. 2021. "Analysis of the Properties of Fractional Heat Conduction in Porous Electrodes of Lithium-Ion Batteries" Entropy 23, no. 2: 195. https://doi.org/10.3390/e23020195
APA StyleLu, X., Li, H., & Chen, N. (2021). Analysis of the Properties of Fractional Heat Conduction in Porous Electrodes of Lithium-Ion Batteries. Entropy, 23(2), 195. https://doi.org/10.3390/e23020195






