Quantum Contextual Advantage Depending on Nonzero Prior Probabilities in State Discrimination of Mixed Qubit States
Abstract
:1. Introduction
2. Preliminaries
2.1. Quantum Theory and Discrimination between Two Mixed Qubit States
2.2. Operational Theory and Preparation-Noncontextual Ontological Model
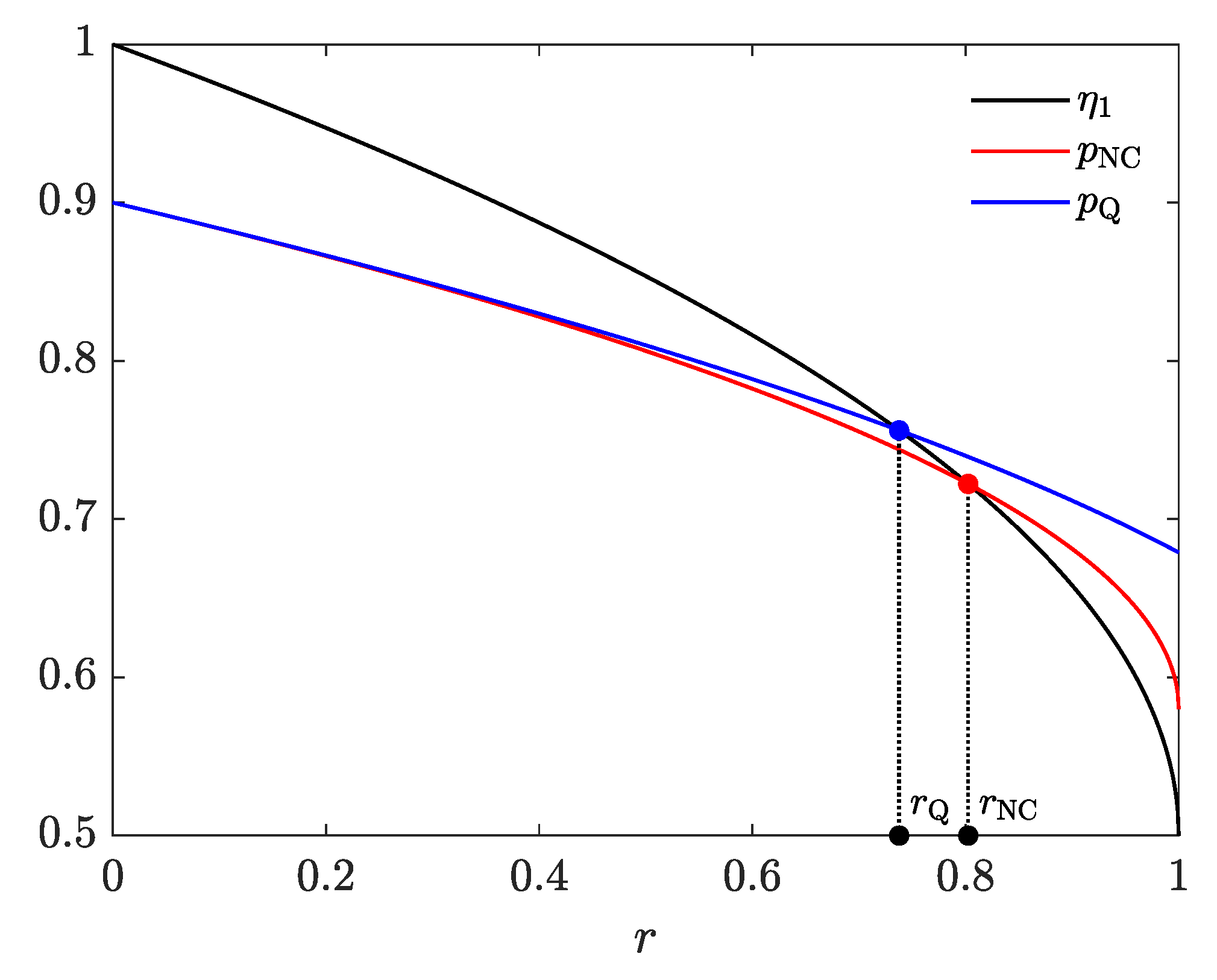
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Abbreviations
| MED | Minimum error discrimination |
References
- Kochen, S.; Specker, E.P. The Problem of Hidden Variables in Quantum Mechanics. J. Math. Mech. 1967, 17, 59–87. [Google Scholar] [CrossRef]
- Spekkens, R.W. Contextuality for preparations, transformations, and unsharp measurements. Phys. Rev. A 2005, 71, 052108. [Google Scholar] [CrossRef] [Green Version]
- Helstrom, C.W. Quantum detection and estimation theory. J. Stat. Phys. 1969, 1, 231–252. [Google Scholar] [CrossRef] [Green Version]
- Holevo, A.S. Probabilistic and Statistical Aspects of Quantum Theory; North-Holland: Amsterdam, The Netherlands, 1982. [Google Scholar]
- Bae, J. Structure of minimum-error quantum state discrimination. New J. Phys. 2013, 15, 073037. [Google Scholar] [CrossRef] [Green Version]
- Ha, D.; Kwon, Y. Complete analysis for three-qubit mixed-state discrimination. Phys. Rev. A 2013, 87, 062302. [Google Scholar] [CrossRef] [Green Version]
- Yuen, H.P.; Kennedy, R.S.; Lax, M. Optimum testing of multiple hypotheses in quantum detection theory. IEEE Trans. Inf. Theory 1975, 21, 125–134. [Google Scholar] [CrossRef]
- Barnett, S.M. Minimum-error discrimination between multiply symmetric states. Phys. Rev. A 2001, 64, 030303. [Google Scholar] [CrossRef]
- Chou, C.L.; Hsu, L.Y. Minimum-error discrimination between symmetric mixed quantum states. Phys. Rev. A 2003, 68, 042305. [Google Scholar] [CrossRef] [Green Version]
- Andersson, E.; Barnett, S.M.; Gilson, C.R.; Hunter, K. Minimum-error discrimination between three mirror-symmetric states. Phys. Rev. A 2002, 65, 052308. [Google Scholar] [CrossRef] [Green Version]
- Chou, C.L. Minimum-error discrimination among mirror-symmetric mixed quantum states. Phys. Rev. A 2004, 70, 062316. [Google Scholar] [CrossRef]
- Bae, J.; Hwang, W.-Y.; Han, Y.-D. No-Signaling Principle Can Determine Optimal Quantum State Discrimination. Phys. Rev. Lett. 2011, 107, 170403. [Google Scholar] [CrossRef] [Green Version]
- Bae, J.; Kim, D.-G.; Kwek, L.-C. Structure of Optimal State Discrimination in Generalized Probabilistic Theories. Entropy 2016, 18, 39. [Google Scholar] [CrossRef] [Green Version]
- Ha, D.; Kwon, Y. Discriminating N-qudit states using geometric structure. Phys. Rev. A 2014, 90, 022320. [Google Scholar] [CrossRef]
- Kim, J.; Ha, D.; Kwon, Y. Uniqueness of Minimax Strategy in View of Minimum Error Discrimination of Two Quantum States. Entropy 2019, 21, 671. [Google Scholar] [CrossRef] [Green Version]
- Ivanovic, I.D. How to differentiate between non-orthogonal states. Phys. Lett. A 1987, 123, 257–259. [Google Scholar] [CrossRef]
- Dieks, D. Overlap and distinguishability of quantum states. Phys. Lett. A 1988, 126, 303–306. [Google Scholar] [CrossRef]
- Peres, A. How to differentiate between non-orthogonal states. Phys. Lett. A 1988, 128, 19. [Google Scholar] [CrossRef]
- Jaeger, G.; Shimony, A. Optimal distinction between two non-orthogonal quantum states. Phys. Lett. A 1995, 197, 83–87. [Google Scholar] [CrossRef]
- Bergou, J.A. Optimal Unambiguous Discrimination of Pure Quantum States. Phys. Rev. Lett. 2012, 108, 250502. [Google Scholar] [CrossRef] [Green Version]
- Ha, D.; Kwon, Y. Analysis of optimal unambiguous discrimination of three pure quantum states. Phys. Rev. A 2015, 91, 062312. [Google Scholar] [CrossRef]
- Ha, D.; Kwon, Y. A minimal set of measurements for qudit-state tomography based on unambiguous discrimination. Quant. Inf. Process. 2018, 17, 232. [Google Scholar] [CrossRef]
- Namkung, M.; Kwon, Y. Understanding of Various Type of Unambiguous Discrimination in View of Coherence Distribution. Entropy 2020, 22, 1422. [Google Scholar] [CrossRef]
- Fiurášek, J.; Ježek, M. Optimal discrimination of mixed quantum states involving inconclusive results. Phys. Rev. A 2003, 67, 012321. [Google Scholar] [CrossRef] [Green Version]
- Herzog, U. Optimal state discrimination with a fixed rate of inconclusive results: Analytical solutions and relation to state discrimination with a fixed error rate. Phys. Rev. A 2012, 86, 032314. [Google Scholar] [CrossRef] [Green Version]
- Bagan, E.; Muñoz-Tapia, R.; Olivares-Rentería, G.A.; Bergou, J.A. Optimal discrimination of quantum states with a fixed rate of inconclusive outcomes. Phys. Rev. A 2012, 86, 040303. [Google Scholar] [CrossRef] [Green Version]
- Ha, D.; Kwon, Y. An optimal discrimination of two mixed qubit states with a fixed rate of inconclusive results. Quant. Inf. Process. 2017, 16, 273. [Google Scholar] [CrossRef] [Green Version]
- Bennett, C.H. Quantum cryptography using any two nonorthogonal states. Phys. Rev. Lett. 1992, 68, 3121. [Google Scholar] [CrossRef] [PubMed]
- Bergou, J.A.; Feldman, E.; Hillery, M. Extracting Information from a Qubit by Multiple Observers: Toward a Theory of Sequential State Discrimination. Phys. Rev. Lett. 2013, 111, 100501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Namkung, M.; Kwon, Y. Optimal sequential state discrimination between two mixed quantum states. Phys. Rev. A 2017, 96, 022318. [Google Scholar] [CrossRef]
- Namkung, M.; Kwon, Y. Sequential state discrimination of coherent states. Sci. Rep. 2018, 8, 16915. [Google Scholar] [CrossRef] [Green Version]
- Namkung, M.; Kwon, Y. Analysis of Optimal Sequential State Discrimination for Linearly Independent Pure Quantum States. Sci. Rep. 2018, 8, 6515. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Namkung, M.; Kwon, Y. Almost minimum error discrimination of N-ary weak coherent states by Jaynes-Cummings Hamiltonian dynamics. Sci. Rep. 2019, 9, 19664. [Google Scholar] [CrossRef] [Green Version]
- Namkung, M.; Kwon, Y. Generalized sequential state discrimination for multiparty QKD and its optical implementation. Sci. Rep. 2020, 10, 8247. [Google Scholar] [CrossRef] [PubMed]
- Schmid, D.; Spekkens, R.W. Contextual Advantage for State Discrimination. Phys. Rev. X 2018, 8, 011015. [Google Scholar] [CrossRef] [Green Version]
- Ha, D.; Kwon, Y. Quantum nonlocality without entanglement: Explicit dependence on prior probabilities of nonorthogonal mirror-symmetric states. NPJ Quant. Inf. 2021, 7, 81. [Google Scholar] [CrossRef]
- Ha, D.; Kim, J.S. Quantum nonlocality without entanglement depending on nonzero prior probabilities in optimal unambiguous discrimination. Sci. Rep. 2021, 11, 17695. [Google Scholar] [CrossRef]
- Hunter, K. Measurement does not always aid state discrimination. Phys. Rev. A 2003, 68, 012306. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shin, J.; Ha, D.; Kwon, Y. Quantum Contextual Advantage Depending on Nonzero Prior Probabilities in State Discrimination of Mixed Qubit States. Entropy 2021, 23, 1583. https://doi.org/10.3390/e23121583
Shin J, Ha D, Kwon Y. Quantum Contextual Advantage Depending on Nonzero Prior Probabilities in State Discrimination of Mixed Qubit States. Entropy. 2021; 23(12):1583. https://doi.org/10.3390/e23121583
Chicago/Turabian StyleShin, Jaehee, Donghoon Ha, and Younghun Kwon. 2021. "Quantum Contextual Advantage Depending on Nonzero Prior Probabilities in State Discrimination of Mixed Qubit States" Entropy 23, no. 12: 1583. https://doi.org/10.3390/e23121583
APA StyleShin, J., Ha, D., & Kwon, Y. (2021). Quantum Contextual Advantage Depending on Nonzero Prior Probabilities in State Discrimination of Mixed Qubit States. Entropy, 23(12), 1583. https://doi.org/10.3390/e23121583





