Energy and Magnetic Moment of a Quantum Charged Particle in Time-Dependent Magnetic and Electric Fields of Circular and Plane Solenoids
Abstract
1. Introduction
2. Basic Definitions and Equations
2.1. Main Physical Quantities
2.2. Equations Describing the Time Evolution
3. The Circular Gauge: General
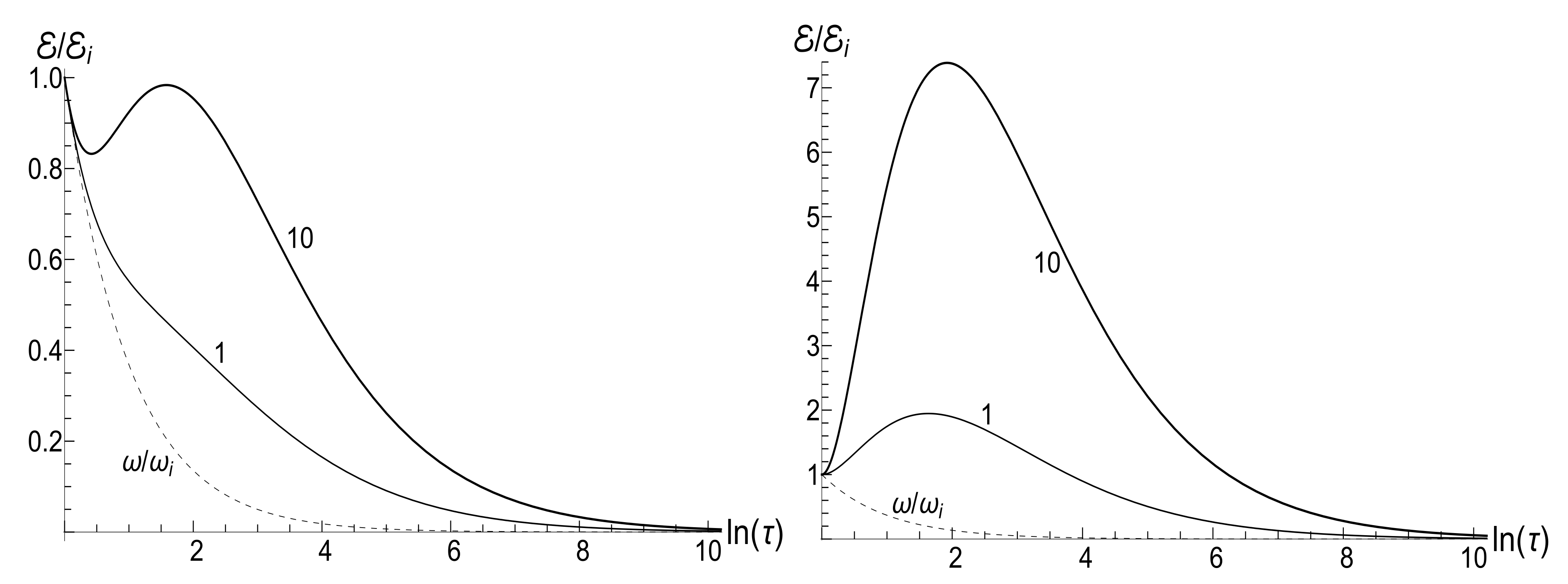
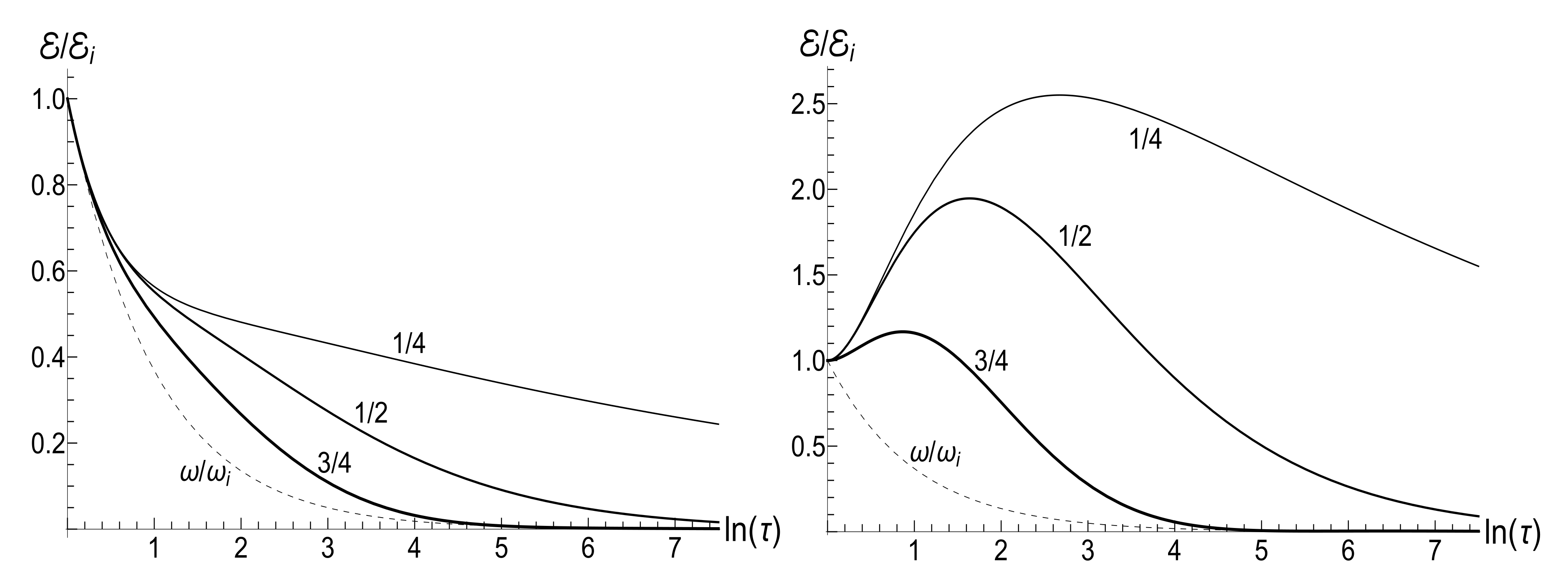
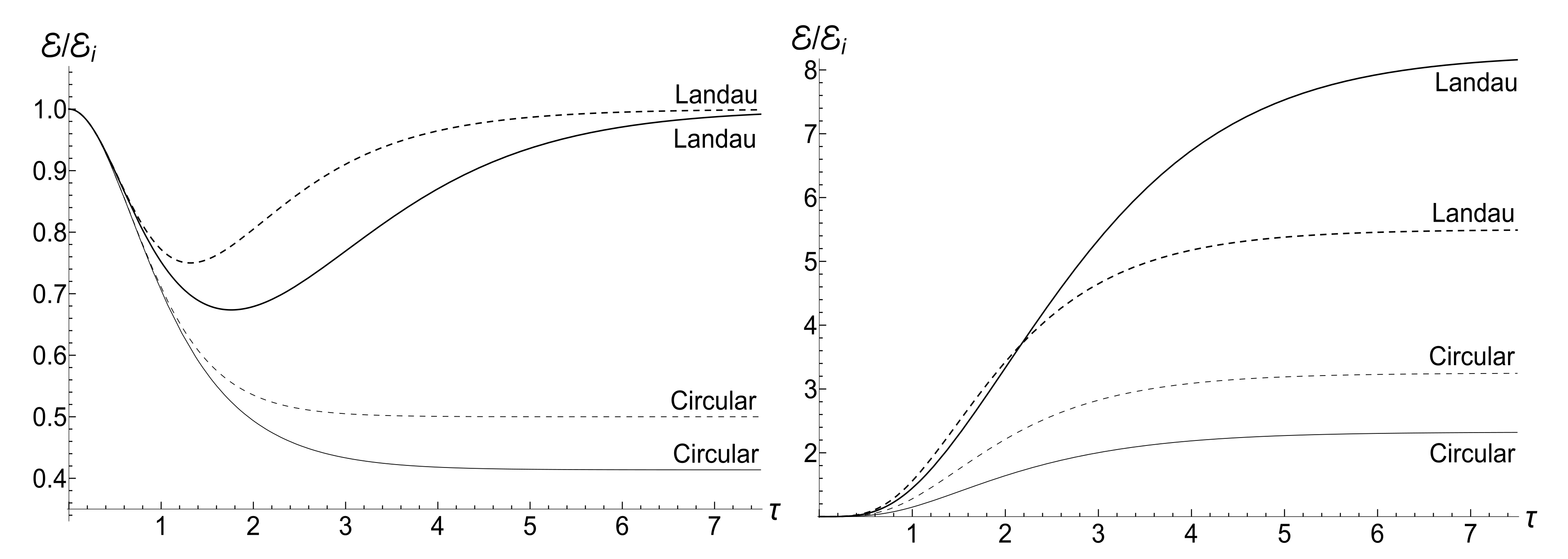
3.1. Evolution of the Mean Energy
3.1.1. Adiabatic Regime
3.1.2. Sudden Jump of the Magnetic Field
3.1.3. Parametric Resonance
3.2. Energy Fluctuations
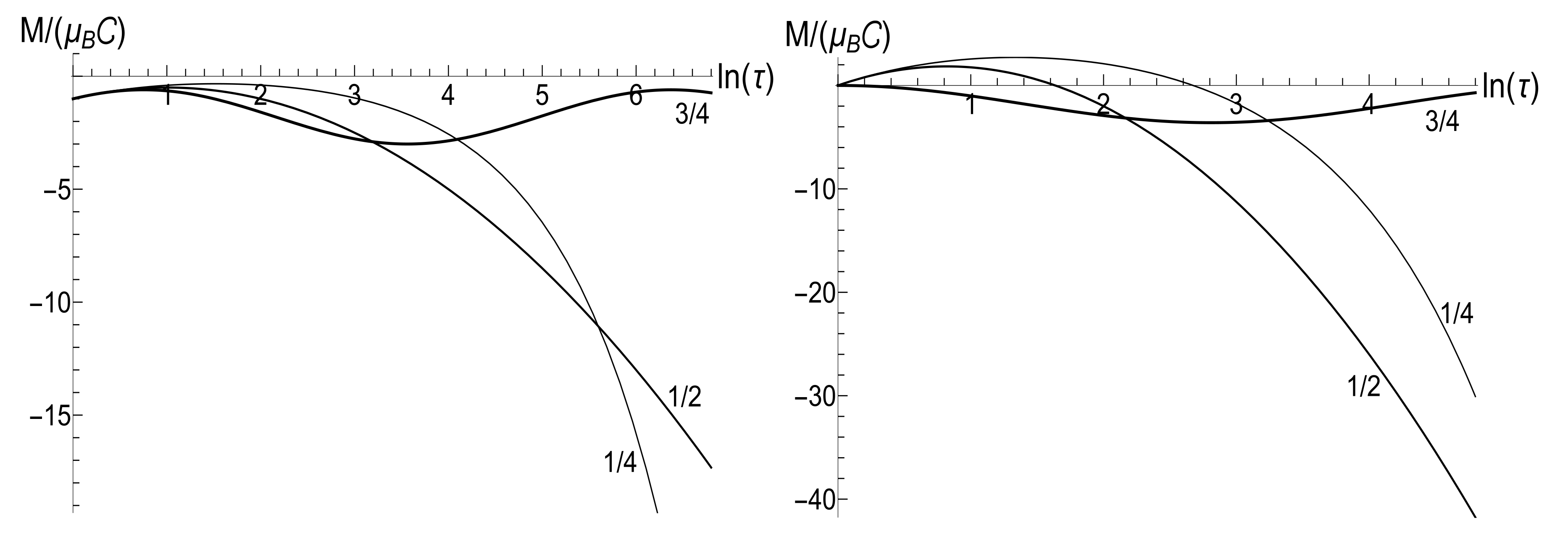
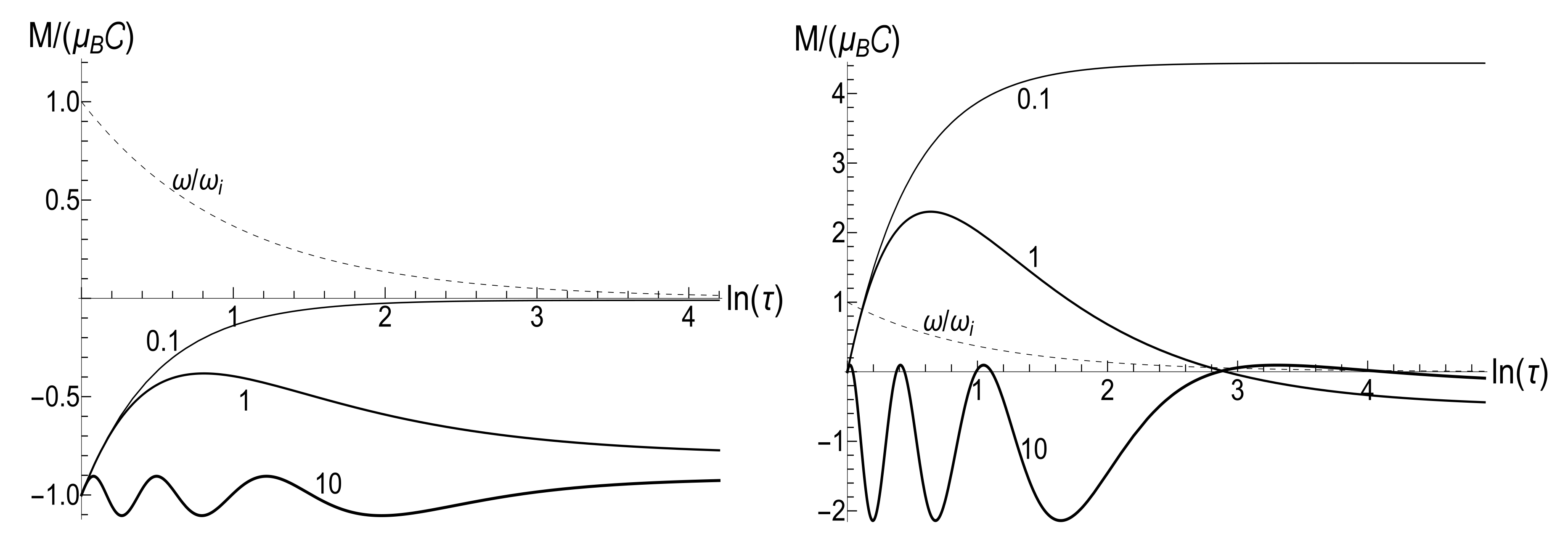
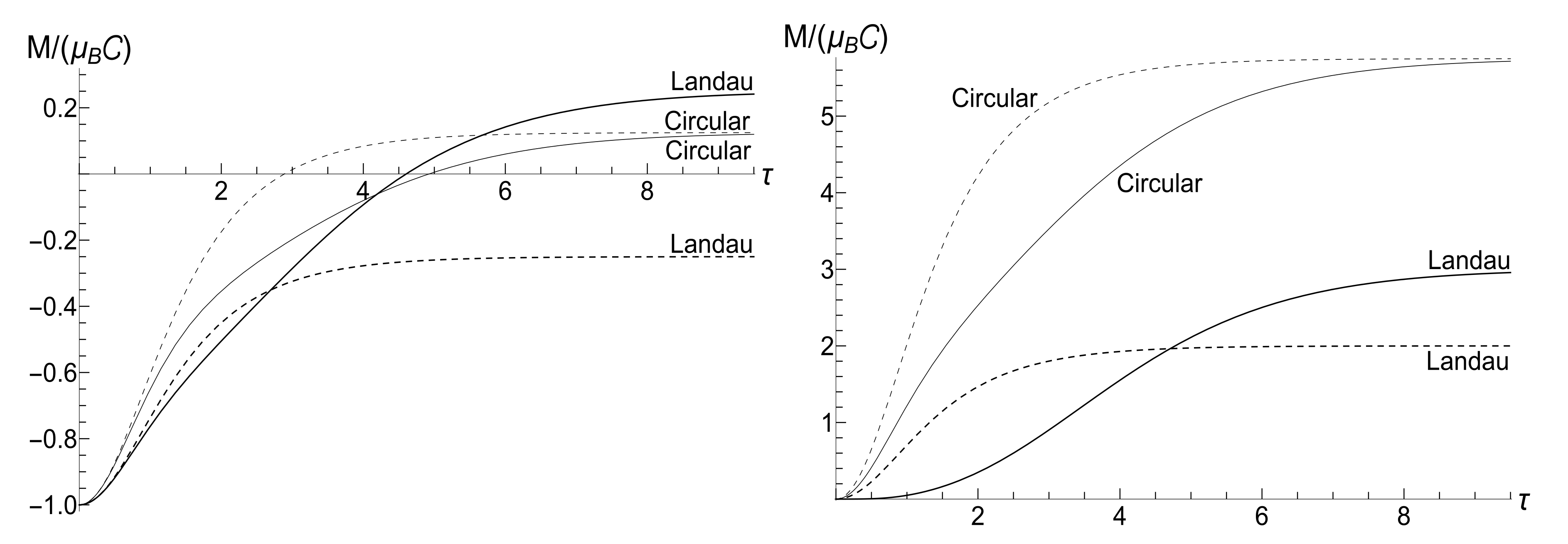
3.3. Evolution of the Mean Magnetic Moment
3.3.1. The Case of Sudden Jump
3.3.2. Parametric Resonance
3.4. Magnetic Moment Fluctuations
4. Explicit Solutions of the Oscillator Equation in Terms of Elementary Functions
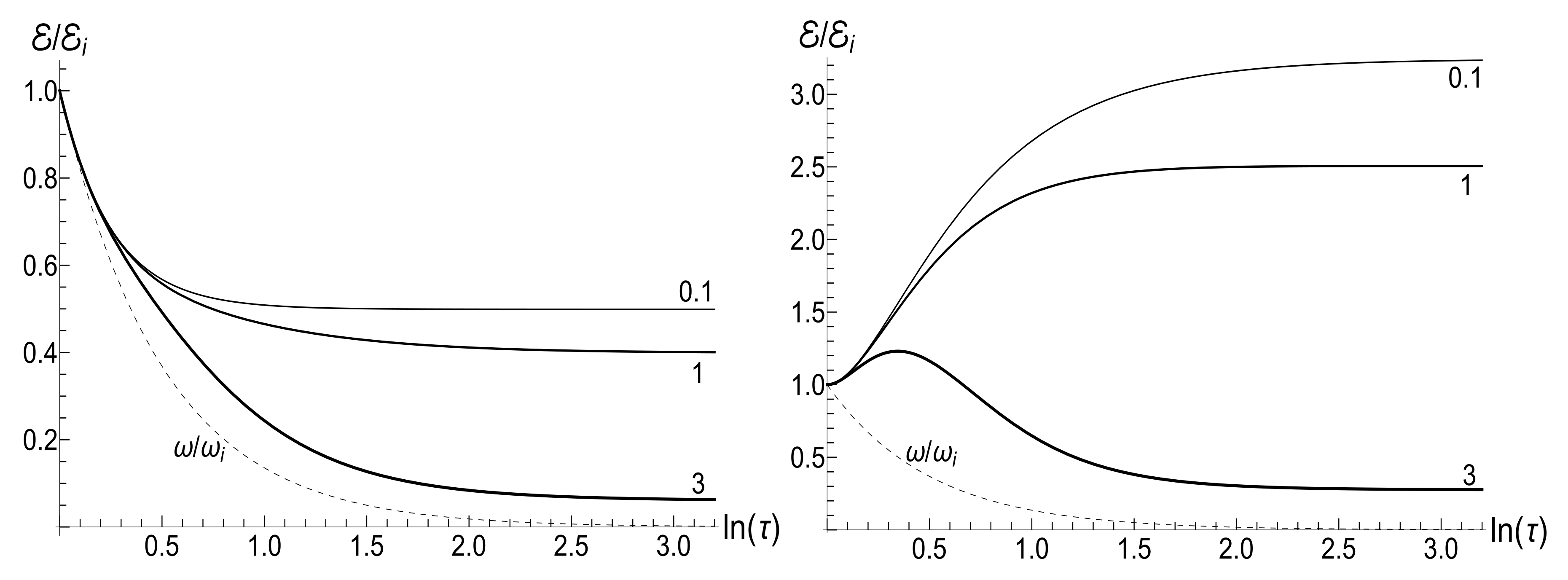
4.1. Inverse Linear Decrease of Magnetic Field
4.1.1. Fast Field Variation
4.1.2. Slow Field Variation
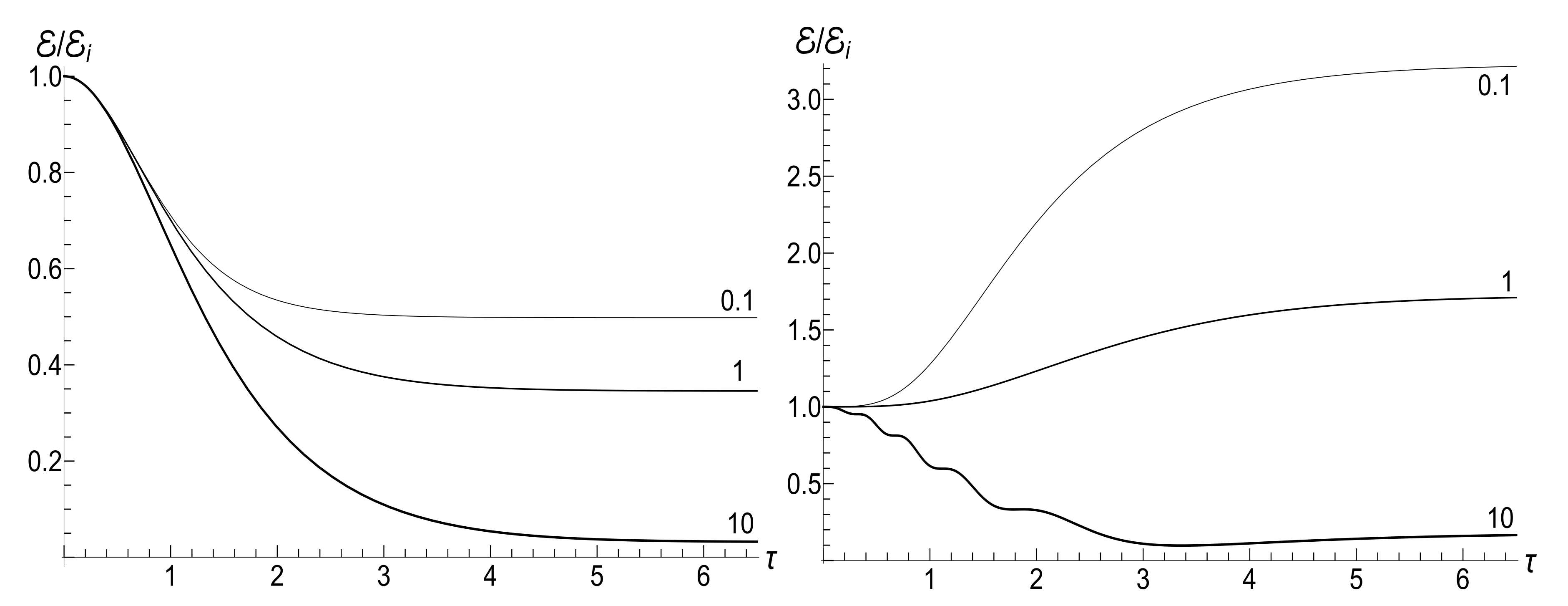
4.2. Inverse Quadratic Decrease of Magnetic Field
4.3. Exponential-Like Decrease of Frequency to a Final Value
4.3.1. A Decrease to Zero Final Frequency
5. Exact Solutions in Terms of the Confluent Hypergeometric and Cylindrical Functions
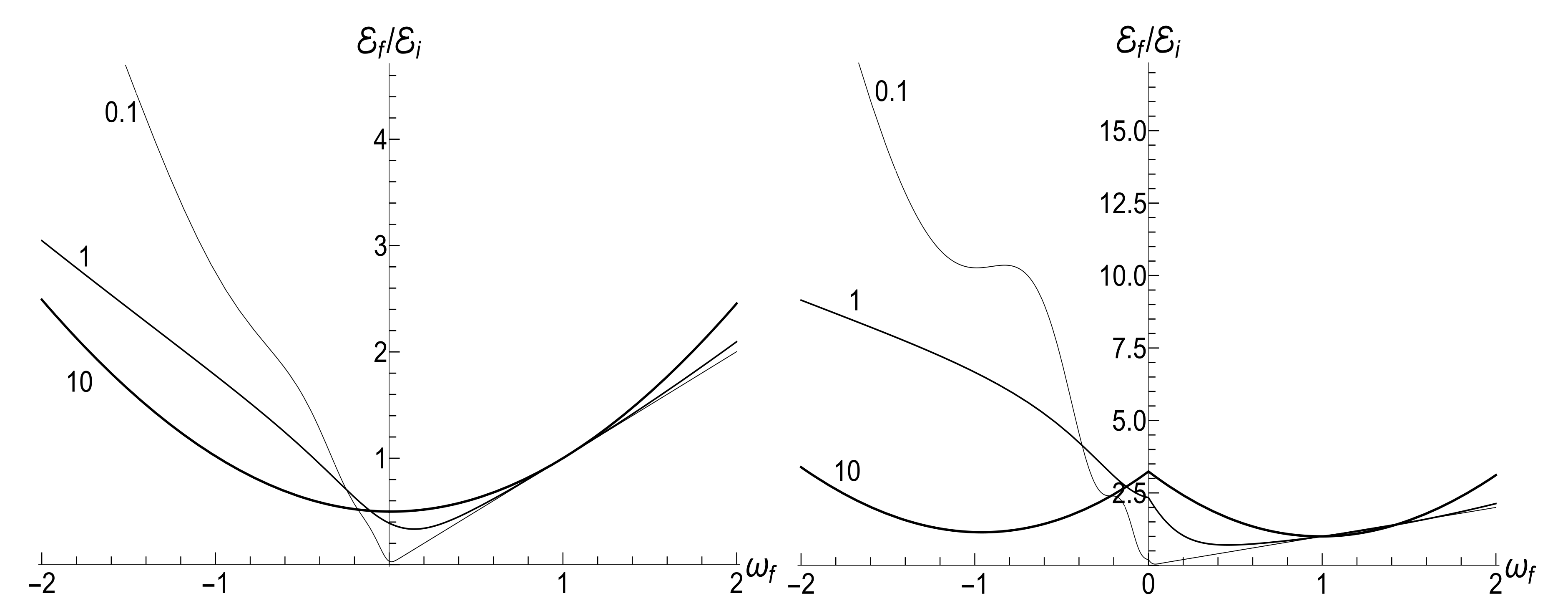
5.1. Mean Energy
5.2. Mean Magnetic Moment in the Asymptotic Regime
5.3. Exponential Switching off the Field: Solutions in Terms of the Hankel Functions
5.3.1. Mean Energy
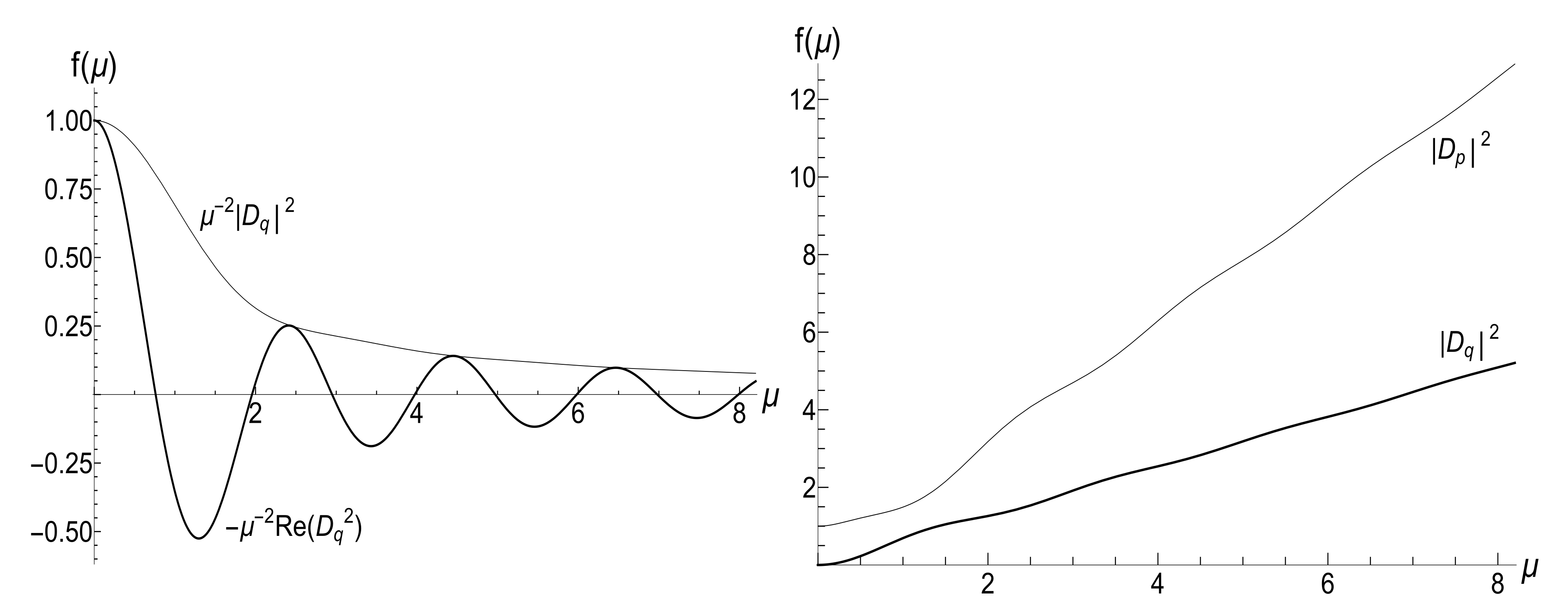
6. The Epstein–Eckart Profiles: Solutions in Terms of the Gauss Hypergeometric Functions
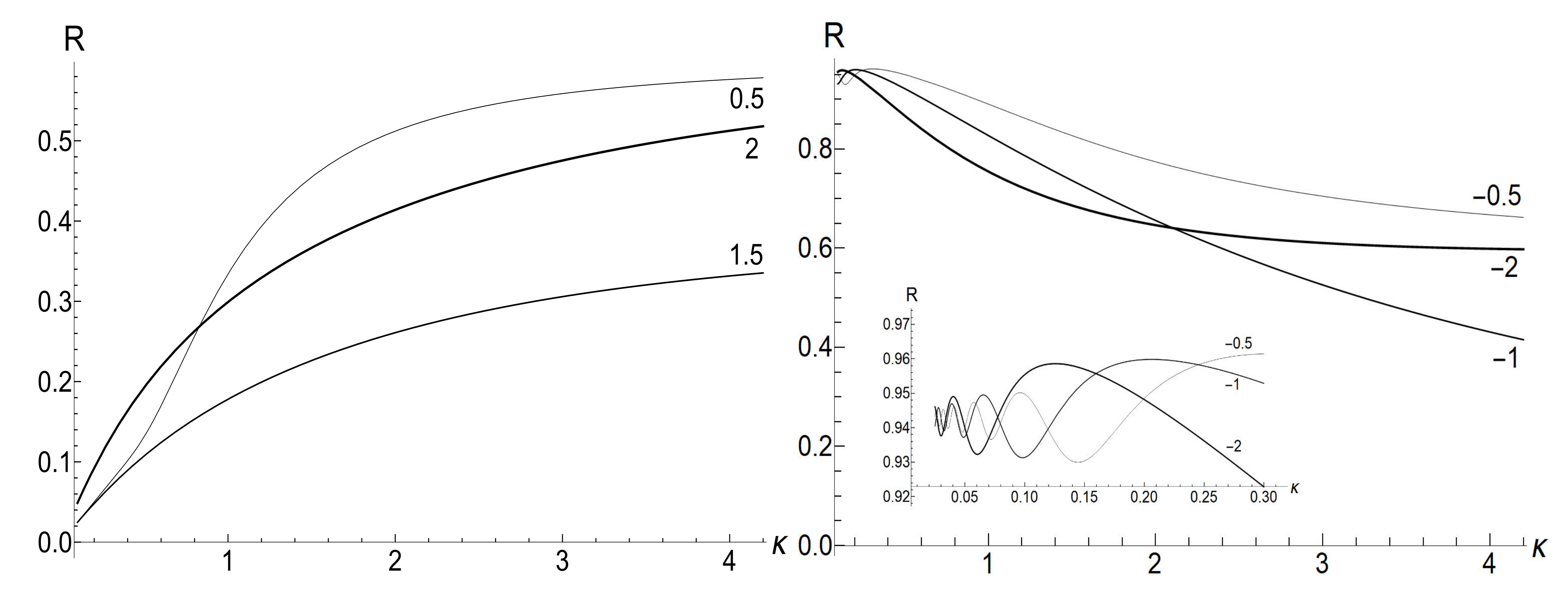
6.1. Evolution on the Whole Time Axis
6.2. “Mild” Transition to the Exponential Decay on the Semi-Axis
6.2.1. Mean Energy
6.2.2. Mean Magnetic Moment
7. Landau Gauge: General Formulas
7.1. Mean Energy
7.1.1. Adiabatic Evolution
7.1.2. Non-Adiabatic Evolution
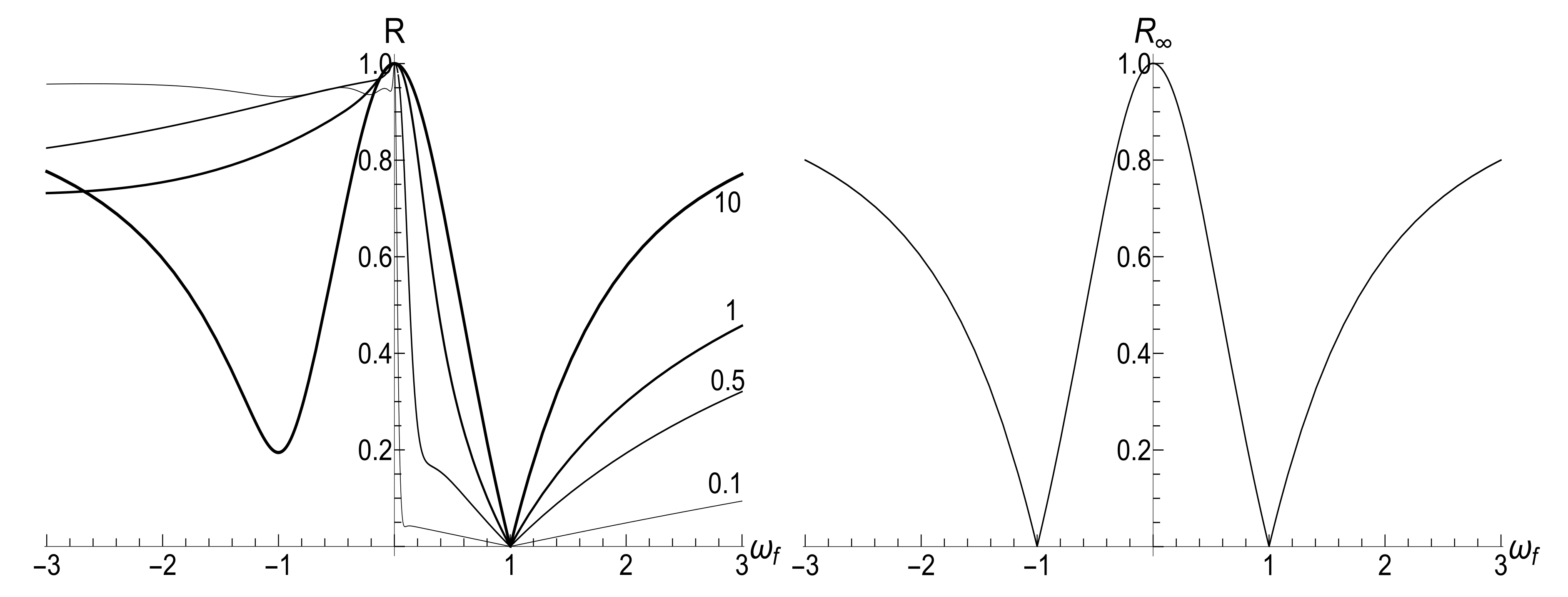
7.1.3. Sudden Jump
7.1.4. Parametric Resonance
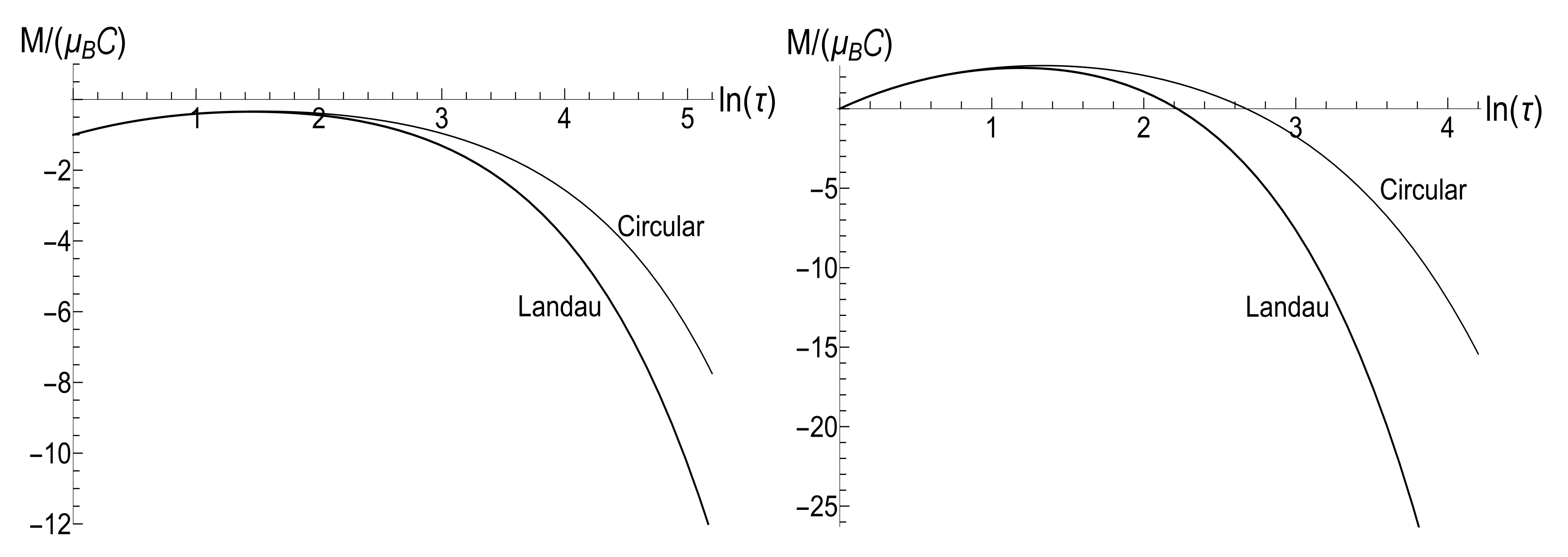
7.2. Mean Magnetic Moment
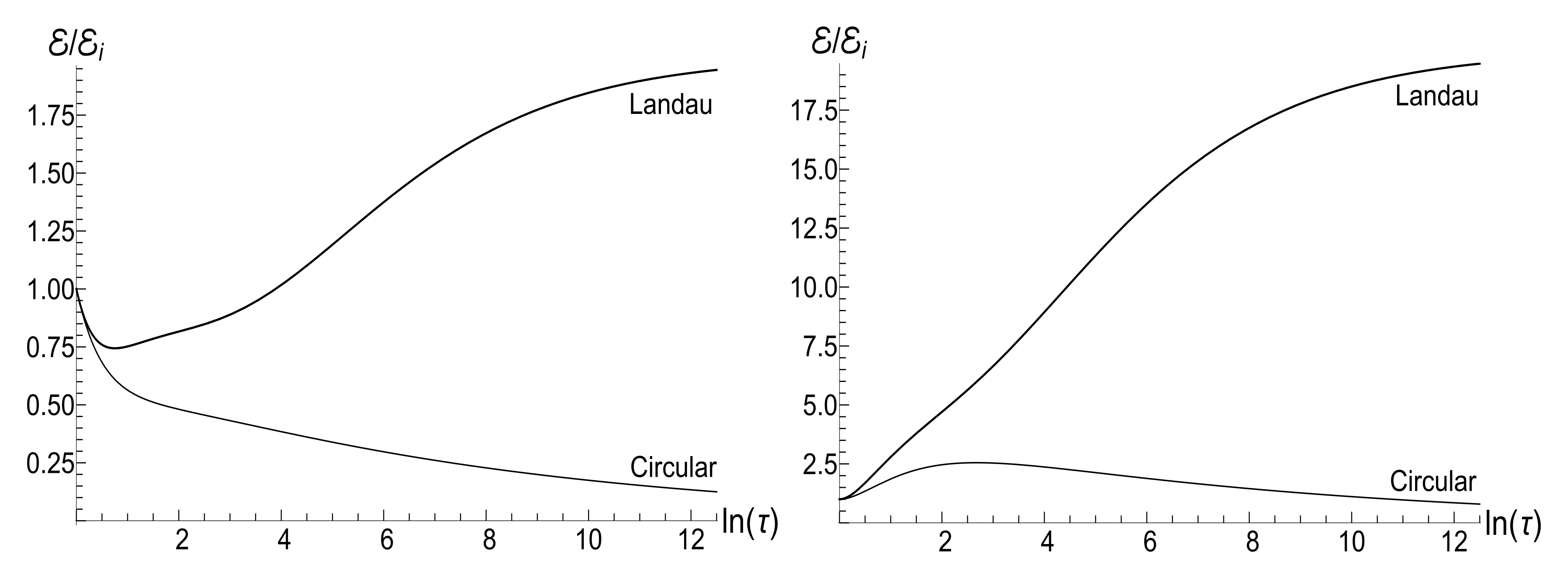
8. Landau Gauge: Explicit Examples
8.1. Inverse Linear Decrease of Magnetic Field
8.1.1. Fast Variations
8.1.2. Slow Variations
8.1.3. Intermediate Case
8.2. Exponential-Like Decrease of the Magnetic Field
8.2.1. Evolution of the Mean Energy
8.2.2. Evolution of the Mean Magnetic Moment
8.3. Dynamics of “Fast Jump to Zero”
9. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Details of General Solutions for the Circular Gauge
Appendix B. The Fourth-Order Moments in Terms of the Second-Order Ones for the Gaussian States
Appendix C. Asymptotic Formulas for in the Case of Exponentially Varying Frequency on the Time Semi-Axis
Appendix D. Analytic Corrections to the Sudden Jump Approximation for in the Case of Exponentially Varying Frequency on the Time Semi-Axis
Appendix E. Solution for the Epstein–Eckart Profile on the Whole Line
Appendix F. Asymptotic Functions Describing the Magnetic Moment in the Landau Gauge
Appendix G. Time-Dependent Landau Levels
Appendix H. Non-Equvalence of Time-Dependent Gauges
References
- Kennard, E.H. Zur Quantenmechanik einfacher Bewegungstypen. Z. Phys. 1927, 44, 326–352. [Google Scholar] [CrossRef]
- Darwin, C.G. Free motion in wave mechanics. Proc. R. Soc. Lond. A 1927, 117, 258–293. [Google Scholar]
- Fock, V. Bemerkung zur Quantelung des harmonischen Oszillators im Magnetfeld. Z. Phys. 1928, 47, 446–448. [Google Scholar] [CrossRef]
- Landau, L. Diamagnetismus der Metalle. Z. Phys. 1930, 64, 629–637. [Google Scholar] [CrossRef]
- Page, L. Deflection of electrons by a magnetic field on the wave mechanics. Phys. Rev. 1930, 36, 444–456. [Google Scholar] [CrossRef]
- Darwin, C.G. The diamagnetism of the free electron. Math. Proc. Camb. Phil. Soc. 1931, 27, 86–90. [Google Scholar] [CrossRef]
- Lewis, H.R., Jr.; Riesenfeld, W.B. An exact quantum theory of the time-dependent harmonic oscillator and of a charged particle in a time-dependent electromagnetic field. J. Math. Phys. 1969, 10, 1458–1473. [Google Scholar] [CrossRef]
- Malkin, I.A.; Man’ko, V.I.; Trifonov, D.A. Invariants and evolution of coherent states for charged particle in time-dependent magnetic field. Phys. Lett. A 1969, 30, 414–416. [Google Scholar] [CrossRef]
- Malkin, I.A.; Man’ko, V.I.; Trifonov, D.A. Coherent states and transition probabilities in a time-dependent electromagnetic field. Phys. Rev. D 1970, 2, 1371–1385. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Malkin, I.A.; Man’ko, V.I. Coherent states of a charged particle in a time-dependent uniform electromagnetic field of a plane current. Physica 1972, 59, 241–256. [Google Scholar] [CrossRef]
- Agayeva, R.G. Non-adiabatic parametric excitation of oscillator-type systems. J. Phys. A Math. Gen. 1980, 13, 1685–1699. [Google Scholar] [CrossRef]
- Abdalla, M.S. Charged harmonic oscillator in the presence of electric and magnetic fields. Nuovo Cim. B 1988, 101, 267–283. [Google Scholar] [CrossRef]
- Abdalla, M.S. Charged particle in the presence of a variable magnetic field. Phys. Rev. A 1988, 37, 4026–4029. [Google Scholar] [CrossRef]
- Jannussis, A.; Vlahos, E.; Skaltsas, D.; Kliros, G.; Bartzis, V. Squeezed states in the presence of a time-dependent magnetic field. Nuovo Cim. B 1989, 104, 53–66. [Google Scholar] [CrossRef]
- De Souza Dutra, A.; Farina de Souza, C.; Claudio de Albuquerque, L. The propagator for a charged oscillator with a time-dependent mass in a time-varying electromagnetic field. Phys. Lett. A 1991, 156, 371–376. [Google Scholar] [CrossRef]
- Baseia, B. On the generation of squeezing for a charged oscillator in a magnetic field. Phys. Lett. A 1992, 170, 311–314. [Google Scholar] [CrossRef]
- Baseia, B.; Mizrahi, S.S.; Moussa, M.H.Y. Generation of squeezing for a charged oscillator and a charged particle in a time dependent electromagnetic field. Phys. Rev. A 1992, 46, 5885–5889. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Man’ko, O.V.; Man’ko, V.I.; Polynkin, P.G.; Rosa, L. δ-kicked Landau levels. J. Phys. A Math. Gen. 1995, 28, 197–208. [Google Scholar] [CrossRef]
- Delgado, F.C.; Mielnik, B. Magnetic control of squeezing effects. J. Phys. A Math. Gen. 1998, 31, 309–320. [Google Scholar]
- Yüce, C. Exact solution of the time-dependent harmonic plus an inverse harmonic potential with a time-dependent electromagnetic field. Ann. Phys. 2003, 308, 599–604. [Google Scholar] [CrossRef]
- Abdalla, M.S.; Choi, J.-R. Propagator for the time-dependent charged oscillator via linear and quadratic invariants. Ann. Phys. 2007, 322, 2795–2810. [Google Scholar] [CrossRef]
- Menouar, S.; Maamache, M.; Choi, J.R. An alternative approach to exact wave functions for time-dependent coupled oscillator model of charged particle in variable magnetic field. Ann. Phys. 2010, 325, 1708–1719. [Google Scholar] [CrossRef][Green Version]
- Man’ko, V.I.; Zhebrak, E.D. Tomographic probability representation for states of charge moving in varying field. Opt. Spectrosc. 2012, 113, 624–629. [Google Scholar] [CrossRef]
- Zhebrak, E.D. Tomographic probability representation in the problem of transitions between Landau levels. Phys. Scr. 2013, T153, 014063. [Google Scholar] [CrossRef][Green Version]
- Menouar, S.; Choi, J.R. A hybrid approach for quantizing complicated motion of a charged particle in time-varying magnetic field. Ann. Phys. 2015, 353, 307–316. [Google Scholar] [CrossRef]
- Aguiar, V.; Guedes, I. Entropy and information of a spinless charged particle in time-varying magnetic fields. J. Math. Phys. 2016, 57, 092103. [Google Scholar] [CrossRef]
- Ying, Z.-J.; Wang, S.-J.; Zhang, W.-Z. Exact solution to Landau system with time-dependent electromagnetic fields. Chin. Phys. Lett. 1999, 16, 391–393. [Google Scholar] [CrossRef]
- Choi, J.R. Exact quantum theory of noninteracting electrons with time-dependent effective mass in a time-dependent magnetic field. J. Phys. Condens. Matter 2003, 15, 823–832. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Man’ko, V.I.; Polynkin, P.G. Geometrical squeezed states of a charged particle in a time-dependent magnetic field. Phys. Lett. A 1994, 188, 232–238. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Horovits, M.B. Squeezing of relative and center of orbit coordinates of a charged particle by step-wise variations of a uniform magnetic field with an arbitrary linear vector potential. J. Rus. Laser Res. 2018, 39, 389–400. [Google Scholar] [CrossRef]
- Johnson, M.H.; Lippmann, B.A. Motion in a constant magnetic field. Phys. Rev. 1949, 76, 828–832. [Google Scholar] [CrossRef]
- Dulock, V.A.; McIntosh, H.V. Degeneracy of cyclotron motion. J. Math. Phys. 1966, 7, 1401–1412. [Google Scholar] [CrossRef]
- Malkin, I.A.; Man’ko, V.I. Coherent states of a charged particle in a magnetic field. Sov. Phys.-JETP 1969, 28, 527–532. [Google Scholar]
- Feldman, A.; Kahn, A.H. Landau diamagnetism from the coherent states of an electron in a uniform magnetic field. Phys. Rev. B 1970, 1, 4584–4589. [Google Scholar] [CrossRef]
- Tam, W.G. Coherent states and the invariance group of a charged particle in a uniform magnetic field. Physica 1971, 54, 557–572. [Google Scholar] [CrossRef]
- Avron, J.E.; Herbst, I.W.; Simon, B. Separaltion of center of mass in homogeneous magnetic fields. Ann. Phys. 1978, 114, 431–451. [Google Scholar] [CrossRef]
- Johnson, B.R.; Hirschfelder, J.O.; Yang, K.-H. Interaction of atoms, molecules, and ions with constant electric and magnetic fields. Rev. Mod. Phys. 1983, 55, 109–153. [Google Scholar] [CrossRef]
- Von Baltz, R. Guiding center motion of two interacting n=0 Landau electrons in two dimensions. Phys. Lett. A 1984, 105, 371–373. [Google Scholar] [CrossRef]
- Kowalski, K.; Rembieliński, J. Coherent states of a charged particle in a uniform magnetic field. J. Phys. A Math. Gen. 2005, 38, 8247–8258. [Google Scholar] [CrossRef][Green Version]
- Mielnik, B.; Ramírez, A. Magnetic operations: A little fuzzy mechanics? Phys. Scr. 2011, 84, 045008. [Google Scholar] [CrossRef]
- Dodonov, V.V. Coherent states and their generalizations for a charged particle in a magnetic field. In Coherent States and Their Applications: A Contemporary Panorama; Springer Proceedings in Physics; Antoine, J.-P., Bagarello, F., Gazeau, J.-P., Eds.; Springer: Berlin, Germany, 2018; Volume 205, pp. 311–338. [Google Scholar]
- Champel, T.; Florens, S. A solvable model of Landau quantization breakdown. Eur. Phys. J. B 2019, 92, 124. [Google Scholar] [CrossRef]
- Wakamatsu, M.; Kitadono, Y.; Zou, L.; Zhang, P.M. The physics of helical electron beam in a uniform magnetic field as a testing ground of gauge principle. Phys. Lett. A 2020, 384, 126415. [Google Scholar] [CrossRef]
- Kitadono, Y.; Wakamatsu, M.; Zou, L.; Zhang, P.M. Role of guiding centre in Landau level system and mechanical and pseudo orbital angular momenta. Int. J. Mod. Phys. A 2020, 35, 2050096. [Google Scholar] [CrossRef]
- Fletcher, R.J.; Shaffer, A.; Wilson, C.C.; Patel, P.B.; Yan, Z.; Crépel, V.; Mukherjee, B.; Zwierlein, M.W. Geometric squeezing into the lowest Landau level. Science 2021, 372, 1318–1322. [Google Scholar] [CrossRef]
- Konstantinou, G.; Moulopoulos, K. Generators of dynamical symmetries and the correct gauge transformation in the Landau level problem: Use of pseudomomentum and pseudo-angular momentum. Eur. J. Phys. 2016, 37, 065401. [Google Scholar] [CrossRef]
- Li, C.-F.; Wang, Q. The quantum behavior of an electron in a uniform magnetic field. Physica B 1999, 269, 22–27. [Google Scholar] [CrossRef]
- Greenshields, C.R.; Stamps, R.L.; Franke-Arnold, S.; Barnett, S.M. Is the angular momentum of an electron conserved in a uniform magnetic field? Phys. Rev. Lett. 2014, 113, 240404. [Google Scholar] [CrossRef]
- Greenshields, C.R.; Stamps, R.L.; Franke-Arnold, S. Parallel axis theorem for free-space electron wavefunctions. New J. Phys. 2015, 17, 093015. [Google Scholar] [CrossRef]
- Wakamatsu, M.; Kitadono, Y.; Zhang, P.-M. The issue of gauge choice in the Landau problem and the physics of canonical and mechanical orbital angular momenta. Ann. Phys. 2018, 392, 287–322. [Google Scholar] [CrossRef]
- Van Enk, S.J. Angular momentum in the fractional quantum Hall effect. Am. J. Phys. 2020, 88, 286–291. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. The Classical Theory of Fields, 3rd ed.; Pergamon: Oxford, UK, 1971; pp. 101–103. [Google Scholar]
- Jackson, J.D. Classical Electrodynamics; Wiley: New York, NY, USA, 1999. [Google Scholar]
- Felderhof, B.U.; Raval, S.P. Diamagnetism of a confined electron gas. Physica A 1976, 82, 151–162. [Google Scholar] [CrossRef]
- March, N.H.; Tosi, M.P. Thermally averaged orbital diamagnetism of a localized Wigner oscillator. Nuovo Cim. D 1985, 6, 521–526. [Google Scholar] [CrossRef]
- Stewart, A.M. General gauge independence of diamagnetism plus paramagnetism. Aust. J. Phys. 2000, 53, 613–629. [Google Scholar] [CrossRef]
- Friar, J.L.; Fallieros, S. Diamagnetism, gauge transformations, and sum rules. Am. J. Phys. 1981, 49, 847–849. [Google Scholar] [CrossRef]
- Ishikawa, Y.; Fukuyama, H. Orbital magnetism and current distribution of two-dimensional electrons under confining potential. J. Phys. Soc. Jpn. 1999, 68, 2405–2413. [Google Scholar] [CrossRef][Green Version]
- Bliokh, K.Y.; Schattschneider, P.; Verbeeck, J.; Nori, F. Electron vortex beams in a magnetic field: A new twist on Landau levels and Aharonov-Bohm states. Phys. Rev. X 2012, 2, 041011. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Ivanov, I.P.; Guzzinati, G.; Clark, L.; van Boxem, R.; Béché, A.; Juchtmans, R.; Alonso, M.A.; Schattschneider, P.; Nori, F.; et al. Theory and applications of free-electron vortex states. Phys. Rep. 2017, 690, 1–70. [Google Scholar] [CrossRef]
- Wakamatsu, M.; Kitadono, Y.; Zou, L.-P.; Zhang, P.-M. Revisiting the compatibility problem between the gauge principle and the observability of the canonical orbital angular momentum in the Landau problem. Ann. Phys. 2021, 434, 168647. [Google Scholar] [CrossRef]
- Dodonov, V.V. Parametric excitation and generation of nonclassical states in linear media. In Theory of Nonclassical States of Light; Dodonov, V.V., Man’ko, V.I., Eds.; Taylor & Francis: London, UK, 2003; pp. 153–218. [Google Scholar]
- Dodonov, V.V.; Horovits, M.B. Change of energy and magnetic moment of a quantum charged particle after a fast jump of the magnetic field in solenoids of arbitrary cross sections. Physica A 2021, 571, 125843. [Google Scholar] [CrossRef]
- Hacyan, S. The quantum sling and the Schrödinger cat. Found. Phys. Lett. 1996, 9, 225–233. [Google Scholar] [CrossRef]
- Parker, L. Quantized fields and particle creation in expanding universes, II. Phys. Rev. D 1971, 3, 346–356. [Google Scholar] [CrossRef]
- Janszky, J.; Yushin, Y.Y. Squeezing via frequency jump. Opt. Commun. 1986, 59, 151–154. [Google Scholar] [CrossRef]
- Graham, R. Squeezing and frequency changes in harmonic oscillations. J. Mod. Opt. 1987, 34, 873–879. [Google Scholar] [CrossRef]
- Bechler, A. Generation of squeezed states in a homogeneous magnetic field. Phys. Lett. A 1988, 130, 481–482. [Google Scholar] [CrossRef]
- Ma, X.; Rhodes, W. Squeezing in harmonic oscillators with time-dependent frequencies. Phys. Rev. A 1989, 39, 1941–1947. [Google Scholar] [CrossRef]
- Lo, C.F. Squeezing by tuning the oscillator frequency. J. Phys. A Math. Gen. 1990, 23, 1155–1165. [Google Scholar] [CrossRef]
- Baseia, B.; De Brito, A.L.; Bagnato, V.S. Squeezing and antisqueezing for a harmonic oscillator having a sudden change of mass. Phys. Rev. A 1992, 45, 5308–5310. [Google Scholar] [CrossRef]
- Janszky, J.; Adam, P. Strong squeezing by repeated frequency jumps. Phys. Rev. A 1992, 46, 6091–6092. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Klimov, A.B.; Nikonov, D.E. Quantum phenomena in nonstationary media. Phys. Rev. A 1993, 47, 4422–4429. [Google Scholar] [CrossRef]
- Olendski, O. A charged particle in a time-varying magnetic field. J. Phys. A Math. Gen. 1993, 26, 7651–7661. [Google Scholar] [CrossRef]
- Kiss, T.; Janszky, J.; Adam, P. Time evolution of harmonic oscillators with time-dependent parameters: A step-function approximation. Phys. Rev. A 1994, 49, 4935–4942. [Google Scholar] [CrossRef]
- Sassaroli, E.; Srivastava, Y.N.; Widom, A. Photon production by the Dynamical casimir effect. Phys. Rev. A 1994, 50, 1027–1034. [Google Scholar] [CrossRef]
- Kira, M.; Tittonen, I.; Lai, W.K.; Stenholm, S. Semiclassical computations of time-dependent tunneling. Phys. Rev. A 1995, 51, 2826–2837. [Google Scholar] [CrossRef]
- Tittonen, I.; Stenholm, S.; Jex, J. Effect of a phase step on two-level atoms in a cavity. Opt. Commun. 1996, 124, 271–276. [Google Scholar] [CrossRef]
- Mendonça, J.T.; Guerreiro, A.; Martins, A.M. Quantum theory of time refraction. Phys. Rev. A 2000, 62, 033805. [Google Scholar] [CrossRef]
- Minguzzi, A.; Gangardt, D.M. Exact coherent states of a harmonically confined Tonks—Girardeau gas. Phys. Rev. Lett. 2005, 94, 240404. [Google Scholar] [CrossRef]
- Del Campo, A. Fermionization and bosonization of expanding one-dimensional anyonic fluids. Phys. Rev. A 2008, 78, 045602. [Google Scholar] [CrossRef]
- Hoffmann, K.H.; Salamon, P.; Rezek, Y.; Kosloff, R. Time-optimal controls for frictionless cooling in harmonic traps. EPL Europhys. Lett. 2011, 96, 60015. [Google Scholar] [CrossRef]
- Abah, O.; Roβnagel, J.; Jacob, G.; Deffner, S.; Schmidt-Kaler, F.; Singer, K.; Lutz, E. Single-ion heat engine at maximum power. Phys. Rev. Lett. 2012, 109, 203006. [Google Scholar] [CrossRef]
- Rajabpour, M.A.; Sotiriadis, S. Quantum quench of the trap frequency in the harmonic Calogero model. Phys. Rev. A 2014, 89, 033620. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Horovits, M.B. A quantum charged particle under sudden jumps of the magnetic field and shape of non-circular solenoids. Quantum Rep. 2019, 1, 193–207. [Google Scholar] [CrossRef]
- Vicari, E. Particle-number scaling of the quantum work statistics and Loschmidt echo in Fermi gases with time-dependent traps. Phys. Rev. A 2019, 99, 043603. [Google Scholar] [CrossRef]
- Tibaduiza, D.M.; Pires, L.; Szilard, D.; Zarro, C.A.D.; Farina, C.; Rego, A.L.C. A time-dependent harmonic oscillator with two frequency jumps: An exact algebraic solution. Braz. J. Phys. 2020, 50, 634–646. [Google Scholar] [CrossRef]
- Martínez-Tibaduiza, D.; Pires, L.; Farina, C. Time-dependent quantum harmonic oscillator: A continuous route from adiabatic to sudden changes. J. Phys. B At. Mol. Opt. Phys. 2021, 54, 205401. [Google Scholar] [CrossRef]
- Louisell, W.H.; Yariv, A.; Siegman, A.E. Quantum fluctuations and noise in parametric processes. I. Phys. Rev. 1961, 124, 1646–1654. [Google Scholar] [CrossRef]
- Mollow, B.R.; Glauber, R.J. Quantum theory of parametric amplification. I. Phys. Rev. 1967, 160, 1076–1096. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Man’ko, V.I. Evolution of multidimensional systems. Magnetic properties of ideal gases of charged particles. In Invariants and the Evolution of Nonstationary Quantum Systems; Proceedings of Lebedev Physics Institute; Markov, M.A., Ed.; Nova Science: Commack, NY, USA, 1989; Volume 183, pp. 263–414. [Google Scholar]
- Hillery, M.; O’Connell, R.F.; Scully, M.O.; Wigner, E.P. Distribution functions in physics: Fundamentals. Phys. Rep. 1984, 106, 121–167. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Dodonov, A.V. Magnetic-moment probability distribution of a quantum charged particle in thermodynamic equilibrium. Phys. Rev. A 2020, 102, 042216. [Google Scholar] [CrossRef]
- Seymour, P.W.; Leipnik, R.B.; Nicholson, A.F. Charged particle motion in a time-dependent axially symmetric magnetic field. Aust. J. Phys. 1965, 18, 553–565. [Google Scholar] [CrossRef]
- Lewis, H.R. Class of exact invariants for classical and quantum time-dependent harmonic oscillators. J. Math. Phys. 1968, 9, 1976–1986. [Google Scholar] [CrossRef]
- Eliezer, C.J.; Gray, A. A note on the time-dependent harmonic oscillator. SIAM J. Appl. Math. 1976, 30, 463–468. [Google Scholar] [CrossRef]
- Bagrov, V.G.; Gitman, D.M.; Pereira, A.S. Coherent states of systems with quadratic Hamiltonians. Braz. J. Phys. 2015, 45, 369–375. [Google Scholar] [CrossRef]
- Erdélyi, A. (Ed.) Bateman Manuscript Project: Higher Transcendental Functions; McGraw-Hill: New York, NY, USA, 1953. [Google Scholar]
- Eckart, C. The penetration of a potential barrier by electrons. Phys. Rev. 1930, 35, 1303–1309. [Google Scholar] [CrossRef]
- Epstein, P.S. Reflection of waves in an inhomogeneous absorbing medium. Proc. Nat. Acad. Sci. USA 1930, 16, 627–637. [Google Scholar] [CrossRef]
- Prudnikov, A.P.; Brychkov, Y.A.; Marichev, O.I. Integrals and Series. Volume 3. More Special Functions; Gordon & Breach: New York, NY, USA, 1990; Appendix II.3. [Google Scholar]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products, 7th ed.; Academic: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Varró, S. Coherent states of an electron in a homogeneous constant magnetic field and the zero magnetic field limit. J. Phys. A Math. Gen. 1984, 17, 1631–1638. [Google Scholar] [CrossRef]
- Dodonov, V.V. Magnetization dynamics of a harmonically confined quantum charged particle in time dependent magnetic fields inside a circular solenoid. J. Phys. A Math. Theor. 2021, 54, 295304. [Google Scholar] [CrossRef]
- Malkin, I.A.; Man’ko, V.I.; Trifonov, D.A. Linear adiabatic invariants and coherent states. J. Math. Phys. 1973, 14, 576–582. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Man’ko, O.V. Quantum damped oscillator in a magnetic field. Phys. A 1985, 130, 353–366. [Google Scholar] [CrossRef]
- Li, X.L.; Ford, G.W.; O’Connell, R.F. Charged oscillator in a heat bath in the presence of a magnetic field. Phys. Rev. A 1990, 42, 4519–4527. [Google Scholar] [CrossRef]
- Li, X.L.; O’Connell, R.F. Green’s function and position correlation function for a charged oscillator in a heat bath and a magnetic field. Phys. A 1996, 224, 639–668. [Google Scholar] [CrossRef]
- Smirnov, A.Y. Radiation-induced reduction of a wave packet in a magnetic field. Phys. A 1998, 253, 161–177. [Google Scholar] [CrossRef]
- Schuch, D.; Moshinsky, M. Coherent states and dissipation for the motion of a charged particle in a constant magnetic field. J. Phys. A Math. Gen. 2003, 36, 6571–6585. [Google Scholar] [CrossRef]
- Genkin, M.; Lindroth, E. Environmental effects on the phase space dynamics and decoherence time scale of a charged particle in a Penning trap. J. Phys. A Math. Theor. 2009, 42, 385302. [Google Scholar] [CrossRef]
- Bandyopadhyay, M. Dissipative cyclotron motion of a charged quantum-oscillator and third law. J. Stat. Phys. 2010, 140, 603–618. [Google Scholar] [CrossRef]
- Kalandarov, S.A.; Abdurakhmanov, I.B.; Kanokov, Z.; Adamian, G.G.; Antonenko, N.V. Angular momentum of open quantum systems in external magnetic field. Phys. Rev. A 2019, 99, 062109. [Google Scholar] [CrossRef]
- Pachón, L.A.; Brumer, P. Quantum driven dissipative parametric oscillator in a blackbody radiation field. J. Math. Phys. 2014, 55, 012103. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Malkin, I.A.; Man’ko, V.I. Integrals of the motion, Green functions and coherent states of dynamical systems. Int. J. Theor. Phys 1975, 14, 37–54. [Google Scholar] [CrossRef]
- Dodonov, V.V. Invariant quantum states of quadratic Hamiltonians. Entropy 2021, 23, 634. [Google Scholar] [CrossRef]
- Swenson, R.J. The correct relation between wavefunctions in two gauges. Am. J. Phys. 1989, 57, 381–382. [Google Scholar] [CrossRef]





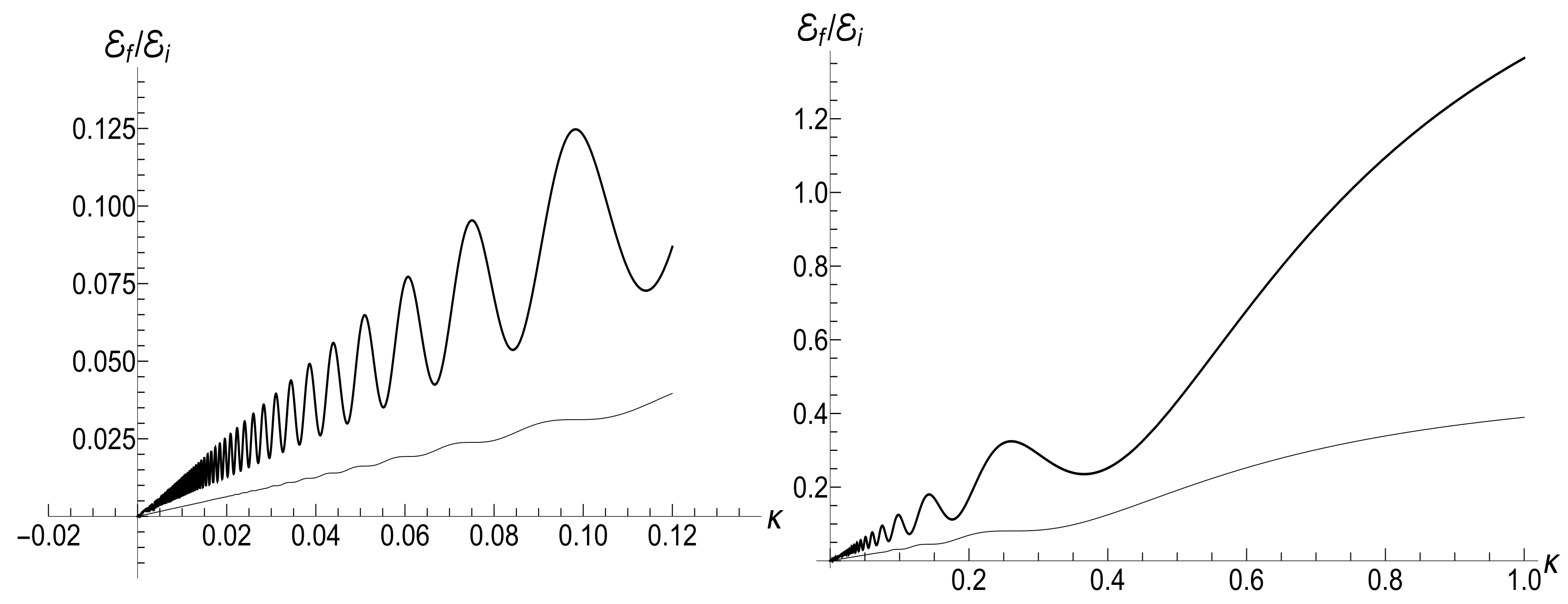
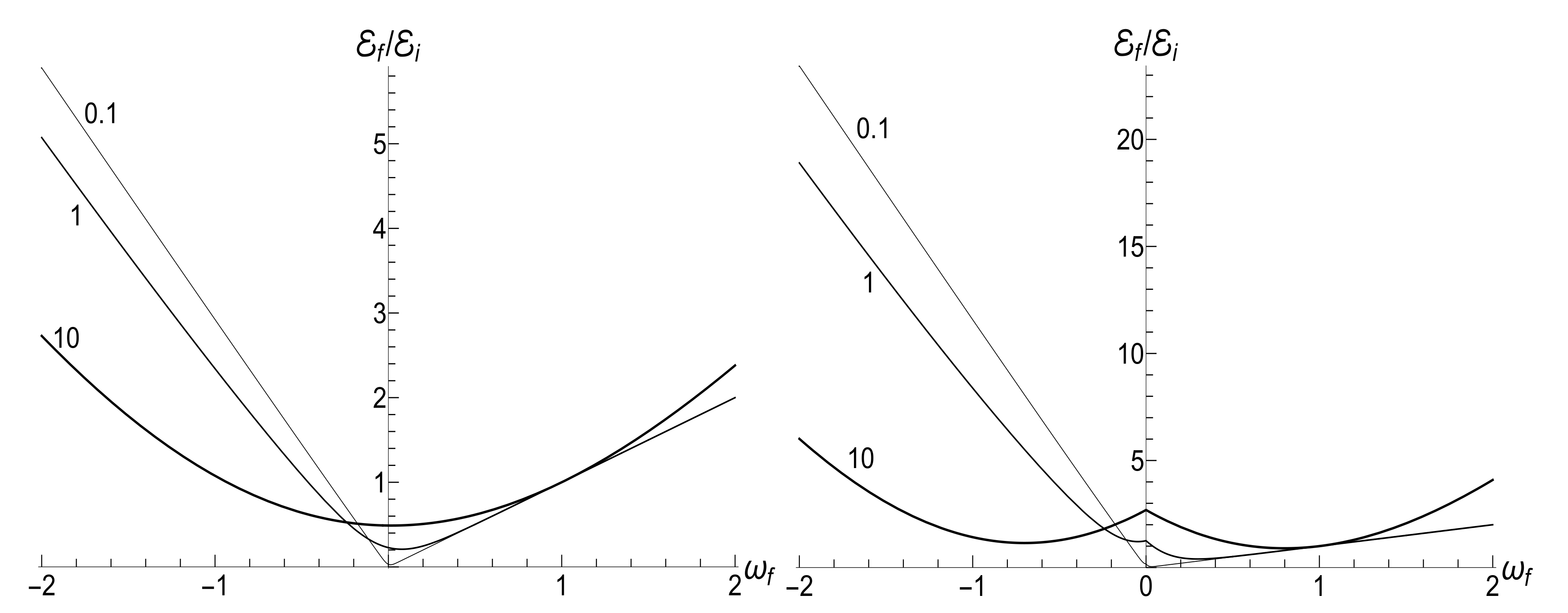
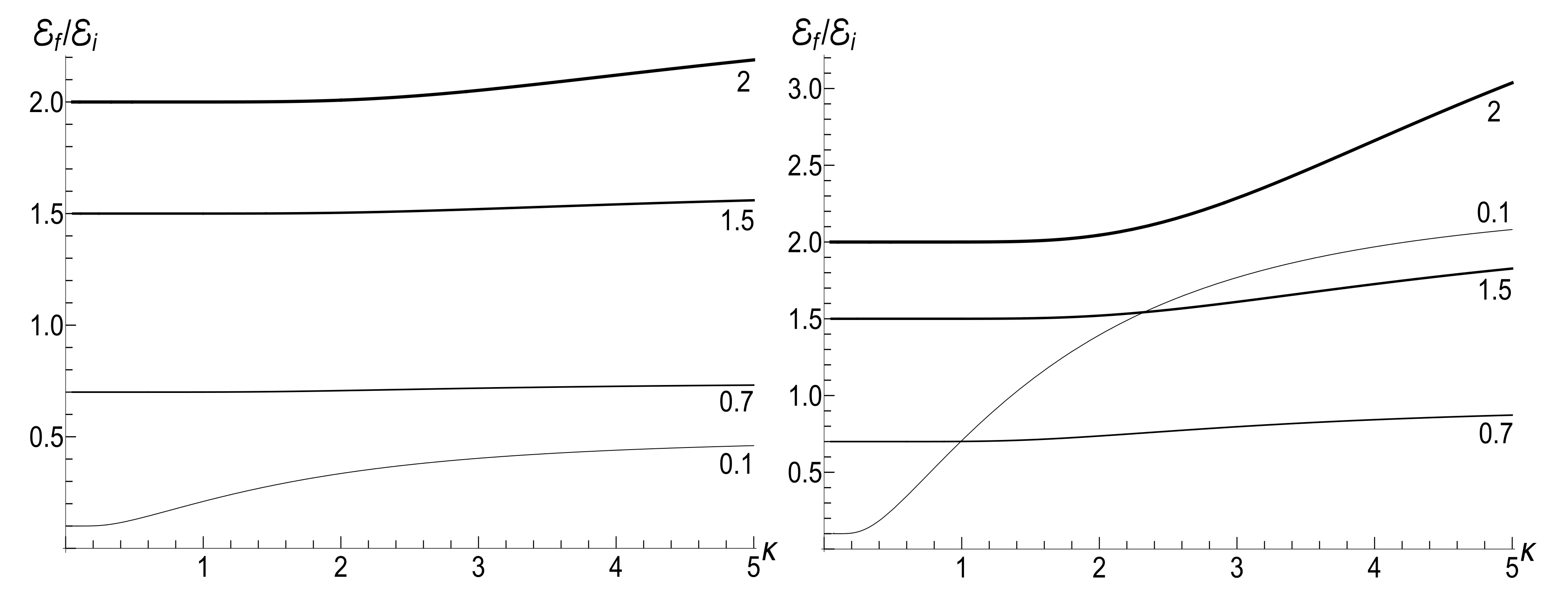
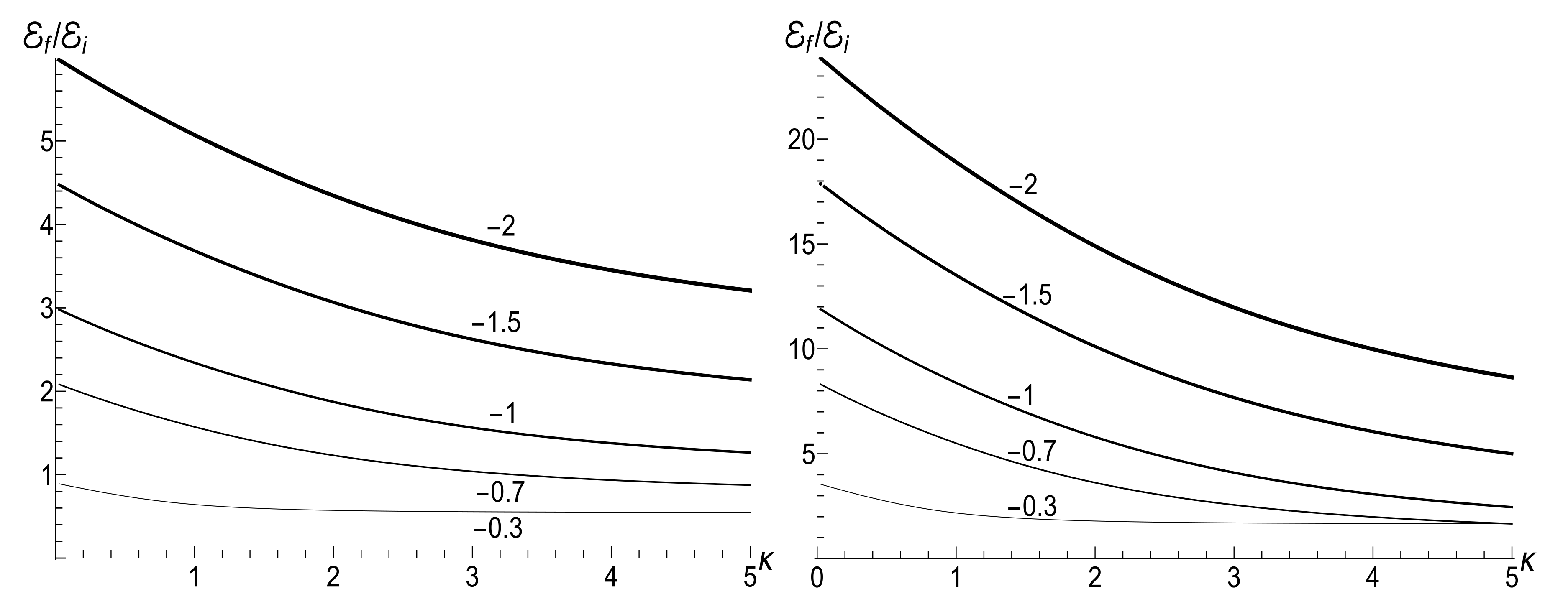

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dodonov, V.V.; Horovits, M.B. Energy and Magnetic Moment of a Quantum Charged Particle in Time-Dependent Magnetic and Electric Fields of Circular and Plane Solenoids. Entropy 2021, 23, 1579. https://doi.org/10.3390/e23121579
Dodonov VV, Horovits MB. Energy and Magnetic Moment of a Quantum Charged Particle in Time-Dependent Magnetic and Electric Fields of Circular and Plane Solenoids. Entropy. 2021; 23(12):1579. https://doi.org/10.3390/e23121579
Chicago/Turabian StyleDodonov, Viktor V., and Matheus B. Horovits. 2021. "Energy and Magnetic Moment of a Quantum Charged Particle in Time-Dependent Magnetic and Electric Fields of Circular and Plane Solenoids" Entropy 23, no. 12: 1579. https://doi.org/10.3390/e23121579
APA StyleDodonov, V. V., & Horovits, M. B. (2021). Energy and Magnetic Moment of a Quantum Charged Particle in Time-Dependent Magnetic and Electric Fields of Circular and Plane Solenoids. Entropy, 23(12), 1579. https://doi.org/10.3390/e23121579






