Lindblad Dynamics and Disentanglement in Multi-Mode Bosonic Systems
Abstract
1. Introduction
2. Lindblad Dynamics
2.1. Master Equation
2.2. Characteristic Function
3. Two-Mode System
3.1. Exact Dynamics of Averages and Covariance Matrix
3.2. Symplectic Eigenvalues and Logarithmic Negativity
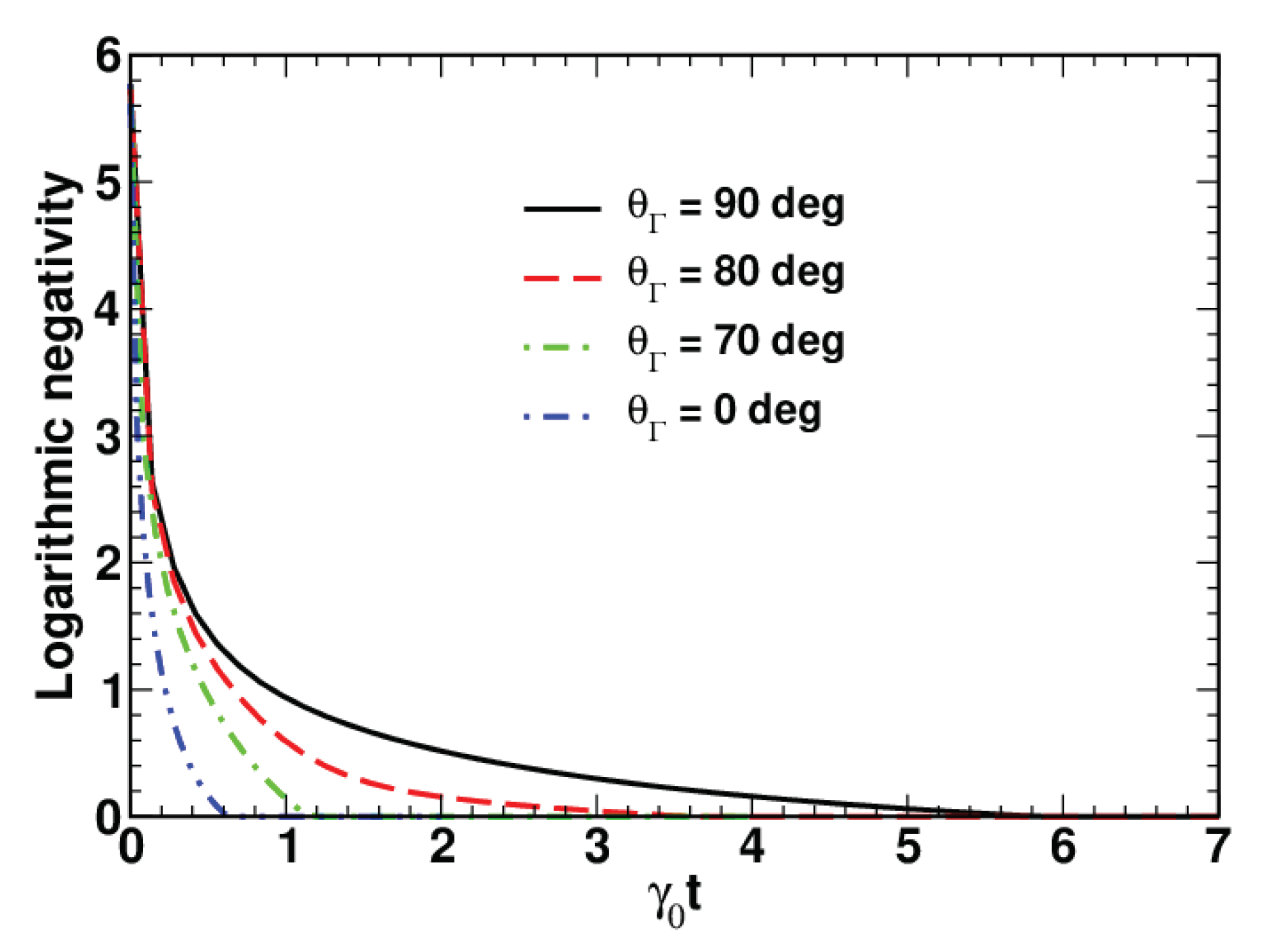
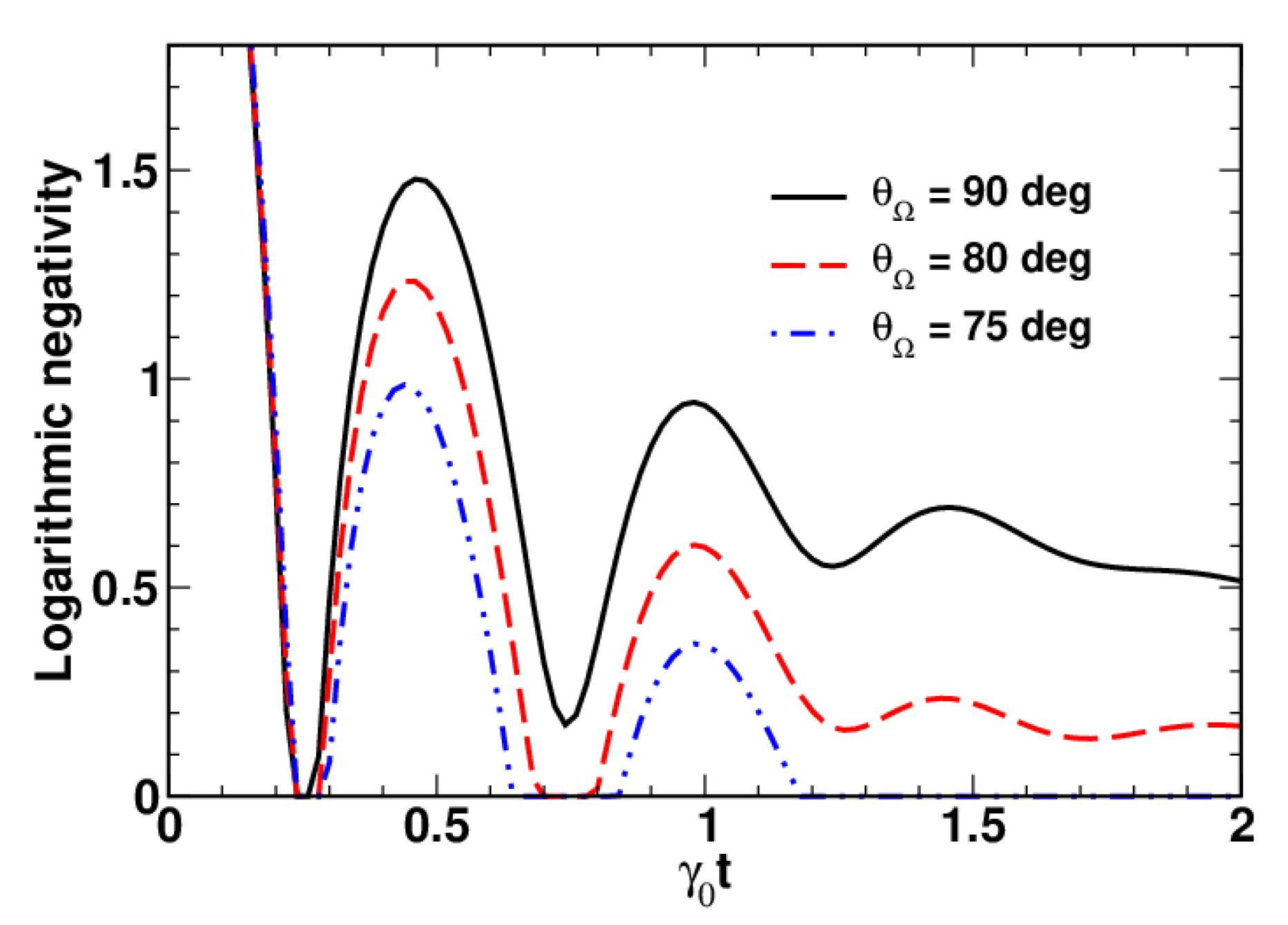
3.3. Numerical Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Stinespring, W.F. Positive functions on C*-algebras. Proc. Am. Math. Soc. 1955, 6, 211–216. [Google Scholar]
- Choi, M.D. Completely positive linear maps on complex matrices. Linear Algebra Its Appl. 1975, 10, 285–290. [Google Scholar] [CrossRef]
- Kraus, K. States, Effects, and Operations: Fundamental Notions of Quantum Theory; Lecture Notes in Physics; Springer: Heidelberg/Berlin, Germany, 1983; Volume 190. [Google Scholar]
- Holevo, A.S. On the mathematical theory of quantum communication channels. Probl. Peredachi Informatsii 1972, 8, 62–71. [Google Scholar] [CrossRef]
- Caruso, F.; Giovannetti, V.; Lupo, C.; Mancini, S. Quantum channels and memory effects. Rev. Mod. Phys. 2014, 86, 1203. [Google Scholar] [CrossRef]
- Carmichael, H. An Open Systems Approach to Quantum Optics; Springer: Heidelberg/Berlin, Germany, 1993. [Google Scholar]
- Omnès, R. General theory of the decoherence effect in quantum mechanics. Phys. Rev. A 1997, 56, 3383. [Google Scholar] [CrossRef]
- Breuer, H.P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Rivas, A.; Huelga, S.F. Open Quantum Systems: An Introduction; SpringerBriefs in Physics; Springer: Heidelberg/Berlin, Germany, 2012. [Google Scholar]
- Rivas, A.; Huelga, S.F.; Plenio, M.B. Quantum non-Markovianity: Characterization, quantification and detection. Rep. Prog. Phys. 2014, 77, 094001. [Google Scholar] [CrossRef]
- de Vega, I.; Alonso, D. Dynamics of non-Markovian open quantum systems. Rev. Mod. Phys. 2017, 89, 015001. [Google Scholar] [CrossRef]
- Kossakowski, A. On quantum statistical mechanics of non-Hamiltonian systems. Rep. Math. Phys. 1972, 3, 247–274. [Google Scholar] [CrossRef]
- Lindblad, G. On the generators of quantum dynamical semigroups. Commun. Math. Phys. 1976, 48, 119–130. [Google Scholar] [CrossRef]
- Gorini, V.; Kossakowski, A.; Sudarshan, E.C.G. Completely positive dynamical semigroups of N-level systems. J. Math. Phys. 1976, 17, 821–825. [Google Scholar] [CrossRef]
- Pearle, P. Simple derivation of the Lindblad equation. Eur. J. Phys. 2012, 33, 805. [Google Scholar] [CrossRef]
- Albash, T.; Boixo, S.; Lidar, D.A.; Zanardi, P. Quantum adiabatic Markovian master equations. New J. Phys. 2012, 14, 123016. [Google Scholar] [CrossRef]
- McCauley, G.; Cruikshank, B.; Bondar, D.I.; Jacobs, K. Accurate Lindblad-form master equation for weakly damped quantum systems across all regimes. Npj Quantum Inf. 2020, 6, 74. [Google Scholar] [CrossRef]
- Manzano, D. A short introduction to the Lindblad master equation. Aip Adv. 2020, 10, 025106. [Google Scholar] [CrossRef]
- Arévalo-Aguilar, L.M.; Moya-Cessa, H. Solution to the master equation for a quantized cavity mode. Quantum Semiclassical Opt. J. Eur. Opt. Soc. Part B 1998, 10, 671. [Google Scholar] [CrossRef]
- Klimov, A.B.; Romero, J.L. An algebraic solution of Lindblad-type master equations. J. Opt. B Quantum Semiclassical Opt. 2003, 5, S316. [Google Scholar] [CrossRef]
- Lu, H.X.; Yang, J.; Zhang, Y.D.; Chen, Z.B. Algebraic approach to master equations with superoperator generators of su(1, 1) and su(2) Lie algebras. Phys. Rev. A 2003, 67, 024101. [Google Scholar] [CrossRef]
- Tay, B.A.; Petrosky, T. Biorthonormal eigenbasis of a Markovian master equation for the quantum Brownian motion. J. Math. Phys. 2008, 49, 113301. [Google Scholar] [CrossRef]
- Honda, D.; Nakazato, H.; Yoshida, M. Spectral resolution of the Liouvillian of the Lindblad master equation for a harmonic oscillator. J. Math. Phys. 2010, 51, 072107. [Google Scholar] [CrossRef]
- Tay, B.A. Eigenvalues of the Liouvillians of quantum master equation for a harmonic oscillator. Phys. A 2020, 556, 124768. [Google Scholar] [CrossRef]
- Shishkov, V.Y.; Andrianov, E.S.; Pukhov, A.A.; Vinogradov, A.P.; Lisyansky, A.A. Perturbation theory for Lindblad superoperators for interacting open quantum systems. Phys. Rev. A 2020, 102, 032207. [Google Scholar] [CrossRef]
- Benatti, F.; Floreanini, R. Entangling oscillators through environment noise. J. Phys. A Math. Gen. 2006, 39, 2689. [Google Scholar] [CrossRef]
- Teuber, L.; Scheel, S. Solving the quantum master equation of coupled harmonic oscillators with Lie-algebra methods. Phys. Rev. A 2020, 101, 042124. [Google Scholar] [CrossRef]
- Hiroshima, T. Decoherence and entanglement in two-mode squeezed vacuum states. Phys. Rev. A 2004, 63, 022305. [Google Scholar] [CrossRef]
- Serafini, A.; Illuminati, F.; Paris, M.G.A.; De Siena, S. Entanglement and purity of two-mode Gaussian states in noisy channels. Phys. Rev. A 2004, 69, 022318. [Google Scholar] [CrossRef]
- de Castro, A.; Siqueira, R.; Dodonov, V. Effect of dissipation and reservoir temperature on squeezing exchange and emergence of entanglement between two coupled bosonic modes. Phys. Lett. A 2008, 372, 367–374. [Google Scholar] [CrossRef]
- Chou, C.H.; Yu, T.; Hu, B.L. Exact master equation and quantum decoherence of two coupled harmonic oscillators in a general environment. Phys. Rev. E 2008, 77, 011112. [Google Scholar] [CrossRef]
- Paz, J.P.; Roncaglia, A.J. Dynamics of the entanglement between two oscillators in the same environment. Phys. Rev. Lett. 2008, 100, 220401. [Google Scholar] [CrossRef]
- Paz, J.P.; Roncaglia, A.J. Dynamical phases for the evolution of the entanglement between two oscillators coupled to the same environment. Phys. Rev. A 2009, 79, 032102. [Google Scholar] [CrossRef]
- Barbosa, F.A.S.; de Faria, A.J.; Coelho, A.S.; Cassemiro, K.N.; Villar, A.S.; Nussenzveig, P.; Martinelli, M. Disentanglement in bipartite continuous-variable systems. Phys. Rev. A 2011, 84, 052330. [Google Scholar] [CrossRef]
- Figueiredo, E.; Linhares, C.; Malbouisson, A.; Malbouisson, J. Time evolution of entanglement in a cavity at finite temperature. Phys. A Stat. Mech. Its Appl. 2016, 462, 1261–1272. [Google Scholar] [CrossRef]
- Linowski, T.; Gneiting, C.; Rudnicki, L. Stabilizing entanglement in two-mode Gaussian states. Phys. Rev. A 2020, 102, 042405. [Google Scholar] [CrossRef]
- Vendromin, C.; Dignam, M.M. Continuous-variable entanglement in a two-mode lossy cavity: An analytic solution. Phys. Rev. A 2021, 103, 022418. [Google Scholar] [CrossRef]
- Adesso, G.; Illuminati, F. Entanglement in continuous-variable systems: Recent advances and current perspectives. J. Phys. A Math. Theor. 2007, 40, 7821. [Google Scholar] [CrossRef]
- Miroshnichenko, G.P.; Kiselev, A.D.; Trifanov, A.L.; Gleim, A.V. Algebraic approach to electro-optic modulation of light: Exactly solvable multimode quantum model. J. Opt. Soc. Am. B 2017, 34, 1177–1190. [Google Scholar] [CrossRef]
- Kozubov, A.; Gaidash, A.; Miroshnichenko, G. Quantum model of decoherence in the polarization domain for the fiber channel. Phys. Rev. A 2019, 99, 053842. [Google Scholar] [CrossRef]
- Gaidash, A.; Kozubov, A.; Miroshnichenko, G. Dissipative dynamics of quantum states in the fiber channel. Phys. Rev. A 2020, 102, 023711. [Google Scholar] [CrossRef]
- Mintert, F.; Carvalho, A.R.; Kuś, M.; Buchleitner, A. Measures and dynamics of entangled states. Phys. Rep. 2005, 415, 207–259. [Google Scholar] [CrossRef]
- Horodecki, R.; Horodecki, P.; Horodecki, M.; Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 2009, 81, 865. [Google Scholar] [CrossRef]
- Aolita, L.; de Melo, F.; Davidovich, L. Open-system dynamics of entanglement: A key issues review. Rep. Prog. Phys. 2015, 78, 042001. [Google Scholar] [CrossRef]
- Rajagopal, A.K.; Rendell, R.W. Decoherence, correlation, and entanglement in a pair of coupled quantum dissipative oscillators. Phys. Rev. A 2001, 63, 022116. [Google Scholar] [CrossRef]
- Życzkowski, K.; Horodecki, P.; Horodecki, M.; Horodecki, R. Dynamics of quantum entanglement. Phys. Rev. A 2001, 65, 012101. [Google Scholar] [CrossRef]
- Dodd, P.J.; Halliwell, J.J. Disentanglement and decoherence by open system dynamics. Phys. Rev. A 2004, 69, 052105. [Google Scholar] [CrossRef]
- Yu, T.; Eberly, J.H. Finite-time disentanglement via spontaneous emission. Phys. Rev. Lett. 2004, 93, 140404. [Google Scholar] [CrossRef]
- Yu, T.; Eberly, J.H. Quantum open system theory: Bipartite aspects. Phys. Rev. Lett. 2006, 97, 140403. [Google Scholar] [CrossRef] [PubMed]
- Yu, T.; Eberly, J.H. Sudden death of entanglement. Science 2009, 323, 598–601. [Google Scholar] [CrossRef] [PubMed]
- Passos, M.H.M.; Balthazar, W.F.; Khoury, A.Z.; Hor-Meyll, M.; Davidovich, L.; Huguenin, J.A.O. Experimental investigation of environment-induced entanglement using an all-optical setup. Phys. Rev. A 2018, 97, 022321. [Google Scholar] [CrossRef]
- Chakraborty, S.; Sarma, A.K. Delayed sudden death of entanglement at exceptional points. Phys. Rev. A 2019, 100, 063846. [Google Scholar] [CrossRef]
- Shearer, M.; Levy, R. Partial Differential Equations: An Introduction to Theory and Applications; Princeton University Press: Princeton, NJ, USA, 2015. [Google Scholar]
- Kozubov, A.V.; Gaidash, A.A.; Miroshnichenko, G.P.; Kiselev, A.D. Quantum dynamics of mixed polarization states: Effects of environment-mediated intermode coupling. J. Opt. Soc. Am. B 2021, 38, 2603–2611. [Google Scholar]
- Goldberg, A.Z.; e la Hoz, P.; Björk, G.; Klimov, A.B.; Grassl, M.; Leuchs, G.; Sánchez-Soto, L.L. Quantum concepts in optical polarization. Adv. Opt. Photon. 2021, 13, 1–73. [Google Scholar] [CrossRef]
- Serafini, A. Quantum Continuous Variables: A Primer of Theoretical Methods; CRC Press, Taylor & Francis Group: Boca Raton, FL, USA, 2017. [Google Scholar]
- Simon, R. Peres-Horodecki separability criterion for continuous variable systems. Phys. Rev. Lett. 2000, 84, 2726. [Google Scholar] [CrossRef] [PubMed]
- Duan, L.M.; Giedke, G.; Cirac, J.L.; Zoller, P. Inseparability criterion for continuous variable systems. Phys. Rev. Lett. 2000, 84, 2722. [Google Scholar] [CrossRef]
- Shchukin, E.; Vogel, W. Inseparability criteria for continuous bipartite quantum states. Phys. Rev. Lett. 2005, 95, 230502. [Google Scholar] [CrossRef]
- Gessner, M.; Pezzè, L.; Smerzi, A. Efficient entanglement criteria for discrete, continuous, and hybrid variables. Phys. Rev. A 2016, 94, 020101. [Google Scholar] [CrossRef]
- Bowen, W.P.; Schnabel, R.; Lam, P.K.; Ralph, T.C. Experimental characterization of continuous-variable entanglement. Phys. Rev. A 2004, 69, 012304. [Google Scholar] [CrossRef]
- Simon, D.S.; Jaeger, G.; Sergienko, A.V. Entangled-coherent-state quantum key distribution with entanglement witnessing. Phys. Rev. A 2014, 89, 012315. [Google Scholar] [CrossRef]
- Jaeger, G.; Simon, D.; Sergienko, A.V. Coherent state quantum key distribution based on entanglement sudden death. Quantum Inf. Process. 2016, 15, 1117–1133. [Google Scholar] [CrossRef]
- Adesso, G.; Illuminati, F. Gaussian measures of entanglement versus negativities: Ordering of two-mode Gaussian states. Phys. Rev. A 2005, 72, 032334. [Google Scholar] [CrossRef]
- Marian, P.; Marian, T.A. Bures distance as a measure of entanglement for symmetric two-mode Gaussian states. Phys. Rev. A 2008, 77, 062319. [Google Scholar] [CrossRef]
- Marian, P.; Marian, T.A. Entanglement of formation for an arbitrary two-mode Gaussian state. Phys. Rev. Lett. 2008, 101, 220403. [Google Scholar] [CrossRef]
- Vidal, G.; Werner, R.F. Computable measure of entanglement. Phys. Rev. A 2002, 65, 032314. [Google Scholar] [CrossRef]
- Hertz, A.; Cerf, N.J.; De Bièvre, S. Relating the entanglement and optical nonclassicality of multimode states of a bosonic quantum field. Phys. Rev. A 2020, 102, 032413. [Google Scholar] [CrossRef]
- Lvovsky, A.I.; Raymer, M.G. Continuous-variable optical quantum-state tomography. Rev. Mod. Phys. 2009, 81, 299. [Google Scholar] [CrossRef]
- Joshi, C.; Jonson, M.; Öhberg, P.; Andersson, E. Constructive role of dissipation for driven coupled bosonic modes. Phys. Rev. A 2013, 87, 062304. [Google Scholar] [CrossRef]
- Xu, J.S.; Sun, K.; Li, C.F.; Xu, X.Y.; Guo, G.C.; Andersson, E.; Lo Franco, R.; Compagno, G. Experimental recovery of quantum correlations in absence of system-environment back-action. Nat. Commun. 2013, 4, 2851. [Google Scholar] [CrossRef]
- Shen, L.T.; Shi, Z.C.; Wu, H.Z.; Yang, Z.B. Dynamics of entanglement in jaynes–cummings nodes with nonidentical qubit-field coupling strengths. Entropy 2017, 19, 331. [Google Scholar] [CrossRef]
- Shen, L.T.; Shi, Z.C.; Yang, Z.B. Coherent state control to recover quantum entanglement and coherence. Entropy 2019, 21, 917. [Google Scholar] [CrossRef]
- Bellomo, B.; Lo Franco, R.; Compagno, G. Long-time preservation of nonlocal entanglement. Adv. Sci. Lett. 2009, 2, 459–462. [Google Scholar] [CrossRef][Green Version]
- Mortezapour, A.; Lo Franco, R. Protecting quantum resources via frequency modulation of qubits in leaky cavities. Sci. Rep. 2018, 8, 4304. [Google Scholar] [CrossRef] [PubMed]
- Man, Z.Y.; Xia, Y.J.; Lo Franco, R. Cavity-based architecture to preserve quantum coherence and entanglement. Sci. Rep. 2015, 5, 13843. [Google Scholar] [CrossRef]
- Nosrati, F.; Mortezapour, A.; Lo Franco, R. Validating and controlling quantum enhancement against noise by the motion of a qubit. Phys. Rev. A 2020, 101, 012331. [Google Scholar] [CrossRef]
- Ghiu, I.; Grimaudo, R.; Mihaescu, T.; Isar, A.; Messina, A. Quantum correlation dynamics in controlled two-coupled-qubit systems. Entropy 2020, 22, 785. [Google Scholar] [CrossRef] [PubMed]
- Nosrati, F.; Castellini, A.; Compagno, G.; Lo Franco, R. Robust entanglement preparation against noise by controlling spatial indistinguishability. Npj Quantum Inf. 2020, 6, 39. [Google Scholar] [CrossRef]
- Nosrati, F.; Castellini, A.; Compagno, G.; Lo Franco, R. Dynamics of spatially indistinguishable particles and quantum entanglement protection. Phys. Rev. A 2020, 102, 062429. [Google Scholar] [CrossRef]
- Piccolini, M.; Nosrati, F.; Compagno, G.; Livreri, P.; Morandotti, R.; Lo Franco, R. Entanglement robustness via spatial deformation of identical particle wave functions. Entropy 2021, 23, 708. [Google Scholar] [CrossRef] [PubMed]
- Deng, X.; Liu, Y.; Wang, M.; Su, X.; Peng, K. Sudden death and revival of Gaussian Einstein–Podolsky–Rosen steering in noisy channels. Npj Quantum Inf. 2021, 7, 65. [Google Scholar] [CrossRef]
- Brody, D.C.; Graefe, E.M. Mixed-state evolution in the presence of gain and loss. Phys. Rev. Lett. 2012, 109, 230405. [Google Scholar] [CrossRef]
- Brody, D.C.; Gibbons, G.W.; Meier, D.M. Time-optimal navigation through quantum wind. New J. Phys. 2015, 17, 033048. [Google Scholar] [CrossRef]
- Brody, D.C.; Longstaff, B. Evolution speed of open quantum dynamics. Phys. Rev. Res. 2019, 1, 033127. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kiselev, A.D.; Ali, R.; Rybin, A.V. Lindblad Dynamics and Disentanglement in Multi-Mode Bosonic Systems. Entropy 2021, 23, 1409. https://doi.org/10.3390/e23111409
Kiselev AD, Ali R, Rybin AV. Lindblad Dynamics and Disentanglement in Multi-Mode Bosonic Systems. Entropy. 2021; 23(11):1409. https://doi.org/10.3390/e23111409
Chicago/Turabian StyleKiselev, Alexei D., Ranim Ali, and Andrei V. Rybin. 2021. "Lindblad Dynamics and Disentanglement in Multi-Mode Bosonic Systems" Entropy 23, no. 11: 1409. https://doi.org/10.3390/e23111409
APA StyleKiselev, A. D., Ali, R., & Rybin, A. V. (2021). Lindblad Dynamics and Disentanglement in Multi-Mode Bosonic Systems. Entropy, 23(11), 1409. https://doi.org/10.3390/e23111409





