Phase-Coherent Dynamics of Quantum Devices with Local Interactions
Abstract
1. Introduction
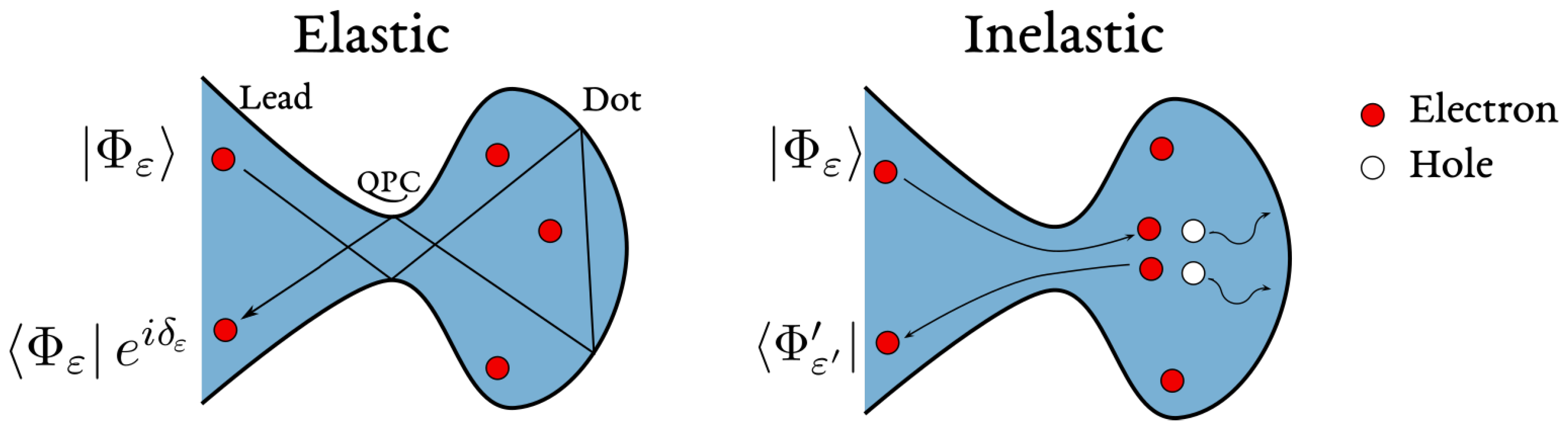
2. Phase-Coherence in Quantum Devices with Local Interactions
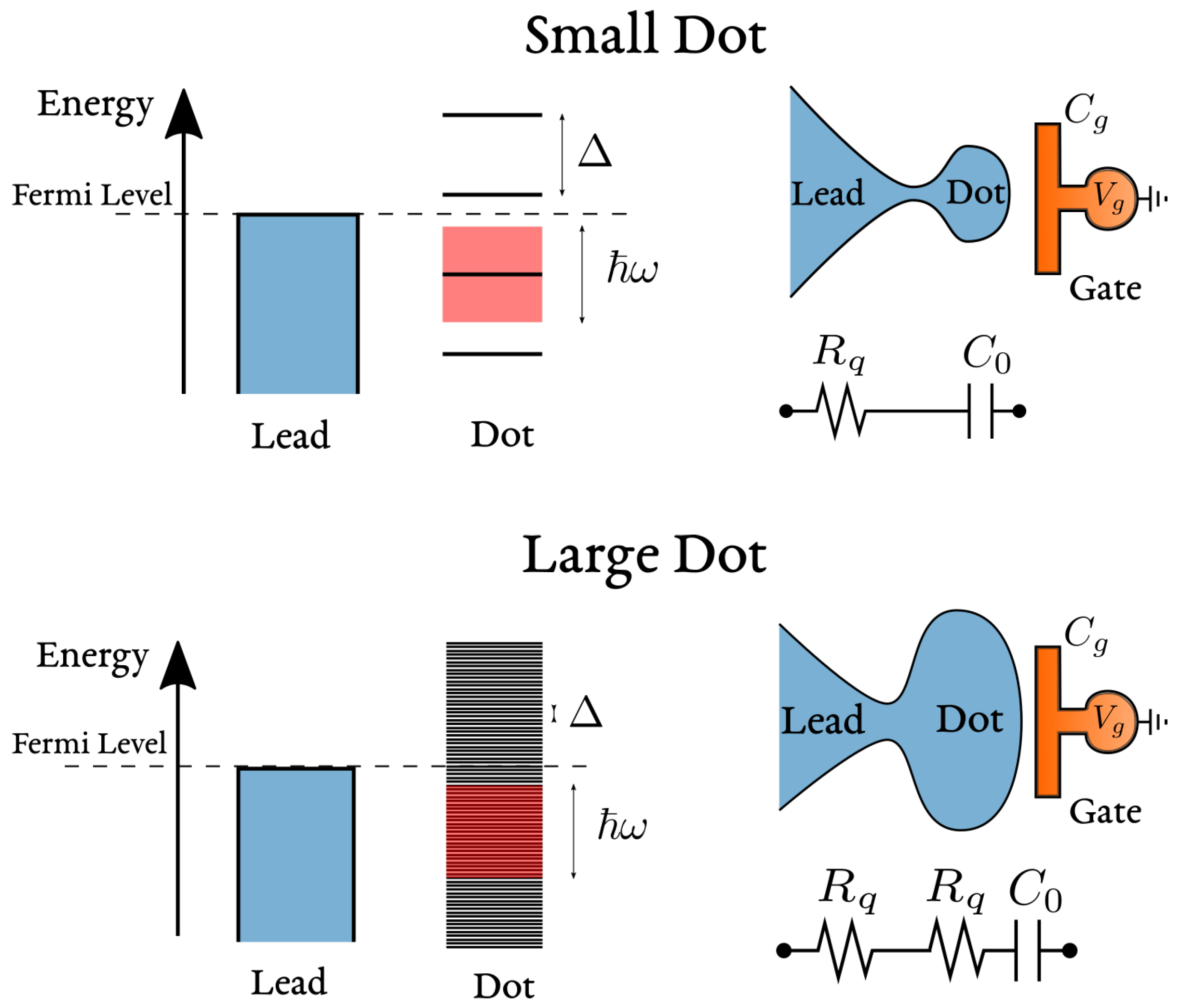
3. What Are Local Fermi Liquids and Why Are They Important to Understand Quantum-Dot Devices?
3.1. The Local Fermi Liquid
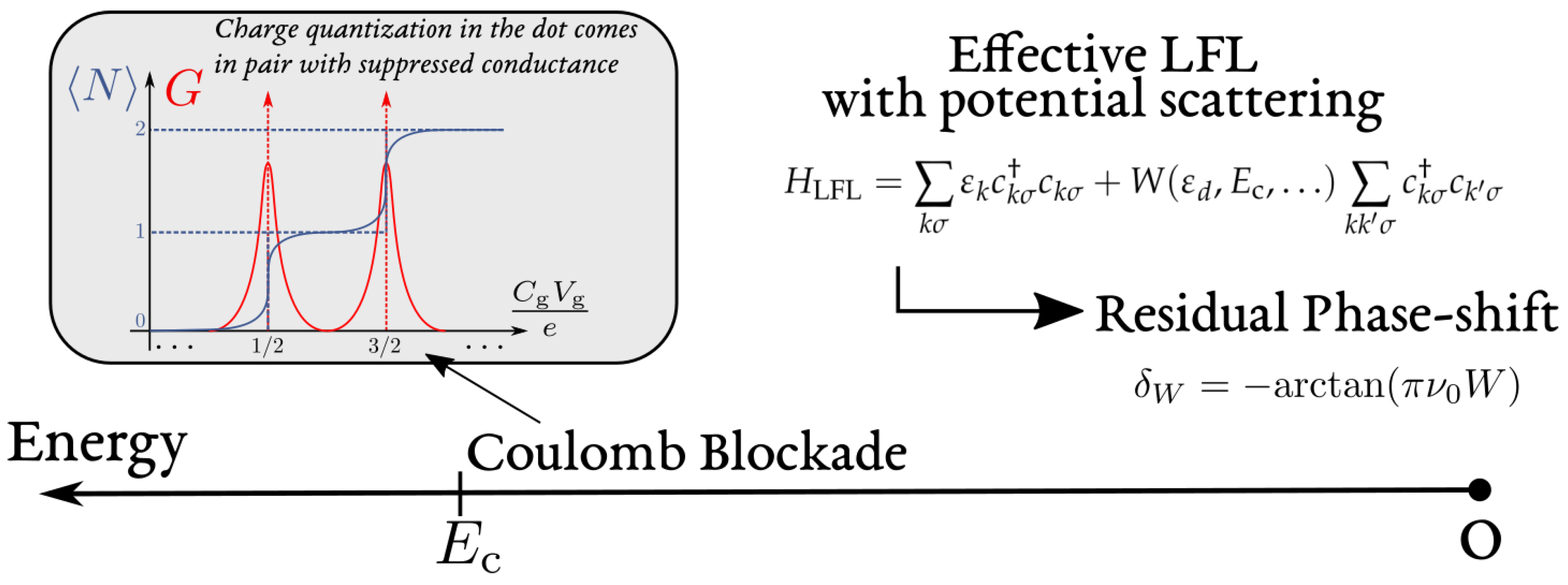
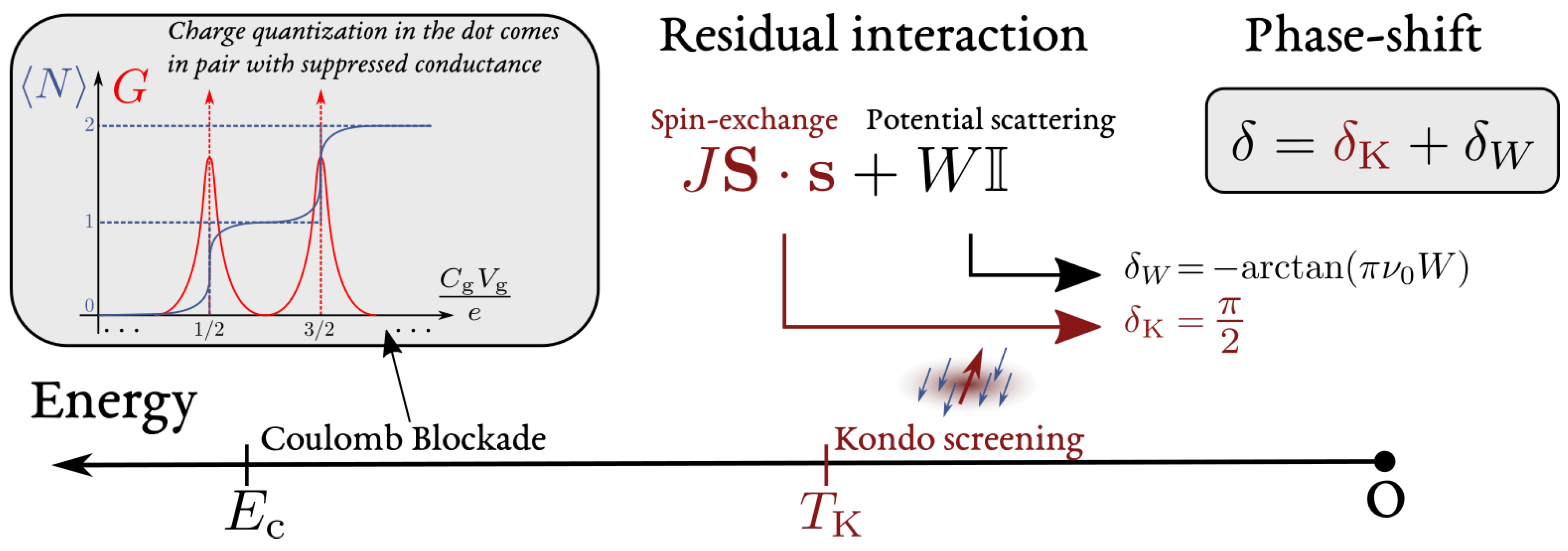
3.2. The Role of the Friedel Sum Rule in Local Fermi Liquid Theories
3.3. Derivation of the LFL Theory in the Coulomb Blockade and Anderson Model
3.3.1. Coulomb Blockade Model
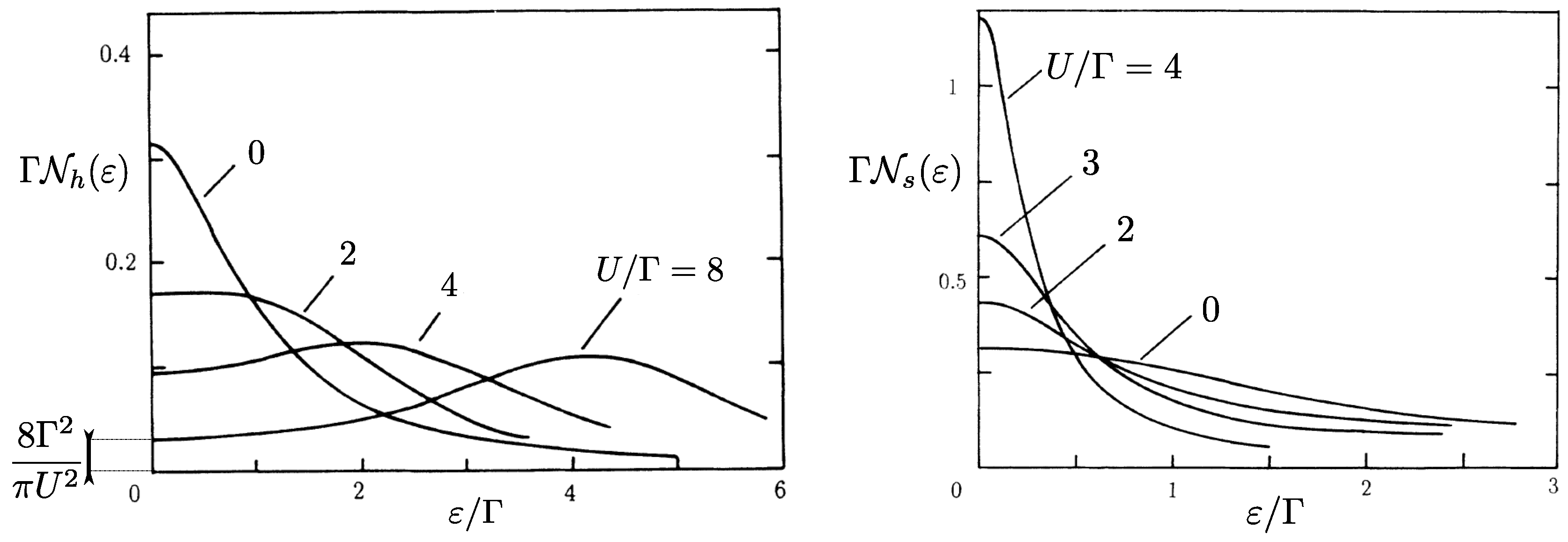
3.3.2. Anderson Impurity Model
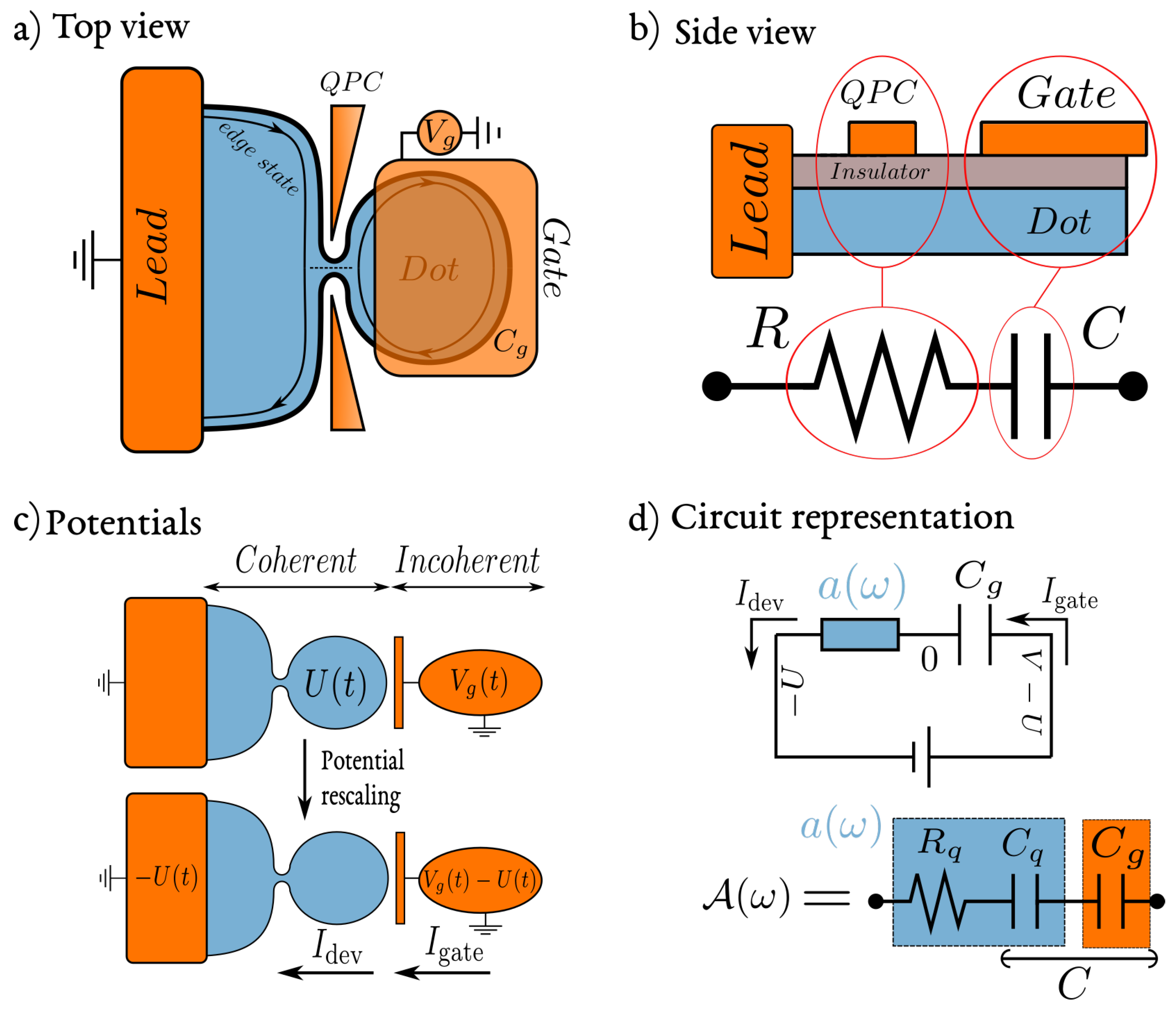
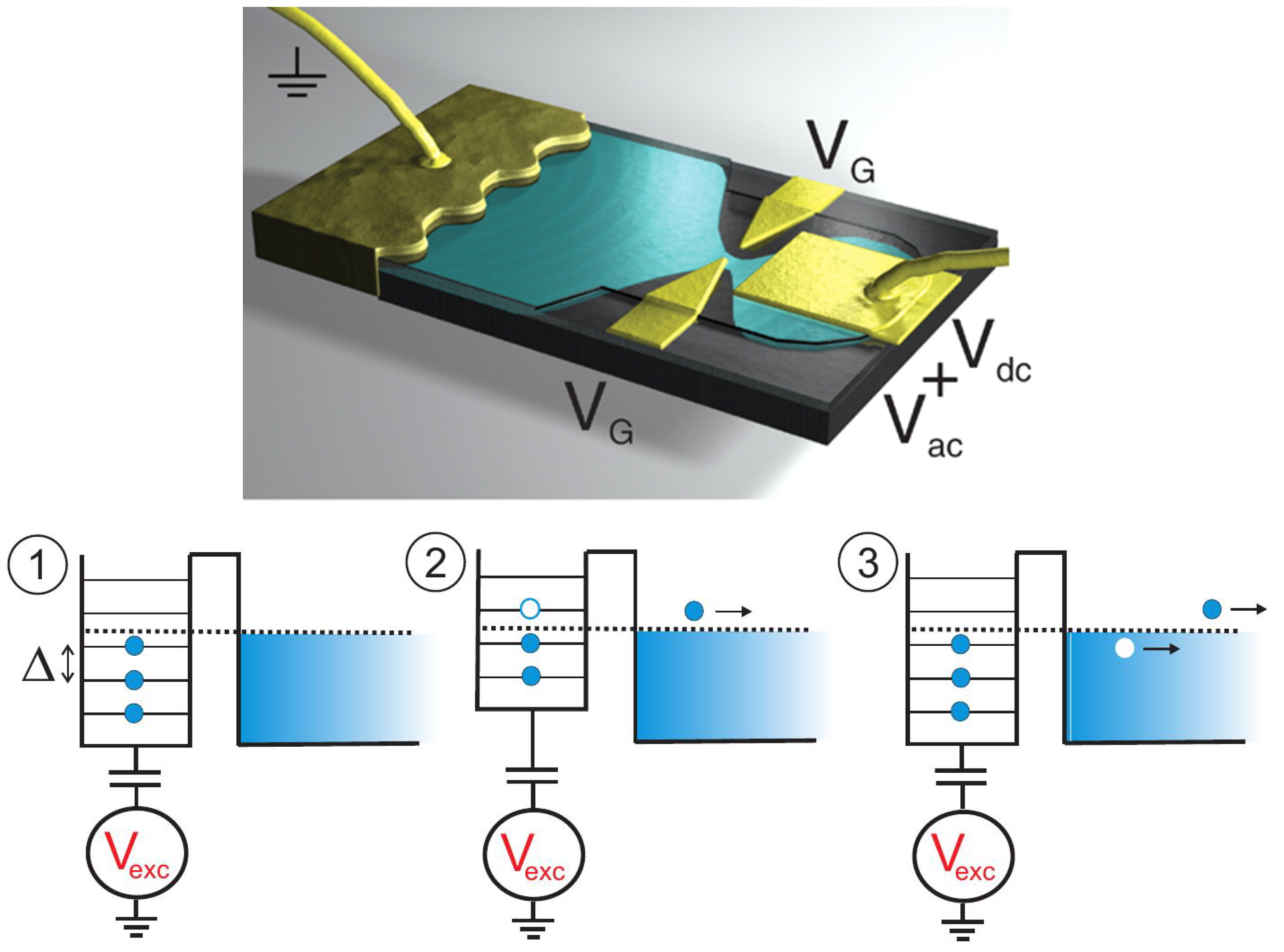
4. The Mesoscopic Capacitor
- The total capacitance C is given by the static charge susceptibility of the cavity and does not generally correspond to a series of a geometric and quantum contribution, proportional to the density of states in the cavity. For instance, in Kondo regimes, the charge susceptibility of the cavity remains small, because of frozen charge fluctuations, while the density of states increases below the Kondo temperature [49]. This effect was directly probed in a recent experiment with a quantum dot device embedded in circuit-QED architecture [145];
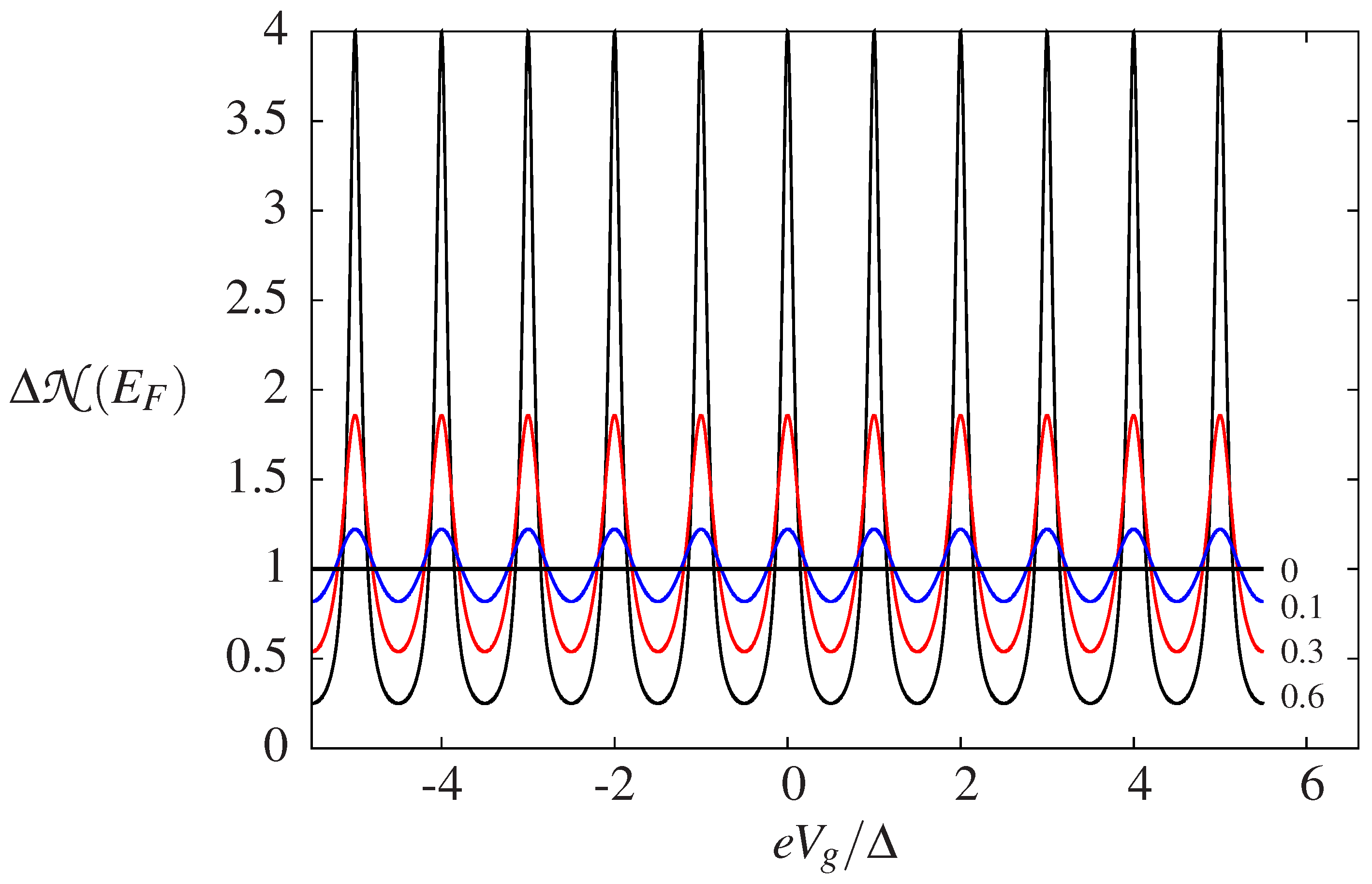
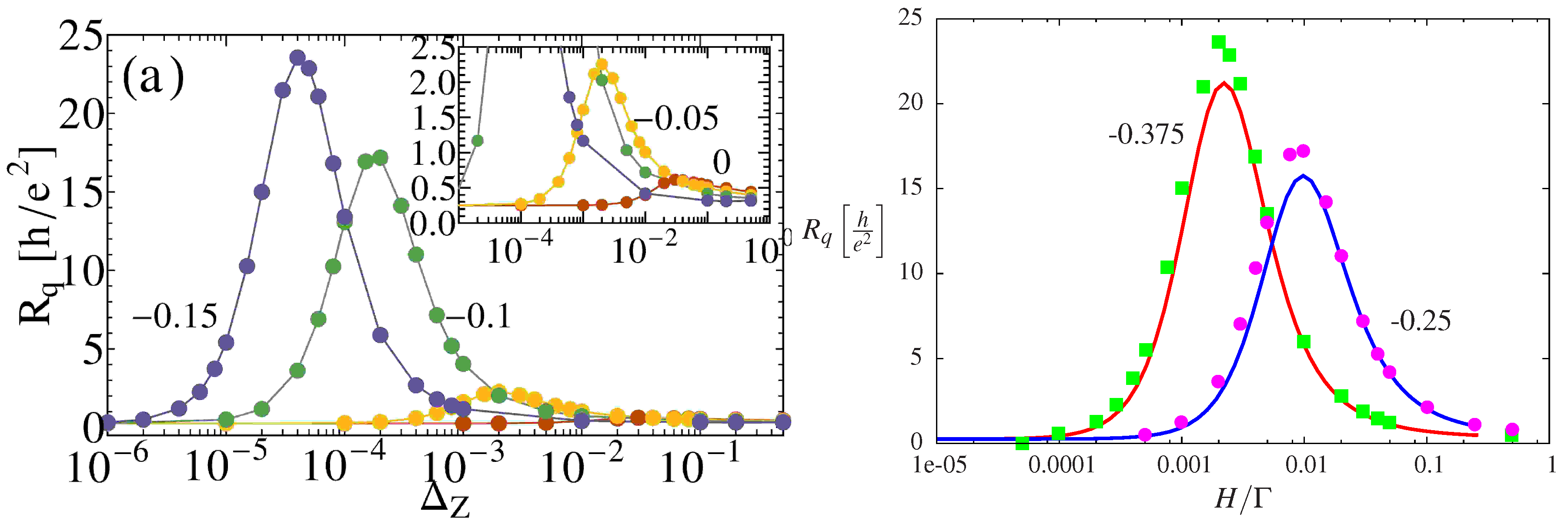
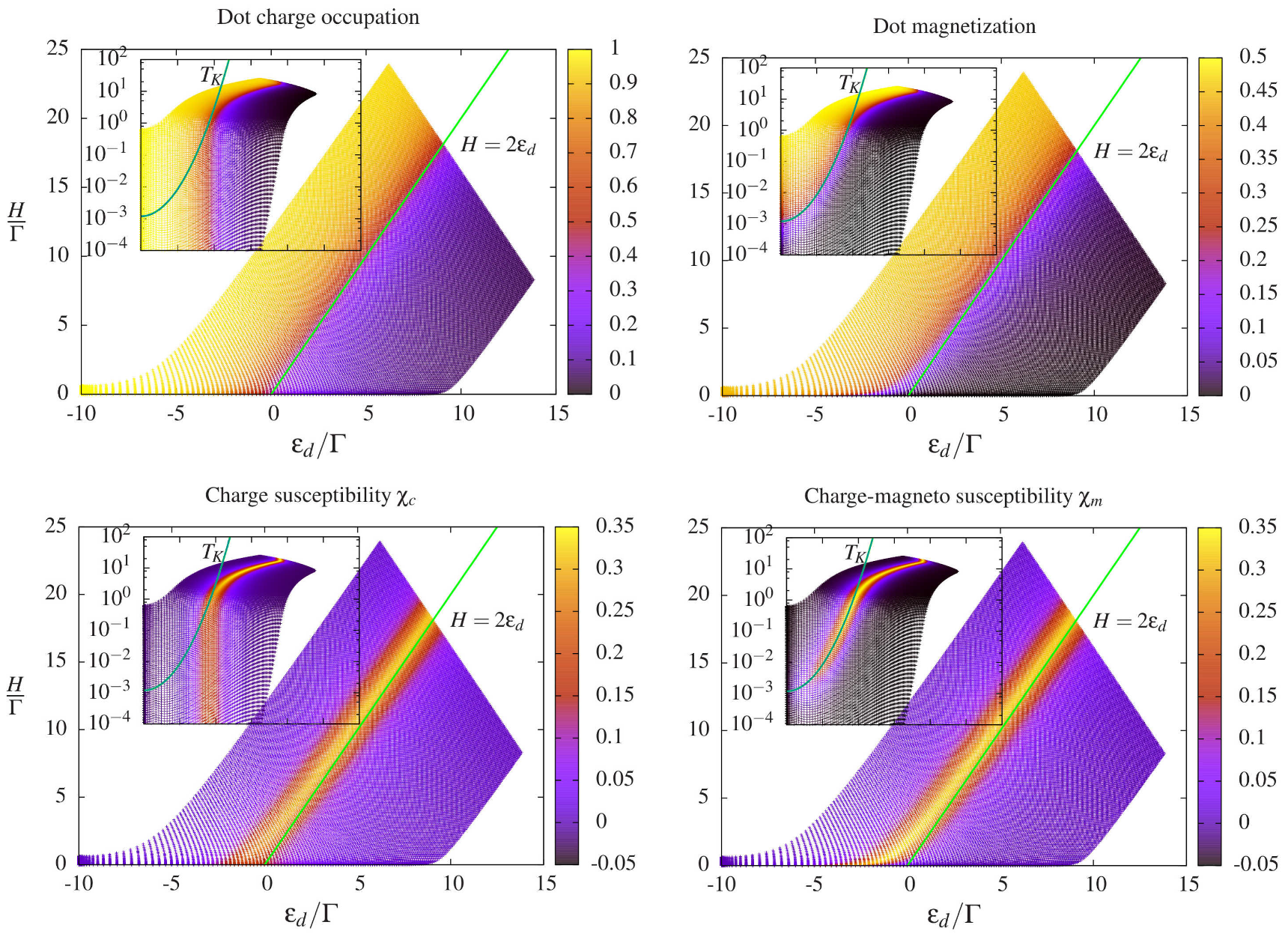
- The LFL approach shows various non-trivial dissipative effects triggered by strong correlations. In particular, it predicts a mesoscopic crossover between two universal regimes in which [65] by increasing the dot size, also at charge degeneracy, in which the CBM maps on the Kondo model [101]. It also predicts giant dissipative regimes, described by giant universal peaks in , triggered by the destruction of the Kondo singlet by a magnetic field [67,147];
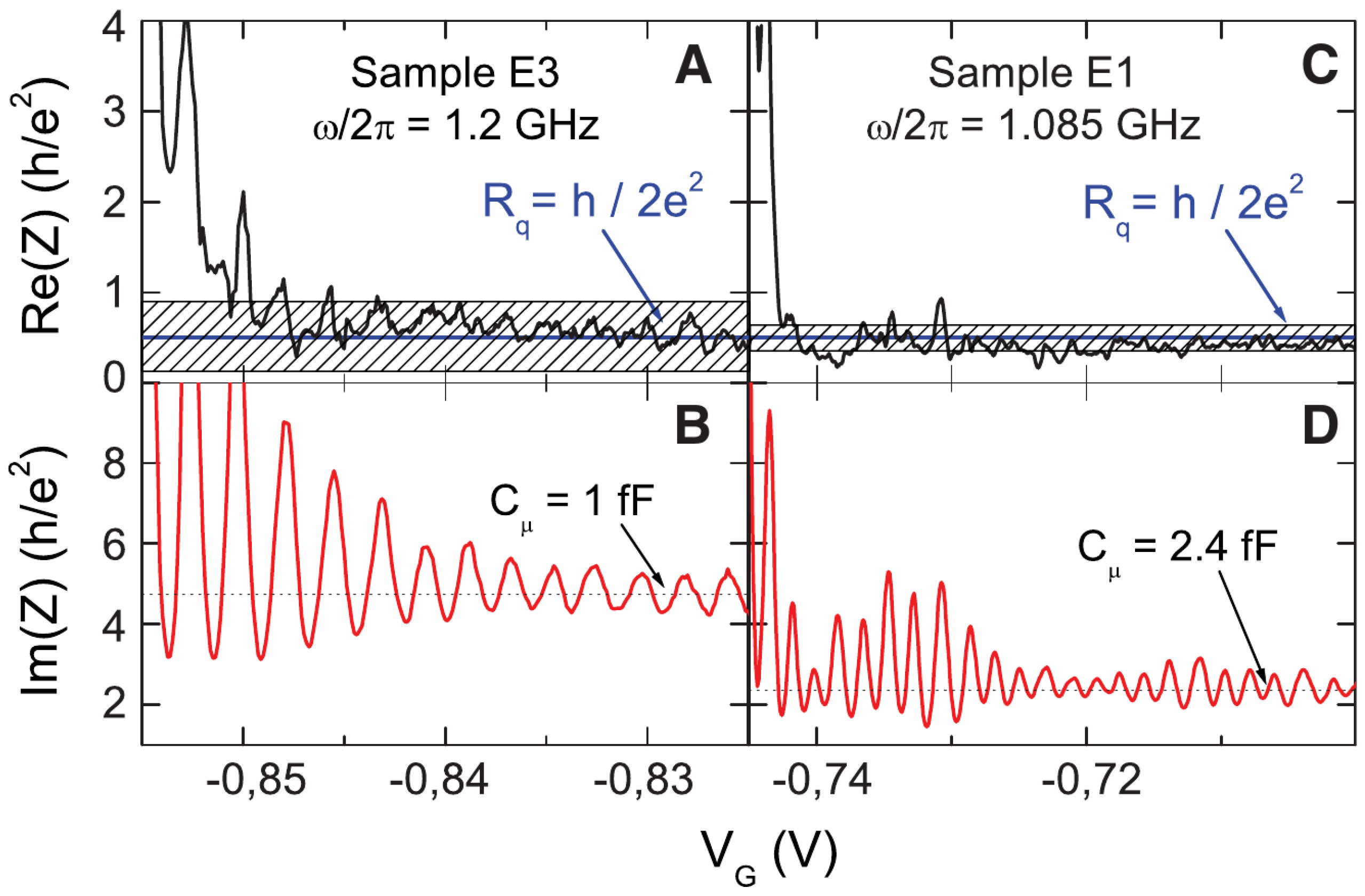
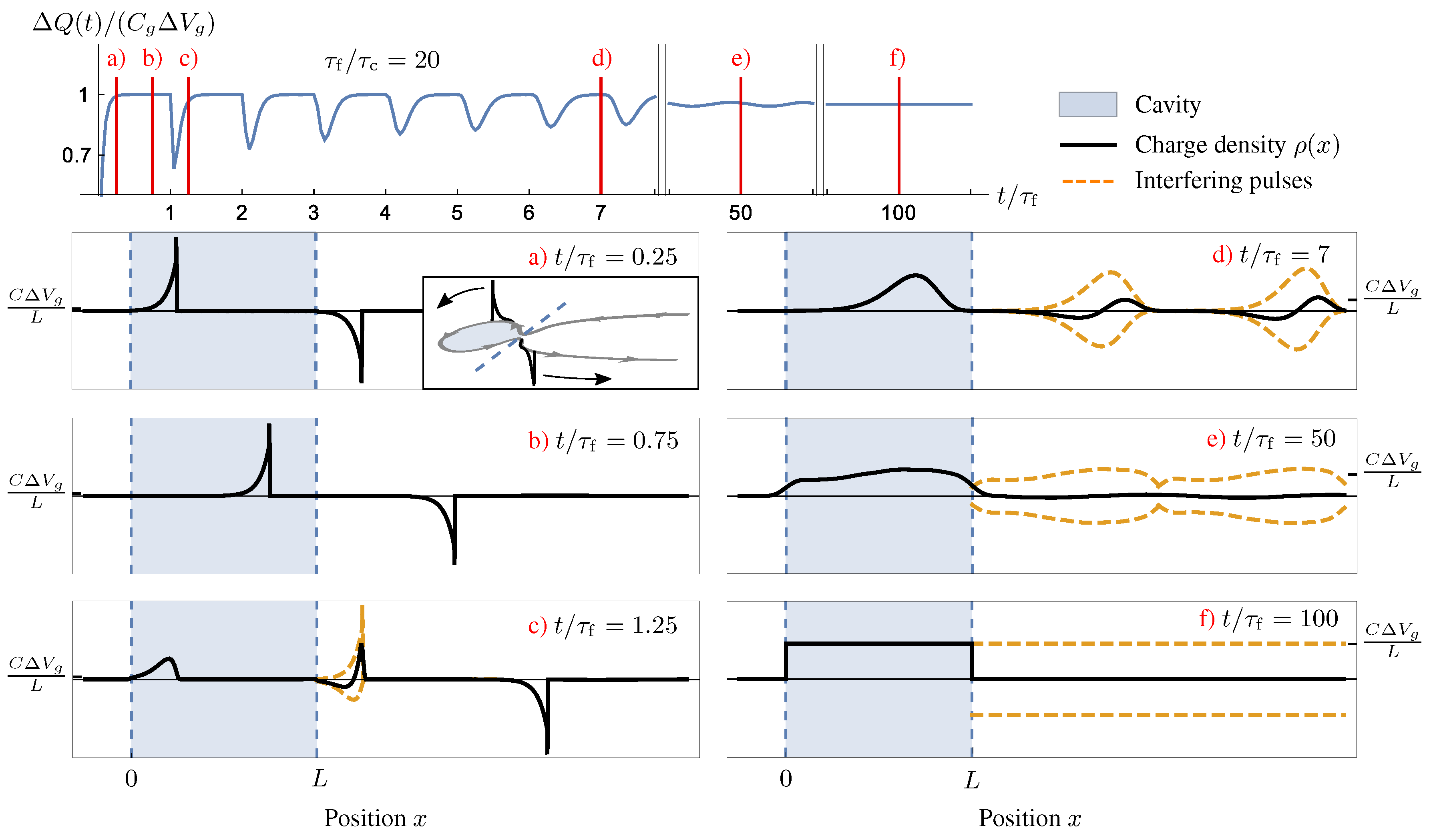
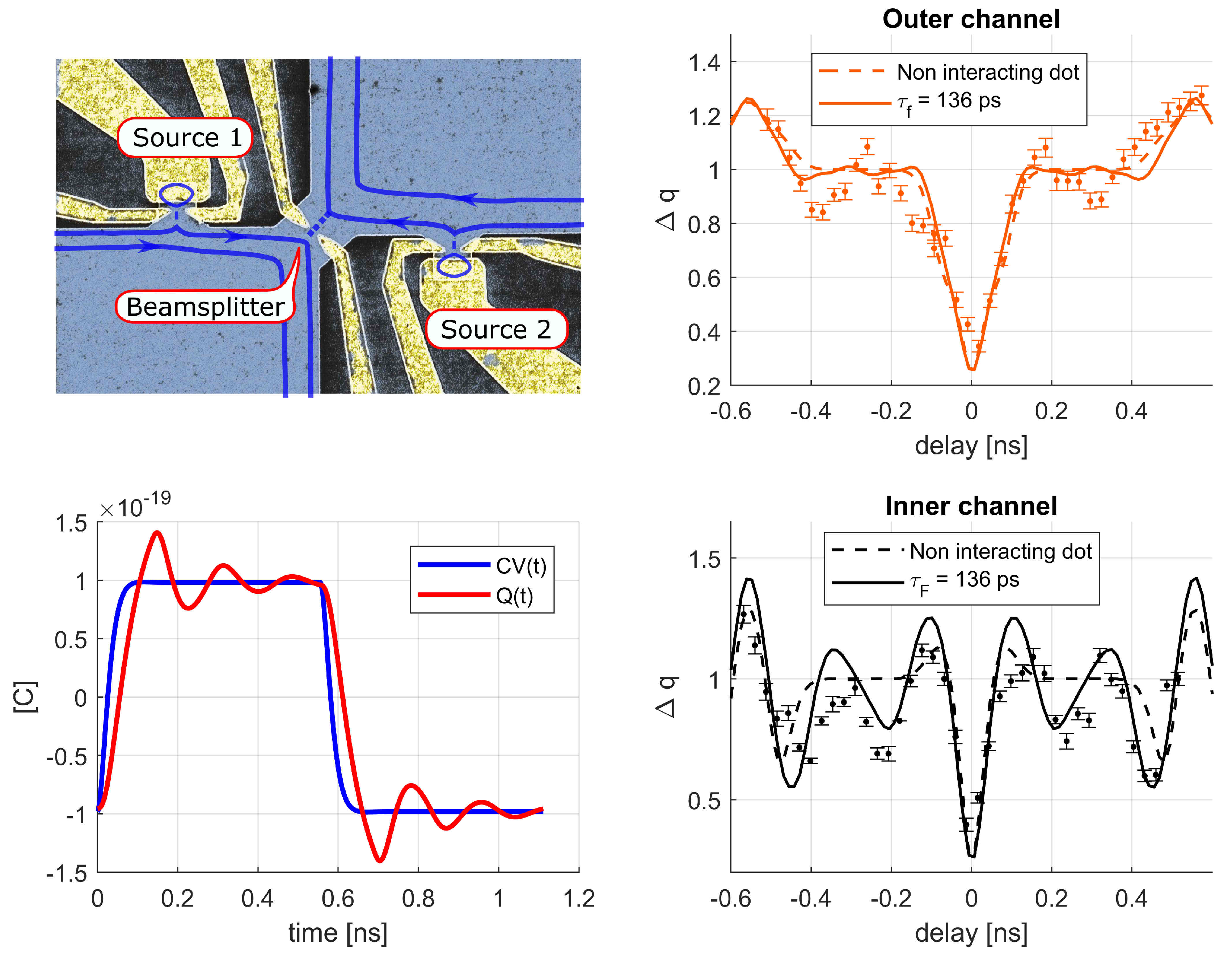
- In proper out-of-equilibrium regimes, interactions and inelastic effects become unavoidable and circuit analogies, such as Equation (31), do not capture the dynamic behavior of the mesoscopic capacitor [148]. We show here how previously published data [25] also show a previously overlooked signature of non-trivial many-body dynamics induced by interactions.
4.1. Hamiltonian Description of the Quantum RC Circuit: Differential Capacitance and Korringa–Shiba Relation
4.2. The Origin of the Differential Capacitance as a ‘Quantum’ Capacitance as far as Interactions are Neglected
4.3. The Physical Origin of the Universal Charge Relaxation Resistance
4.4. The Open-Dot Limit
4.5. The Tunneling Limit and the Quasi-Static Approximation
4.6. The LFL Theory of Large Quantum Dots: The Mesoscopic Crossover
4.7. The Multi-Channel Case and Universal Effects Triggered by Kondo Correlations
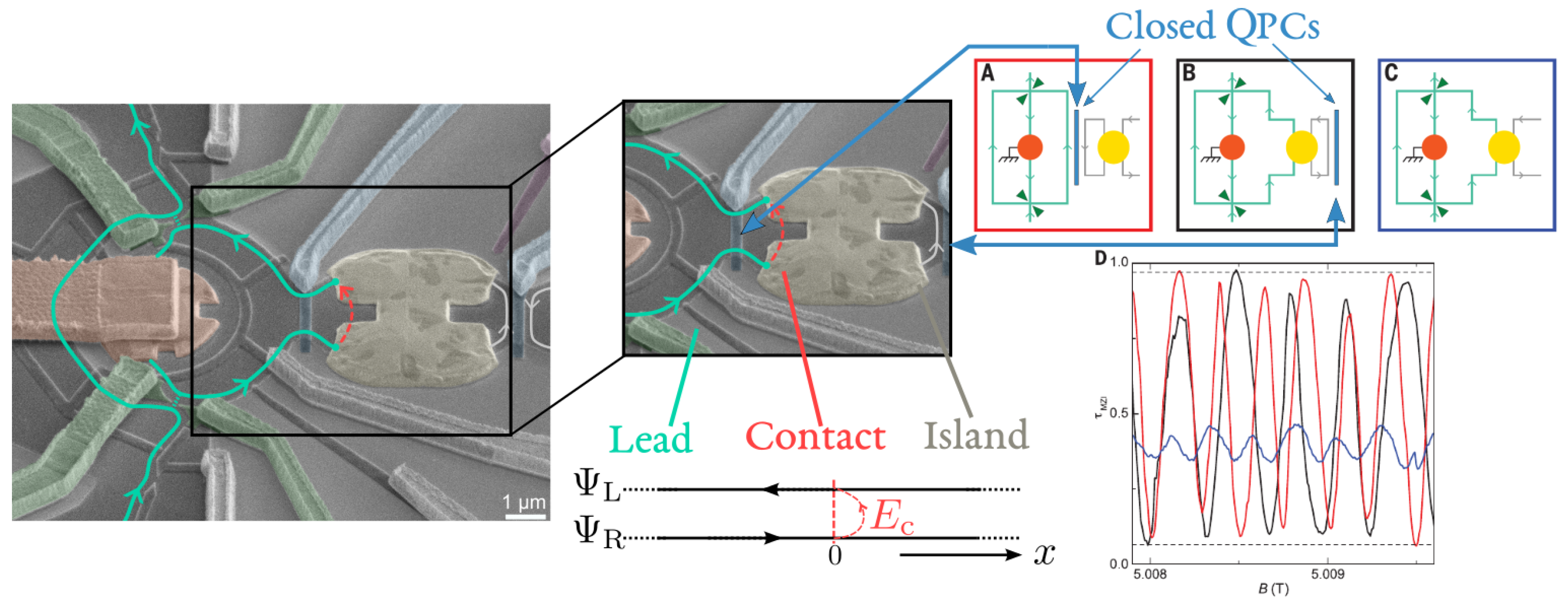
5. What about Out-Of-Equilibrium Regimes? A New Twist on Experiments
Experimental Signatures of the Effects of Interaction in Quantum Cavities Driven out of Equilibrium
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| QPC | quantum point contact |
| LFL | local Fermi liquid |
| CBM | Coulomb blockade model |
| AIM | Anderson impurity model |
| SW | Schrieffer–Wolff |
| DC | direct current |
| 2DEG | two-dimensional electron gas |
| KS | Korringa–Shiba |
| AC | alternate current |
| NRG | numerical renormalization group |
Appendix A. Scattering Theory, Phase-Shifts and the Friedel Sum Rule
Appendix A.1. General Definitions
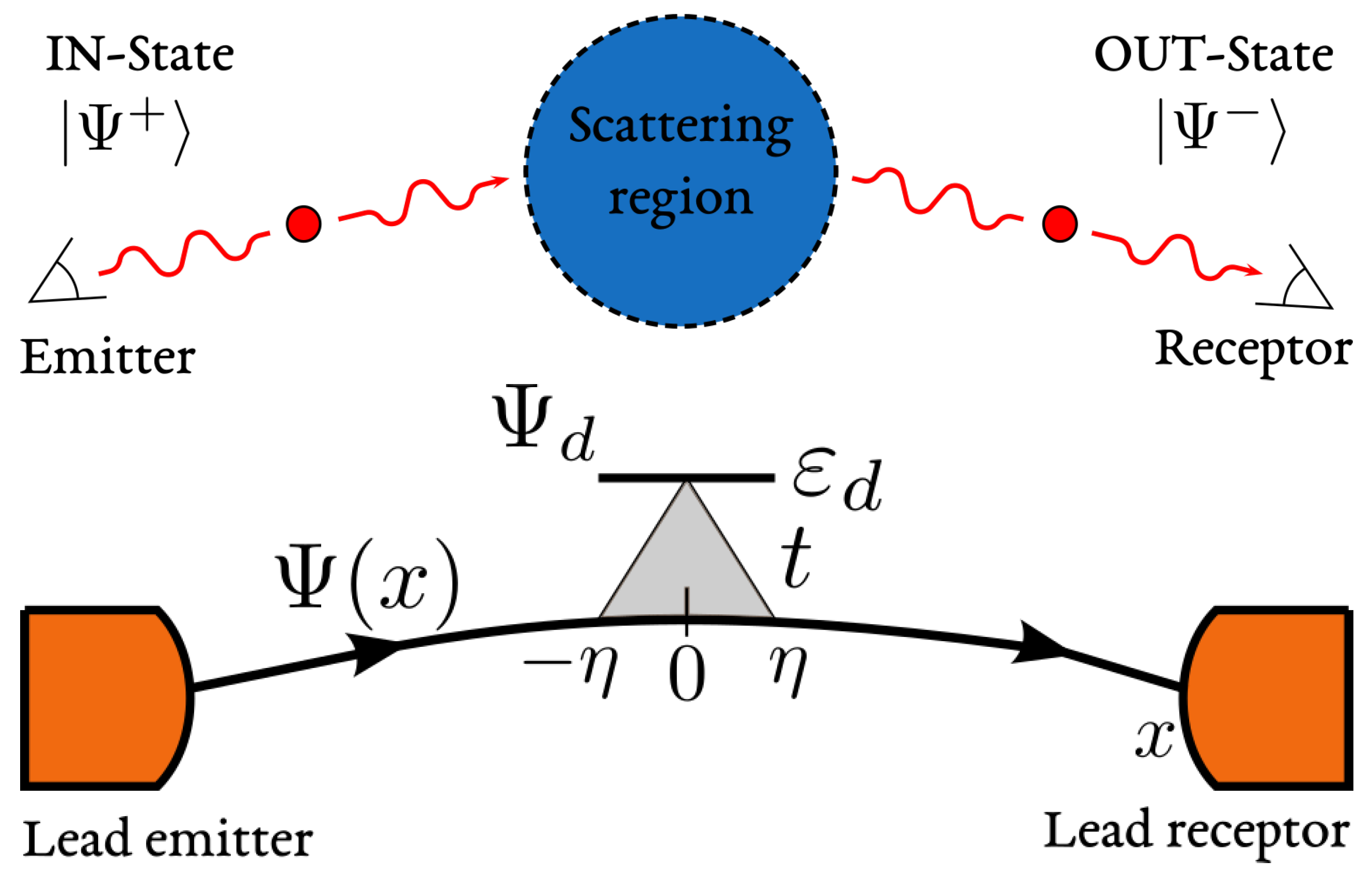
Appendix A.2. The Friedel Sum Rule
Appendix A.3. Illustration on the Resonant Level Model
Appendix A.4. T-Matrix in the Potential Scattering Hamiltonian
Appendix B. Self-Consistent Description- of a 2DEG Quantum RC Circuit
Appendix B.1. Self-Consistent Theory
Appendix B.2. Hamiltonian Description of the Quantum RC Circuit with a Resonant Level Model
Multi-Level Case
Appendix C. Useful Results of Linear Response Theory
Appendix C.1. Parity of the Dynamical Charge Susceptibility
Appendix C.2. Energy Dissipation in the Linear Response Regime
References
- Averin, D.V.; Likharev, K.K. Coulomb blockade of single-electron tunneling, and coherent oscillations in small tunnel junctions. J. Low Temp. Phys. 1986, 62, 345–373. [Google Scholar] [CrossRef]
- Grabert, H.; Devoret, M.; Kastner, M. Single Charge Tunneling: Coulomb Blockade Phenomena in Nanostructures. Phys. Today 1993, 46, 62. [Google Scholar] [CrossRef]
- Aleiner, I.; Brouwer, P.; Glazman, L. Quantum effects in Coulomb blockade. Phys. Rep. 2002, 358, 309–440. [Google Scholar] [CrossRef]
- Loss, D.; DiVincenzo, D.P. Quantum computation with quantum dots. Phys. Rev. A 1998, 57, 120–126. [Google Scholar] [CrossRef]
- Vandersypen, L.M.K.; Bluhm, H.; Clarke, J.S.; Dzurak, A.S.; Ishihara, R.; Morello, A.; Reilly, D.J.; Schreiber, L.R.; Veldhorst, M. Interfacing spin qubits in quantum dots and donors—hot, dense, and coherent. NPJ Quantum Inf. 2017, 3, 1–10. [Google Scholar] [CrossRef]
- Vinet, M.; Hutin, L.; Bertrand, B.; Barraud, S.; Hartmann, J.M.; Kim, Y.J.; Mazzocchi, V.; Amisse, A.; Bohuslavskyi, H.; Bourdet, L.; et al. Towards scalable silicon quantum computing. In Proceedings of the 2018 IEEE International Electron Devices Meeting (IEDM), San Francisco, CA, USA, 1–5 December 2018; pp. 6–1918. [Google Scholar] [CrossRef]
- Xiang, Z.L.; Ashhab, S.; You, J.Q.; Nori, F. Hybrid quantum circuits: Superconducting circuits interacting with other quantum systems. Rev. Mod. Phys. 2013, 85, 623–653. [Google Scholar] [CrossRef]
- Levitov, L.S.; Lee, H.; Lesovik, G.B. Electron counting statistics and coherent states of electric current. J. Math. Phys. 1996, 37, 4845–4866. [Google Scholar] [CrossRef]
- Ivanov, D.A.; Lee, H.W.; Levitov, L.S. Coherent states of alternating current. Phys. Rev. B 1997, 56, 6839–6850. [Google Scholar] [CrossRef]
- Keeling, J.; Klich, I.; Levitov, L.S. Minimal Excitation States of Electrons in One-Dimensional Wires. Phys. Rev. Lett. 2006, 97, 116403. [Google Scholar] [CrossRef]
- Dubois, J.; Jullien, T.; Portier, F.; Roche, P.; Cavanna, A.; Jin, Y.; Wegscheider, W.; Roulleau, P.; Glattli, D. Minimal-excitation states for electron quantum optics using levitons. Nature 2013, 502, 659–663. [Google Scholar] [CrossRef]
- Jullien, T.; Roulleau, P.; Roche, B.; Cavanna, A.; Jin, Y.; Glattli, D. Quantum tomography of an electron. Nature 2014, 514, 603–607. [Google Scholar] [CrossRef] [PubMed]
- Rech, J.; Ferraro, D.; Jonckheere, T.; Vannucci, L.; Sassetti, M.; Martin, T. Minimal Excitations in the Fractional Quantum Hall Regime. Phys. Rev. Lett. 2017, 118, 076801. [Google Scholar] [CrossRef] [PubMed]
- Hermelin, S.; Takada, S.; Yamamoto, M.; Tarucha, S.; Wieck, A.D.; Saminadayar, L.; Bäuerle, C.; Meunier, T. Electrons surfing on a sound wave as a platform for quantum optics with flying electrons. Nature 2011, 477, 435–438. [Google Scholar] [CrossRef] [PubMed]
- Bertrand, B.; Hermelin, S.; Takada, S.; Yamamoto, M.; Tarucha, S.; Ludwig, A.; Wieck, A.D.; Bäuerle, C.; Meunier, T. Fast spin information transfer between distant quantum dots using individual electrons. Nat. Nanotechnol. 2016, 11, 672–676. [Google Scholar] [CrossRef] [PubMed]
- Bertrand, B.; Hermelin, S.; Mortemousque, P.A.; Takada, S.; Yamamoto, M.; Tarucha, S.; Ludwig, A.; Wieck, A.D.; Bäuerle, C.; Meunier, T. Injection of a single electron from static to moving quantum dots. Nanotechnology 2016, 27, 214001. [Google Scholar] [CrossRef] [PubMed]
- Takada, S.; Edlbauer, H.; Lepage, H.V.; Wang, J.; Mortemousque, P.A.; Georgiou, G.; Barnes, C.H.W.; Ford, C.J.B.; Yuan, M.; Santos, P.V.; et al. Sound-driven single-electron transfer in a circuit of coupled quantum rails. Nat. Commun. 2019, 10, 4557. [Google Scholar] [CrossRef]
- Van Zanten, D.M.T.; Basko, D.M.; Khaymovich, I.M.; Pekola, J.P.; Courtois, H.; Winkelmann, C.B. Single Quantum Level Electron Turnstile. Phys. Rev. Lett. 2016, 116, 166801. [Google Scholar] [CrossRef]
- Basko, D.M. Landau-Zener-Stueckelberg Physics with a Singular Continuum of States. Phys. Rev. Lett. 2017, 118, 016805. [Google Scholar] [CrossRef]
- Gabelli, J.; Fève, G.; Berroir, J.M.; Plaçais, B.; Cavanna, A.; Etienne, B.; Jin, Y.; Glattli, D.C. Violation of Kirchhoff’s Laws for a Coherent RC Circuit. Science 2006, 313, 499–502. [Google Scholar] [CrossRef]
- Gabelli, J.; Fève, G.; Berroir, J.M.; Plaçais, B. A coherentRCcircuit. Rep. Prog. Phys. 2012, 75, 126504. [Google Scholar] [CrossRef]
- Fève, G.; Mahé, A.; Berroir, J.M.; Kontos, T.; Plaçais, B.; Glattli, D.C.; Cavanna, A.; Etienne, B.; Jin, Y. An On-Demand Coherent Single-Electron Source. Science 2007, 316, 1169–1172. [Google Scholar] [CrossRef] [PubMed]
- Mahé, A.; Parmentier, F.D.; Bocquillon, E.; Berroir, J.M.; Glattli, D.C.; Kontos, T.; Plaçais, B.; Fève, G.; Cavanna, A.; Jin, Y. Current correlations of an on-demand single-electron emitter. Phys. Rev. B 2010, 82, 201309. [Google Scholar] [CrossRef]
- Parmentier, F.D.; Bocquillon, E.; Berroir, J.M.; Glattli, D.C.; Plaçais, B.; Fève, G.; Albert, M.; Flindt, C.; Büttiker, M. Current noise spectrum of a single-particle emitter: Theory and experiment. Phys. Rev. B 2012, 85, 165438. [Google Scholar] [CrossRef]
- Freulon, V.; Marguerite, A.; Berroir, J.M.; Plaçais, B.; Cavanna, A.; Jin, Y.; Fève, G. Hong-Ou-Mandel experiment for temporal investigation of single-electron fractionalization. Nat. Commun. 2015, 6, 1–6. [Google Scholar] [CrossRef] [PubMed]
- Marguerite, A.; Cabart, C.; Wahl, C.; Roussel, B.; Freulon, V.; Ferraro, D.; Grenier, C.; Berroir, J.M.; Plaçais, B.; Jonckheere, T.; et al. Decoherence and relaxation of a single electron in a one-dimensional conductor. Phys. Rev. B 2016, 94, 115311. [Google Scholar] [CrossRef]
- Leicht, C.; Mirovsky, P.; Kaestner, B.; Hohls, F.; Kashcheyevs, V.; Kurganova, E.V.; Zeitler, U.; Weimann, T.; Pierz, K.; Schumacher, H.W. Generation of energy selective excitations in quantum Hall edge states. Semicond. Sci. Technol. 2011, 26, 055010. [Google Scholar] [CrossRef]
- Battista, F.; Samuelsson, P. Spectral distribution and wave function of electrons emitted from a single-particle source in the quantum Hall regime. Phys. Rev. B 2012, 85, 075428. [Google Scholar]
- Fletcher, J.D.; See, P.; Howe, H.; Pepper, M.; Giblin, S.P.; Griffiths, J.P.; Jones, G.A.C.; Farrer, I.; Ritchie, D.A.; Janssen, T.J.B.M.; et al. Clock-Controlled Emission of Single-Electron Wave Packets in a Solid-State Circuit. Phys. Rev. Lett. 2013, 111, 216807. [Google Scholar] [CrossRef]
- Waldie, J.; See, P.; Kashcheyevs, V.; Griffiths, J.P.; Farrer, I.; Jones, G.A.C.; Ritchie, D.A.; Janssen, T.J.B.M.; Kataoka, M. Measurement and control of electron wave packets from a single-electron source. Phys. Rev. B 2015, 92, 125305. [Google Scholar] [CrossRef]
- Kataoka, M.; Johnson, N.; Emary, C.; See, P.; Griffiths, J.P.; Jones, G.A.C.; Farrer, I.; Ritchie, D.A.; Pepper, M.; Janssen, T.J.B.M. Time-of-Flight Measurements of Single-Electron Wave Packets in Quantum Hall Edge States. Phys. Rev. Lett. 2016, 116, 126803. [Google Scholar] [CrossRef]
- Johnson, N.; Fletcher, J.; Humphreys, D.; See, P.; Griffiths, J.; Jones, G.; Farrer, I.; Ritchie, D.; Pepper, M.; Janssen, T.; et al. Ultrafast voltage sampling using single-electron wavepackets. Appl. Phys. Lett. 2017, 110, 102105. [Google Scholar] [CrossRef]
- Roussely, G.; Arrighi, E.; Georgiou, G.; Takada, S.; Schalk, M.; Urdampilleta, M.; Ludwig, A.; Wieck, A.D.; Armagnat, P.; Kloss, T.; et al. Unveiling the bosonic nature of an ultrashort few-electron pulse. Nat. Commun. 2018, 9, 2811. [Google Scholar] [CrossRef] [PubMed]
- Schoelkopf, R.; Wahlgren, P.; Kozhevnikov, A.; Delsing, P.; Prober, D. The radio-frequency single-electron transistor (RF-SET): A fast and ultrasensitive electrometer. Science 1998, 280, 1238–1242. [Google Scholar] [CrossRef] [PubMed]
- Bäuerle, C.; Glattli, D.C.; Meunier, T.; Portier, F.; Roche, P.; Roulleau, P.; Takada, S.; Waintal, X. Coherent control of single electrons: A review of current progress. Rep. Prog. Phys. 2018, 81, 056503. [Google Scholar] [CrossRef]
- Akkermans, E.; Montambaux, G. Mesoscopic Physics of Electrons and Photons; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Altshuler, B.L.; Aronov, A.G.; Khmelnitsky, D.E. Effects of electron-electron collisions with small energy transfers on quantum localisation. J. Phys. C Solid State Phys. 1982, 15, 7367. [Google Scholar] [CrossRef]
- Pierre, F.; Gougam, A.B.; Anthore, A.; Pothier, H.; Esteve, D.; Birge, N.O. Dephasing of electrons in mesoscopic metal wires. Phys. Rev. B 2003, 68, 085413. [Google Scholar] [CrossRef]
- Huard, B.; Anthore, A.; Birge, N.O.; Pothier, H.; Esteve, D. Effect of Magnetic Impurities on Energy Exchange between Electrons. Phys. Rev. Lett. 2005, 95, 036802. [Google Scholar] [CrossRef]
- Mallet, F.; Ericsson, J.; Mailly, D.; Ünlübayir, S.; Reuter, D.; Melnikov, A.; Wieck, A.D.; Micklitz, T.; Rosch, A.; Costi, T.A.; et al. Scaling of the Low-Temperature Dephasing Rate in Kondo Systems. Phys. Rev. Lett. 2006, 97, 226804. [Google Scholar] [CrossRef]
- Saminadayar, L.; Mohanty, P.; Webb, R.A.; Degiovanni, P.; Bäuerle, C. Electron coherence at low temperatures: The role of magnetic impurities. Phys. E Low-Dimens. Syst. Nanostruct. 2007, 40, 12–24. [Google Scholar] [CrossRef]
- Niimi, Y.; Baines, Y.; Capron, T.; Mailly, D.; Lo, F.Y.; Wieck, A.D.; Meunier, T.; Saminadayar, L.; Bäuerle, C. Quantum coherence at low temperatures in mesoscopic systems: Effect of disorder. Phys. Rev. B 2010, 81, 245306. [Google Scholar] [CrossRef]
- Pines, D.; Nozières, P. Theory Of Quantum Liquids|Normal Fermi Liquids; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Coleman, P. Introduction to Many-Body Physics; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Landauer, R. Electrical resistance of disordered one-dimensional lattices. Philos. Mag. 1970, 21, 863–867. [Google Scholar] [CrossRef]
- Landauer, R. Spatial variation of currents and fields due to localized scatterers in metallic conduction. IBM J. Res. Dev. 1988, 32, 306–316. [Google Scholar] [CrossRef]
- Büttiker, M.; Imry, Y.; Landauer, R.; Pinhas, S. Generalized many-channel conductance formula with application to small rings. Phys. Rev. B 1985, 31, 6207–6215. [Google Scholar] [CrossRef] [PubMed]
- Nozières, P. A “fermi-liquid” description of the Kondo problem at low temperatures. J. Low Temp. Phys. 1974, 17, 31–42. [Google Scholar] [CrossRef]
- Hewson, A.C. The Kondo Problem to Heavy Fermions; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Duprez, H.; Sivre, E.; Anthore, A.; Aassime, A.; Cavanna, A.; Gennser, U.; Pierre, F. Transmitting the quantum state of electrons across a metallic island with Coulomb interaction. Science 2019, 366, 1243–1247. [Google Scholar] [CrossRef] [PubMed]
- Büttiker, M.; Prêtre, A.; Thomas, H. Dynamic conductance and the scattering matrix of small conductors. Phys. Rev. Lett. 1993, 70, 4114–4117. [Google Scholar] [CrossRef] [PubMed]
- Büttiker, M.; Thomas, H.; Prêtre, A. Mesoscopic capacitors. Phys. Lett. A 1993, 180, 364–369. [Google Scholar] [CrossRef]
- Prêtre, A.; Thomas, H.; Büttiker, M. Dynamic admittance of mesoscopic conductors: Discrete-potential model. Phys. Rev. B 1996, 54, 8130–8143. [Google Scholar] [CrossRef]
- Nigg, S.E.; López, R.; Büttiker, M. Mesoscopic Charge Relaxation. Phys. Rev. Lett. 2006, 97, 206804. [Google Scholar] [CrossRef]
- Büttiker, M.; Nigg, S.E. Mesoscopic capacitance oscillations. Nanotechnology 2006, 18, 044029. [Google Scholar] [CrossRef]
- Büttiker, M.; Nigg, S.E. Role of coherence in resistance quantization. Eur. Phys. J. Spec. Top. 2009, 172, 247–255. [Google Scholar] [CrossRef]
- Mora, C.; Vitushinsky, P.; Leyronas, X.; Clerk, A.A.; Le Hur, K. Theory of nonequilibrium transport in the SU(N) Kondo regime. Phys. Rev. B 2009, 80, 155322. [Google Scholar] [CrossRef]
- Mora, C. Fermi-liquid theory for SU(N) Kondo model. Phys. Rev. B 2009, 80, 125304. [Google Scholar] [CrossRef]
- Mora, C.; Moca, C.P.; von Delft, J.; Zaránd, G. Fermi-liquid theory for the single-impurity Anderson model. Phys. Rev. B 2015, 92, 075120. [Google Scholar] [CrossRef]
- Oguri, A.; Hewson, A.C. Higher-Order Fermi-Liquid Corrections for an Anderson Impurity Away from Half Filling. Phys. Rev. Lett. 2018, 120, 126802. [Google Scholar] [CrossRef]
- Oguri, A.; Hewson, A.C. Higher-order Fermi-liquid corrections for an Anderson impurity away from half filling: Equilibrium properties. Phys. Rev. B 2018, 97, 045406. [Google Scholar] [CrossRef]
- Oguri, A.; Hewson, A.C. Higher-order Fermi-liquid corrections for an Anderson impurity away from half filling: Nonequilibrium transport. Phys. Rev. B 2018, 97, 035435. [Google Scholar] [CrossRef]
- Filippone, M.; Moca, C.P.; Weichselbaum, A.; von Delft, J.; Mora, C. At which magnetic field, exactly, does the Kondo resonance begin to split? A Fermi liquid description of the low-energy properties of the Anderson model. Phys. Rev. B 2018, 98, 075404. [Google Scholar] [CrossRef]
- Teratani, Y.; Sakano, R.; Oguri, A. Fermi liquid theory for nonlinear transport through a multilevel Anderson impurity. arXiv 2020, arXiv:2001.08348. [Google Scholar]
- Mora, C.; Le Hur, K. Universal resistances of the quantum resistance-capacitance circuit. Nat. Phys. 2010, 6, 697–701. [Google Scholar] [CrossRef]
- Filippone, M.; Mora, C. Fermi liquid approach to the quantum RC circuit: Renormalization group analysis of the Anderson and Coulomb blockade models. Phys. Rev. B 2012, 86, 125311. [Google Scholar] [CrossRef]
- Filippone, M.; Le Hur, K.; Mora, C. Giant Charge Relaxation Resistance in the Anderson Model. Phys. Rev. Lett. 2011, 107, 176601. [Google Scholar] [CrossRef] [PubMed]
- Filippone, M.; Le Hur, K.; Mora, C. Admittance of the SU(2) and SU(4) Anderson quantum RC circuits. Phys. Rev. B 2013, 88, 045302. [Google Scholar] [CrossRef]
- Dutt, P.; Schmidt, T.L.; Mora, C.; Le Hur, K. Strongly correlated dynamics in multichannel quantum RC circuits. Phys. Rev. B 2013, 87, 155134. [Google Scholar] [CrossRef]
- Clerk, A.A.; Brouwer, P.W.; Ambegaokar, V. Interaction-Induced Restoration of Phase Coherence. Phys. Rev. Lett. 2001, 87, 186801. [Google Scholar] [CrossRef]
- Idrisov, E.G.; Levkivskyi, I.P.; Sukhorukov, E.V. Dephasing in a Mach-Zehnder Interferometer by an Ohmic Contact. Phys. Rev. Lett. 2018, 121, 026802. [Google Scholar] [CrossRef]
- Matveev, K.A. Coulomb blockade at almost perfect transmission. Phys. Rev. B 1995, 51, 1743–1751. [Google Scholar] [CrossRef]
- Haldane, F.D.M. ‘Luttinger liquid theory’ of one-dimensional quantum fluids. I. Properties of the Luttinger model and their extension to the general 1D interacting spinless Fermi gas. J. Phys. C Solid State Phys. 1981, 14, 2585. [Google Scholar] [CrossRef]
- Haldane, F.D.M. Effective Harmonic-Fluid Approach to Low-Energy Properties of One-Dimensional Quantum Fluids. Phys. Rev. Lett. 1981, 47, 1840–1843. [Google Scholar] [CrossRef]
- Delft, J.v.; Schoeller, H. Bosonization for beginners—Refermionization for experts. Ann. Der Phys. 1998, 7, 225–305. [Google Scholar] [CrossRef][Green Version]
- Giamarchi, T. Quantum Physics in One Dimension; Oxford University Press: New York, NY, USA, 2004; Volume 121. [Google Scholar]
- Aleiner, I.L.; Glazman, L.I. Mesoscopic charge quantization. Phys. Rev. B 1998, 57, 9608–9641. [Google Scholar] [CrossRef]
- Brouwer, P.W.; Lamacraft, A.; Flensberg, K. Nonequilibrium theory of Coulomb blockade in open quantum dots. Phys. Rev. B 2005, 72, 075316. [Google Scholar] [CrossRef]
- Altland, A.; Simons, B. Condensed Matter Field Theory; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Clerk, A.A. Aspects of Andreev Scattering and Kondo Physics in Mesoscopic Systems. Ph.D. Thesis, Cornell University, Ithaca, NY, USA, 2011. [Google Scholar]
- Schuster, R.; Buks, E.; Heiblum, M.; Mahalu, D.; Umansky, V.; Shtrikman, H. Phase measurement in a quantum dot via a double-slit interference experiment. Nature 1997, 385, 417–420. [Google Scholar] [CrossRef]
- Edlbauer, H.; Takada, S.; Roussely, G.; Yamamoto, M.; Tarucha, S.; Ludwig, A.; Wieck, A.D.; Meunier, T.; Bäuerle, C. Non-universal transmission phase behaviour of a large quantum dot. Nat. Commun. 2017, 8, 1710. [Google Scholar] [CrossRef] [PubMed]
- Wick, G.C. The Evaluation of the Collision Matrix. Phys. Rev. 1950, 80, 268–272. [Google Scholar] [CrossRef]
- Büttiker, M. Quantized transmission of a saddle-point constriction. Phys. Rev. B 1990, 41, 7906–7909. [Google Scholar] [CrossRef]
- Mora, C.; Le Hur, K. Probing dynamics of Majorana fermions in quantum impurity systems. Phys. Rev. B 2013, 88, 241302. [Google Scholar] [CrossRef]
- Friedel, J. On some electrical and magnetic properties of metallic solid solutions. Can. J. Phys. 1956, 34, 1190–1211. [Google Scholar] [CrossRef]
- Langreth, D.C. Friedel Sum Rule for Anderson’s Model of Localized Impurity States. Phys. Rev. 1966, 150, 516–518. [Google Scholar] [CrossRef]
- Rontani, M. Friedel Sum Rule for an Interacting Multiorbital Quantum Dot. Phys. Rev. Lett. 2006, 97, 076801. [Google Scholar] [CrossRef]
- Ringel, Z.; Imry, Y.; Entin-Wohlman, O. Delayed currents and interaction effects in mesoscopic capacitors. Phys. Rev. B 2008, 78, 165304. [Google Scholar] [CrossRef]
- Brouwer, P.W. Scattering approach to parametric pumping. Phys. Rev. B 1998, 58, R10135–R10138. [Google Scholar] [CrossRef]
- Karki, D.B.; Mora, C.; von Delft, J.; Kiselev, M.N. Two-color Fermi-liquid theory for transport through a multilevel Kondo impurity. Phys. Rev. B 2018, 97, 195403. [Google Scholar] [CrossRef]
- Anderson, P.W. Localized Magnetic States in Metals. Phys. Rev. 1961, 124, 41–53. [Google Scholar] [CrossRef]
- Pustilnik, M.; Glazman, L. Kondo effect in quantum dots. J. Phys. Condens. Matter 2004, 16, R513–R537. [Google Scholar] [CrossRef]
- Schrieffer, J.R.; Wolff, P.A. Relation between the Anderson and Kondo Hamiltonians. Phys. Rev. 1966, 149, 491–492. [Google Scholar] [CrossRef]
- Goldhaber-Gordon, D.; Shtrikman, H.; Mahalu, D.; Abusch-Magder, D.; Meirav, U.; Kastner, M.A. Kondo effect in a single-electron transistor. Nature 1998, 391, 156. [Google Scholar] [CrossRef]
- Bruhat, L.E.; Viennot, J.J.; Dartiailh, M.C.; Desjardins, M.M.; Cottet, A.; Kontos, T. Scaling laws of the Kondo problem at finite frequency. Phys. Rev. B 2018, 98, 075121. [Google Scholar] [CrossRef]
- Bruus, H.; Flensberg, K. Many-Body Quantum Theory in Condensed Matter Physics. An Introduction; Oxford University Press: New York, NY, USA, 2004. [Google Scholar]
- Coqblin, B.; Schrieffer, J.R. Exchange Interaction in Alloys with Cerium Impurities. Phys. Rev. 1969, 185, 847–853. [Google Scholar] [CrossRef]
- Grabert, H. Charge fluctuations in the single-electron box: Perturbation expansion in the tunneling conductance. Phys. Rev. B 1994, 50, 17364–17377. [Google Scholar] [CrossRef]
- Grabert, H. Rounding of the Coulomb Staircase by the tunneling conductance. Phys. B Condens. Matter 1994, 194, 1011–1012. [Google Scholar] [CrossRef]
- Matveev, K.A. Quantum fluctuations of the charge of a metal particle under the Coulomb blockade conditions. Zh. Eksp. Teor. Fiz. 1990, 99, 1598. [Google Scholar]
- Tsvelick, A.; Wiegmann, P. Exact results in the theory of magnetic alloys. Adv. Phys. 1983, 32, 453–713. [Google Scholar] [CrossRef]
- Wiegmann, P.B.; Tsvelick, A.M. Exact solution of the Anderson model: I. J. Phys. C Solid State Phys. 1983, 16, 2281–2319. [Google Scholar] [CrossRef]
- Krishna-murthy, H.R.; Wilkins, J.W.; Wilson, K.G. Renormalization-group approach to the Anderson model of dilute magnetic alloys. I. Static properties for the symmetric case. Phys. Rev. B 1980, 21, 1003–1043. [Google Scholar] [CrossRef]
- Krishna-murthy, H.R.; Wilkins, J.W.; Wilson, K.G. Renormalization-group approach to the Anderson model of dilute magnetic alloys. II. Static properties for the asymmetric case. Phys. Rev. B 1980, 21, 1044–1083. [Google Scholar] [CrossRef]
- Affleck, I. Conformal Field Theory Approach to the Kondo Effect. arXiv 1995, arXiv:Cond-mat/9512099. [Google Scholar]
- Cardy, J.L. Boundary conditions, fusion rules and the Verlinde formula. Nucl. Phys. B 1989, 324, 581–596. [Google Scholar] [CrossRef]
- Affleck, I.; Ludwig, A.W.W. Critical theory of overscreened Kondo fixed points. Nucl. Phys. B 1991, 360, 641–696. [Google Scholar] [CrossRef]
- Affleck, I.; Ludwig, A.W.W. Exact conformal-field-theory results on the multichannel Kondo effect: Single-fermion Green’s function, self-energy, and resistivity. Phys. Rev. B 1993, 48, 7297–7321. [Google Scholar] [CrossRef]
- Lesage, F.; Saleur, H. Perturbation of infra-red fixed points and duality in quantum impurity problems. Nucl. Phys. B 1999, 546, 585–620. [Google Scholar] [CrossRef]
- Lesage, F.; Saleur, H. Strong-Coupling Resistivity in the Kondo Model. Phys. Rev. Lett. 1999, 82, 4540–4543. [Google Scholar] [CrossRef]
- Haldane, F.D.M. Theory of the atomic limit of the Anderson model. I. Perturbation expansions re-examined. J. Phys. C 1978, 11, 5015. [Google Scholar] [CrossRef]
- Haldane, F.D.M. Scaling Theory of the Asymmetric Anderson Model. Phys. Rev. Lett. 1978, 40, 416–419. [Google Scholar] [CrossRef]
- Sørensen, E.S.; Affleck, I. Scaling theory of the Kondo screening cloud. Phys. Rev. B 1996, 53, 9153–9167. [Google Scholar] [CrossRef]
- Barzykin, V.; Affleck, I. The Kondo Screening Cloud: What Can We Learn from Perturbation Theory? Phys. Rev. Lett. 1996, 76, 4959–4962. [Google Scholar] [CrossRef]
- Affleck, I.; Simon, P. Detecting the Kondo Screening Cloud Around a Quantum Dot. Phys. Rev. Lett. 2001, 86, 2854–2857. [Google Scholar] [CrossRef]
- Affleck, I.; Borda, L.; Saleur, H. Friedel oscillations and the Kondo screening cloud. Phys. Rev. B 2008, 77, 180404. [Google Scholar] [CrossRef]
- Suhl, H. Dispersion Theory of the Kondo Effect. Phys. Rev. 1965, 138, A515–A523. [Google Scholar] [CrossRef]
- Glazman, L.; Raikh, M. Resonant Kondo transparency of a barrier with quasilocal impurity states. JETP Lett. 1988, 47, 105. [Google Scholar]
- Goldhaber-Gordon, D.; Göres, J.; Kastner, M.A.; Shtrikman, H.; Mahalu, D.; Meirav, U. From the Kondo Regime to the Mixed-Valence Regime in a Single-Electron Transistor. Phys. Rev. Lett. 1998, 81, 5225–5228. [Google Scholar] [CrossRef]
- Takada, S.; Bäuerle, C.; Yamamoto, M.; Watanabe, K.; Hermelin, S.; Meunier, T.; Alex, A.; Weichselbaum, A.; von Delft, J.; Ludwig, A.; et al. Transmission Phase in the Kondo Regime Revealed in a Two-Path Interferometer. Phys. Rev. Lett. 2014, 113, 126601. [Google Scholar] [CrossRef] [PubMed]
- Borzenets, I.V.; Shim, J.; Chen, J.C.H.; Ludwig, A.; Wieck, A.D.; Tarucha, S.; Sim, H.S.; Yamamoto, M. Observation of the Kondo screening cloud. Nature 2020, 579, 210–213. [Google Scholar] [CrossRef] [PubMed]
- Cragg, D.; Lloyd, P. Potential Scattering and the Kondo Problem. J. Phys. C 1978, 11, L597. [Google Scholar] [CrossRef]
- Lloyd, P.; Cragg, D.M. The Nozieres-Wilson relation in the low-temperature Kondo problem. J. Phys. C 1979, 12, 3289. [Google Scholar] [CrossRef]
- Cragg, D.M.; Lloyd, P. Universality and the renormalisability of rotationally invariant Kondo Hamiltonians. J. Phys. C 1979, 12, 3301. [Google Scholar] [CrossRef]
- Horvatié, B.; Zlatié, V. Equivalence of the perturbative and Bethe-Ansatz solution of the symmetric Anderson Hamiltonian. J. De Phys. 1985, 46, 1459–1467. [Google Scholar] [CrossRef]
- Nozières, P.; Blandin, A. Kondo effect in real metals. J. De Phys. 1980, 41, 193–211. [Google Scholar] [CrossRef]
- Cox, D.L.; Zawadowski, A. Exotic Kondo effects in metals: Magnetic ions in a crystalline electric field and tunnelling centres. Adv. Phys. 1998, 47, 599–942. [Google Scholar] [CrossRef]
- Petta, J.; Johnson, A.; Taylor, J.; Laird, E.; Yacoby, A.; Lukin, M.; Marcus, C.; Hanson, M.; Gossard, A. Coherent manipulation of coupled electron spins in semiconductor quantum dots. Science 2005, 309, 2180–2184. [Google Scholar] [CrossRef]
- Koppens, F.; Buizert, C.; Tielrooij, K.; Vink, I.; Nowack, K.; Meunier, T.; Kouwenhoven, L.; Vandersypen, L. Driven coherent oscillations of a single electron spin in a quantum dot. Nature 2006, 442, 766–771. [Google Scholar] [CrossRef] [PubMed]
- Bocquillon, E.; Parmentier, F.D.; Grenier, C.; Berroir, J.M.; Degiovanni, P.; Glattli, D.C.; Plaçais, B.; Cavanna, A.; Jin, Y.; Fève, G. Electron Quantum Optics: Partitioning Electrons One by One. Phys. Rev. Lett. 2012, 108, 196803. [Google Scholar] [CrossRef] [PubMed]
- Bocquillon, E.; Freulon, V.; Berroir, J.M.; Degiovanni, P.; Plaçais, B.; Cavanna, A.; Jin, Y.; Fève, G. Coherence and Indistinguishability of Single Electrons Emitted by Independent Sources. Science 2013, 339, 1054–1057. [Google Scholar] [CrossRef] [PubMed]
- Bocquillon, E.; Freulon, V.; Parmentier, F.D.; Berroir, J.M.; Plaçais, B.; Wahl, C.; Rech, J.; Jonckheere, T.; Martin, T.; Grenier, C.; et al. Electron quantum optics in ballistic chiral conductors. Ann. Der Phys. 2014, 526, 1–30. [Google Scholar] [CrossRef]
- Grenier, C.; Hervé, R.; Bocquillon, E.; Parmentier, F.; Plaçais, B.; Berroir, J.; Fève, G.; Degiovanni, P. Single-electron quantum tomography in quantum Hall edge channels. New J. Phys. 2011, 13, 093007. [Google Scholar] [CrossRef]
- Bocquillon, E.; Freulon, V.; Berroir, J.M.; Degiovanni, P.; Plaçais, B.; Cavanna, A.; Jin, Y.; Fève, G. Separation of neutral and charge modes in one-dimensional chiral edge channels. Nat. Commun. 2013, 4, 1–7. [Google Scholar] [CrossRef]
- Safi, I.; Schulz, H.J. Transport in an inhomogeneous interacting one-dimensional system. Phys. Rev. B 1995, 52, R17040–R17043. [Google Scholar] [CrossRef]
- Safi, I.; Schulz, H.J. Transport Through a Single-Band Wire Connected to Measuring Leads. In Quantum Transport in Semiconductor Submicron Structures; Kramer, B., Ed.; NATO ASI Series; Springer: Dordrecht, The Netherlands, 1996; pp. 159–172. [Google Scholar] [CrossRef][Green Version]
- Safi, I. A dynamic scattering approach for a gated interacting wire. Eur. Phys. J. B Condens. Matter Complex Syst. 1999, 12, 451–455. [Google Scholar] [CrossRef][Green Version]
- Oreg, Y.; Finkel’stein, A.M. Interedge Interaction in the Quantum Hall Effect. Phys. Rev. Lett. 1995, 74, 3668–3671. [Google Scholar] [CrossRef]
- Blanter, Y.M.; Hekking, F.W.J.; Büttiker, M. Interaction Constants and Dynamic Conductance of a Gated Wire. Phys. Rev. Lett. 1998, 81, 1925–1928. [Google Scholar] [CrossRef]
- Fazio, R.; Hekking, F.W.J.; Khmelnitskii, D.E. Anomalous Thermal Transport in Quantum Wires. Phys. Rev. Lett. 1998, 80, 5611–5614. [Google Scholar] [CrossRef]
- Sukhorukov, E.V.; Cheianov, V.V. Resonant Dephasing in the Electronic Mach-Zehnder Interferometer. Phys. Rev. Lett. 2007, 99, 156801. [Google Scholar] [CrossRef] [PubMed]
- Grenier, C.; Hervé, R.; Fève, G.; Degiovanni, P. Electron quantum optics in quantum Hall edge channels. Mod. Phys. Lett. B 2011, 25, 1053–1073. [Google Scholar] [CrossRef]
- Bertrand, B.; Hermelin, S.; Takada, S.; Yamamoto, M.; Tarucha, S.; Ludwig, A.; Wieck, A.; Bäuerle, C.; Meunier, T. Long-range spin transfer using individual electrons. arXiv 2015, arXiv:1508.04307. [Google Scholar]
- Desjardins, M.M.; Viennot, J.J.; Dartiailh, M.C.; Bruhat, L.E.; Delbecq, M.R.; Lee, M.; Choi, M.S.; Cottet, A.; Kontos, T. Observation of the frozen charge of a Kondo resonance. Nature 2017, 545, 71–74. [Google Scholar] [CrossRef]
- Shiba, H. Korringa Relation for the Impurity Nuclear Spin-Lattice Relaxation in Dilute Kondo Alloys. Prog. Theor. Phys. 1975, 54, 967–981. [Google Scholar] [CrossRef]
- Lee, M.; López, R.; Choi, M.S.; Jonckheere, T.; Martin, T. Effect of many-body correlations on mesoscopic charge relaxation. Phys. Rev. B 2011, 83, 201304. [Google Scholar] [CrossRef]
- Litinski, D.; Brouwer, P.W.; Filippone, M. Interacting mesoscopic capacitor out of equilibrium. Phys. Rev. B 2017, 96, 085429. [Google Scholar] [CrossRef]
- Kubo, R.; Toda, M.; Hashitsume, N. Statistical Physics: Nonequilibrium Statistical Mechanics; Springer: Berlin/Heidelberg, Germany, 1992; Volume 2. [Google Scholar]
- Schiró, M.; Le Hur, K. Tunable hybrid quantum electrodynamics from nonlinear electron transport. Phys. Rev. B 2014, 89, 195127. [Google Scholar] [CrossRef]
- Le Hur, K.; Henriet, L.; Petrescu, A.; Plekhanov, K.; Roux, G.; Schiró, M. Many-body quantum electrodynamics networks: Non-equilibrium condensed matter physics with light. C. R. Phys. 2016, 17, 808–835. [Google Scholar] [CrossRef]
- Le Hur, K.; Henriet, L.; Herviou, L.; Plekhanov, K.; Petrescu, A.; Goren, T.; Schiro, M.; Mora, C.; Orth, P.P. Driven dissipative dynamics and topology of quantum impurity systems. C. R. Phys. 2018, 19, 451–483. [Google Scholar] [CrossRef]
- Delbecq, M.R.; Schmitt, V.; Parmentier, F.D.; Roch, N.; Viennot, J.J.; Fève, G.; Huard, B.; Mora, C.; Cottet, A.; Kontos, T. Coupling a Quantum Dot, Fermionic Leads, and a Microwave Cavity on a Chip. Phys. Rev. Lett. 2011, 107, 256804. [Google Scholar] [CrossRef] [PubMed]
- Delbecq, M.; Bruhat, L.; Viennot, J.; Datta, S.; Cottet, A.; Kontos, T. Photon-mediated interaction between distant quantum dot circuits. Nat. Commun. 2013, 4, 1400. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.Y.; Petersson, K.D.; Stehlik, J.; Taylor, J.M.; Petta, J.R. Photon Emission from a Cavity-Coupled Double Quantum Dot. Phys. Rev. Lett. 2014, 113, 036801. [Google Scholar] [CrossRef] [PubMed]
- Bruhat, L.; Viennot, J.; Dartiailh, M.; Desjardins, M.; Kontos, T.; Cottet, A. Cavity photons as a probe for charge relaxation resistance and photon emission in a quantum dot coupled to normal and superconducting continua. Phys. Rev. X 2016, 6, 021014. [Google Scholar] [CrossRef]
- Mi, X.; Cady, J.V.; Zajac, D.M.; Stehlik, J.; Edge, L.F.; Petta, J.R. Circuit quantum electrodynamics architecture for gate-defined quantum dots in silicon. Appl. Phys. Lett. 2017, 110, 043502. [Google Scholar] [CrossRef]
- Viennot, J.J.; Delbecq, M.R.; Bruhat, L.E.; Dartiailh, M.C.; Desjardins, M.M.; Baillergeau, M.; Cottet, A.; Kontos, T. Towards hybrid circuit quantum electrodynamics with quantum dots. C. R. Phys. 2016, 17, 705–717. [Google Scholar] [CrossRef]
- Deng, G.W.; Henriet, L.; Wei, D.; Li, S.X.; Li, H.O.; Cao, G.; Xiao, M.; Guo, G.C.; Schiro, M.; Hur, K.L.; et al. A Quantum Electrodynamics Kondo Circuit with Orbital and Spin Entanglement. arXiv 2015, arXiv:1509.06141. [Google Scholar]
- Van Wees, B.J.; van Houten, H.; Beenakker, C.W.J.; Williamson, J.G.; Kouwenhoven, L.P.; van der Marel, D.; Foxon, C.T. Quantized conductance of point contacts in a two-dimensional electron gas. Phys. Rev. Lett. 1988, 60, 848–850. [Google Scholar] [CrossRef]
- Wharam, D.; Pepper, M.; Ahmed, H.; Frost, J.; Hasko, D.; Peacock, D.; Ritchie, D.; Jones, G. Addition of the one-dimensional quantised ballistic resistance. J.Phys. C 1988, 21, L887. [Google Scholar] [CrossRef]
- Nigg, S.E.; Büttiker, M. Quantum to classical transition of the charge relaxation resistance of a mesoscopic capacitor. Phys. Rev. B 2008, 77, 085312. [Google Scholar] [CrossRef]
- Imry, Y. Directions in Condensed Matter Physics; Grinstein, G., Mazenco, G., Eds.; World Scientific: Singapore, 1986. [Google Scholar]
- Landauer, R. Electrical transport in open and closed systems. Z. Phys. B 1987, 68, 217–228. [Google Scholar] [CrossRef]
- Rodionov, Y.I.; Burmistrov, I.S.; Ioselevich, A.S. Charge relaxation resistance in the Coulomb blockade problem. Phys. Rev. B 2009, 80, 035332. [Google Scholar] [CrossRef]
- Rodionov, Y.I.; Burmistrov, I.S. Out-of-equilibrium admittance of single electron box under strong Coulomb blockade. JETP Lett. 2010, 92, 696–702. [Google Scholar] [CrossRef][Green Version]
- Ngo Dinh, S.; Bagrets, D.A.; Mirlin, A.D. Nonequilibrium functional bosonization of quantum wire networks. Ann. Phys. 2012, 327, 2794–2852. [Google Scholar] [CrossRef]
- Ngo Dinh, S.; Bagrets, D.A.; Mirlin, A.D. Analytically solvable model of an electronic Mach-Zehnder interferometer. Phys. Rev. B 2013, 87, 195433. [Google Scholar] [CrossRef]
- Garst, M.; Wölfle, P.; Borda, L.; von Delft, J.; Glazman, L. Energy-resolved inelastic electron scattering off a magnetic impurity. Phys. Rev. B 2005, 72, 205125. [Google Scholar] [CrossRef]
- Kawakami, N.; Okiji, A. Density of states for elementary excitations in the Kondo problem. Phys. Rev. B 1990, 42, 2383–2392. [Google Scholar] [CrossRef]
- Lansbergen, G.P.; Tettamanzi, G.C.; Verduijn, J.; Collaert, N.; Biesemans, S.; Blaauboer, M.; Rogge, S. Tunable Kondo Effect in a Single Donor Atom. Nano Lett. 2010, 10, 455–460. [Google Scholar] [CrossRef]
- Tettamanzi, G.C.; Verduijn, J.; Lansbergen, G.P.; Blaauboer, M.; Calderón, M.J.; Aguado, R.; Rogge, S. Magnetic-Field Probing of an SU(4) Kondo Resonance in a Single-Atom Transistor. Phys. Rev. Lett. 2012, 108, 046803. [Google Scholar] [CrossRef]
- Borda, L.; Zaránd, G.; Hofstetter, W.; Halperin, B.I.; von Delft, J. SU(4) Fermi Liquid State and Spin Filtering in a Double Quantum Dot System. Phys. Rev. Lett. 2003, 90, 026602. [Google Scholar] [CrossRef]
- Le Hur, K.; Simon, P. Smearing of charge fluctuations in a grain by spin-flip assisted tunneling. Phys. Rev. B 2003, 67, 201308. [Google Scholar] [CrossRef]
- Zaránd, G.; Brataas, A.; Goldhaber-Gordon, D. Kondo effect and spin filtering in triangular artificial atoms. Solid State Commun. 2003, 126, 463–466. [Google Scholar] [CrossRef][Green Version]
- López, R.; Sánchez, D.; Lee, M.; Choi, M.S.; Simon, P.; Le Hur, K. Probing spin and orbital Kondo effects with a mesoscopic interferometer. Phys. Rev. B 2005, 71, 115312. [Google Scholar] [CrossRef]
- Filippone, M.; Moca, C.P.; Zaránd, G.; Mora, C. Kondo temperature of SU(4) symmetric quantum dots. Phys. Rev. B 2014, 90, 121406. [Google Scholar] [CrossRef]
- Ludovico, M.F.; Lim, J.S.; Moskalets, M.; Arrachea, L.; Sánchez, D. Dynamical energy transfer in ac-driven quantum systems. Phys. Rev. B 2014, 89, 161306. [Google Scholar] [CrossRef]
- Ludovico, M.F.; Battista, F.; von Oppen, F.; Arrachea, L. Adiabatic response and quantum thermoelectrics for ac-driven quantum systems. Phys. Rev. B 2016, 93, 075136. [Google Scholar] [CrossRef]
- Romero, J.I.; Roura-Bas, P.; Aligia, A.A.; Arrachea, L. Nonlinear charge and energy dynamics of an adiabatically driven interacting quantum dot. Phys. Rev. B 2017, 95, 235117. [Google Scholar] [CrossRef]
- Benyamini, A.; Hamo, A.; Kusminskiy, S.V.; Oppen, F.v.; Ilani, S. Real-space tailoring of the electron–phonon coupling in ultraclean nanotube mechanical resonators. Nat. Phys. 2014, 10, 151–156. [Google Scholar] [CrossRef]
- Bode, N.; Kusminskiy, S.V.; Egger, R.; von Oppen, F. Scattering Theory of Current-Induced Forces in Mesoscopic Systems. Phys. Rev. Lett. 2011, 107, 036804. [Google Scholar] [CrossRef]
- Micchi, G.; Avriller, R.; Pistolesi, F. Mechanical Signatures of the Current Blockade Instability in Suspended Carbon Nanotubes. Phys. Rev. Lett. 2015, 115, 206802. [Google Scholar] [CrossRef] [PubMed]
- Micchi, G.; Avriller, R.; Pistolesi, F. Electromechanical transition in quantum dots. Phys. Rev. B 2016, 94, 125417. [Google Scholar] [CrossRef]
- Avriller, R.; Pistolesi, F. Andreev Bound-State Dynamics in Quantum-Dot Josephson Junctions: A Washing Out of the 0-pi Transition. Phys. Rev. Lett. 2015, 114, 037003. [Google Scholar] [CrossRef] [PubMed]
- Pistolesi, F. Bistability of a slow mechanical oscillator coupled to a laser-driven two-level system. Phys. Rev. A 2018, 97, 063833. [Google Scholar] [CrossRef]
- Schaeverbeke, Q.; Avriller, R.; Frederiksen, T.; Pistolesi, F. Single-Photon Emission Mediated by Single-Electron Tunneling in Plasmonic Nanojunctions. Phys. Rev. Lett. 2019, 123, 246601. [Google Scholar] [CrossRef]
- Hamamoto, Y.; Jonckheere, T.; Kato, T.; Martin, T. Dynamic response of a mesoscopic capacitor in the presence of strong electron interactions. Phys. Rev. B 2010, 81, 153305. [Google Scholar] [CrossRef]
- Hamamoto, Y.; Jonckheere, T.; Kato, T.; Martin, T. Quantum phase transition of dynamical resistance in a mesoscopic capacitor. J. Physics Conf. Ser. 2011, 334, 012033. [Google Scholar] [CrossRef]
- Burmistrov, I.S.; Rodionov, Y.I. Charge relaxation resistance in the cotunneling regime of multichannel Coulomb blockade: Violation of Korringa-Shiba relation. Phys. Rev. B 2015, 92, 195412. [Google Scholar] [CrossRef]
- Keeling, J.; Shytov, A.V.; Levitov, L.S. Coherent Particle Transfer in an On-Demand Single-Electron Source. Phys. Rev. Lett. 2008, 101, 196404. [Google Scholar] [CrossRef]
- Ol’khovskaya, S.; Splettstoesser, J.; Moskalets, M.; Büttiker, M. Shot Noise of a Mesoscopic Two-Particle Collider. Phys. Rev. Lett. 2008, 101, 166802. [Google Scholar] [CrossRef]
- Moskalets, M.; Samuelsson, P.; Büttiker, M. Quantized Dynamics of a Coherent Capacitor. Phys. Rev. Lett. 2008, 100, 086601. [Google Scholar] [CrossRef] [PubMed]
- Sasaoka, K.; Yamamoto, T.; Watanabe, S. Single-electron pumping from a quantum dot into an electrode. Appl. Phys. Lett. 2010, 96. [Google Scholar] [CrossRef]
- Moskalets, M.; Haack, G.; Büttiker, M. Single-electron source: Adiabatic versus nonadiabatic emission. Phys. Rev. B 2013, 87, 125429. [Google Scholar] [CrossRef]
- Splettstoesser, J.; Governale, M.; König, J.; Büttiker, M. Charge and spin dynamics in interacting quantum dots. Phys. Rev. B 2010, 81, 165318. [Google Scholar] [CrossRef]
- Contreras-Pulido, L.D.; Splettstoesser, J.; Governale, M.; König, J.; Büttiker, M. Time scales in the dynamics of an interacting quantum dot. Phys. Rev. B 2012, 85, 075301. [Google Scholar] [CrossRef]
- Kashuba, O.; Schoeller, H.; Splettstoesser, J. Nonlinear adiabatic response of interacting quantum dots. EPL (Europhys. Lett.) 2012, 98, 57003. [Google Scholar] [CrossRef]
- Alomar, M.; Lim, J.S.; Sánchez, D. Time-dependent current of interacting quantum capacitors subjected to large amplitude pulses. J. Phys. Conf. Ser. 2015, 647, 012049. [Google Scholar] [CrossRef]
- Alomar, M.I.; Lim, J.S.; Sánchez, D. Coulomb-blockade effect in nonlinear mesoscopic capacitors. Phys. Rev. B 2016, 94, 165425. [Google Scholar] [CrossRef]
- Vanherck, J.; Schulenborg, J.; Saptsov, R.B.; Splettstoesser, J.; Wegewijs, M.R. Relaxation of quantum dots in a magnetic field at finite bias—Charge, spin, and heat currents. Phys. Status Solidi (b) 2017, 254. [Google Scholar] [CrossRef]
- Maslov, D.L.; Stone, M. Landauer conductance of Luttinger liquids with leads. Phys. Rev. B 1995, 52, R5539–R5542. [Google Scholar] [CrossRef] [PubMed]
- Ponomarenko, V.V. Renormalization of the one-dimensional conductance in the Luttinger-liquid model. Phys. Rev. B 1995, 52, R8666–R8667. [Google Scholar] [CrossRef] [PubMed]
- Cuniberti, G.; Sassetti, M.; Kramer, B. AC conductance of a quantum wire with electron-electron interactions. Phys. Rev. B 1998, 57, 1515–1526. [Google Scholar] [CrossRef]
- Marguerite, A. Two-Particle Interferometry for Quantum Signal Processing. Ph.D. Thesis, Université Pierre et Marie Curie, Paris, France, 2017. [Google Scholar]
- Degiovanni, P.; Grenier, C.; Fève, G.; Altimiras, C.; le Sueur, H.; Pierre, F. Plasmon scattering approach to energy exchange and high-frequency noise in v = 2 quantum Hall edge channels. Phys. Rev. B 2010, 81, 121302. [Google Scholar] [CrossRef]
- Fu, L. Electron Teleportation via Majorana Bound States in a Mesoscopic Superconductor. Phys. Rev. Lett. 2010, 104, 056402. [Google Scholar] [CrossRef] [PubMed]
- Shi, Z.; Brouwer, P.W.; Flensberg, K.; Glazman, L.I.; von Oppen, F. Long distance coherence of Majorana wires. arXiv 2020, arXiv:2002.01539. [Google Scholar]
- Vijay, S.; Hsieh, T.H.; Fu, L. Majorana Fermion Surface Code for Universal Quantum Computation. Phys. Rev. X 2015, 5, 041038. [Google Scholar] [CrossRef]
- Plugge, S.; Landau, L.A.; Sela, E.; Altland, A.; Flensberg, K.; Egger, R. Roadmap to Majorana surface codes. Phys. Rev. B 2016, 94, 174514. [Google Scholar] [CrossRef]
- Litinski, D.; Kesselring, M.S.; Eisert, J.; von Oppen, F. Combining Topological Hardware and Topological Software: Color-Code Quantum Computing with Topological Superconductor Networks. Phys. Rev. X 2017, 7, 031048. [Google Scholar] [CrossRef]
- Moca, C.P.; Mora, C.; Weymann, I.; Zaránd, G. Noise of a Chargeless Fermi Liquid. Phys. Rev. Lett. 2018, 120, 016803. [Google Scholar] [CrossRef]
- Ferrier, M.; Arakawa, T.; Hata, T.; Fujiwara, R.; Delagrange, R.; Weil, R.; Deblock, R.; Sakano, R.; Oguri, A.; Kobayashi, K. Universality of non-equilibrium fluctuations in strongly correlated quantum liquids. Nat. Phys. 2016, 12, 230–235. [Google Scholar] [CrossRef]
- Delagrange, R.; Luitz, D.J.; Weil, R.; Kasumov, A.; Meden, V.; Bouchiat, H.; Deblock, R. Manipulating the magnetic state of a carbon nanotube Josephson junction using the superconducting phase. Phys. Rev. B 2015, 91, 241401. [Google Scholar] [CrossRef]
- Delagrange, R.; Weil, R.; Kasumov, A.; Ferrier, M.; Bouchiat, H.; Deblock, R. 0_pi quantum transition in a carbon nanotube Josephson junction: Universal phase dependence and orbital degeneracy. Phys. Rev. B 2016, 93, 195437. [Google Scholar] [CrossRef]
- Delagrange, R.; Basset, J.; Bouchiat, H.; Deblock, R. Emission noise and high frequency cut-off of the Kondo effect in a quantum dot. Phys. Rev. B 2018, 97, 041412. [Google Scholar] [CrossRef]
- Crépieux, A.; Sahoo, S.; Duong, T.Q.; Zamoum, R.; Lavagna, M. Emission Noise in an Interacting Quantum Dot: Role of Inelastic Scattering and Asymmetric Coupling to the Reservoirs. Phys. Rev. Lett. 2018, 120, 107702. [Google Scholar] [CrossRef] [PubMed]
- Saminadayar, L.; Glattli, D.C.; Jin, Y.; Etienne, B. Observation of the e/3 Fractionally Charged Laughlin Quasiparticle. Phys. Rev. Lett. 1997, 79, 2526–2529. [Google Scholar] [CrossRef]
- Reznikov, M.; Picciotto, R.d.; Griffiths, T.G.; Heiblum, M.; Umansky, V. Observation of quasiparticles with one-fifth of an electron’s charge. Nature 1999, 399, 238–241. [Google Scholar] [CrossRef]
- Kapfer, M.; Roulleau, P.; Santin, M.; Farrer, I.; Ritchie, D.A.; Glattli, D.C. A Josephson relation for fractionally charged anyons. Science 2019, 363, 846–849. [Google Scholar] [CrossRef] [PubMed]
- Bisognin, R.; Bartolomei, H.; Kumar, M.; Safi, I.; Berroir, J.M.; Bocquillon, E.; Plaçais, B.; Cavanna, A.; Gennser, U.; Jin, Y.; et al. Microwave photons emitted by fractionally charged quasiparticles. Nat. Commun. 2019, 10, 1–7. [Google Scholar] [CrossRef]
- Chamon, C.D.C.; Freed, D.E.; Wen, X.G. Tunneling and quantum noise in one-dimensional Luttinger liquids. Phys. Rev. B 1995, 51, 2363–2379. [Google Scholar] [CrossRef]
- Bena, C.; Safi, I. Emission and absorption noise in the fractional quantum Hall effect. Phys. Rev. B 2007, 76, 125317. [Google Scholar] [CrossRef]
- Safi, I.; Joyez, P. Time-dependent theory of nonlinear response and current fluctuations. Phys. Rev. B 2011, 84, 205129. [Google Scholar] [CrossRef]
- Safi, I. Driven quantum circuits and conductors: A unifying perturbative approach. Phys. Rev. B 2019, 99, 045101. [Google Scholar] [CrossRef]
- Safi, I.; Devillard, P.; Martin, T. Partition Noise and Statistics in the Fractional Quantum Hall Effect. Phys. Rev. Lett. 2001, 86, 4628–4631. [Google Scholar] [CrossRef] [PubMed]
- Guyon, R.; Devillard, P.; Martin, T.; Safi, I. Klein factors in multiple fractional quantum Hall edge tunneling. Phys. Rev. B 2002, 65, 153304. [Google Scholar] [CrossRef]
- Kim, E.A.; Lawler, M.; Vishveshwara, S.; Fradkin, E. Signatures of Fractional Statistics in Noise Experiments in Quantum Hall Fluids. Phys. Rev. Lett. 2005, 95, 176402. [Google Scholar] [CrossRef] [PubMed]
- Bena, C.; Nayak, C. Effects of non-Abelian statistics on two-terminal shot noise in a quantum Hall liquid in the Pfaffian state. Phys. Rev. B 2006, 73, 155335. [Google Scholar] [CrossRef]
- Carrega, M.; Ferraro, D.; Braggio, A.; Magnoli, N.; Sassetti, M. Spectral noise for edge states at the filling factor$\upnu$= 5/2. New J. Phys. 2012, 14, 023017. [Google Scholar] [CrossRef]
- Ferraro, D.; Carrega, M.; Braggio, A.; Sassetti, M. Multiple quasiparticle Hall spectroscopy investigated with a resonant detector. New J. Phys. 2014, 16, 043018. [Google Scholar] [CrossRef]
- Ferraro, D.; Braggio, A.; Merlo, M.; Magnoli, N.; Sassetti, M. Relevance of Multiple Quasiparticle Tunneling between Edge States at v = p/(2np+1). Phys. Rev. Lett. 2008, 101, 166805. [Google Scholar] [CrossRef] [PubMed]
- Crépieux, A.; Devillard, P.; Martin, T. Photoassisted current and shot noise in the fractional quantum Hall effect. Phys. Rev. B 2004, 69, 205302. [Google Scholar] [CrossRef]
- Roussel, B.; Degiovanni, P.; Safi, I. Perturbative fluctuation dissipation relation for nonequilibrium finite-frequency noise in quantum circuits. Phys. Rev. B 2016, 93, 045102. [Google Scholar] [CrossRef]
- Bartolomei, H.; Kumar, M.; Bisognin, R.; Marguerite, A.; Berroir, J.M.; Bocquillon, E.; Plaçais, B.; Cavanna, A.; Dong, Q.; Gennser, U.; et al. Fractional statistics in anyon collisions. Science 2020, 368, 173–177. [Google Scholar] [CrossRef] [PubMed]
- Glattli, D.C.; Nath, J.; Taktak, I.; Roulleau, P.; Bauerle, C.; Waintal, X. Design of a Single-Shot Electron detector with sub-electron sensitivity for electron flying qubit operation. arXiv 2020, arXiv:2002.03947. [Google Scholar]
- Wagner, G.; Nguyen, D.X.; Kovrizhin, D.L.; Simon, S.H. Driven quantum dot coupled to a fractional quantum Hall edge. Phys. Rev. B 2019, 100, 245111. [Google Scholar] [CrossRef]
- Weinberg, S. The Quantum Theory of Fields: Foundations; Cambridge University Press: Cambridge, UK, 1996; Volume 1. [Google Scholar]
- Newton, R.G. Scattering Theory of Waves and Particles; Dover Publications: Mineola, NY, USA, 2002. [Google Scholar]
- Filippone, M.; Brouwer, P.W. Tunneling into quantum wires: Regularization of the tunneling Hamiltonian and consistency between free and bosonized fermions. Phys. Rev. B 2016, 94, 235426. [Google Scholar] [CrossRef]
- Gabelli, J. Mise en évidence de la Cohérence Quantique des Conducteurs en Régime Dynamique. Ph.D. Thesis, Université Pierre et Marie Curie-Paris VI, Paris, France, 2006. [Google Scholar]
- Fève, G. Quantification du Courant Alternatif: La Boîte Quantique Comme Source D’électrons Uniques Subnanoseconde. Ph.D. Thesis, Université Pierre et Marie Curie-Paris VI, Paris, France, 2006. [Google Scholar]
- Wigner, E.P. Lower Limit for the Energy Derivative of the Scattering Phase Shift. Phys. Rev. 1955, 98, 145–147. [Google Scholar] [CrossRef]
- Cohen-Tannoudji, C.; Dupont-Roc, J.; Grynberg, G. Photons and Atoms: Introduction to Quantum Electrodynamics; Wiley: Hoboken, NJ, USA, 1989. [Google Scholar]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Filippone, M.; Marguerite, A.; Le Hur, K.; Fève, G.; Mora, C. Phase-Coherent Dynamics of Quantum Devices with Local Interactions. Entropy 2020, 22, 847. https://doi.org/10.3390/e22080847
Filippone M, Marguerite A, Le Hur K, Fève G, Mora C. Phase-Coherent Dynamics of Quantum Devices with Local Interactions. Entropy. 2020; 22(8):847. https://doi.org/10.3390/e22080847
Chicago/Turabian StyleFilippone, Michele, Arthur Marguerite, Karyn Le Hur, Gwendal Fève, and Christophe Mora. 2020. "Phase-Coherent Dynamics of Quantum Devices with Local Interactions" Entropy 22, no. 8: 847. https://doi.org/10.3390/e22080847
APA StyleFilippone, M., Marguerite, A., Le Hur, K., Fève, G., & Mora, C. (2020). Phase-Coherent Dynamics of Quantum Devices with Local Interactions. Entropy, 22(8), 847. https://doi.org/10.3390/e22080847




