Knudsen Number Effects on Two-Dimensional Rayleigh–Taylor Instability in Compressible Fluid: Based on a Discrete Boltzmann Method
Abstract
:1. Introduction
2. Discrete Boltzmann Model
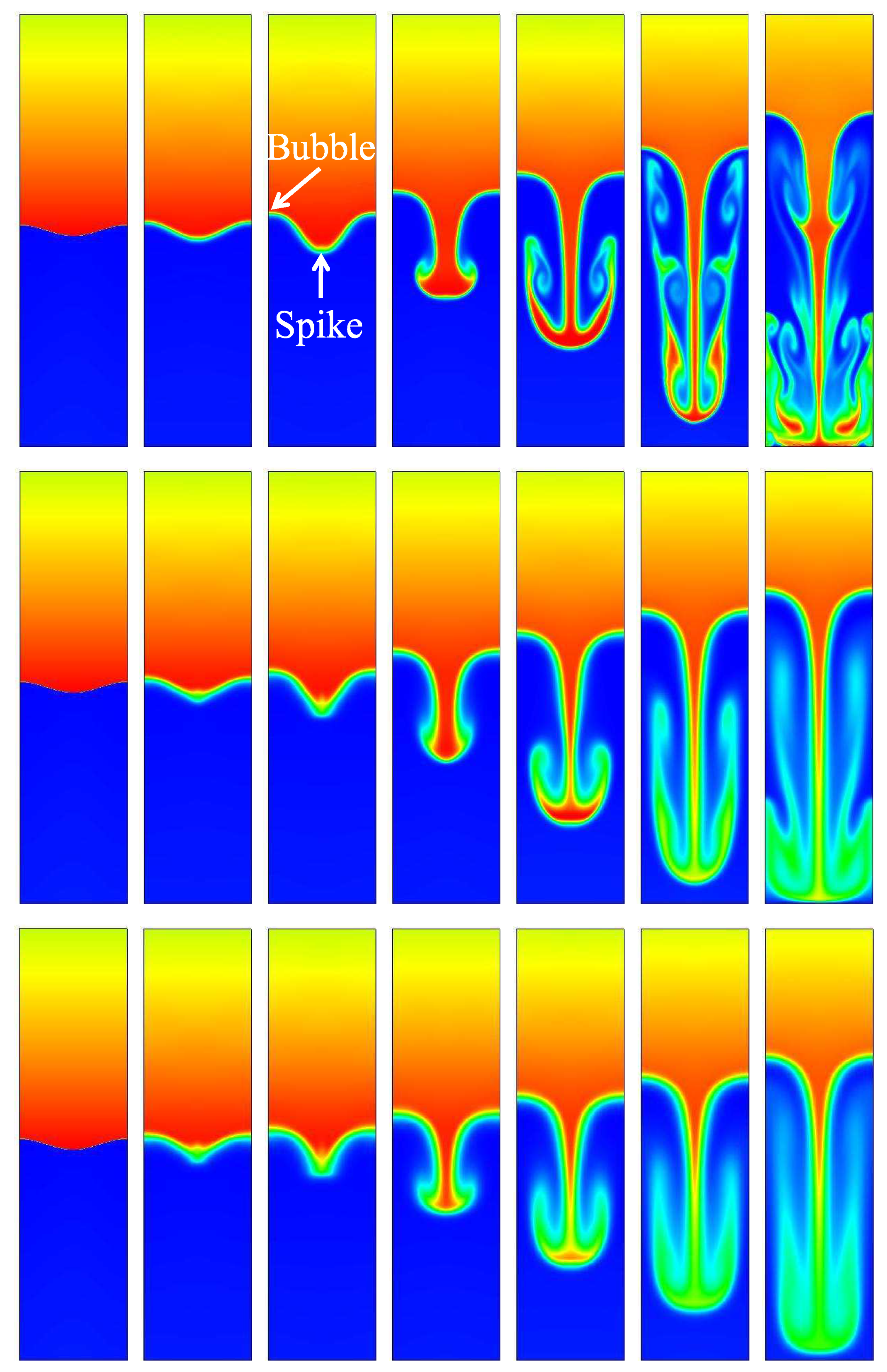
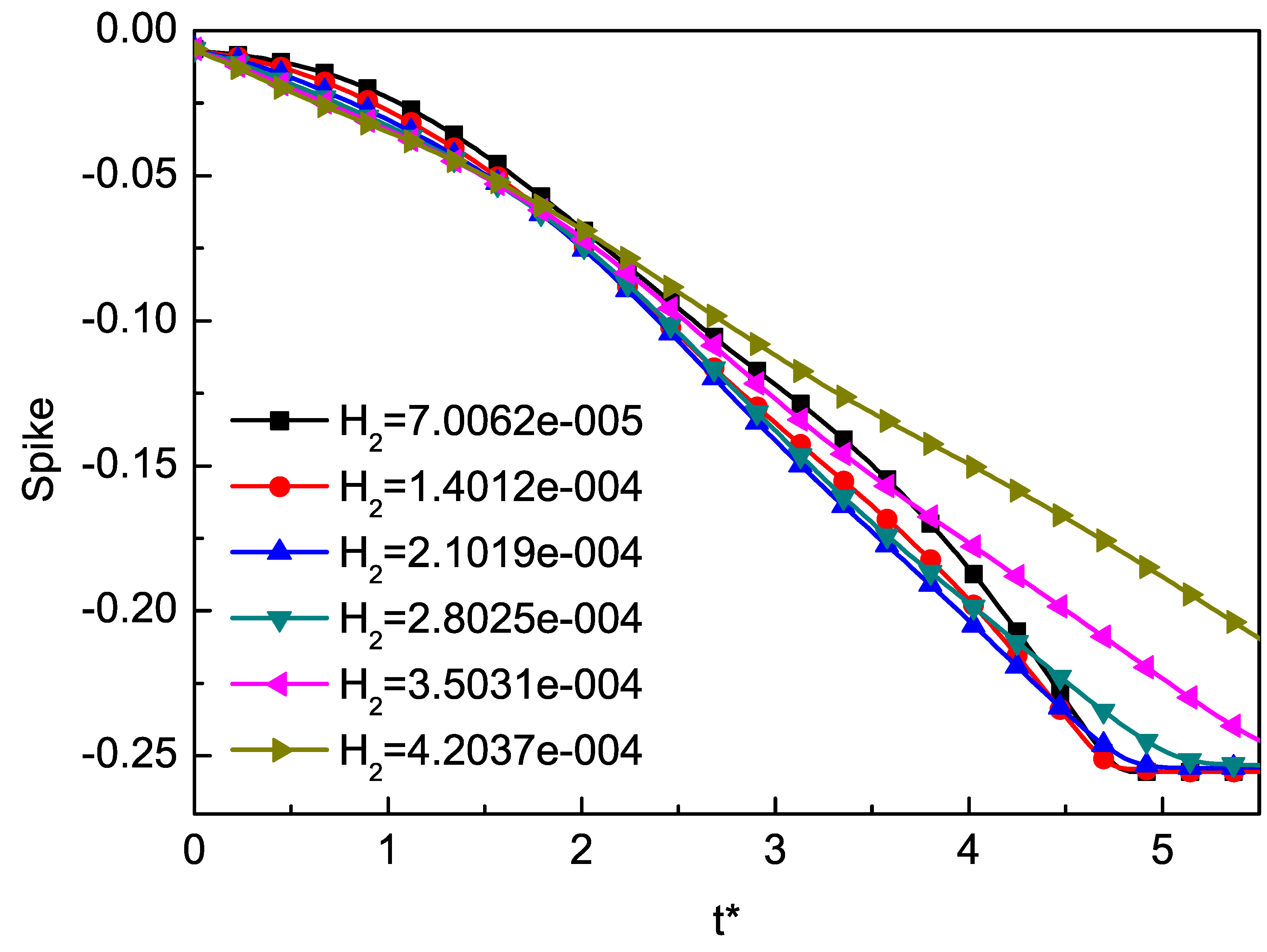
3. Numerical Simulations
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Kull, H.J. Theory of the Rayleigh–Taylor instability. Phys. Rep. 1991, 206, 197–325. [Google Scholar] [CrossRef]
- Betti, R.; Goncharov, V.N.; McCrory, R.L.; Verdon, C.P. Growth rates of the ablative Rayleigh–Taylor instability in inertial confinement fusion. Phys. Plasmas 1998, 5, 1446–1454. [Google Scholar] [CrossRef]
- Lindl, J.D.; McCrory, R.L.; Campbell, E.M. Rogress toward ignition and burn propagation in inertial confinement fusion. Phys. Today 1992, 45, 32–40. [Google Scholar] [CrossRef] [Green Version]
- Wang, L.; Ye, W.; He, X.; Wu, J.; Fan, Z.; Xue, C.; Jia, G. Theoretical and simulation research of hydrodynamic instabilities in inertial-confinement fusion implosions. Sci. China Phys. Mech. 2017, 60, 055201. [Google Scholar] [CrossRef]
- Cabot, W.H.; Cook, A.W. Reynolds number effects on Rayleigh–Taylor instability with possible implications for type Ia supernovae. Nat. Phys. 2006, 2, 562–568. [Google Scholar] [CrossRef]
- Huang, L.; Jian, G.D.; Qiu, X.M.; Peng, X.D.; Wang, S.Q. Effects of compressibility on the finite Larmor radius stabilized Rayleigh–Taylor instability in Z-pinch implosions. Acta Phys. Sin. 2008, 15, 022103. [Google Scholar] [CrossRef]
- Wang, L.F.; Yang, B.L.; Ye, W.H.; He, X.T. Stabilization of the Rayleigh–Taylor instability in quantum magnetized plasmas. Phys. Plasmas 2012, 19, 072704. [Google Scholar]
- Milinković, K.; Padding, J.T.; Dijkstra, M. Hydrodynamic Rayleigh–Taylor-like instabilities in sedimenting colloidal mixtures. Soft Matter 2011, 7, 11177–11186. [Google Scholar]
- Park, H.S.; Lorenz, K.T.; Cavallo, R.M.; Pollaine, S.M.; Prisbrey, S.T.; Rudd, R.E.; Becker, R.C.; Bernier, J.V.; Remington, B.A. Viscous Rayleigh–Taylor instability experiments at high pressure and strain rate. Phys. Rev. Lett. 2010, 104, 135504. [Google Scholar] [CrossRef]
- White, J.; Oakley, J.; Anderson, M.; Bonazza, R. Experimental measurements of the nonlinear Rayleigh–Taylor instability using a magnetorheological fluid. Phys. Rev. E 2010, 81, 026303. [Google Scholar] [CrossRef]
- Wykes, M.S.D.; Dalziel, S.B. Efficient mixing in stratified flows: Experimental study of a Rayleigh–Taylor unstable interface within an otherwise stable stratification. J. Fluid Mech. 2014, 756, 1027–1057. [Google Scholar] [CrossRef]
- Rigon, G.; Casner, A.; Albertazzi, B.; Michel, T.; Mabey, P.; Falize, E.; Ballet, J.; Som, L.V.B.; Pikuz, S.; Sakawa, Y.; et al. Rayleigh–Taylor instability experiments on the LULI2000 laser in scaled conditions for young supernova remnants. Phys. Rev. E 2019, 100, 021201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Polavarapu, R.; Roach, P.; Banerjee, A. Rayleigh–Taylor-instability experiments with elastic-plastic materials. Phys. Rev. E 2019, 99, 053104. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.B.; Tao, J.J.; He, X.T. Unified decomposition method to study Rayleigh–Taylor instability in liquids and solids. Phys. Rev. E 2018, 97, 063109. [Google Scholar] [CrossRef] [PubMed]
- Ye, W.H.; Zhang, W.Y.; Chen, G.N.; Jin, C.Q.; Zhang, J. Numerical simulations of the FCT method on Rayleigh–Taylor and Richtmyer-Meshkov instabilities. Chin. J. Comput. Phys. 1998, 15, 277. [Google Scholar]
- Dervieux, A.; Thomasset, F. A finite element method for the simulation of a Rayleigh–Taylor instability. In Approximation Methods for Navier–Stokes Problems; Rautmann, R., Ed.; Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 1980; Volume 771, pp. 145–158. [Google Scholar]
- Terashima, H.; Tryggvason, G. A front-tracking/ghost-fluid method for fluid interfaces in compressible flows. J. Comput. Phys. 2009, 228, 4012–4037. [Google Scholar] [CrossRef]
- Gallis, M.A.; Koehler, T.P.; Torczynski, J.R.; Plimpton, S.J. Direct simulation Monte Carlo investigation of the Rayleigh–Taylor instability. Phys. Rev. Fluids 2016, 1, 043403. [Google Scholar] [CrossRef]
- Guo, K.L.; Chen, R.H.; Li, Y.L.; Tian, W.X.; Su, G.H.; Qiu, S.Z. Numerical simulation of Rayleigh–Taylor Instability with periodic boundary condition using MPS method. Prog. Nucl. Energ. 2018, 109, 130–144. [Google Scholar] [CrossRef]
- Shadloo, M.S.; Zainali, A.; Yildiz, M. Simulation of single mode Rayleigh–Taylor instability by SPH method. Comput. Mech. 2013, 51, 699. [Google Scholar] [CrossRef]
- Tryggvason, G. Numerical Simulations of the Rayleigh–Taylor Instability. J. Comput. Phys. 1998, 75, 253–282. [Google Scholar] [CrossRef] [Green Version]
- Miles, A.R.; Edwards, M.J.; Greenough, J.A. Effect of initial conditions on two-dimensional Rayleigh–Taylor instability and transition to turbulence in planar blast-wave-driven systems. Phys. Plasmas 2004, 11, 5278–5296. [Google Scholar] [CrossRef]
- Banerjee, A.; Andrews, M.J. 3D Simulations to investigate initial condition effects on the growth of Rayleigh–Taylor mixing. Int. J. Heat. Mass Tran. 2009, 52, 3906–3917. [Google Scholar] [CrossRef]
- Liu, W.H.; Wang, L.F.; Ye, W.H.; He, X.T. Nonlinear saturation amplitudes in classical Rayleigh–Taylor instability at arbitrary Atwood numbers. Phys. Plasmas 2012, 19, 042705. [Google Scholar]
- Rozanov, V.B.; Kuchugov, P.A.; Zmitrenko, N.V.; Yanilkin, Y.V. Effect of initial conditions on the development of Rayleigh–Taylor instability. J. Russ. Laser Res. 2015, 36, 139–150. [Google Scholar] [CrossRef]
- Sagert, I.; Howell, J.; Staber, A.; Strother, T.; Colbry, D.; Bauer, W. Knudsen-number dependence of two-dimensional single-mode Rayleigh–Taylor fluid instabilities. Phys. Rev. E. 2015, 92, 013009. [Google Scholar] [CrossRef] [Green Version]
- Yan, R.; Betti, R.; Sanz, J.; Aluie, H.; Liu, B.; Frank, A. Three-dimensional single-mode nonlinear ablative Rayleigh–Taylor instability. Phys. Plasmas 2016, 23, 022701. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Q.; Jiang, L.F. Kinetic and thermal energy dissipation rates in two-dimensional Rayleigh–Taylor turbulence. Phys. Fluids 2016, 28, 045109. [Google Scholar] [CrossRef]
- Aslangil, D.; Banerjee, A.; Lawrie, A.G.W. Numerical investigation of initial condition effects on Rayleigh–Taylor instability with acceleration reversals. Phys. Rev. E 2016, 94, 053114. [Google Scholar] [CrossRef] [Green Version]
- Zhang, H.; Betti, R.; Yan, R.; Zhao, D.; Shvarts, D.; Aluie, H. Self-similar multimode bubble-front evolution of the ablative Rayleigh–Taylor instability in two and three dimensions. Phys. Rev. Lett. 2018, 121, 185002. [Google Scholar] [CrossRef] [Green Version]
- Hu, Z.X.; Zhang, Y.S.; Tian, B.; He, Z.; Li, L. Effect of viscosity on two-dimensional single-mode Rayleigh–Taylor instability during and after the reacceleration stage. Phys. Fluids 2019, 31, 104108. [Google Scholar]
- Kord, A.; Capecelatro, J. Optimal perturbations for controlling the growth of a Rayleigh–Taylor instability. J. Fluid Mech. 2019, 876, 150–185. [Google Scholar] [CrossRef]
- Liu, W.H.; Chen, Y.L.; Huang, Y.; Mei, Y.; Ye, W.H. Mode-coupling branches in single-mode classical Rayleigh–Taylor instability for arbitrary atwood numbers. Results Phys. 2019, 12, 1142–1148. [Google Scholar] [CrossRef]
- Doludenko, A.; Fortova, S.; Shepelev, V.; Son, E. Numerical simulation of the Rayleigh–Taylor instability of inviscid and viscous fluid. Phys. Scripta 2019, 94, 094003. [Google Scholar] [CrossRef]
- Luo, T.F.; Wang, J.C.; Xie, C.Y.; Wan, M.P.; Chen, S.Y. Effects of compressibility and Atwood number on the single-mode Rayleigh–Taylor instability. Phys. Fluids 2020, 31, 012110. [Google Scholar]
- Zhou, Y. Rayleigh–Taylor and Richtmyer-Meshkov instability induced flow, turbulence, and mixing. I. Phys. Rep. 2017, 720, 1–136. [Google Scholar]
- Zhou, Y. Rayleigh–Taylor and Richtmyer-Meshkov instability induced flow, turbulence, and mixing. II. Phys. Rep. 2017, 723, 1–160. [Google Scholar] [CrossRef]
- Peng, Y.; Shu, C.; Chew, Y.T. Simplified thermal lattice Boltzmann model for incompressible thermal flows. Phys. Rev. E 2003, 68, 026701. [Google Scholar] [CrossRef] [PubMed]
- Wei, Y.K.; Wang, Z.D.; Dou, H.S.; Qian, Y.H.; Yan, W.W. Simulations of natural convection heat transfer in an enclosure at different Rayleigh number using lattice Boltzmann method. Comput. Fluids 2016, 124, 30–38. [Google Scholar] [CrossRef]
- Wei, Y.K.; Yang, H.; Lin, Z.; Wang, Z.D.; Qian, Y.H. A novel two-dimensional coupled lattice Boltzmann model for thermal incompressible flows. Appl. Math. Comput. 2018, 339, 556–567. [Google Scholar] [CrossRef]
- Wang, Z.D.; Wei, Y.K.; Qian, Y.H. A bounce back-immersed boundary-lattice Boltzmann model for curved boundary. Appl. Math. Model. 2020, 81, 428–440. [Google Scholar] [CrossRef]
- Guo, Z.L.; Zhao, T.S. Lattice Boltzmann model for incompressible flows through porous media. Phys. Rev. E 2002, 66, 036304. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.H.; Kang, Q.J.; Leonardi, C.R.; Schmieschek, S.; Narváez, A.; Jones, B.D.; Harting, J. Multiphase lattice Boltzmann simulations for porous media applications. Comput. Geosci. 2016, 20, 777–805. [Google Scholar] [CrossRef] [Green Version]
- He, Y.L.; Liu, Q.; Li, Q.; Tao, W.Q. Lattice Boltzmann methods for single-phase and solid-liquid phase-change heat transfer in porous media: A review. Int. J. Heat Mass Tran. 2016, 129, 160–197. [Google Scholar] [CrossRef] [Green Version]
- Chen, S.Y.; Chen, H.D.; Martnez, D.; Matthaeus, W. Lattice Boltzmann model for simulation of magnetohydrodynamics. Phys. Rev. Lett. 1991, 67, 3776–3779. [Google Scholar] [CrossRef] [PubMed]
- Dellar, P.J. Lattice Boltzmann magnetohydrodynamics with current-dependent resistivity. J. Comput. Phys. 2013, 237, 115–131. [Google Scholar] [CrossRef]
- Li, D.M.; Lai, H.L.; Shi, B.C. Mesoscopic simulation of the (2+1)-dimensional wave equation with nonlinear damping and source terms using the lattice Boltzmann BGK model. Entropy 2019, 21, 390. [Google Scholar] [CrossRef] [Green Version]
- Li, D.M.; Lai, H.L.; Lin, C.D. Mesoscopic simulation of the two-component system of coupled sine-Gordon equations with lattice Boltzmann method. Entropy 2019, 21, 542. [Google Scholar] [CrossRef] [Green Version]
- Lim, C.Y.; Shu, C.; Niu, X.D.; Chew, Y.T. Application of lattice Boltzmann method to simulate microchannel flows. Phys. Fluids 2002, 14, 2299–2308. [Google Scholar] [CrossRef]
- Tang, G.H.; Tao, W.Q.; He, Y.L. Lattice Boltzmann method for simulating gas flow in microchannels. Int. J. Mod. Phys. C 2004, 15, 335–347. [Google Scholar] [CrossRef] [Green Version]
- Li, Q.; He, Y.L.; Tang, G.H.; Tao, W.Q. Lattice Boltzmann modeling of microchannel flows in the transition flow regime. Microfluid. nanofluid. 2011, 10, 607–618. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D. Entropy generation of nanofluid in the presence of magnetic field using Lattice Boltzmann Method. Phys. A 2015, 417, 273–286. [Google Scholar] [CrossRef]
- Li, Q.; Luo, K.H.; Kang, Q.J.; He, Y.L.; Chen, Q.; Liu, Q. Lattice Boltzmann methods for multiphase flow and phase-change heat transfer. Prog. Energ. Combust. 2016, 52, 62–105. [Google Scholar] [CrossRef] [Green Version]
- He, X.Y.; Chen, S.Y.; Zhang, R.Y. A lattice Boltzmann scheme for incompressible multiphase flow and its application in simulation of Rayleigh–Taylor instability. J. Comput. Phys. 1999, 152, 642–663. [Google Scholar] [CrossRef]
- Scagliarini, A.; Biferale, L.; Sbragaglia, M.; Sugiyama, K.; Toschi, F. Lattice Boltzmann methods for thermal flows: Continuum limit and applications to compressible Rayleigh–Taylor systems. Phys. Fluids 2010, 22, 055101. [Google Scholar] [CrossRef]
- Li, Q.; Luo, K.H.; Gao, Y.J.; He, Y.L. Additional interfacial force in lattice Boltzmann models for incompressible multiphase flows. Phys. Rev. E 2012, 85, 026704. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y.; Shu, C.; Huang, H.B.; Teo, C.J. Multiphase lattice Boltzmann flux solver for incompressible multiphase flows with large density ratio. J. Comput. Phys. 2015, 280, 404–423. [Google Scholar] [CrossRef]
- Liang, H.; Li, Q.X.; Shi, B.C.; Chai, Z.H. Lattice boltzmann simulation of three-dimensional Rayleigh–Taylor instability. Phys. Rev. E 2016, 93, 033113. [Google Scholar] [CrossRef]
- Wei, Y.K.; Dou, H.S.; Qian, Y.H.; Wang, Z.D. A novel two-dimensional coupled lattice Boltzmann model for incompressible flow in application of turbulence Rayleigh–Taylor instability. J. Comput. Phys. 2017, 156, 97–102. [Google Scholar] [CrossRef]
- Yang, X.Y.; He, H.J.; Xu, J.; Wei, Y.K.; Zhang, H. Entropy generation rates in two-dimensional Rayleigh–Taylor turbulence mixing. Entropy 2018, 20, 738. [Google Scholar] [CrossRef] [Green Version]
- Liang, H.; Hu, X.L.; Huang, X.F.; Xu, J.R. Direct numerical simulations of multi-mode immiscible Rayleigh–Taylor instability with high Reynolds numbers. Phys. Fluids 2019, 31, 112104. [Google Scholar]
- Xu, A.G.; Zhang, G.C.; Gan, Y.B.; Chen, F.; Yu, X.J. Lattice Boltzmann modeling and simulation of compressible flows. Front. Phys. 2012, 7, 582–600. [Google Scholar] [CrossRef] [Green Version]
- Gan, Y.B.; Xu, A.G.; Zhang, G.C.; Yang, Y. Lattice BGK kinetic model for high-speed compressible flows: Hydrodynamic and non-equilibrium behaviors. Europhys. Lett. 2013, 103, 24003. [Google Scholar] [CrossRef] [Green Version]
- Xu, A.G.; Zhang, G.C.; Zhang, Y.D. Discrete Boltzmann Modeling of Compressible Flows. In Kinetic Theory; Kyzas, G.Z., Mitropoulos, A.C., Eds.; IntechOpen: London, UK, 2018; Chapter 2. [Google Scholar]
- Lin, C.D.; Luo, K.H.; Fei, L.L.; Succi, S. A multi-component discrete Boltzmann model for non-equilibrium reactive flows. Sci. Rep. 2017, 7, 14580. [Google Scholar] [CrossRef] [PubMed]
- Xu, A.G.; Zhang, G.C.; Zhang, Y.D.; Wang, P.; Ying, Y.J. Discrete Boltzmann model for implosion- and explosionrelated compressible flow with spherical symmetry. Front. Phys. 2018, 13, 135102. [Google Scholar] [CrossRef] [Green Version]
- Lin, C.D.; Luo, K.H. Mesoscopic simulation of non-equilibrium detonation with discrete Boltzmann method. Combust. Flame 2018, 198, 356–362. [Google Scholar] [CrossRef]
- Zhang, Y.D.; Xu, A.G.; Zhang, G.C.; Chen, Z.H.; Wang, P. Discrete ellipsoidal statistical BGK model and Burnett equations. Front. Phys. 2018, 13, 135101. [Google Scholar] [CrossRef] [Green Version]
- Gan, Y.B.; Xu, A.G.; Zhang, G.C.; Lin, C.D.; Lai, H.L.; Liu, Z.P. Nonequilibrium and morphological characterizations of Kelvin–Helmholtz instability in compressible flows. Front. Phys. 2019, 14, 43602. [Google Scholar] [CrossRef] [Green Version]
- Lin, C.D.; Luo, K.H.; Gan, Y.B.; Liu, Z.P. Kinetic simulation of non-equilibrium Kelvin–Helmholtz instability. Commun. Theor. Phys. 2019, 71, 132–142. [Google Scholar] [CrossRef]
- Liu, H.; Kang, W.; Zhang, Q.; Zhang, Y.; Duan, H.L.; He, X.T. Molecular dynamics simulations of microscopic structure of ultra strong shock waves in dense helium. Front. Phys. 2016, 11, 115206. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, Y.; Kang, W.; Zhang, P.; Duan, H.L.; He, X.T. Molecular dynamics simulation of strong shock waves propagating in dense deuterium, taking into consideration effects of excited electrons. Phys. Rev. E 2016, 95, 023201. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.D.; Xu, A.G.; Zhang, G.C.; Chen, Z.H.; Wang, P. Discrete Boltzmann method for non-equilibrium flows: Based on Shakhov model. Comput. Phys. Commun. 2019, 238, 50–65. [Google Scholar] [CrossRef] [Green Version]
- Lai, H.L.; Xu, A.G.; Zhang, G.C.; Gan, Y.B.; Ying, Y.J.; Succi, S. Nonequilibrium thermohydrodynamic effects on the Rayleigh–Taylor instability in compressible flows. Phys. Rev. E 2016, 94, 023106. [Google Scholar] [CrossRef] [PubMed]
- Chen, F.; Xu, A.G.; Zhang, G.C. Viscosity, heat conductivity, and Prandtl number effects in the Rayleigh–Taylor Instability. Front. Phys. 2016, 11, 114703. [Google Scholar] [CrossRef] [Green Version]
- Lin, C.D.; Xu, A.G.; Zhang, G.C.; Luo, K.H.; Li, Y.J. Discrete Boltzmann modeling of Rayleigh–Taylor instability in two-component compressible flows. Phys. Rev. E 2017, 96, 053305. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, F.; Xu, A.G.; Zhang, G.C. Collaboration and competition between Richtmyer-Meshkov instability and Rayleigh–Taylor instability. Phys. Fluids 2018, 30, 102105. [Google Scholar]
- Li, D.M.; Lai, H.L.; Xu, A.G.; Zhang, G.C.; Lin, C.D.; Gan, Y.B. Discrete Boltzmann simulation of Rayleigh–Taylor instability in compressible flows. Acta Phys. Sin. 2018, 67, 080501. [Google Scholar]
- Zhang, G.; Xu, A.G.; Li, Y.J.; Lai, H.L.; Hu, X.M. Particle tracking manifestation of compressible flow and mixing induced by Rayleigh–Taylor instability. arXiv 2019, arXiv:1912.13181. [Google Scholar]
- He, X.Y.; Shan, X.W.; Doolen, G.D. Discrete Boltzmann equation model for nonideal gases. Phys. Rev. E 1998, 57, R13. [Google Scholar] [CrossRef]
- Watari, M. Finite difference lattice Boltzmann method with arbitrary specific heat ratio applicable to supersonic flow simulations. Phys. A 2007, 382, 502–522. [Google Scholar] [CrossRef]




© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ye, H.; Lai, H.; Li, D.; Gan, Y.; Lin, C.; Chen, L.; Xu, A. Knudsen Number Effects on Two-Dimensional Rayleigh–Taylor Instability in Compressible Fluid: Based on a Discrete Boltzmann Method. Entropy 2020, 22, 500. https://doi.org/10.3390/e22050500
Ye H, Lai H, Li D, Gan Y, Lin C, Chen L, Xu A. Knudsen Number Effects on Two-Dimensional Rayleigh–Taylor Instability in Compressible Fluid: Based on a Discrete Boltzmann Method. Entropy. 2020; 22(5):500. https://doi.org/10.3390/e22050500
Chicago/Turabian StyleYe, Haiyan, Huilin Lai, Demei Li, Yanbiao Gan, Chuandong Lin, Lu Chen, and Aiguo Xu. 2020. "Knudsen Number Effects on Two-Dimensional Rayleigh–Taylor Instability in Compressible Fluid: Based on a Discrete Boltzmann Method" Entropy 22, no. 5: 500. https://doi.org/10.3390/e22050500
APA StyleYe, H., Lai, H., Li, D., Gan, Y., Lin, C., Chen, L., & Xu, A. (2020). Knudsen Number Effects on Two-Dimensional Rayleigh–Taylor Instability in Compressible Fluid: Based on a Discrete Boltzmann Method. Entropy, 22(5), 500. https://doi.org/10.3390/e22050500





