Effects of Chemical Species and Nonlinear Thermal Radiation with 3D Maxwell Nanofluid Flow with Double Stratification—An Analytical Solution
Abstract
1. Introduction
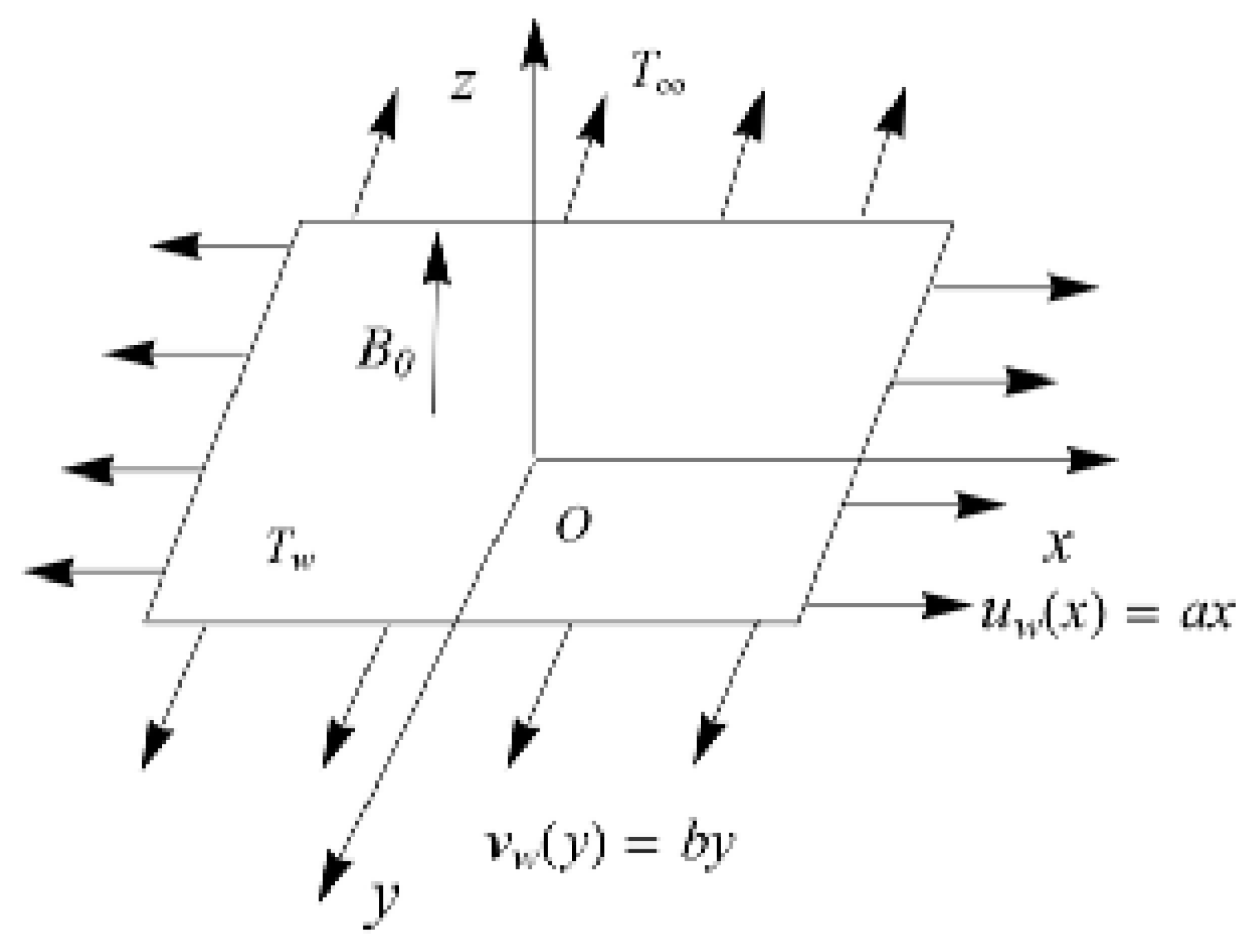
2. Mathematical Modeling
3. Solution by HAM
4. Entropy Generation Analysis
- Fluid friction irreversibility (FFI)
- Diffusive irreversibility (DI)
- Conduction effect or heat transfer irreversibility (HTI)
5. Convergence Analysis
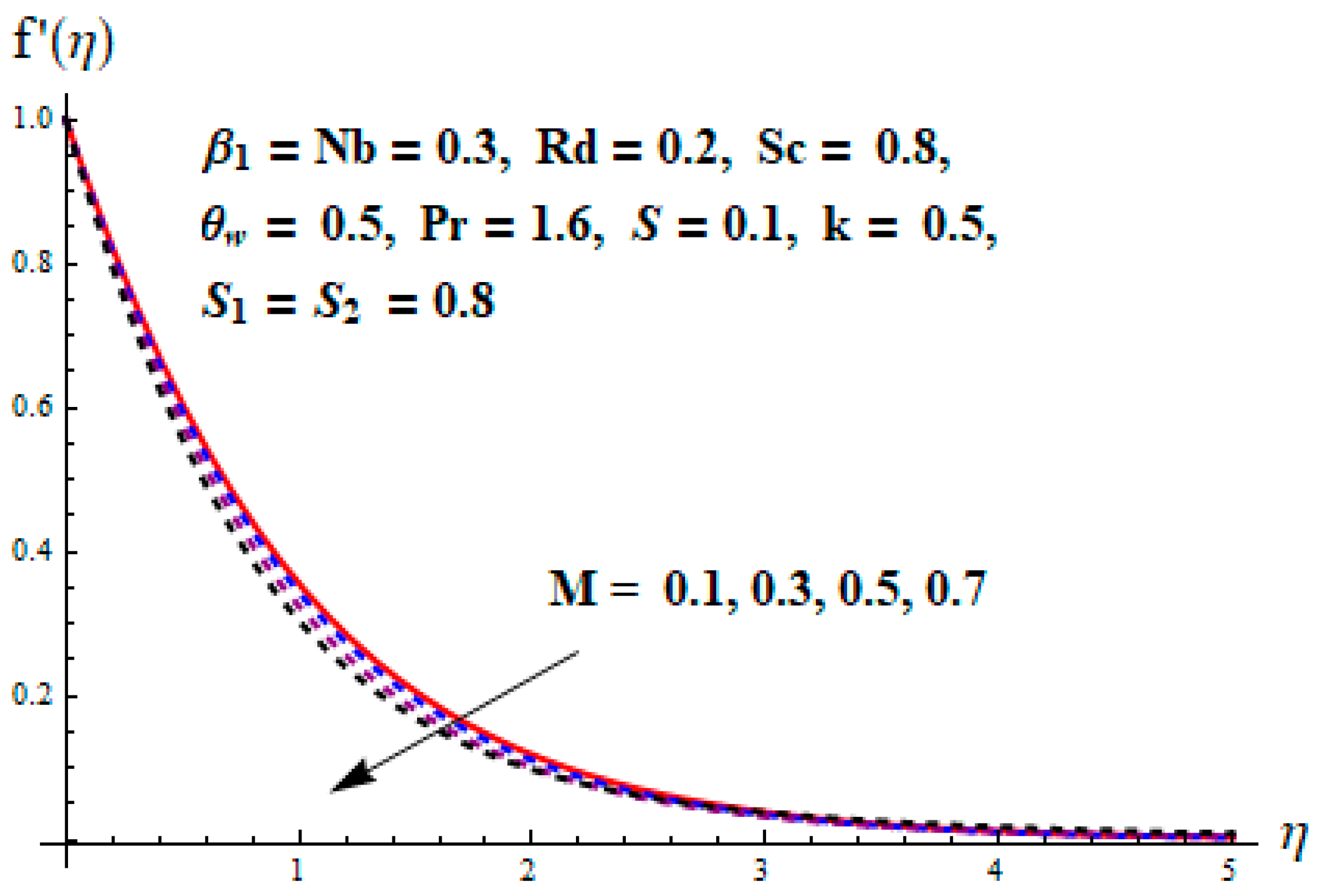
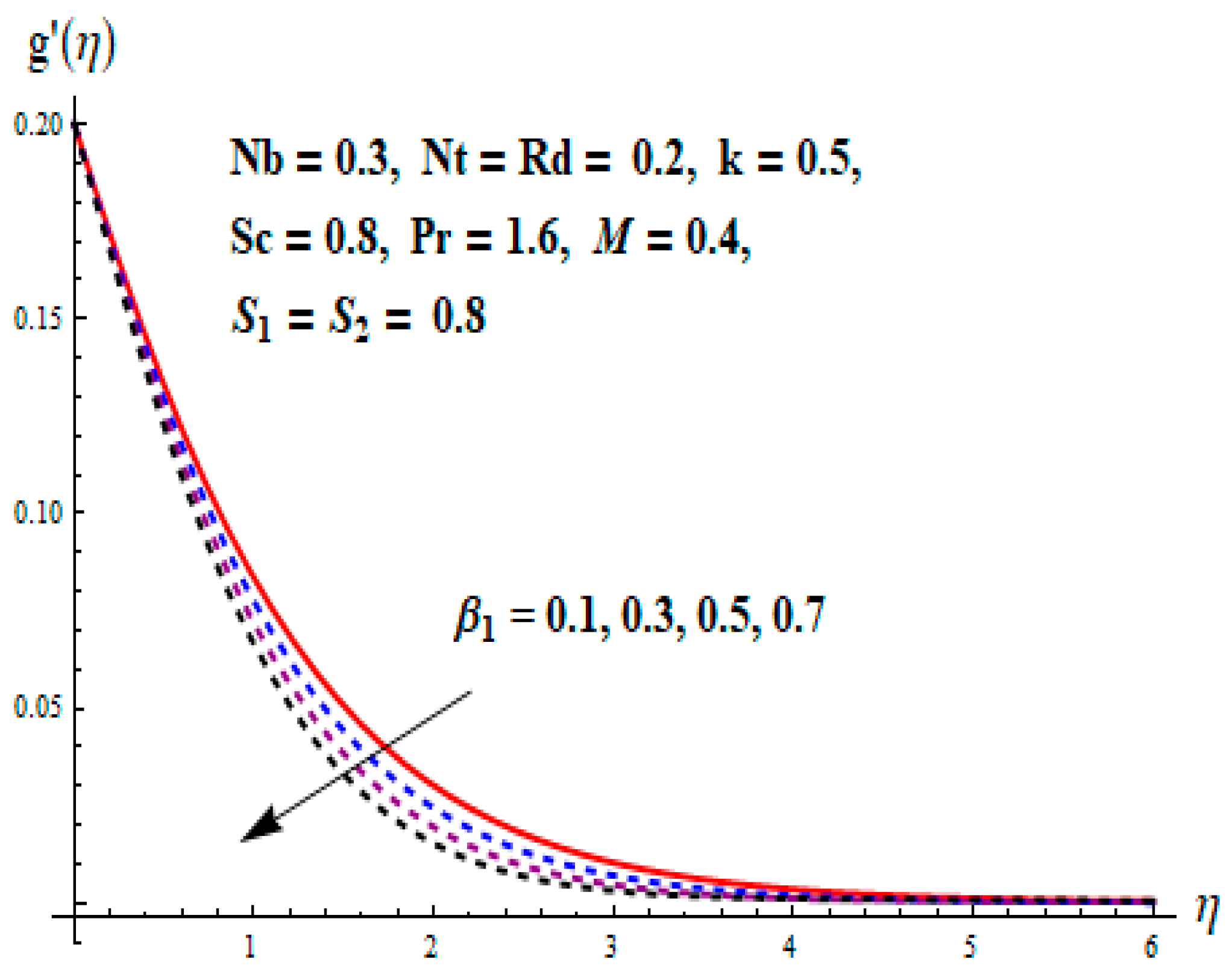
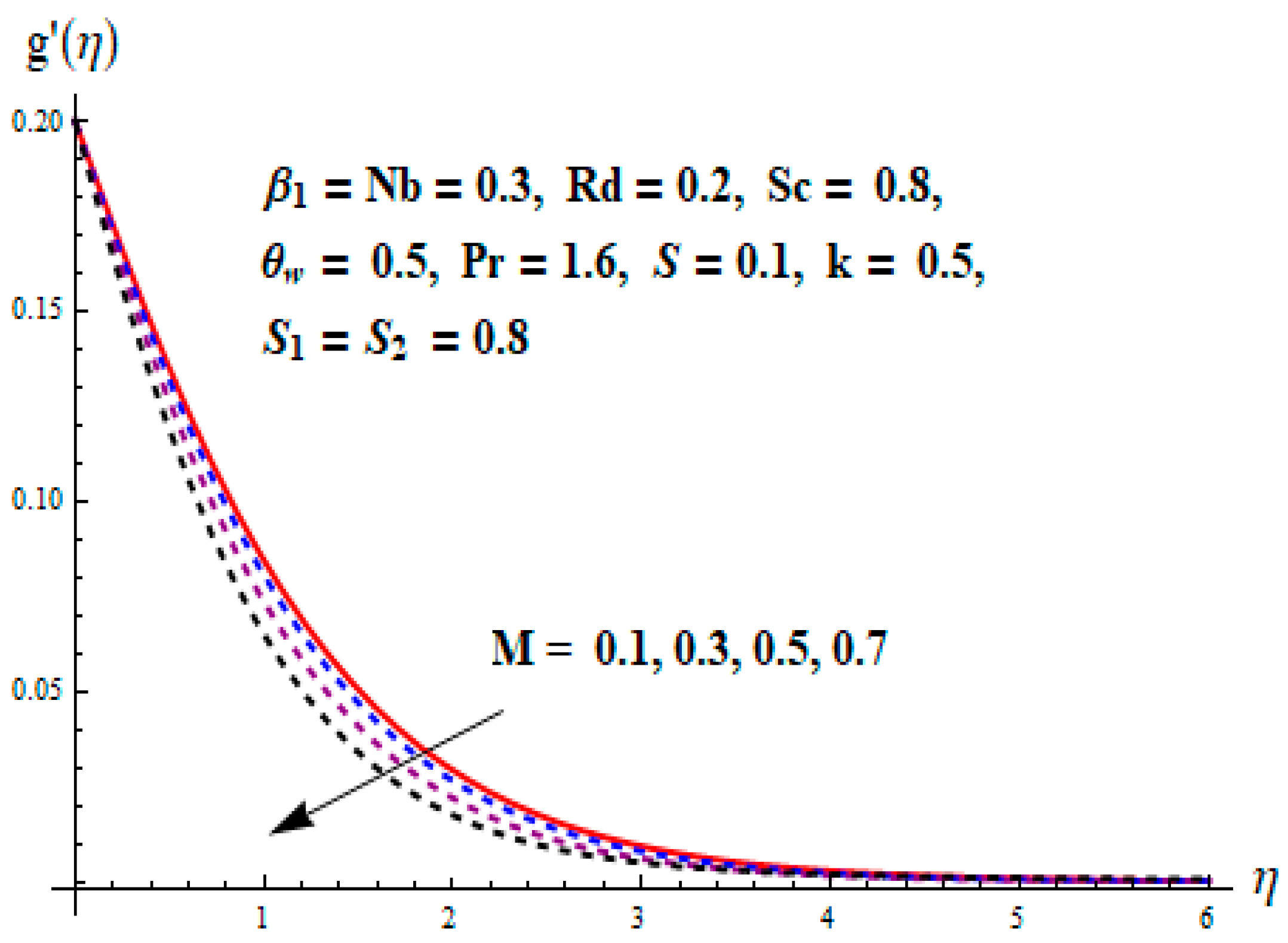
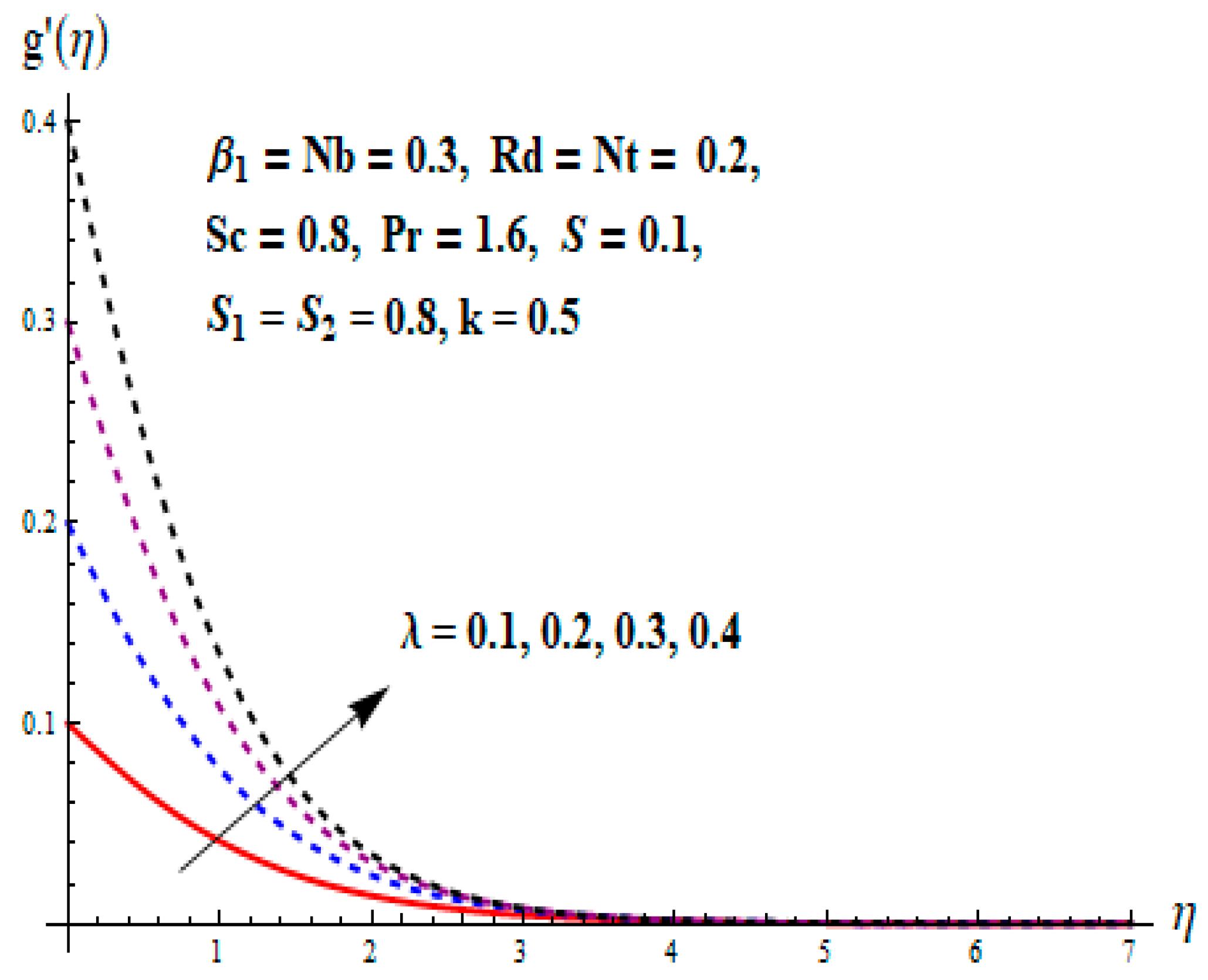
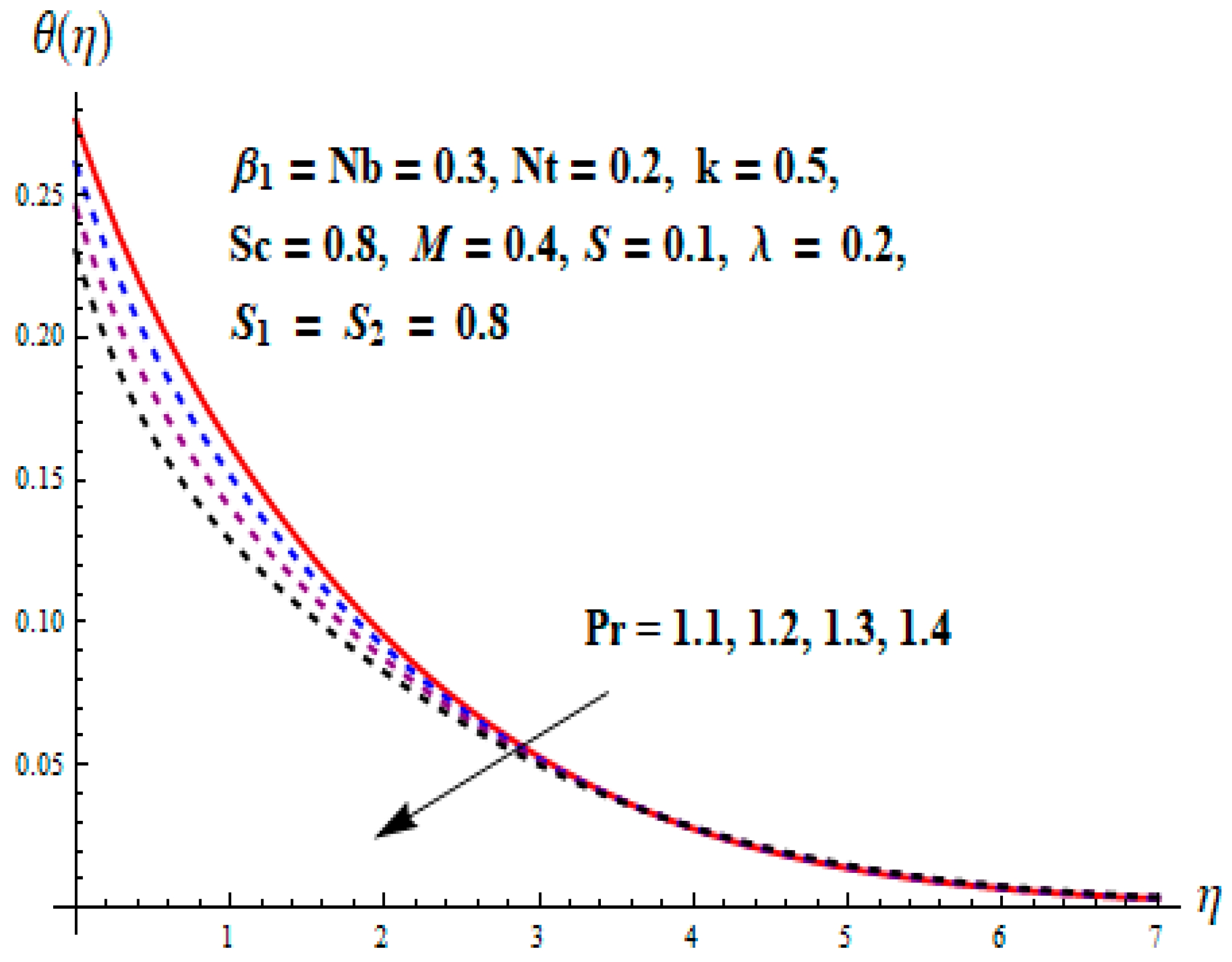
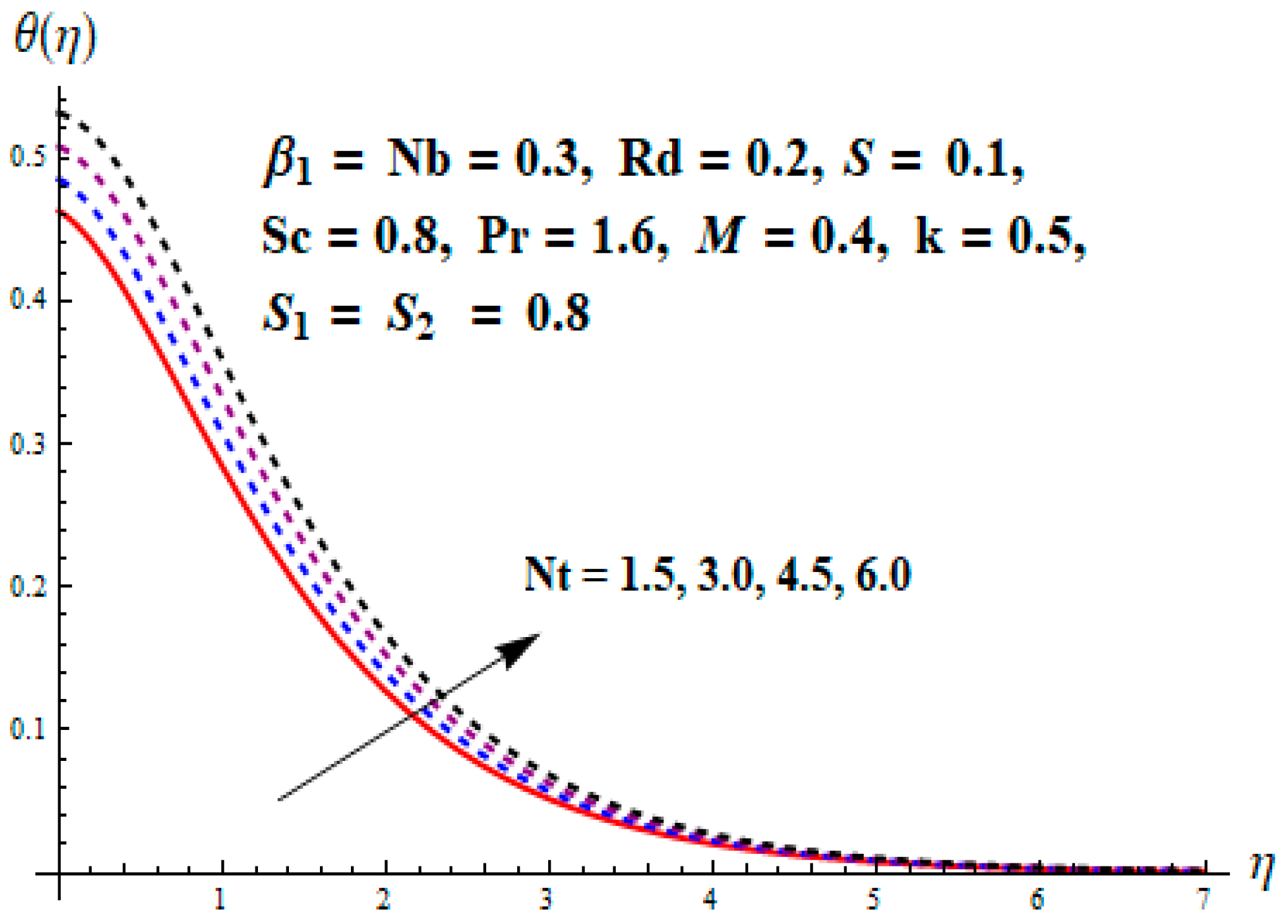
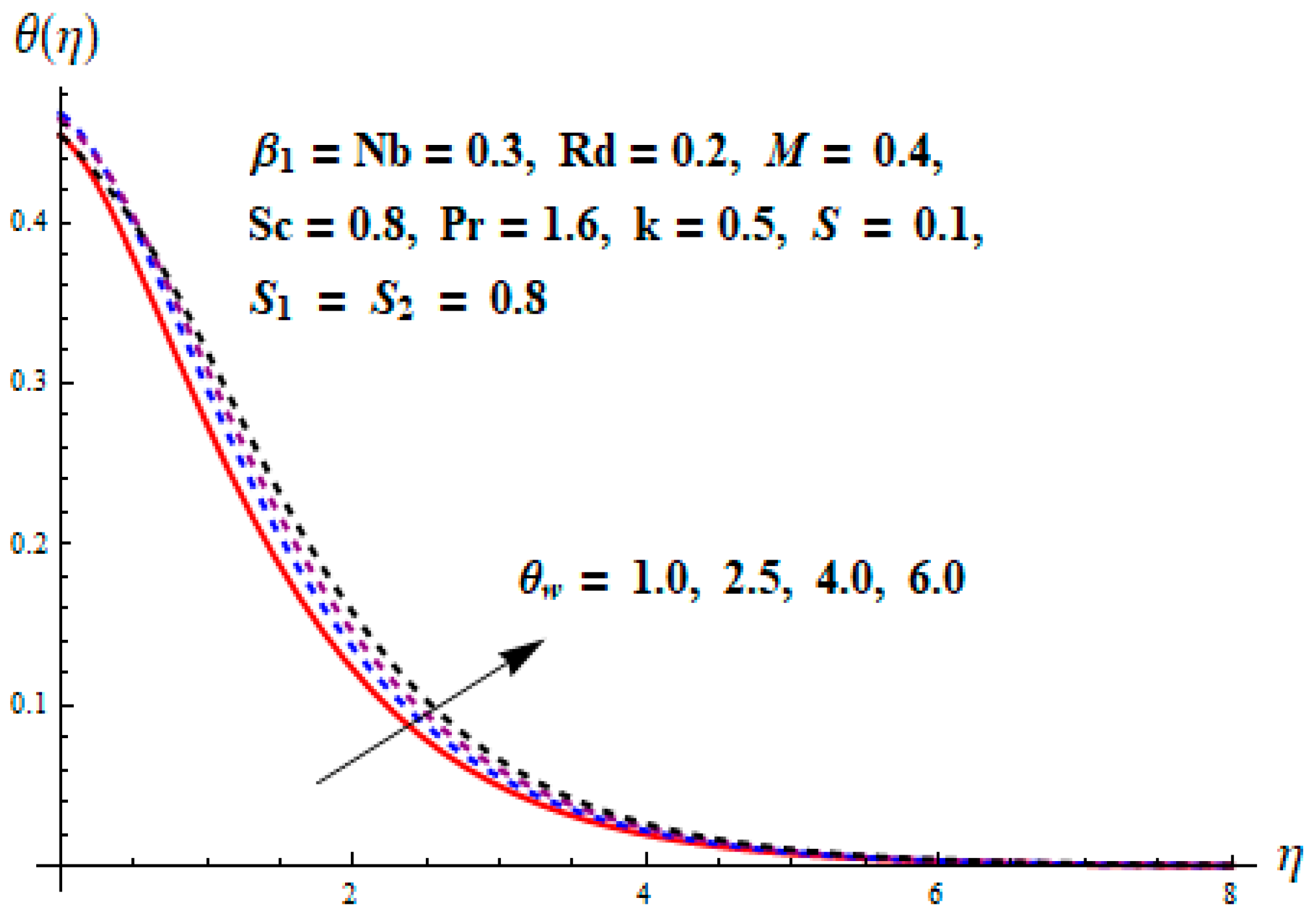
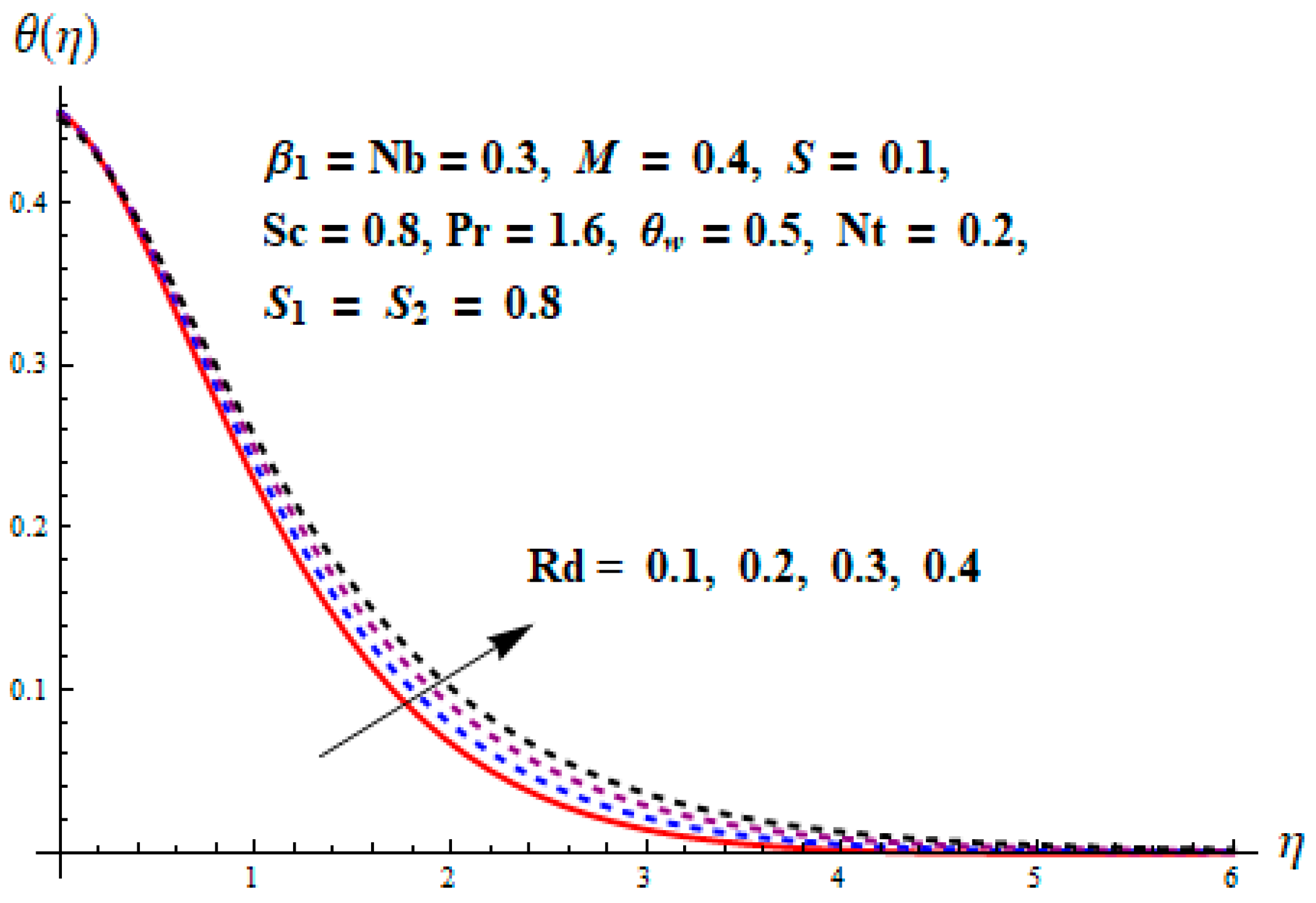
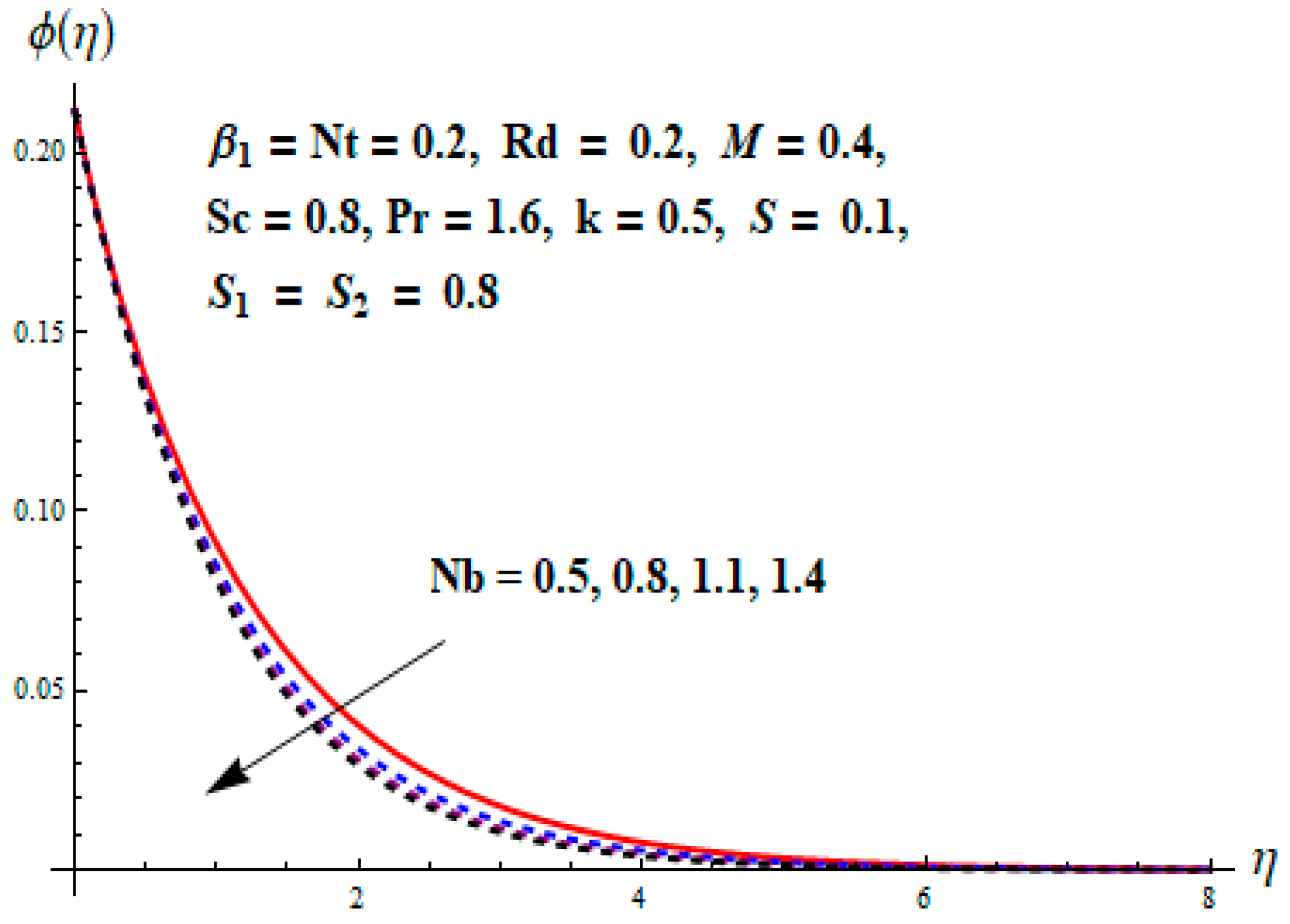
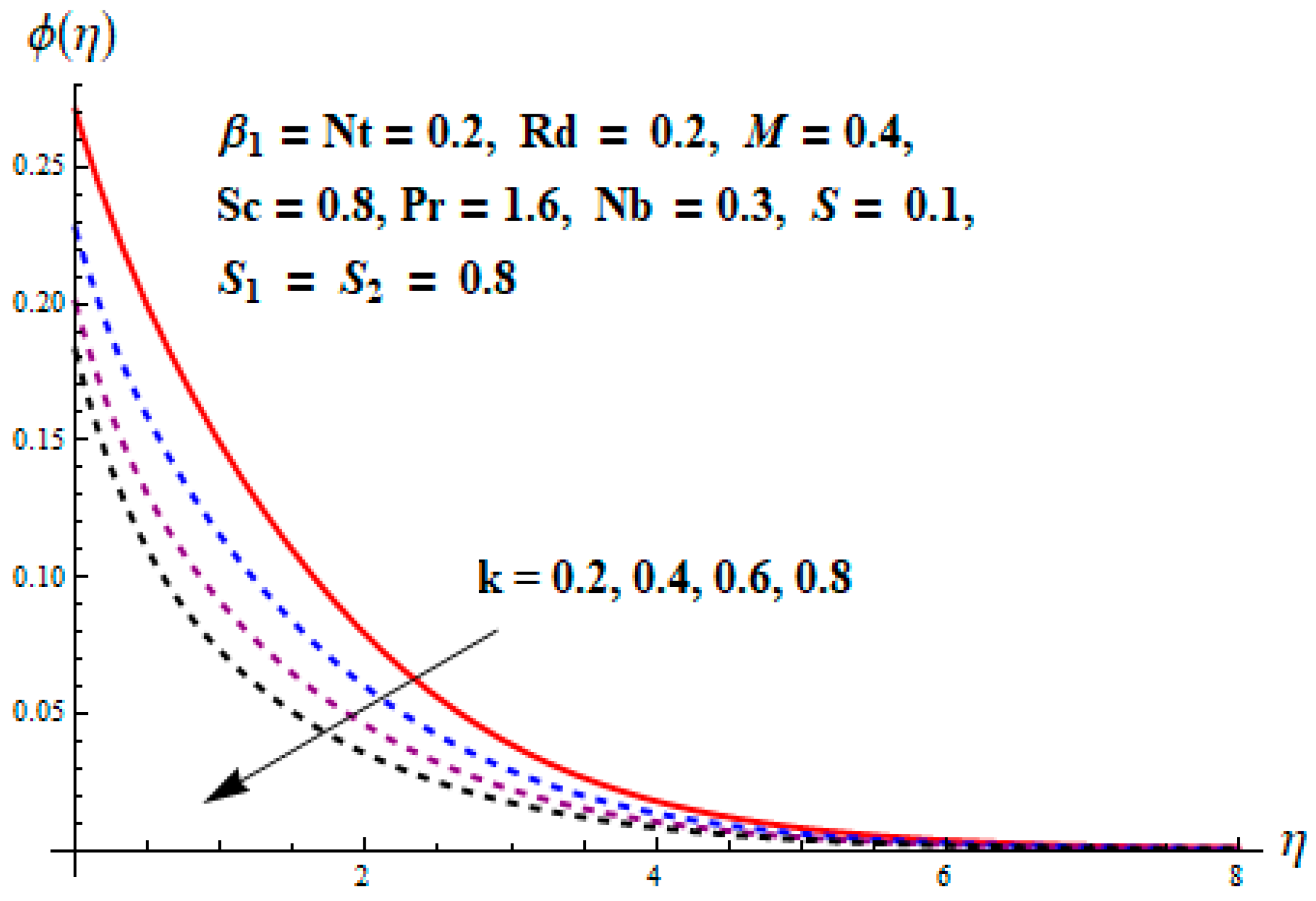
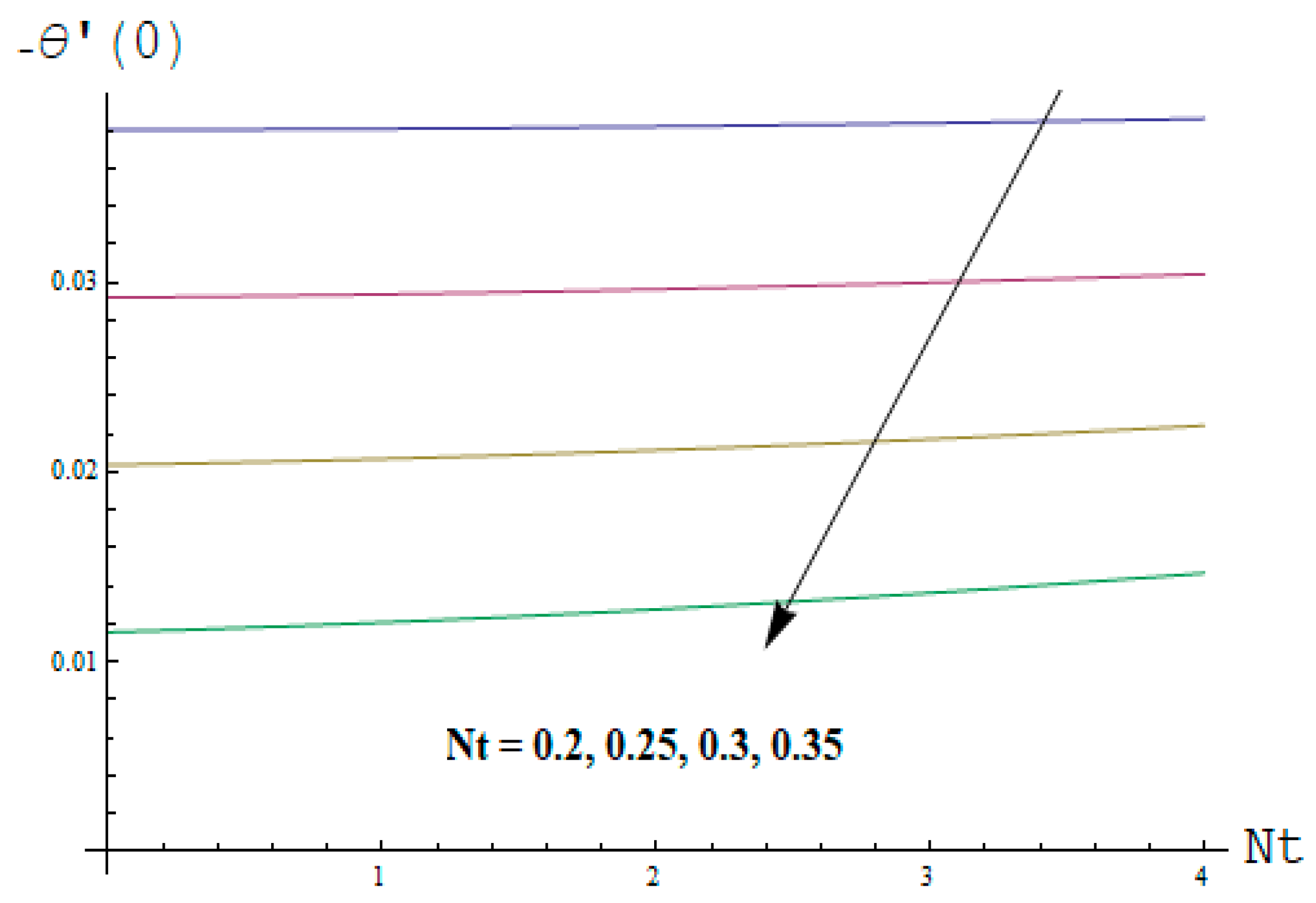
6. Results with Discussion
7. Concluding Remarks
- ➢
- Impacts of Brownian motion on the concentration and temperature distributions are different.
- ➢
- Strong magnetic impact hinders the fluid velocity.
- ➢
- Enhancing the estimation of Deborah’s number will lead to an enhancement in the velocity distributions.
- ➢
- Concentration profile declines for the large estimates of the chemical reaction parameter.
- ➢
- The thermally stratified parameter reduces the temperature field.
- ➢
- Fluid temperature is enhanced for nonlinear radiative heat flux.
- ➢
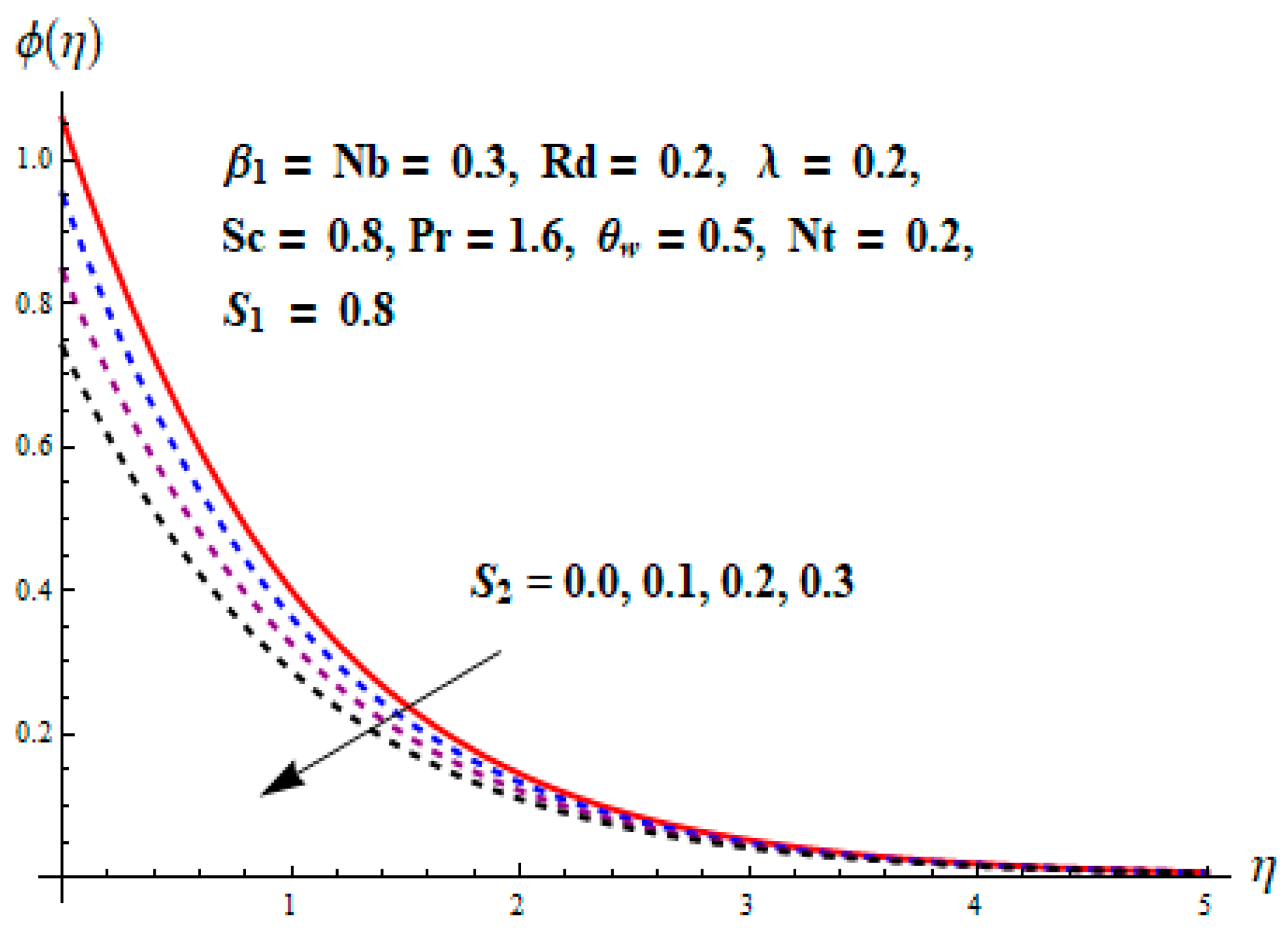
- The parameter of solutal stratification decreases the concentration distribution.
- ➢
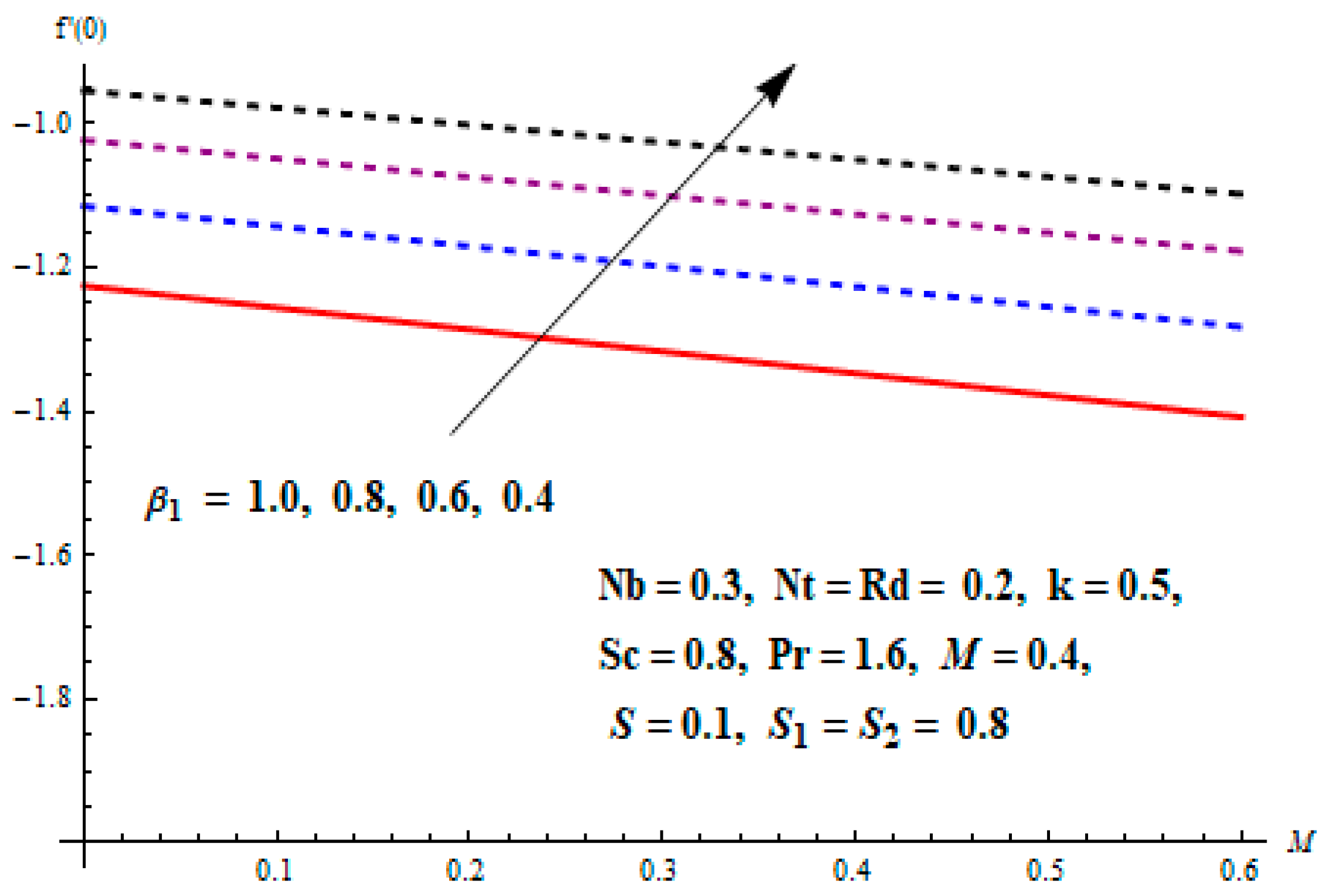
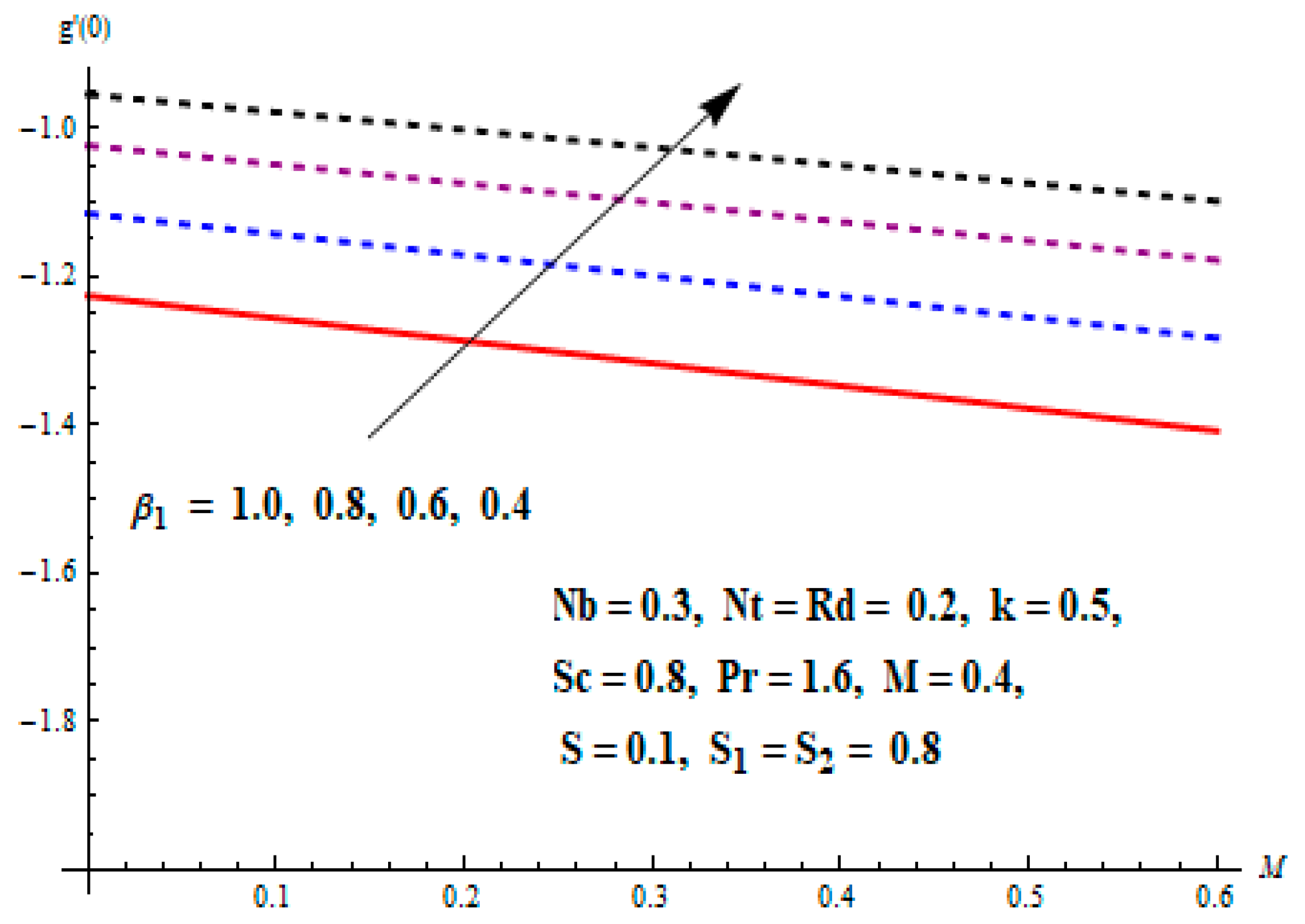
- The heat transfer rate reduces for mounting estimations of and .
- ➢
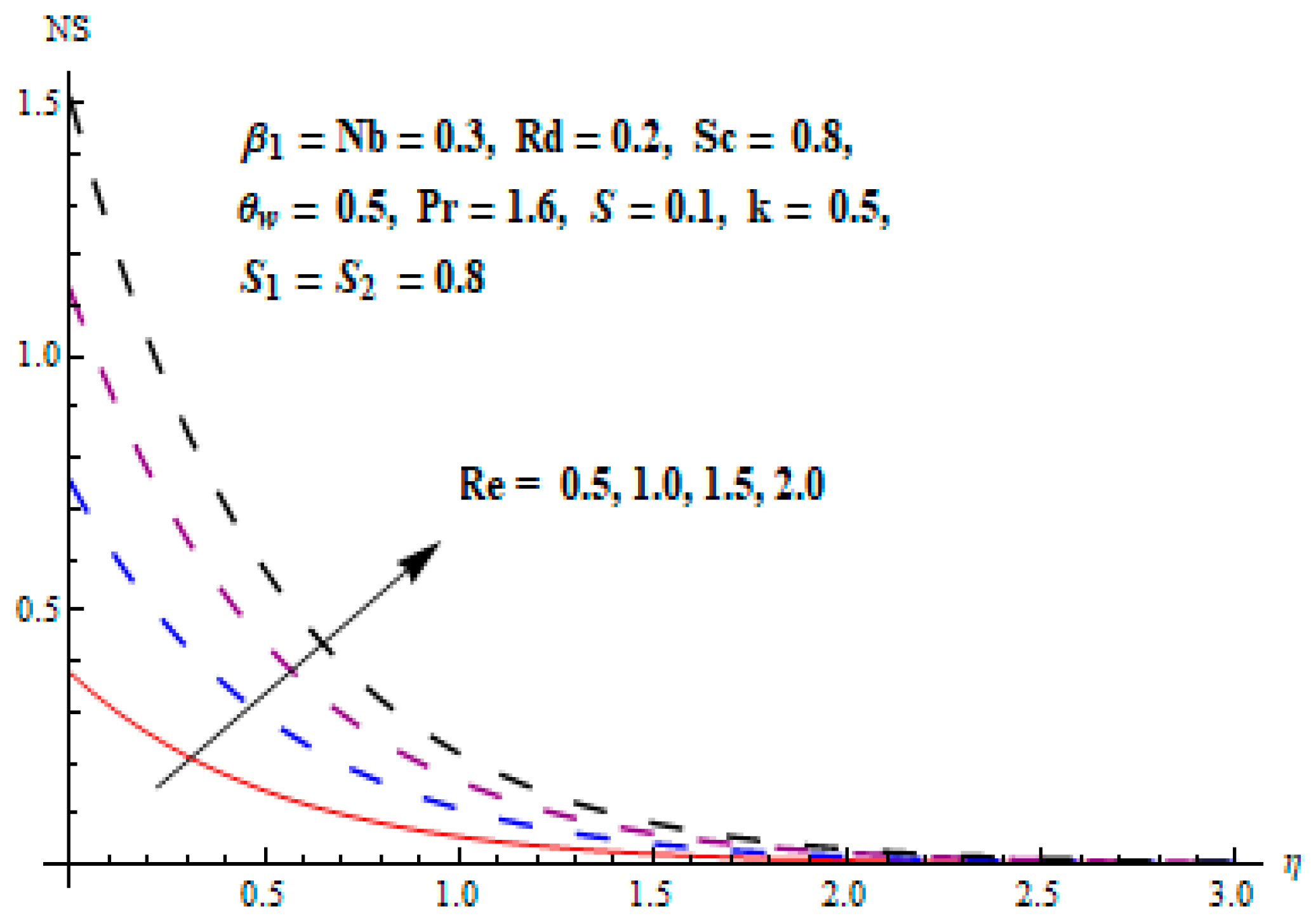
- Large estimates of Reynolds and Brinkman numbers boost the entropy of the system.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Coordinate axis | |
| Velocity components | |
| Magnetic field strength | |
| Dynamic viscosity | |
| Fluid parameter | |
| Base fluid’s density | |
| Radiative heat flux | |
| Kinematic viscosity | |
| Fluid’s heat capacity | |
| Heat generation/absorption coefficient | |
| Fluid temperature | |
| Specific heat capacity | |
| Thermal diffusivity of fluid | |
| Thermal conductivity | |
| Temperature away from the surface | |
| Nanoparticles concentration | |
| Concentration away from the surface | |
| Coefficient of mean absorption | |
| Local Reynolds number | |
| Reaction rate | |
| Reference concentration | |
| Convective fluid temperature | |
| Convective fluid concentration | |
| Stefan-Boltzman constant | |
| Wall heat flux | |
| Thermophoretic diffusion coefficient | |
| Chemical reaction parameter | |
| Magnetic parameter | |
| Deborah number expressing relaxation time | |
| Ratio parameter for temperature | |
| Effective heat capacity of nanoparticles | |
| Brownian diffusivity coefficient | |
| Heat absorption/generation parameter | |
| Non-dimensional temperature | |
| Non-dimensional concentration | |
| Non-dimensional velocity | |
| Schmidt number | |
| Similarity variable | |
| Reference temperature | |
| Brownian motion | |
| Thermophoresis parameter | |
| Prandtl number | |
| Parameter for radiation | |
| Nusselt number | |
| Parameter of thermal stratification | |
| Parameter of concentration stratification | |
| Brinkmann number | |
| Dimensionless entropy number | |
| Dimensionless entropy generation rate | |
| Hartmann number | |
| Volumetric entropy generation per unit length |
References
- Muhammad, T.; Alsaedi, A.; Shehzad, S.A.; Hayat, T. A revised model for Darcy-Forchheimer flow of Maxwell nanofluid subject to convective boundary condition. Chin. J. Phys. 2017, 55, 963–976. [Google Scholar] [CrossRef]
- Hussain, T.; Hussain, S.; Hayat, T. Impact of double stratification and magnetic field in the mixed convective radiative flow of Maxwell nanofluid. J. Mol. Liq. 2016, 220, 870–878. [Google Scholar] [CrossRef]
- Bai, Y.; Liu, X.; Zhang, Y.; Zhang, M. Stagnation-point heat and mass transfer of MHD Maxwell nanofluids over a stretching surface in the presence of thermophoresis. J. Mol. Liq. 2016, 224, 1172–1180. [Google Scholar] [CrossRef]
- Jusoh, R.; Nazar, R.; Pop, I. Flow and heat transfer of magnetohydrodynamic three-dimensional Maxwell nanofluid over a permeable stretching/shrinking surface with convective boundary conditions. Int. J. Mech. Sci. 2017, 124, 166–173. [Google Scholar] [CrossRef]
- Hayat, T.; Qayyum, S.; Waqas, M.; Alsaedi, A. Thermally radiative stagnation point flow of Maxwell nanofluid due to unsteady convectively heated stretched surface. J. Mol. Liq. 2016, 224, 801–810. [Google Scholar] [CrossRef]
- Farooq, U.; Lu, D.; Munir, S.; Ramzan, M.; Suleman, M.; Hussain, S. MHD flow of Maxwell fluid with nanomaterials due to an exponentially stretching surface. Sci. Rep. 2019, 9, 1–11. [Google Scholar] [CrossRef]
- Ramzan, M.; Bilal, M.; Chung, J.D. Soret and Dufour effects on three dimensional upper-convected Maxwell fluid with chemical reaction and non-linear radiative heat flux. Int. J. Chem. React. Eng. 2017, 15, 3. [Google Scholar] [CrossRef]
- Aziz, A.; Shams, M. Entropy generation in MHD Maxwell nanofluid flow with variable thermal conductivity, thermal radiation, slip conditions, and heat source. AIP Adv. 2020, 10, 015038. [Google Scholar] [CrossRef]
- Ramzan, M.; Bilal, M.; Chung, J.D.; Farooq, U. Mixed convective flow of Maxwell nanofluid past a porous vertical stretched surface—An optimal solution. Result. Phys. 2016, 6, 1072–1079. [Google Scholar] [CrossRef]
- Ramzan, M.; Bilal, M.; Chung, J.D. Influence of homogeneous-heterogeneous reactions on MHD 3D Maxwell fluid flow with Cattaneo-Christov heat flux and convective boundary condition. J. Mol. Liq. 2017, 230, 415–422. [Google Scholar] [CrossRef]
- Rehman, K.U.; Al-Mdallal, Q.M.; Malik, M.Y. Symmetry analysis on thermally magnetized fluid flow regime with heat source/sink. Case Stud. Therm. Eng. 2019, 14, 100452. [Google Scholar] [CrossRef]
- Aman, S.; Khan, I.; Ismail, Z.; Salleh, M.Z.; Al-Mdallal, Q.M. Heat transfer enhancement in free convection flow of CNTs Maxwell nanofluids with four different types of molecular liquids. Sci. Rep. 2017, 7, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Khan, M.; Salahuddin, T.; Tanveer, A.; Malik, M.Y.; Hussain, A. Change in internal energy of thermal diffusion stagnation point Maxwell nanofluid flow along with solar radiation and thermal conductivity. Chin. J. Chem. Eng. 2019, 27, 2352–2358. [Google Scholar] [CrossRef]
- Ganesh, N.V.; Kameswaran, P.K.; Al-Mdallal, Q.M.; Hakeem, A.K.; Ganga, B. Non-Linear thermal radiative marangoni boundary layer flow of gamma Al2O3 nanofluids past a stretching sheet. J. Nanofluid. 2018, 7, 944–950. [Google Scholar] [CrossRef]
- Ganesh, N.V.; Chamkha, A.J.; Al-Mdallal, Q.M.; Kameswaran, P.K. Magneto-Marangoni nano-boundary layer flow of water and ethylene glycol based γ Al2O3 nanofluids with non-linear thermal radiation effects. Case Stud. Therm. Eng. 2018, 12, 340–348. [Google Scholar] [CrossRef]
- Ganesh, N.V.; Al-Mdallal, Q.M.; Reena, K.; Aman, S. Blasius and Sakiadis slip flow of H2O–C2H6O2 (50: 50) based nanoliquid with different geometry of boehmite alumina nanoparticles. Case Stud. Therm. Eng. 2019, 16, 100546. [Google Scholar] [CrossRef]
- Abdelsalam, S.; Bhatti, M. Anomalous reactivity of thermo-bioconvective nanofluid towards oxytactic microorganisms. Appl. Math. Mech. 2020. [Google Scholar] [CrossRef]
- Yang, L.; Huang, J.N.; Ji, W.; Mao, M. Investigations of a new combined application of nanofluids in heat recovery and air purification. Powder Techol. 2020, 360, 956–966. [Google Scholar] [CrossRef]
- Sohail, M.; Naz, R.; Abdelsalam, S.I. On the onset of entropy generation for a nanofluid with thermal radiation and gyrotactic microorganisms through 3D flows. Phys. Scr. 2020, 95, 045206. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Bhatti, M.M. New insight into AuNP applications in tumour treatment and cosmetics through wavy annuli at the nanoscale. Sci. Rep. 2019, 9, 1–14. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Bhatti, M.M. The study of non-Newtonian nanofluid with Hall and ion slip effects on peristaltically induced motion in a non-uniform channel. RSC Adv. 2018, 8, 7904–7915. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Jafaryar, M.; Shafee, A.; Babazadeh, H. Acceleration of discharge process of clean energy storage unit with insertion of porous foam considering nanoparticle enhanced paraffin. J. Clean. Prod. 2020, 261, 121206. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Rezaeianjouybari, B.; Darzi, M.; Shafee, A.; Li, Z.; Nguyen, T.K. Application of nano-refrigerant for boiling heat transfer enhancement employing an experimental study. Int. J. Heat Mass Transf. 2019, 141, 974–980. [Google Scholar] [CrossRef]
- Elmaboud, Y.A.; Abdelsalam, S.I.; Mekheimer, K.S.; Vafai, K. Electromagnetic flow for two-layer immiscible fluids. Eng. Sci. Technol. Int. J. 2019, 22, 237–248. [Google Scholar] [CrossRef]
- Eldesoky, I.M.; Abdelsalam, S.I.; El-Askary, W.A.; El-Refaey, A.M.; Ahmed, M.M. Joint effect of magnetic field and heat transfer on particulate fluid suspension in a catheterized wavy tube. BioNanoScience 2019, 9, 723–739. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Bhatti, M.M.; Zeeshan, A.; Riaz, A.; Bég, O.A. Metachronal propulsion of a magnetised particle-fluid suspension in a ciliated channel with heat and mass transfer. Phys. Scr. 2019, 94, 115301. [Google Scholar] [CrossRef]
- Eldesoky, I.M.; Abdelsalam, S.I.; El-Askary, W.A.; Ahmed, M.M. Concurrent Development of Thermal Energy with Magnetic Field on a Particle-Fluid Suspension Through a Porous Conduit. BioNanoScience 2019, 9, 186–202. [Google Scholar] [CrossRef]
- Aman, S.; Al-Mdallal, Q.; Khan, I. Heat transfer and second order slip effect on MHD flow of fractional Maxwell fluid in a porous medium. J. King Saud Univ.-Sci. 2020, 32, 450–458. [Google Scholar] [CrossRef]
- Ganesh, N.V.; Al-Mdallal, Q.M.; Kameswaran, P.K. Numerical study of MHD effective Prandtl number boundary layer flow of γ Al2O3 nanofluids past a melting surface. Case Stud. Therm. Eng. 2019, 13, 100413. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Bhatti, M.M. The impact of impinging TiO2 nanoparticles in Prandtl nanofluid along with endoscopic and variable magnetic field effects on peristaltic blood flow. Multidiscip. Model. Mat. Struct. 2018, 14, 530–548. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Vafai, K. Combined effects of magnetic field and rheological properties on the peristaltic flow of a compressible fluid in a microfluidic channel. Eur. J. Mech.-B/Fluid. 2017, 65, 398–411. [Google Scholar] [CrossRef]
- Elkoumy, S.R.; Barakat, E.I.; Abdelsalam, S.I. Hall and transverse magnetic field effects on peristaltic flow of a Maxwell fluid through a porous medium. Global J. Pure Appl. Math 2013, 9, 187–203. [Google Scholar]
- Mekheimer, K.S.; Komy, S.R.; Abdelsalam, S.I. Simultaneous effects of magnetic field and space porosity on compressible Maxwell fluid transport induced by a surface acoustic wave in a microchannel. Chin. Phys. B 2013, 22, 124702. [Google Scholar] [CrossRef]
- Ibrahim, W.; Makinde, O.D. The effect of double stratification on boundary-layer flow and heat transfer of nanofluid over a vertical plate. Comput. Fluids 2013, 86, 433–441. [Google Scholar] [CrossRef]
- Abbasi, F.M.; Shehzad, S.A.; Hayat, T.; Ahmad, B. Doubly stratified mixed convection flow of Maxwell nanofluid with heat generation/absorption. J. Magn. Magn. Mater. 2016, 404, 159–165. [Google Scholar] [CrossRef]
- Srinivasacharya, D.; Upendar, M. Effect of double stratification on MHD free convection in a micropolar fluid. J. Egypt. Math. Soc. 2013, 21, 370–378. [Google Scholar] [CrossRef]
- Hayat, T.; Hussain, T.; Shehzad, S.A.; Alsaedi, A. Thermal and concentration stratifications effects in radiative flow of Jeffrey fluid over a stretching sheet. PLoS ONE 2014, 9, e107858. [Google Scholar] [CrossRef]
- Mahanthesh, B.; Gireesha, B.J.; Raju, C.S.K. Cattaneo-Christov heat flux on UCM nanofluid flow across a melting surface with double stratification and exponential space dependent internal heat source. Inform. Med. Unlocked 2017, 9, 26–34. [Google Scholar] [CrossRef]
- Ramzan, M.; Gul, H.; Chung, J.D. Double stratified radiative Jeffery magneto nanofluid flow along an inclined stretched cylinder with chemical reaction and slip condition. Eur. Phys. J. Plus 2017, 132, 456. [Google Scholar] [CrossRef]
- Ramzan, M.; Ullah, N.; Chung, J.D.; Lu, D.; Farooq, U. Buoyancy effects on the radiative magneto Micropolar nanofluid flow with double stratification, activation energy and binary chemical reaction. Sci. Rep. 2017, 7, 12901. [Google Scholar] [CrossRef]
- Alshomrani, A.S.; Ramzan, M. Upshot of magnetic dipole on the flow of nanofluid along a stretched cylinder with gyrotactic microorganism in a stratified medium. Phys. Scr. 2019, 95, 025702. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.M.; Rashidi, M.M.; Hafidzuddin, E.H.; Wahi, N. Magnetohydrodynamics (MHD) stagnation point flow past a shrinking/stretching surface with double stratification effect in a porous medium. J. Therm. Anal. Calorim. 2020, 139, 3635–3648. [Google Scholar]
- Ramzan, M.; Shaheen, N. Thermally stratified Darcy Forchheimer nanofluid flow comprising carbon nanotubes with effects of Cattaneo-Christov heat flux and homogeneous-heterogeneous reactions. Phys. Scr. 2019, 95, 015701. [Google Scholar] [CrossRef]
- Ramzan, M.; Bilal, M.; Kanwal, S.; Chung, J.D. Effects of variable thermal conductivity and non-linear thermal radiation past an Eyring Powell nanofluid flow with chemical Reaction. Commun. Theor. Phys. 2017, 67, 723. [Google Scholar] [CrossRef]
- Ramzan, M.; Chung, J.D.; Ullah, N. Radiative magnetohydrodynamic nanofluid flow due to gyrotactic microorganisms with chemical reaction and non-linear thermal radiation. Int. J. Mech. Sci. 2017, 130, 31–40. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, T.; Alsaedi, A.; Alhuthali, M.S. Magnetohydrodynamic three-dimensional flow of viscoelastic nanofluid in the presence of nonlinear thermal radiation. J. Magn. Magn. Mater. 2015, 385, 222–229. [Google Scholar] [CrossRef]
- Lu, D.; Ramzan, M.; Bilal, M.; Chung, J.D.; Farooq, U.; Tahir, S. On three-dimensional MHD Oldroyd-B fluid flow with nonlinear thermal radiation and homogeneous–heterogeneous reaction. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 387. [Google Scholar] [CrossRef]
- Bilal, M.; Ramzan, M. Hall current effect on unsteady rotational flow of carbon nanotubes with dust particles and nonlinear thermal radiation in Darcy–Forchheimer porous media. J. Ther. Anal. Calorim 2019, 138, 3127–3137. [Google Scholar] [CrossRef]
- Lu, D.C.; Ramzan, M.; Bilal, M.; Chung, J.D.; Farooq, U. Upshot of chemical species and nonlinear thermal radiation on Oldroyd-B nanofluid flow past a bi-directional stretched surface with heat generation/absorption in a porous media. Commun. Theor. Phys. 2018, 70, 071. [Google Scholar] [CrossRef]
- Suleman, M.; Ramzan, M.; Zulfiqar, M.; Bilal, M.; Shafee, A.; Chung, J.D.; Farooq, U. Entropy analysis of 3D non-Newtonian MHD nanofluid flow with nonlinear thermal radiation past over exponential stretched surface. Entropy 2018, 20, 930. [Google Scholar] [CrossRef]
- Lu, D.; Ramzan, M.; Ahmad, S.; Shafee, A.; Suleman, M. Impact of nonlinear thermal radiation and entropy optimization coatings with hybrid nanoliquid flow past a curved stretched surface. Coatings 2018, 8, 430. [Google Scholar] [CrossRef]
- Lu, D.; Ramzan, M.; ul Huda, N.; Chung, J.D.; Farooq, U. Nonlinear radiation effect on MHD Carreau nanofluid flow over a radially stretching surface with zero mass flux at the surface. Sci. Rep. 2018, 8, 1–17. [Google Scholar] [CrossRef] [PubMed]
- Bejan, A. Second-law analysis in heat transfer and thermal design. In Advances in Heat Transfer; Elsevier: Amsterdam, The Netherlands, 1982; Volume 15, pp. 1–58. [Google Scholar]
- Lu, D.; Ramzan, M.; Mohammad, M.; Howari, F.; Chung, J.D. A thin film flow of nanofluid comprising carbon nanotubes influenced by Cattaneo-Christov heat flux and entropy generation. Coatings 2019, 9, 296. [Google Scholar] [CrossRef]
- Ramzan, M.; Mohammad, M.; Howari, F.; Chung, J.D. Entropy analysis of carbon nanotubes based nanofluid flow past a vertical cone with thermal radiation. Entropy 2019, 21, 642. [Google Scholar] [CrossRef]
- Weigand, B.; Birkefeld, A. Similarity solutions of the entropy transport equation. Int. J. Therm. Sci. 2009, 48, 1863–1869. [Google Scholar] [CrossRef]
- Hassan, M.; Mohyud-Din, S.T.; Ramzan, M. Study of heat transfer and entropy generation in ferrofluid under low oscillating magnetic field. Indian J. Phys. 2019, 93, 749–758. [Google Scholar] [CrossRef]
- Lai, H.Y.; Liu, C.C. Numerical analysis of entropy generation in mixed convection flow with viscous dissipation effects in vertical channel. Int. Commun. Heat Mass Transf. 2011, 38, 285–290. [Google Scholar]
- Tlili, I.; Ramzan, M.; Kadry, S.; Kim, H.W.; Nam, Y. Radiative MHD nanofluid flow over a moving thin needle with Entropy generation in a porous medium with dust particles and Hall current. Entropy 2020, 22, 354. [Google Scholar] [CrossRef]
- Ramzan, M.; Mohammad, M.; Howari, F. Magnetized suspended carbon nanotubes based nanofluid flow with bio-convection and entropy generation past a vertical cone. Sci. Rep. 2019, 9, 1–15. [Google Scholar] [CrossRef]
- Liao, S.J. The Proposed Homotopy Analysis Technique for the Solution of Nonlinear Problems. Ph.D. Thesis, Shanghai Jiao Tong University, Shanghai, China, 1992. [Google Scholar]
- Liao, S. Homotopy Analysis Method in Nonlinear Differential Equations; Beijing Higher Education Press: Beijing, China, 2012; pp. 153–165. [Google Scholar]
- Liao, S.J. An explicit, totally analytic approximation of Blasius’ viscous flow problems. Int. J. Non-Linear Mech. 1999, 34, 759–778. [Google Scholar] [CrossRef]
- Liao, S.J. Beyond Perturbation: Introduction to the Homotopy Analysis Method; Chapman & Hall/CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Elwakil, E.A.E.; Abdou, M.A. New applications of the homotopy analysis method. Z. Nat. A 2008, 63, 385–392. [Google Scholar] [CrossRef]
- Renuka, A.; Muthtamilselvan, M.; Doh, D.H.; Cho, G.R. Entropy analysis and nanofluid past a double stretchable spinning disk using Homotopy Analysis Method. Math. Comput. Simul. 2020, 171, 152–169. [Google Scholar] [CrossRef]
- Ghiasi, E.K.; Saleh, R. Homotopy analysis method for the Sakiadis flow of a thixotropic fluid. Eur. Phys. J. Plus 2019, 134, 32. [Google Scholar] [CrossRef]
- Biswal, U.; Chakraverty, S. Investigation of Jeffery-Hamel fow for nanofluid in the presence of magnetic field by a new approach in the Optimal Homotopy analysis method. J. Appl. Comput. Mech. 2020. [Google Scholar] [CrossRef]
- Sobamowo, M.G.; Yinusa, A.A.; Akinshilo, A.T. Homotopy analysis method to MHD-slip flow of an upper-convected maxwell viscoelastic nanofluid in a permeable channel embedded in a porous medium. Int. J. Petrochem. Sci. Eng. 2020, 5, 11–20. [Google Scholar]
- Ray, A.K.; Vasu, B.; Murthy, P.V.S.N.; Gorla, R.S. Non-similar solution of Eyring–Powell fluid flow and heat transfer with convective boundary condition: Homotopy Analysis Method. Int. J. Appl. Comput. Math. 2020, 6, 16. [Google Scholar] [CrossRef]
- Bilal, M.; Sagheer, M.; Hussain, S. Three dimensional MHD upper-convected Maxwell nanofluid flow with nonlinear radiative heat flux. Alexandria Eng. J. 2018, 57, 1917–1925. [Google Scholar] [CrossRef]
- Rosseland, S. Astrophysik: Auf Atomtheoretischer Grundlage; Springer: Berlin, Germany, 2013; Volume 11. [Google Scholar]
- Hayat, T.; Muhammad, T.; Shehzad, S.A.; Alsaedi, A. An analytical solution for magnetohydrodynamic Oldroyd-B nanofluid flow induced by a stretching sheet with heat generation/absorption. Int. J. Therm. Sci. 2017, 111, 274–288. [Google Scholar] [CrossRef]
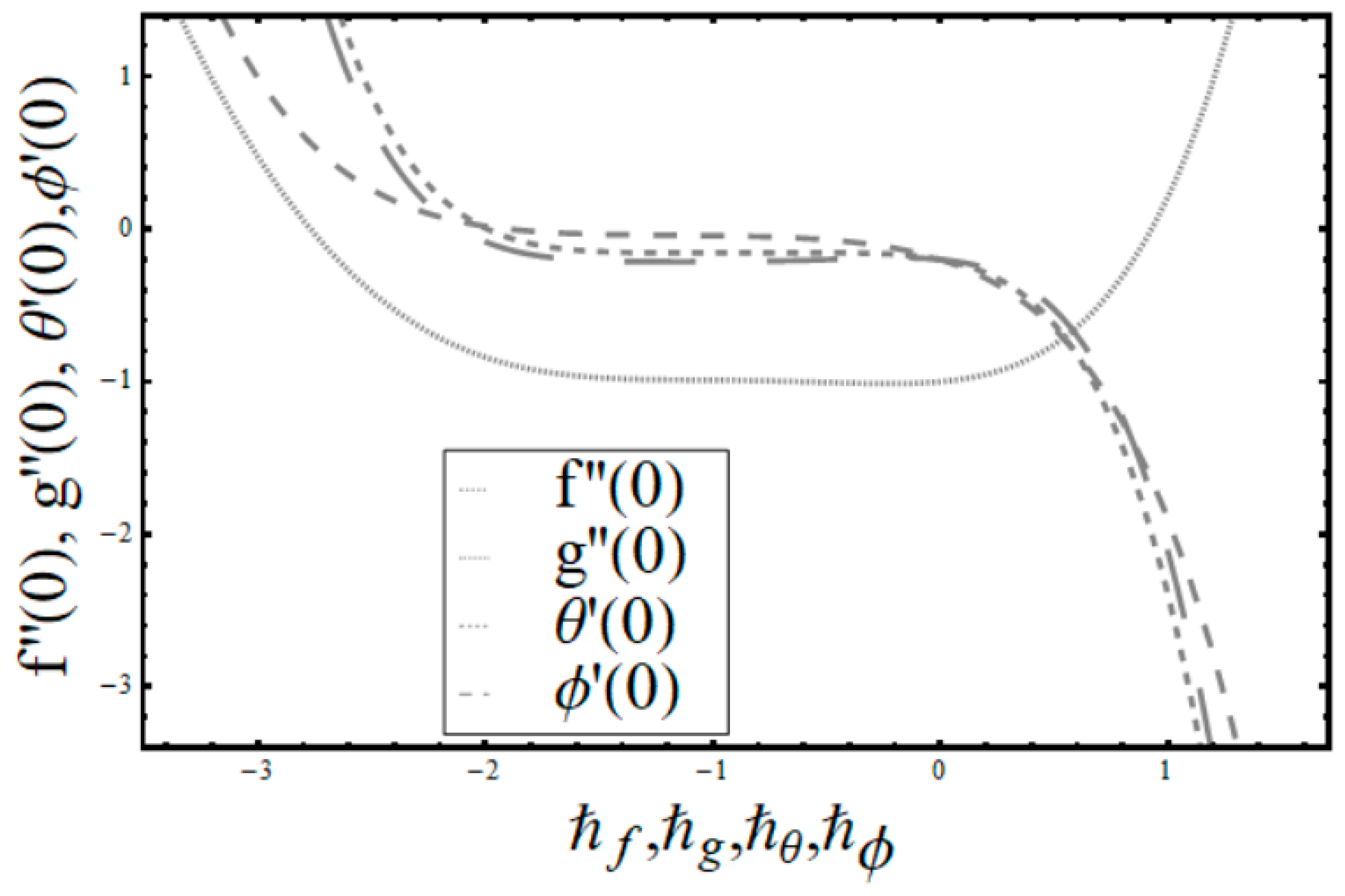







| Approximations Order | ||||
|---|---|---|---|---|
| 1 | 0.99750 | 0.15980 | 0.13620 | 0.18150 |
| 5 | 1.00200 | 0.15390 | 0.09212 | 0.20190 |
| 10 | 0.99880 | 0.15360 | 0.07378 | 0.21070 |
| 20 | 0.99660 | 0.15400 | 0.06439 | 0.21430 |
| 25 | 0.99660 | 0.15400 | 0.06439 | 0.21430 |
| Reference [71] | Present | Reference [71] | Present | Reference [71] | Present | Reference [71] | Present | |||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.5 | 2 | 1.0169493 | 1.0169463 | 0.6127148 | 0.6127137 | 0.5395022 | 0.5395012 | 0.3170918 | 0.3170911 |
| 4 | 1.6016506 | 1.6016498 | 1.0169493 | 1.0169473 | 0.9034844 | 0.9034812 | 0.5510692 | 0.5510653 | ||
| 7 | 2.2439298 | 2.2439277 | 1.4727082 | 1.4727065 | 1.3143535 | 0.3143525 | 0.8208336 | 0.8208313 | ||
| 10 | 2.7550740 | 2.7550733 | 1.8369216 | 1.8369208 | 1.6428361 | 1.6428345 | 1.0373929 | 1.0373912 | ||
| 1 | 0 | 7 | 1.8260260 | 1.8260243 | 1.2025347 | 1.2025333 | 1.0737145 | 1.0737134 | 0.6725491 | 0.6725478 |
| 0.3 | 2.0940290 | 2.0940256 | 1.3782398 | 1.3782376 | 1.2305095 | 1.2305067 | 0.7701323 | 0.7701314 | ||
| 0.6 | 2.3129240 | 2.3129212 | 1.5154397 | 1.5154387 | 1.3609931 | 1.3609911 | 0.8434082 | 0.8434046 | ||
| 1 | 2.5591800 | 2.5591777 | 1.6644187 | 1.6644165 | 1.4836290 | 1.4836267 | 0.9204030 | 0.9204012 | ||
| 0 | 0.5 | 7 | 2.3543502 | 2.3543499 | 1.5922506 | 1.5302056 | 1.4275931 | 1.4275911 | 0.9108748 | 0.9108735 |
| 0.5 | 2.2966491 | 2.2966478 | 1.5302076 | 1.5302054 | 1.3684014 | 1.3684004 | 0.8638003 | 0.8638000 | ||
| 1.0 | 2.2439499 | 2.2439477 | 1.4727082 | 1.4727057 | 1.3143533 | 1.3143501 | 0.8208334 | 0.8208325 | ||
| 1.5 | 2.1950047 | 2.1950034 | 1.4200796 | 1.4200773 | 1.2649215 | 1.2649201 | 0.7818260 | 0.7818245 | ||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tlili, I.; Naseer, S.; Ramzan, M.; Kadry, S.; Nam, Y. Effects of Chemical Species and Nonlinear Thermal Radiation with 3D Maxwell Nanofluid Flow with Double Stratification—An Analytical Solution. Entropy 2020, 22, 453. https://doi.org/10.3390/e22040453
Tlili I, Naseer S, Ramzan M, Kadry S, Nam Y. Effects of Chemical Species and Nonlinear Thermal Radiation with 3D Maxwell Nanofluid Flow with Double Stratification—An Analytical Solution. Entropy. 2020; 22(4):453. https://doi.org/10.3390/e22040453
Chicago/Turabian StyleTlili, Iskander, Sania Naseer, Muhammad Ramzan, Seifedine Kadry, and Yunyoung Nam. 2020. "Effects of Chemical Species and Nonlinear Thermal Radiation with 3D Maxwell Nanofluid Flow with Double Stratification—An Analytical Solution" Entropy 22, no. 4: 453. https://doi.org/10.3390/e22040453
APA StyleTlili, I., Naseer, S., Ramzan, M., Kadry, S., & Nam, Y. (2020). Effects of Chemical Species and Nonlinear Thermal Radiation with 3D Maxwell Nanofluid Flow with Double Stratification—An Analytical Solution. Entropy, 22(4), 453. https://doi.org/10.3390/e22040453








