Analytical Analysis of Heat Transfer and Entropy Generation in a Tube Filled with Double-Layer Porous Media
Abstract
1. Introduction
2. Problem Descriptions
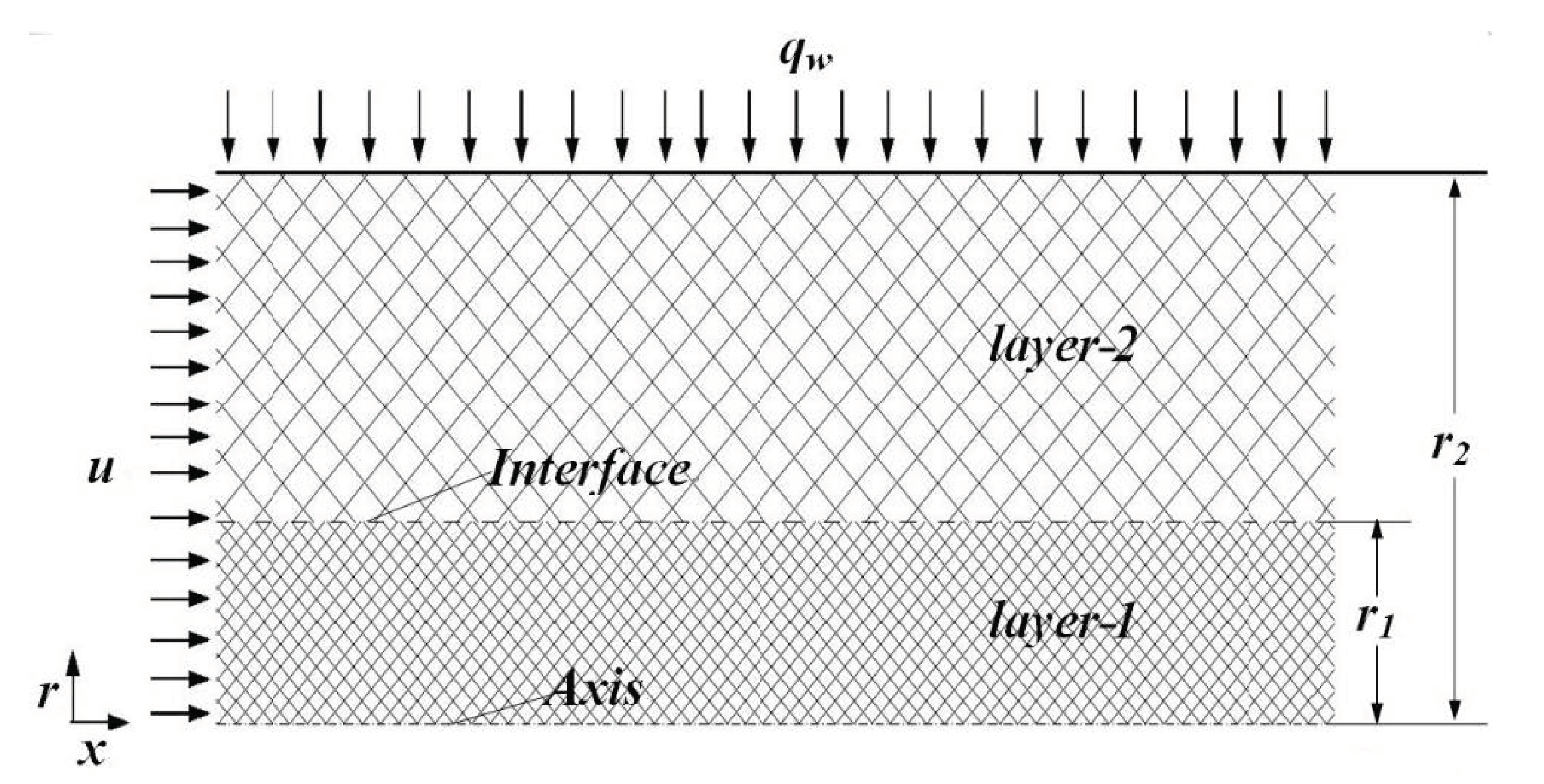
2.1. Physical Problem
- Each porous layer is homogenous and isotropic.
- The flow and heat transfer are steady and fully developed.
- The pertinent physical properties are constant.
- The gravity is neglected.
- The no-slip condition is used for the velocity boundary condition.
- The internal heat source in the energy equation is not considered.
2.2. Governing Equations
2.3. Boundary Conditions
2.4. Normalization
3. Analytical Solutions
3.1. Velocity Solutions
3.2. Temperature Distribution
3.3. Dimensionless Parameters
3.4. Entropy Generation Rate
4. Results and Discussion
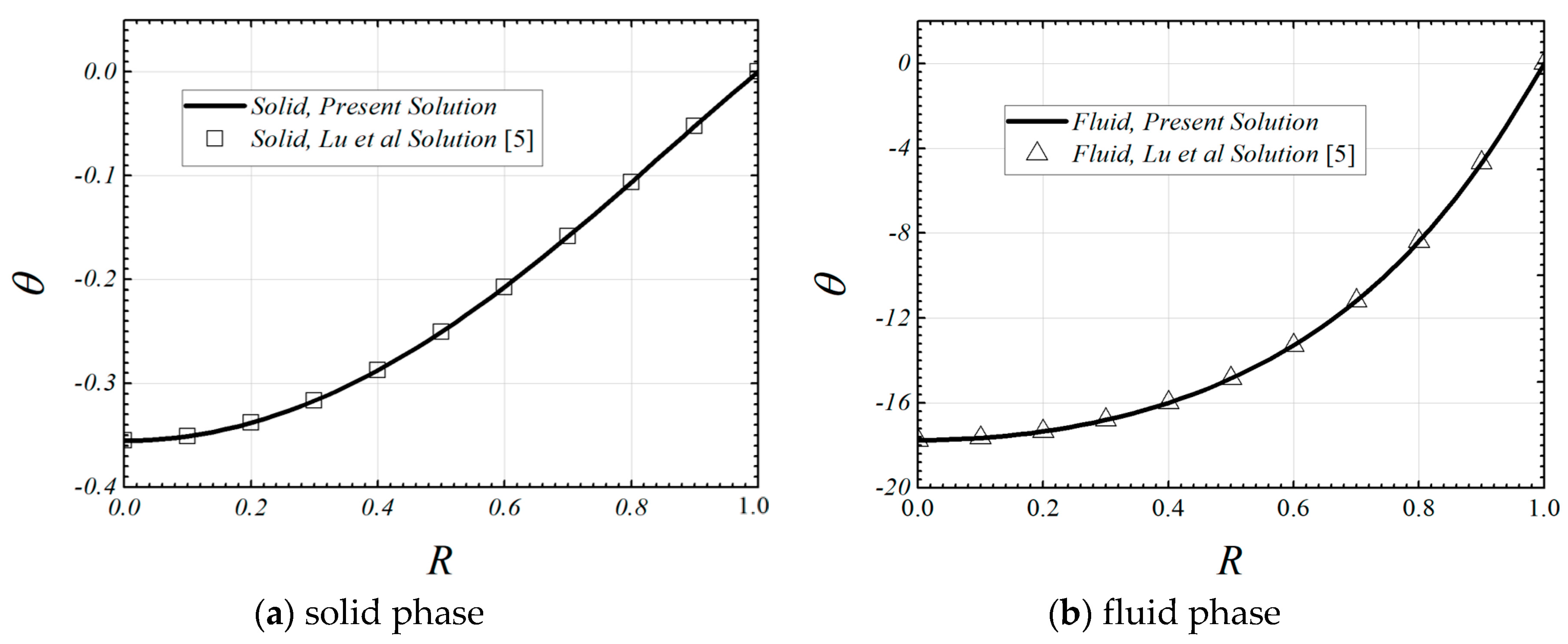
4.1. Validation of Solutions
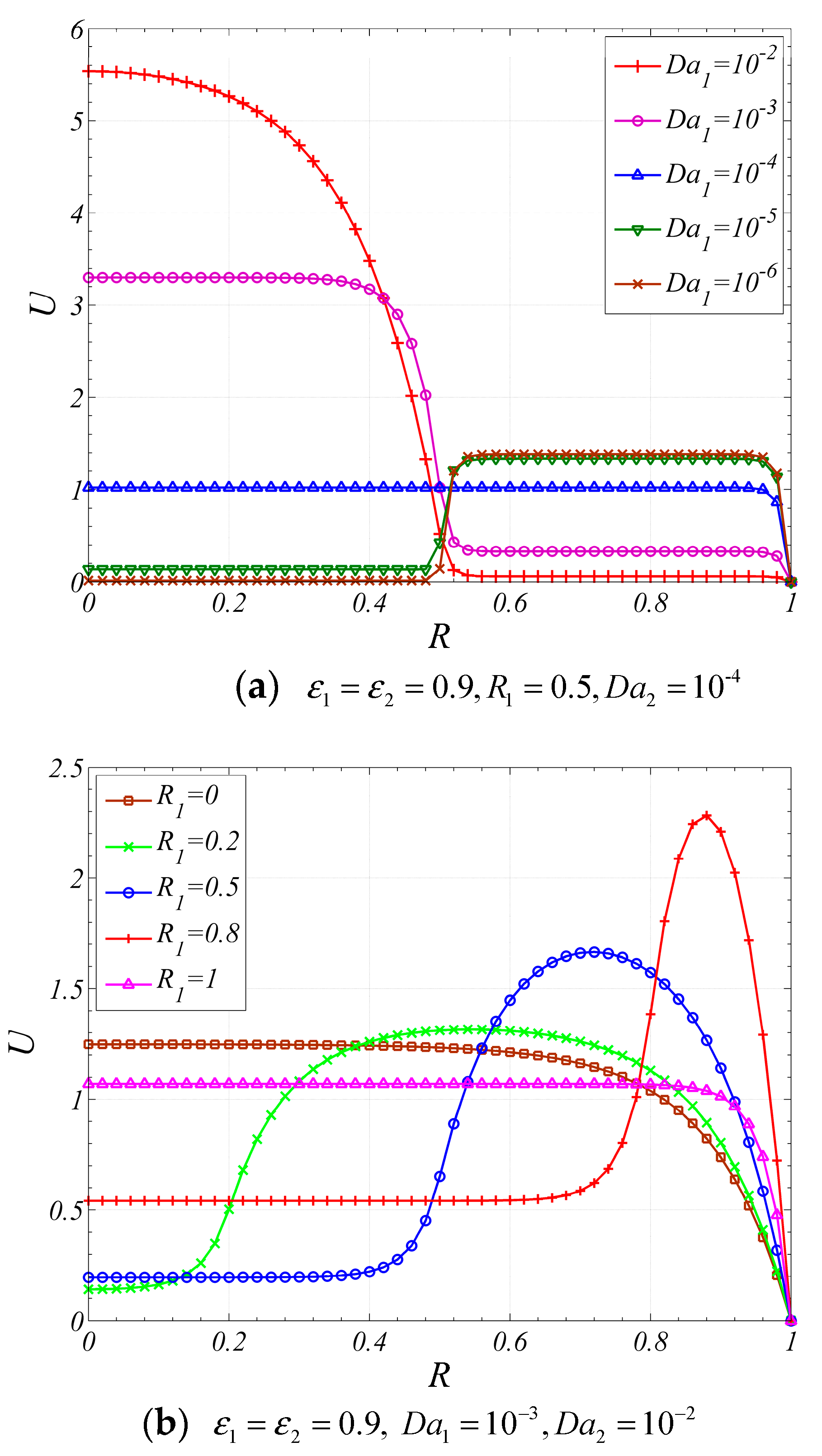
4.2. Velocity Distribution
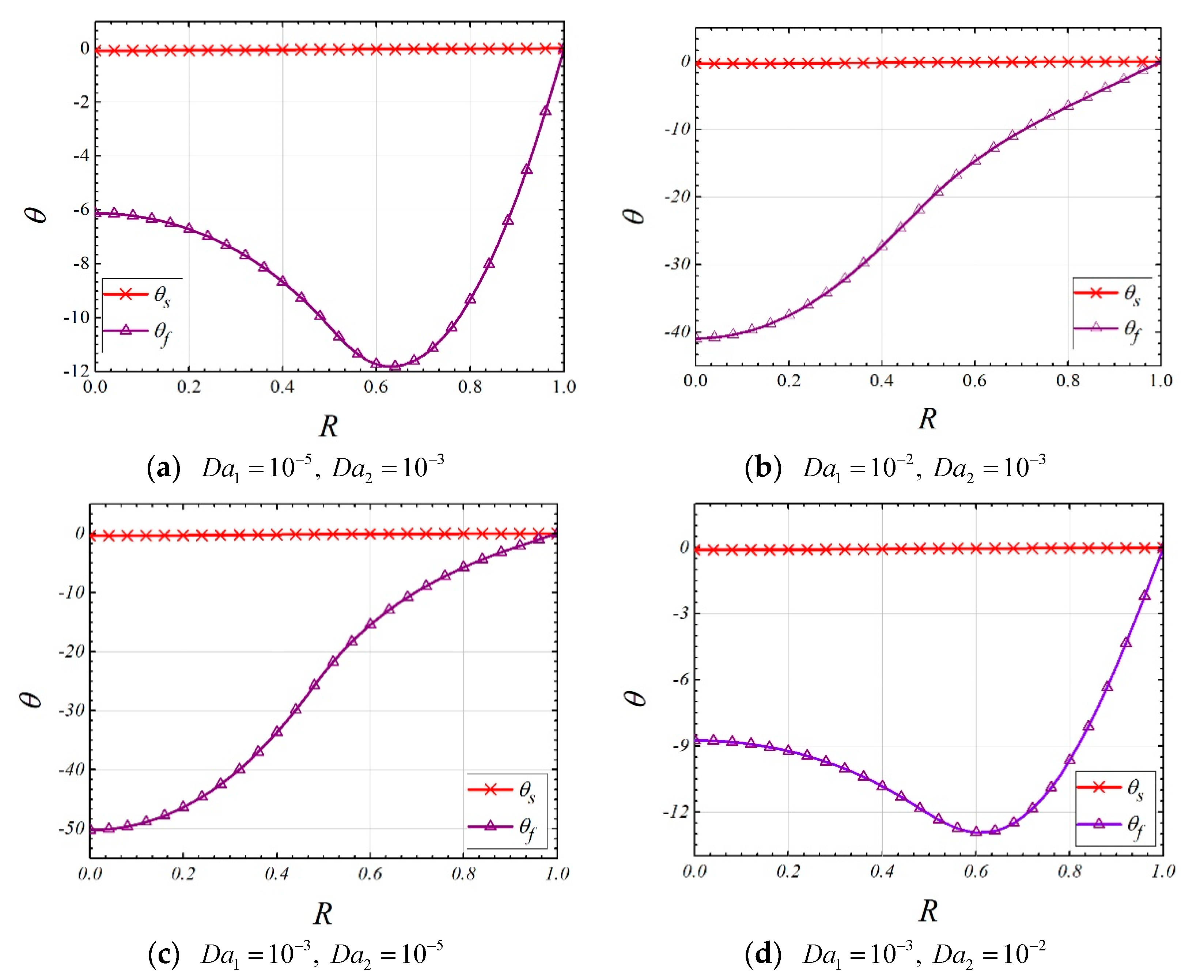
4.3. Temperature Distribution
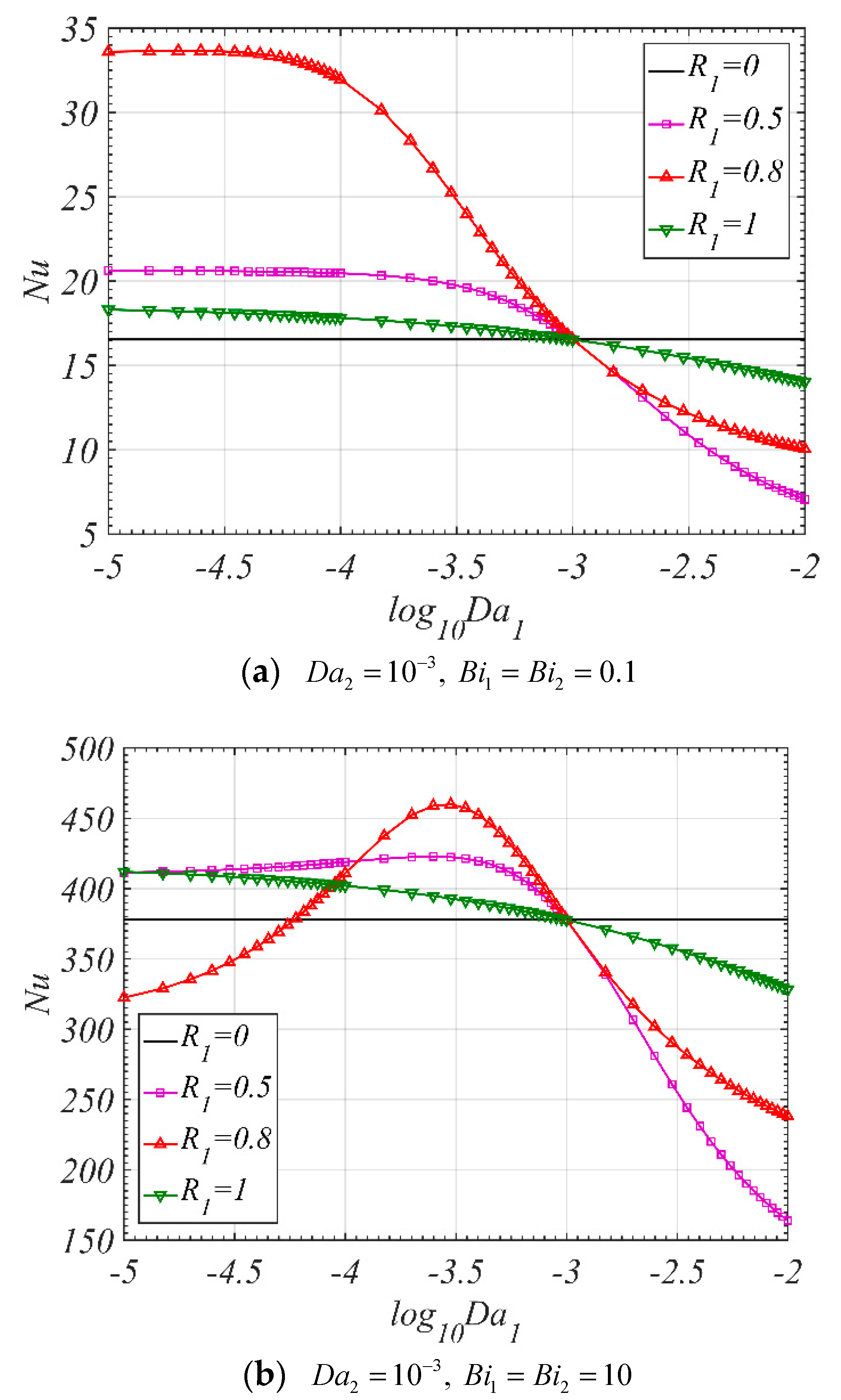
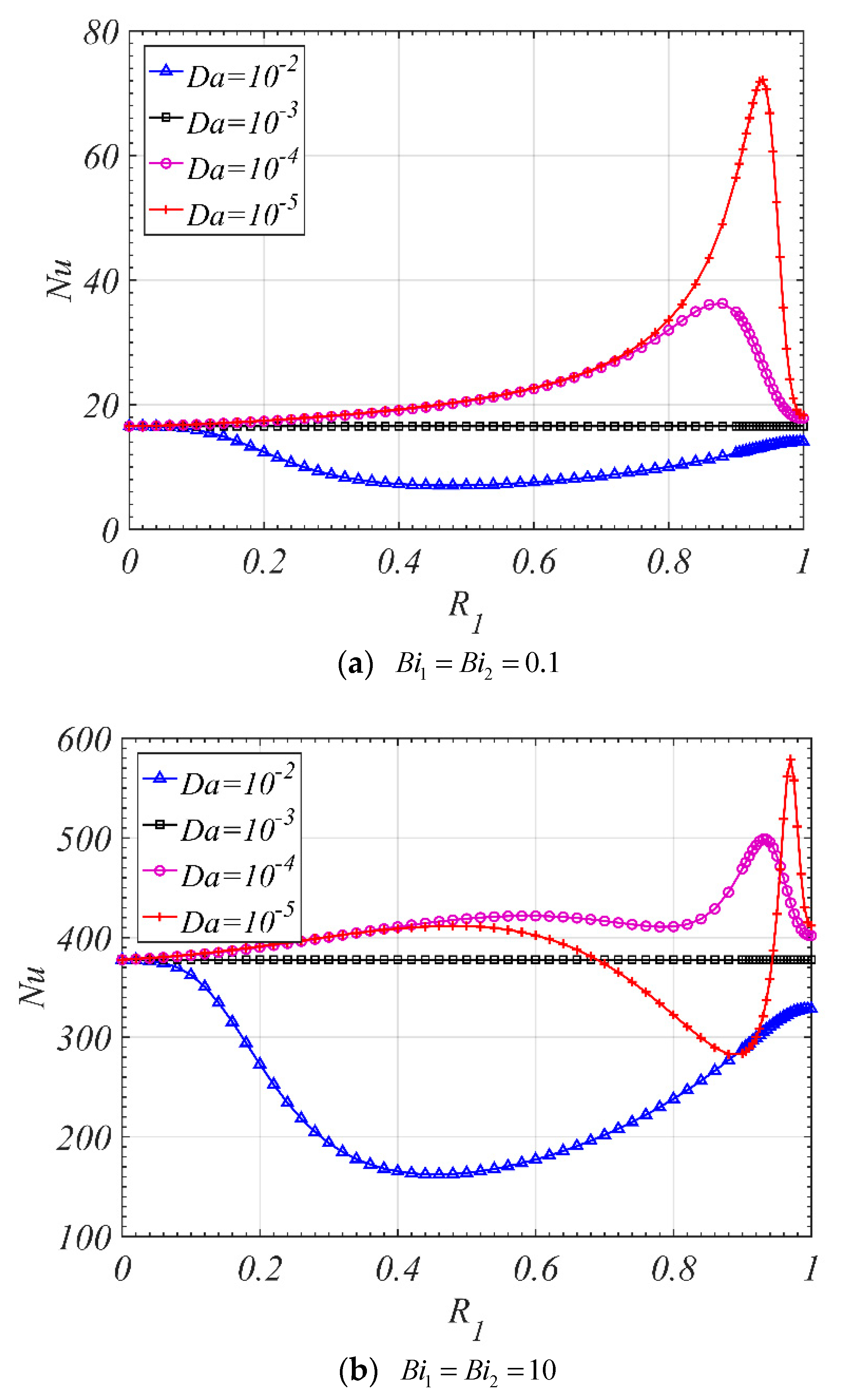
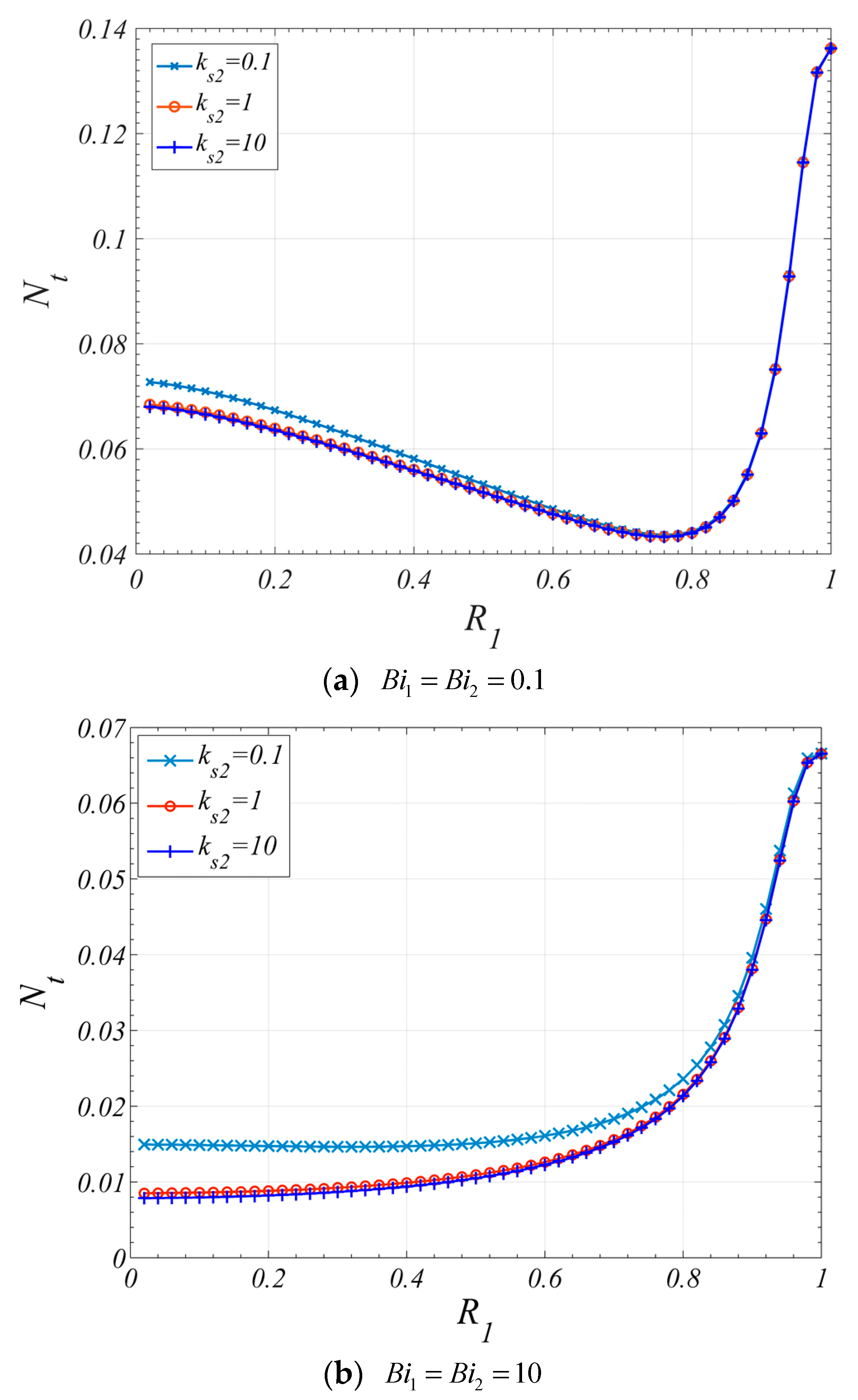
4.4. Heat Transfer Performance
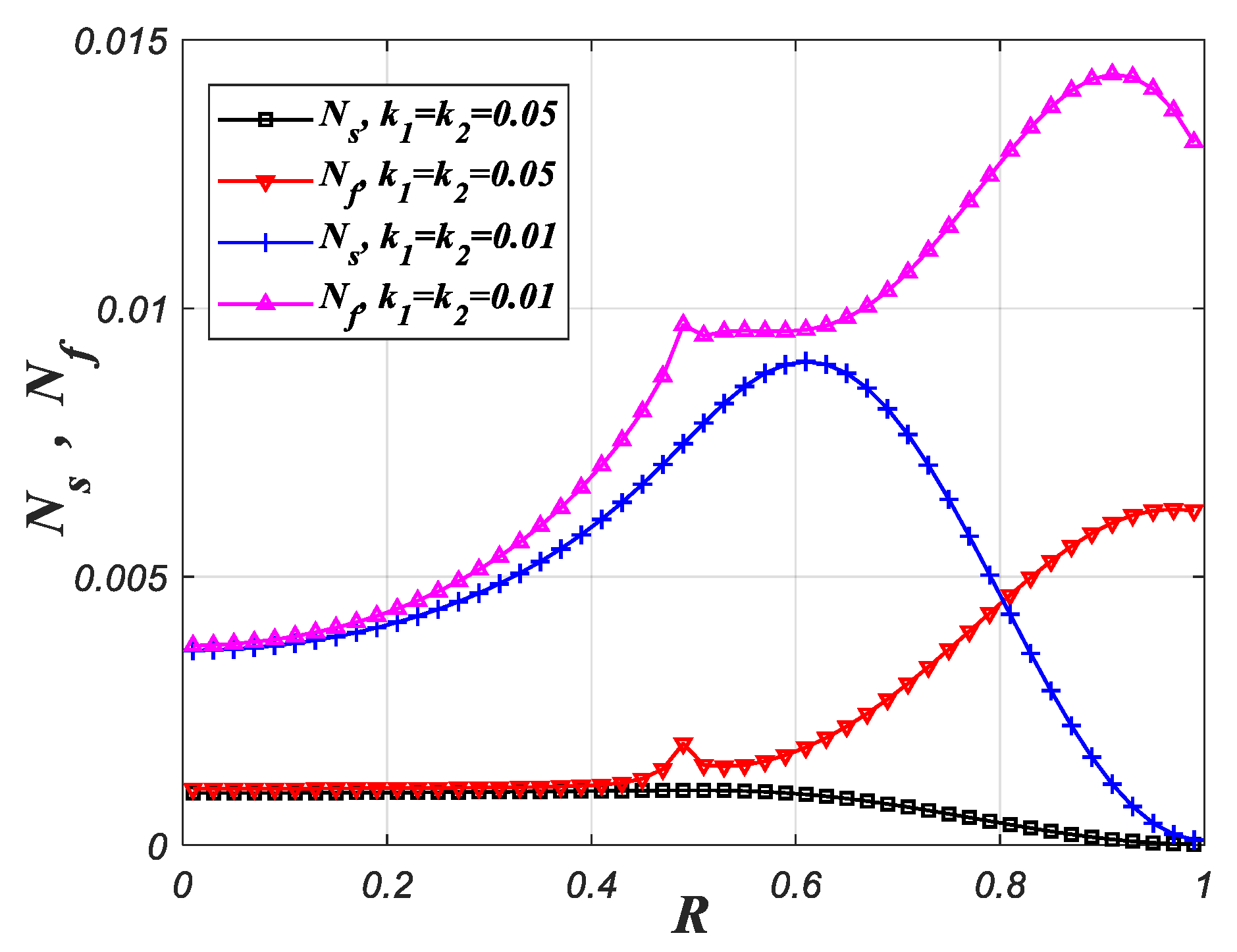
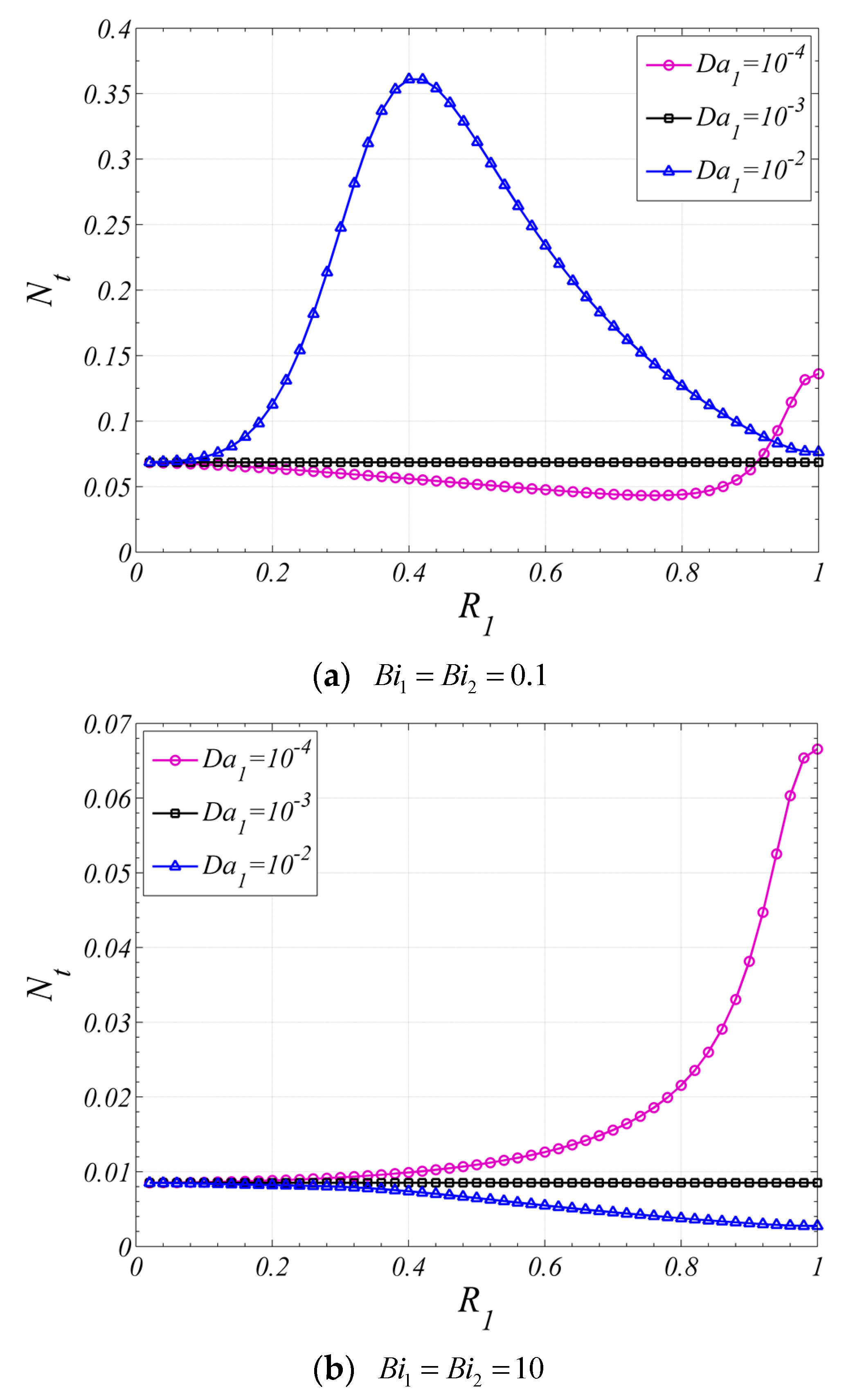
4.5. Local and Total Entropy Generation Rate
5. Conclusions
- (1)
- A more uniform temperature distribution of fluid phase within the tube filled with double-layer porous media can be obtained by decreasing or increasing .
- (2)
- When is less than , the Nusselt number for the tube filled with double-layer porous media can be larger than that for the tube filled with corresponding single layer porous medium. However, when is larger than , the Nusselt number for the tube filled with double-layer porous media can be less than that for the tube filled with corresponding single layer porous medium.
- (3)
- When is less than and the Biot number is small, the total entropy generation rate for the tube filled with double-layer porous media can be less than that for the tube filled with corresponding single layer porous medium.
- (4)
- When is less than , the maximum value of the Nusselt number and the minimum value of the total entropy generation rate for the tube filled with double-layer porous media can be obtained by properly selecting the pertinent parameters, such as Darcy number, Biot number, and dimensionless interfacial radius. However, it should be noted that the dimensionless interfacial radius corresponding to the maximum value of the Nusselt number is different from that corresponding to the minimum value of the total entropy generation rate.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| interfacial area per unit volume of the porous media, m−1 | |
| Biot number | |
| Brinkman number | |
| heat capacity of fluid, J·kg−1·K−1 | |
| Darcy number | |
| dimensionless wall temperature, defined in Equation (15) | |
| heat transfer coefficient, W·m−2·K−1 | |
| permeability, m2 | |
| ratio of effective fluid thermal conductivity to that of solid | |
| ratio of effective solid thermal conductivity of two layers | |
| dimensionless local entropy generation rate within the fluid phase | |
| dimensionless local entropy generation rate within the solid phase | |
| dimensionless total entropy generation rate within the tube | |
| overall Nusselt number | |
| dimensionless pressure drop | |
| Peclet number | |
| pressure, N·m−2 | |
| dimensionless radius | |
| radius, m | |
| local entropy generation rate within the fluid phase | |
| local entropy generation rate within the solid phase | |
| temperature, K | |
| thermal conductivity ratio, | |
| dimensionless velocity | |
| velocity, m/s | |
| Greek symbols | |
| porosity | |
| dimensionless temperature | |
| dynamic viscosity, kg·m−1·s−1 | |
| density, kg·m−3 | |
| Subscripts | |
| bulk | |
| effective | |
| fluid phase | |
| mass average | |
| solid phase | |
| wall | |
| layer 1 | |
| layer 2 | |
Appendix A.
References
- Kim, S.; Kim, D.; Lee, D. On the local thermal equilibrium in microchannel heat sinks. Int. J. Heat Mass Transf. 2000, 43, 1735–1748. [Google Scholar] [CrossRef]
- Jing, D.; Song, J. Hydraulic and thermal performances of laminar flow in fractal treelike branching microchannel network with wall velocity slip. Fractals 2020, 28, 2050022. [Google Scholar] [CrossRef]
- Jing, D.; Yi, S. Electroosmotic flow in tree-like branching microchannel network. Fractals 2019, 27, 1950095. [Google Scholar] [CrossRef]
- Pavel, B.I.; Mohamad, A.A. Experimental Investigation of the Potential of Metallic Porous Inserts in Enhancing Forced Convective Heat Transfer. ASME J. Heat Transf. 2004, 126, 540–545. [Google Scholar] [CrossRef]
- Lu, W.; Zhao, C.; Tassou, S. Thermal analysis on metal-foam filled heat exchangers. Part I: Metal-foam filled pipes. Int. J. Heat Mass Transf. 2006, 49, 27512761. [Google Scholar] [CrossRef]
- Yang, K.; Vafai, K. Analysis of temperature gradient bifurcation in porous media—An exact solution. Int. J. Heat Mass Transf. 2010, 53, 4316–4325. [Google Scholar] [CrossRef]
- Yang, K.; Vafai, K. Analysis of heat flux bifurcation inside porous media incorporating inertial and dispersion effects—An exact solution. Int. J. Heat Mass Transf. 2011, 54, 5286–5297. [Google Scholar] [CrossRef]
- Xu, H.; Qu, Z.; Tao, W. Thermal transport analysis in parallel-plate channel filled with open-celled metallic foams. Int. J. Heat Mass Transf. 2011, 38, 868873. [Google Scholar] [CrossRef]
- Dehghan, M.; Jamal-Abad, M.T.; Rashidi, S. Analytical interpretation of the local thermal non-equilibrium condition of porous media imbedded in tube heat exchangers. Energy Convers. Manag. 2014, 85, 264–271. [Google Scholar] [CrossRef]
- Zhang, J.; Qu, Z.; Xu, H.; Tao, W. Semi-analytical solution for fully developed forced convection in metal-foam filled tube with uniform wall temperature. Sci. China Technol. Sci. 2014, 57, 2487–2499. [Google Scholar] [CrossRef]
- Dehghan, M.; Valipour, M.S.; Saedodin, S. Temperature-dependent conductivity in forced convection of heat exchangers filled with porous media: A perturbation solution. Energy Convers. Manag. 2015, 91, 259–266. [Google Scholar] [CrossRef]
- Yang, K.; You, X.; Wang, J.; Vafai, K. Analysis of two approaches for an adiabatic boundary condition in porous media. Int. J. Numer. Methods Heat Fluid Flow 2016, 26, 977–998. [Google Scholar] [CrossRef]
- Torabi, M.; Karimi, N.; Zhang, K. Heat transfer and second law analyses of forced convection in a channel partially filled by porous media and featuring internal heat sources. Energy 2015, 93, 106–127. [Google Scholar] [CrossRef]
- Torabi, M.; Karimi, N.; Zhang, K.; Peterson, G. Generation of entropy and forced convection of heat in a conduit partially filled with porous media—local thermal non-equilibrium and exothermicity effects. Appl. Therm. Eng. 2016, 106, 518–536. [Google Scholar] [CrossRef]
- Torabi, M.; Zhang, K.; Yang, G.; Wang, J.; Wu, P. Heat transfer and entropy generation analyses in a channel partially filled with porous media using local thermal non-equilibrium model. Energy 2015, 82, 922–938. [Google Scholar] [CrossRef]
- Yang, K.; Chen, H.; Wang, J. Analysis of heat transfer and entropy generation in a channel partially filled with N-layer porous media. ASME J. Heat Transf. 2018, 140, 082601. [Google Scholar] [CrossRef]
- Yang, K.; Vafai, K. Restrictions on the validity of the thermal conditions at the porous-fluid interface: An exact solution. ASME J. Heat Transf. 2011, 133, 112601. [Google Scholar] [CrossRef]
- Mahmoudi, Y.; Karimi, N.; Mazaheri, K. Analytical investigation of heat transfer enhancement in a channel partially filled with porous material under local thermal non-equilibrium condition: Effect of different boundary conditions at porous fluid interface. Int. J. Heat Mass Transf. 2014, 70, 875–891. [Google Scholar] [CrossRef]
- Mohamed, R.E.; Al-Hossainy, A.F. FEM for blood-based SWCNTs flow through a circular cylinder in a porous medium with electromagnetic radiation. Commun. Theor. Phys. 2019, 71, 1425. [Google Scholar]
- Buonomo, B.; Manca, O.; Lauriat, G. Forced convection in micro-channels filled with porous media in local thermal non-equilibrium conditions. Int. J. Therm. Sci. 2014, 77, 206–222. [Google Scholar] [CrossRef]
- Nield, D.A.; Kuznetsov, A.V. Effects of heterogeneity in forced convection in a porous medium: Parallel plate channel or circular duct. Int. J. Heat Mass Transf. 2000, 43, 4119–4134. [Google Scholar] [CrossRef]
- Nield, D.A. Effects of heterogeneity in forced convection in a porous medium: Parallel-plate channel, asymmetric property variation, and asymmetric heating. J. Porous Media 2001, 4, 137148. [Google Scholar] [CrossRef]
- Nield, D.A.; Kuznetsov, A.V. Effects of Temperature-Dependent Viscosity in Forced Convection in a Porous Medium: Layered-Medium Analysis. J. Porous Media 2003, 6, 213–222. [Google Scholar] [CrossRef]
- Sundaravadivelu, K.; Tso, C.P. Influence of viscosity variations on the forced convection flow through two types of heterogeneous porous media with isoflux boundary condition. Int. J. Heat Mass Transf. 2003, 46, 2329–2339. [Google Scholar] [CrossRef]
- Nield, D.A.; Kuznetsov, A.V. Interaction of Transverse Heterogeneity and Thermal Development of Forced Convection in a Porous Medium. Transp. Porous Media 2004, 57, 103–111. [Google Scholar] [CrossRef]
- Nield, D.A.; Kuznetsov, A.V. Optimization of forced convection heat transfer in a composite porous medium channel. Transp. Porous Media 2013, 99, 349–357. [Google Scholar] [CrossRef]
- Wildi-Tremblay, P.; Gosselin, L. Layered porous media architecture for maximal cooling. Int. J. Heat Mass Transf. 2007, 50, 464–478. [Google Scholar] [CrossRef]
- Zheng, Z.J.; Li, M.J.; He, Y.L. Optimization of porous insert configurations for heat transfer enhancement in tubes based on genetic algorithm and CFD. Int. J. Heat Mass Transf. 2015, 87, 376–379. [Google Scholar] [CrossRef]
- Chu, L.; Fwa, T.F. Functional sustainability of single- and double-layer porous asphalt pavements. Constr. Build. Mater. 2019, 197, 436–443. [Google Scholar] [CrossRef]
- Misra, J.C.; Mallick, B.; Steinmann, P. Temperature distribution and entropy generation during Darcy–Forchheimer–Brinkman electrokinetic flow in a microfluidic tube subject to a prescribed heat flux. Meccanica 2020, 55, 1079–1098. [Google Scholar] [CrossRef]
- Nield, D.A. Effects of heterogeneity in forced convection in a porous medium: Parallel-plate channel, Brinkman model. J. Porous Media 2003, 6, 257–266. [Google Scholar] [CrossRef]
- Nield, D.A.; Kuznetsov, A.V. The interaction of thermal nonequilibrium and heterogeneous conductivity effects in forced convection in layered porous channels. Int. J. Heat Mass Transf. 2001, 44, 4369–4373. [Google Scholar] [CrossRef]
- Xu, H.; Qu, Z.; Tao, W. Analytical solution of forced convective heat transfer in tubes partially filled with metallic foam using the two-equation model. Int. J. Heat Mass Transf. 2011, 54, 3846–3855. [Google Scholar] [CrossRef]
- Allan, F.M.; Hamdan, M.H. Fluid Mechanics of the Interface Region between Two Porous Layers. Appl. Math. Comput. 2002, 128, 37–43. [Google Scholar] [CrossRef]
- Vafai, K.; Thiyagaraja, R. Analysis of Flow and Heat Transfer at the Interface Region of a Porous Medium. Int. J. Heat Mass Transf. 1987, 30, 1391–1405. [Google Scholar] [CrossRef]
- Mahjoob, S.; Vafai, K. Analysis of Bioheat Transport Through a Dual Layer Biological Media. ASME J. Heat Transf. 2010, 132, 031101. [Google Scholar] [CrossRef]
- Zwillinger, D. CRC Standard Mathematical Tables and Formulae, 32nd ed.; CRC Press: New York, NY, USA, 2011; pp. 454–462. [Google Scholar]
- Bejan, A. Entropy Generation through Heat and Fluid Flow; John Wiley & Sons: New York, NY, USA, 1982; pp. 98–105. [Google Scholar]
- Pavel, B.I.; Mohamad, A.A. An experimental and numerical study on heat transfer enhancement for gas heat exchangers fitted with porous media. Int. J. Heat Mass Transf. 2004, 47, 4939–4952. [Google Scholar] [CrossRef]




| (Present Study) | ([39]) | ||
|---|---|---|---|
| 10−2 | 1.0 | 5.9812 | 5.9848 |
| 10−3 | 1.0 | 7.1380 | 7.1360 |
| 10−4 | 1.0 | 7.6957 | 7.6955 |
| 10−5 | 1.0 | 7.9002 | 7.9012 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, K.; Huang, W.; Li, X.; Wang, J. Analytical Analysis of Heat Transfer and Entropy Generation in a Tube Filled with Double-Layer Porous Media. Entropy 2020, 22, 1214. https://doi.org/10.3390/e22111214
Yang K, Huang W, Li X, Wang J. Analytical Analysis of Heat Transfer and Entropy Generation in a Tube Filled with Double-Layer Porous Media. Entropy. 2020; 22(11):1214. https://doi.org/10.3390/e22111214
Chicago/Turabian StyleYang, Kun, Wei Huang, Xin Li, and Jiabing Wang. 2020. "Analytical Analysis of Heat Transfer and Entropy Generation in a Tube Filled with Double-Layer Porous Media" Entropy 22, no. 11: 1214. https://doi.org/10.3390/e22111214
APA StyleYang, K., Huang, W., Li, X., & Wang, J. (2020). Analytical Analysis of Heat Transfer and Entropy Generation in a Tube Filled with Double-Layer Porous Media. Entropy, 22(11), 1214. https://doi.org/10.3390/e22111214




