The Secret Key Capacity of a Class of Noisy Channels with Correlated Sources
Abstract
1. Introduction
1.1. Related Work
1.2. Contributions and Organization of the Paper
1.3. Notation and Conventions
2. Preliminaries
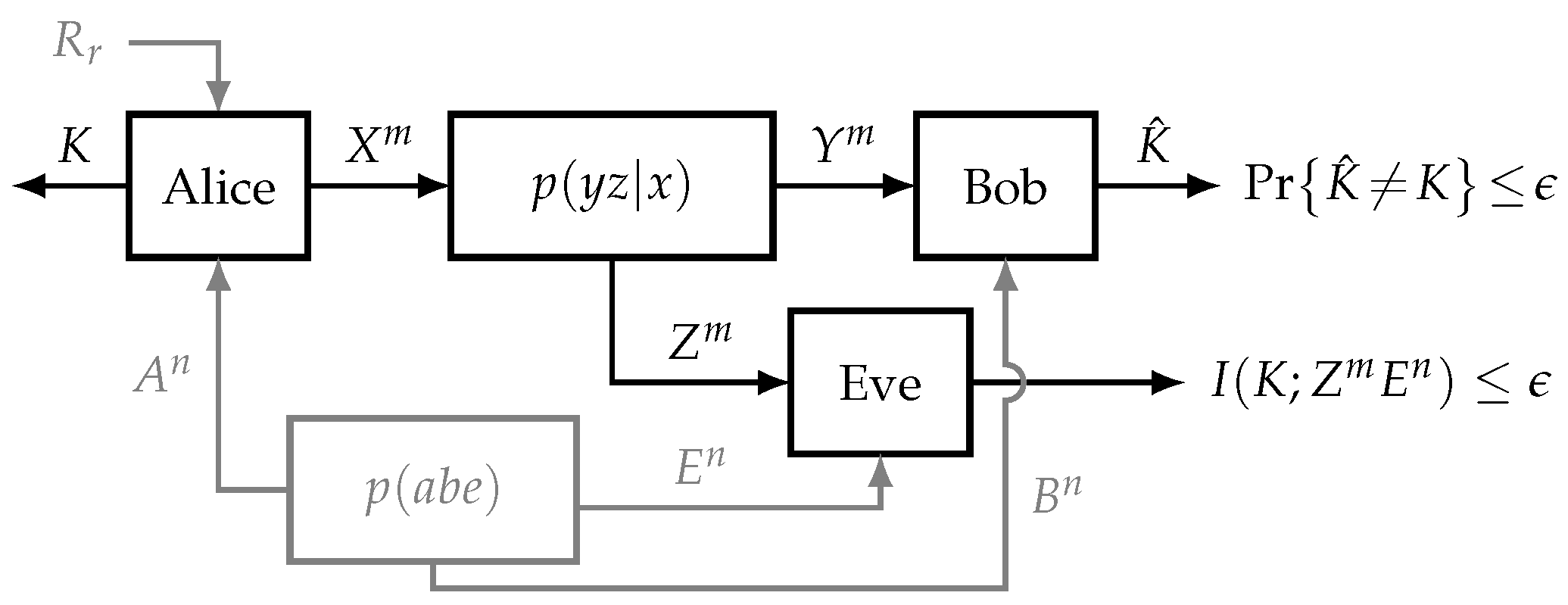
2.1. Problem Definition
- a key set , where is the rate of the secret key;
- a source of local randomness at Alice;
- an encoding function ;
- a key generation function ; and
- a key generation function .
2.2. Inner Bound
3. Main Results
3.1. Outer Bound
3.2. Optimal Characterization of the Secret Key Rate
3.2.1. Eve Has a Less Noisy Channel
3.2.2. Eve Has a Less Noisy Source
3.2.3. Bob Has a Less Noisy Channel and Source
4. Secret Key Agreement over a Wiretap Channel with BEC/BSC Sources
4.1. System Model
- If , E is a degraded version of B, i.e., .
- If , B is less noisy than E, i.e., .
- If , B is more capable than E.
4.2. Performance of the Coding Scheme
5. Summary and Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| i.i.d. | independent and identically distributed |
| r.h.s. | right-hand side |
| w.r.t. | with respect to |
Appendix A. Proof of Theorem 2
- Equation (A4a) stems from the uniformity of the keys in Equation (A2d).
- Equation (A4b) is due to the security condition in Equation (A2c).
- Equation (A4c) follows from Equations (A1) and (A2b), and Fano’s inequality, .
- Equation (A5a) is due to Csiszár sum identity.
- Equation (A5b) follows from the definition of the auxiliary RVs and .
- Equation (A5c) introduces the auxiliary RV J uniformly distributed over and independent of all the other variables.
- Equation (A5d) stems from the definition of random variables , , , and .
- Equation (A14a) follows from the Markov chain in Equation (A12).
- Equation (A14b) stems from due to Equations (A1) and (A2b), and .
- Equation (A15a) stems from the Markov chain .
- Equation (A15b) follows from the sources being i.i.d., i.e., .
- Equation (A15c) is due to the auxiliary RV .
- Equation (A15d) introduces the auxiliary RV J uniformly distributed over and independent of all the other variables.
- Equation (A15e) follows from the independence of J and .
- Equation (A15f) stems from the definition of random variables , , and .
Appendix B. Proof of Proposition 5
References
- Wyner, A.D. The Wire-Tap Channel. Bell Syst. Tech. J. 1975, 54, 1355–1387. [Google Scholar] [CrossRef]
- Csiszár, I.; Körner, J. Broadcast Channels with Confidential Messages. IEEE Trans. Inf. Theory 1978, 24, 339–348. [Google Scholar] [CrossRef]
- Shannon, C.E. Communication Theory of Secrecy Systems. Bell Syst. Tech. J. 1949, 28, 656–715. [Google Scholar] [CrossRef]
- Chorti, A.; Hollanti, C.; Belfiore, J.C.; Poor, H.V. Physical Layer Security: A Paradigm Shift in Data Confidentiality. In Physical and Data-Link Security Techniques for Future Communication Systems; Baldi, M., Tomasin, S., Eds.; Lecture Notes in Electrical Engineering; Springer International Publishing: Cham, Switzerland, 2016; Volume 358, pp. 1–15. [Google Scholar] [CrossRef]
- Narayan, P.; Tyagi, H. Multiterminal Secrecy by Public Discussion. In Foundations and Trends® in Communications and Information Theory; Now Publishers Inc.: Hanover, MA, USA, 2016; Volume 13, pp. 129–275. [Google Scholar] [CrossRef]
- Maurer, U.M. Secret Key Agreement by Public Discussion from Common Information. IEEE Trans. Inf. Theory 1993, 39, 733–742. [Google Scholar] [CrossRef]
- Ahlswede, R.; Csiszár, I. Common Randomness in Information Theory and Cryptography—Part I: Secret Sharing. IEEE Trans. Inf. Theory 1993, 39, 1121–1132. [Google Scholar] [CrossRef]
- Csiszár, I.; Narayan, P. Common Randomness and Secret Key Generation with a Helper. IEEE Trans. Inf. Theory 2000, 46, 344–366. [Google Scholar] [CrossRef]
- Csiszár, I.; Narayan, P. Secrecy Capacities for Multiterminal Channel Models. IEEE Trans. Inf. Theory 2008, 54, 2437–2452. [Google Scholar] [CrossRef]
- Csiszár, I.; Narayan, P. Secrecy Generation for Multiaccess Channel Models. IEEE Trans. Inf. Theory 2013, 59, 17–31. [Google Scholar] [CrossRef]
- Gohari, A.A.; Anantharam, V. Information-Theoretic Key Agreement of Multiple Terminals—Part I. IEEE Trans. Inf. Theory 2010, 56, 3973–3996. [Google Scholar] [CrossRef]
- Gohari, A.A.; Anantharam, V. Information-Theoretic Key Agreement of Multiple Terminals—Part II: Channel Model. IEEE Trans. Inf. Theory 2010, 56, 3997–4010. [Google Scholar] [CrossRef]
- Khisti, A.; Diggavi, S.N.; Wornell, G.W. Secret-Key Generation Using Correlated Sources and Channels. IEEE Trans. Inf. Theory 2012, 58, 652–670. [Google Scholar] [CrossRef]
- Prabhakaran, V.M.; Eswaran, K.; Ramchandran, K. Secrecy via Sources and Channels. IEEE Trans. Inf. Theory 2012, 58, 6747–6765. [Google Scholar] [CrossRef]
- Bunin, A.; Piantanida, P.; Shamai, S. The Gaussian Wiretap Channel with Correlated Sources at the Terminals: Secret Communication and Key Generation. In Proceedings of the 2016 ICSEE International Conference on the Science of Electrical Engineering, Eilat, Israel, 16–18 November 2016; pp. 1–5. [Google Scholar]
- Salimi, S.; Skoglund, M.; Golic, J.D.; Salmasizadeh, M.; Aref, M.R. Key Agreement over a Generalized Multiple Access Channel Using Noiseless and Noisy Feedback. IEEE J. Sel. Areas Commun. 2013, 31, 1765–1778. [Google Scholar] [CrossRef]
- Tyagi, H. Common Information and Secret Key Capacity. IEEE Trans. Inf. Theory 2013, 59, 5627–5640. [Google Scholar] [CrossRef]
- Hayashi, M.; Tyagi, H.; Watanabe, S. Secret Key Agreement: General Capacity and Second-Order Asymptotics. IEEE Trans. Inf. Theory 2016, 62, 3796–3810. [Google Scholar] [CrossRef]
- Courtade, T.A.; Halford, T.R. Coded Cooperative Data Exchange for a Secret Key. IEEE Trans. Inf. Theory 2016, 62, 3785–3795. [Google Scholar] [CrossRef]
- Boche, H.; Schaefer, R.F.; Poor, H.V. On the Computability of the Secret Key Capacity under Rate Constraints. In Proceedings of the 2019 IEEE International Conference on Acoustics, Speech and Signal Processing (ICASSP), Brighton, UK, 12–17 May 2019; pp. 2427–2431. [Google Scholar] [CrossRef]
- Chen, Y.; Vinck, A.J.H. Wiretap Channel with Side Information. IEEE Trans. Inf. Theory 2008, 54, 395–402. [Google Scholar] [CrossRef]
- Liu, W.; Chen, B. Wiretap Channel with Two-Sided Channel State Information. In Proceedings of the 2007 41st Asilomar Conference on Signals, Systems and Computers (ACSSC), Pacific Grove, CA, USA, 4–7 November 2007; pp. 893–897. [Google Scholar] [CrossRef]
- Gelfand, S.I.; Pinsker, M.S. Coding for Channel with Random Parameters. Probl. Control Inf. Theory 1980, 9, 19–31. [Google Scholar]
- Muramatsu, J. General Formula for Secrecy Capacity of Wiretap Channel with Noncausal State. In Proceedings of the 2014 IEEE International Symposium on Information Theory (ISIT), Honolulu, HI, USA, 29 June–4 July 2014; pp. 21–25. [Google Scholar] [CrossRef]
- Goldfeld, Z.; Cuff, P.; Permuter, H.H. Wiretap Channels with Random States Non-Causally Available at the Encoder. arXiv 2016, arXiv:1608.00743. [Google Scholar]
- Zibaeenejad, A. Key Generation over Wiretap Models with Non-Causal Side Information. IEEE Trans. Inf. Forensics Secur. 2015, 10, 1456–1471. [Google Scholar] [CrossRef]
- Bunin, A.; Goldfeld, Z.; Permuter, H.H.; Shamai, S.; Cuff, P.; Piantanida, P. Key and Message Semantic-Security over State-Dependent Channels. IEEE Trans. Inf. Forensics Secur. 2018. [Google Scholar] [CrossRef]
- Bassi, G.; Piantanida, P.; Shamai, S. The Wiretap Channel with Generalized Feedback: Secure Communication and Key Generation. IEEE Trans. Inf. Theory 2019, 65, 2213–2233. [Google Scholar] [CrossRef]
- El Gamal, A.; Kim, Y.H. Network Information Theory; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Bassi, G.; Piantanida, P.; Shamai, S. Secret Key Generation over Noisy Channels with Common Randomness. In Proceedings of the 2016 IEEE International Symposium on Information Theory (ISIT), Barcelona, Spain, 10–15 July 2016; pp. 510–514. [Google Scholar] [CrossRef]
- Bassi, G.; Piantanida, P.; Shamai, S. Secret Key Generation over Noisy Channels with Correlated Sources. arXiv 2016, arXiv:1609.08330. [Google Scholar]
- Cao, D.; Kang, W. Secret key generation from correlated sources and secure link. In Proceedings of the 2017 9th International Conference on Wireless Communications and Signal Processing (WCSP), Nanjing, China, 11–13 October 2017; pp. 1–5. [Google Scholar] [CrossRef]
- Nair, C. Capacity Regions of Two New Classes of 2-Receiver Broadcast Channels. In Proceedings of the 2009 IEEE International Symposium on Information Theory (ISIT), Seoul, Korea, 28 June–3 July 2009; pp. 1839–1843. [Google Scholar] [CrossRef]

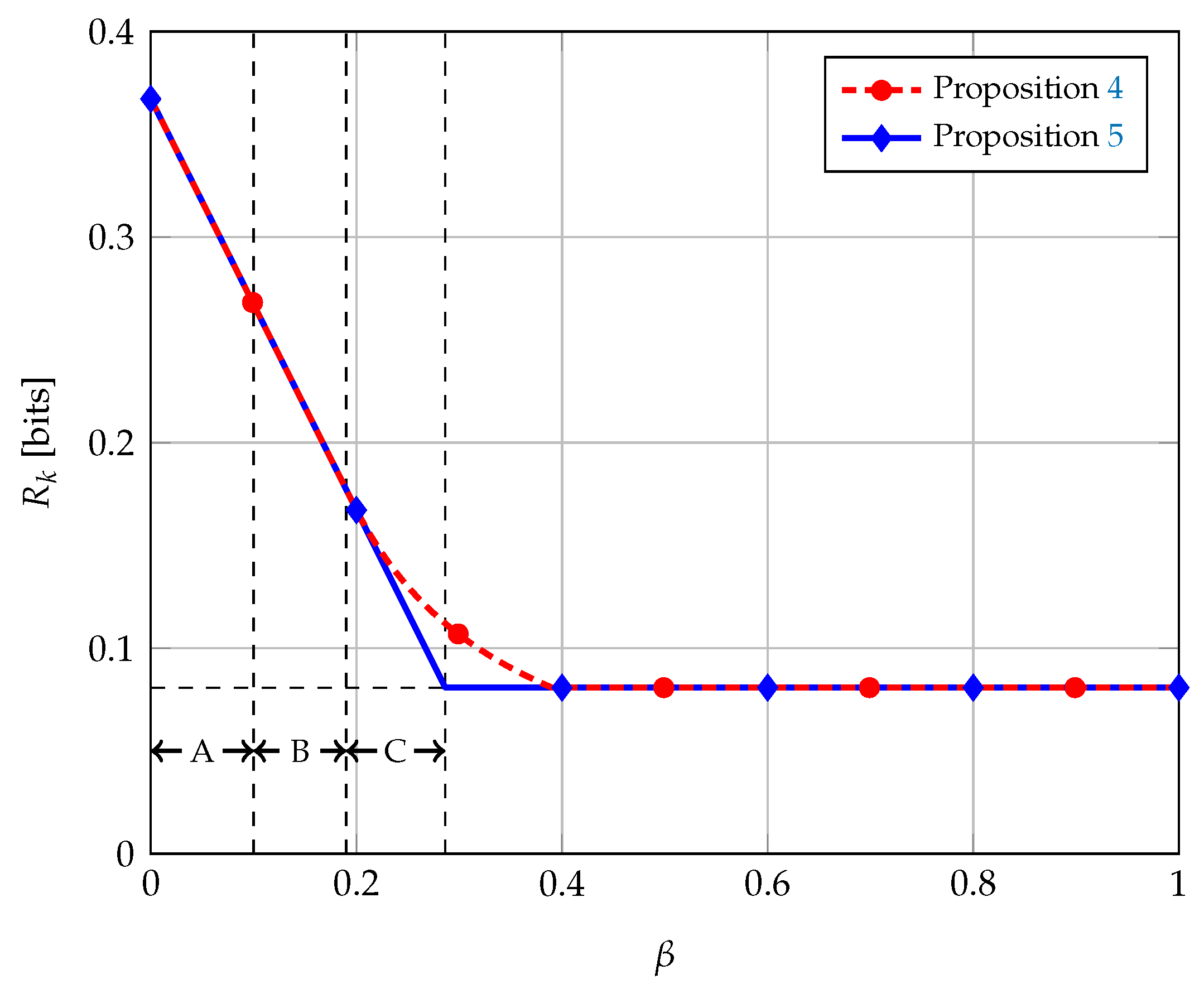
| Proposition 1 | Proposition 2 | Proposition 3 | ||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bassi, G.; Piantanida, P.; Shamai, S. The Secret Key Capacity of a Class of Noisy Channels with Correlated Sources. Entropy 2019, 21, 732. https://doi.org/10.3390/e21080732
Bassi G, Piantanida P, Shamai S. The Secret Key Capacity of a Class of Noisy Channels with Correlated Sources. Entropy. 2019; 21(8):732. https://doi.org/10.3390/e21080732
Chicago/Turabian StyleBassi, Germán, Pablo Piantanida, and Shlomo Shamai (Shitz). 2019. "The Secret Key Capacity of a Class of Noisy Channels with Correlated Sources" Entropy 21, no. 8: 732. https://doi.org/10.3390/e21080732
APA StyleBassi, G., Piantanida, P., & Shamai, S. (2019). The Secret Key Capacity of a Class of Noisy Channels with Correlated Sources. Entropy, 21(8), 732. https://doi.org/10.3390/e21080732




.png)

