Quantum Games with Unawareness with Duopoly Problems in View
Abstract
1. Introduction
2. Preliminaries
2.1. Normal form Games with Unawareness
- 1.
- For every ,
- 2.
- For every ,
- 3.
- If , then
2.2. Extended Nash Equilibrium
- 1.
- σ is rationalizable for G if and only if is part of an extended rationalizable profile in .
- 2.
- σ is a Nash equilibrium for G if and only if is part of on an extended Nash equilibrium for and this ENE satisfies .
3. Quantum Cournot’s Duopoly
3.1. Classical Case
- the set of players is ,
- the strategy set of player i is ,
- player i’s payoff function is given by formulawhere represents the price of the product,and a marginal cost c satisfies .
3.2. Quantum Case
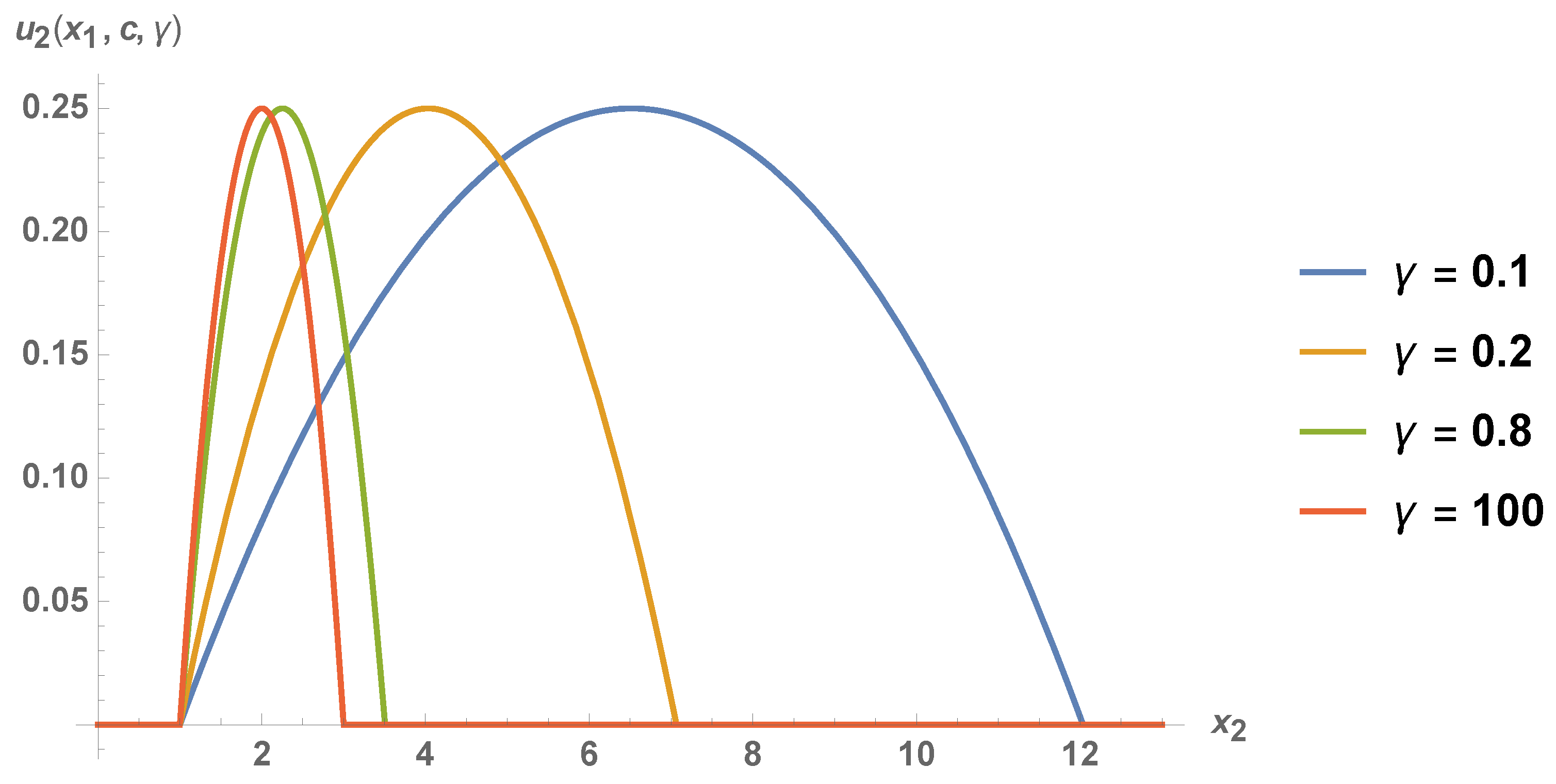
4. Quantum Cournot Duopoly with Unawareness
5. General Framework
6. Bertrand Price Competition
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Eisert, J.; Wilkens, M.; Lewenstein, M. Quantum games and quantum strategies. Phys. Rev. Lett. 1999, 83, 3077–3080. [Google Scholar] [CrossRef]
- Yu, T.; Ben-Av, R. Evolutionarily stable sets in quantum penny flip games. Quantum. Inf. Process 2013, 12, 2143. [Google Scholar] [CrossRef]
- Nawaz, A.; Toor, A.H. Evolutionarily stable strategies in quantum HawkDove game. Chin. Phys. Lett. 2010, 27, 5. [Google Scholar] [CrossRef]
- Iqbal, A.; Toor, A.H. Evolutionarily stable strategies in quantum games. Phys. Lett. A 2001, 280, 249. [Google Scholar] [CrossRef]
- Pykacz, J.; Fra̧ckiewicz, P. Arbiter as a third man in classical and quantum games. Int. J. Theor. Phys. 2010, 49, 3243. [Google Scholar] [CrossRef]
- Meyer, D.A. Quantum strategies. Phys. Rev. Lett. 1999, 82, 1052. [Google Scholar] [CrossRef]
- Fra̧ckiewicz, P. Quantum information approach to normal representation of extensive games. Int. J. Quantum Inf. 2012, 10, 1250048. [Google Scholar] [CrossRef]
- Fra̧ckiewicz, P.; Sładkowski, J. Quantum information approach to the ultimatum game. Int. J. Theor. Phys. 2014, 53, 3248. [Google Scholar] [CrossRef]
- La Mura, P. Correlated equilibria of classical strategic games with quantum signals. Int. J. Quantum Inf. 2005, 3, 183. [Google Scholar] [CrossRef]
- Wei, Z.; Zhang, S. Full characterization of quantum correlated equilibria. Quantum Inf. Comput. 2013, 13, 846. [Google Scholar]
- Fra̧ckiewicz, P. On quantum game approach to correlated equilibrium. In Proceedings of the 4th Global Virtual Conference, Žilina, Slovakia, 18–22 April 2016. [Google Scholar] [CrossRef]
- Iqbal, A.; Toor, A.H. Quantum repeated games. Phys. Lett. A 2002, 300, 541. [Google Scholar] [CrossRef]
- Fra̧ckiewicz, P. Quantum repeated games revisited. J. Phys. A Math. Theor. 2012, 45, 085307. [Google Scholar] [CrossRef]
- Iqbal, A.; Toor, A.H. Quantum cooperative games. Phys. Lett. A 2002, 293, 103. [Google Scholar] [CrossRef]
- Liao, X.P.; Ding, X.Z.; Fang, M.F. Improving the payoffs of cooperators in three-player cooperative game using weak measurements. Quantum Inf. Process 2015, 14, 4395. [Google Scholar] [CrossRef]
- Fra̧ckiewicz, P. Quantum games with unawareness. Entropy 2018, 20, 555. [Google Scholar] [CrossRef]
- Fra̧ckiewicz, P. Quantum Penny Flip game with unawareness. Quantum Inf. Process 2019, 18, 15. [Google Scholar] [CrossRef]
- Fra̧ckiewicz, P. Remarks on quantum duopoly schemes. Quantum Inf. Process 2016, 15, 121. [Google Scholar] [CrossRef]
- Fra̧ckiewicz, P.; Sladkowski, J. Quantum approach to Bertrand duopoly. Quantum Inf. Process 2016, 15, 3637. [Google Scholar] [CrossRef]
- Feinberg, Y. Games with Unawareness; Working Paper No. 2122; Stanford Graduate School of Business: Stanford, CA, USA, 2012. [Google Scholar]
- Li, H.; Du, J.; Massar, S. Continuous-variable quantum games. Phys. Lett. A 2002, 306, 73–78. [Google Scholar] [CrossRef]
- Nash, J. Non-cooperative games. Ann. Math. 1951, 54, 286. [Google Scholar] [CrossRef]
- Cournot, A. Recherches sur les Principes Mathématiques de la Théorie des Richesses; Hachette: Paris, France, 1838. [Google Scholar]
- Fra̧ckiewicz, P. Quantum approach to Cournot-type competition. Int. J. Theor. Phys. 2018, 57, 353. [Google Scholar] [CrossRef]
- Bertrand, J. Théorie mathématique de la richesse sociale. Journal des Savants 1883, 67, 499–508. [Google Scholar]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frąckiewicz, P.; Bilski, J. Quantum Games with Unawareness with Duopoly Problems in View. Entropy 2019, 21, 1097. https://doi.org/10.3390/e21111097
Frąckiewicz P, Bilski J. Quantum Games with Unawareness with Duopoly Problems in View. Entropy. 2019; 21(11):1097. https://doi.org/10.3390/e21111097
Chicago/Turabian StyleFrąckiewicz, Piotr, and Jakub Bilski. 2019. "Quantum Games with Unawareness with Duopoly Problems in View" Entropy 21, no. 11: 1097. https://doi.org/10.3390/e21111097
APA StyleFrąckiewicz, P., & Bilski, J. (2019). Quantum Games with Unawareness with Duopoly Problems in View. Entropy, 21(11), 1097. https://doi.org/10.3390/e21111097




