Thermodynamic Analysis of Irreversible Desiccant Systems
Abstract
:1. Introduction
2. Modelling
2.1. Thermal Cycle Efficiency
2.2. Energy and Mass Balance
2.3. Entropy Balance
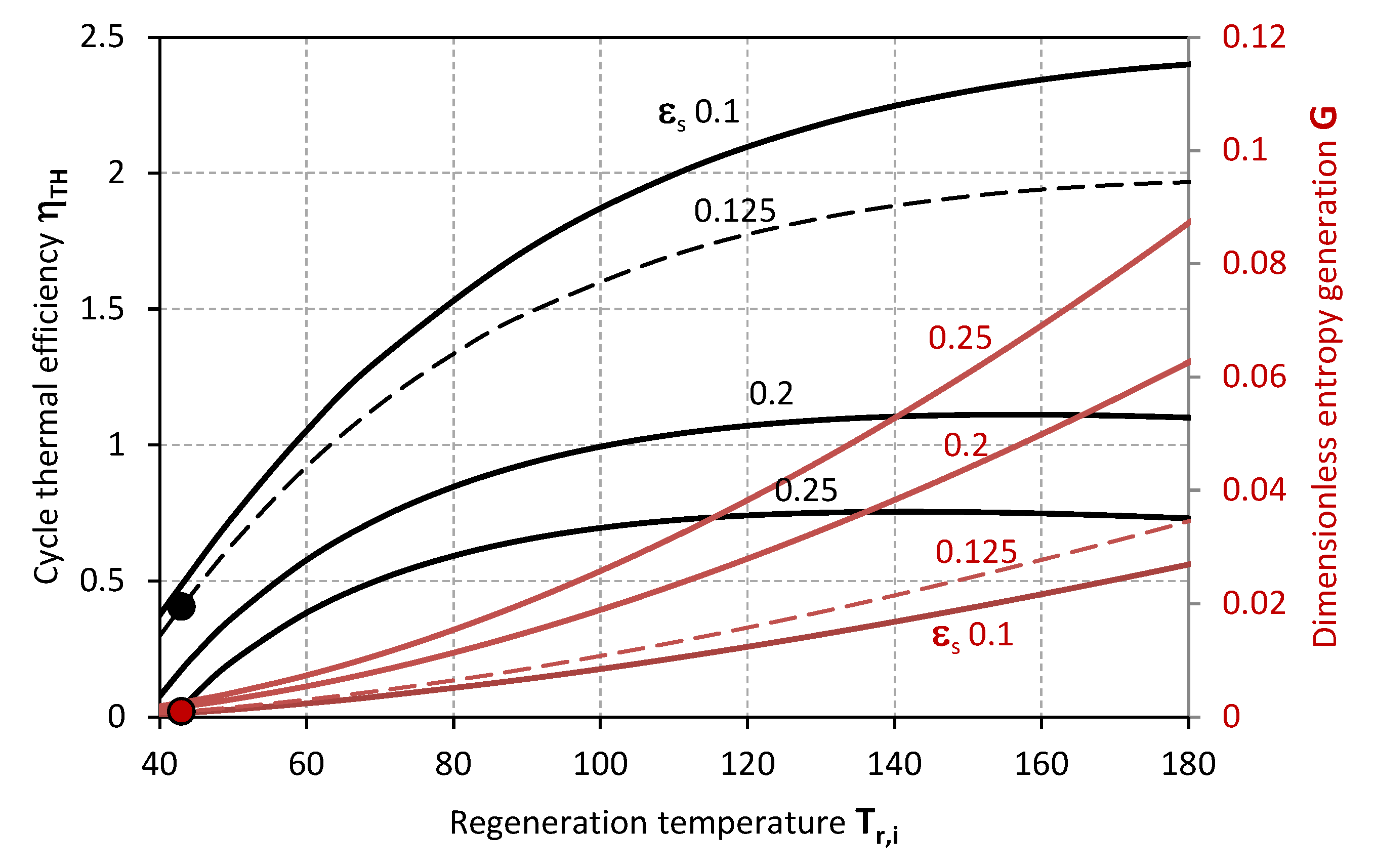
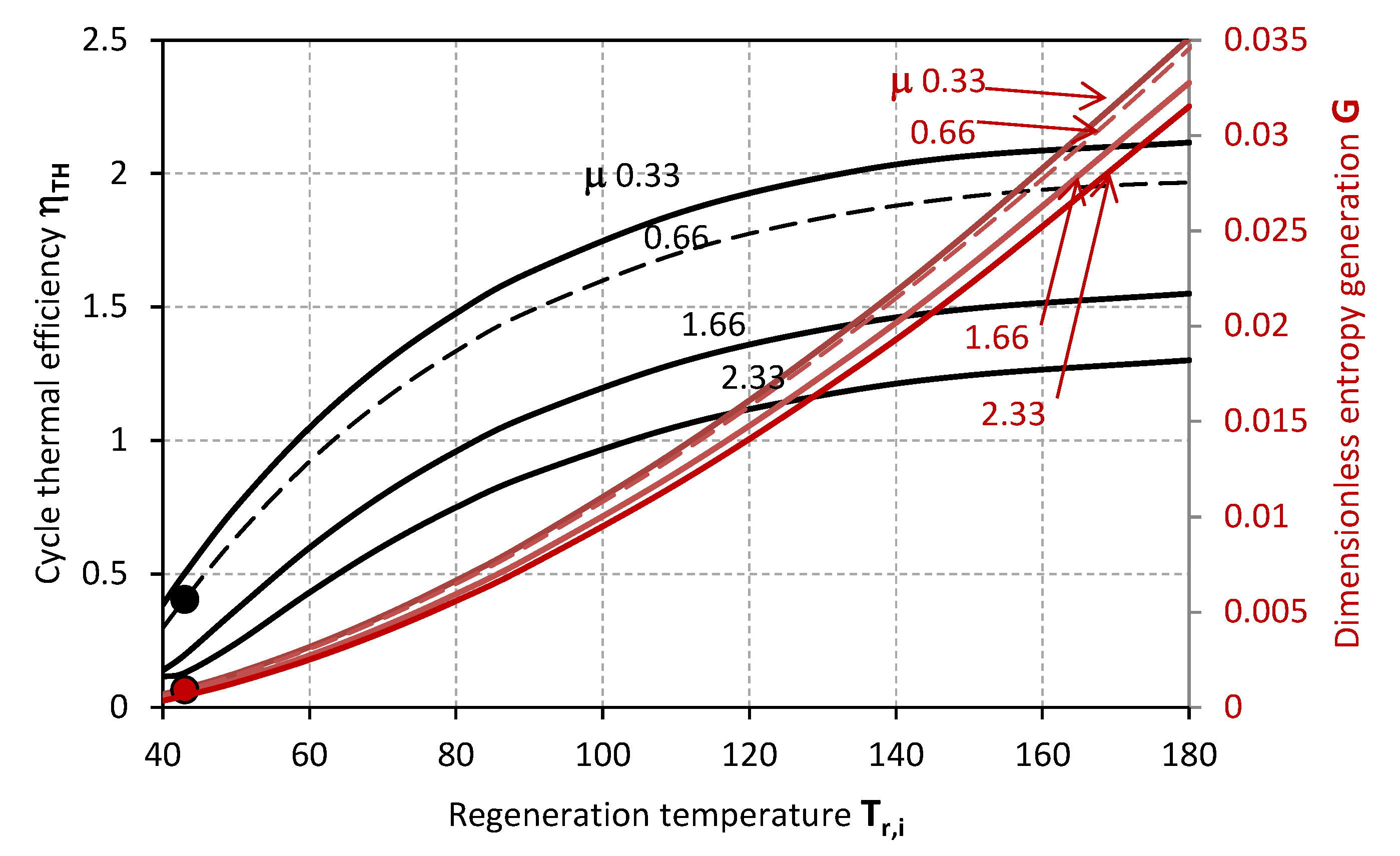
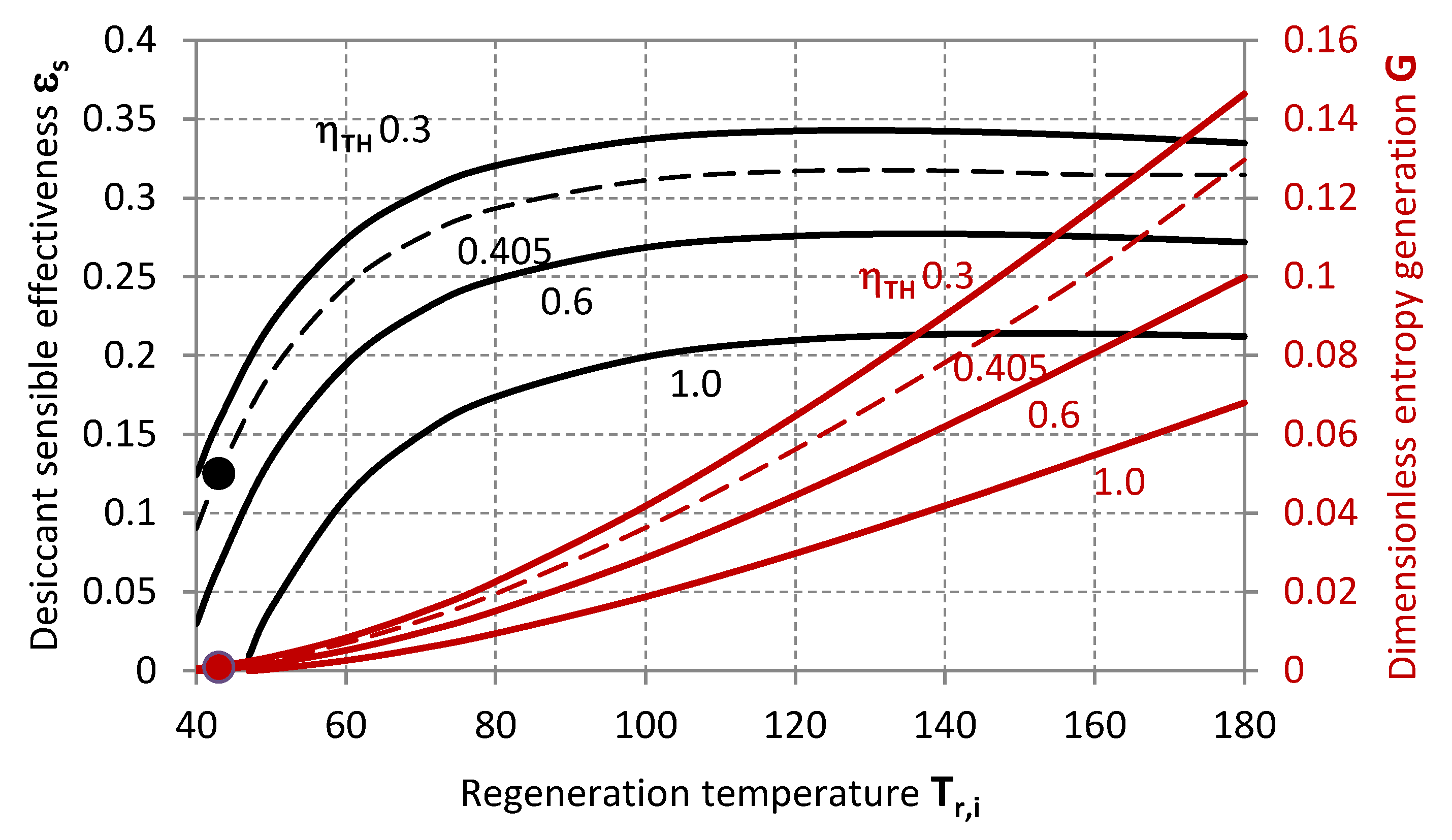
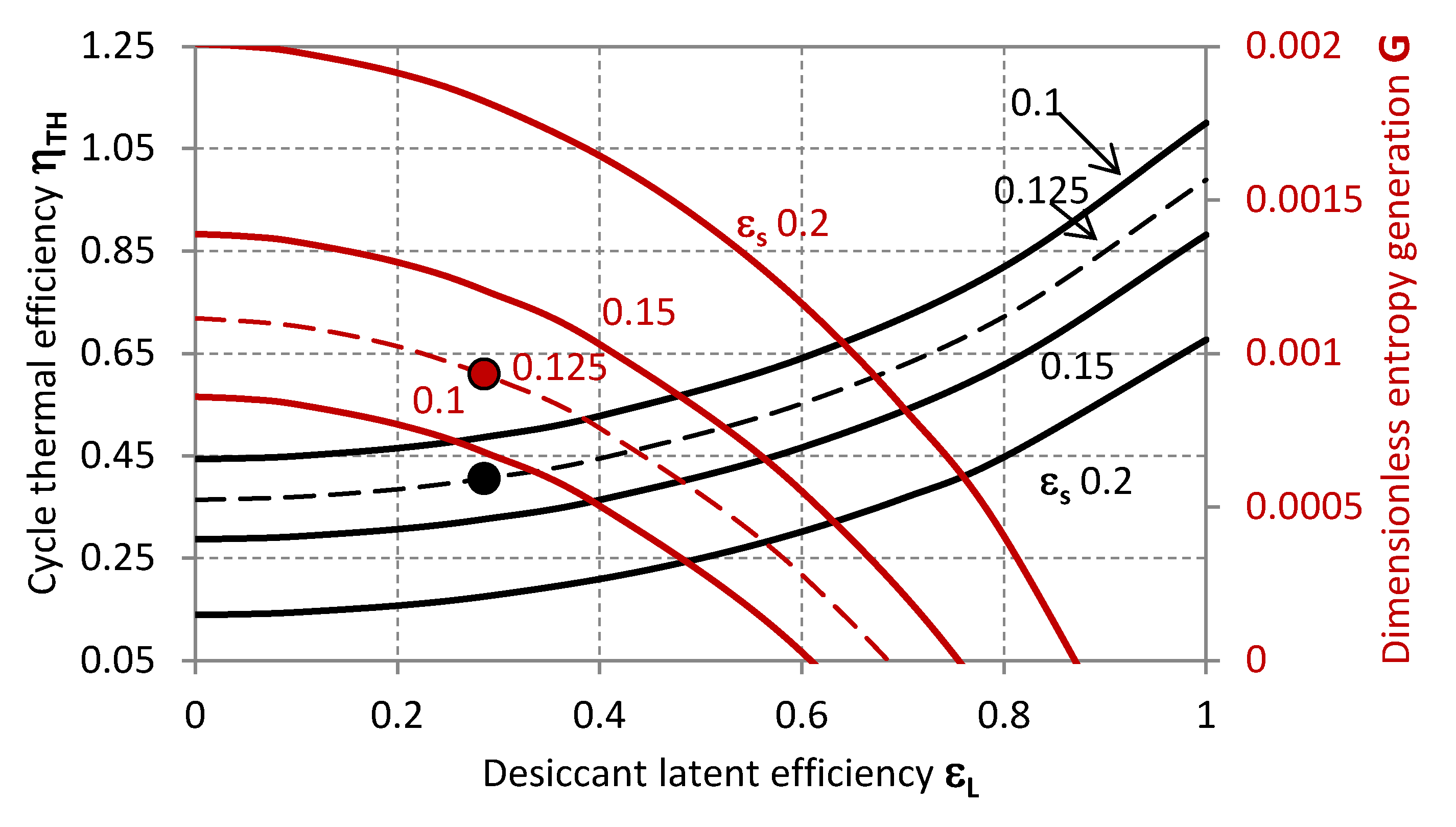
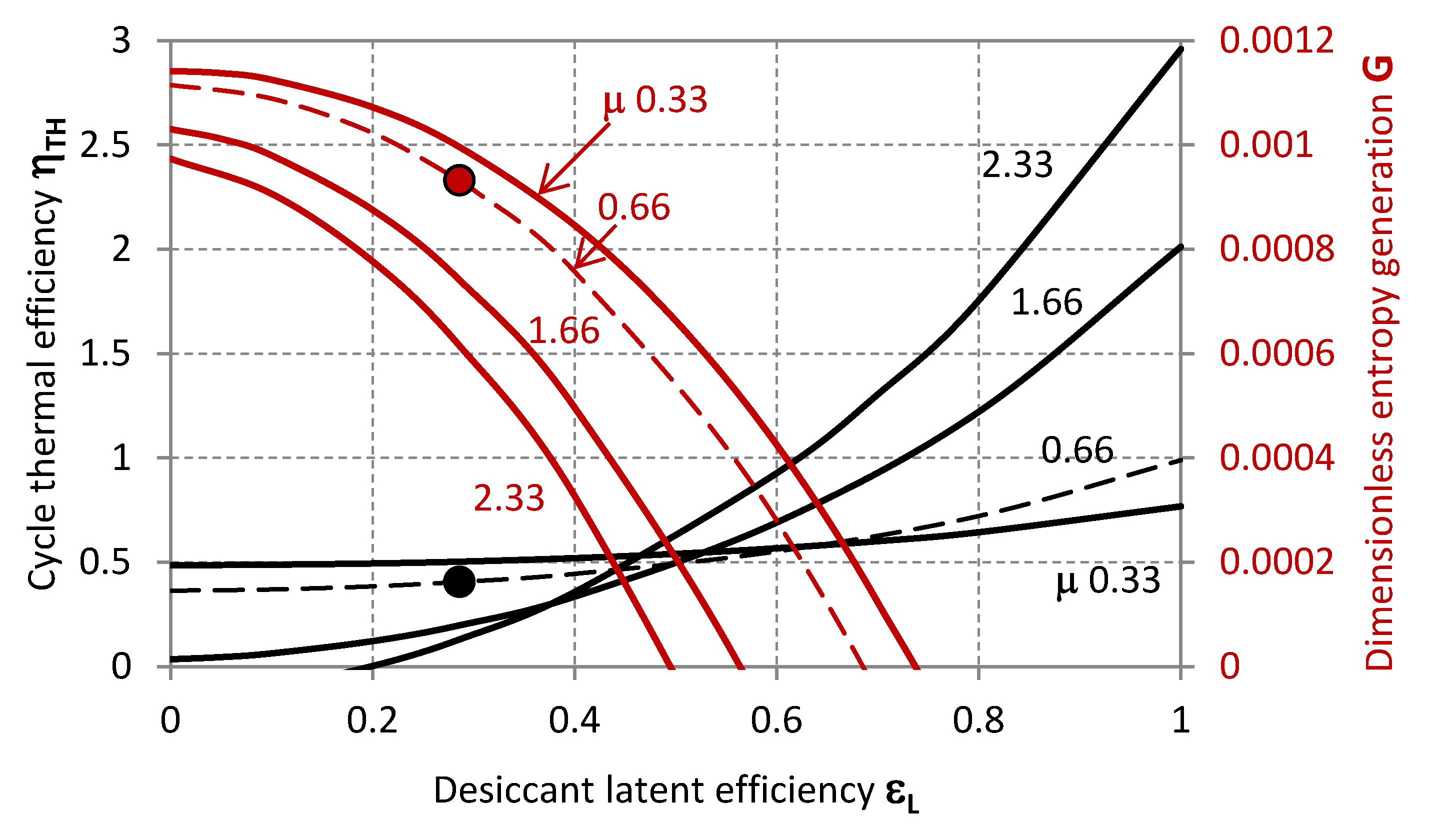
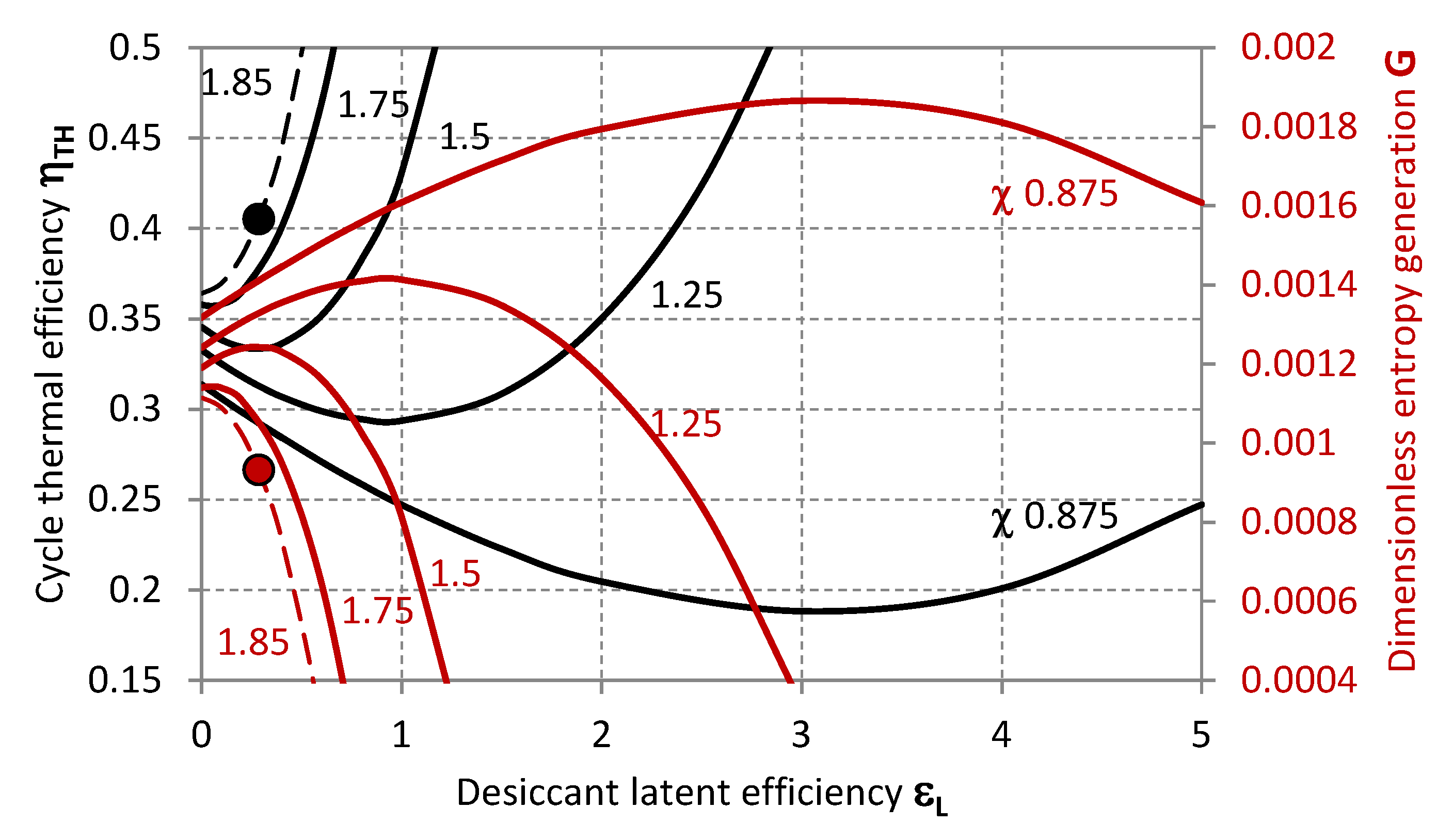
3. Thermodynamic Analysis
4. Conclusions
- A maximal efficiency can be identified for a certain inlet temperature of the regeneration air stream Tr,i, which moves to lower values as εs increases;
- Ceteris paribus, at operative conditions of low εL working with higher ratios of the process to the regeneration flow rates μ, is detrimental to the thermal efficiency of the cycle, whereas the opposite can be generally advised at εL higher than 0.5;
- A maximal entropy generation per unit dry air stream flow rate corresponding to a minimal efficiency can be identified for a certain dehumidification capacity εL, when the ratio of the inlet absolute humidity of the process stream to the regeneration stream χ and the inlet temperature of the regeneration air stream Tr,i are fixed; as χ increases, the value of εL moves to lower values;
- The experimental operability of the system from Mohan et al. [32] exhibits limited cycle thermal performance;
- Higher cycle efficiency values can be achieved provided that the desiccant material is compatible with higher regeneration temperatures and the component structure, with lower flow rate ratio μ, lower sensible effectiveness εs, and higher latent effectiveness εL.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| COP | coefficient of performance |
| cp | specific heat at constant pressure, [J∙kg−1·K−1] |
| cV | specific heat at constant volume, [J∙kg−1·K−1] |
| G | dimensionless entropy generation |
| ṁ | mass flow rate, [kg∙s−1] |
| R | gas constant, [J∙mol−1·K−1] |
| S | entropy, [J∙K−1·s−1] |
| s | specific entropy, [J∙kg−1·K−1] |
| T | temperature, [K] |
| Y | air absolute humidity |
| z | dry air specific heat ratio |
| zv | vapour specific air ratio |
| zM | dry to vapour specific air ratio |
| Greek Symbol | |
| ε | effectiveness |
| η | cycle efficiency |
| μ | mass flow rate ratio |
| γ | specific heat ratio |
| χ | absolute humidity ratio |
| Subscripts | |
| a | absolute |
| amb | related to ambient |
| d | dry air |
| gen | irreversibility rate |
| H | high temperature |
| i | inlet value |
| L | latent/related to mass transfer |
| min | minimum |
| o | outlet value |
| p | related to process stream |
| r | related to regeneration stream |
| R | conditioned space (low temperature) |
| s | sensible |
| TH | thermal |
| v | related to vapour |
References
- Abdel-Salam, A.H.; Simonson, C.J. State-of-the-art in liquid desiccant air conditioning equipment and systems. Renew. Sustain. Energy Rev. 2016, 58, 1152–1183. [Google Scholar] [CrossRef]
- Pesaran, A.A. A Review of desiccant dehumidification technology. In Proceedings of the Electric Dehumidification: Energy Efficient Humidity Control for Commercial and Institutional Buildings Conference, New Orleans, LA, USA, 2–3 June 1993. [Google Scholar]
- Tu, R.; Hwang, Y.; Cao, T.; Hou, M.; Xiao, H. Investigation of adsorption isotherms and rotational speeds for low temperature regeneration of desiccant wheel systems. Int. J. Refrig. 2018, 86, 495–509. [Google Scholar] [CrossRef]
- Jani, D.B.; Mishra, M.; Sahoo, P.K. A critical review on application of solar energy as renewable regeneration heat source in solid desiccant—Vapor compression hybrid cooling system. J. Build. Eng. 2018, 18, 107–124. [Google Scholar] [CrossRef]
- Ge, T.S.; Dai, Y.J.; Wang, R.Z. Analysis on integrated low grade condensation heat powered desiccant coated vapor compression system. Appl. Therm. Eng. 2018, 138, 307–318. [Google Scholar] [CrossRef]
- Giampieri, A.; Ma, Z.; Smallbone, A.; Roskilly, A.P. Thermodynamics and economics of liquid desiccants for heating, ventilation and air-conditioning—An overview. Appl. Energy 2018, 220, 455–479. [Google Scholar] [CrossRef]
- Rafique, M.M.; Gandhidasan, P.; Bahaidarah, H.M.S. Liquid desiccant materials and dehumidifiers—A review. Renew. Sustain. Energy Rev. 2016, 56, 179–195. [Google Scholar] [CrossRef]
- Fu, H.X.; Liu, X.H. Review of the impact of liquid desiccant dehumidification on indoor air quality. Build. Environ. 2017, 116, 158–172. [Google Scholar] [CrossRef]
- Wu, X.N.; Ge, T.S.; Dai, Y.J.; Wang, R.Z. Review on substrate of solid desiccant dehumidification system. Renew. Sustain. Energy Rev. 2018, 82, 3236–3249. [Google Scholar] [CrossRef]
- Deng, Y.Q. Ionic Liquids: Property, Preparation and Application; China Petrochemical Press: Beijing, China, 2006. [Google Scholar]
- Luo, Y.M.; Shao, S.Q.; Xu, H.B.; Tian, C.Q. Dehumidification performance of [EMIM]BF4. Appl. Therm. Eng. 2011, 31, 2772–2777. [Google Scholar] [CrossRef]
- Luo, Y.; Shao, S.; Qin, F.; Tian, C.; Yang, H. Investigation on feasibility of ionic liquids used in solar liquid desiccant air conditioning system. Sol. Energy 2012, 86, 2718–2724. [Google Scholar] [CrossRef]
- Zegenhagen, M.T.; Ricart, C.; Meyer, T.; Kühn, R.; Ziegler, F. Experimental investigation of a liquid desiccant system for air dehumidification working with ionic liquids. Energy Procedia 2015, 70, 544–551. [Google Scholar] [CrossRef]
- Varela, R.J.; Giannetti, N.; Yamaguchi, S.; Saito, K.; Wang, X.M.; Nakayama, H. Experimental investigation of the wetting characteristics of an aqueous ionic liquid solution on an aluminum fin-tube substrate. Int. J. Refrig. 2018, 88, 472–482. [Google Scholar] [CrossRef]
- Giannetti, N.; Varela, R.J.; Ariyadi, H.M.; Yamaguchi, S.; Saito, K.; Wang, X.M.; Nakayama, H. Semi-theoretical prediction of the wetting characteristics of aqueous ionic liquid solution on an aluminum finned-tube desiccant contactor. ASME J. Fluids Eng. 2018, 140, 121109. [Google Scholar] [CrossRef]
- Yamaguchi, S.; Saito, K. Numerical and experimental performance analysis of rotary desiccant wheels. Int. J. Heat Mass Transf. 2013, 60, 51–60. [Google Scholar] [CrossRef]
- Varela, R.J.; Yamaguchi, S.; Giannetti, N.; Saito, K.; Harada, M.; Miyauchi, H. General correlations for the heat and mass transfer coefficients in an air-solution contactor of a liquid desiccant system and an experimental case application. Int. J. Heat Mass Transf. 2018, 120, 851–860. [Google Scholar] [CrossRef]
- Giannetti, N.; Yamaguchi, S.; Saito, K. Wetting behavior of a liquid film on an internally-cooled desiccant contactor. Int. J. Heat Mass Transf. 2016, 101, 958–969. [Google Scholar] [CrossRef]
- Ge, T.S.; Ziegler, F.; Wang, R.Z. A mathematical model for predicting the performance of a compound desiccant wheel (A model of compound desiccant wheel). Appl. Therm. Eng. 2010, 30, 1005–1015. [Google Scholar] [CrossRef]
- Jeong, J.; Yamaguchi, S.; Saito, K.; Kawai, S. Performance analysis of desiccant dehumidification systems driven by low-grade heat source. Int. J. Refrig. 2011, 34, 928–945. [Google Scholar] [CrossRef]
- Yamaguchi, S.; Jeong, J.; Saito, K.; Miyauchi, H.; Harada, M. Hybrid liquid desiccant air-conditioning system: Experiments and simulations. Appl. Therm. Eng. 2011, 31, 3741–3747. [Google Scholar] [CrossRef]
- Giannetti, N.; Arnas, A.; Rocchetti, A.; Saito, K. Thermodynamic analysis of irreversible heat-transformers. Makara J. Technol. 2015, 19, 90–96. [Google Scholar] [CrossRef]
- Giannetti, N.; Rocchetti, A.; Saito, K. Thermodynamic optimization of three-thermal irreversible systems. Int. J. Heat Technol. 2016, 34, S83–S90. [Google Scholar] [CrossRef]
- Lavan, Z.Z.; Monnier, J.B.; Worek, W.M. Second law analysis of desiccant cooling systems. J. Sol. Energy Eng. 1982, 104, 229–236. [Google Scholar] [CrossRef]
- Pons, M.; Kodama, A. Entropic analysis of adsorption open cycles for air conditioning. Part 1: First and second law analyses. Int. J. Energy Res. 2000, 24, 251–262. [Google Scholar] [CrossRef]
- Kanoğlu, M.; Bolattürk, A.; Altuntop, N. Effect of ambient conditions on the first and second law performance of an open desiccant cooling process. Renew. Energy 2007, 32, 931–946. [Google Scholar] [CrossRef]
- La, D.; Li, Y.; Dai, Y.; Ge, T.; Wang, R. Effect of irreversible processes on the thermodynamic performance of open-cycle desiccant cooling cycles. Energy Convers. Manag. 2013, 67, 44–56. [Google Scholar] [CrossRef]
- Caliskan, H.; Hepbasli, A.; Dincer, I.; Maisotsenko, V. Thermodynamic performance assessment of a novel air cooling cycle: Maisotsenko cycle. Int. J. Refrig. 2011, 34, 980–990. [Google Scholar] [CrossRef]
- Saghafifar, M.; Gadalla, M. Performance assessment of integrated PV/T and solid desiccant air-conditioning systems for cooling buildings using Maisotsenko cooling cycle. Sol. Energy 2016, 127, 79–95. [Google Scholar] [CrossRef]
- Pandelidis, D.; Anisimov, S.; Worek, W.M.; Drąg, P. Numerical analysis of a desiccant system with cross-flow Maisotsenko cycle heat and mass exchanger. Energy Build. 2016, 123, 136–150. [Google Scholar] [CrossRef]
- Giannetti, N.; Rocchetti, A.; Saito, K.; Yamaguchi, S. Entropy parameters for desiccant wheel design. Appl. Therm. Eng. 2015, 75, 826–838. [Google Scholar] [CrossRef]
- Mohan, B.S.; Tiwari, S.; Maiya, M.P. Experimental investigations on performance of liquid desiccant-vapor compression hybrid air conditioner. Appl. Therm. Eng. 2015, 77, 153–162. [Google Scholar] [CrossRef]


| Mohan et al. [32] | |||||
|---|---|---|---|---|---|
| md,r | 0.3 | kg/s | md,p | 0.2 | kg/s |
| Yr,i | 0.015 | kg/kga | Yp,i | 0.008 | kg/kga |
| Tr,i | 43 | oC | Tp,i | 11 | oC |
| Tr,o | 46.80 | oC | Tp,o | 15 | oC |
| Yr,o | 0.01633 | kg/kga | Yp,o | 0.006 | kg/kga |
| Tamb | 30 | oC | χ | 1.875 | - |
| Yamb | 0.008 | kg/kga | εs | 0.125 | - |
| μ | 0.6667 | - | εL | 0.2857 | - |
| z | 0.9977 | - | zM | 1.984 | - |
| zv | 0.9902 | - | |||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giannetti, N.; Yamaguchi, S.; Rocchetti, A.; Saito, K. Thermodynamic Analysis of Irreversible Desiccant Systems. Entropy 2018, 20, 595. https://doi.org/10.3390/e20080595
Giannetti N, Yamaguchi S, Rocchetti A, Saito K. Thermodynamic Analysis of Irreversible Desiccant Systems. Entropy. 2018; 20(8):595. https://doi.org/10.3390/e20080595
Chicago/Turabian StyleGiannetti, Niccolò, Seiichi Yamaguchi, Andrea Rocchetti, and Kiyoshi Saito. 2018. "Thermodynamic Analysis of Irreversible Desiccant Systems" Entropy 20, no. 8: 595. https://doi.org/10.3390/e20080595
APA StyleGiannetti, N., Yamaguchi, S., Rocchetti, A., & Saito, K. (2018). Thermodynamic Analysis of Irreversible Desiccant Systems. Entropy, 20(8), 595. https://doi.org/10.3390/e20080595






