Uncertainty Relations for Coarse–Grained Measurements: An Overview
Abstract
:1. Introduction
2. Uncertainty Relations
2.1. Heisenberg (or Variance) Uncertainty Relation
2.2. Entropic URs
2.2.1. Shannon-entropy UR
2.2.2. Rényi-Entropy URs
3. Utility of Uncertainty Relations in Quantum Physics
4. Realistic Coarse-Grained Measurements of Continuous Distributions
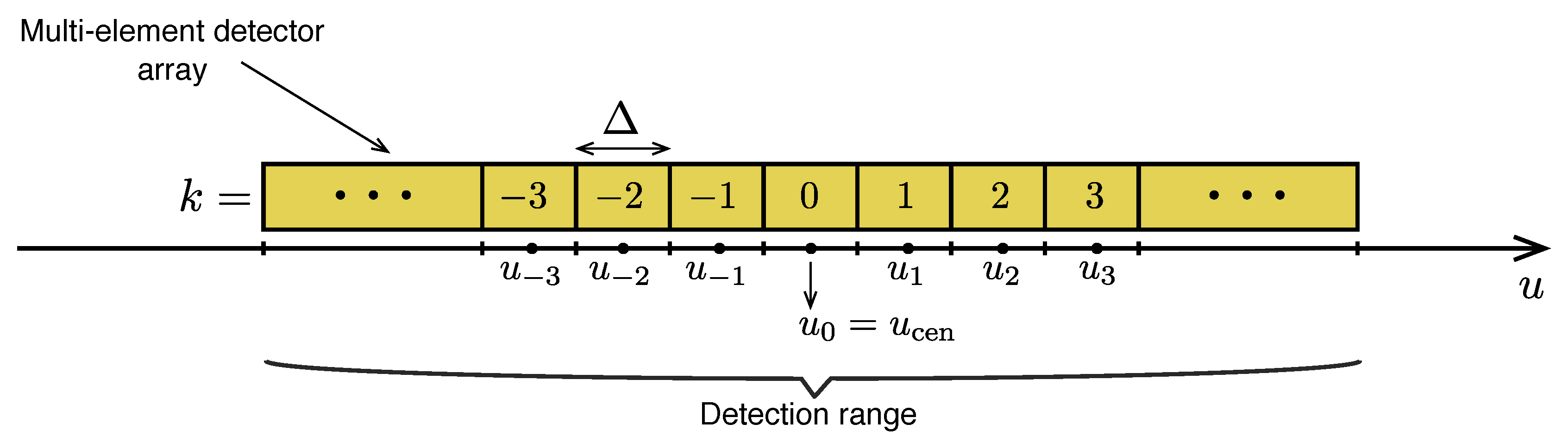
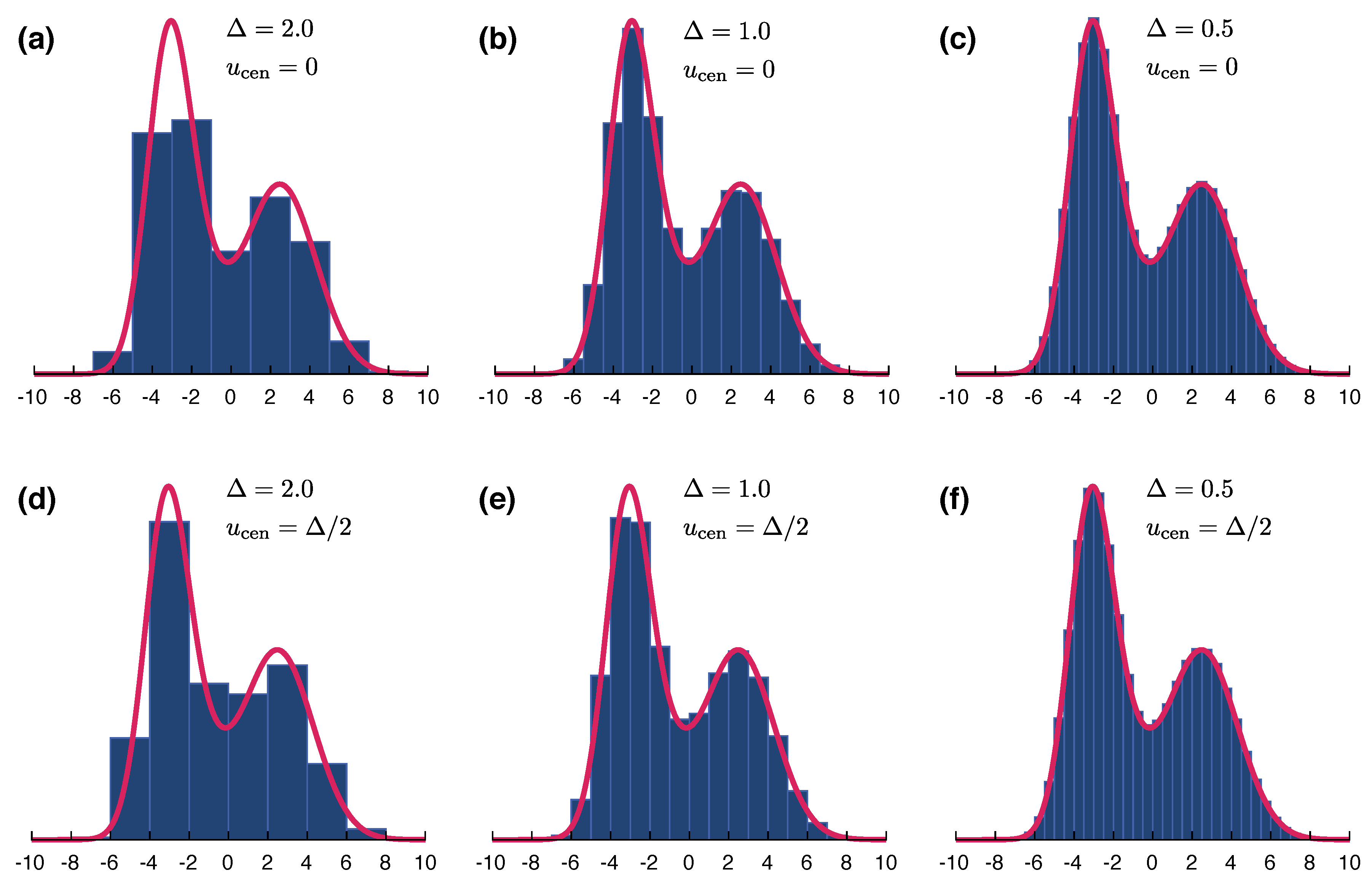
4.1. Coarse-Graining Models
4.1.1. Standard Coarse Graining
4.1.2. Periodic Coarse Graining
4.2. Mutual Unbiasedness in Coarse-Grained Measurements
5. UR for Coarse-Grained Observables
5.1. URs Proved Only for CCOs
5.2. URs Valid for General Observables, and , Defined in Equation (3)
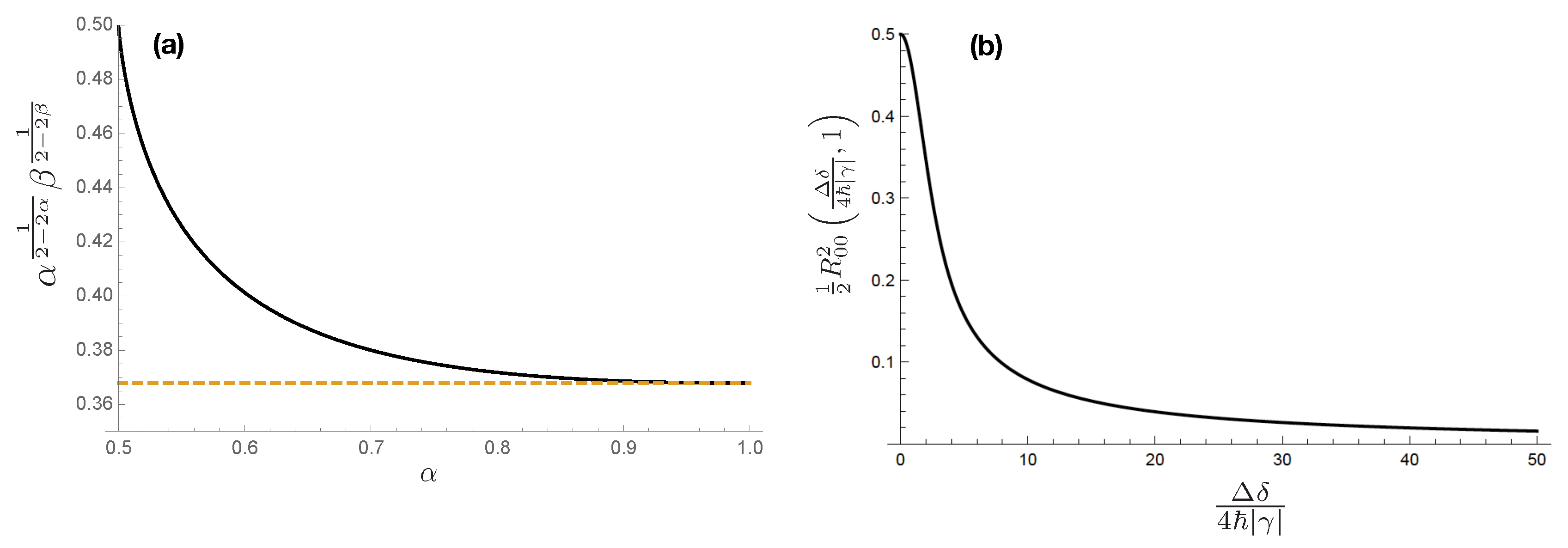
5.3. Coarse-Grained URs Merged with the Majorization Approach
5.4. Other Coarse-Grained URs
6. Applications of Coarse-Grained Measurements and Coarse-Grained Uncertainty Relations
7. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Abbreviations
| CV | Continuous variable |
| UR | Uncertainty relation |
| QIT | Quantum information theory |
| CCR | Canonical commutation relation |
| CCO | Canonically conjugate operators |
| probability distribution function | |
| EPR | Einstein-Podolsky-Rosen |
| PPT | Positive partial transposition |
| PCG | Periodic coarse graining |
| MU | Maassen-Uffink |
| HF | Histogram function |
Appendix A
Appendix B
References
- Wheeler, J.A.; Zurek, W.H. (Eds.) Quantum Theory and Measurement; Princeton University Press: Princeton, NJ, USA, 1983. [Google Scholar]
- Scully, M.O.; Englert, B.G.; Walther, H. Quantum optical tests of complementarity. Nature 1991, 351, 111–116. [Google Scholar] [CrossRef]
- Kim, Y.H.; Kulik, S.; Shih, Y.; Scully, M. Delayed Choice Quantum Eraser. Phys. Rev. Lett. 2000, 84, 1. [Google Scholar] [CrossRef] [PubMed]
- Bertet, P.; Osnaghi, S.; Rauschenbeutel, A.; Nogues, G.; Auffeves, A.; Brune, M.; Raimond, J.M.; Haroche, S. A complementarity experiment with an interferometer at the quantum-classical boundary. Nature 2001, 411, 166–170. [Google Scholar] [CrossRef] [PubMed]
- Walborn, S.P.; Cunha, M.O.T.; Pádua, S.; Monken, C.H. Double-slit quantum eraser. Phys. Rev. A 2002, 65, 0338. [Google Scholar] [CrossRef]
- Mandel, L. Coherence and indistinguishability. Opt. Lett. 1991, 16, 1882–1883. [Google Scholar] [CrossRef] [PubMed]
- Englert, B.G. Fringe Visibility and Which-Way Information: An Inequality. Phys. Rev. Lett. 1996, 77, 2154. [Google Scholar] [CrossRef] [PubMed]
- Ozaktas, H.M.; Zalevsky, Z.; Kutay, M.A. The Fractional Fourier Transform: with Applications in Optics and Signal Processing; John Wiley and Sons Ltd.: New York, NY, USA, 2001. [Google Scholar]
- Coles, P.J.; Berta, M.; Tomamichel, M.; Wehner, S. Entropic uncertainty relations and their applications. Rev. Mod. Phys. 2017, 89, 848–858. [Google Scholar] [CrossRef]
- Braunstein, S.L.; van Loock, P. Quantum information with continuous variables. Rev. Mod. Phys. 2005, 77, 513. [Google Scholar] [CrossRef]
- Adesso, G.; Ragy, S.; Lee, A.R. Continuous Variable Quantum Information: Gaussian States and Beyond. Open Syst. Inf. Dyn. 2014, 21, 1440001. [Google Scholar] [CrossRef] [Green Version]
- Bialynicki-Birula, I.; Rudnicki, Ł. Entropic Uncertainty Relations in Quantum Physics. In Statistical Complexity: Applications in Electronic Structure Chapter 1; Sen, K., Ed.; Springer: Dordrecht, The Netherlands, 2011; pp. 1–34. [Google Scholar]
- Wehner, S.; Winter, A. Entropic uncertainty relations—A survey. New J. Phys. 2010, 12, 025009. [Google Scholar] [CrossRef]
- Sperling, J.; Vogel, W. Verifying continuous-variable entanglement in finite spaces. Phys. Rev. A 2009, 79, 052313. [Google Scholar] [CrossRef]
- Willard, J. Elementary Principles in Statistical Mechanics; Scribner’s sons: New York, NY, USA, 1902. [Google Scholar]
- Ehrenfest, P.; Ehrenfest, T. Begriffliche Grundlagen der Statistischen Auffassung in der Mechanik; B. G. Teubner: Leipzig, Germany, 1912. [Google Scholar]
- Ehrenfest, P.; Ehrenfest, T. The Conceptual Foundations of the Statistical Approach in Mechanics; Dover: New York, NY, USA, 1990. [Google Scholar]
- Mackey, M. Time’s Arrow: The Origins of Thermodynamical Behavior; Springer: New York, NY, USA, 1992. [Google Scholar]
- Kofler, J.; Brukner, C. Classical World Arising out of Quantum Physics under the Restriction of Coarse-Grained Measurements. Phys. Rev. Lett. 2007, 99, 180403. [Google Scholar] [CrossRef] [PubMed]
- Kofler, J.; Brukner, C.V. Conditions for Quantum Violation of Macroscopic Realism. Phys. Rev. Lett. 2008, 101, 090403. [Google Scholar] [CrossRef] [PubMed]
- Raeisi, S.; Sekatski, P.; Simon, C. Coarse Graining Makes It Hard to See Micro-Macro Entanglement. Phys. Rev. Lett. 2011, 107, 250401. [Google Scholar] [CrossRef] [PubMed]
- Wang, T.; Ghobadi, R.; Raeisi, S.; Simon, C. Precision requirements for observing macroscopic quantum effects. Phys. Rev. A 2013, 88, 062114. [Google Scholar] [CrossRef]
- Jeong, H.; Lim, Y.; Kim, M.S. Coarsening Measurement References and the Quantum-to-Classical Transition. Phys. Rev. Lett. 2014, 112, 010402. [Google Scholar] [CrossRef] [PubMed]
- Rudnicki, L.; Walborn, S.P.; Toscano, F. Optimal uncertainty relations for extremely coarse-grained measurements. Phys. Rev. A 2012, 85, 042115. [Google Scholar] [CrossRef]
- Ray, M.R.; van Enk, S.J. Missing data outside the detector range. II. Application to time-frequency entanglement. Phys. Rev. A 2013, 88, 062327. [Google Scholar] [CrossRef]
- Tasca, D.S.; Rudnicki, L.; Gomes, R.M.; Toscano, F.; Walborn, S.P. Reliable Entanglement Detection under Coarse-Grained Measurements. Phys. Rev. Lett. 2013, 110, 210502. [Google Scholar] [CrossRef] [PubMed]
- Tasca, D.S.; Walborn, S.P.; Toscano, F.; Souto Ribeiro, P.H. Observation of tunable Popescu-Rohrlich correlations through postselection of a Gaussian state. Phys. Rev. A 2009, 80, 030101. [Google Scholar] [CrossRef]
- Semenov, A.A.; Vogel, W. Fake violations of the quantum Bell-parameter bound. Phys. Rev. A 2011, 83, 032119. [Google Scholar] [CrossRef]
- Ray, M.R.; van Enk, S.J. Missing data outside the detector range: Continuous-variable entanglement verification and quantum cryptography. Phys. Rev. A 2013, 88, 042326. [Google Scholar] [CrossRef]
- Bialynicki-Birula, I. Entropic Uncertainty Relations. Phys. Lett. 1984, 103, 253–254. [Google Scholar] [CrossRef]
- Bialynicki-Birula, I. Formulation of the uncertainty relations in terms of the Rényi entropies. Phys. Rev. A 2006, 74, 52101. [Google Scholar] [CrossRef]
- Rudnicki, Ł.; Walborn, S.P.; Toscano, F. Heisenberg uncertainty relation for coarse-grained observables. EPL 2012, 97, 38003. [Google Scholar] [Green Version]
- Heisenberg, W. Über den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. Z. Phys. 1927, 43, 172–198. [Google Scholar] [CrossRef]
- Busch, P.; Heinonen, T.; Lahti, P. Heisenberg’s uncertainty principle. Phys. Rep. 2007, 452, 155–176. [Google Scholar] [CrossRef] [Green Version]
- Ozawa, M. Universally valid reformulation of the Heisenberg uncertainty principle on noise and disturbance in measurement. Phys. Rev. A 2003, 67, 042105. [Google Scholar] [CrossRef]
- Ozawa, M. Uncertainty relations for noise and disturbance in generalized quantum measurements. Ann. Phys. 2004, 311, 350–416. [Google Scholar] [CrossRef] [Green Version]
- Ozawa, M. Universal uncertainty principle in the measurement operator formalism. J. Opt. B Quantum Semiclass. Opt. 2005, 7, S672–S681. [Google Scholar] [CrossRef]
- Werner, R.F. The Uncertainty Relation for Joint Measurement of Postion and Momentum. Quantum Inf. Comput. 2004, 4, 546–562. [Google Scholar]
- Busch, P.; Heinonen, T.; Lahti, P. Noise and disturbance in quantum measurement. Phys. Lett. A 2004, 320, 261–270. [Google Scholar] [CrossRef] [Green Version]
- Busch, P.; Lahti, P.; Werner, R.F. Proof of Heisenberg’s Error-Disturbance Relation. Phys. Rev. Lett. 2013, 111, 160405. [Google Scholar] [CrossRef] [PubMed]
- Korzekwa, K.; Lostaglio, M.; Jennings, D.; Rudolph, T. Quantum and classical entropic uncertainty relations. Phys. Rev. A 2014, 89, 042122. [Google Scholar] [CrossRef]
- Arthurs, E.; Kelly, J.L. On the Simultaneous Measurement of a Pair of Conjugate Observables. Bell Syst. Tech. J. 1965, 44, 725–729. [Google Scholar] [CrossRef]
- Davies, E.B. Quantum Theory of Open Systems; Academic Press London: New York, NY, USA, 1976; 171p. [Google Scholar]
- Busch, P. Indeterminacy relations and simultaneous measurements in quantum theory. Int. J. Theor. Phys. 1985, 24, 63–92. [Google Scholar] [CrossRef]
- Arthurs, E.; Goodman, M.S. Quantum correlations: A generalized Heisenberg uncertainty relation. Phys. Rev. Lett. 1988, 60, 2447–2449. [Google Scholar] [CrossRef] [PubMed]
- Ishikawa, S. Uncertainty relations in simultaneous measurements for arbitrary observables. Rep. Math. Phys. 1991, 29, 257–273. [Google Scholar] [CrossRef]
- Raymer, M. Uncertainty principle for joint measurement of noncommuting variables. Am. J. Phys. 1994, 62, 986. [Google Scholar] [CrossRef]
- Ozawa, M. Uncertainty relations for joint measurements of noncommuting observables. Phys. Lett. A 2004, 320, 367–374. [Google Scholar] [CrossRef] [Green Version]
- Tasca, D.S.; Gomes, R.M.; Toscano, F.; Souto Ribeiro, P.H.; Walborn, S.P. Continuous-variable quantum computation with spatial degrees of freedom of photons. Phys. Rev. A 2011, 83, 052325. [Google Scholar] [CrossRef]
- Dutta, B.; Mukunda, N.; Simon, R. The real symplectic groups in quantum mechanics and optics. Pramana 1995, 45, 471–497. [Google Scholar] [Green Version]
- Kennard, E.H. Zur Quantenmechanik einfacher Bewegungstypen. Z. Phys. 1927, 44, 326–352. [Google Scholar] [CrossRef]
- Weyl, H. Gruppentheorie und Quantenmechanik (Leipzig: S Hirzel) Weyl H 1950 The Theory of Groups and Quantum Mechanics; Dover: New York, NY, USA, 1928. [Google Scholar]
- Robertson, H. The uncertainty principle. Phys. Rev. 1929, 34, 163–164. [Google Scholar] [CrossRef]
- Schrödinger, E. On Heisenberg’s Uncertainty Principle. Phys. Math. 1930, 19, 296–303. [Google Scholar]
- Simon, R.; Mukunda, N.; Dutta, B. Quantum-noise matrix for multimode systems: U (n) invariance, squeezing, and normal forms. Phys. Rev. A 1994, 49, 1567. [Google Scholar] [CrossRef] [PubMed]
- Solomon Ivan, J.; Sabapathy, K.K.; Mukunda, N.; Simon, R. Invariant theoretic approach to uncertainty relations for quantum systems. arXiv, 2012; arXiv:1205.5132v1. [Google Scholar]
- Simon, R. Peres-Horodecki Separability Criterion for Continuous Variable Systems. Phys. Rev. Lett. 2000, 84, 2726–2729. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huang, Y. Entropic uncertainty relations in multidimensional position and momentum spaces. Phys. Rev. A 2011, 83, 052124. [Google Scholar] [CrossRef]
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Cover, T.M.; Thomas, J.A. Elements of Information Theory; John Wiley and Sons: New York, NY, USA, 2006. [Google Scholar]
- Bialynicki-Birula, I.; Mycielski, J. Uncertainty Relations for Information Entropy in Wave Mechanics. Commun. Math. Phys. 1975, 44, 129. [Google Scholar] [CrossRef]
- Beckner, W. Inequalities in Fourier Analysis. Ann. Math. 1975, 102, 159–182. [Google Scholar] [CrossRef]
- Babenko, K.I. IAn inequality in the theory of Fourier integrals. Izv. Akad. Nauk SSSR Ser. Mater. 1961, 25, 531–542. [Google Scholar]
- Hirschman, I.I. A Note on Entropy. Am. J. Math. 1957, 79, 152–156. [Google Scholar] [CrossRef]
- Guanlei, X.; Xiaotong, W.; Xiaogang, X. Generalized entropic uncertainty principle on fractional Fourier transform. Signal Process. 2009, 89, 2692–2697. [Google Scholar] [CrossRef]
- Narcowich, F.J. Geometry and uncertainty. J. Math. Phys. 1990, 31, 354–364. [Google Scholar] [CrossRef]
- Slusher, R.E.; Hollberg, L.W.; Yurke, B.; Mertz, J.C.; Valley, J.F. Observation of Squeezed States Generated by Four-Wave Mixing in an Optical Cavity. Phys. Rev. Lett. 1985, 55, 2409–2412. [Google Scholar] [CrossRef] [PubMed]
- Shchukin, E.; Richter, T.; Vogel, W. Nonclassicality criteria in terms of moments. Phys. Rev. A 2005, 71, 011802. [Google Scholar] [CrossRef]
- Vogel, W. Nonclassical States: An Observable Criterion. Phys. Rev. Lett. 2000, 84, 1849–1852. [Google Scholar] [CrossRef] [PubMed]
- Richter, T.; Vogel, W. Nonclassicality of Quantum States: A Hierarchy of Observable Conditions. Phys. Rev. Lett. 2002, 89, 283601. [Google Scholar] [CrossRef] [PubMed]
- Kiesel, T.; Vogel, W.; Hage, B.; DiGuglielmo, J.; Samblowski, A.; Schnabel, R. Experimental test of nonclassicality criteria for phase-diffused squeezed states. Phys. Rev. A 2009, 79, 022122. [Google Scholar] [CrossRef]
- Ryl, S.; Sperling, J.; Agudelo, E.; Mraz, M.; Köhnke, S.; Hage, B.; Vogel, W. Unified nonclassicality criteria. Phys. Rev. A 2015, 92, 011801. [Google Scholar] [CrossRef]
- Reid, M.D.; Drummond, P.D. Quantum Correlations of Phase in Nondegenerate Parametric Oscillation. Phys. Rev. Lett. 1988, 60, 2731–2733. [Google Scholar] [CrossRef] [PubMed]
- Reid, M.D. Demonstration of the Einstein-Podolsky-Rosen paradox using nondegenerate parametric amplification. Phys. Rev. A 1989, 40, 913–923. [Google Scholar] [CrossRef]
- Einstein, A.; Podolsky, D.; Rosen, N. Can Quantum-Mechanical Description of Physical Reality Be Considered Complete? Phys. Rev. 1935, 47, 777. [Google Scholar] [CrossRef]
- Ou, Z.Y.; Pereira, S.F.; Kimble, H.J.; Peng, K.C. Realization of the Einstein-Podolsky-Rosen paradox for continuous variables. Phys. Rev. Lett. 1992, 68, 3663–3666. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wiseman, H.M.; Jones, S.J.; Doherty, A.C. Steering, Entanglement, Nonlocality, and the Einstein-Podolsky-Rosen Paradox. Phys. Rev. Lett. 2007, 98, 140402. [Google Scholar] [CrossRef] [PubMed]
- Jones, S.J.; Wiseman, H.M.; Doherty, A.C. Entanglement, Einstein-Podolsky-Rosen correlations, Bell nonlocality, and steering. Phys. Rev. A 2007, 76, 052116. [Google Scholar] [CrossRef]
- Cavalcanti, D.; Skrzypczyk, P. Quantum steering: a review with focus on semidefinite programming. Rep. Prog. Phys. 2017, 80, 024001. [Google Scholar] [CrossRef] [PubMed]
- Schrödinger, E. The Present Status of Quantum Mechanics. Naturwissenschaften 1935, 23, 807. [Google Scholar] [CrossRef]
- Horodecki, R.; Horodecki, P.; Horodecki, M.; Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 2009, 81, 865–942. [Google Scholar] [CrossRef]
- Gühne, O.; Tóth, G. Entanglement detection. Phys. Rep. 2009, 474, 1–75. [Google Scholar] [CrossRef] [Green Version]
- Brunner, N.; Cavalcanti, D.; Pironio, S.; Scarani, V.; Wehner, S. Bell nonlocality. Rev. Mod. Phys. 2014, 86, 419–478. [Google Scholar] [CrossRef]
- Ji, S.W.; Lee, J.; Park, J.; Nha, H. Steering criteria via covariance matrices of local observables in arbitrary-dimensional quantum systems. Phys. Rev. A 2015, 92, 062130. [Google Scholar] [CrossRef]
- Walborn, S.P.; Salles, A.; Gomes, R.M.; Toscano, F.; Souto Ribeiro, P.H. Revealing Hidden Einstein-Podolsky-Rosen Nonlocality. Phys. Rev. Lett. 2011, 106, 130402. [Google Scholar] [CrossRef] [PubMed]
- Schneeloch, J.; Dixon, P.B.; Howland, G.A.; Broadbent, C.J.; Howell, J.C. Violation of Continuous-Variable Einstein-Podolsky-Rosen Steering with Discrete Measurements. Phys. Rev. Lett. 2013, 110, 130407. [Google Scholar] [CrossRef] [PubMed]
- Schneeloch, J.; Howland, G.A. Quantifying high-dimensional entanglement with Einstein-Podolsky-Rosen correlations. Phys. Rev. A 2018, 97, 042338. [Google Scholar] [CrossRef] [Green Version]
- Schneeloch, J.; Tison, C.C.; Fanto, M.L.; Alsing, P.M.; Howland, G.A. Quantifying entanglement in a 68-billion dimensional quantum system. arXiv, 2018; arXiv:1804.04515. [Google Scholar]
- Reid, M.D. Quantum cryptography with a predetermined key, using continuous-variable Einstein-Podolsky-Rosen correlations. Phys. Rev. A 2000, 62, 062308. [Google Scholar] [CrossRef]
- Grosshans, F.; Cerf, N.J. Continuous-Variable Quantum Cryptography is Secure against Non-Gaussian Attacks. Phys. Rev. Lett. 2004, 92, 047905. [Google Scholar] [CrossRef] [PubMed]
- Branciard, C.; Cavalcanti, E.G.; Walborn, S.P.; Scarani, V.; Wiseman, H.M. One-sided device-independent quantum key distribution: Security, feasibility, and the connection with steering. Phys. Rev. A 2012, 85, 010301. [Google Scholar] [CrossRef]
- Kogias, I.; Skrzypczyk, P.; Cavalcanti, D.; Acín, A.; Adesso, G. Hierarchy of Steering Criteria Based on Moments for All Bipartite Quantum Systems. Phys. Rev. Lett. 2015, 115, 210401. [Google Scholar] [CrossRef] [PubMed]
- Silberhorn, C.; Lam, P.K.; Weiß, O.; König, F.; Korolkova, N.; Leuchs, G. Generation of Continuous Variable Einstein-Podolsky-Rosen Entanglement via the Kerr Nonlinearity in an Optical Fiber. Phys. Rev. Lett. 2001, 86, 4267–4270. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bowen, W.P.; Schnabel, R.; Lam, P.K.; Ralph, T.C. Experimental Investigation of Criteria for Continuous Variable Entanglement. Phys. Rev. Lett. 2003, 90, 043601. [Google Scholar] [CrossRef] [PubMed]
- D’Angelo, M.; Kim, Y.H.; Kulik, S.P.; Shih, Y. Identifying Entanglement Using Quantum Ghost Interference and Imaging. Phys. Rev. Lett. 2004, 92, 233601. [Google Scholar] [CrossRef] [PubMed]
- Howell, J.C.; Bennink, R.S.; Bentley, S.J.; Boyd, R.W. Realization of the Einstein-Podolsky-Rosen Paradox Using Momentum- and Position-Entangled Photons from Spontaneous Parametric Down Conversion. Phys. Rev. Lett. 2004, 92, 210403. [Google Scholar] [CrossRef] [PubMed]
- Tasca, D.S.; Walborn, S.P.; Souto Ribeiro, P.H.; Toscano, F.; Pellat-Finet, P. Propagation of transverse intensity correlations of a two-photon state. Phys. Rev. A 2009, 79, 033801. [Google Scholar] [CrossRef]
- Duan, L.M.; Giedke, G.; Cirac, J.I.; Zoller, P. Inseparability Criterion for Continuous Variable Systems. Phys. Rev. Lett. 2000, 84, 2722–2725. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mancini, S.; Giovannetti, V.; Vitali, D.; Tombesi, P. Entangling Macroscopic Oscillators Exploiting Radiation Pressure. Phys. Rev. Lett. 2002, 88, 120401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Giovannetti, V.; Mancini, S.; Vitali, D.; Tombesi, P. Characterizing the entanglement of bipartite quantum systems. Phys. Rev. A 2003, 67, 022320. [Google Scholar] [CrossRef] [Green Version]
- Zhang, C.J.; Nha, H.; Zhang, Y.S.; Guo, G.C. Entanglement detection via tighter local uncertainty relations. Phys. Rev. A 2010, 81, 012324. [Google Scholar] [CrossRef]
- Peres, A. Separability Criterion for Density Matrices. Phys. Rev. Lett. 1996, 77, 1413. [Google Scholar] [CrossRef] [PubMed]
- Horedecki, M.; Horodecki, P.; Horodecki, R. Separability of mixed states: necessary and sufficient conditions. Phys. Lett. A 1996, 223, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Nha, H.; Zubairy, M.S. Uncertainty Inequalities as Entanglement Criteria for Negative Partial-Transpose States. Phys. Rev. Lett. 2008, 101, 130402. [Google Scholar] [CrossRef] [PubMed]
- Walborn, S.P.; Taketani, B.G.; Salles, A.; Toscano, F.; de Matos Filho, R.L. Entropic Entanglement Criteria for Continuous Variables. Phys. Rev. Lett. 2009, 103, 160505. [Google Scholar] [CrossRef] [PubMed]
- Saboia, A.; Toscano, F.; Walborn, S.P. Family of continuous-variable entanglement criteria using general entropy functions. Phys. Rev. A 2011, 83, 032307. [Google Scholar] [CrossRef]
- Toscano, F.; Saboia, A.; Avelar, A.T.; Walborn, S.P. Systematic construction of genuine-multipartite-entanglement criteria in continuous-variable systems using uncertainty relations. Phys. Rev. A 2015, 92, 052316. [Google Scholar] [CrossRef]
- Werner, R.F. Quantum states with Einstein-Podolsky-Rosen correlations admitting a hidden-variable model. Phys. Rev. A 1989, 40, 4277–4281. [Google Scholar] [CrossRef]
- Vidal, G.; Werner, R.F. Computable measure of entanglement. Phys. Rev. A 2002, 65, 032314. [Google Scholar] [CrossRef] [Green Version]
- Werner, R.F.; Wolf, M.M. Bound Entangled Gaussian States. Phys. Rev. Lett. 2001, 86, 3658–3661. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Horodecki, M.; Horodecki, P.; Horodecki, R. Mixed-State Entanglement and Distillation: Is there a “Bound” Entanglement in Nature? Phys. Rev. Lett. 1998, 80, 5239–5242. [Google Scholar] [CrossRef] [Green Version]
- Bennett, C.H.; Bernstein, H.J.; Popescu, S.; Schumacher, B. Concentrating partial entanglement by local operations. Phys. Rev. A 1996, 53, 2046. [Google Scholar] [CrossRef] [PubMed]
- Giedke, G.; Kraus, B.; Duan, L.M.; Zoller, P.; Cirac, I.J.; Lewenstein, M. Separability and Distillability of bipartite Gaussian States–the Complete Story. Fortschr. Phys. 2001, 49, 973–980. [Google Scholar] [CrossRef]
- Giedke, G.; Kraus, B.; Duan, L.M.; Lewenstein, M.; Cirac, I.J. Entanglement Criteria for All Bipartite Gaussian States. Phys. Rev. Lett. 2001, 87, 167904. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hyllus, P.; Eisert, J. Optimal entanglement witnesses for continuous-variable systems. New J. Phys. 2006, 8, 51. [Google Scholar] [CrossRef]
- Nha, H. Entanglement condition via su(2) and su(1,1) algebra using Schrödinger-Robertson uncertainty relation. Phys. Rev. A 2007, 76, 014305. [Google Scholar] [CrossRef]
- Agarwal, G.S.; Biswas, A. Inseparability inequalities for higher order moments for bipartite systems. New J. Phys. 2005, 7, 211. [Google Scholar] [CrossRef]
- Hillery, M.; Zubairy, M.S. Entanglement Conditions for Two-Mode States. Phys. Rev. Lett. 2006, 96, 050503. [Google Scholar] [CrossRef] [PubMed]
- Paul, E.C.; Tasca, D.S.; Rudnicki, L.; Walborn, S.P. Detecting entanglement through direct measurement of biphoton characteristic functions. 2018; submitted for publication. [Google Scholar]
- Paul, E.C.; Tasca, D.S.; Rudnicki, L.; Walborn, S.P. Detecting entanglement of continuous variables with three mutually unbiased bases. Phys. Rev. A 2016, 94, 012303. [Google Scholar] [CrossRef]
- Shchukin, E.; Vogel, W. Inseparability Criteria for Continuous Bipartite Quantum States. Phys. Rev. Lett. 2005, 95, 230502. [Google Scholar] [CrossRef] [PubMed]
- Van Loock, P.; Furusawa, A. Detecting genuine multipartite continuous-variable entanglement. Phys. Rev. A 2003, 67, 052315. [Google Scholar] [CrossRef]
- Sun, Q.; Nha, H.; Zubairy, M.S. Entanglement criteria and nonlocality for multimode continuous-variable systems. Phys. Rev. A 2009, 80, 020101. [Google Scholar] [CrossRef]
- Shchukin, E.; Vogel, W. Conditions for multipartite continuous-variable entanglement. Phys. Rev. A 2006, 74, 030302. [Google Scholar] [CrossRef]
- Villar, A.S.; Cruz, L.S.; Cassemiro, K.N.; Martinelli, M.; Nussenzveig, P. Generation of Bright Two-Color Continuous Variable Entanglement. Phys. Rev. Lett. 2005, 95, 243603. [Google Scholar] [CrossRef] [PubMed]
- Coelho, A.S.; Barbosa, F.A.S.; Cassemiro, K.N.; Villar, A.S.; Martinelli, M.; Nussenzveig, P. Three-Color Entanglement. Science 2009, 6, 823–826. [Google Scholar] [CrossRef] [PubMed]
- Tasca, D.S.; Walborn, S.P.; Ribeiro, P.H.S.; Toscano, F. Detection of transverse entanglement in phase space. Phys. Rev. A 2008, 78, 010304. [Google Scholar] [CrossRef]
- Shalm, L.K.; Hamel, D.R.; Yan, Z.; Simon, C.; Resch, K.J.; Jennewein, T. Three-photon energy-time entanglement. Nat. Phys. 2012, 9, 19–22. [Google Scholar] [CrossRef]
- MacLean, J.P.W.; Donohue, J.M.; Resch, K.J. Direct Characterization of Ultrafast Energy-Time Entangled Photon Pairs. Phys. Rev. Lett. 2018, 120, 053601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gomes, R.M.; Salles, A.; Toscano, F.; Ribeiro, P.H.S.; Walborn, S.P. Quantum Entanglement Beyond Gaussian Criteria. Proc. Natl. Acad. Sci. USA 2009, 106, 21517–21520. [Google Scholar] [CrossRef] [PubMed]
- Edgar, M.; Tasca, D.; Izdebski, F.; Warburton, R.; Leach, J.; Agnew, M.; Buller, G.; Boyd, R.; Padgett, M. Imaging high-dimensional spatial entanglement with a camera. Nat. Commun. 2012, 3, 984. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Aspden, R.S.; Tasca, D.S.; Boyd, R.W.; Padgett, M.J. EPR-based ghost imaging using a single-photon-sensitive camera. New J. Phys. 2013, 15, 073032. [Google Scholar] [CrossRef] [Green Version]
- Moreau, P.A.; Devaux, F.; Lantz, E. Einstein-Podolsky-Rosen Paradox in Twin Images. Phys. Rev. Lett. 2014, 113, 160401. [Google Scholar] [CrossRef] [PubMed]
- Tentrup, T.B.H.; Hummel, T.; Wolterink, T.A.W.; Uppu, R.; Mosk, A.P.; Pinkse, P.W.H. Transmitting more than 10 bit with a single photon. Opt. Express 2017, 25, 2826–2833. [Google Scholar] [CrossRef] [PubMed]
- Warburton, R.E.; Izdebski, F.; Reimer, C.; Leach, J.; Ireland, D.G.; Padgett, M.; Buller, G.S. Single-photon position to time multiplexing using a fiber array. Opt. Express 2011, 19, 2670–2675. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leach, J.; Warburton, R.E.; Ireland, D.G.; Izdebski, F.; Barnett, S.M.; Yao, A.M.; Buller, G.S.; Padgett, M.J. Quantum correlations in position, momentum, and intermediate bases for a full optical field of view. Phys. Rev. A 2012, 85, 013827. [Google Scholar] [CrossRef]
- Durt, T.; Englert, B.G.; Bengtsson, I.; Życzkowski, K. On Mutually Unbiased Bases. Int. J. Quant. Inf. 2010, 8, 535–640. [Google Scholar] [CrossRef]
- Aharonov, Y.; Pendleton, H.; Petersen, A. Modular variables in quantum theory. Int. J. Theor. Phys. 1969, 2, 213–230. [Google Scholar] [CrossRef]
- Busch, P.; Lahti, P.J. To what extent do position and momentum commute? Phys. Lett. A 1986, 115, 259–264. [Google Scholar] [CrossRef]
- Reiter, H.; Thirring, W. Are x and p incompatible observables? Found. Phys. 1989, 19, 1037–1039. [Google Scholar] [CrossRef]
- Ylinen, K. Commuting functions of the position and momentum observables on locally compact abelian groups. J. Math. Anal. Appl. 1989, 137, 185–192. [Google Scholar] [CrossRef]
- Tasca, D.S.; Sánchez, P.; Walborn, S.P.; Rudnicki, L. Mutual Unbiasedness in Coarse-Grained Continuous Variables. Phys. Rev. Lett. 2018, 120, 040403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Paul, E.C.; Walborn, S.P.; Tasca, D.S.; Rudnicki, L. Mutually Unbiased Coarse-Grained Measurements of Two or More Phase-Space Variables. Phys. Rev. A 2018, 97, 052103. [Google Scholar] [CrossRef]
- Vallone, G.; Marangon, D.G.; Tomasin, M.; Villoresi, P. Quantum randomness certified by the uncertainty principle. Phys. Rev. A 2014, 90, 052327. [Google Scholar] [CrossRef]
- Spengler, C.; Huber, M.; Brierley, S.; Adaktylos, T.; Hiesmayr, B.C. Entanglement detection via mutually unbiased bases. Phys. Rev. A 2012, 86, 022311. [Google Scholar] [CrossRef]
- Krenn, M.; Huber, M.; Fickler, R.; Lapkiewicz, R.; Ramelow, S.; Zeilinger, A. Generation and confirmation of a (100 × 100)-dimensional entangled quantum system. Proc. Natl. Acad. Sci. USA 2014, 111, 6243–6247. [Google Scholar] [CrossRef] [PubMed]
- Erker, P.; Krenn, M.; Huber, M. Quantifying high dimensional entanglement with two mutually unbiased bases. Quantum 2017, 1, 22. [Google Scholar] [CrossRef] [Green Version]
- Fernández-Pérez, A.; Klimov, A.B.; Saavedra, C. Quantum process reconstruction based on mutually unbiased basis. Phys. Rev. A 2011, 83, 052332. [Google Scholar] [CrossRef]
- Giovannini, D.; Romero, J.; Leach, J.; Dudley, A.; Forbes, A.; Padgett, M.J. Characterization of High-Dimensional Entangled Systems via Mutually Unbiased Measurements. Phys. Rev. Lett. 2013, 110, 143601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Weigert, S.; Wilkinson, M. Mutually unbiased bases for continuous variables. Phys. Rev. A 2008, 78, 020303. [Google Scholar] [CrossRef]
- Kraus, K. Complementary observables and uncertainty relations. Phys. Rev. D 1987, 35, 3070–3075. [Google Scholar] [CrossRef]
- Grassl, M.; McNulty, D.; Mišta, L.; Paterek, T. Small sets of complementary observables. Phys. Rev. A 2017, 95, 823–826. [Google Scholar] [CrossRef]
- Rudnicki, L.; Tasca, D.S.; Walborn, S.P. Uncertainty relations for characteristic functions. Phys. Rev. A 2016, 93, 022109. [Google Scholar] [CrossRef]
- Deutsch, D. Uncertainty in Quantum Measurements. Phys. Rev. Lett. 1983, 50, 631–633. [Google Scholar] [CrossRef]
- Maassen, H.; Uffink, J.B.M. Generalized entropic uncertainty relations. Phys. Rev. Lett. 1988, 60, 1103–1106. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Friedland, S.; Gheorghiu, V.; Gour, G. Universal Uncertainty Relations. Phys. Rev. Lett. 2013, 111, 230401. [Google Scholar] [CrossRef] [PubMed]
- Puchała, Z.; Rudnicki, Ł.; Życzkowski, K. Majorization entropic uncertainty relations. J. Phys. A Math. Theor. 2013, 46, 272002. [Google Scholar] [Green Version]
- Coles, P.J.; Piani, M. Improved entropic uncertainty relations and information exclusion relations. Phys. Rev. A 2014, 89, 022112. [Google Scholar] [CrossRef]
- Rudnicki, L.; Puchała, Z.; Życzkowski, K. Strong majorization entropic uncertainty relations. Phys. Rev. A 2014, 89, 052115. [Google Scholar] [CrossRef]
- Bosyk, G.M.; Zozor, S.; Portesi, M.; Osán, T.M.; Lamberti, P.W. Geometric approach to extend Landau-Pollak uncertainty relations for positive operator-valued measures. Phys. Rev. A 2014, 90, 052114. [Google Scholar] [CrossRef]
- Zozor, S.; Bosyk, G.M.; Portesi, M. General entropy-like uncertainty relations in finite dimensions. J. Phys. A Math. Theor. 2014, 47, 495302. [Google Scholar] [CrossRef] [Green Version]
- Kaniewski, J.M.K.; Tomamichel, M.; Wehner, S. Entropic uncertainty from effective anticommutators. Phys. Rev. A 2014, 90, 012332. [Google Scholar] [CrossRef]
- Puchała, Z.; Rudnicki, Ł.; Krawiec, A.; Życzkowski, K. Majorization uncertainty relations for mixed quantum states. J. Phys. A Math. Theor. 2018, 51, 175306. [Google Scholar] [Green Version]
- Brandão, F.; Horodecki, M.; Ng, N.; Oppenheim, J.; Wehner, S. The second laws of quantum thermodynamics. Proc. Natl. Acad. Sci. USA 2015, 112, 3275–3279. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Partovi, M.H. Entropic Formulation of Uncertainty for Quantum Measurements. Phys. Rev. Lett. 1983, 50, 1883–1885. [Google Scholar] [CrossRef]
- Rudnicki, Ł. Shannon entropy as a measure of uncertainty in positions and momenta. J. Russ. Laser Res. 2011, 32, 393. [Google Scholar] [CrossRef]
- Partovi, M.H. Majorization formulation of uncertainty in quantum mechanics. Phys. Rev. A 2011, 84, 052117. [Google Scholar] [CrossRef]
- Schürmann, T.; Hoffmann, I. A Closer Look at the Uncertainty Relation of Position and Momentum. Found. Phys. 2009, 39, 958–963. [Google Scholar] [CrossRef] [Green Version]
- Schürmann, T. A note on entropic uncertainty relations of position and momentum. J. Russ. Laser Res. 2012, 33, 52–54. [Google Scholar] [CrossRef] [Green Version]
- Wilk, G.; Włodarczyk, Z. Uncertainty relations in terms of the Tsallis entropy. Phys. Rev. A 2009, 79, 062108. [Google Scholar] [CrossRef]
- Bialynicki-Birula, I.; Rudnicki, L. Comment on “Uncertainty relations in terms of the Tsallis entropy”. Phys. Rev. A 2010, 81, 026101. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I. Handbook of Mathematical Functions; Dover: New York, NY, USA, 1964. [Google Scholar]
- Lassance, N. Optimal RRnyi Entropy Portfolios. SSRN Electron. J. 2017, 1–15. [Google Scholar] [CrossRef]
- Rudnicki, L. Majorization approach to entropic uncertainty relations for coarse-grained observables. Phys. Rev. A 2015, 91, 032123. [Google Scholar] [CrossRef]
- Fuchs, W. On the eigenvalues of an integral equation arising in the theory of band-limited signals. J. Math. Anal. Appl. 1964, 9, 317–330. [Google Scholar] [CrossRef]
- Ballentine, L. Quantum Mechanics: A Modern Development; World Scientific: Singapore, 1998. [Google Scholar]
- Kofler, J.; Brukner, Č. A Coarse-Grained Schrödinger Cat; IOS Press: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Bialynicki-Birula, I.; Madajczyk, J. Entropic uncertainty relations for angular distributions. Phys. Lett. A 1985, 108, 384–386. [Google Scholar] [CrossRef]
- Furrer, F.; Berta, M.; Tomamichel, M.; Scholz, V.B.; Christandl, M. Position-momentum uncertainty relations in the presence of quantum memory. J. Math. Phys. 2014, 55, 122205. [Google Scholar] [CrossRef] [Green Version]
- Berta, M.; Matthias Christandl and, R.C.; Renes, J.M.; Renner, R. The uncertainty principle in the presence of quantum memory. Nat. Phys. 2010, 6, 659. [Google Scholar] [CrossRef]
- Rastegin, A.E. On entropic uncertainty relations in the presence of a minimal length. Ann. Phys. 2017, 382, 170–180. [Google Scholar] [CrossRef] [Green Version]
- Tasca, D.S.; Rudnicki, L.; Aspden, R.S.; Padgett, M.J.; Souto Ribeiro, P.H.; Walborn, S.P. Testing for entanglement with periodic coarse graining. Phys. Rev. A 2018, 97, 042312. [Google Scholar] [CrossRef] [Green Version]
- Park, J.; Ji, S.W.; Lee, J.; Nha, H. Gaussian states under coarse-grained continuous variable measurements. Phys. Rev. A 2014, 89, 042102. [Google Scholar] [CrossRef]
- Gilchrist, A.; Deuar, P.; Reid, M.D. Contradiction of Quantum Mechanics with Local Hidden Variables for Quadrature Phase Amplitude Measurements. Phys. Rev. Lett. 1998, 80, 3169–3172. [Google Scholar] [CrossRef] [Green Version]
- Gilchrist, A.; Deuar, P.; Reid, M.D. Contradiction of quantum mechanics with local hidden variables for quadrature phase measurements on pair-coherent states and squeezed macroscopic superpositions of coherent states. Phys. Rev. A 1999, 60, 4259–4271. [Google Scholar] [CrossRef] [Green Version]
- Munro, W.J. Optimal states for Bell-inequality violations using quadrature-phase homodyne measurements. Phys. Rev. A 1999, 59, 4197–4201. [Google Scholar] [CrossRef] [Green Version]
- García-Patrón, R.; Fiurášek, J.; Cerf, N.J.; Wenger, J.; Tualle-Brouri, R.; Grangier, P. Proposal for a Loophole-Free Bell Test Using Homodyne Detection. Phys. Rev. Lett. 2004, 93, 130409. [Google Scholar] [CrossRef] [PubMed]
- Wenger, J.; Hafezi, M.; Grosshans, F.; Tualle-Brouri, R.; Grangier, P. Maximal violation of Bell inequalities using continuous-variable measurements. Phys. Rev. A 2003, 67, 012105. [Google Scholar] [CrossRef]
- Aharanov, Y.; Rohrlich, D. Quantum Paradoxes; Wiley: Berlin, Germany, 2005. [Google Scholar]
- Vernaz-Gris, P.; Ketterer, A.; Keller, A.; Walborn, S.P.; Coudreau, T.; Milman, P. Continuous discretization of infinite-dimensional Hilbert spaces. Phys. Rev. A 2014, 89, 052311. [Google Scholar] [CrossRef] [Green Version]
- Ketterer, A.; Keller, A.; Walborn, S.P.; Coudreau, T.; Milman, P. Quantum information processing in phase space: A modular variables approach. Phys. Rev. A 2016, 94, 022325. [Google Scholar] [CrossRef]
- Gneiting, C.; Hornberger, K. Detecting Entanglement in Spatial Interference. Phys. Rev. Lett. 2011, 106, 210501. [Google Scholar] [CrossRef] [PubMed]
- Carvalho, M.A.D.; Ferraz, J.; Borges, G.F.; de Assis, P.L.; Pádua, S.; Walborn, S.P. Experimental observation of quantum correlations in modular variables. Phys. Rev. A 2012, 86, 032332. [Google Scholar] [CrossRef]
- Barros, M.R.; Farías, O.J.; Keller, A.; Coudreau, T.; Milman, P.; Walborn, S.P. Detecting multipartite spatial entanglement with modular variables. Phys. Rev. A 2015, 92, 022308. [Google Scholar] [CrossRef]
- Massar, S.; Pironio, S. Greenberger-Horne-Zeilinger paradox for continuous variables. Phys. Rev. A 2001, 64, 062108. [Google Scholar] [CrossRef]
- Plastino, A.R.; Cabello, A. State-independent quantum contextuality for continuous variables. Phys. Rev. A 2010, 82, 022114. [Google Scholar] [CrossRef]
- Asadian, A.; Budroni, C.; Steinhoff, F.E.S.; Rabl, P.; Gühne, O. Contextuality in phase space. arXiv, 2015; arXiv:1502.05799. [Google Scholar]
- Laversanne-Finot, A.; Ketterer, A.; Barros, M.R.; Walborn, S.P.; Coudreau, T.; Keller, A.; Milman, P. General conditions for maximal violation of non-contextuality in discrete and continuous variables. J. Phys. A Math. Theor. 2017, 50, 155304. [Google Scholar] [CrossRef] [Green Version]
- Asadian, A.; Erker, P.; Huber, M.; Klöckl, C. Heisenberg-Weyl Observables: Bloch vectors in phase space. Phys. Rev. A 2016, 94, 010301. [Google Scholar] [CrossRef]
- Chang, L.N.; Lewis, Z.; Minic, D.; Takeuchi, T. On the Minimal Length Uncertainty Relation and the Foundations of String Theory. Adv. High Energy Phys. 2011, 2011, 493514. [Google Scholar] [CrossRef]
- Tawfik, A.N.; Diab, A.M. Review on Generalized Uncertainty Principle. Rep. Prog. Phys. 2015, 78, 126001. [Google Scholar] [CrossRef] [PubMed]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Toscano, F.; Tasca, D.S.; Rudnicki, Ł.; Walborn, S.P. Uncertainty Relations for Coarse–Grained Measurements: An Overview. Entropy 2018, 20, 454. https://doi.org/10.3390/e20060454
Toscano F, Tasca DS, Rudnicki Ł, Walborn SP. Uncertainty Relations for Coarse–Grained Measurements: An Overview. Entropy. 2018; 20(6):454. https://doi.org/10.3390/e20060454
Chicago/Turabian StyleToscano, Fabricio, Daniel S. Tasca, Łukasz Rudnicki, and Stephen P. Walborn. 2018. "Uncertainty Relations for Coarse–Grained Measurements: An Overview" Entropy 20, no. 6: 454. https://doi.org/10.3390/e20060454
APA StyleToscano, F., Tasca, D. S., Rudnicki, Ł., & Walborn, S. P. (2018). Uncertainty Relations for Coarse–Grained Measurements: An Overview. Entropy, 20(6), 454. https://doi.org/10.3390/e20060454




