New Forms of Quantum Value Indefiniteness Suggest That Incompatible Views on Contexts Are Epistemic
Abstract
:1. Quantum Contexts as Views on States
2. Probabilities on Contexts in Quantum Mechanics
- A1
- probabilities are real-valued and non-negative: , and for all , or, equivalently, ;
- A2
- probabilities of mutually-exclusive observables within contexts are additive:
- A3
- probabilities within one context add up to one: .
- (i)
- For each closed subspace spanned by the vectors in the context , take the projection of onto .
- (ii)
- Take the absolute square of the length (norm) of this projection and identify it with the probability of finding the quantum system that is in state to be in state ; that is (the symbol “†” stands for the Hermitian adjoint):
3. Contexts in Partition Logics and Their Probabilities
4. Probabilities on Pastings or Stitchings of Contexts
- (i)
- Enumerate all truth assignments (or two--valued measures or states) .
- (ii)
- The (quasi)classical probabilities are obtained by the formation of the convex sum over all such states obtained in (i), with and .
- (ii)
- The Bell-type bounds on probabilities and expectations are obtained by bundling these truth assignments into vectors, one per two-valued measure, with the coordinates representing the respective values of those states on the atoms (propositions, observables) of the logic; and by subsequently solving the hull problem for a convex polytope whose vertices are identified with the vectors formed by all truth assignments [20,21,22,23].
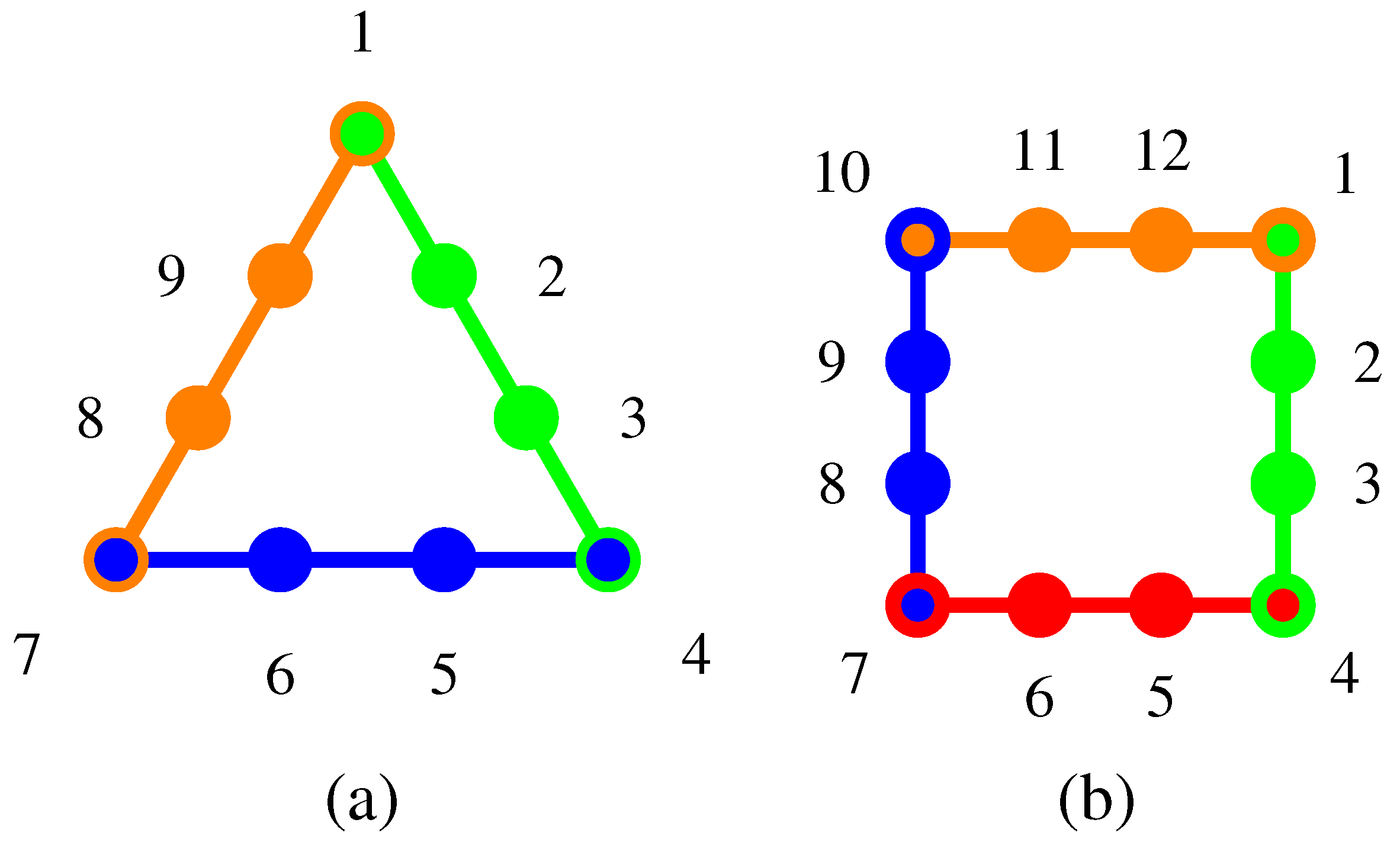
4.1. Triangular and Square Logics in Four Dimensions
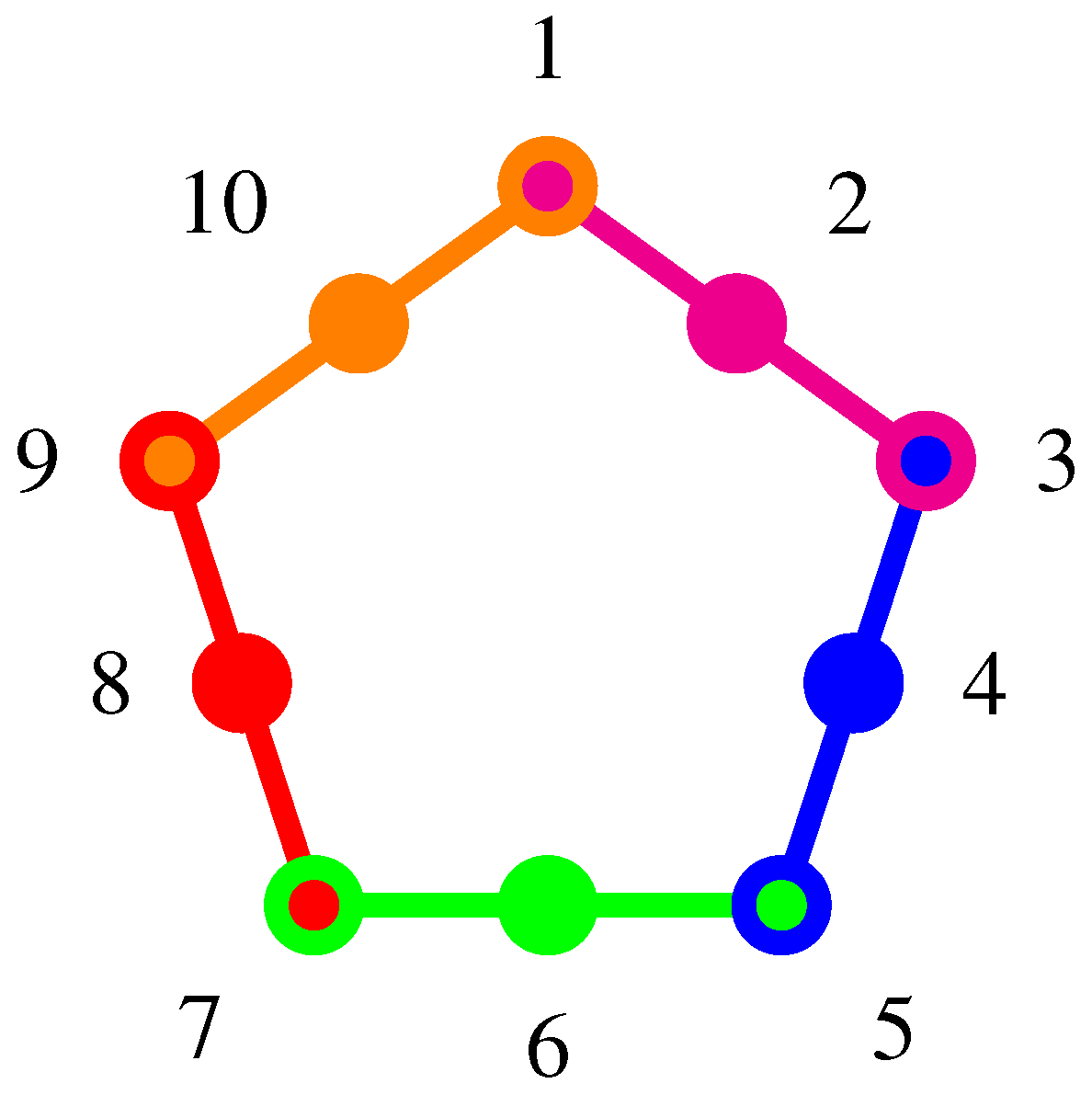
4.2. Pentagon (Pentagram) Logic
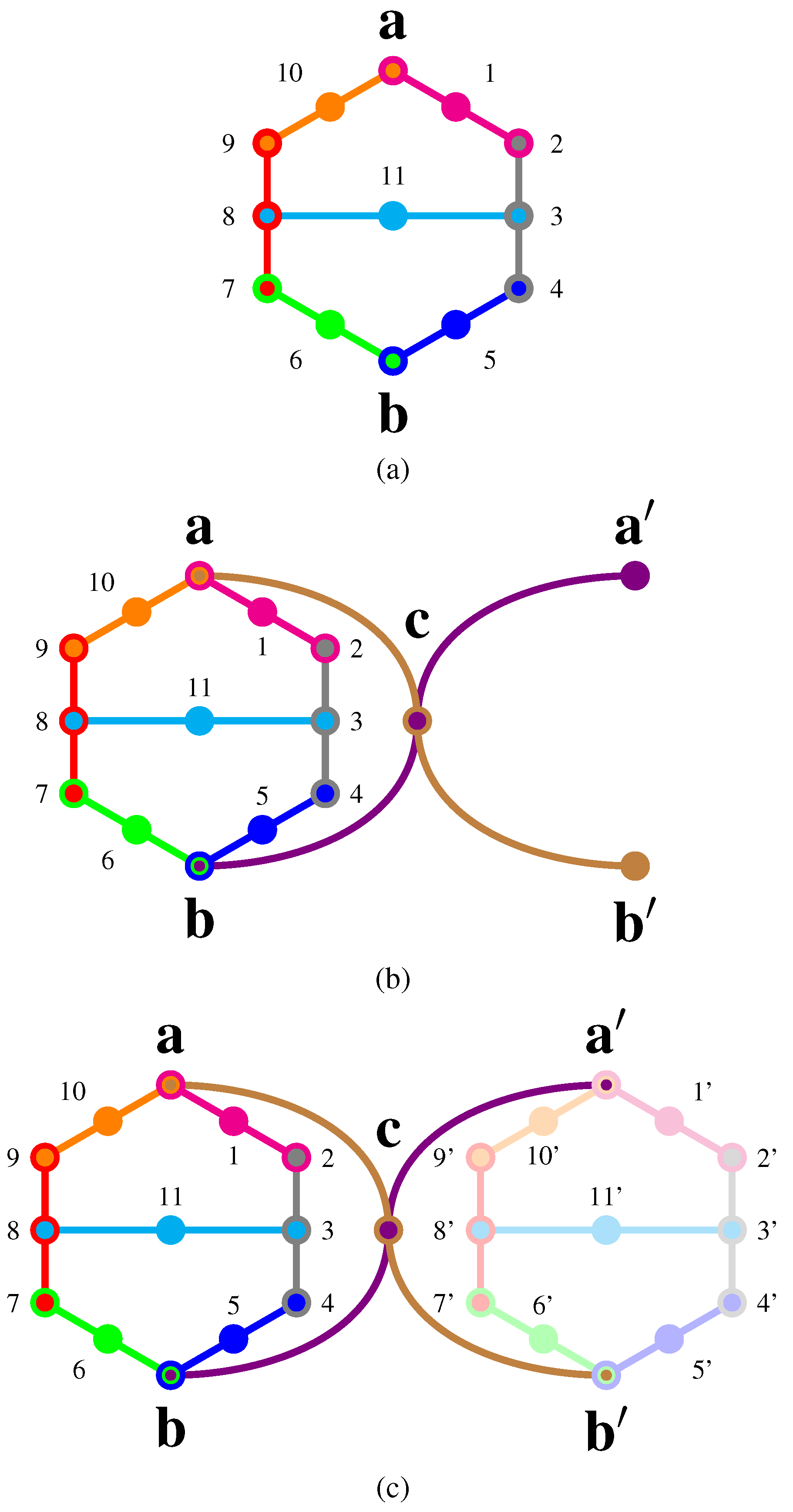
4.3. Specker Bug Logic with the True-Implies-False Property
4.4. Combo of Specker Bug Logic with the True-Implies-True, as Well as Inseparability Properties
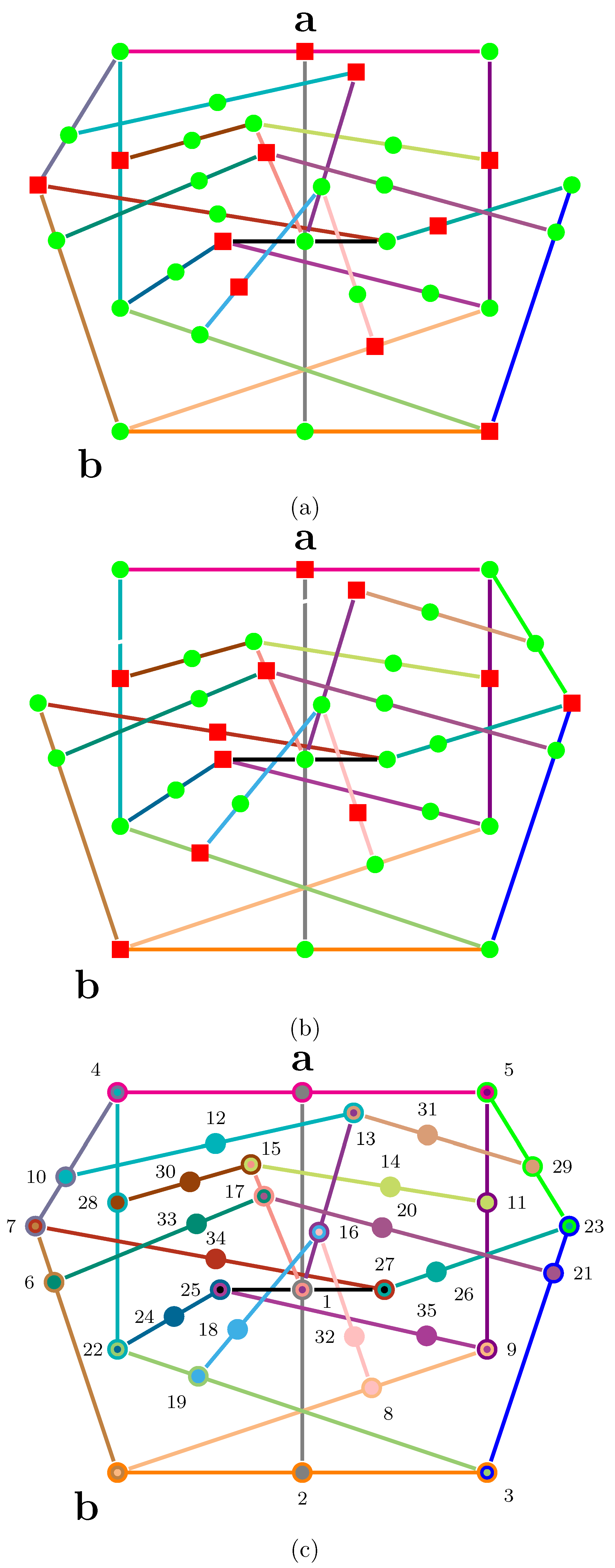
4.5. Logics Inducing Partial Value (In)Definiteness
- (i)
- Find a logic (collection of intertwined contexts of observables) exhibiting a true-implies-false property on the two atoms and .
- (ii)
- Find another logic exhibiting a true-implies-true property on the same two atoms and .
- (iii)
- Then, join (paste) these logics into a larger logic, which, given , neither allows to be true nor false. Consequently, must be value indefinite.
5. Propositional Logic Does Not Uniquely Determine Probabilities
6. Some Platonist Afterthoughts
Acknowledgments
Conflicts of Interest
Appendix A. Two-Valued States, (Quasi)Classical Probabilities on the Triangular Logic in Four Dimensions
Appendix B. Truth Assignments, (Quasi)Classical Probabilities on the Square Logic in Four Dimensions
Appendix C. Two-Valued States, (Quasi)Classical Probabilities on the Pentagon (Pentagram) Logic in Three Dimensions
Appendix D. Truth Assignments, (Quasi)Classical Probabilities on the Specker Bug Combo Logic
Appendix E. Truth Assignments, (Quasi)Classical Probabilities on Truth-Implies-Value Indefiniteness Logic in Three Dimensions
References
- Gleason, A.M. Measures on the closed subspaces of a Hilbert space. J. Math. Mech. 1957, 6, 885–893. [Google Scholar] [CrossRef]
- Mermin, D.N. Quantum Computer Science; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Halmos, P.R. Finite-Dimensional Vector Spaces; Undergraduate Texts in Mathematics; Springer: New York, NY, USA, 1958. [Google Scholar]
- Svozil, K. Randomness & Undecidability in Physics; World Scientific: Singapore, 1993. [Google Scholar]
- Dvurečenskij, A.; Pulmannová, S.; Svozil, K. Partition Logics, Orthoalgebras and Automata. Helv. Phys. Acta 1995, 68, 407–428. [Google Scholar]
- Svozil, K. Quantum Logic; Springer: Singapore, 1998. [Google Scholar]
- Svozil, K. Logical equivalence between generalized urn models and finite automata. Int. J. Theor. Phys. 2005, 44, 745–754. [Google Scholar] [CrossRef]
- Svozil, K. Contexts in quantum, classical and partition logic. In Handbook of Quantum Logic and Quantum Structures; Engesser, K., Gabbay, D.M., Lehmann, D., Eds.; Elsevier: Amsterdam, The Netherland, 2009; pp. 551–586. [Google Scholar]
- Svozil, K. Generalized event structures and probabilities. In Information and Complexity; Burgin, M., Calude, C.S., Eds.; World Scientific Series in Information Studies; World Scientific: Singapore, 2016; Volume 6, Chapter 11; pp. 276–300. [Google Scholar] [CrossRef]
- Svozil, K. Physical [A]Causality. Determinism, Randomness and Uncaused Events; Springer: Cham, Switzerland; Berlin/Heidelberg, Germany; New York, NY, USA, 2018. [Google Scholar]
- Chevalier, G. Commutators and decompositions of orthomodular lattices. Order 1989, 6, 181–194. [Google Scholar] [CrossRef]
- Moore, E.F. Gedanken-Experiments on Sequential Machines. In Automata Studies; Shannon, C.E., McCarthy, J., Eds.; Princeton University Press: Princeton, NJ, USA, 1956; pp. 129–153. [Google Scholar]
- Schaller, M.; Svozil, K. Automaton partition logic versus quantum logic. Int. J. Theor. Phys. 1995, 34, 1741–1750. [Google Scholar] [CrossRef]
- Schaller, M.; Svozil, K. Automaton logic. Int. J. Theor. Phys. 1996, 35. [Google Scholar] [CrossRef]
- Wright, R. Generalized urn models. Found. Phys. 1990, 20, 881–903. [Google Scholar] [CrossRef]
- Svozil, K. Staging quantum cryptography with chocolate balls. Am. J. Phys. 2006, 74, 800–803. [Google Scholar] [CrossRef]
- Svozil, K. Non-contextual chocolate ball versus value indefinite quantum cryptography. Theor. Comput. Sci. 2014, 560, 82–90. [Google Scholar] [CrossRef]
- Svozil, K. On generalized probabilities: Correlation polytopes for automaton logic and generalized urn models, extensions of quantum mechanics and parameter cheats. arXiv, 2000; arXiv:quant-ph/0012066. [Google Scholar]
- Boole, G. On the Theory of Probabilities. Philos. Trans. R. Soc. Lond. 1862, 152, 225–252. [Google Scholar] [CrossRef]
- Froissart, M. Constructive generalization of Bell’s inequalities. Il Nuovo Cimento B 1981, 64, 241–251. [Google Scholar] [CrossRef]
- Cirel’son, B.S. Some results and problems on quantum Bell-type inequalities. Hadron. J. Suppl. 1993, 8, 329–345. [Google Scholar]
- Pitowsky, I. The range of quantum probabilities. J. Math. Phys. 1986, 27, 1556–1565. [Google Scholar] [CrossRef]
- Pitowsky, I. George Boole’s ‘Conditions of Possible Experience’ and the Quantum Puzzle. Br. J. Philos. Sci. 1994, 45, 95–125. [Google Scholar] [CrossRef]
- Richard, J. Orthomodular lattices admitting no states. J. Comb. Theory Ser. A 1971, 10, 119–132. [Google Scholar]
- Fukuda, K. cdd and cddplus Homepage, cddlib Package cddlib-094h, 2000. Available online: http://www.inf.ethz.ch/personal/fukudak/cdd_home/ (accessed on 1 July 2017).
- Wright, R. The state of the pentagon. A nonclassical example. In Mathematical Foundations of Quantum Theory; Marlow, A.R., Ed.; Academic Press: New York, NY, USA, 1978; pp. 255–274. [Google Scholar]
- Kalmbach, G. Orthomodular Lattices (London Mathematical Society Monographs); Academic Press: London, UK; New York, NY, USA, 1983; Volume 18. [Google Scholar]
- Beltrametti, E.G.; Maçzyński, M.J. On the range of non-classical probability. Rep. Math. Phys. 1995, 36. [Google Scholar] [CrossRef]
- Klyachko, A.A.; Can, M.A.; Binicioğlu, S.; Shumovsky, A.S. Simple Test for Hidden Variables in Spin-1 Systems. Phys. Rev. Lett. 2008, 101, 020403. [Google Scholar] [CrossRef] [PubMed]
- Bub, J.; Stairs, A. Contextuality and Nonlocality in ‘No Signaling’ Theories. Found. Phys. 2009, 39. [Google Scholar] [CrossRef]
- Bub, J.; Stairs, A. Contextuality in Quantum Mechanics: Testing the Klyachko Inequality. arXiv, 2010; arXiv:1006.0500. [Google Scholar]
- Badzia̧g, P.; Bengtsson, I.; Cabello, A.; Granström, H.; Larsson, J.A. Pentagrams and Paradoxes. Found. Phys. 2011, 41. [Google Scholar] [CrossRef]
- Kochen, S.; Specker, E.P. Logical Structures arising in quantum theory. In The Theory of Models, Proceedings of the 1963 International Symposium at Berkeley; North Holland: Amsterdam, The Netherland; New York, NY, USA; Oxford, UK, 1965; pp. 177–189. [Google Scholar]
- Kochen, S.; Specker, E.P. The Problem of Hidden Variables in Quantum Mechanics. J. Math. Mech. 1967, 17, 59–87. [Google Scholar] [CrossRef]
- Redhead, M. Incompleteness, Nonlocality, and Realism: A Prolegomenon to the Philosophy of Quantum Mechanics; Clarendon Press: Oxford, UK, 1990. [Google Scholar]
- Pitowsky, I. Betting on the outcomes of measurements: A Bayesian theory of quantum probability. Stud. Hist. Philos. Sci. Part B Stud. Hist. Philos. Mod. Phys. 2003, 34, 395–414. [Google Scholar] [CrossRef]
- Pitowsky, I. Quantum Mechanics as a Theory of Probability. In Physical Theory and Its Interpretation; Demopoulos, W., Pitowsky, I., Eds.; The Western Ontario Series in Philosophy of Science; Springer: Dordrecht, The Netherlands, 2006; Volume 72, pp. 213–240. [Google Scholar]
- Belinfante, F.J. A Survey of Hidden-Variables Theories; International Series of Monographs in Natural Philosophy; Pergamon Press, Elsevier: Oxford, UK; New York, NY, USA, 1973; Volume 55. [Google Scholar]
- Stairs, A. Quantum logic, realism, and value definiteness. Philos. Sci. 1983, 50, 578–602. [Google Scholar] [CrossRef]
- Getting contextual and nonlocal elements-of-reality the easy way. Am. J. Phys. 1993, 61, 443–447. [CrossRef]
- Pták, P.; Pulmannová, S. Orthomodular Structures as Quantum Logics. Intrinsic Properties, State Space and Probabilistic Topics; Fundamental Theories of Physics; Kluwer Academic Publishers, Springer: Dordrecht, The Netherlands, 1991; Volume 44. [Google Scholar]
- Navara, M.; Rogalewicz, V. The pasting constructions for orthomodular posets. Math. Nachr. 1991, 154, 157–168. [Google Scholar] [CrossRef]
- Johansen, H.B. Comment on Getting contextual and nonlocal elements-of-reality the easy way. Am. J. Phys. 1994, 62, 471. [Google Scholar] [CrossRef]
- Vermaas, P.E. Comment on Getting contextual and nonlocal elements-of-reality the easy way. Am. J. Phys. 1994, 62, 658. [Google Scholar] [CrossRef]
- Cabello, A.; Portillo, J.R.; Solís, A.; Svozil, K. Minimal true-implies-false and true-implies-true sets of propositions in noncontextual hidden variable theories. arXiv, 2013; arXiv:1805.00796. [Google Scholar]
- Svozil, K. Quantum Scholasticism: On Quantum Contexts, Counterfactuals, and the Absurdities of Quantum Omniscience. Inf. Sci. 2009, 179, 535–541. [Google Scholar] [CrossRef]
- Cabello, A. A simple proof of the Kochen-Specker theorem. Eur. J. Phys. 1994, 15, 179–183. [Google Scholar] [CrossRef]
- Cabello, A. Pruebas Algebraicas de Imposibilidad de Variables Ocultas en Mecánica Cuántica. Ph.D. Thesis, Universidad Complutense de Madrid, Madrid, Spain, 1996. [Google Scholar]
- Tkadlec, J. Greechie diagrams of small quantum logics with small state spaces. Int. J. Theor. Phys. 1998, 37, 203–209. [Google Scholar] [CrossRef]
- Svozil, K.; Tkadlec, J. Greechie diagrams, nonexistence of measures in quantum logics and Kochen–Specker type constructions. J. Math. Phys. 1996, 37, 5380–5401. [Google Scholar] [CrossRef]
- Abbott, A.A.; Calude, C.S.; Svozil, K. A variant of the Kochen-Specker theorem localising value indefiniteness. J. Math. Phys. 2015, 56, 102201. [Google Scholar] [CrossRef]
- Pitowsky, I. Substitution and Truth in Quantum Logic. Philos. Sci. 1982, 49. [Google Scholar] [CrossRef]
- Hardy, L. Quantum mechanics, local realistic theories, and Lorentz-invariant realistic theories. Phys. Rev. Lett. 1992, 68, 2981–2984. [Google Scholar] [CrossRef] [PubMed]
- Hardy, L. Nonlocality for two particles without inequalities for almost all entangled states. Phys. Rev. Lett. 1993, 71, 1665–1668. [Google Scholar] [CrossRef] [PubMed]
- Boschi, D.; Branca, S.; De Martini, F.; Hardy, L. Ladder Proof of Nonlocality without Inequalities: Theoretical and Experimental Results. Phys. Rev. Lett. 1997, 79, 2755–2758. [Google Scholar] [CrossRef]
- Cabello, A.; García-Alcaine, G. A hidden-variables versus quantum mechanics experiment. J. Phys. A Math. Gen. Phys. 1995, 28. [Google Scholar] [CrossRef]
- Cabello, A.; Estebaranz, J.M.; García-Alcaine, G. Bell-Kochen-Specker theorem: A proof with 18 vectors. Phys. Lett. A 1996, 212, 183–187. [Google Scholar] [CrossRef]
- Cabello, A. No-hidden-variables proof for two spin- particles preselected and postselected in unentangled states. Phys. Rev. A 1997, 55, 4109–4111. [Google Scholar] [CrossRef]
- Chen, J.L.; Cabello, A.; Xu, Z.P.; Su, H.Y.; Wu, C.; Kwek, L.C. Hardy’s paradox for high-dimensional systems. Phys. Rev. A 2013, 88, 062116. [Google Scholar] [CrossRef]
- Cabello, A.; Badziag, P.; Terra Cunha, M.; Bourennane, M. Simple Hardy-Like Proof of Quantum Contextuality. Phys. Rev. Lett. 2013, 111, 180404. [Google Scholar] [CrossRef] [PubMed]
- Pitowsky, I. Infinite and finite Gleason’s theorems and the logic of indeterminacy. J. Math. Phys. 1998, 39, 218–228. [Google Scholar] [CrossRef]
- Hrushovski, E.; Pitowsky, I. Generalizations of Kochen and Specker’s theorem and the effectiveness of Gleason’s theorem. Stud. Hist. Philos. Sci. Part B Stud. Hist. Philos. Mod. Phys. 2004, 35, 177–194. [Google Scholar] [CrossRef]
- Abbott, A.A.; Calude, C.S.; Conder, J.; Svozil, K. Strong Kochen-Specker theorem and incomputability of quantum randomness. Phys. Rev. A 2012, 86, 062109. [Google Scholar] [CrossRef]
- Abbott, A.A.; Calude, C.S.; Svozil, K. Value-indefinite observables are almost everywhere. Phys. Rev. A 2014, 89, 032109. [Google Scholar] [CrossRef]
- Pitowsky, I. Deterministic model of spin and statistics. Phys. Rev. D 1983, 27, 2316–2326. [Google Scholar] [CrossRef]
- Meyer, D.A. Finite precision measurement nullifies the Kochen-Specker theorem. Phys. Rev. Lett. 1999, 83, 3751–3754. [Google Scholar] [CrossRef]
- Schrödinger, E. Die gegenwärtige Situation in der Quantenmechanik. Naturwissenschaften 1935, 23, 807–812. [Google Scholar] [CrossRef]
- London, F.; Bauer, E. The Theory of Observation in Quantum Mechanics. In Quantum Theory and Measurement; Princeton University Press: Princeton, NJ, USA, 1983; pp. 217–259. [Google Scholar]
- Svozil, K. Quantum information via state partitions and the context translation principle. J. Mod. Opt. 2004, 51, 811–819. [Google Scholar] [CrossRef]
- Howard, D. Who Invented the “Copenhagen Interpretation”? A Study in Mythology. Philos. Sci. 2004, 71, 669–682. [Google Scholar] [CrossRef]
- Bohr, N. The quantum postulate and the recent development of atomistic theory. Nature 1928, 121, 580–590. [Google Scholar] [CrossRef]
- Von Neumann, J. Mathematische Grundlagen der Quantenmechanik, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Zeilinger, A. A Foundational Principle for Quantum Mechanics. Found. Phys. 1999, 29, 631–643. [Google Scholar] [CrossRef]
- Myrvold, W.C. Statistical mechanics and thermodynamics: A Maxwellian view. Stud. Hist. Philos. Sci. Part B Stud. Hist. Philos. Mod. Phys. 2011, 42, 237–243. [Google Scholar] [CrossRef]
- Specker, E. Die Logik nicht gleichzeitig entscheidbarer Aussagen. Dialectica 1960, 14, 239–246. [Google Scholar] [CrossRef]
- Kochen, S.; Specker, E.P. The calculus of partial propositional functions. In Proceedings of the 1964 International Congress for Logic, Methodology and Philosophy of Science, Jerusalem, Israel, 26 August–2 September 1964; North Holland: Amsterdam, The Netherlands, 1965; pp. 45–57. [Google Scholar]
- Valentini, A. The de Broglie-Bohm Pilot-Wave Theory; Lecture Series on Foundations of Physics: Scientific Realism; University of Vienna: Vienna, Austria, 2018. [Google Scholar]
- Plato. The Republic; Cambridge Texts in the History of Political Thought; Ferrari, G.R.F., Ed.; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Tkadlec, J.; Czech Technical University in Prague. Personal communication, 23 August 2017.


© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Svozil, K. New Forms of Quantum Value Indefiniteness Suggest That Incompatible Views on Contexts Are Epistemic. Entropy 2018, 20, 406. https://doi.org/10.3390/e20060406
Svozil K. New Forms of Quantum Value Indefiniteness Suggest That Incompatible Views on Contexts Are Epistemic. Entropy. 2018; 20(6):406. https://doi.org/10.3390/e20060406
Chicago/Turabian StyleSvozil, Karl. 2018. "New Forms of Quantum Value Indefiniteness Suggest That Incompatible Views on Contexts Are Epistemic" Entropy 20, no. 6: 406. https://doi.org/10.3390/e20060406
APA StyleSvozil, K. (2018). New Forms of Quantum Value Indefiniteness Suggest That Incompatible Views on Contexts Are Epistemic. Entropy, 20(6), 406. https://doi.org/10.3390/e20060406




