Levitated Nanoparticles for Microscopic Thermodynamics—A Review
Abstract
1. Introduction
2. Forces and Potentials
2.1. Deterministic Forces
2.1.1. Optical Potential
2.1.2. Rotation
2.2. Stochastic Forces
2.2.1. Gas Damping
2.2.2. Radiation Damping
2.2.3. Artificial Damping and Heating
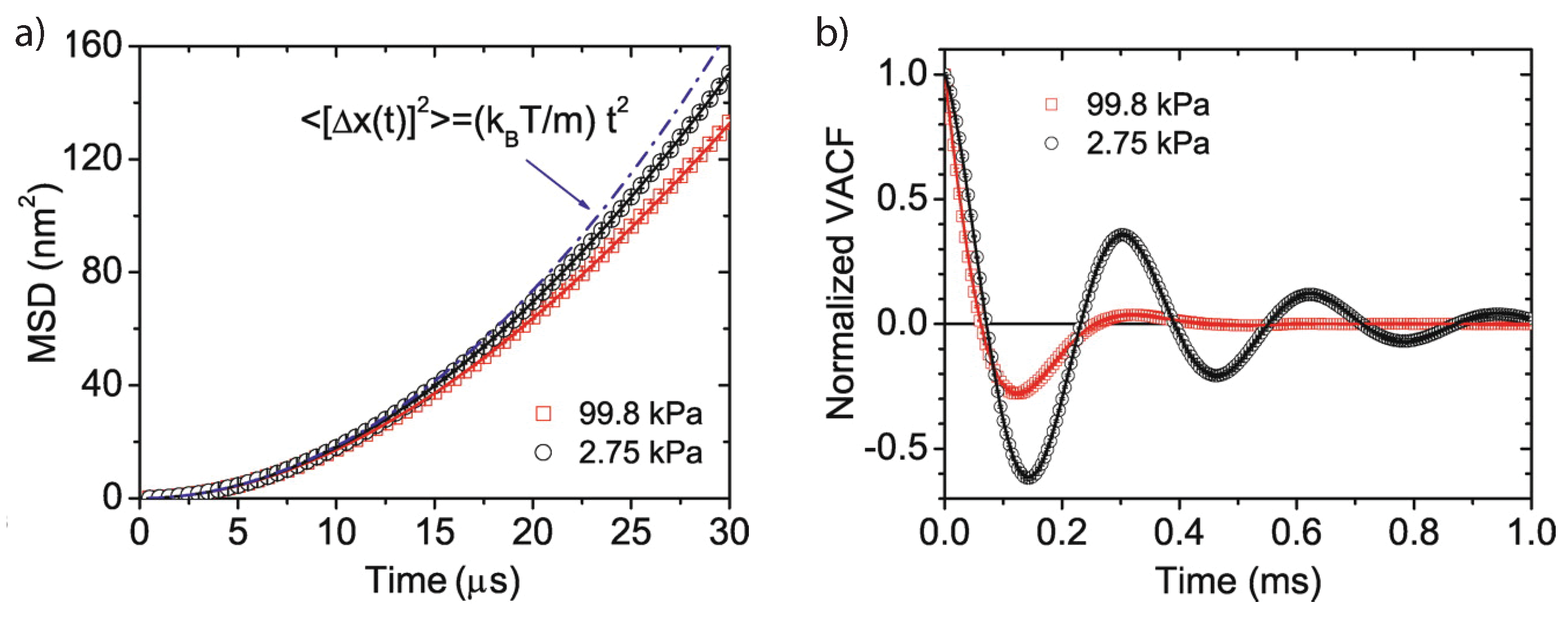
3. Brownian Motion
3.1. Harmonic Brownian Motion
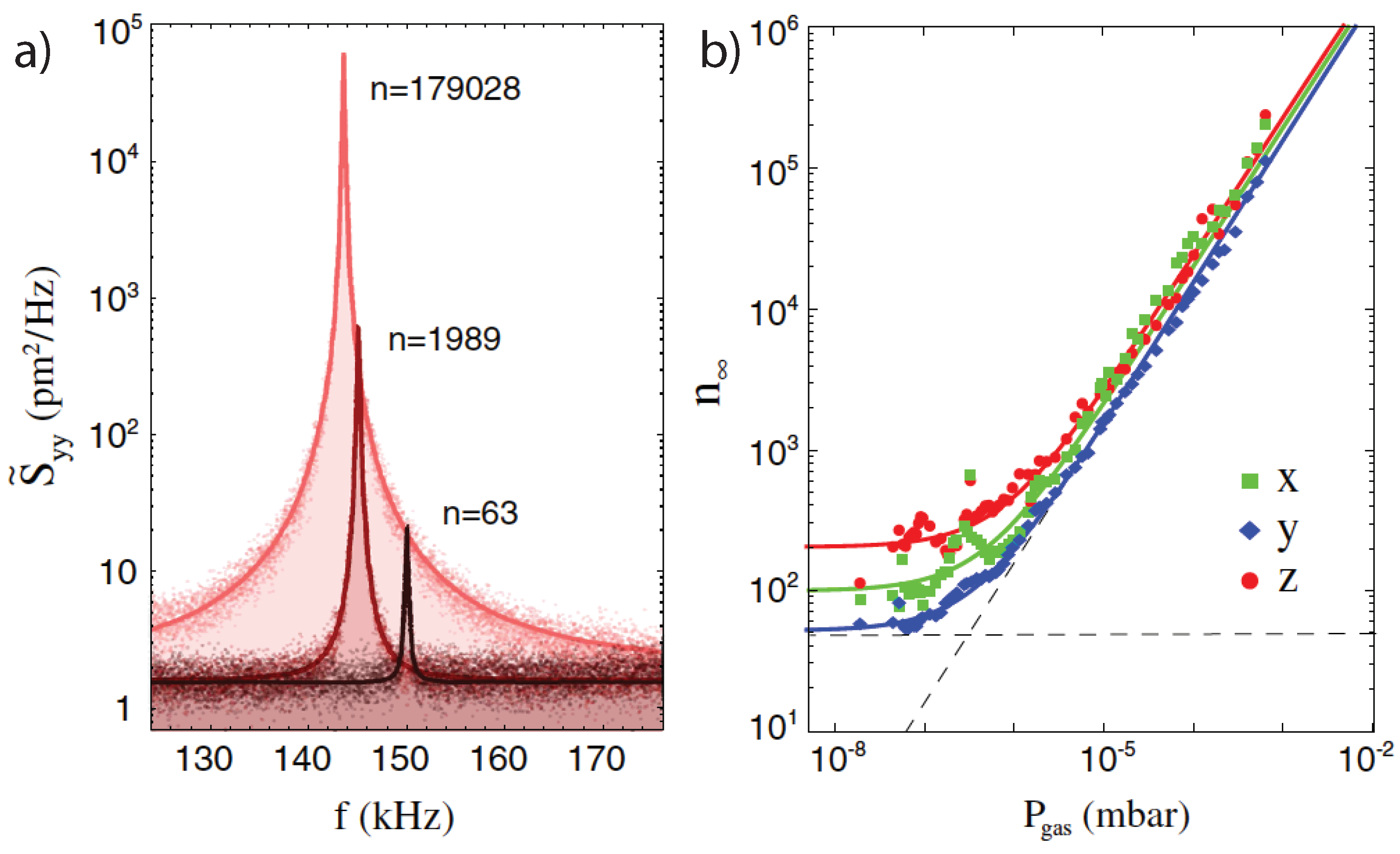
3.2. Power Spectral Density and Calibration
3.3. Quantum Brownian Motion
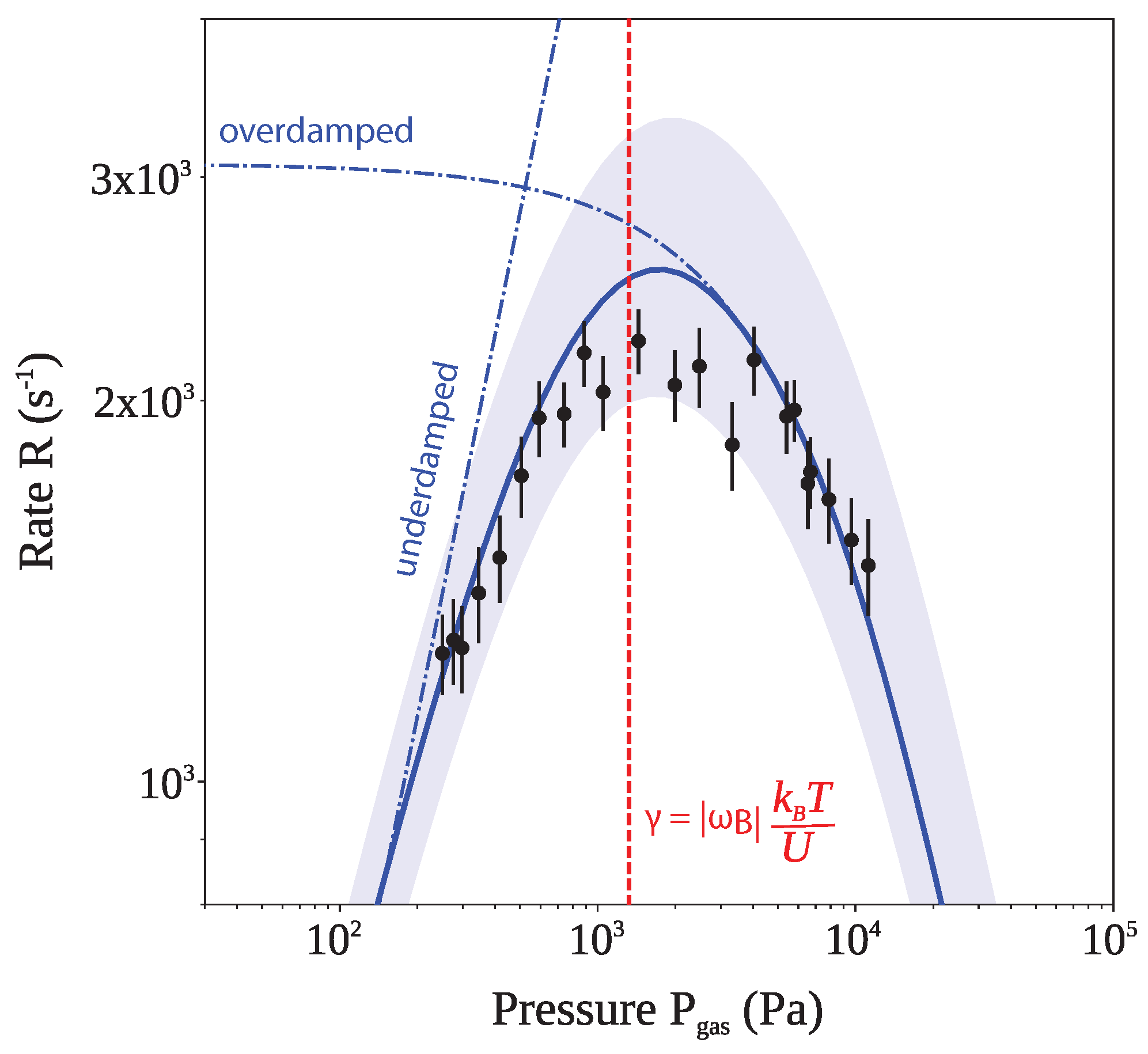
4. Trap Stability and Kramers Turnover
5. Effective Potentials in the Steady State
5.1. Effective Potential for the Energy
5.2. Effective Temperature
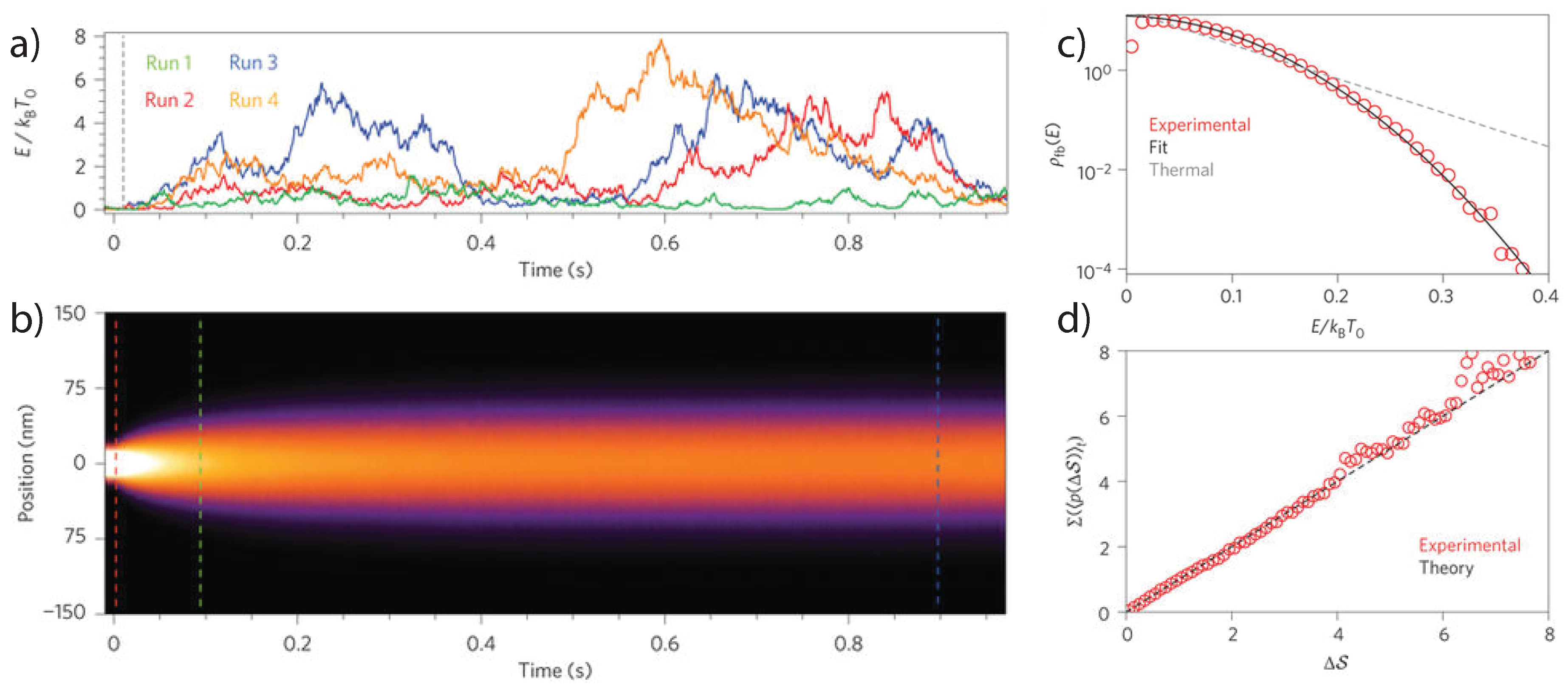
6. Relaxation
7. Fluctuation Theorems
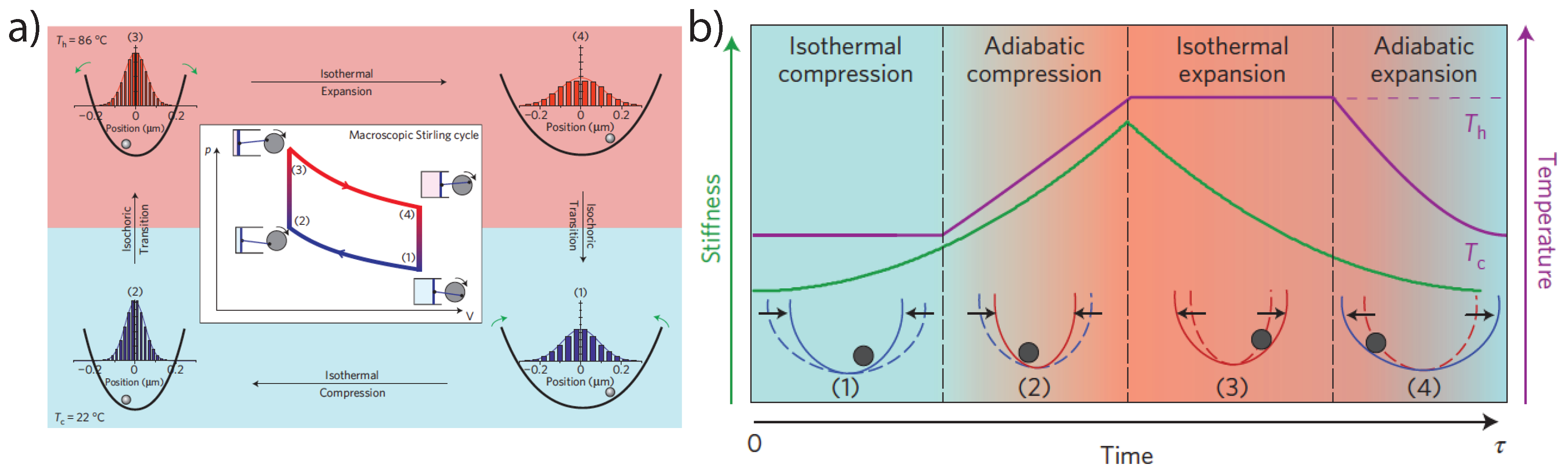
8. Heat Engines
9. Conclusions
Author Contributions
Conflicts of Interest
References
- Brown, R. A Brief Account of Microscopical Observations Made on the Particles Contained in the Pollen of Plants, and on the General Existence of Active Molecules in Organic and Inorganic Bodies. Philos. Mag. 1828, 4, 161–173. [Google Scholar] [CrossRef]
- Einstein, A. Über die von der molekularkinetischen Theorie der Wärme geforderte Bewegung von in ruhenden Flüssigkeiten suspendierten Teilchen. Ann. Phys. 1905, 17, 549–560. [Google Scholar] [CrossRef]
- Ashkin, A. Acceleration and trapping of particles by radiation pressure. Phys. Rev. Lett. 1970, 24, 156–159. [Google Scholar] [CrossRef]
- Ashkin, A. Optical Levitation by Radiation Pressure. Appl. Phys. Lett. 1971, 19, 283–285. [Google Scholar] [CrossRef]
- Ashkin, A.; Dziedzic, J.M. Optical levitation in high vacuum. Appl. Phys. Lett. 1976, 28, 333–335. [Google Scholar] [CrossRef]
- Ashkin, A.; Dziedzic, J.M.; Bjorkholm, J.E.; Chu, S. Observation of a single-beam gradient force optical trap for dielectric particles. Opt. Lett. 1986, 11, 288–290. [Google Scholar] [CrossRef] [PubMed]
- Jones, P.H.; Maragó, O.M.; Volpe, G. Optical Tweezers: Principles and Applications; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Spesyvtseva, S.E.S.; Dholakia, K. Trapping in a Material World. ACS Photon. 2016, 3, 719–736. [Google Scholar] [CrossRef]
- Li, T.; Kheifets, S.; Medellin, D.; Raizen, M.G. Measurement of the Instantaneous Velocity of a Brownian Particle. Science 2010, 328, 1673–1675. [Google Scholar] [CrossRef] [PubMed]
- Gieseler, J.; Deutsch, B.; Quidant, R.; Novotny, L. Subkelvin Parametric Feedback Cooling of a Laser-Trapped Nanoparticle. Phys. Rev. Lett. 2012, 109, 103603. [Google Scholar] [CrossRef] [PubMed]
- Kiesel, N.; Blaser, F.; Delic, U.; Grass, D.; Kaltenbaek, R.; Aspelmeyer, M. Cavity cooling of an optically levitated submicron particle. Proc. Nat. Acad. Sci. USA 2013, 110, 14180–14185. [Google Scholar] [CrossRef] [PubMed]
- Millen, J.; Fonseca, P.Z.G.; Mavrogordatos, T.; Monteiro, T.S.; Barker, P.F. Cavity Cooling a Single Charged Levitated Nanosphere. Phys. Rev. Lett. 2015, 114, 123602. [Google Scholar] [CrossRef] [PubMed]
- Romero-Isart, O.; Juan, M.; Quidant, R.; Cirac, J. Toward quantum superposition of living organisms. New J. Phys. 2010, 12, 033015. [Google Scholar] [CrossRef]
- Chang, D.E.; Regal, C.A.; Papp, S.B.; Wilson, D.J.; Ye, J.; Painter, O.; Kimble, H.J.; Zoller, P. Cavity opto-mechanics using an optically levitated nanosphere. Proc. Nat. Acad. Sci. USA 2010, 107, 1005–1010. [Google Scholar] [CrossRef] [PubMed]
- Barker, P.F. Doppler Cooling a Microsphere. Phys. Rev. Lett. 2010, 105, 073002. [Google Scholar] [CrossRef] [PubMed]
- Yin, Z.Q.; Geraci, A.A.; Li, T. Optomechanics of levitated dielectric particles. Int. J. Mod. Phys. B 2013, 27, 1330018–1330027. [Google Scholar] [CrossRef]
- Vamivakas, N.; Bhattacharya, M.; Barker, P. Levitated Optomechanics. Opt. Photon. News 2016, 27, 42–49. [Google Scholar] [CrossRef]
- Jain, V.; Gieseler, J.; Moritz, C.; Dellago, C.; Quidant, R.; Novotny, L. Direct Measurement of Photon Recoil from a Levitated Nanoparticle. Phys. Rev. Lett. 2016, 116, 243601. [Google Scholar] [CrossRef] [PubMed]
- Li, T.; Raizen, M.G. Brownian motion at short time scales. Ann. Phys. 2013, 525, 281–295. [Google Scholar] [CrossRef]
- Hebestreit, E.; Reimann, R.; Frimmer, M.; Novotny, L. Measuring the Internal Temperature of a Levitated Nanoparticle in High Vacuum. Phys. Rev. A 2018, 043803. [Google Scholar] [CrossRef]
- Lukas, N.; Hecht, B. Principles of Nano-Optics, 2nd ed.; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Albaladejo, S.; Marqués, M.; Laroche, M.; Sáenz, J. Scattering Forces from the Curl of the Spin Angular Momentum of a Light Field. Phys. Rev. Lett. 2009, 102, 113602. [Google Scholar] [CrossRef] [PubMed]
- Gieseler, J.; Spasenović, M.; Novotny, L.; Quidant, R. Nonlinear Mode Coupling and Synchronization of a Vacuum-Trapped Nanoparticle. Phys. Rev. Lett. 2014, 112, 103603. [Google Scholar] [CrossRef] [PubMed]
- Millen, J.; Deesuwan, T.; Barker, P.; Anders, J. Nanoscale temperature measurements using non-equilibrium Brownian dynamics of a levitated nanosphere. Nat. Nanotech. 2014, 9, 425–429. [Google Scholar] [CrossRef] [PubMed]
- Gieseler, J.; Novotny, L.; Quidant, R. Thermal nonlinearities in a nanomechanical oscillator. Nat. Phys. 2013, 9, 806–810. [Google Scholar] [CrossRef]
- Stickler, B.A.; Nimmrichter, S.; Martinetz, L.; Kuhn, S.; Arndt, M.; Hornberger, K. Rotranslational cavity cooling of dielectric rods and disks. Phys. Rev. A 2016, 94, 033818. [Google Scholar] [CrossRef]
- Kuhn, S.; Kosloff, A.; Stickler, B.A.; Patolsky, F.; Hornberger, K.; Arndt, M.; Millen, J. Full rotational control of levitated silicon nanorods. Optica 2017, 4, 356–360. [Google Scholar] [CrossRef]
- Geiselmann, M.; Juan, M.L.; Renger, J.; Say, J.M.; Brown, L.J.; de Abajo, F.J.G.; Koppens, F.; Quidant, R. Three-dimensional optical manipulation of a singleelectron spin. Nat. Nanotech. 2013, 8, 175–179. [Google Scholar] [CrossRef] [PubMed]
- Arita, Y.; Mazilu, M.; Dholakia, K. Laser-induced rotation and cooling of a trapped microgyroscope in vacuum. Nat. Commun. 2013, 4, 2374. [Google Scholar] [CrossRef] [PubMed]
- Bishop, A.I.; Nieminen, T.A.; Heckenberg, N.R.; Rubinsztein-Dunlop, H. Optical Microrheology Using Rotating Laser-Trapped Particles. Phys. Rev. Lett. 2004, 92, 198104. [Google Scholar] [CrossRef] [PubMed]
- Paterson, L.; MacDonald, M.P.; Arlt, J.; Sibbett, W.; Bryant, P.E.; Dholakia, K. Controlled Rotation of Optically Trapped Microscopic Particles. Science 2001, 292, 912–914. [Google Scholar] [CrossRef] [PubMed]
- Kuhn, S.; Asenbaum, P.; Kosloff, A.; Sclafani, M.; Stickler, B.A.; Nimmrichter, S.; Hornberger, K.; Cheshnovsky, O.; Patolsky, F.; Arndt, M. Cavity-Assisted Manipulation of Freely Rotating Silicon Nanorods in High Vacuum. Nano Lett. 2015, 15, 5604–5608. [Google Scholar] [CrossRef] [PubMed]
- Roulet, A.; Nimmrichter, S.; Arrazola, J.M.; Seah, S.; Scarani, V. Autonomous rotor heat engine. Phys. Rev. E 2017, 95, 062131. [Google Scholar] [CrossRef] [PubMed]
- Baffou, G.; Quidant, R. Thermo-plasmonics: using metallic nanostructures as nano-sources of heat. Laser Photon. Rev. 2012, 7, 171–187. [Google Scholar] [CrossRef]
- Baffou, G.; Quidant, R.; García de Abajo, F.J. Nanoscale Control of Optical Heating in Complex Plasmonic Systems. ACS Nano 2010, 4, 709–716. [Google Scholar] [CrossRef] [PubMed]
- Jauffred, L.; Taheri, S.M.R.; Schmitt, R.; Linke, H.; Oddershede, L.B. Optical Trapping of Gold Nanoparticles in Air. Nano Lett. 2015, 15, 4713–4719. [Google Scholar] [CrossRef] [PubMed]
- Rings, D.; Schachoff, R.; Selmke, M.; Cichos, F.; Kroy, K. Hot Brownian Motion. Phys. Rev. Lett. 2010, 105, 090604. [Google Scholar] [CrossRef] [PubMed]
- Rings, D.; Chakraborty, D.; Kroy, K. Rotational hot Brownian motion. New J. Phys. 2012, 14, 053012. [Google Scholar] [CrossRef][Green Version]
- Falasco, G.; Gnann, M.V.; Rings, D.; Kroy, K. Effective temperatures of hot Brownian motion. Phys. Rev. E 2014, 90, 032131. [Google Scholar] [CrossRef] [PubMed]
- Martinetz, L.; Hornberger, K.; Stickler, B.A. Gas-induced friction and diffusion of rigid rotors. Phys. Rev. E 2018. Accepted. [Google Scholar]
- Novotny, L. Radiation damping of a polarizable particle. Phys. Rev. A 2017, 96, 032108. [Google Scholar] [CrossRef]
- Li, T.; Kheifets, S.; Raizen, M. Millikelvin cooling of an optically trapped microsphere in vacuum. Nat. Phys. 2011, 7, 527–530. [Google Scholar] [CrossRef]
- Frimmer, M.; Luszcz, K.; Ferreiro, S.; Jain, V.; Hebestreit, E.; Novotny, L. Controlling the net charge on a nanoparticle optically levitated in vacuum. Phys. Rev. A 2017, 95, 061801. [Google Scholar] [CrossRef]
- Mestres, P.; Martinez, I.A.; Ortiz-Ambriz, A.; Rica, R.A.; Roldan, E. Realization of nonequilibrium thermodynamic processes using external colored noise. Phys. Rev. E 2014, 90, 032116. [Google Scholar] [CrossRef] [PubMed]
- Martínez, I.A.; Roldán, É.; Parrondo, J.M.R.; Petrov, D. Effective heating to several thousand kelvins of an optically trapped sphere in a liquid. Phys. Rev. E 2013, 87, 1246–1258. [Google Scholar] [CrossRef]
- Casas-Vázquez, J.; Jou, D. Temperature in non-equilibrium states: a review of open problems and current proposals. Rep. Prog. Phys. 2003, 66, 1937. [Google Scholar] [CrossRef]
- Gieseler, J.; Quidant, R.; Dellago, C.; Novotny, L. Dynamic relaxation of a levitated nanoparticle from a non-equilibrium steady state. Nat. Nanotech. 2014, 9, 358–364. [Google Scholar] [CrossRef] [PubMed]
- Gnesotto, F.; Mura, F.; Gladrow, J.; Broedersz, C.P. Broken detailed balance and non-equilibrium dynamics in living systems. arXiv, 2017; arXiv:1710.03456v1. [Google Scholar]
- Bechinger, C.; Di Leonardo, R.; Löwen, H.; Reichhardt, C.; Volpe, G.; Volpe, G. Active Particles in Complex and Crowded Environments. Rev. Mod. Phys. 2016, 88, 045006. [Google Scholar] [CrossRef]
- Nicolis, G.; De Decker, Y. Stochastic Thermodynamics of Brownian Motion. Entropy 2017, 19, 434. [Google Scholar] [CrossRef]
- Uhlenbeck, G.E.; Ornstein, L.S. On the Theory of the Brownian Motion. Phys. Rev. 1930, 36, 823–841. [Google Scholar] [CrossRef]
- Wang, M.C.; Uhlenbeck, G.E. On the Theory of the Brownian Motion II. Rev. Mod. Phys. 1945, 17, 323–342. [Google Scholar] [CrossRef]
- Kheifets, S.; Simha, A.; Melin, K.; Li, T.; Raizen, M.G. Observation of Brownian Motion in Liquids at Short Times: Instantaneous Velocity and Memory Loss. Science 2014, 343, 1493–1496. [Google Scholar] [CrossRef] [PubMed]
- Hebestreit, E.; Frimmer, M.; Reimann, R.; Dellago, C.; Ricci, F.; Novotny, L. Calibration and temperature measurement of levitated optomechanical sensors. Rev. Sci. Instrum. 2018, 89, 033111. [Google Scholar] [CrossRef] [PubMed]
- Clerk, A.A.; Girvin, S.M.; Marquardt, F.; Schoelkopf, R.J. Introduction to quantum noise, measurement, and amplification. Rev. Mod. Phys. 2010, 82, 1155–1208. [Google Scholar] [CrossRef]
- Safavi-Naeini, A.H.; Chan, J.; Hill, J.T.; Alegre, T.P.M.; Krause, A.; Painter, O. Observation of quantum motion of a nanomechanical resonator. Phys. Rev. Lett. 2012, 108, 33602. [Google Scholar] [CrossRef] [PubMed]
- Weinstein, A.J.; Lei, C.U.; Wollman, E.E.; Suh, J.; Metelmann, A.; Clerk, A.A.; Schwab, K.C. Observation and Interpretation of Motional Sideband Asymmetry in a Quantum Electromechanical Device. Phys. Rev. X 2014, 4, 041003. [Google Scholar] [CrossRef]
- Underwood, M.; Mason, D.; Lee, D.; Xu, H.; Jiang, L.; Shkarin, A.B.; Børkje, K.; Girvin, S.M.; Harris, J.G.E. Measurement of the motional sidebands of a nanogram-scale oscillator in the quantum regime. Phys. Rev. A 2015, 92, 061801. [Google Scholar] [CrossRef]
- Peterson, R.W.; Purdy, T.P.; Kampel, N.S.; Andrews, R.W.; Yu, P.L.; Lehnert, K.W.; Regal, C.A. Laser Cooling of a Micromechanical Membrane to the Quantum Backaction Limit. Phys. Rev. Lett. 2016, 116, 653–656. [Google Scholar] [CrossRef] [PubMed]
- Kampel, N.S.; Peterson, R.W.; Fischer, R.; Yu, P.L.; Cicak, K.; Simmonds, R.W.; Lehnert, K.W.; Regal, C.A. Improving Broadband Displacement Detection with Quantum Correlations. Phys. Rev. X 2017, 7, 021008. [Google Scholar] [CrossRef]
- Divitt, S.; Rondin, L.; Novotny, L. Cancellation of non-conservative scattering forces in optical traps by counter-propagating beams. Opt. Lett. 2015, 40, 1900–1904. [Google Scholar] [CrossRef] [PubMed]
- Ranjit, G.; Atherton, D.P.; Stutz, J.H.; Cunningham, M.; Geraci, A.A. Attonewton force detection using microspheres in a dual-beam optical trap in high vacuum. Phys. Rev. A 2015, 91, 051805. [Google Scholar] [CrossRef]
- Kramers, H. Brownian motion in a field of force and the diffusion model of chemical reactions. Physica 1940, 7, 284–304. [Google Scholar] [CrossRef]
- Mel’nikov, V. The Kramers problem: Fifty years of development. Phys. Rep. 1991, 209, 1–71. [Google Scholar] [CrossRef]
- Hänggi, P.; Talkner, P.; Borkovec, M. Reaction-rate theory: Fifty years after Kramers. Rev. Mod. Phys. 1990, 62, 251–341. [Google Scholar] [CrossRef]
- Rondin, L.; Gieseler, J.; Ricci, F.; Quidant, R.; Dellago, C.; Novotny, L. Direct measurement of Kramers turnover with a levitated nanoparticle. Nat. Nanotech. 2017, 12, 1130–1133. [Google Scholar] [CrossRef] [PubMed]
- Eichler, A.; Moser, J.; Chaste, J.; Zdrojek, M.; Wilson-Rae, I.; Bachtold, A. Nonlinear damping in mechanical resonators made from carbon nanotubes and graphene. Nat. Nanotech. 2011, 6, 339–342. [Google Scholar] [CrossRef] [PubMed]
- Gieseler, J.; Novotny, L.; Moritz, C.; Dellago, C. Non-equilibrium steady state of a driven levitated particle with feedback cooling. New J. Phys. 2015, 17, 045011. [Google Scholar] [CrossRef]
- Ricci, F.; Rica, R.A.; Spasenovic, M.; Gieseler, J.; Rondin, L.; Novotny, L.; Quidant, R. Optically levitated nanoparticle as a model system for stochastic bistable dynamics. Nat. Commun. 2017, 8, 15141. [Google Scholar] [CrossRef] [PubMed]
- Salazar, D.S.P.; Lira, S.A. Exactly solvable nonequilibrium Langevin relaxation of a trapped nanoparticle. J. Phys. A Math. Theor. 2016, 49, 465001–465018. [Google Scholar] [CrossRef][Green Version]
- Martínez, I.A.; Petrosyan, A.; Guéry-Odelin, D.; Trizac, E.; Ciliberto, S. Engineered swift equilibration of a Brownian? particle. Nat. Phys. 2016, 12, 843–846. [Google Scholar] [CrossRef] [PubMed]
- Chupeau, M.; Ciliberto, S.; Guéry-Odelin, D.; Trizac, E. Engineered Swift Equilibration for Brownian objects: From underdamped to over damped dynamics. arXiv, 2018; arXiv:1802.10512. [Google Scholar]
- Crooks, G.E. Entropy production fluctuation theorem and the nonequilibrium work relation for free energy differences. The Gallavotti-Cohen Fluctuation Theorem and the NonequilibriumWork Relation for Free Energy Differences. Phys. Rev. E 1999, 60, 2721–2726. [Google Scholar] [CrossRef]
- Collin, D.; Ritort, F.; Jarzynski, C.; Smith, S.B.; Tinoco, I.; Bustamante, C. Verification of the Crooks fluctuation theorem and recovery of RNA folding free energies. Nature 2005, 437, 231–234. [Google Scholar] [CrossRef] [PubMed]
- Seifert, U. Stochastic thermodynamics, fluctuation theorems and molecular machines. Rep. Prog. Phys. 2012, 75, 126001. [Google Scholar] [CrossRef] [PubMed]
- Gieseler, J. Dynamics of Optically Levitated Nanoparticles in High Vacuum. PhD Thesis, The Institute of Photonic Sciences, Barcelona, Spain, 2014. [Google Scholar]
- Wang, G.M.; Sevick, E.M.; Mittag, E.; Searles, D.J.; Evans, D.J. Experimental demonstration of violations of the second law of thermodynamics for small systems and short time scales. Phys. Rev. Lett. 2002, 89, 50601. [Google Scholar] [CrossRef] [PubMed]
- Seifert, U. Entropy Production along a Stochastic Trajectory and an Integral Fluctuation Theorem. Phys. Rev. Lett. 2005, 95, 040602. [Google Scholar] [CrossRef] [PubMed]
- Evans, D.J.; Searles, D.J. The Fluctuation Theorem. Adv. Phys. 2002, 51, 1529–1585. [Google Scholar] [CrossRef]
- Hoang, T.M.; Pan, R.; Ahn, J.; Bang, J.; Quan, H.T.; Li, T. Experimental Test of the Differential Fluctuation Theorem and a Generalized Jarzynski Equality for Arbitrary Initial States. Phys. Rev. Lett. 2018, 120, 080602. [Google Scholar] [CrossRef] [PubMed]
- Jarzynski, C. Nonequilibrium Equality for Free Energy Differences. Phys. Rev. Lett. 1997, 78, 2690–2693. [Google Scholar] [CrossRef]
- Liphardt, J.; Dumont, S.; Smith, S.B.; Tinoco, I.; Bustamante, C. Equilibrium information from nonequilibrium measurements in an experimental test of Jarzynski’s equality. Science 2002, 296, 1832–1835. [Google Scholar] [CrossRef] [PubMed]
- Hummer, G.; Szabo, A. Free energy reconstruction from nonequilibrium single-molecule pulling experiments. Proc. Nat. Acad. Sci. USA 2001, 98, 3658–3661. [Google Scholar] [CrossRef] [PubMed]
- Gupta, A.N.; Vincent, A.; Neupane, K.; Yu, H.; Wang, F.; Woodside, M.T. Experimental validation of free-energy-landscape reconstruction from non-equilibrium single- molecule force spectroscopy measurements. Nat. Phys. 2011, 7, 631–634. [Google Scholar] [CrossRef]
- Martinez, I.A.; Roldan, E.; Dinis, L.; Rica, R.A. Colloidal heat engines: a review. Soft Matter 2017, 13, 22–36. [Google Scholar] [CrossRef] [PubMed]
- Ciliberto, S. Experiments in Stochastic Thermodynamics: Short History and Perspectives. Phys. Rev. X 2017, 7, 021051. [Google Scholar] [CrossRef]
- Ritort, F. Single-molecule experiments in biological physics: Methods and applications. J. Phys. Condens. Matter 2006, 18, R531–R583. [Google Scholar] [CrossRef] [PubMed]
- Sekimoto, K. Stochastic Energetics; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Spinney, R.E.; Ford, I.J. Fluctuation relations: a pedagogical overview. ArXiv, 2012. [Google Scholar]
- Schmiedl, T.; Seifert, U. Efficiency at maximum power: An analytically solvable model for stochastic heat engines. Europhys. Lett. 2008, 81, 20003. [Google Scholar] [CrossRef]
- Blickle, V.; Bechinger, C. Realization of a micrometre-sized stochastic heat engine. Nat. Phys. 2011, 8, 143–146. [Google Scholar] [CrossRef]
- Carnot, S. Réflexions sur la Puissance Motrice du Feu; Elsevier: Amsterdam, The Netherland, 1824; Available online: http://www.numdam.org/article/ASENS_1872_2_1__393_0.pdf (accessed on 27 April 2018).
- Shiraishi, N.; Saito, K.; Tasaki, H. Universal Trade-Off Relation between Power and Efficiency for Heat Engines. Phys. Rev. Lett. 2016, 117, 190601. [Google Scholar] [CrossRef] [PubMed]
- Andresen, B.; Salamon, P.; Berry, R.S. Thermodynamics in finite time. Phys. Today 1984, 37, 62–70. [Google Scholar] [CrossRef]
- Novikov, I. The efficiency of atomic power stations. J. Nucl. Energy 1958, 125–128. [Google Scholar]
- Curzon, F.; Ahlborn, B. Efficiency of a Carnot engine at maximum power output. Am. J. Phys. 1975, 43, 22–24. [Google Scholar]
- Berry, M.V. Transitionless quantum driving. J. Phys. A Math. Theor. 2009, 42, 365303–365310. [Google Scholar] [CrossRef]
- Demirplak, M.; Rice, S.A. Adiabatic Population Transfer with Control Fields. J. Phys. Chem. A 2003, 107, 9937–9945. [Google Scholar] [CrossRef]
- Deffner, S.; Jarzynski, C.; del Campo, A. Classical and Quantum Shortcuts to Adiabaticity for Scale-Invariant Driving. Phys. Rev. X 2014, 4, 021013. [Google Scholar] [CrossRef]
- Jarzynski, C. Generating shortcuts to adiabaticity in quantum and classical dynamics. Phys. Rev. A 2013, 88, 24413–24421. [Google Scholar] [CrossRef]
- Del Campo, A. Shortcuts to Adiabaticity by Counterdiabatic Driving. Phys. Rev. Lett. 2013, 111, 100502–100505. [Google Scholar] [CrossRef] [PubMed]
- Tu, Z.C. Stochastic heat engine with the consideration of inertial effects and shortcuts to adiabaticity. Phys. Rev. E 2014, 89, 052148. [Google Scholar] [CrossRef] [PubMed]
- Brandner, K.; Saito, K.; Seifert, U. Thermodynamics of Micro- and Nano-Systems Driven by Periodic Temperature Variations. Phys. Rev. X 2015, 5, 031019. [Google Scholar] [CrossRef]
- Rosinberg, M.L.; Munakata, T.; Tarjus, G. Stochastic thermodynamics of Langevin systems under time-delayed feedback control: Second-law-like inequalities. Phys. Rev. E 2015, 91, 29–31. [Google Scholar] [CrossRef] [PubMed]
- Shiraishi, N.; Tajima, H. Efficiency versus speed in quantum heat engines: Rigorous constraint from Lieb-Robinson bound. Phys. Rev. E 2017, 96, 022138. [Google Scholar] [CrossRef] [PubMed]
- Martínez, I.A.; Roldán, E.; Dinis, L.; Petrov, D.; Rica, R.A. Adiabatic Processes Realized with a Trapped Brownian Particle. Phys. Rev. Lett. 2015, 114, 120601. [Google Scholar] [CrossRef] [PubMed]
- Martínez, I.A.; Roldán, É.; Dinis, L.; Petrov, D.; Parrondo, J.M.R.; Rica, R.A. Brownian Carnot engine. Nat. Phys. 2015, 12, 67–70. [Google Scholar] [CrossRef] [PubMed]
- Dinis, L.; Martínez, I.A.; Roldán, É.; Parrondo, J.M.R.; Rica, R.A. Thermodynamics at the microscale: From effective heating to the Brownian Carnot engine. J. Stat. Mech. Theory Exp. 2016, 5, 054003. [Google Scholar] [CrossRef]
- Huang, R.; Chavez, I.; Taute, K.M.; Lukić, B.; Jeney, S.; Raizen, M.; Florin, E.L. Direct observation of the full transition from ballistic to diffusive Brownian motion in a liquid. Nat. Phys. 2011, 7, 576–580. [Google Scholar] [CrossRef]
- Roßnagel, J.; Abah, O.; Schmidt-Kaler, F.; Singer, K.; Lutz, E. Nanoscale Heat Engine Beyond the Carnot Limit. Phys. Rev. Lett. 2014, 112, 030602. [Google Scholar] [CrossRef] [PubMed]
- Rashid, M.; Tufarelli, T.; Bateman, J.; Vovrosh, J.; Hempston, D.; Kim, M.S.; Ulbricht, H. Experimental Realization of a Thermal Squeezed State of Levitated Optomechanics. Phys. Rev. Lett. 2016, 117, 273601. [Google Scholar] [CrossRef] [PubMed]
- Dechant, A.; Kiesel, N.; Lutz, E. All-Optical Nanomechanical Heat Engine. Phys. Rev. Lett. 2015, 114, 183602. [Google Scholar] [CrossRef] [PubMed]
- Gomez-Marin, A.; Schmiedl, T.; Seifert, U. Optimal protocols for minimal work processes in underdamped stochastic thermodynamics. J. Chem. Phys. 2008, 129, 024114. [Google Scholar] [CrossRef] [PubMed]
- Dechant, A.; Kiesel, N.; Lutz, E. Underdamped stochastic heat engine at maximum efficiency. EPL (Europhys. Lett.) 2017, 119, 50003. [Google Scholar] [CrossRef]
- Browne, W.R.; Feringa, B.L. Making molecular machines work. Nat. Nanotech. 2006, 1, 25–35. [Google Scholar] [CrossRef] [PubMed]
- Erbas-Cakmak, S.; Leigh, D.A.; McTernan, C.T.; Nussbaumer, A.L. Artificial Molecular Machines. Chem. Rev. 2015, 115, 10081–10206. [Google Scholar] [CrossRef] [PubMed]
- Heisenberg, W. Über den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. Z. Phys. 1927, 43, 172–198. [Google Scholar] [CrossRef]
- Landauer, R. Irreversibility and heat generation in the computing process. IBM J. Res. Dev. 1961, 5, 183–191. [Google Scholar] [CrossRef]
- Lutz, E.; Ciliberto, S. Information: From Maxwell’s demon to Landauer’s eraser. Phys. Today 2015, 68, 30–35. [Google Scholar] [CrossRef]
- Bérut, A.; Arakelyan, A.; Petrosyan, A.; Ciliberto, S.; Dillenschneider, R.; Lutz, E. Experimental verification of Landauer’s principle linking information and thermodynamics. Nature 2012, 483, 187–189. [Google Scholar] [CrossRef] [PubMed]
- Braginsky, V.B.; Khalili, F.Y. Quantum Measurement; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- Purdy, T.P.; Peterson, R.W.; Regal, C.A. Observation of Radiation Pressure ShotNoise on aMacroscopic Object. Science 2013, 339, 801–804. [Google Scholar] [CrossRef] [PubMed]
- Talkner, P.; Hänggi, P. Aspects of quantum work. Phys. Rev. E 2016, 93, 022131. [Google Scholar] [CrossRef] [PubMed]

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gieseler, J.; Millen, J. Levitated Nanoparticles for Microscopic Thermodynamics—A Review. Entropy 2018, 20, 326. https://doi.org/10.3390/e20050326
Gieseler J, Millen J. Levitated Nanoparticles for Microscopic Thermodynamics—A Review. Entropy. 2018; 20(5):326. https://doi.org/10.3390/e20050326
Chicago/Turabian StyleGieseler, Jan, and James Millen. 2018. "Levitated Nanoparticles for Microscopic Thermodynamics—A Review" Entropy 20, no. 5: 326. https://doi.org/10.3390/e20050326
APA StyleGieseler, J., & Millen, J. (2018). Levitated Nanoparticles for Microscopic Thermodynamics—A Review. Entropy, 20(5), 326. https://doi.org/10.3390/e20050326





