1. Introduction
In the statistical formulation of quantum mechanics, a wavefunction
has to be square integrable (SI) to ensure the qualification of
as a probability density function. SI solutions to the Schrödinger equation can be used to determine the energy levels in a confined system. Nonsquare-integrable (NSI) solutions to the Schrödinger equation otherwise are ruled out and their role has been unknown till now. To investigate the role of NSI wavefunctions, we need a formulation of quantum mechanics, which does not require the SI condition. Among the nine different formulations of quantum mechanics [
1], there is a formulation known as the quantum Hamilton-Jacobi (H-J) formalism [
2,
3], which meets our purpose. The quantum H-J formalism has been developed since the inception of quantum mechanics along the line of Jordan [
4], Dirac [
5] and Schwinger [
6]. The main advantage of the classical H-J formalism is to give the frequencies of a periodic motion directly without solving the equations of motion. Analogous to its classical counterpart, the advantage of quantum H-J formalism is recognized as a method of finding energy eigenvalues directly without solving the related Schrödinger equation.
Based on the quantum H-J equation, Leacock and Padgett [
2,
3] proposed an ingenious method to evaluate energy eigenvalues by contour integral. This approach to energy eigenvalues
is entirely independent of whether the related wavefunction is SI or not, and allows us to examine the participation of the NSI wavefunctions in the process of energy quantization. Apart from providing energy eigenvalues, quantum H-J formalism like its classical counterpart produces quantum Hamilton dynamics [
7], from which complex quantum trajectories can be solved to describe the quantum motion associated with a given wavefunction. Probability interpretation isolates SI wavefunctions from NSI wavefunctions; on the contrary, under the quantum H-J formalism SI and NSI wavefunctions are indivisible with continuously connected quantum trajectories. Because NSI wavefunctions
fail to serve as probability density functions, we need an alternative operation to replace the expectation (assemble average)
of a quantum observable
. The complex quantum trajectory method developed from the quantum H-J formalism can provide the time average
to substitute for the usual assemble average
.
Based on the time-average operation , which applies to both SI and NSI wavefunctions, we can derive quantization laws more general than those based on the assemble average , which applies only to SI wavefunctions. One of the general results shows that as the total energy of a confined system increases monotonically, the time-average kinetic energy of a confined particle exhibits a stair-like distribution in such a way that the step jumps occur as equal to one of the energy eigenvalue and the flat part of the distribution is formed over the interval . During the process as increases continuously from to the next energy eigenvalue , we note that all the corresponding wavefunctions are NSI, but they all yield the same value of and form the flat part of the stair-like energy distribution. In other words, the transition from the eigenstate to the next eigenstate can be connected smoothly by the NSI wavefunctions with , which otherwise have been ruled out in standard quantum mechanics.
Compared to the complex quantum trajectory derived from the quantum H-J formalism, de Broglie-Bohm (dBB) quantum trajectory [
8,
9,
10] is real-valued. The equivalence between dBB trajectory interpretation and probability interpretation of quantum mechanics has been well developed over the last several decades. Although under the dBB formulation, particles follow continuous trajectories with well-defined two-time position correlations, a recent paper by Gisin [
11] pointed out that Bohmian mechanics makes the same predictions as standard quantum mechanics: the violation of Bell inequalities. The studies of dBB formulation of quantum mechanics [
12,
13,
14] revealed that like the way that thermal probabilities arise in ordinary statistical mechanics, the quantum probabilities
arise dynamically in a similar way that a simple initial ensemble with a non-equilibrium distribution
of particle positions evolves towards the equilibrium distribution via the relaxation process
. Meanwhile, the speed of the convergence of
to
was found to correlate with the degree of chaos of the involved Bohmian trajectories [
15,
16,
17], which in turn was shown to be related to the vortex dynamics generated by nodal points in the wavefunction
[
18,
19]. The degree of chaos produced by many interacting vortices ultimately depends on the number and spatial distribution of the nodal points in the configuration space [
20,
21].
Parallel to the development of real-valued dBB trajectories, the study of complex-valued quantum trajectories has evolved into a quantum trajectory method, which integrates the hydrodynamic equations
on the fly to synthesize the probability density by evolving ensembles of complex quantum trajectories [
22,
23,
24]. Due to the additional degree of freedom given by the imaginary part of a complex trajectory, it is possible to synthesize the quantum probability
by a single complex-valued trajectory, instead of an ensemble of real-valued or complex-valued trajectories [
25].
To date, the trajectory approaches to quantum mechanics, either using real-valued trajectories based on dBB formulation or using complex-valued trajectories based on H-J formulation, mainly deal with SI wavefunctions in order to show their consistency with the probability interpretation. Here we will go beyond SI wavefunctions to find out what will happen, when statistical interpretation is not applicable. On one hand we will use complex trajectories to demonstrate the energy quantization process after releasing the SI condition, and on the other we will apply the quantum Hamilton dynamics to demonstrate the sequential center-saddle bifurcations as the energy quantization proceeds. The combined result manifests a new quantum phenomenon regarding the synchronicity between the energy quantization process and the center-saddle bifurcation process.
NSI wavefunctions not only participate in the quantization and bifurcation process, but also in the formation of spin degree of freedom. In spite of their distinct statistical properties, SI and NSI wavefunctions have similar velocity fields with the only difference in their directions of rotation on the complex plane. As the third goal of the paper, we will contrast quantum trajectories of SI wavefunctions with those of NSI wavefunctions to manifest the invisible spin degree of freedom as a rotational motion on the complex plane.
The remainder of this paper is organized as follows:
Section 2 presents quantum H-J formalism and the related method of determining energy eigenvalues. In
Section 3, time average operation for NSI wavefunctions along a complex quantum trajectory is developed from the quantum H-J formalism to replace the ensemble average. The proposed time average operation is then used in
Section 4 to derive the universal quantization laws regarding the kinetic energy and the quantum potential.
Section 5 demonstrates the participation of NSI wavefunctions in the energy quantization process for a harmonic oscillator.
Section 6 proposes a quantum dynamic description of energy quantization, in terms of which a new phenomenon regarding the synchronicity between quantization and bifurcation is revealed. Finally, both SI and NSI solutions to the Schrödinger equation are considered in
Section 7 and their relations to spin degree of freedom are explained.
2. Quantum Hamilton-Jacobi Formalism
While the quantum H-J theory is general, here we consider its application to bound states, which have quantized energy levels and closed quantum trajectories. The quantum H-J approach to determining energy eigenvalues can be conceived of as an extension of the Wilson-Sommerfeld quantization rule [
26]. In this approach, the quantum energy levels are given exactly by setting the quantum action variable equal to an integer multiple of Planck constant:
where
is called quantum momentum function (QMF) and the contour
is defined on the complex plane with the integer
being the number of poles of
enclosed by
. The QMF
is related to the quantum action function
and the wavefunction
as:
with
satisfying the quantum H-J equation:
and with
satisfying the Schrödinger equation:
It appears that the quantum H-J Equation (3) and the Schrödinger Equation (4) are equivalent expressions via the relation .
Leacock and Padgett [
2,
3] proposed an ingenious method to evaluate
without actually solving
from the quantum H-J Equation (3). They showed that for a given potential
,
can be computed simply by a suitable deformation of the complex contour
and the change of variables in Equation (1). Once
is found, the energy eigenvalues
can be determined by solving
in terms of the integer
via the relation
.
The quantum H-J approach to determining energy eigenvalues has two significant implications. Firstly, this approach suggests that the energy eigenvalue stems from the quantization of the action variable , rather than from the quantization of the total energy itself. Precisely speaking, the energy eigenvalue is the specific energy at which the action variable happens to be an integer multiple of , i.e., . Inspired by this implication, the first goal of this paper is to reveal the internal mechanism causing the quantization of the action variable and find out its relation to the energy quantization.
Secondly, the quantum H-J approach implies that the SI condition is not required throughout the process of determining energy eigenvalues, which means that whether wavefunctions are SI or not is unconcerned upon evaluating eigen energies. Based on this observation, our second goal here is to expose how SI and NSI wavefunctions cooperate to form the observed energy levels within a confining potential. For a given wavefunction
either SI or NSI, the associated quantum dynamics can be described by the quantum Hamilton equations with the quantum Hamiltonian
given by Equation (3):
where
is the complex quantum potential defined by:
Quantum potential
is intrinsic to the quantum state
and is independent of the externally applied potential
. The quantum Hamilton Equations (5) are distinct from the classical ones in two aspects: the complex nature and the state-dependent nature. The complex nature is a consequence of the fact that the canonical variables
solved from Equation (5) are, in general, complex variables. The state-dependent nature means that Equation (5) governs the quantum motion specifically in the quantum state described by
. The Hamilton Equations (5), which is usually regarded as the complex-extension of Bohmian mechanics, can be derived independently by the optimal stochastic control theory [
27].
For a given wavefunction , the complex contour traced by can be solved from Equation (5a), along which the contour integral in Equation (1) then can be evaluated. The second Hamilton Equation (5b) is an alternative expression of the Schrödinger Equation (4) as can be shown by substituting from Equation (2) and from Equation (6).
3. Time Average along a Complex Quantum Trajectory
The necessity of considering time average along a complex quantum trajectory comes from the fact that the action variable
introduced in Equation (1) is equal to the time-average kinetic energy, as will be shown below. For a particle confined by a time-independent potential
, we have wavefunction
and quantum action function
, with which the quantum H-J Equation (3) can be recast into the following form:
This is the energy conservation law in the quantum H-J formalism, indicating that the conserved total energy
comprises three terms: the kinetic energy
, the applied potential
, and the quantum potential
. When expressed in terms of the wavefunction
, Equation (7) and Equation (4) become the time-independent Schrödinger equation:
The energy conservation law (7) is valid for any solution
to the Schrödinger Equation (8), either SI or NSI. The Schrodinger Equation (8) has a continuum of solutions, unless it is supplemented with appropriate boundary conditions. Without loss of generality, we consider
in the form of a potential well with the property
, as
. Due to the presence of the infinite potential, the probability of finding the particle at infinity is zero, i.e.:
This boundary condition gets rid of most of the solutions to Equation (8) and selects out only a discrete set of
and
. Consequently, it is the boundary conditions in standard quantum mechanics that actually enforce the quantization. The boundary condition (9) originates from the fundamental requirement that wavefunctions must be SI, i.e.,:
which allows the normalization of the total probability to unity. If the SI condition (10) or the boundary condition (9) is released, the total energy
will be still conserved, but no longer quantized, because the participation of NSI wavefunctions
in Equation (7) will result in an arbitrary total energy
other than
. However, even if the total energy
is allowed to be varied continuously, there exist intrinsic quantization laws from which the energy eigenvalue
can be recovered. In other words, probability interpretation with the accompanying SI condition is not the only way to arrive at the quantization. This issue was first addressed by Leacock and Padgett [
2,
3] and demonstrated in detail by Bhalla [
28,
29].
Although NSI wavefunctions
fail to serve as probability density functions in the assemble average
, complex quantum trajectories for NSI wavefunction still exist, along which time average of
can be defined to substitute for the assemble average
. The complex quantum trajectory describing the particle’s motion in a confined system can be solved from Equations (5a) and (2), which together with
gives the governing equation as:
where
is a general solution to Equation (8) with given energy
. The resulting complex trajectory
serves as a physical realization of the complex contour
appearing in Equation (1), and allows the contour integral to be evaluated along the particle’s path of motion.
By treating the quantum Hamiltonian defined in Equation (7) as a Lyapunov function, the energy conservation law implies that the autonomous nonlinear system (11) is Lyapunov stable (neutrally stable) with equilibrium points in the form of centers, irrespective of whether is SI or not. The trajectory solved from Equation (11) coincides with the Lyapunov contour lines defined by , which are concentric curves surrounding equilibrium points.
The time average of
along the particle’s trajectory
is defined as:
where
is the period of oscillation of
. The quantum action variable
defined in Equation (1) is a ready example of taking time average along a complex contour. Letting
be a closed trajectory solved from Equation (11), we can rewrite the contour integral (1) in terms of the time-average kinetic energy as:
where
is the angular frequency of the periodic motion. Therefore, the Wilson-Sommerfeld quantization law
is simply an alternative expression of the energy quantization law
.
In general, the time average of an arbitrary function
can be expressed in terms of a contour integral by using Equations (11) and (12):
where
is the closed contour traced by
on the complex plane, and the symbol “prime” denotes the differentiation with respect to
. Since QMF
can be expressed as a function of
, we simply write
as
in the integrand. According to the residue theorem, the value of
is determined only by the poles of the integrand enclosed by the contour
and is independent of the actual form of
. We will see below that the discrete change of the number of poles in the integrant leads to the quantization of
.
4. General Quantization Laws without SI Condition
Let be a general solution to the Schrödinger Equation (8) with a given energy . We can treat the time average as a function of the total energy by noting that is computed by Equation (14) with wavefunction , which in turn depends on the energy . The time average is said to be quantized, if its value manifests a stair-like distribution as the total energy increases monotonically. We will derive several energy quantization laws originating from such a stair-like behavior of , which are universal for all confined quantum systems. The energy quantization defined here denotes the discrete change of the considered energy, which is different from the definition in standard quantum mechanics, where energy quantization denotes the discrete energies satisfying the SI condition (10).
Firstly, we consider the quantization of the time-average kinetic energy. By substituting
into Equation (14), we obtain:
To evaluate the above contour integral, we recall a formula from the residue theorem:
where
and
are, respectively, the numbers of zero and pole of
enclosed by the contour
. Using this formula in Equation (15) yields:
where the integer
is the difference between the numbers of zero and pole of
. It appears that the time average of the particle’s kinetic energy in a confined potential is an integer multiple of
. This is an universal quantization law independent of the confining potential
. Using Equation (17) in Equation (13), we recover the Wilson-Sommerfeld quantization law
.
The other quantized energy is the quantum potential
. The evaluation of Equation (14) with
gives:
Applying Formula (16) once again, we arrive at the second energy quantization law:
where integer
is the difference between the numbers of zero and pole of
. Like the quantization of
, Equation (19) reveals that the value of
is an integer multiple of
, irrespective of the confining potential
.
The Kinetic energy
and the quantum potential energy
, individually, are quantized quantities, and their combination leads to another quantization law. This can be verified from the combination of Equations (15) and (18):
where in the integrand can be simplified further as:
With the above simplification and the Formula (16), Equation (20) yields a new quantization law:
where integer
is the difference between the numbers of zero and pole of
.
The three integers,
,
and
, are solely determined by the wavefunction
, which in turn is solved from Equation (8) with a prescribed energy
. As
increases, the three integers can only change discretely in response to the continuous change of
. Let
be the sequence of specific energies at which the integer
experiences a step jump,
. With increasing
, the value of
then assumes a stair-like distribution described by:
The values of and have a similar distribution. It is noted that the wavefunction solved from Equation (8) with an energy in the interval is generally NSI. Our next task is to clarify the roles of these NSI wavefunctions in the quantization process of and .
5. Energy Quantization beyond SI Wavefunctions
To elucidate how SI and NSI wavefunctions cooperate to form the observed quantization levels, we consider the typical quantum motion under a quadratic confining potential
. The related Schrödinger equation in dimensionless form is:
where the total energy
is allowed to be any positive real number. A general solution to the Schrödinger Equation (23), which takes into account NSI wavefunctions, can be expressed in terms of the Whittaker function
as:
where
is the hypergeometric function, and
is the Gamma function. Detailed discussions on the above-mentioned special functions can be found in standard textbooks of physical mathematics [
30]. For a given energy
, the obtained solution
is generally NSI, except for the energy eigenvalues
, at which Equation (24b) becomes:
Depending on whether is even or odd, simplification of is given respectively by:
:
where we note
in Equation (25) for negative integer
. Combining the above two equations yields the eigenfunctions
for the quantum harmonic oscillator. The eigenfunctions
are the only solutions to the Schrödinger Equation (23), satisfying the boundary condition (9) and the SI condition (10).
All the existing discussions on energy quantization in the harmonic oscillator focus on the SI eigenfunctions and their linear combinations. Here we are interested in the energy quantization related to the NSI wavefunctions described by Equation (24) with
. According to Equations (17) and (21), the quantization of
and
is determined by the numbers of zero and pole of
and
. Examining the expression for
given by Equation (24b), we find that
and
do not have any pole over the entire complex plane, because the hypergeometric function
and its derivative are analytic functions for any
. Accordingly, we have
, and:
Hence the three quantum numbers,
,
and
, can be determined by the two independent integers:
and
, the numbers of zero of
and
, respectively. Regarding the computation of
, we can find the zero of
by solving the roots of the Whittaker function according to Equation (24a):
For a given energy
, the resulting root is denoted by
, and
is the number of
satisfying Equation (28). The blue line in
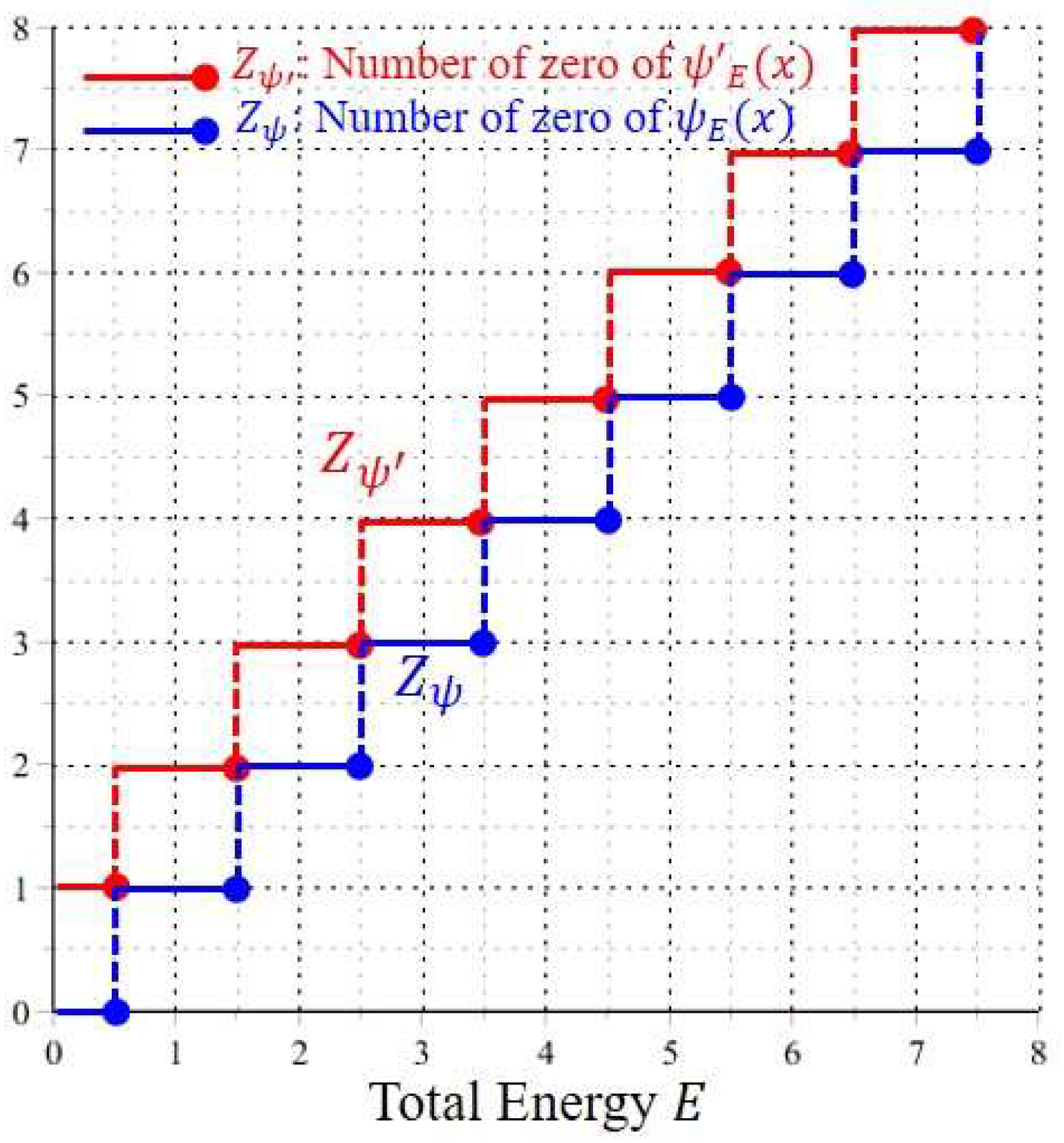
Figure 1 illustrates the variation of
with respect to the energy
. Similarly,
can be found by solving the roots of
:
The resulting root is denoted by
and the number of
satisfying Equation (29) for a given energy
gives the value of
. The red line in
Figure 1 illustrates the variation of
with respect to the energy
.
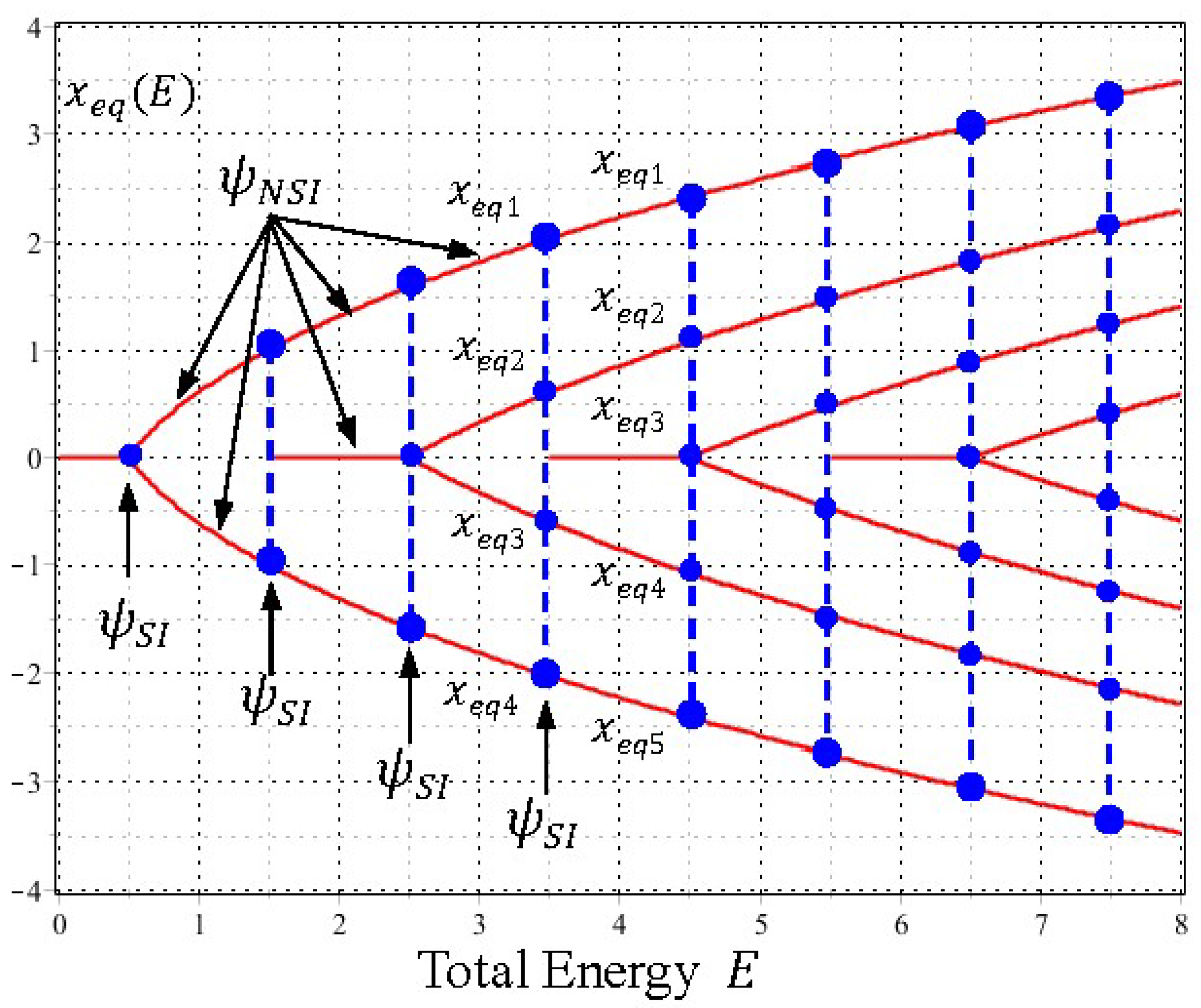
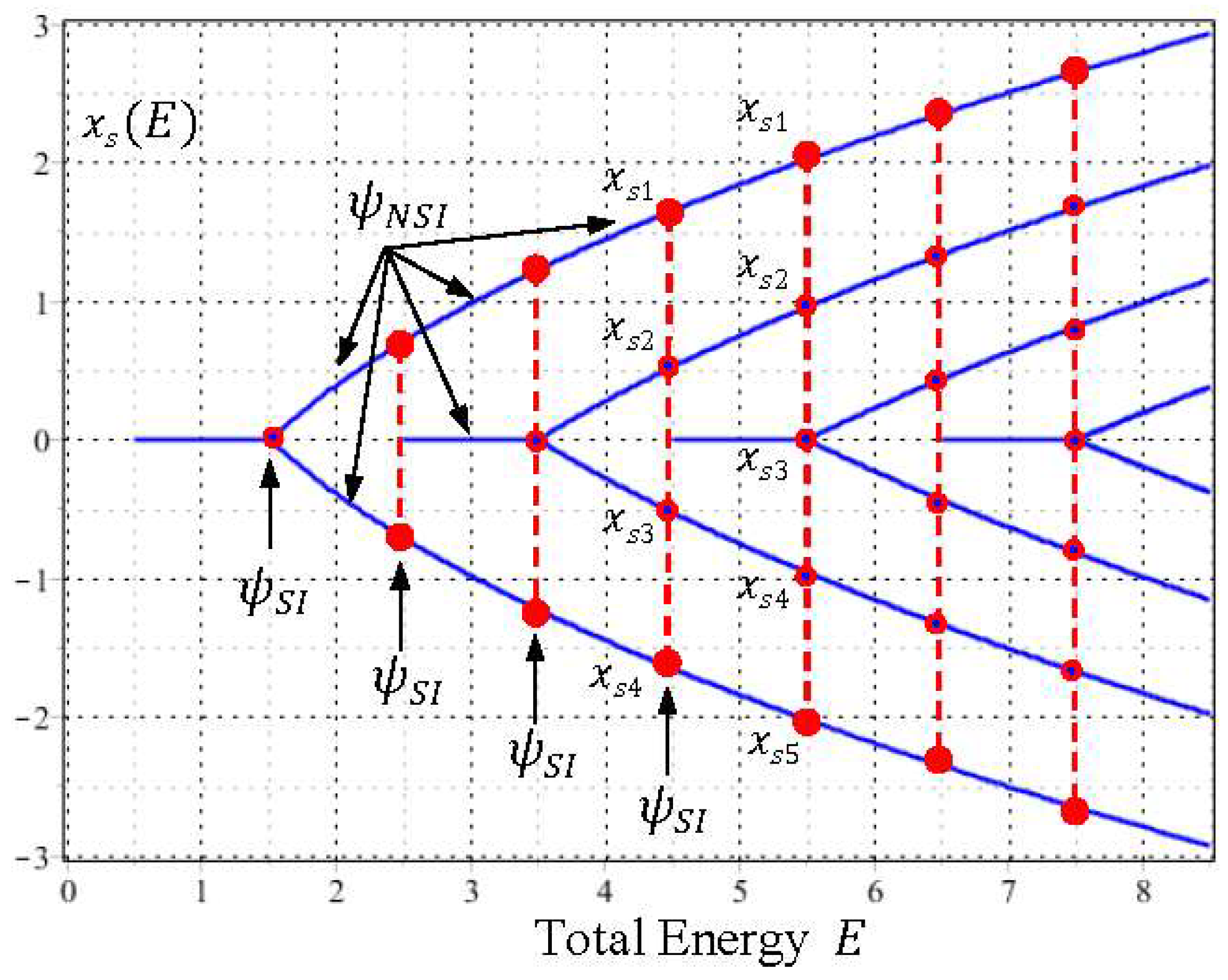
As can be seen from
Figure 1, when the total energy
increases monotonically,
and
exhibit a stair-like distribution in the form of:
and
,
, as
. Based on the above distributions of
and
, the quantization laws derived in Equations (17), (19) and (21) now become:
when the total energy
falls in the interval
. All the energies in Equation (31) have been expressed in terms of the multiples of
. Consequently, as we increase the total energy
monotonically,
and
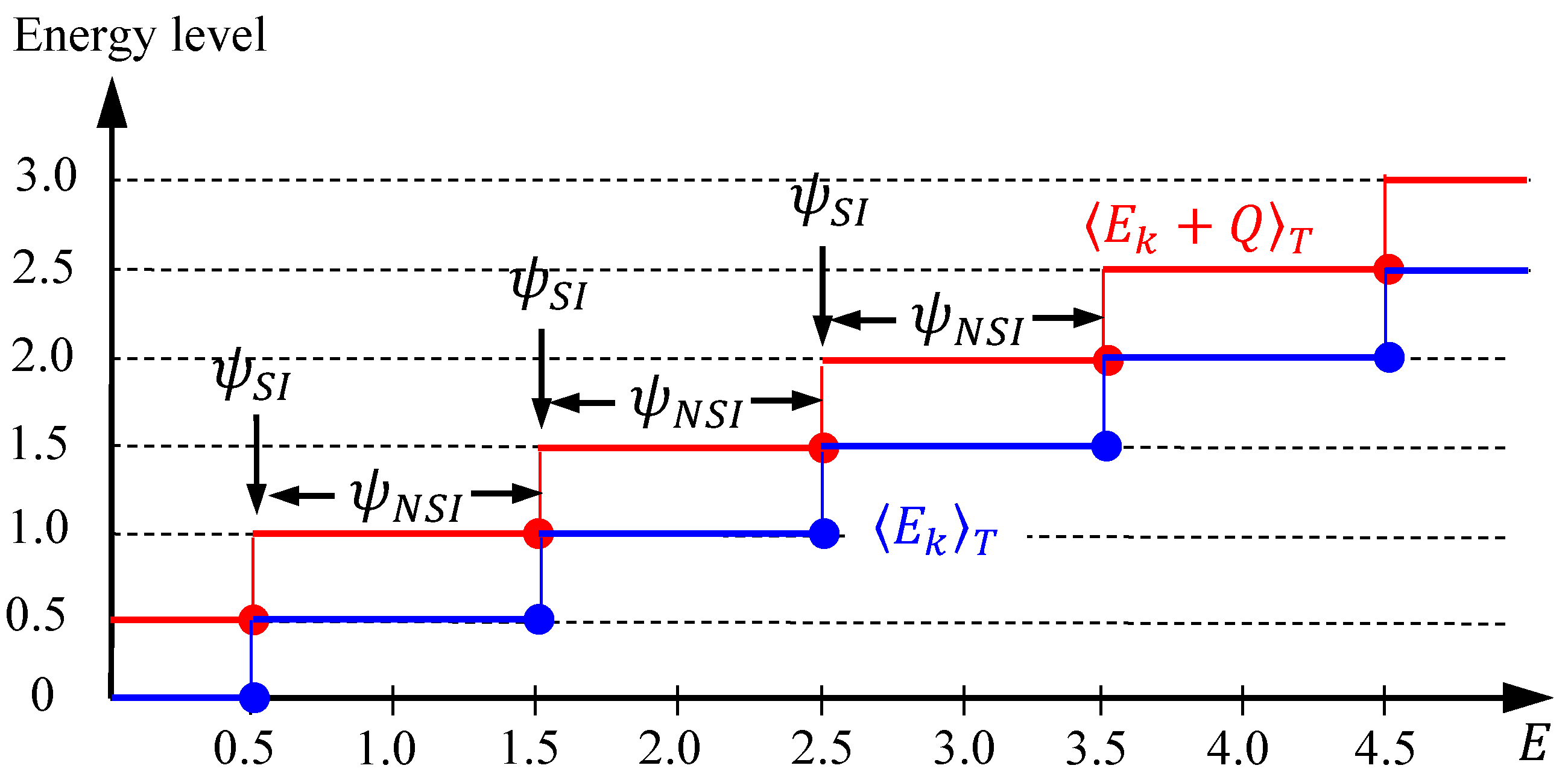
increase in a stair-like manner with the step levels given by Equation (31), as shown in
Figure 2. Up to this stage, the two components
and
in the energy conservation law (7) have been found to be quantized, while the third component, i.e., the externally applied potential
, is not a quantized quantity, which otherwise changes continuously with
via the relation:
The most noticeable point is that the step change of
and
occurs at the specific energies
, which coincide with the energy eigenvalues of the harmonic oscillator. In other words, the role of the SI condition amounts to determining the discrete energy
at which the numbers of zero of
and
exhibit a step jump, while the role of the NSI wavefunctions
with
is to form the flat parts of the stair-like distribution as shown in
Figure 1 and
Figure 2, where the numbers of zero of
and
, or equivalently the time-average energies
and
, keep unchanged.
6. Quantum Bifurcation beyond SI Wavefunctions
As the total energy increases, the wavefunction transits repeatedly from NSI states to a SI state, once coincides with an energy eigenvalue . In this section, we will show that the encounter with an energy eigenvalue not only causes a step jump of and , but also causes a nonlinear phenomenon - quantum bifurcation, where the number of equilibrium points of the quantum dynamics experiences an instantaneous change.
With
given by Equation (24a), the quantum dynamics (11) assumes the following dimensionless form:
where the total energy
is treated as a free parameter, whose critical values for the occurrence of bifurcation are to be identified. The quantum trajectories
solved from Equation (33) provide us with a quantitative comparison between SI and NSI wavefunctions, which otherwise cannot be compared under the probability interpretation of
.
As can be seen from Equation (33), the equilibrium point
of the quantum dynamics is equal to the zero of
, while the singular point
is just the zero of
. Hence the step changes of
and
shown in
Figure 1 also imply the step changes of the numbers of the equilibrium points
and the singular points
, respectively. In other words, we can say that the following two processes occur synchronously as
increases monotonically: one process is the quantization of
and
regarding the step changes of
and
as discussed previously, and the other is the bifurcation of the quantum dynamics (33) regarding the step changes of the equilibrium points and singular points, as to be discussed below.
(1) SI wavefunctions:
Firstly, we consider the special cases that the total energy
happens to be one of the eigen energies:
,
, and
. The related eigenfunctions and the eigen-dynamics derived from Equation (33) are given by:
These three equations describe the velocity fields and their solutions give the eigen-trajectories for the first three SI states of the harmonic oscillator. It can be shown that the equilibrium points of Equation (34) are centers, while their singular points are saddles. For instance,
in Equation (34a) has an equilibrium point at the origin with solution given by
, whose trajectories on the complex plane are concentric circles around the equilibrium point, showing that
is a center. To show singular points in Equation (34) are saddles, we consider the following complex-valued system with a singular point at the origin:
where
is analytic at
. In a neighborhood of the origin, Equation (35) can be approximated by
, where
is the residue of
evaluated at
. The substitution of
and
into
leads to the equivalent real-valued nonlinear system:
Its solution is a set of hyperbolas expressed by
, showing that the singular point
in Equation (35) are saddles. The centers and saddles of Equation (34) generated by the SI wavefunctions
with
,
, and
are illustrated in
Figure 3, which displays the distribution and movement of the centers and saddles of the quantum dynamics (33) on the horizontal
axis, as the total energy
changes continuously along the vertical axis. Detailed discussions on the quantum trajectories of the SI wavefunctions
for a harmonic oscillator were reported in the literature [
31,
32]. Here our concern is the quantum trajectories of the NSI wavefunctions
with
. Quantum trajectories in the first three quantization intervals of
will be examined below, from which a global picture of center-saddle bifurcation can be drawn.
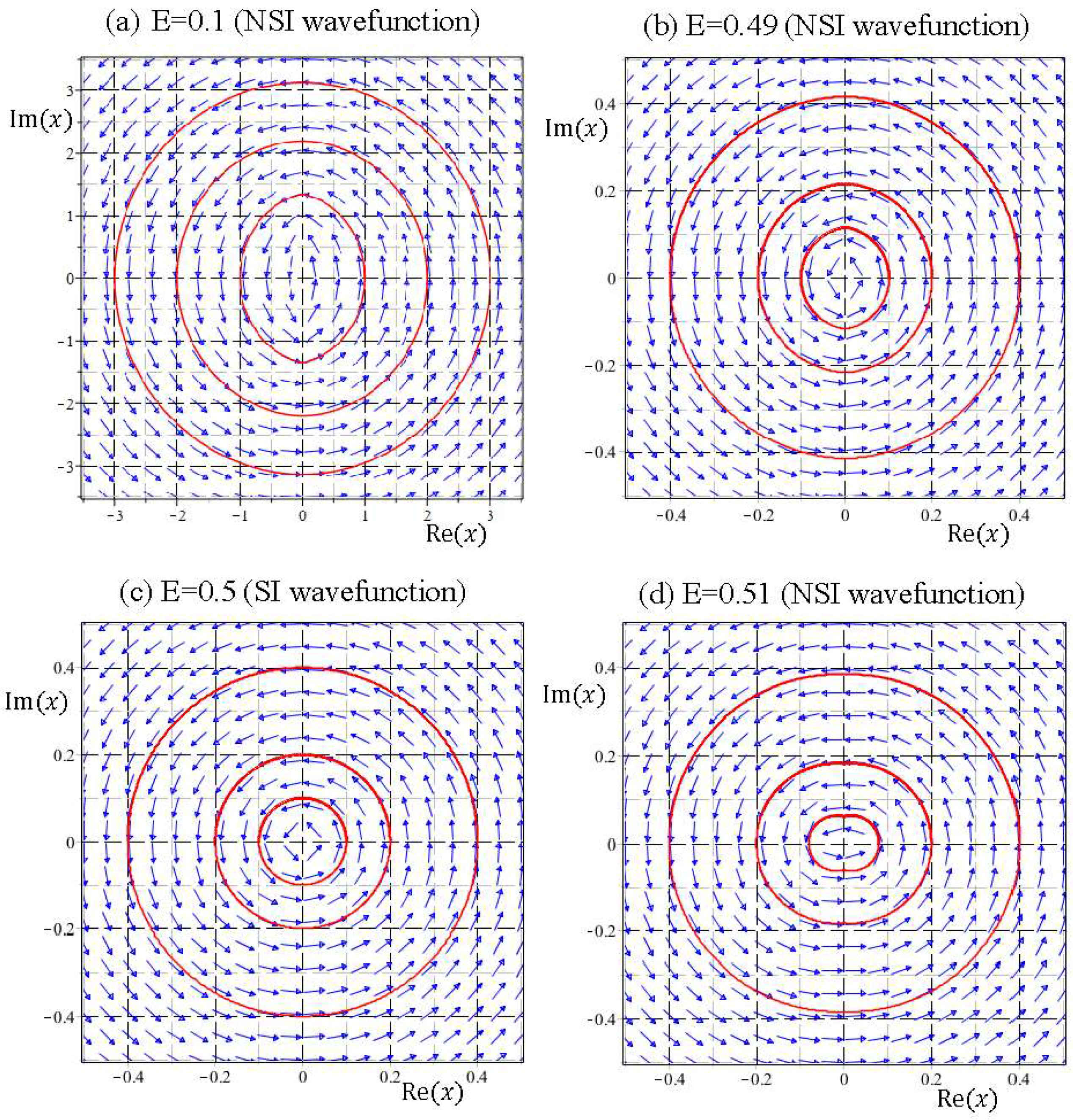
(2) NSI wavefunctions with :
The wavefunction
in this range of energy is NSI, except for
. It seems to be a reasonable conjecture that NSI wavefunctions naturally give rise to unbound quantum trajectories; however, this is not the case.
Figure 4 illustrates the quantum trajectories solved from Equation (33) for the NSI wavefunctions
with
,
, and
. In spite of being generated by NSI wavefunctions, the resulting quantum trajectories are bound with slight deviations from the eigen-trajectories of
, which are concentric circles around the equilibrium point at the origin, as described by Equation (34a). It appears that SI eigenfunctions are not isolated from the neighboring NSI wavefunctions, because their quantum trajectories can be deformed continuously into each other.
(3) NSI wavefunctions with :
According to
Figure 1, the number of equilibrium points
of the quantum dynamics (33), jumps from one to two as
across the energy eigenvalue
. This bifurcation phenomenon is illustrated in
Figure 5. There is only one equilibrium point at the origin in the energy interval
, while beyond the bifurcation point
, two equilibrium points come out from the origin. Particular attention is paid to the quantum trajectories of
depicted in
Figure 4d. At first glance, it looks like that the quantum trajectories of
have a single equilibrium point at the origin. However, the enlargement of
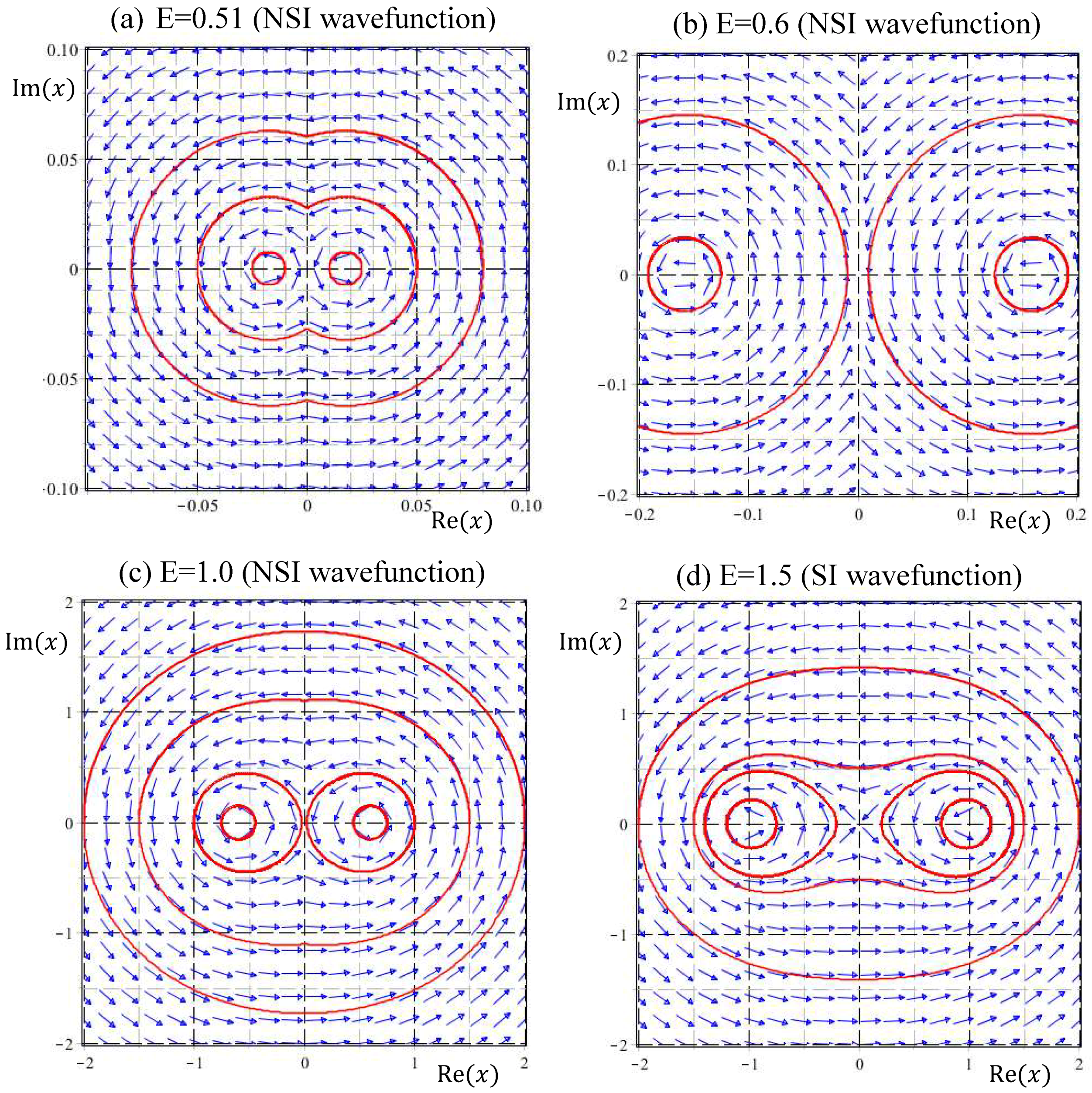
Figure 4d near the origin as illustrated in
Figure 5a indicates that the single equilibrium point at the origin for
splits into two equilibrium points as
increases to 0.51. When
increases to 3/2, the two equilibrium points (two centers) move further to
, as described by the quantum dynamics (34b) and illustrated in
Figure 5d.
Coincident with the splitting of the equilibrium point at the bifurcation point
, a singular point emerges from the origin in the form of a saddle point. The resulting saddle point pattern in the vicinity of the origin is clearly manifested in
Figure 5. It turns out that at the bifurcation energy
, two kinds of bifurcation occur simultaneously: one bifurcation regards the change of the number of equilibrium points from a single center at the origin into a pair of centers moving apart along the positive and negative real axis as
increases from
to
, and the other bifurcation regards the change from a center into a saddle at the origin.
(4) NSI wavefunctions with :
At the energy eigenvalue
, the value of
experiences the second step jump, and a new bifurcation is expected to form here. This prediction is confirmed in
Figure 6a, where the enlargement of the velocity field near the origin shows that the saddle-point singularity at the origin for
now transforms into a center for
. As
increases further to
and
, flow circulation around the origin as a center becomes more apparent. Counting the new equilibrium point emerging from the origin and the already existing pair of centers, the number of equilibrium points increases from two to three as
across
, and remains three in the interval
. Coincident with the emergence of a new center from the origin at
, the singular saddle point previously residing at the origin now splits into a pair of saddles with their separation increasing with
. The two saddles move to
when
increases to
, as described by Equation (34c) and illustrated in
Figure 6d.
(5) Center-Saddle Bifurcation
When we proceed further, the bifurcations of the equilibrium centers
and the singular saddles
of the quantum dynamics (33) occur alternatively as
increases. To gain a global picture of the bifurcation pattern, we solve the equilibrium points
and the singular points
from Equations (29) and (28), respectively, and then plot them as functions of
. The resulting plots generate two sequences of pitchfork bifurcation diagram as shown in
Figure 7 and
Figure 8 for
and
, respectively. It can be seen that the bifurcations of
and
occur alternatively at the critical energies
in such a way that the branches of
bifurcate sequentially at
, while the branches of
bifurcate sequentially at
.
Furthermore, it is worth noting that except for the bifurcation points (the blue dots in
Figure 7 and the red dots in
Figure 8), the sequential bifurcation diagram is constructed entirely by the NSI wavefunctions
with
. Without the participation of the NSI wavefunctions, adjacent eigenfunctions lose their interconnection and a continuous description of the bifurcation sequence becomes impossible. The other perspective of center-saddle bifurcation can be gained from
Figure 3, where we can see that centers and saddles appear alternatively at the origin as the total energy
increases monotonically along the vertical axis.
(6) Synchronicity between quantization and bifurcation
We recall that the number of
at each
is just the number of zero of
, which gives the quantization level of
. This relation indicates that the bifurcation of the equilibrium center point
and the quantization of
occur synchronously. Similarly, because the number of
at each
is the number of zero of
, which gives the quantization level of
, the bifurcation of the singular saddle point
is thus synchronous with the quantization of
.
Table 1 lists the numbers of saddles and centers, and the energy levels of
and
for the first several energy intervals. As can be seen, the numbers of saddles and centers change synchronously with the change of
and
. It is noted that as energy
varies continuously during the quantization and bifurcation processes, the instantaneous changes of energy levels and equilibrium points are triggered by the SI condition
.
7. Spin Degree of Freedom beyond SI Wavefunctions
The role of the Schrödinger equation has long been considered as describing spinless particles only, because the Schrödinger charge current for the s-states of a hydrogen-like atom vanishes and produces no intrinsic angular momentum. However, based on the observation that in the absence of a magnetic field, the Pauli equation reduces to the Schrödinger equation, it was pointed out [
33] that the Schrödinger equation must be regarded as describing an electron in an eigenstate of spin and not, as universally supposed, an electron without spin. According to the dBB trajectory approach [
34,
35], spin is interpreted as a dynamical property of electron motion and is attributed to a circulating movement of a point, i.e., to a pure orbital motion, but not to an extended spinning object. To be consistent with the Dirac theory and with the condition of Lorentz invariance, Holland [
34] proposed that the Schrödinger charge current must be supplemented by a spin magnetization current, which is generated by a circulating flow of energy in the wave field of the electron [
36,
37].
The NSI solutions to the Schrödinger equation considered in the present paper might give an alternative explanation for the origin of particle’s spin motion. The Schrödinger Equation (8) with given energy
actually has two independent solutions. It is surprising to find that the quantum trajectories generated by the two independent solutions are indistinguishable, except for their directions of rotation. Inspecting the quantum trajectories shown in
Figure 4,
Figure 5 and
Figure 6, it appears that all the trajectories, either generated by SI or NSI wavefunctions, rotate counterclockwise (CCW). In fact, all the trajectories produced by the general wavefunctions given by Equation (24) rotate in the same direction, because Equation (24) only gives one of the independent solutions. A complete general solution to the Schrödinger Equation (23) comprises two independent parts:
where
is the solution considered previously and
is the other independent solution, whose quantum trajectories rotate clockwise (CW). The wavefunction
represents the second half of solutions to the Schrödinger Equation (23), which is NSI for any energy
and we usually take the neglect of it as granted.
The consideration of the wavefunctions
helps to identify the additional degree of freedom independent of the particle’s orbital motion. To highlight the difference between
and
, their velocity fields computed by Equation (11) with
are illustrated in
Figure 9a,b, respectively. The velocity field of
is identical to
Figure 4c, which shows circular flows surrounding the origin counterclockwise. By contrast, the velocity field of
depicted in
Figure 9b appears to be clockwise circulation around the origin. The quantum trajectories generated by
are almost indistinguishable from those generated by
, and the only difference between them is the directions of rotation. In addition to the orbital motion, the new degree of freedom manifested in the combination of
and
is the dual directions of rotation, which is otherwise invisible along a single trajectory generated by either
or
. Due to their same spatial motion with dual directions,
and
can be reasonably recognized as the same spatial solution to the Schrödinger equation but with opposing directions of spin.
The reason underlying the opposite rotation of
and
can be explained by using the asymptotic expansion property for the Whittaker function:
With this property, the asymptotic expansions of
and
take the following forms:
The asymptotic quantum dynamics of
and
then can be derived as:
Irrespective to the energy
, both of the asymptotic quantum dynamics converge to the ground-state quantum dynamics (34a) with the only difference in their rotation direction. This similarity in the velocity field of
and
has been ignored in the literature. Probability interpretation is only concerned with the square-integrable condition of
and
, as shown in
Figure 9c, which obviously fails to explain the similarity in the quantum dynamics of
and
.
The factor dominating the similarity in the quantum dynamics of
and
can be explained by Equation (5b):
The total potential
, comprising the applied potential
and the quantum potential
, dominates the time evolution of the QMF
. For the case of a harmonic oscillator,
turns out to be (in dimensionless form):
The evaluations of
at
and
for
are plotted in
Figure 9d. Despite of the adverse nature between SI wavefunction
and NSI wavefunction
, their total potentials exhibit a high degree of resemblance, which explains the observed similarity in the velocity fields of
Figure 9a,b. The comparisons regarding the magnitudes of
and the total potential
for
and
are shown in
Figure 10, where we observe that except for the region neighboring the origin,
is close to
and they become identical as
. This asymptotic identity leads to the dual velocity fields derived in Equation (40).
Schrödinger equation is a second-order differential equation with respect to its spatial coordinates, and its complete solution should be composed of two independent solutions. The common belief that Schrödinger equation is unable to describe spin motion seems to stem from our disregard of one of the independent solution. While probability interpretation of wavefunctions excludes the NSI solution from the general solution , the spin degree of freedom is removed at the same time. Under the framework of quantum H-J formalism, we have seen that by incorporating with to form a general wavefunction as expressed by Equation (37), both spatial and spin motion can be described by the Schrödinger equation, and even for one-dimensional quantum motion, the spin degree of freedom can be manifested as the dual rotations on the complex plane.