Slow Dynamics and Structure of Supercooled Water in Confinement
Abstract
1. Introduction
2. Results and Discussion
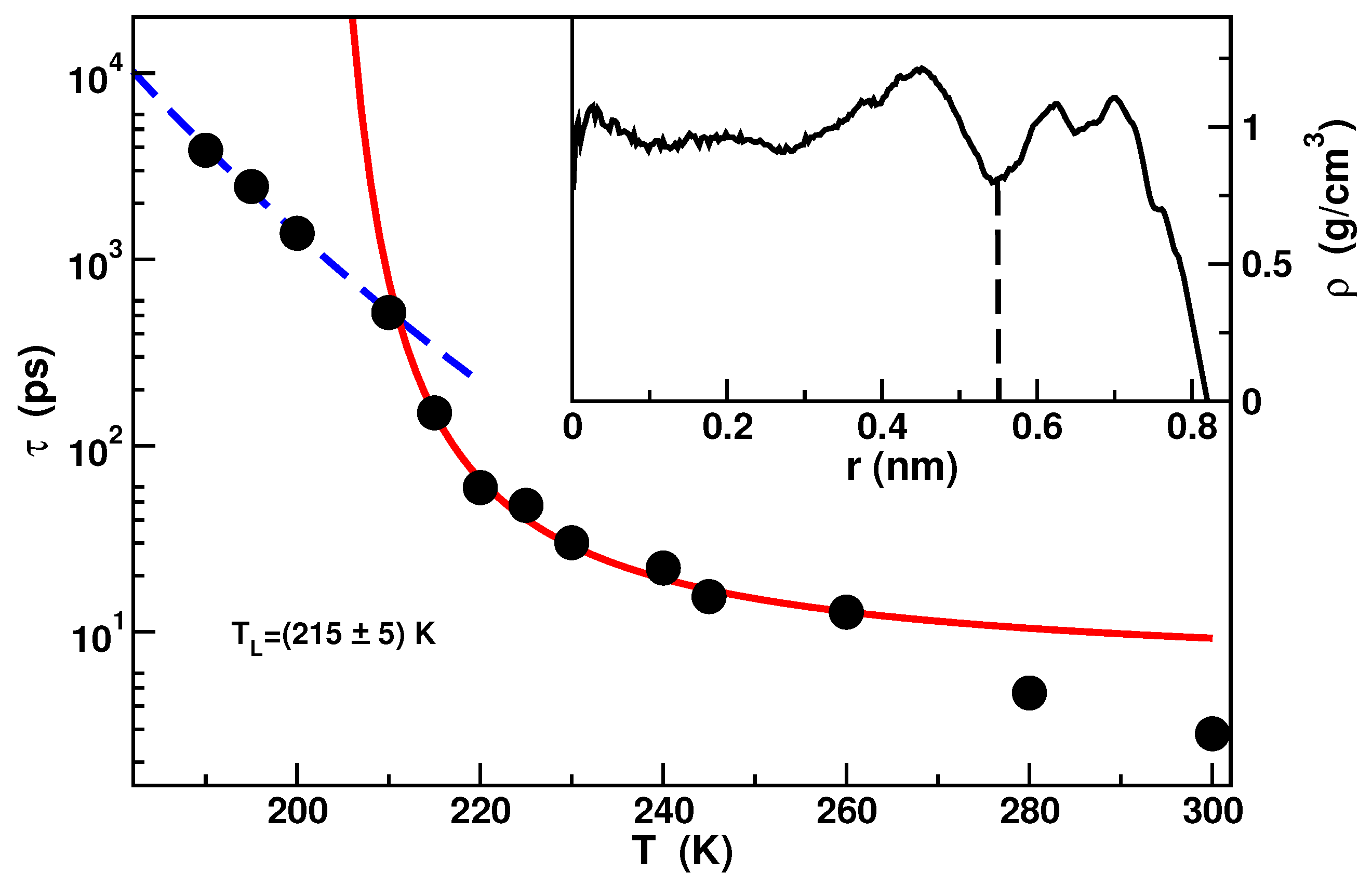
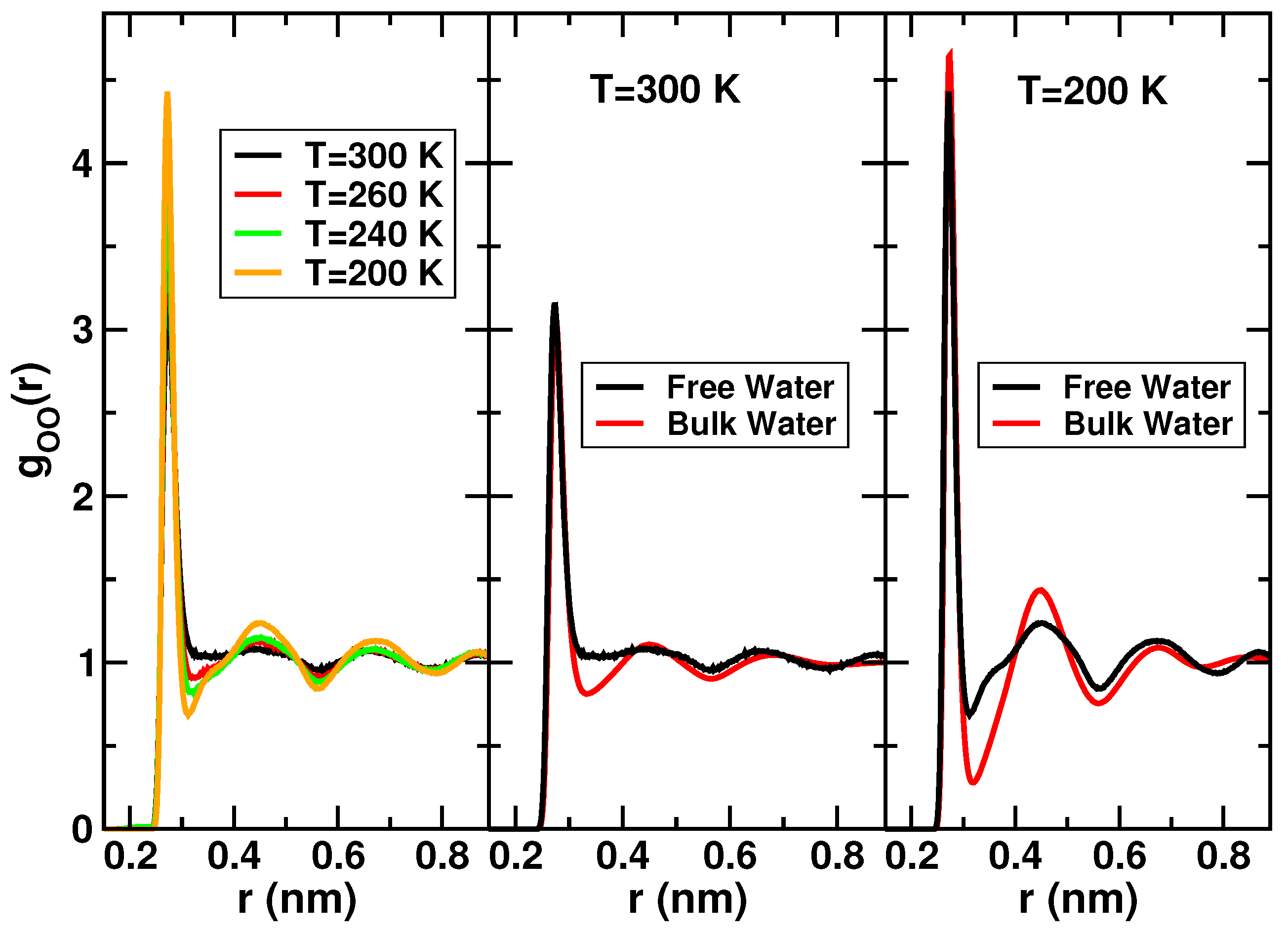
2.1. Confined Water
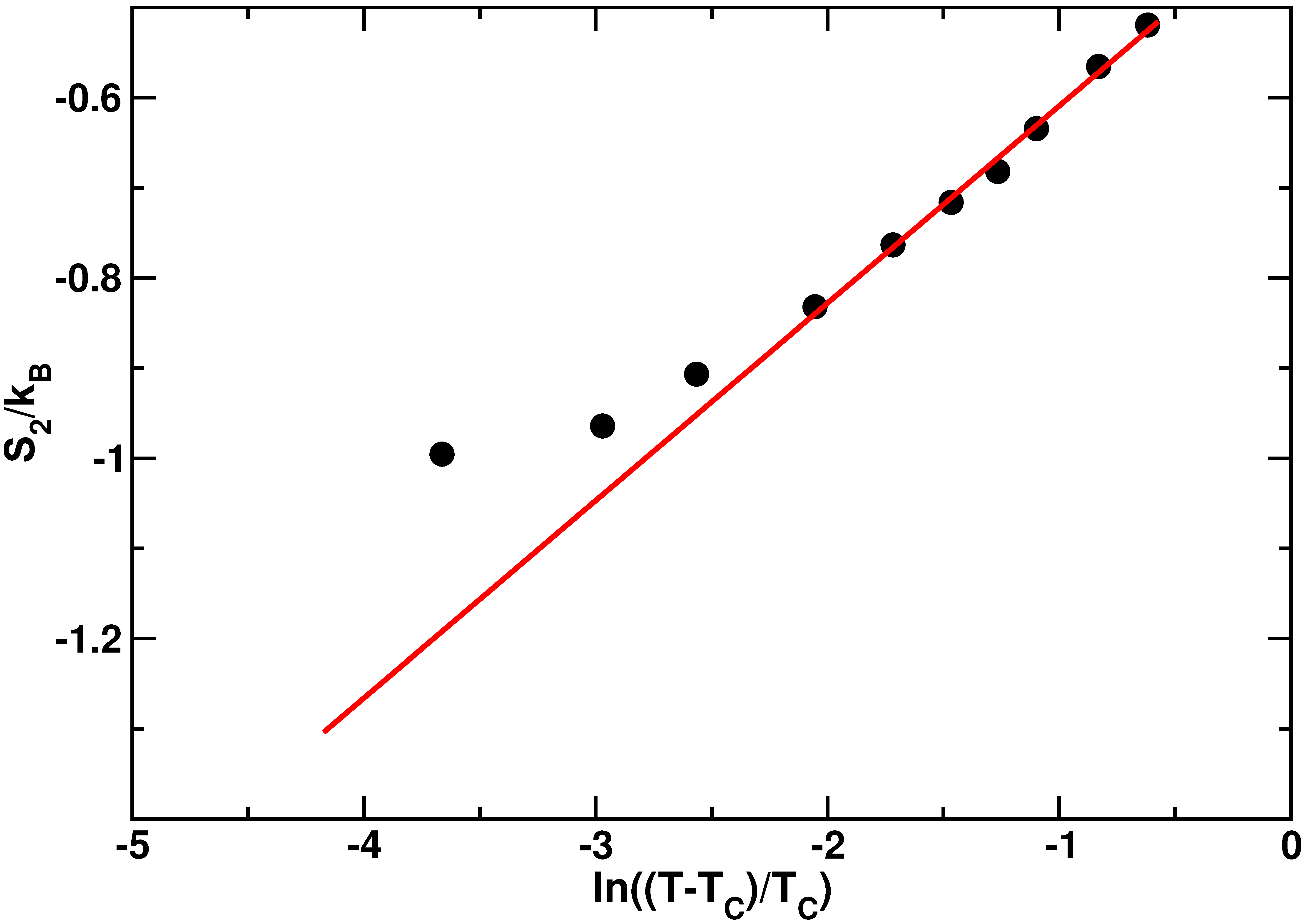
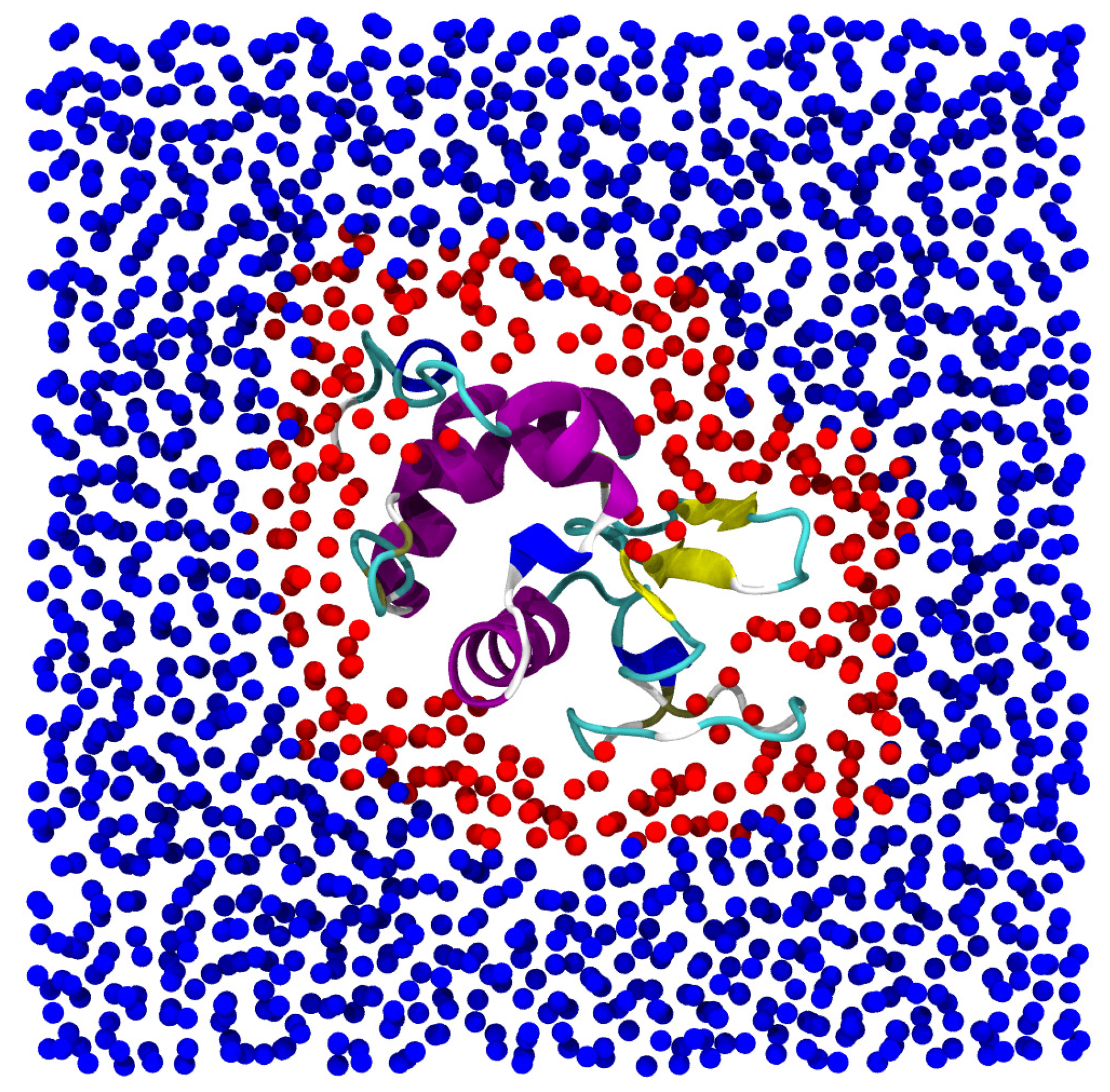
2.2. Protein Hydration Water
3. Methods
4. Conclusions
Conflicts of Interest
Abbreviations
| LLCP | Liquid-Liquid Critical Point |
| LDA | Low Density Amorphous |
| HDA | High Density Amorphous |
| LDL | Low Density Liquid |
| HDL | High Density Liquid |
| MCT | Mode Coupling Theory |
| VFT | Vogel-Fulcher-Tammann |
| FSC | Fragile to Strong Crossover |
| PDT | Protein Dynamical Transition |
| MD | Molecular Dynamics |
| SISF | Self Intermediate Scattering Function |
| RDF | Radial Distribution Functions |
References
- Franks, F. Water: A Matrix of Life, 2nd ed.; RSC Paperbacks, The Royal Society of Chemistry: Cambridge, UK, 2000. [Google Scholar]
- Ball, P. Water: Water-An enduring mystery. Nature 2008, 452, 291–292. [Google Scholar] [CrossRef] [PubMed]
- Rasaiah, J.C.; Garde, S.; Hummer, G. Water in nonpolar confinement: From nanotubes to proteins and beyond. Annu. Rev. Phys. Chem. 2008, 59, 713–740. [Google Scholar] [CrossRef] [PubMed]
- Sakai, A.; Matsumoto, T.; Hirai, D.; Niino, T. Newly developed encapsulation-dehydration protocol for plantcryopreservation. Cryoletters 1999, 21, 53–62. [Google Scholar]
- Kauzmann, W. Some factors in the interpretation of protein denaturation. Adv. Protein Chem. 1959, 14, 1–63. [Google Scholar] [PubMed]
- Gallo, P.; Amann-Winkel, K.; Angell, C.A.; Anisimov, M.A.; Caupin, F.; Chakravarty, C.; Lascaris, E.; Loerting, T.; Panagiotopoulos, A.Z.; Russo, J.; et al. Water: A Tale of Two Liquids. Chem. Rev. 2016, 116, 7463–7500. [Google Scholar] [CrossRef] [PubMed]
- Stanley, H.; Kumar, P.; Xu, L.; Yan, Z.; Mazza, M.; Buldyrev, S.; Chen, S.H.; Mallamace, F. The puzzling unsolved mysteries of liquid water: Some recent progress. Phys. A Stat. Mech. Its Appl. 2007, 386, 729–743. [Google Scholar] [CrossRef][Green Version]
- Debenedetti, P.G. Supercooled and glassy water. J. Phys. Condens. Matter 2003, 15, R1669–R1726. [Google Scholar] [CrossRef]
- Sellberg, J.A.; Huang, C.; McQueen, T.A.; Loh, N.D.; Laksmono, H.; Schlesinger, D.; Sierra, R.G.; Nordlund, D.; Hampton, C.Y.; Starodub, D.; et al. Ultrafast X-ray probing of water structure below the homogeneous ice nucleation temperature. Nature 2014, 510, 381–384. [Google Scholar] [CrossRef] [PubMed]
- Holten, V.; Bertrand, C.E.; Anisimov, M.A.; Sengers, J.V. Thermodynamics of supercooled water. J. Chem. Phys. 2012, 136, 094507. [Google Scholar] [CrossRef] [PubMed]
- Speedy, R.J.; Angell, C.A. Isothermal compressibility of supercooled water and evidence for a thermodynamic singularity at 45 ∘C. J. Chem. Phys. 1976, 65, 851. [Google Scholar] [CrossRef]
- Mishima, O.; Stanley, H.E. The relationship between liquid, supercooled and glassy water. Nature 1998, 396, 329–335. [Google Scholar]
- Mishima, O.; Calvert, L.D.; Whalley, E. An apparently first-order transition between two amorphous phases of ice induced by pressure. Nature 1985, 314, 76–78. [Google Scholar] [CrossRef]
- Winkel, K.; Elsaesser, M.; Mayer, E.; Loerting, T. Water polyamorphism: Reversibility and (dis)continuity. J. Chem. Phys. 2008, 128, 044510. [Google Scholar] [CrossRef] [PubMed]
- Kim, C.U.; Barstow, B.; Tate, M.V.; Gruner, S.M. Evidence for liquid water during the high-density to low-density amorphous ice transition. Proc. Natl. Acad. Sci. USA 2009, 106, 4596–4600. [Google Scholar] [CrossRef] [PubMed]
- Winkel, K.; Mayer, E.; Loerting, T. Equilibrated High-Density Amorphous Ice and Its First-Order Transition to the Low-Density Form. J. Phys. Chem. B 2011, 115, 14141–14148. [Google Scholar] [CrossRef] [PubMed]
- Klotz, S.; Strässle, T.; Nelmes, R.; Loveday, J.; Hamel, G.; Rousse, G.; Canny, B.; Chervin, J.; Saitta, A. Nature of the polyamorphic transition in ice under pressure. Phys. Rev. Lett. 2005, 94, 025506. [Google Scholar] [CrossRef] [PubMed]
- Stanley, H.E. Unsolved Mysteries of Water in Its Liquid and Glass States. MRS Bull. 1999, 24, 22–30. [Google Scholar] [CrossRef]
- Mishima, O.; Stanley, H.E. Decompression-induced melting of ice IV and the liquid-liquid transition in water. Nature 1998, 392, 164–168. [Google Scholar] [CrossRef]
- Speedy, R.J. Stability-limit conjecture. An interpretation of the properties of water. J. Chem. Phys. 1982, 86, 982–991. [Google Scholar] [CrossRef]
- Poole, P.H.; Sciortino, F.; Essmann, U.; Stanley, H.E. Phase behaviour of metastable water. Nature 1992, 360, 324–328. [Google Scholar] [CrossRef]
- Holten, V.; Anisimov, M. Entropy-driven liquid-liquid separation in supercooled water. Sci. Rep. 2012, 2, 713. [Google Scholar] [CrossRef] [PubMed]
- Nilsson, A.; Pettersson, L.G.M. Perspective on the structure of liquid water. Chem. Phys. 2011, 389, 1–34. [Google Scholar] [CrossRef]
- Harrington, S.; Poole, P.H.; Sciortino, F.; Stanley, H.E. Equation of state of supercooled water simulated using the extended simple point charge intermolecular potential. J. Chem. Phys. 1997, 107, 7443–7450. [Google Scholar] [CrossRef]
- Yamada, M.; Mossa, S.; Stanley, H.; Sciortino, F. Interplay between Time-Temperature Transformation and the Liquid-Liquid Phase Transition in Water. Phys. Rev. Lett. 2002, 88, 195701. [Google Scholar] [CrossRef] [PubMed]
- Poole, P.H.; Saika-Voivod, I.; Sciortino, F. Density minimum and liquid-liquid phase transition. J. Phys. Condens. Matter 2005, 17, L431. [Google Scholar] [CrossRef]
- Paschek, D. How the liquid-liquid transition affects hydrophobic hydration in deeply supercooled water. Phys. Rev. Lett. 2005, 94, 217802. [Google Scholar] [CrossRef] [PubMed]
- Corradini, D.; Rovere, M.; Gallo, P. A route to explain water anomalies from results on an aqueous solution of salt. J. Chem. Phys. 2010, 132, 134508. [Google Scholar] [CrossRef] [PubMed]
- Abascal, J.L.F.; Vega, C. Widom line and the liquid-liquid critical point for the TIP4P/2005 water model. J. Chem. Phys. 2010, 133, 234502. [Google Scholar] [CrossRef] [PubMed]
- Palmer, J.; Car, R.; Debenedetti, P. The liquid-liquid transition in supercooled ST2 water: A comparison between umbrella sampling and well-tempered metadynamics. Faraday Discuss. 2013, 167, 77. [Google Scholar] [CrossRef] [PubMed]
- Palmer, J.; Martelli, F.; Liu, Y.; Car, R.; Panagiotopoulos, A.Z.; Debenedetti, P.G. Metastable liquid-liquid transition in a molecular model of water. Nature 2014, 510, 385. [Google Scholar] [CrossRef] [PubMed]
- Poole, P.H.; Bowles, R.K.; Saika-Voivod, I.; Sciortino, F. Free energy surface of ST2 water near the liquid-liquid phase transition. J. Chem. Phys. 2013, 138, 034505. [Google Scholar] [CrossRef] [PubMed]
- Gallo, P.; Sciortino, F. Ising Universality Class for the Liquid-Liquid Critical Point of a One Component Fluid: A Finite-Size Scaling Test. Phys. Rev. Lett. 2012, 109, 177801. [Google Scholar] [CrossRef] [PubMed]
- McMillan, P.F.; Stanley, E.H. Fluid phases: Going supercritical. Nat. Phys. 2010, 6, 479–480. [Google Scholar] [CrossRef]
- Xu, L.; Kumar, P.; Buldyrev, S.V.; Chen, S.H.; Poole, P.H.; Sciortino, F.; Stanley, H.E. Relation between the Widom line and the dynamic crossover in systems with a liquid-liquid phase transition. Proc. Natl. Acad. Sci. USA 2005, 102, 16558–16562. [Google Scholar] [CrossRef] [PubMed]
- Franzese, G.; Stanley, H.E. The Widom line of supercooled water. J. Phys. Condens. Matter 2007, 19, 205126. [Google Scholar] [CrossRef]
- Gallo, P.; Sciortino, F.; Tartaglia, P.; Chen, S.H. Slow Dynamics of Water Molecules in Supercooled States. Phys. Rev. Lett. 1996, 76, 2730–2733. [Google Scholar] [CrossRef] [PubMed]
- Sciortino, F.; Gallo, P.; Tartaglia, P.; Chen, S.H. Supercooled water and the kinetic glass transition. Phys. Rev. E 1996, 54, 6331. [Google Scholar] [CrossRef]
- Torre, R.; Bartolini, P.; Righini, R. Structural relaxation in supercooled water by time-resolved spectroscopy. Nature 2004, 428, 296–299. [Google Scholar] [CrossRef] [PubMed]
- Götze, W.; Sjogren, L. Relaxation processes in supercooled liquids. Rep. Prog. Phys. 1992, 55, 241–376. [Google Scholar] [CrossRef]
- Götze, W. Complex Dynamics of Glass-Forming Liquids; Oxford University Press: New York, NY, USA, 2009; pp. 1–656. [Google Scholar]
- Dehaoui, A.; Issenmann, B.; Caupin, F. Viscosity of deeply supercooled water and its coupling to molecular diffusion. Proc. Natl. Acad. Sci. USA 2015, 112, 12020–12025. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Chen, S.H.; Faraone, A.; Yen, C.W.; Mou, C.Y. Pressure dependence of fragile-to-strong transition and a possible second critical point in supercooled confined water. Phys. Rev. Lett. 2005, 95, 117802. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Chen, S.H.; Faraone, A.; Yen, C.W.; Mou, C.Y.; Kolesnikov, A.I.; Mamontov, E.; Leao, J. Quasielastic and inelastic neutron scattering investigation of fragile-to-strong crossover in deeply supercooled water confined in nanoporous silica matrices. J. Phys. Condens. Matter 2006, 18, S2261. [Google Scholar] [CrossRef]
- Mallamace, F.; Broccio, M.; Corsaro, C.; Faraone, A.; Wanderlingh, U.; Liu, L.; Mou, C.; Chen, S.H. The fragile-to-strong dynamic crossover transition in confined water: Nuclear magnetic resonance results. J. Chem. Phys. 2006, 124, 161102. [Google Scholar] [CrossRef] [PubMed]
- Faraone, A.; Liu, L.; Mou, C.Y.; Yen, C.W.; Chen, S.H. Fragile-to- strong liquid transition in deeply supercooled confined water. J. Chem. Phys. 2004, 121, 10843–10846. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Lagi, M.; Fratini, E.; Baglioni, P.; Mamontov, E.; Chen, S.H. Dynamic susceptibility of supercooled water and its relation to the dynamic crossover phenomenon. Phys. Rev. E 2009, 79, 040201. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Le, P.; Ito, K.; Leão, J.B.; Tyagi, M.; Chen, S.H. Dynamic crossover in deeply cooled water confined in MCM-41 at 4 kbar and its relation to the liquid-liquid transition hypothesis. J. Chem. Phys. 2015, 143, 114508. [Google Scholar] [CrossRef] [PubMed]
- Starr, F.W.; Sciortino, F.; Stanley, H.E. Dynamics of simulated water under pressure. Phys. Rev. E 1999, 60, 6757–6768. [Google Scholar] [CrossRef]
- Gallo, P.; Rovere, M. Mode coupling and fragile to strong transition in supercooled TIP4P water. J. Chem. Phys. 2012, 137, 164503. [Google Scholar] [CrossRef] [PubMed]
- Gallo, P.; Rovere, M.; Chen, S.H. Dynamic Crossover in Supercooled Confined Water: Understanding Bulk Properties through Confinement. J. Phys. Chem. Lett. 2010, 1, 729–733. [Google Scholar] [CrossRef]
- De Marzio, M.; Camisasca, G.; Rovere, M.; Gallo, P. Mode Coupling Theory And Fragile To Strong Transition in Supercooled TIP4P/2005 Water. J. Chem. Phys. 2016, 144, 074503. [Google Scholar] [CrossRef] [PubMed]
- Franzese, G.; Bianco, V. Water at Biological and Inorganic Interfaces. Food Biophys. 2013, 8, 153–169. [Google Scholar] [CrossRef]
- Chen, S.H.; Liu, L.; Fratini, E.; Baglioni, P.; Faraone, A.; Mamontov, E.; Fomina, M. Observation of fragile-to-strong dynamic crossover in protein hydration water. Proc. Natl. Acad. Sci. USA 2006, 103, 9012–9016. [Google Scholar] [CrossRef] [PubMed]
- Kumar, P.; Yan, Z.; Xu, L.; Mazza, M.G.; Buldyrev, S.V.; Chen, S.H.; Sastry, S.; Stanley, H.E. Glass transition in biomolecules and the liquid-liquid critical point of water. Phys. Rev. Lett. 2006, 97, 177802. [Google Scholar] [CrossRef] [PubMed]
- Amann-Winkel, K.; Boehmer, R.; Fujara, F.; Gainaru, C.; Geil, B.; Loerting, T. Colloquium: Water’s controversial glass transitions. Rev. Mod. Phys. 2016, 88, 011002. [Google Scholar] [CrossRef]
- Laage, D.; Elsaesser, T.; Hynes, J.T. Water Dynamics in the Hydration Shells of Biomolecules. Chem. Rev. 2017. [Google Scholar] [CrossRef] [PubMed]
- Gallo, P.; Rovere, M.; Chen, S.H. Anomalous dynamics of water confined in MCM-41 at different hydrations. J. Phys. Condens. Matter 2010, 22, 284102. [Google Scholar] [CrossRef] [PubMed]
- Gallo, P.; Rovere, M.; Chen, S.H. Water confined in MCM-41: A mode coupling theory analysis. J. Phys. Condens. Matter 2012, 24, 064109. [Google Scholar] [CrossRef] [PubMed][Green Version]
- De Marzio, M.; Camisasca, G.; Conde, M.M.; Rovere, M.; Gallo, P. Structural properties and fragile to strong transition in confined water. J. Chem. Phys 2016, 146, 084505. [Google Scholar] [CrossRef] [PubMed]
- Gallo, P.; Ricci, M.; Rovere, M. Layer analysis of the structure of water confined in vycor glass. J. Chem. Phys. 2002, 116, 342–346. [Google Scholar] [CrossRef]
- Rosenfeld, Y. A quasi-universal scaling law for atomic transport in simple fluids. J. Phys. Condens. Matter 1999, 11, 5415–5427. [Google Scholar] [CrossRef]
- Jabes, B.S.; Agarwal, M.; Chakravarty, C. Tetrahedral order, pair correlation entropy, and waterlike liquid state anomalies: Comparison of GeO2 with BeF2, SiO2, and H2O. J. Chem. Phys. 2010, 132, 234507. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, M.; Singh, M.; Sharma, R.; Alam, M.P.; Chakravarty, C. Relationship between Structure, Entropy, and Diffusivity in Water and Water-Like Liquids. J. Phys. Chem. B 2010, 114, 6995–7001. [Google Scholar] [CrossRef] [PubMed]
- Sharma, R.; Agarwal, M.; Chakravarty, C. Estimating the entropy of liquids from atom–atom radial distribution functions: Silica, beryllium fluoride and water. Mol. Phys. 2008, 106, 1925–1938. [Google Scholar] [CrossRef]
- Mittal, J.; Errington, J.R.; Truskett, T.M. Relationship between thermodynamics and dynamics of supercooled liquids. J. Chem. Phys. 2006, 125, 076102. [Google Scholar] [CrossRef] [PubMed]
- Errington, J.R.; Truskett, T.M.; Mittal, J. Excess-entropy-based anomalies for a waterlike fluid. J. Chem. Phys. 2006, 125, 244502. [Google Scholar] [CrossRef] [PubMed]
- Scala, A.; Starr, F.W.; Nave, E.L.; Sciortino, F.; Stanley, H.E. Configurational entropy and diffusivity of supercooled water. Nature 2000, 406, 166–169. [Google Scholar] [PubMed]
- Fomin, Y.D.; Ryzhov, V.N.; Gribova, N.V. Breakdown of excess entropy scaling for systems with thermodynamic anomalies. Phys. Rev. E 2010, 81, 061201. [Google Scholar] [CrossRef] [PubMed]
- Johnson, M.E.; Head-Gordon, T. Assessing thermodynamic-dynamic relationships for waterlike liquids. J. Chem. Phys. 2009, 130, 214510. [Google Scholar] [CrossRef] [PubMed]
- Nandi, M.K.; Banerjee, A.; Sengupta, S.; Sastry, S.; Bhattacharyya, S.M. Unraveling the success and failure of mode coupling theory from consideration of entropy. J. Chem. Phys. 2015, 143, 174504. [Google Scholar] [CrossRef] [PubMed]
- Gallo, P.; Rovere, M. Relation between the two-body entropy and the relaxation time in supercooled water. Phys. Rev. E 2015, 91, 012107. [Google Scholar] [CrossRef] [PubMed]
- Corradini, D.; Strekalova, E.G.; Stanley, H.E.; Gallo, P. Microscopic mechanism of protein cryopreservation in an aqueous solution with trehalose. Sci. Rep. 2013, 3, 1218. [Google Scholar] [CrossRef] [PubMed]
- Camisasca, G.; De Marzio, M.; Corradini, D.; Gallo, P. Two structural relaxations in protein hydration water and their dynamic crossovers. J. Chem. Phys. 2016, 145, 044503. [Google Scholar] [CrossRef] [PubMed]
- Magno, A.; Gallo, P. Understanding the Mechanisms of Bioprotection: A Comparative Study of Aqueous Solutions of Trehalose and Maltose upon Supercooling. J. Phys. Chem. Lett. 2011, 2, 977–982. [Google Scholar] [CrossRef]
- Comez, L.; Lupi, L.; Morresi, A.; Paolantoni, M.; Sassi, P.; Fioretto, D. More Is Different: Experimental Results on the Effect of Biomolecules on the Dynamics of Hydration Water. J. Phys. Chem. Lett. 2013, 4, 1188–1192. [Google Scholar] [CrossRef] [PubMed]
- Paolantoni, M.; Comez, L.; Gallina, M.E.; Sassi, P.; Scarponi, F.; Fioretto, D.; Morresi, A. Light scattering spectra of water in trehalose aqueous solutions: Evidence for two different solvent relaxation processes. J. Phys. Chem. B 2009, 113, 7874–7878. [Google Scholar] [CrossRef] [PubMed]
- Starr, F.W.; Harrington, S.; Sciortino, F.; Stanley, H.E. Slow Dynamics of Water under Pressure. Phys. Rev. Lett. 1999, 82, 3629–3632. [Google Scholar] [CrossRef]
- Mallamace, F.; Chen, S.H.; Broccio, M.; Corsaro, C.; Crupi, V.; Majolino, D.; Venuti, V.; Baglioni, P.; Fratini, E.; Vannucci, C.; et al. Role of the solvent in the dynamical transitions of proteins: the case of the lysozyme-water system. J. Chem. Phys. 2007, 127, 045104. [Google Scholar] [CrossRef] [PubMed]
- Lagi, M.; Chu, X.; Kim, C.; Mallamace, F.; Baglioni, P.; Chen, S.H. The low-temperature dynamic crossover phenomenon in protein hydration water: Simulations vs. experiments. J. Phys. Chem. B 2008, 112, 1571–1575. [Google Scholar] [CrossRef] [PubMed]
- Spohr, E.; Hartnig, C.; Gallo, P.; Rovere, M. Water in porous glasses. A computer simulation study. J. Mol. Liq. 1999, 80, 165–178. [Google Scholar] [CrossRef]
- Pronk, S.; Páll, S.; Schulz, R.; Larsson, P.; Bjelkmar, P.; Apostolov, R.; Shirts, M.R.; Smith, J.C.; Kasson, P.M.; van der Spoel, D.; et al. GROMACS 4.5: A high-throughput and highly parallel open source molecular simulation toolkit. Bioinformatics 2013, 29, 845–854. [Google Scholar] [CrossRef] [PubMed]
- MacKerell, A.D.; Banavali, N.K. All-atom empirical force field for nucleic acids: II. Application to molecular dynamics simulations of DNA and RNA in solution. J. Comput. Chem. 2000, 21, 105–120. [Google Scholar] [CrossRef]
- MacKerell, A.D.; Feig, M.; Brooks, C.L. Extending the treatment of backbone energetics in protein force fields: limitations of gas-phase quantum mechanics in reproducing protein conformational distributions in molecular dynamics simulations. J. Comput. Chem. 2004, 25, 1400–1415. [Google Scholar] [CrossRef] [PubMed]
- Berendsen, H.J.C.; Grigera, J.R.; Straatsma, T.P. The missing term in effective pair potentials. J. Phys. Chem. 1987, 91, 6269–6271. [Google Scholar] [CrossRef]


© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Camisasca, G.; De Marzio, M.; Rovere, M.; Gallo, P. Slow Dynamics and Structure of Supercooled Water in Confinement. Entropy 2017, 19, 185. https://doi.org/10.3390/e19040185
Camisasca G, De Marzio M, Rovere M, Gallo P. Slow Dynamics and Structure of Supercooled Water in Confinement. Entropy. 2017; 19(4):185. https://doi.org/10.3390/e19040185
Chicago/Turabian StyleCamisasca, Gaia, Margherita De Marzio, Mauro Rovere, and Paola Gallo. 2017. "Slow Dynamics and Structure of Supercooled Water in Confinement" Entropy 19, no. 4: 185. https://doi.org/10.3390/e19040185
APA StyleCamisasca, G., De Marzio, M., Rovere, M., & Gallo, P. (2017). Slow Dynamics and Structure of Supercooled Water in Confinement. Entropy, 19(4), 185. https://doi.org/10.3390/e19040185




