Applications of the Fuzzy Sumudu Transform for the Solution of First Order Fuzzy Differential Equations
Abstract
:1. Introduction
2. Preliminaries
- U is upper semi-continuous,
- U is fuzzy convex, i.e., U(λx + (1 − λ)y) ≥ min{U(x), U(y)} for all x, y ∈ ℝ, λ ∈ [0, 1],
- U is normal, i.e., ∃x0 ∈ ℝ for which U(x0) = 1,
- supp U = {x ∈ ℝ|U(x) > 0} is the support of the U, and its closure, i.e. cl(supp U) is compact.
- is a bounded non-decreasing left continuous function in (0, 1] and right continuous at zero,
- is a bounded non-increasing left continuous function in (0, 1] and right continuous at zero,
- .≤ .
- addition,
- subtraction,
- scalar multiplication,
- D(U ⊕ W, V ⊕ W) = D(U, V), ∀U, V, W ∈ (ℝ),
- D(k ⊙ U, k ⊙ V) = |k|D(U, V), ∀k ∈ ℝ, U, V ∈ (ℝ),
- D(U ⊕ V, W ⊕ E) ≤ D(U, W) + D(V, E), ∀U, V, W, E ∈ (ℝ),
- (D, (ℝ)) is a complete metric space.
- for all h > 0 sufficiently small, there exist f(x0 + h) −H f(x0), f(x0) −H f(x0 − h) and the limits (in the metric D):or
- for all h > 0 sufficiently small, there exist f(x0)−H f(x0 + h), f(x0 − h)−H f(x0) and the limits (in the metric D):(h and −h in the denominators mean and, respectively.)
3. Fuzzy Sumudu Transform
3.1. Duality Properties of the Fuzzy Laplace and Fuzzy Sumudu Transform
3.2. Fundamental Theorems and Properties of the Fuzzy Sumudu Transform
4. Procedure for Solving Fuzzy Differential Equations
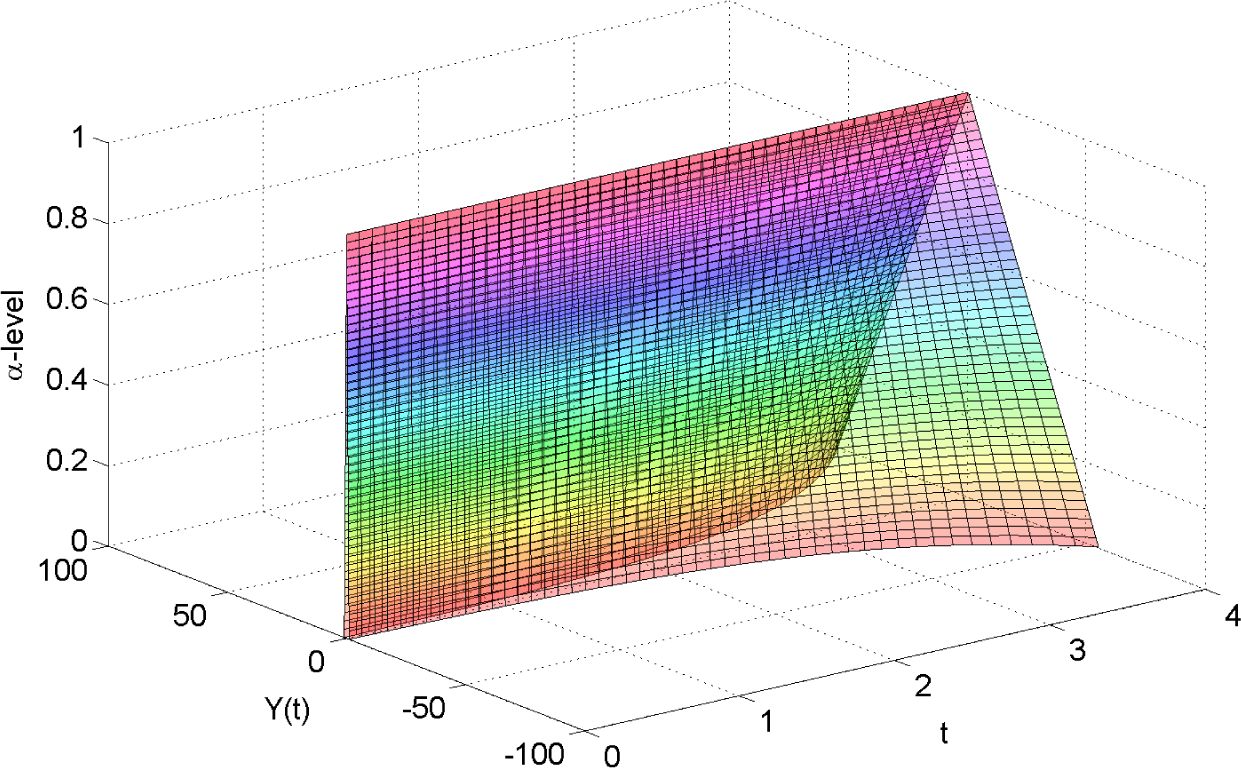
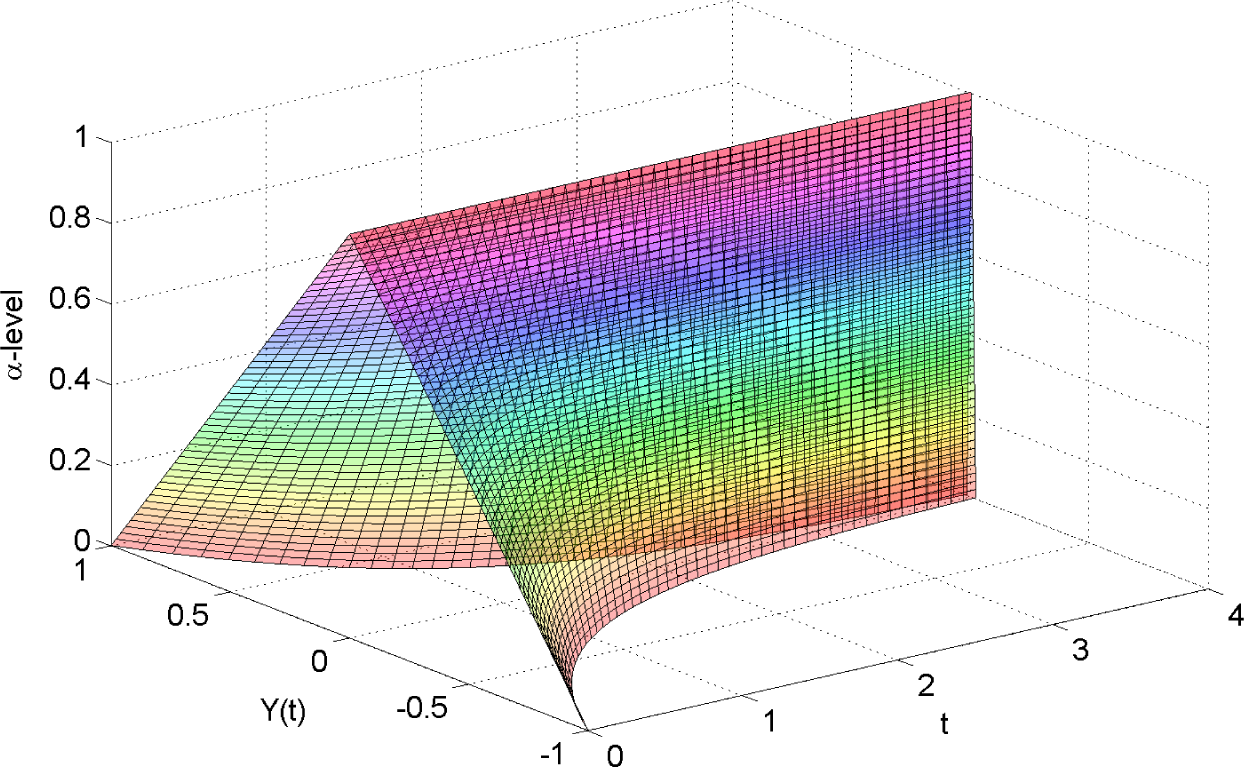
5. A Numerical Example
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Davis, J.A.; McNamara, D.E.; Cottrell, D.M.; Campos, J. Image processing with the radial Hilbert transform: Theory and experiments. Opt. Lett. 2000, 25, 99–101. [Google Scholar]
- Namias, V. The fractional order Fourier transform and its application to quantum mechanics. IMA J. Appl. Math. 1980, 25, 241–265. [Google Scholar]
- Saitoh, S. The Weierstrass transform and an isometry in the heat equation. Appl. Anal. 1983, 16, 1–6. [Google Scholar]
- Ghaemi, F.; Yunus, R.; Ahmadian, A.; Salahshour, S.; Suleiman, M.; Saleh, S.F. Application of Fuzzy Fractional Kinetic Equations to Modelling of the Acid Hydrolysis Reaction. Abstr. Appl. Anal. 2013, 2013, 610314. [Google Scholar]
- Spinelli, R. Numerical inversion of a Laplace transform. SIAM J. Numer. Anal. 1966, 3, 636–649. [Google Scholar]
- Layman, J.W. The Hankel transform and some of its properties. J. Integer Seq. 2001, 4, 1–11. [Google Scholar]
- Tranter, C. The use of the Mellin transform in finding the stress distribution in an infinite wedge. Q. J. Mech. Appl. Math. 1948, 1, 125–130. [Google Scholar]
- Watugala, G.K. Sumudu transforms—A new integral transform to solve differential equations and control engineering problems. Int. J. Math. Educ. Sci. Technol. 1993, 24, 35–43. [Google Scholar]
- Watugala, G.K. Sumudu transforms-a new integral transform to solve differential equations and control engineering problems. Math. Eng. Ind. 1998, 6, 319–329. [Google Scholar]
- Weerakoon, S. Application of Sumudu transform to partial differential equations. Int. J. Math. Educ. Sci. Technol. 1994, 25, 277–283. [Google Scholar]
- Weerakoon, S. Complex inversion formula for Sumudu transform. Int. J. Math. Educ. Sci. Technol. 1998, 29, 618–621. [Google Scholar]
- Asiru, M.A. Sumudu transform and the solution of integral equations of convolution type. Int. J. Math. Educ. Sci. Technol. 2001, 32, 906–910. [Google Scholar]
- Belgacem, F.B.M.; Karaballi, A.A.; Kalla, S.L. Analytical investigations of the Sumudu transform and applications to integral production equations. Math. Probl. Eng. 2003, 2003, 103–118. [Google Scholar]
- Belgacem, F.B.M.; Karaballi, A.A. Sumudu transform fundamental properties investigations and applications. Int. J. Stoch. Anal. 2006, 2006. [Google Scholar] [CrossRef]
- Belgacem, F.B.M. Sumudu transform applications to Bessel functions and equations. Appl. Math. Sci. 2010, 4, 3665–3686. [Google Scholar]
- Agwa, H.A.; Ali, F.M.; Kılıçman, A. A new integral transform on time scales and its application. Adv. Differ. Equ. 2012, 2012. [Google Scholar] [CrossRef]
- Kılıçman, A.; Eltayeb, H.; Ismail, M.R. A note on integral transforms and differential equations. Malays. J. Math. Sci. 2012, 6, 1–18. [Google Scholar]
- Asiru, M.A. Further properties of the Sumudu transform and its application. Int. J. Math. Educ. Sci. Technol. 2002, 33, 441–449. [Google Scholar]
- Eltayeb, H.; Kılıçman, A. A note on the Sumudu transforms and differential equations. Appl. Math. Sci. 2010, 4, 1089–1098. [Google Scholar]
- Belgacem, F.B.M. Introducing and analyzing deeper Sumudu properties. Nonlinear Stud. 2006, 13, 23–41. [Google Scholar]
- Rathore, S.; Kumar, D.; Singh, J.; Gupta, S. Homotopy analysis Sumudu transform method for nonlinear equations. Int. J. Ind. Math. 2012, 4, 301–314. [Google Scholar]
- Zadeh, L.A. Fuzzy sets. Inf. Control. 1965, 8, 338–353. [Google Scholar]
- Chang, S.S.L.; Zadeh, L.A. On fuzzy mapping and control. IEEE Trans. Syst. Man Cybern. 1972, SMC-2, 30–34. [Google Scholar]
- Dubois, D.; Prade, H. Towards fuzzy differential calculus part 1: Integration of fuzzy mappings. Fuzzy Sets Syst. 1982, 8, 1–17. [Google Scholar]
- Dubois, D.; Prade, H. Towards fuzzy differential calculus part 2: Integration on fuzzy intervals. Fuzzy Sets Syst. 1982, 8, 105–116. [Google Scholar]
- Dubois, D.; Prade, H. Towards fuzzy differential calculus part 3: Differentiation. Fuzzy Sets Syst. 1982, 8, 225–233. [Google Scholar]
- Puri, M.L.; Ralescu, D.A. Differentials of fuzzy functions. J. Math. Anal. Appl. 1983, 91, 552–558. [Google Scholar]
- Goetschel, R., Jr.; Voxman, W. Elementary fuzzy calculus. Fuzzy Sets Syst. 1986, 18, 31–43. [Google Scholar]
- Kaleva, O. Fuzzy differential equations. Fuzzy Sets Syst. 1987, 24, 301–317. [Google Scholar]
- Ding, Z.; Ma, M.; Kandel, A. Existence of the solutions of fuzzy differential equations with parameters. Inf. Sci. 1997, 99, 205–217. [Google Scholar]
- Salahshour, S. Nth-order fuzzy differential equations under generalized differentiability. J. Fuzzy Set Valued Anal. 2011, 2011. [Google Scholar] [CrossRef]
- Shahriyar, M.; Ismail, F.; Aghabeigi, S.; Ahmadian, A.; Salahshour, S. An eigenvalue-eigenvector method for solving a system of fractional differential equations with uncertainty. Math. Probl. Eng. 2013, 2013, 579761. [Google Scholar]
- Arshad, S.; Lupulescu, V. On the fractional differential equations with uncertainty. Nonlinear Anal. Theory Methods Appl. 2011, 74, 3685–3693. [Google Scholar]
- Allahviranloo, T.; Salahshour, S.; Abbasbandy, S. Explicit solutions of fractional differential equations with uncertainty. Soft Comput. 2012, 16, 297–302. [Google Scholar]
- Allahviranloo, T.; Ahmadi, M.B. Fuzzy Laplace transforms. Soft Comput. 2010, 14, 235–243. [Google Scholar]
- Salahshour, S.; Allahviranloo, T. Applications of fuzzy Laplace transforms. Soft Comput. 2013, 17, 145–158. [Google Scholar]
- Jafarian, A.; Golmankhaneh, A.K.; Baleanu, D. On fuzzy fractional Laplace transformation. Adv. Math. Phys. 2014, 2014. [Google Scholar] [CrossRef]
- Salahshour, S.; Allahviranloo, T.; Abbasbandy, S. Solving fuzzy fractional differential equations by fuzzy Laplace transforms. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 1372–1381. [Google Scholar]
- Salahshour, S.; Khezerloo, M.; Hajighasemi, S.; Khorasany, M. Solving fuzzy integral equations of the second kind by fuzzy Laplace transform method. Int. J. Ind. Math. 2012, 4, 21–29. [Google Scholar]
- Muhammad Ali, H.F.; Haydar, A.K. On fuzzy Laplace transforms for fuzzy differential equations of the third order. J. Kerbala Univ. 2013, 11, 251–256. [Google Scholar]
- Salahshour, S.; Haghi, E. Solving fuzzy heat equation by fuzzy Laplace transforms, Information Processing and Management of Uncertainty in Knowledge-Based Systems; Springer: Berlin/Heidelberg, Germany, 2010; pp. 512–521.
- Ahmad, M.Z.; Abdul Rahman, N.A. Explicit solution of fuzzy differential equations by mean of fuzzy Sumudu transform. Int. J. Appl. Phys. Math. 2015, 5, 86–93. [Google Scholar]
- Alam Khan, N.; Abdul Razzaq, O.; Ayyaz, M. On the solution of fuzzy differential equations by fuzzy Sumudu transform. Nonlinear Eng. 2015, 4, 49–60. [Google Scholar]
- Xu, J.; Liao, Z.; Hu, Z. A class of linear differential dynamical systems with fuzzy initial condition. Fuzzy Sets Syst. 2007, 158, 2339–2358. [Google Scholar]
- Zimmerman, H.J. Fuzzy Set Theory and Its Applications; Kluwer Academic Publisher and Dordrecht: Dordrecht, The Netherlands, 1991. [Google Scholar]
- Friedman, M.; Ma, M.; Kandel, A. Numerical solutions of fuzzy differential and integral equations. Fuzzy Sets Syst. 1999, 106, 35–48. [Google Scholar]
- Ma, M.; Friedman, M.; Kandel, A. Numerical solutions of fuzzy differential equations. Fuzzy Sets Syst. 1999, 105, 133–138. [Google Scholar]
- Ahmad, M.Z.; de Baets, B. A Predator-Prey Model with Fuzzy Initial Populations. Proceedings of the Joint 2009 International Fuzzy Systems Association World Congress and 2009 European Society of Fuzzy Logic and Technology Conference, Lisbon, Portugal, 20–24 July 2009; pp. 1311–1314.
- Dubois, D.J. Fuzzy Sets and Systems: Theory and Applications; Academic Press: Waltham, MA, USA, 1980. [Google Scholar]
- Wu, H.C. The improper fuzzy Riemann integral and its numerical integration. Inf. Sci. 1998, 111, 109–137. [Google Scholar]
- Wu, H.C. The fuzzy Riemann integral and its numerical integration. Fuzzy Sets Syst. 2000, 110, 1–25. [Google Scholar]
- Bede, B.; Gal, S.G. Generalizations of the differentiability of fuzzy-number-valued functions with applications to fuzzy differential equations. Fuzzy Sets Syst. 2005, 151, 581–599. [Google Scholar]
- Bede, B.; Rudas, I.J.; Bencsik, A.L. First order linear fuzzy differential equations under generalized differentiability. Inf. Sci. 2007, 177, 1648–1662. [Google Scholar]
- Chalco-Cano, Y.; Román-Flores, H. On new solutions of fuzzy differential equations. Chaos Solitons Fractals. 2008, 38, 112–119. [Google Scholar]
- Das, M.; Talukdar, D. Method For Solving Fuzzy Integro-Differential Equation By Using Fuzzy Laplace Transformation. Int. J. Sci. Technol. Res. 2014, 3, 291–295. [Google Scholar]
- Chalco-Cano, Y.; Román-Flores, H. Comparation between some approaches to solve fuzzy differential equations. Fuzzy Sets Syst. 2009, 160, 1517–1527. [Google Scholar]
- Kaleva, O. A note on fuzzy differential equations. Nonlinear Anal. Theory Methods Appl. 2006, 64, 895–900. [Google Scholar]
© 2015 by the authors; licensee MDPI, Basel, Switzerland This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahman, N.A.A.; Ahmad, M.Z. Applications of the Fuzzy Sumudu Transform for the Solution of First Order Fuzzy Differential Equations. Entropy 2015, 17, 4582-4601. https://doi.org/10.3390/e17074582
Rahman NAA, Ahmad MZ. Applications of the Fuzzy Sumudu Transform for the Solution of First Order Fuzzy Differential Equations. Entropy. 2015; 17(7):4582-4601. https://doi.org/10.3390/e17074582
Chicago/Turabian StyleRahman, Norazrizal Aswad Abdul, and Muhammad Zaini Ahmad. 2015. "Applications of the Fuzzy Sumudu Transform for the Solution of First Order Fuzzy Differential Equations" Entropy 17, no. 7: 4582-4601. https://doi.org/10.3390/e17074582
APA StyleRahman, N. A. A., & Ahmad, M. Z. (2015). Applications of the Fuzzy Sumudu Transform for the Solution of First Order Fuzzy Differential Equations. Entropy, 17(7), 4582-4601. https://doi.org/10.3390/e17074582




