Modeling Soil Moisture Profiles in Irrigated Fields by the Principle of Maximum Entropy
Abstract
:1. Introduction
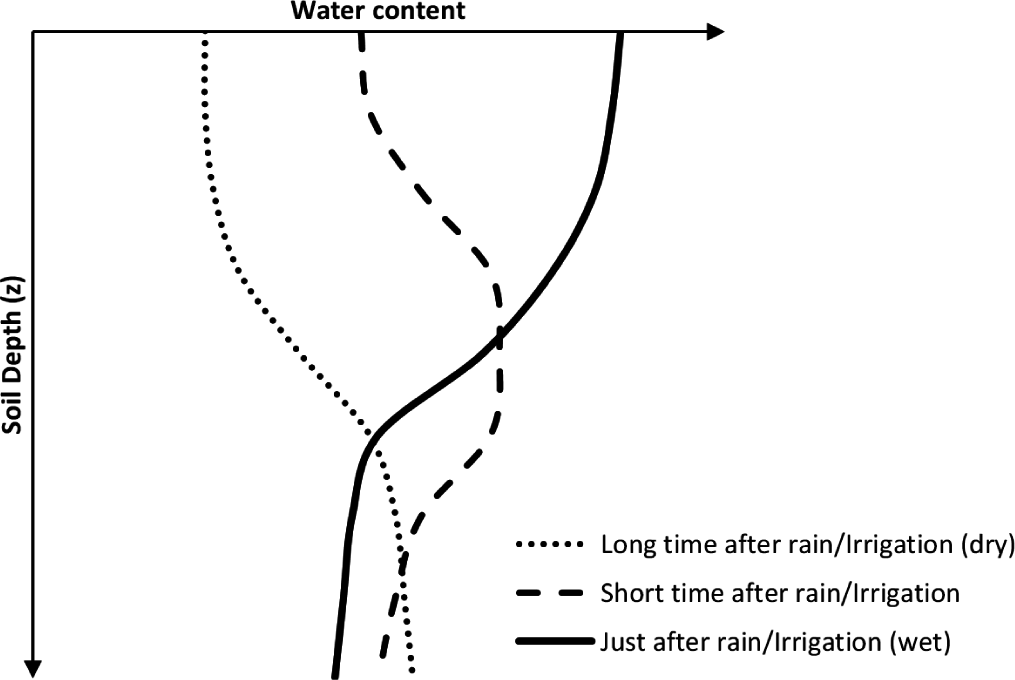
2. Soil Moisture States and Irrigation
3. Entropy Applied to Soil Moisture States
4. Soil Moisture Profile Development Models
4.1. Development of Soil Moisture Profiles from POME
4.2. Physically-Based Soil Moisture Model
4.3. Temporal Evolution of Soil Moisture Profiles by POME
5. Study Area
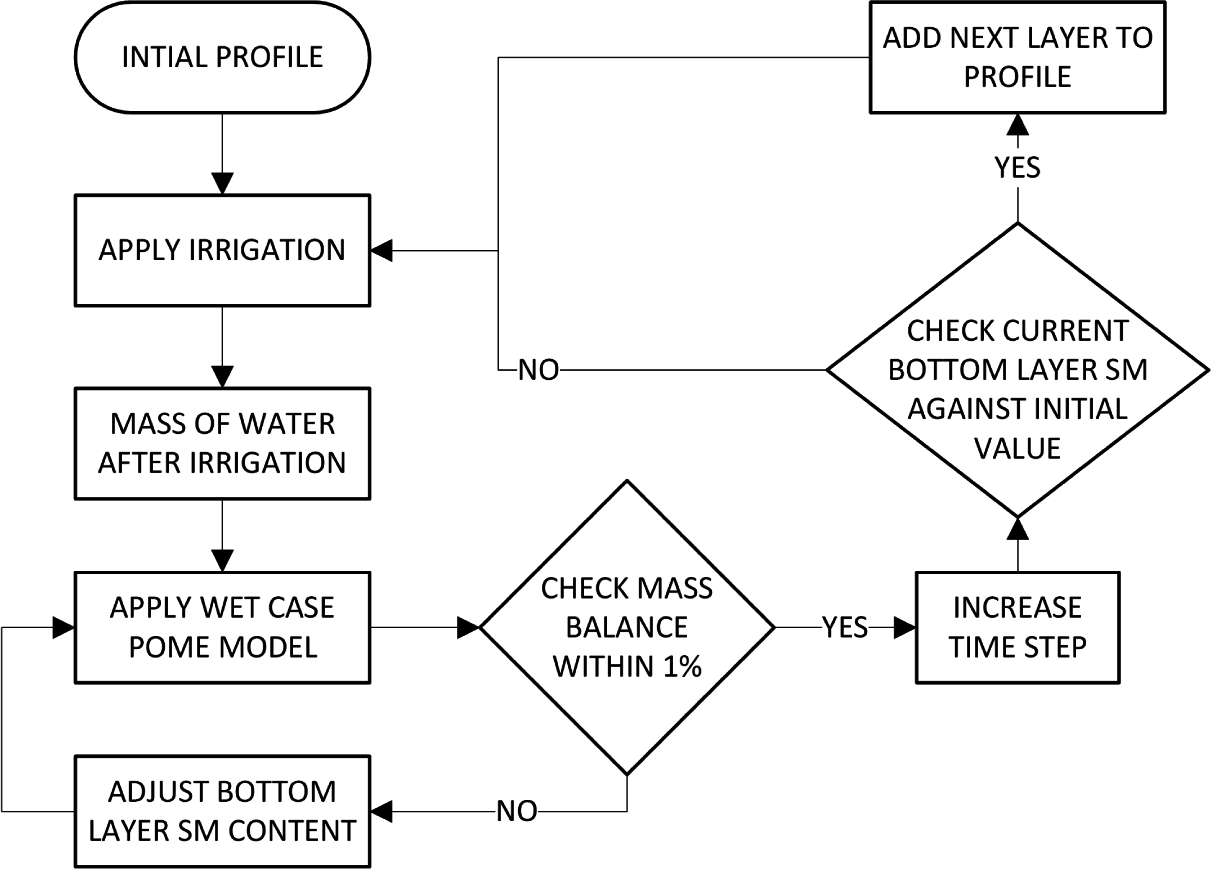
6. Methodology
- Verification of the entropy soil moisture profiles with all known input parameters for all cases shown in Figure 1 using observed data from the USDA SCAN site located beside the field.
- Verification of the entropy soil moisture profiles with derived input parameters at the SCAN site.
- Application of the entropy method to simulate a complete irrigation cycle of the center pivot on the field and comparison with a physical model.
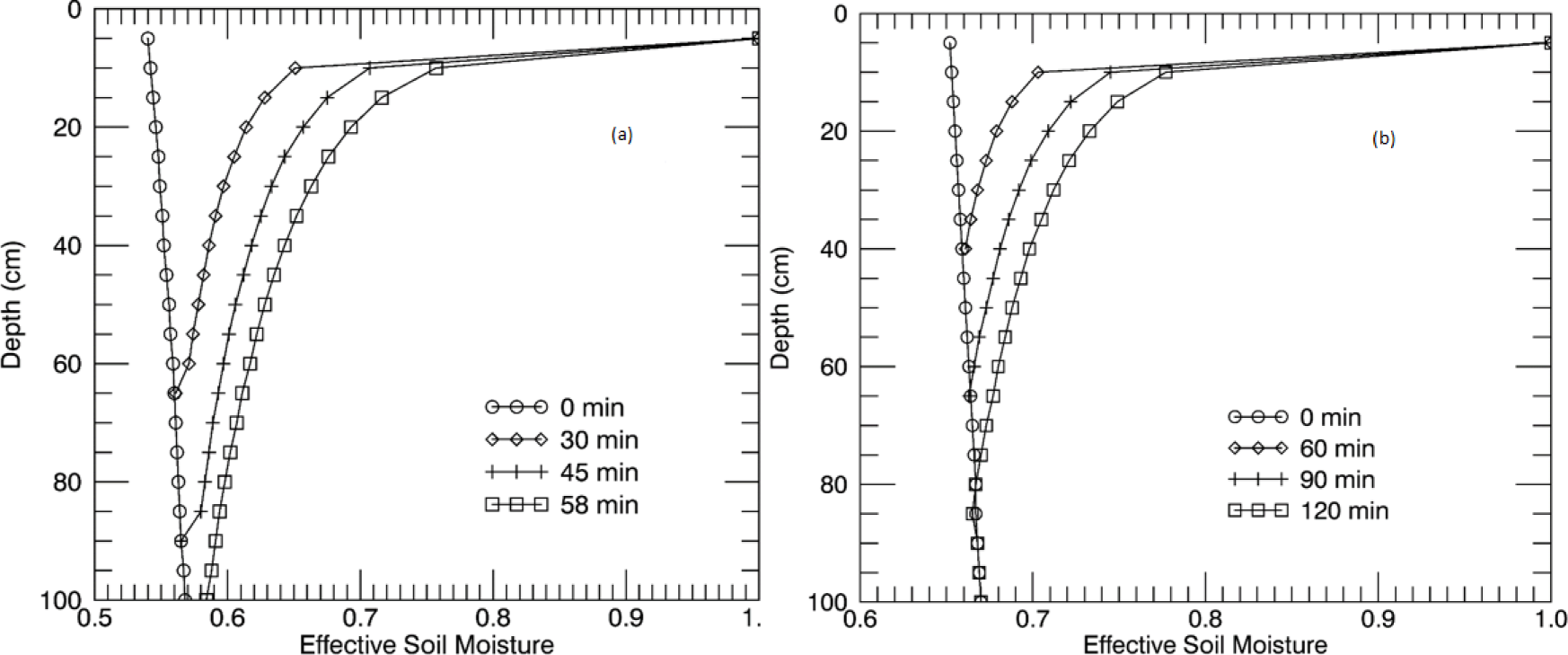
6.1. Irrigation Phase Simulation
6.2. Drying Phase Simulation
7. Results and Discussions
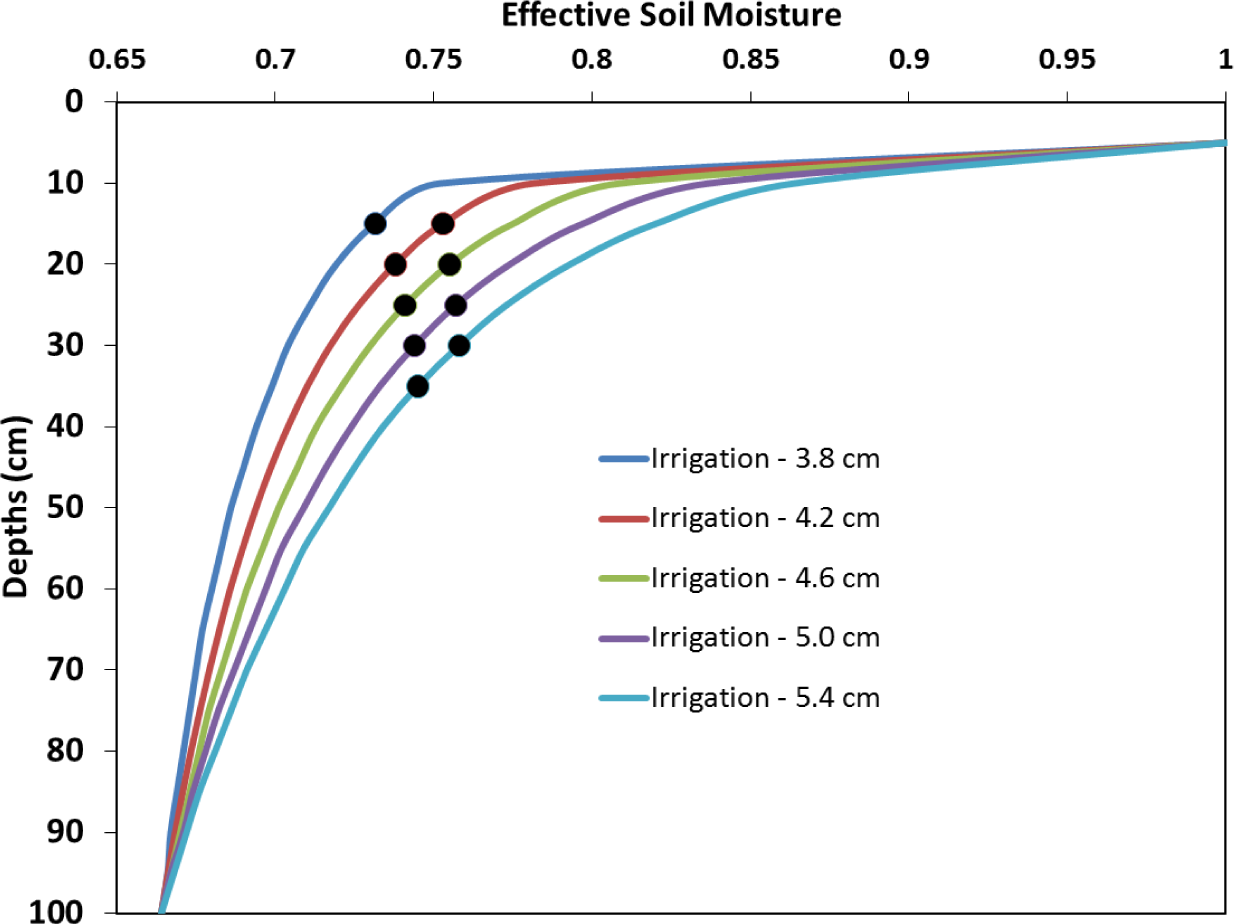
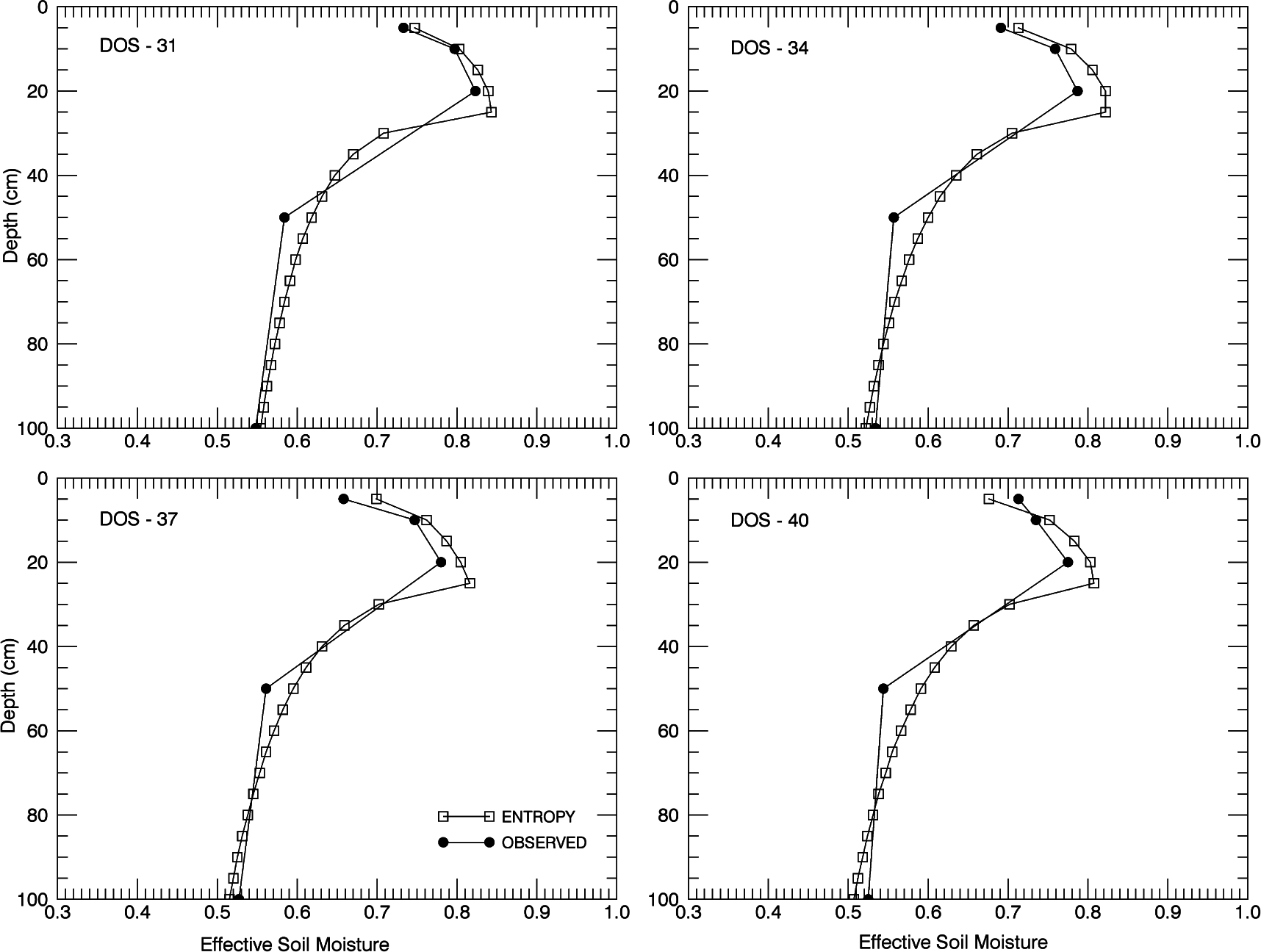
7.1. Verification of Entropy Profiles
7.1.1. SCAN Site Verification
7.1.2. Entropy Validation with Derived Parameters
7.2. Application of the POME Model to an Irrigation Cycle
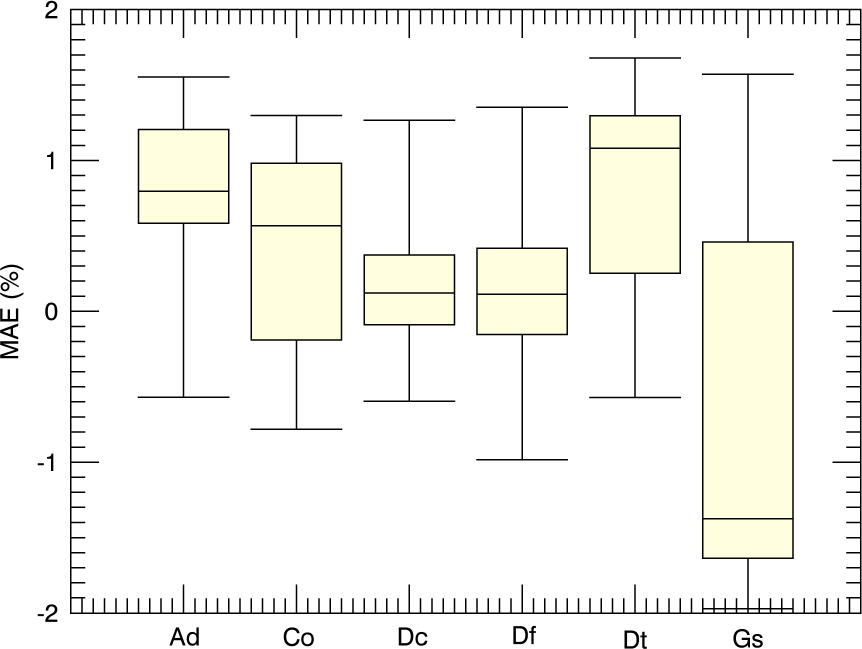
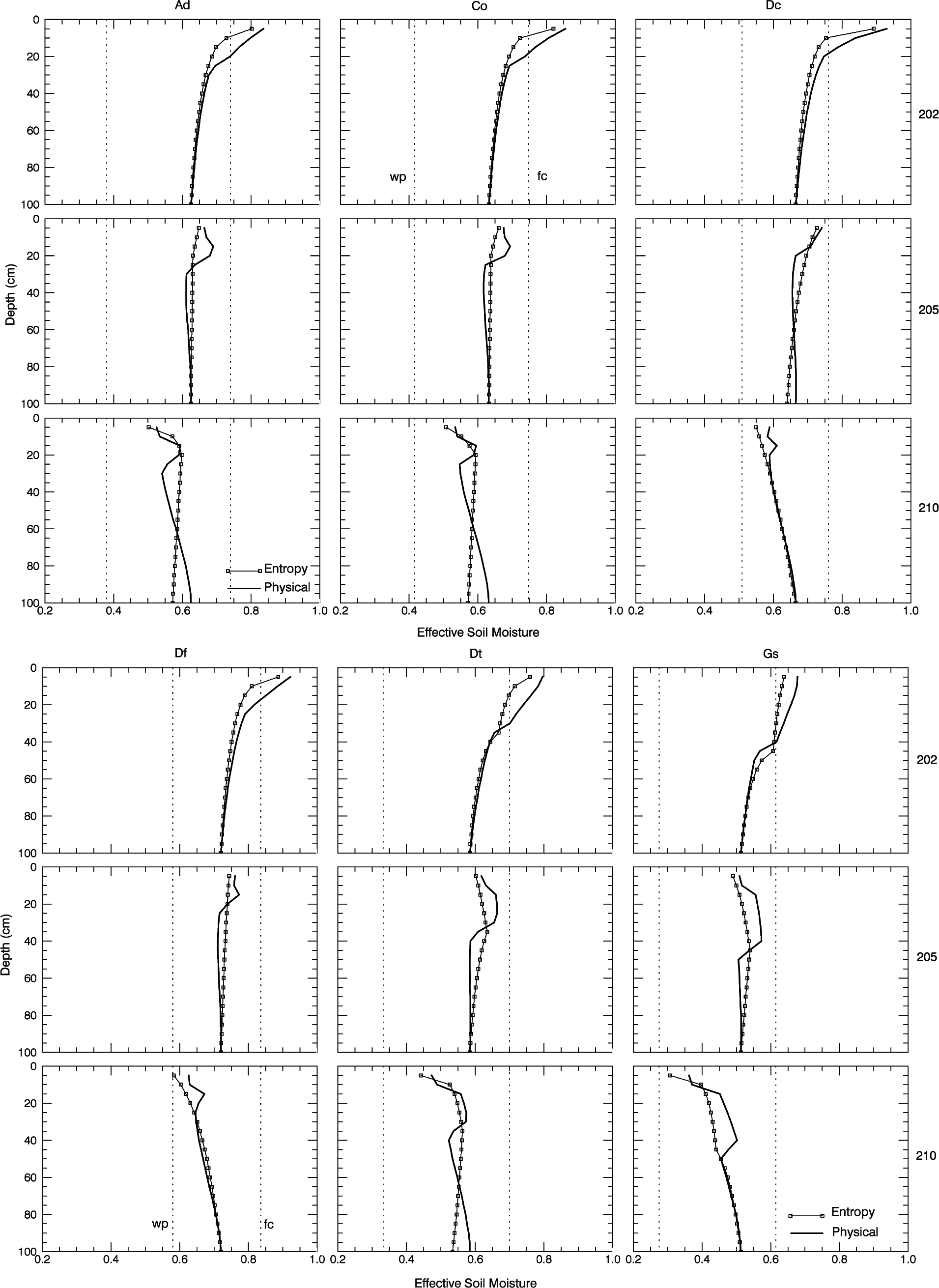
7.3. Comparison to Physically Based Model
8. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix
References
- Burt, C.; Clemmens, A.; Strelkoff, T.; Solomon, K.; Bliesner, R.; Hardy, L.; Howell, T.; Eisenhauer, D. Irrigation Performance Measures: Efficiency and Uniformity. J. Irrig. Drain. Eng. 1997, 123, 423–442. [Google Scholar]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop Evapotranspiration—Guidelines for Computing Crop Water Requirements—FAO Irrigation and Drainage Paper 56; FAO: Rome, Italy, 1998; Volume 300, p. 6541. [Google Scholar]
- Fereres, E.; Connor, D. Sustainable water management in agriculture. In Challenges of the New Water Policies for the XXI Century, Proceedings of the Seminar on Challenges of the New Water Policies for the 21st Century, Valencia, Spain, 29–31 October 2002; p. 164.
- Ozdogan, M.; Gutman, G. A new methodology to map irrigated areas using multi-temporal MODIS and ancillary data: An application example in the continental US. Remote Sens. Environ. 2008, 112, 3520–3537. [Google Scholar]
- Schaible, G.D.; Aillery, M.P. Water Conservation in Irrigated Agriculture: Trends and Challenges in the Face of Emerging Demands; US Department of Agriculture, Economic Research Service: Washington, DC, USA, 2012. [Google Scholar]
- Kenny, J.F.; Barber, N.L.; Hutson, S.S.; Linsey, K.S.; Lovelace, J.K.; Maupin, M.A. Estimated Use of Water in the United States in 2005; US Geological Survey: Reston, VA, USA, 2009. [Google Scholar]
- Fereres, E.; Soriano, M.A. Deficit irrigation for reducing agricultural water use. J. Exp. Bot. 2007, 58, 147–159. [Google Scholar]
- Zhang, H.; Oweis, T.Y.; Garabet, S.; Pala, M. Water-use efficiency and transpiration efficiency of wheat under rain-fed conditions and supplemental irrigation in a Mediterranean-type environment. Plant Soil 1998, 201, 295–305. [Google Scholar]
- Sammis, T.; Sharma, P.; Shukla, M.; Wang, J.; Miller, D. A water-balance drip-irrigation scheduling model. Agric. Water Manag. 2012, 113, 30–37. [Google Scholar]
- Starks, P.J.; Heathman, G.C.; Ahuja, L.R.; Ma, L. Use of limited soil property data and modeling to estimate root zone soil water content. J. Hydrol. 2003, 272, 131–147. [Google Scholar]
- Scott, C.A.; Bastiaanssen, W.G.M.; Ahmad, M.U.D. Mapping Root Zone Soil Moisture Using Remotely Sensed Optical Imagery. J. Irrig. Drain. Eng. 2003, 129, 326–335. [Google Scholar]
- Kondratyev, K.Y.; Melentyev, V.V.; Rabinovich, Y.I.; Shulgina, E.M. Passive Microwave Remote Sensing of Soil Moisture. Proceedings of the 11th International Symposium on Remote Sensing Environment, University of Michigan, Ann Arbor, MI, USA, 25–29 April 1977.
- Arya, L.M.; Richter, J.C.; Paris, J.F. Estimating profile water storage from surface zone soil moisture measurements under bare field conditions. Water Resour. Res. 1983, 19, 403–412. [Google Scholar]
- Srivastava, S.; Yograjan, N.; Jayaraman, V.; Rao, P.; Chandrasekhar, M. On the relationship between ERS-1 SAR/backscatter and surface/sub-surface soil moisture variations in vertisols. Acta Astronaut. 1997, 40, 693–699. [Google Scholar]
- Kostov, K.G.; Jackson, T.J. Estimating profile soil moisture from surface-layer measurements: A review. Proc. SPIE 1993, 1941, 125–136. [Google Scholar]
- Jackson, T.J. Profile soil moisture from surface measurements. J. Irrig. Drain. Div. Am. Soc. Civil Eng. 1980, 106, 81–92. [Google Scholar]
- Reutov, E.; Shutko, A. Prior-knowledge based- soil moisture determination by microwave radiometry. Soviet J. Remote Sens. 1986, 5, 100–125. [Google Scholar]
- Singh, V. Hydrologic Systems: Watershed Modeling; Prentice Hall: Englewood, NJ, USA, 1988; Volume 2. [Google Scholar]
- De Troch, F.; Troch, P.; Su, Z.; Lin, D. Application of remote sensing for hydrological modelling. In Distributed Hydrological Modelling; Springer: Dordrecht, The Netherlands, 1996; pp. 165–191. [Google Scholar]
- Assouline, S.; Tessier, D.; Bruand, A. A conceptual model of the soil water retention curve. Water Resour. Res. 1998, 34, 223–231. [Google Scholar]
- Pachepsky, Y.; Guber, A.; Jacques, D.; Simunek, J.; Van Genuchten, M.T.; Nicholson, T.; Cady, R. Information content and complexity of simulated soil water fluxes. Geoderma 2006, 134, 253–266. [Google Scholar]
- Al-Hamdan, O.Z.; Cruise, J.F. Soil Moisture Profile Development from Surface Observations by Principle of Maximum Entropy. J. Hydrol. Eng. 2010, 15, 327–337. [Google Scholar]
- Koekkoek, E.; Booltink, H. Neural network models to predict soil water retention. Eur. J. Soil Sci. 1999, 50, 489–495. [Google Scholar]
- Kornelsen, K.C.; Coulibaly, P. Root-zone soil moisture estimation using data-driven methods. Water Resour. Res. 2014, 50, 2946–2962. [Google Scholar]
- Huang, C.; Li, X.; Lu, L.; Gu, J. Experiments of one-dimensional soil moisture assimilation system based on ensemble Kalman filter. Remote Sens. Environ. 2008, 112, 888–900. [Google Scholar]
- Moore, R.J. The probability-distributed principle and runoff production at point and basin scales. Hydrol. Sci. J. 1985, 30, 273–297. [Google Scholar]
- Mays, D.C.; Faybishenko, B.A.; Finsterle, S. Information entropy to measure temporal and spatial complexity of unsaturated flow in heterogeneous media. Water Resour. Res. 2002, 38, 49.1–49.11. [Google Scholar]
- Singh, V.P. Entropy theory for derivation of infiltration equations. Water Resour. Res. 2010, 46. [Google Scholar] [CrossRef]
- Pan, F.; Pachepsky, Y.A.; Guber, A.K.; Hill, R.L. Information and complexity measures applied to observed and simulated soil moisture time series. Hydrol. Sci. J. 2011, 56, 1027–1039. [Google Scholar]
- Mishra, V.; Cruise, J.F.; Mecikalski, J.R.; Hain, C.R.; Anderson, M.C. A remote-sensing driven tool for estimating crop stress and yields. Remote Sens. 2013, 5, 3331–3356. [Google Scholar]
- Bras, R.L. Hydrology: An Introduction to Hydrologic Science; Addison-Wesley: Reading, MA, USA, 1990. [Google Scholar]
- Singh, V.P. Elementary Hydrology; Pearson College Division: Engelwood Cliffs, NJ, USA, 1992. [Google Scholar]
- Clapp, R.B.; Hornberger, G.M. Empirical equations for some soil hydraulic properties. Water Resour. Res. 1978, 14, 601–604. [Google Scholar]
- Heber Green, W.; Ampt, G.A. Studies on Soil Phyics. J. Agric. Sci. 1911, 4, 1–24. [Google Scholar]
- Kostiakov, A.N. On the dynamics of the coefficient of water-percolation in soils and on the necessity for studying it from a dynamic point of view for purposes of amelioration. Proceedings of the Transactions of 6th Congress of International Soil Science Society, Moscow, Russia; 1932; pp. 17–21. [Google Scholar]
- Horton, R.E. The Interpretation Application of Runoff Plat Experiments with Reference to Soil Erosion Problems. Soil Sci. Soc. Am. J. 1939, 3, 340. [Google Scholar]
- Holtan, H. A Concept for Infiltration Estimates in Watershed Engineering; USDA-ARS Bulletin 41–51; Washington, DC, USA, 1961. [Google Scholar]
- Singh, V. The use of entropy in hydrology and water resources. Hydrol. Process. 1997, 11, 587–626. [Google Scholar]
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar]
- Jaynes, E.T. Information theory and statistical mechanics-1. Phys. Rev. 1957, 106, 620–630. [Google Scholar]
- Jaynes, E.T. Information Theory Statistical Mechanics-II. Phys. Rev. 1957, 108, 171–190. [Google Scholar]
- Barbe, D.E.; Cruise, J.F.; Singh, V.P. Solution of Three Constraint Entropy Based Velocity Distribution. J. Hydraul. Eng. 1991, 117, 1389–1396. [Google Scholar]
- Singh, V.P. Hydrologic synthesis using entropy theory: Review. J. Hydrol. Eng. 2011, 16, 421–433. [Google Scholar]
- Chiu, C.L. Entropy and Probability Concepts in Hydraulics. J. Hydraul. Eng. 1987, 113, 583–599. [Google Scholar]
- Yadav, B.K.; Mathur, S.; Siebel, M.A. Soil moisture dynamics modeling considering the root compensation mechanism for water uptake by plants. J. Hydrol. Eng. 2009, 14, 913–922. [Google Scholar]
- Neitsch, S.L.; Arnold, J.G.; Kiniry, J.R.; Williams, J.R. SWAT Theoretical Documentation Version 2009; Technical Report Technical Report 406; Texas Water Resources Institute: College Station, TX, USA, 2011. [Google Scholar]
- Feddes, R.A.; Kowalik, P.J.; Zaradny, H.; others. Simulation of Field Water Use and Crop Yield; Centre for Agricultural Publishing and Documentation: Wageningen, The Netherlands, 1978. [Google Scholar]
- Paudel, K.P.; Limaye, A.S.; Hatch, L.U.; Cruise, J.F.; Musleh, F. Development of an optimal water allocation decision tool for the major crops during the water deficit period in the southeast United States. Nat. Resour. Model. 2005, 18, 281–306. [Google Scholar]
- Nash, J.; Sutcliffe, J. River flow forecasting through conceptual models part I—A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar]
- ASCE Task Committee Defination of Criteria for Evaluation of Watershed Models. J. Irrig. Drain. Eng. 1993, 119, 429–442.
- Poulovassilis, A.; Anadranistakis, M.; Liakatas, A.; Alexandris, S.; Kerkides, P. Semi-empirical approach for estimating actual evapotranspiration in Greece. Agric. Water Manag. 2001, 51, 143–152. [Google Scholar]
- Wu, J.; Zhang, R.; Gui, S. Modeling soil water movement with water uptake by roots. Plant Soil 1999, 215, 7–17. [Google Scholar]
- Çakir, R. Effect of water stress at different development stages on vegetative and reproductive growth of corn. Field Crops Res. 2004, 89, 1–16. [Google Scholar]
- Luo, Y.; He, C.; Sophocleous, M.; Yin, Z.; Hongrui, R.; Ouyang, Z. Assessment of crop growth and soil water modules in SWAT2000 using extensive field experiment data in an irrigation district of the Yellow River Basin. J. Hydrol. 2008, 352, 139–156. [Google Scholar]
- Taylor, T.; Ravet, F. A Meteorologically Driven Maize Stress Indicator Model; AgRISTARS EW/CCA Rep. EW-U1-04119, JSC-17399; Lyndon B. Johnson Space Center: Houston, TX, USA, 1981. [Google Scholar]
- Veenhof, D.; McBride, R. A preliminary performance evaluation of a soil water balance model (SWATRE) on corn producing croplands in the RM of Haldimand-Norfolk. In Soil Compaction Susceptibility and Compaction Risk Assessment for Corn Production; Centre for Land and Biological Resources Research AAFC: Ottawa, ON, USA, 1994; pp. 112–142. [Google Scholar]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar]
- Perrochet, P. Water uptake by plant roots—A simulation model, I. Conceptual model. J. Hydrol. 1987, 95, 55–61. [Google Scholar]
- Upchurch, D.; Ritchie, J. Battery-operated color video camera for root observations in mini-rhizotrons. Agron. J. 1984, 76, 1015–1017. [Google Scholar]
- Merrill, S.; Doering, E.; Reichman, G. Application of a minirhizotron with flexible, pressurized walls to a study of corn root growth. In Minirhizotron Observation Tubes: Methods and Applications for Measuring Rhizosphere Dynamics; American Society of Agronomy: Madison, WI, USA, 1987; pp. 131–143. [Google Scholar]
- Li, K.; De Jong, R.; Boisvert, J. An exponential root-water-uptake model with water stress compensation. J. Hydrol. 2001, 252, 189–204. [Google Scholar]
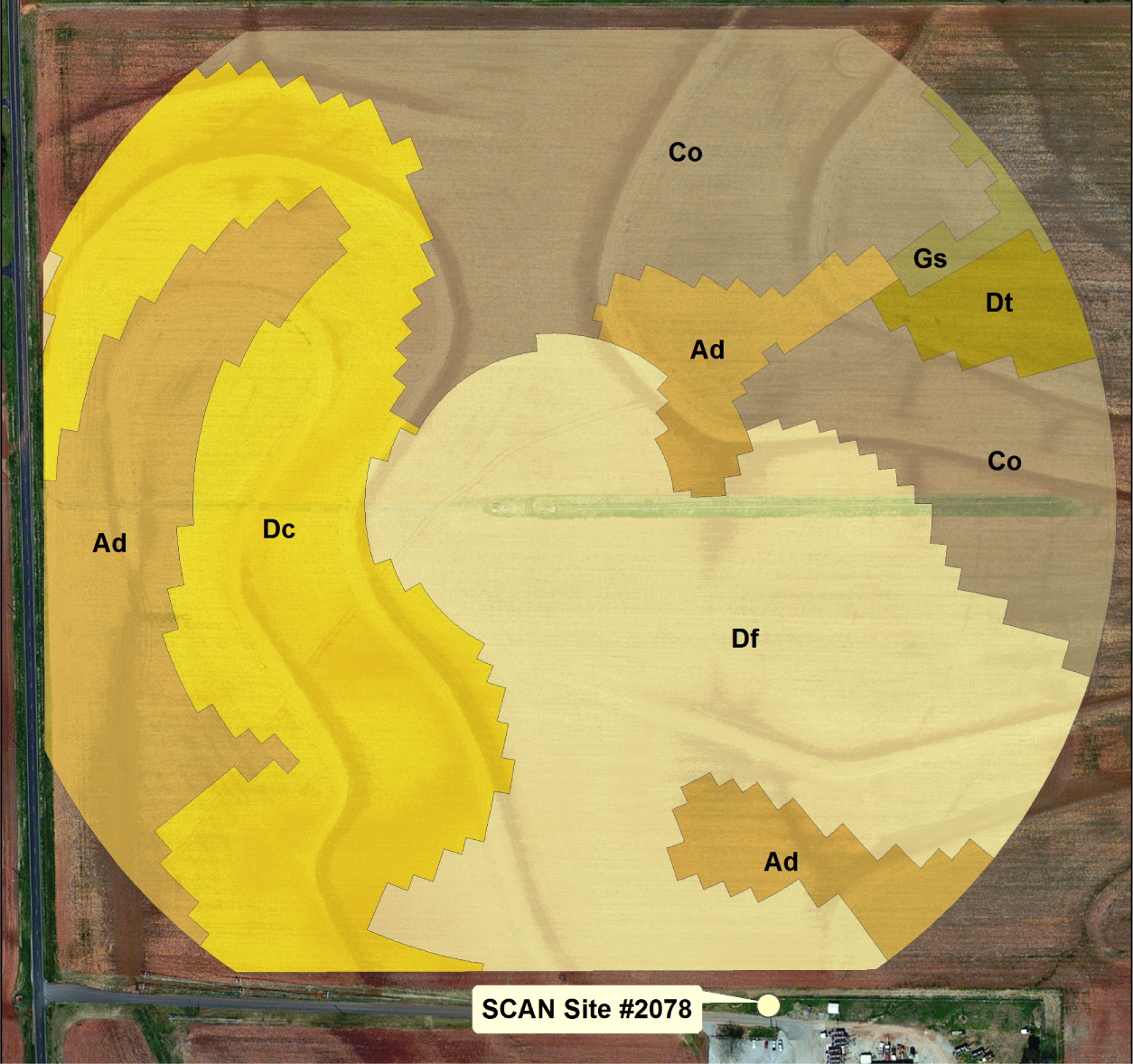





| Soil Type | Sand% | Clay% | θwp (cm3/cm3) | θfc (cm3/cm3) | θsat (cm3/cm3) | Ksat (mm/h) | Bulk Density (gm/cm3) |
|---|---|---|---|---|---|---|---|
| Ad (SIL) | 08.5 | 33.3 | 0.204 | 0.379 | 0.512 | 6.30 | 1.29 |
| Co (SIL) | 17.9 | 35.1 | 0.216 | 0.372 | 0.498 | 5.11 | 1.33 |
| Dt (SIL) | 18.4 | 26.7 | 0.174 | 0.347 | 0.490 | 8.11 | 1.35 |
| Gs (SIL) | 40.8 | 20.6 | 0.137 | 0.288 | 0.459 | 15.49 | 1.43 |
| Df (SICL) | 09.5 | 53.3 | 0.310 | 0.432 | 0.534 | 2.52 | 1.24 |
| Dc (SIC) | 18.4 | 47.6 | 0.286 | 0.414 | 0.507 | 1.92 | 1.31 |
| SCAN | 08.7 | 48.8 | 0.287 | 0.426 | 0.508 | 1.41 | 1.30 |
| SCAN Site 2078
| |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Time/day | 10 cm
| 20 cm
| 50 cm
| ||||||||
| Xobs | Xm | e (%) | Xobs | Xm | e (%) | Xobs | Xm | e (%) | |||
| Wet Case (50-cm Depth) | 16:00 | 0.722 | 0.757 | 4.75 | 0.730 | 0.722 | −1.02 | – | – | – | 0.56 |
| 17:00 | 0.826 | 0.817 | −1.14 | 0.730 | 0.747 | 2.32 | – | – | – | 0.97 | |
| 18:00 | 0.896 | 0.838 | −6.47 | 0.735 | 0.768 | 4.57 | – | – | – | 0.75 | |
| 19:00 | 0.898 | 0.842 | −6.19 | 0.764 | 0.790 | 3.28 | – | – | – | 0.81 | |
| 20:00 | 0.896 | 0.853 | −4.74 | 0.787 | 0.803 | 2.08 | – | – | – | 0.91 | |
| 21:00 | 0.935 | 0.910 | −2.72 | 0.863 | 0.859 | −0.47 | – | – | – | 0.98 | |
| 22:00 | 0.935 | 0.897 | −4.08 | 0.891 | 0.865 | −2.82 | – | – | – | 0.94 | |
| 23:00 | 0.928 | 0.886 | −4.48 | 0.886 | 0.858 | −3.13 | – | – | – | 0.92 | |
| 00:00 | 0.923 | 0.875 | −5.13 | 0.881 | 0.851 | −3.39 | – | – | – | 0.89 | |
| 01:00 | 0.923 | 0.867 | −6.02 | 0.878 | 0.847 | −3.60 | – | – | – | 0.85 | |
| 02:00 | 0.920 | 0.861 | −6.48 | 0.876 | 0.842 | −3.87 | – | – | – | 0.81 | |
| Mean e | −3.88 | −0.55 | 0.86 | ||||||||
| MAE (%) | 4.74 | 2.78 | – | ||||||||
| Dry Case (100-cm Depth) | 88 | 0.582 | 0.601 | 3.26 | 0.653 | 0.632 | −3.16 | 0.665 | 0.665 | 0.0 | 0.87 |
| 89 | 0.562 | 0.583 | 3.88 | 0.636 | 0.619 | −2.67 | 0.658 | 0.655 | −0.42 | 0.91 | |
| 90 | 0.549 | 0.558 | 1.58 | 0.616 | 0.602 | −2.26 | 0.653 | 0.651 | −0.34 | 0.98 | |
| 91 | 0.549 | 0.566 | 3.05 | 0.621 | 0.606 | −2.33 | 0.653 | 0.650 | −0.48 | 0.96 | |
| 92 | 0.547 | 0.555 | 1.48 | 0.616 | 0.597 | −3.11 | 0.643 | 0.644 | 0.16 | 0.97 | |
| 93 | 0.539 | 0.542 | 0.47 | 0.609 | 0.586 | −3.68 | 0.636 | 0.639 | 0.55 | 0.97 | |
| 94 | 0.537 | 0.535 | −0.41 | 0.604 | 0.582 | −3.71 | 0.634 | 0.637 | 0.61 | 0.97 | |
| 95 | 0.532 | 0.524 | −1.55 | 0.591 | 0.570 | −3.56 | 0.626 | 0.630 | 0.70 | 0.98 | |
| 96 | 0.524 | 0.512 | −2.66 | 0.586 | 0.565 | −3.68 | 0.614 | 0.623 | 1.55 | 0.96 | |
| Mean e | 1.01 | −3.13 | 0.26 | 0.95 | |||||||
| MAE (%) | 2.04 | 3.13 | 0.54 | – | |||||||
| Dynamic Case (100-cm Depth) | 29 | 0.757 | 0.727 | −3.89 | – | – | – | 0.556 | 0.574 | 3.23 | – |
| 30 | 0.835 | 0.823 | −1.38 | – | – | – | 0.602 | 0.629 | 4.48 | – | |
| 31 | 0.797 | 0.778 | −2.34 | – | – | – | 0.584 | 0.620 | 6.18 | – | |
| 32 | 0.775 | 0.748 | −3.53 | – | – | – | 0.578 | 0.611 | 5.71 | – | |
| 33 | 0.759 | 0.733 | −3.67 | – | – | – | 0.557 | 0.595 | 6.77 | – | |
| 34 | 0.759 | 0.734 | −3.41 | – | – | – | 0.557 | 0.591 | 6.05 | – | |
| 35 | 0.747 | 0.715 | −4.29 | – | – | – | 0.553 | 0.587 | 6.17 | – | |
| 36 | 0.752 | 0.719 | −4.35 | – | – | – | 0.551 | 0.574 | 4.23 | – | |
| 37 | 0.747 | 0.711 | −4.82 | – | – | – | 0.561 | 0.581 | 3.51 | – | |
| 38 | 0.738 | 0.757 | 2.63 | – | – | – | 0.548 | 0.564 | 2.83 | – | |
| 39 | 0.738 | 0.754 | 2.22 | – | – | – | 0.548 | 0.563 | 2.65 | – | |
| 40 | 0.735 | 0.750 | 2.01 | – | – | – | 0.544 | 0.559 | 2.74 | – | |
| Mean e | −2.07 | 4.55 | – | ||||||||
| MAE (%) | 3.21 | 4.55 | – | ||||||||
| SCAN site 2078
| |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| depth (cm) | DOS
| ||||||||||
| 31 | 32 | 33 | 34 | 35 | 36 | 37 | 38 | 39 | 40 | ||
| Xobs | 5 | 0.733 | 0.705 | 0.700 | 0.691 | 0.681 | 0.671 | 0.658 | 0.658 | 0.700 | 0.713 |
| 10 | 0.797 | 0.775 | 0.759 | 0.759 | 0.725 | 0.752 | 0.747 | 0.738 | 0.738 | 0.735 | |
| 20 | 0.823 | 0.804 | 0.794 | 0.787 | 0.783 | 0.785 | 0.780 | 0.783 | 0.778 | 0.775 | |
| 50 | 0.584 | 0.578 | 0.557 | 0.557 | 0.553 | 0.551 | 0.561 | 0.548 | 0.548 | 0.544 | |
| 100 | 0.548 | 0.539 | 0.537 | 0.534 | 0.532 | 0.530 | 0.527 | 0.525 | 0.521 | 0.525 | |
| Xm | 5 | 0.747 | 0.725 | 0.721 | 0.713 | 0.707 | 0.705 | 0.699 | 0.687 | 0.685 | 0.677 |
| 10 | 0.803 | 0.792 | 0.785 | 0.779 | 0.774 | 0.772 | 0.762 | 0.752 | 0.752 | 0.752 | |
| 20 | 0.839 | 0.837 | 0.829 | 0.822 | 0.816 | 0.813 | 0.805 | 0.805 | 0.803 | 0.803 | |
| 50 | 0.618 | 0.608 | 0.597 | 0.600 | 0.594 | 0.595 | 0.595 | 0.591 | 0.591 | 0.590 | |
| 100 | 0.554 | 0.540 | 0.531 | 0.522 | 0.522 | 0.515 | 0.515 | 0.508 | 0.507 | 0.507 | |
| e(%) | 5 | 1.95 | 2.98 | 2.96 | 3.25 | 3.90 | 5.10 | 6.16 | 4.34 | −2.15 | −5.03 |
| 10 | 0.79 | 2.18 | 3.49 | 2.71 | 3.68 | 2.64 | 2.00 | 1.95 | 1.95 | 2.28 | |
| 20 | 1.98 | 4.10 | 4.34 | 4.45 | 4.38 | 3.61 | 3.12 | 2.87 | 3.24 | 3.56 | |
| 50 | 5.82 | 5.19 | 7.13 | 7.67 | 7.44 | 8.05 | 6.06 | 7.76 | 7.76 | 8.63 | |
| 100 | 1.11 | 0.22 | −1.03 | −2.29 | −1.87 | −2.77 | −2.35 | −3.26 | −2.60 | −3.45 | |
| Mean e | – | 2.33 | 2.93 | 3.38 | 3.16 | 3.51 | 3.32 | 3.00 | 2.73 | 1.63 | 1.20 |
| MAE (%) | – | 2.33 | 2.93 | 3.79 | 4.07 | 4.25 | 4.43 | 3.94 | 4.04 | 3.54 | 4.59 |
| – | 0.97 | 0.95 | 0.93 | 0.92 | 0.87 | 0.92 | 0.92 | 0.93 | 0.94 | 0.91 | |
| Soil Type | Irrigation Rate (cm/min) | Total time to Saturate (min) | Effective SM
| Total Water (cm) Initial/Final | |
|---|---|---|---|---|---|
| Surface Initial/Final | Bottom Initial/Final | ||||
| Ad (SIL) | 0.104 | 56 | 0.55/1.0 | 0.57/.625 | 29.5/35.3 |
| Co (SIL) | 0.104 | 58 | 0.57/1.0 | 0.55/0.632 | 28.5/34.5 |
| Dt (SIL) | 0.102 | 58 | 0.52/1.0 | 0.55/0.584 | 27.0/32.6 |
| Gs (SIL) | 0.101 | 59 | 0.44/1.0 | 0.47/0.513 | 21.5/27.1 |
| Dc (SIC) | 0.019 | 141 | 0.65/1.0 | 0.66/0.665 | 35.4/38.1 |
| Df (SICL) | 0.035 | 115 | 0.71/1.0 | 0.68/0.720 | 36.3/40.2 |
| Initial | 200 | 201 | 202 | 203 | 204 | 205 | 206 | 207 | 208 | 209 | 210 | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ad (IP at 20 cm) | A | 1 | 0.80 | 0.72 | 0.69 | 0.66 | 0.65 | 0.63 | 0.62 | 0.59 | 0.56 | 0.53 | 0.50 |
| B | 0.70 | 0.69 | 0.67 | 0.65 | 0.64 | 0.63 | 0.62 | 0.62 | 0.64 | 0.62 | 0.61 | 0.60 | |
| C | 0.63 | 0.63 | 0.63 | 0.62 | 0.62 | 0.62 | 0.62 | 0.62 | 0.58 | 0.58 | 0.58 | 0.57 | |
| D | 35.3 | 34.3 | 33.8 | 33.5 | 33.0 | 32.8 | 32.5 | 32.2 | 31.7 | 31.2 | 30.7 | 30.3 | |
| AET | – | 0.56 | 0.60 | 0.53 | 0.42 | 0.25 | 0.27 | 0.32 | 0.521 | 0.48 | 0.45 | 0.41 | |
| Co (IP at 20 cm) | A | 1 | 0.82 | 0.74 | 0.70 | 0.68 | 0.66 | 0.64 | 0.63 | 0.60 | 0.56 | 0.54 | 0.51 |
| B | 0.72 | 0.69 | 0.67 | 0.66 | 0.64 | 0.64 | 0.63 | 0.63 | 0.64 | 0.63 | 0.61 | 0.59 | |
| C | 0.63 | 0.63 | 0.63 | 0.63 | 0.63 | 0.63 | 0.63 | 0.63 | 0.59 | 0.58 | 0.58 | 0.57 | |
| D | 34.3 | 33.7 | 33.2 | 32.9 | 32.5 | 32.3 | 32.0 | 31.7 | 30.5 | 30.1 | 29.9 | 29.4 | |
| AET | – | 0.56 | 0.59 | 0.53 | 0.42 | 0.25 | 0.27 | 0.31 | 0.51 | 0.47 | 0.44 | 0.40 | |
| Dc (No IP) | A | 1 | 0.89 | 0.82 | 0.77 | 0.74 | 0.73 | 0.71 | 0.68 | 0.64 | 0.61 | 0.58 | 0.55 |
| B | – | – | – | – | – | – | – | – | – | – | – | – | |
| C | 0.67 | 0.67 | 0.67 | 0.67 | 0.64 | 0.64 | 0.64 | 0.64 | 0.67 | 0.66 | 0.66 | 0.66 | |
| D | 38.1 | 37.2 | 36.9 | 36.7 | 36.3 | 35.8 | 35.4 | 35.2 | 35.1 | 34.0 | 33.7 | 33.3 | |
| AET | – | 0.57 | 0.61 | 0.55 | 0.44 | 0.27 | 0.29 | 0.33 | 0.55 | 0.50 | 0.46 | 0.42 | |
| Df (No IP) | A | 1 | 0.89 | 0.82 | 0.79 | 0.76 | 0.74 | 0.73 | 0.69 | 0.67 | 0.64 | 0.61 | 0.58 |
| B | – | – | – | – | – | – | – | – | – | – | – | – | |
| C | 0.72 | 0.72 | 0.72 | 0.72 | 0.72 | 0.70 | 0.70 | 0.72 | 0.72 | 0.72 | 0.72 | 0.72 | |
| D | 40.2 | 39.2 | 39.0 | 38.7 | 38.4 | 37.5 | 37.3 | 37.0 | 36.5 | 36.1 | 35.7 | 35.4 | |
| AET | – | 0.55 | 0.59 | 0.53 | 0.42 | 0.25 | 0.27 | 0.31 | 0.51 | 0.44 | 0.41 | 0.37 | |
| Dt (IP at 35 cm) | A | 1 | 0.76 | 0.68 | 0.65 | 0.62 | 0.60 | 0.58 | 0.56 | 0.53 | 0.50 | 0.47 | 0.44 |
| B | 0.65 | 0.67 | 0.66 | 0.64 | 0.65 | 0.63 | 0.63 | 0.62 | 0.61 | 0.59 | 0.58 | 0.56 | |
| C | 0.58 | 0.58 | 0.58 | 0.58 | 0.58 | 0.58 | 0.58 | 0.58 | 0.56 | 0.56 | 0.53 | 0.53 | |
| D | 32.6 | 31.8 | 31.3 | 30.8 | 30.7 | 30.3 | 30.0 | 29.8 | 29.0 | 28.5 | 27.7 | 27.3 | |
| AET | – | 0.59 | 0.64 | 0.57 | 0.46 | 0.29 | 0.32 | 0.37 | 0.57 | 0.50 | 0.47 | 0.44 | |
| Gs (IP at 45 cm) | A | 1 | 0.64 | 0.58 | 0.54 | 0.51 | 0.49 | 0.47 | 0.45 | 0.41 | 0.37 | 0.34 | 0.31 |
| B | 0.59 | 0.61 | 0.61 | 0.59 | 0.54 | 0.53 | 0.52 | 0.51 | 0.50 | 0.48 | 0.46 | 0.44 | |
| C | 0.51 | 0.51 | 0.51 | 0.51 | 0.51 | 0.51 | 0.51 | 0.51 | 0.51 | 0.51 | 0.51 | 0.51 | |
| D | 27.1 | 26.9 | 26.4 | 25.9 | 24.8 | 24.6 | 24.1 | 23.7 | 23.1 | 22.5 | 22.1 | 21.7 | |
| AET | – | 0.59 | 0.64 | 0.57 | 0.46 | 0.29 | 0.30 | 0.35 | 0.59 | 0.52 | 0.49 | 0.45 | |
| Soil Type | Physical model Result
| Redistribution Technique Result inflection point depths (cm) | |
|---|---|---|---|
| First 2 prominent inflection point depths (cm) (initial) | First 2 prominent inflection point depths (cm) (final) | ||
| Ad (SIL) | 20, 00 | 15, 25 | 20 |
| Co (SIL) | 25, 00 | 15, 25 | 20 |
| Dt (SIL) | 30, 00 | 15, 35 | 30–40 |
| Gs (SIL) | 40, 00 | 15, 40 | 45–50 |
| Dc (SIC) | 00, 00 | 15, 00 | 00 |
| Df (SICL) | 00, 00 | 15, 00 | 00 |
| Soil Type | Water added | Total ET | ∆S | ∆(S + ET ) | ∆SM | ∆SM100cm |
|---|---|---|---|---|---|---|
| Ad (SIL) | 5.8 | 4.81 | 0.8 | 5.61 | 0.19 | 0.137 |
| Co (SIL) | 6.0 | 4.75 | 0.9 | 5.65 | 0.35 | 0.198 |
| Dt (SIL) | 5.6 | 5.22 | 0.3 | 5.52 | 0.08 | 0.081 |
| Gs (SIL) | 5.6 | 5.25 | 0.2 | 5.45 | 0.15 | 0.096 |
| Dc (SIC) | 2.7 | 4.99 | −2.1 | 2.89 | −0.19 | 0.011 |
| Df (SICL) | 3.9 | 4.65 | −0.9 | 3.75 | 0.15 | 0.095 |
© 2015 by the authors; licensee MDPI, Basel, Switzerland This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mishra, V.; Ellenburg, W.L.; Al-Hamdan, O.Z.; Bruce, J.; Cruise, J.F. Modeling Soil Moisture Profiles in Irrigated Fields by the Principle of Maximum Entropy. Entropy 2015, 17, 4454-4484. https://doi.org/10.3390/e17064454
Mishra V, Ellenburg WL, Al-Hamdan OZ, Bruce J, Cruise JF. Modeling Soil Moisture Profiles in Irrigated Fields by the Principle of Maximum Entropy. Entropy. 2015; 17(6):4454-4484. https://doi.org/10.3390/e17064454
Chicago/Turabian StyleMishra, Vikalp, Walter L. Ellenburg, Osama Z. Al-Hamdan, Josh Bruce, and James F. Cruise. 2015. "Modeling Soil Moisture Profiles in Irrigated Fields by the Principle of Maximum Entropy" Entropy 17, no. 6: 4454-4484. https://doi.org/10.3390/e17064454
APA StyleMishra, V., Ellenburg, W. L., Al-Hamdan, O. Z., Bruce, J., & Cruise, J. F. (2015). Modeling Soil Moisture Profiles in Irrigated Fields by the Principle of Maximum Entropy. Entropy, 17(6), 4454-4484. https://doi.org/10.3390/e17064454





